Simplified Cost Functions Meet Advanced Muscle Models to Streamline Muscle Force Estimation
Abstract
1. Introduction
2. Methodology
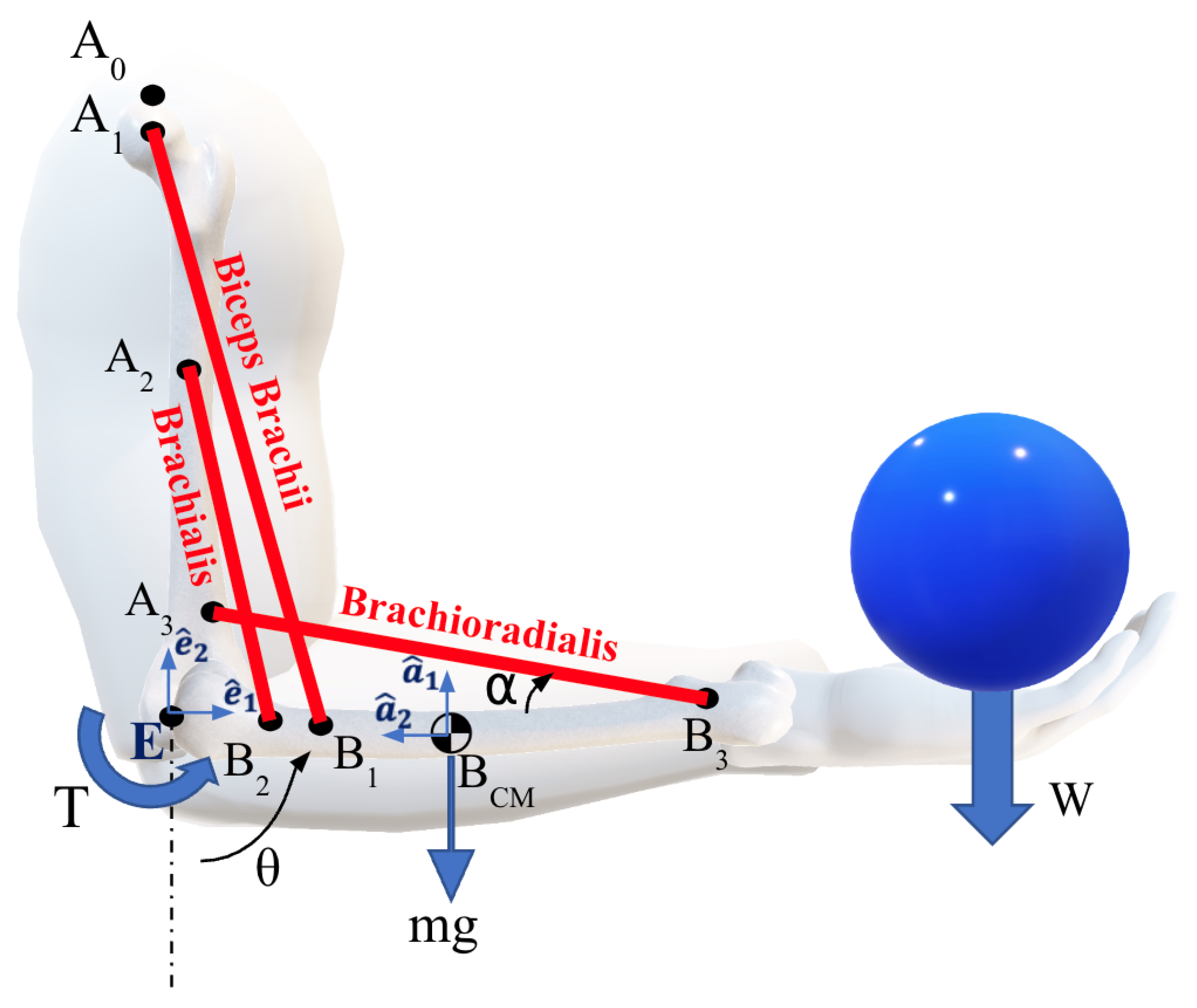
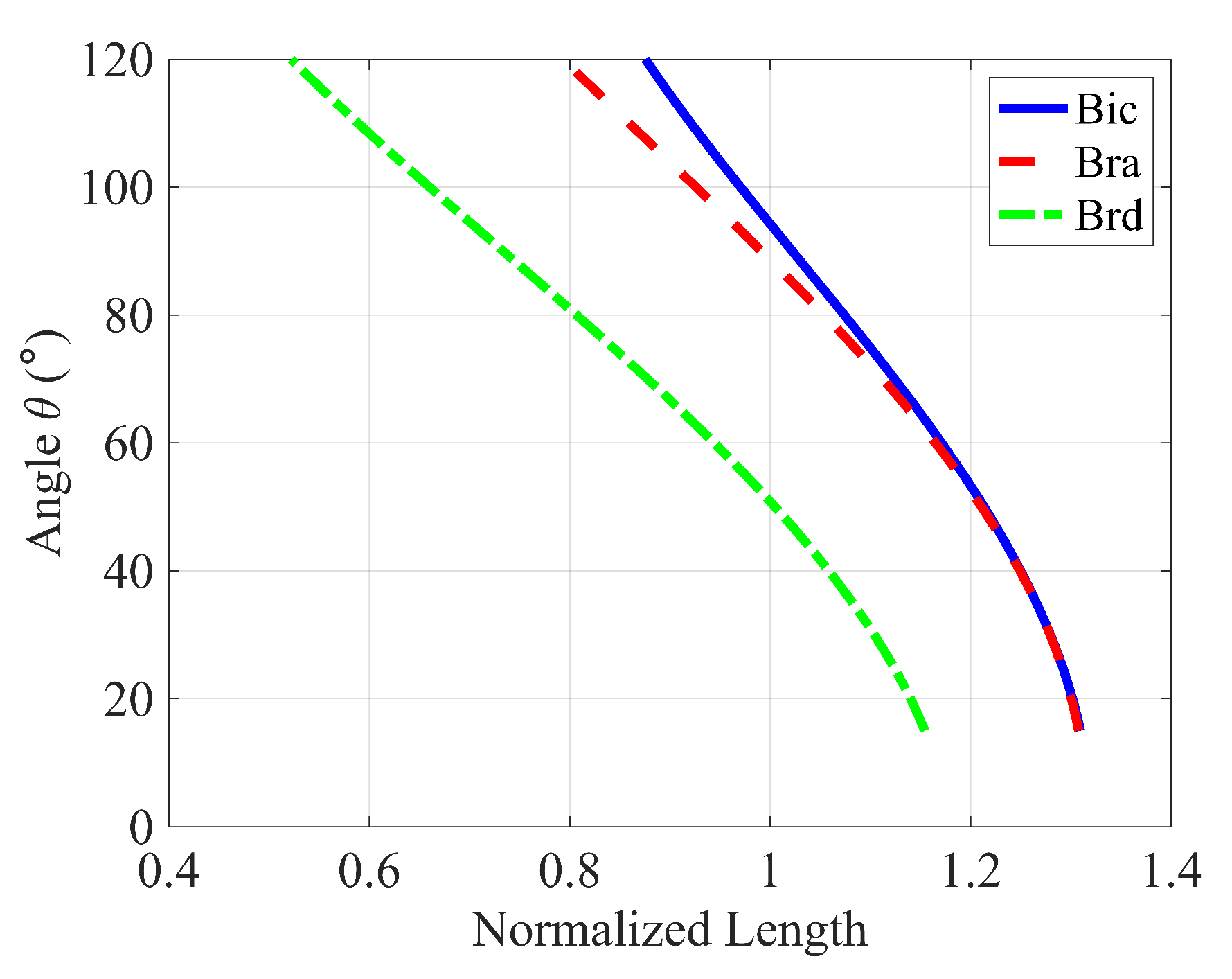
2.1. Biomechanical Model
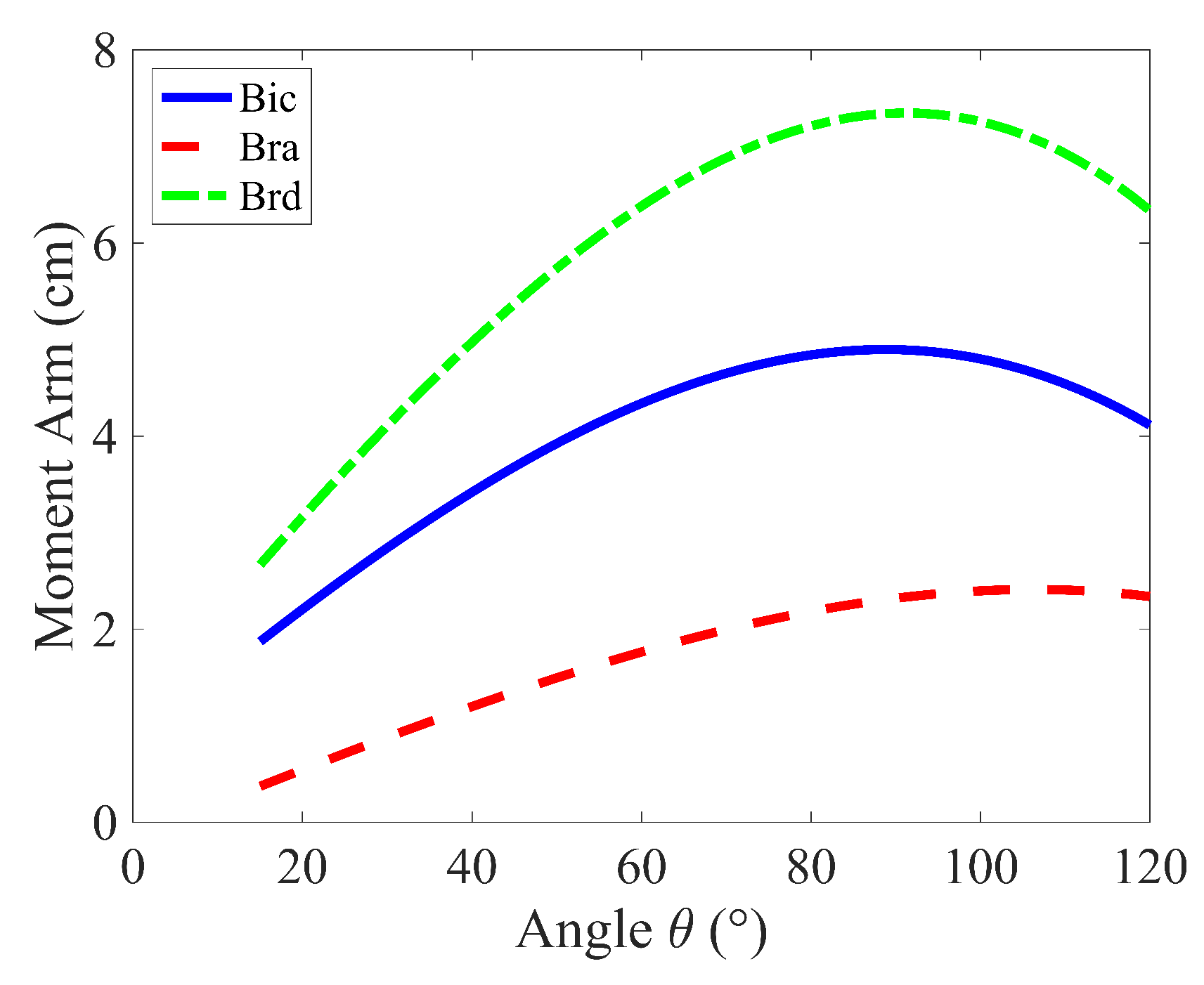
2.2. Calculation of Forces and Torque at the Elbow Joint
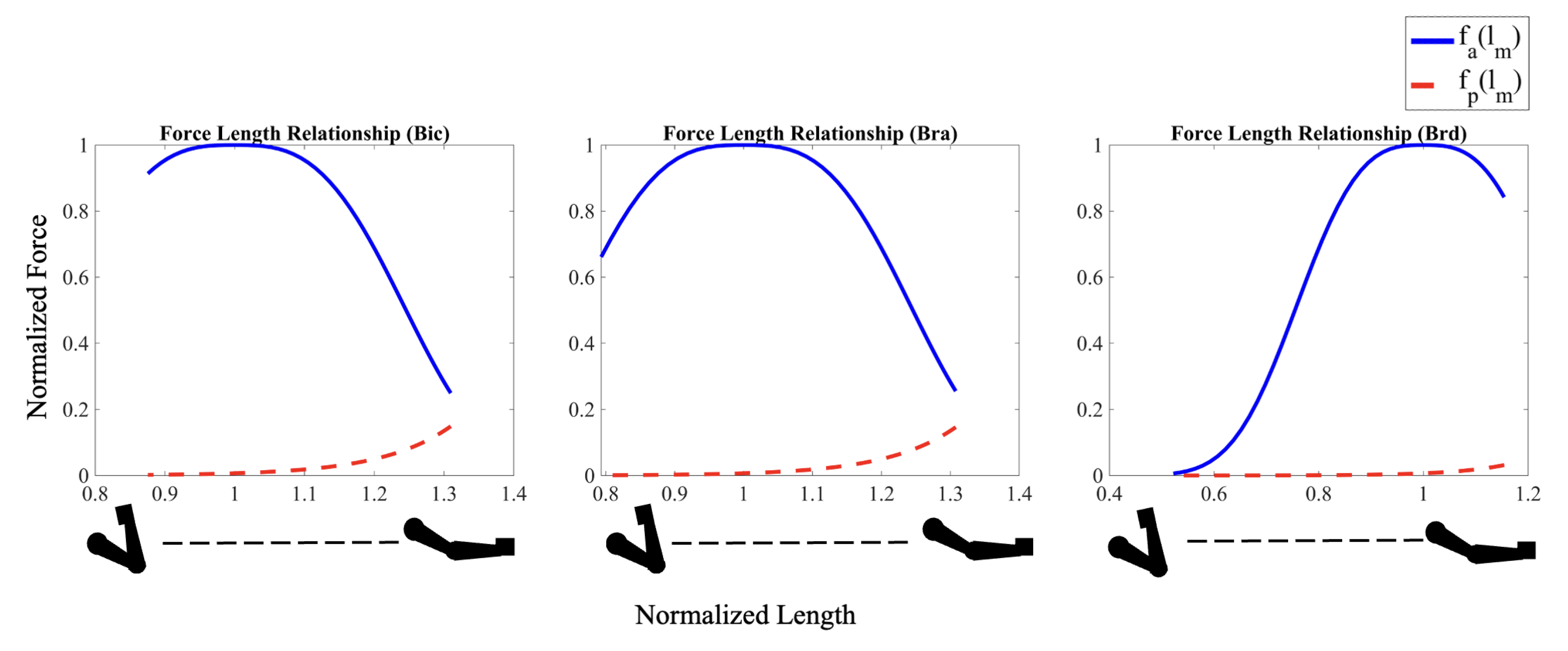
2.3. Muscle-Force Models
- 1.
- Simple Force Model [33]:This model considers muscle force as a vector directed from its insertion to its origin, without considering the effects of the skeletal muscle’s force–length relationship or the speed of muscle contraction. Since this model does not attempt to address the physiological properties of skeletal muscle, it serves as a control from which the effects of more complex models can be evaluated.
- 2.
- Hill-Type Active Model [25]:Hill-type models have been widely used as a foundational representation of skeletal muscle mechanics, incorporating the effects of muscle fiber length and contraction speed to estimate maximum force generation [25]. The muscle force equation is:where represents maximum isometric force, is muscle activation, captures the active force–length relationship and captures the force–velocity relationship.
- 3.
- Hill-Type Active and Passive [34]:This comprehensive model builds on the Hill-Type Active model to represent both active and passive muscle contraction behaviors [34]. The muscle force is given by:Here, the additional term represents the passive force–length property, adding another layer of complexity by considering the intrinsic force–length properties of muscle fibers.
- •
- Constant tendon length—In this approximation, muscle lengths exceed realistic bounds, and force–length properties are inaccurately represented at the beginning and end of the motion.
- •
- Linear muscle contraction—In this adaptation, the muscle length is assumed to change at a constant rate. As a result, however, the force–velocity value is constant and is not correctly represented.
- •
- Linear tendon length change—In this adaptation, while the rate of tendon length change remains constant, muscle length changes non-linearly, providing more realistic force–length and force–velocity values.
- •
- Exponential tendon length change—In this one, the rate of change of tendon length varies exponentially, offering the most accurate representation of the muscle model.
2.4. Muscle-Force Constraints and Cost Functions
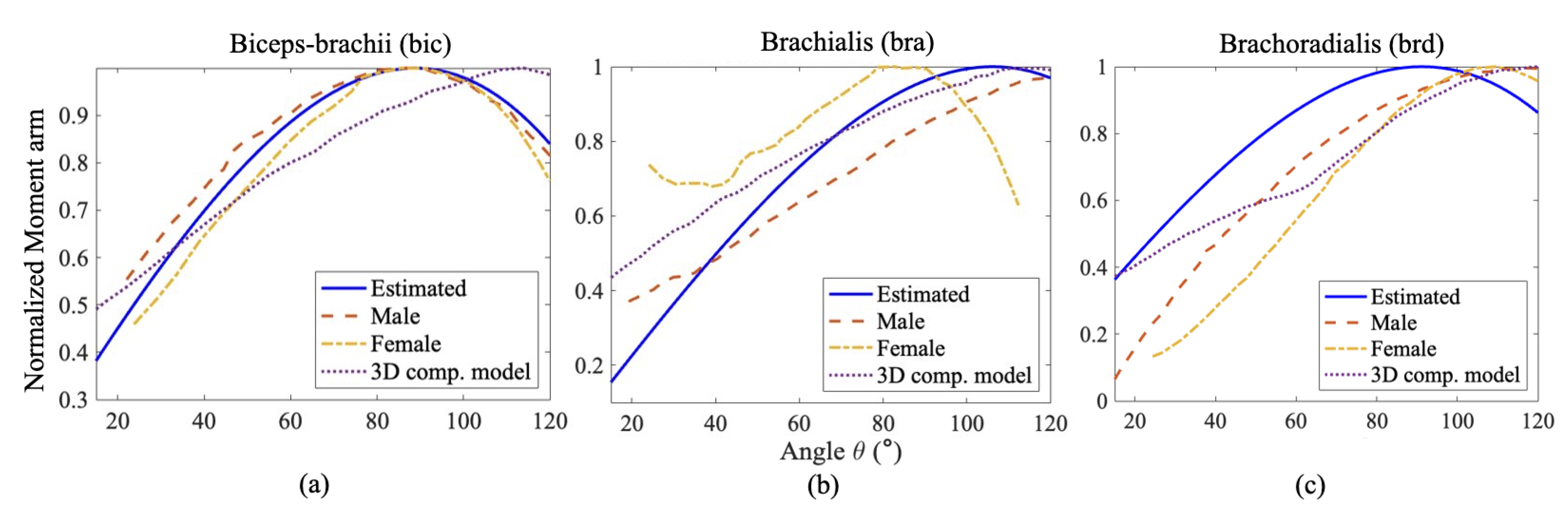
2.5. Model Validation
3. Results
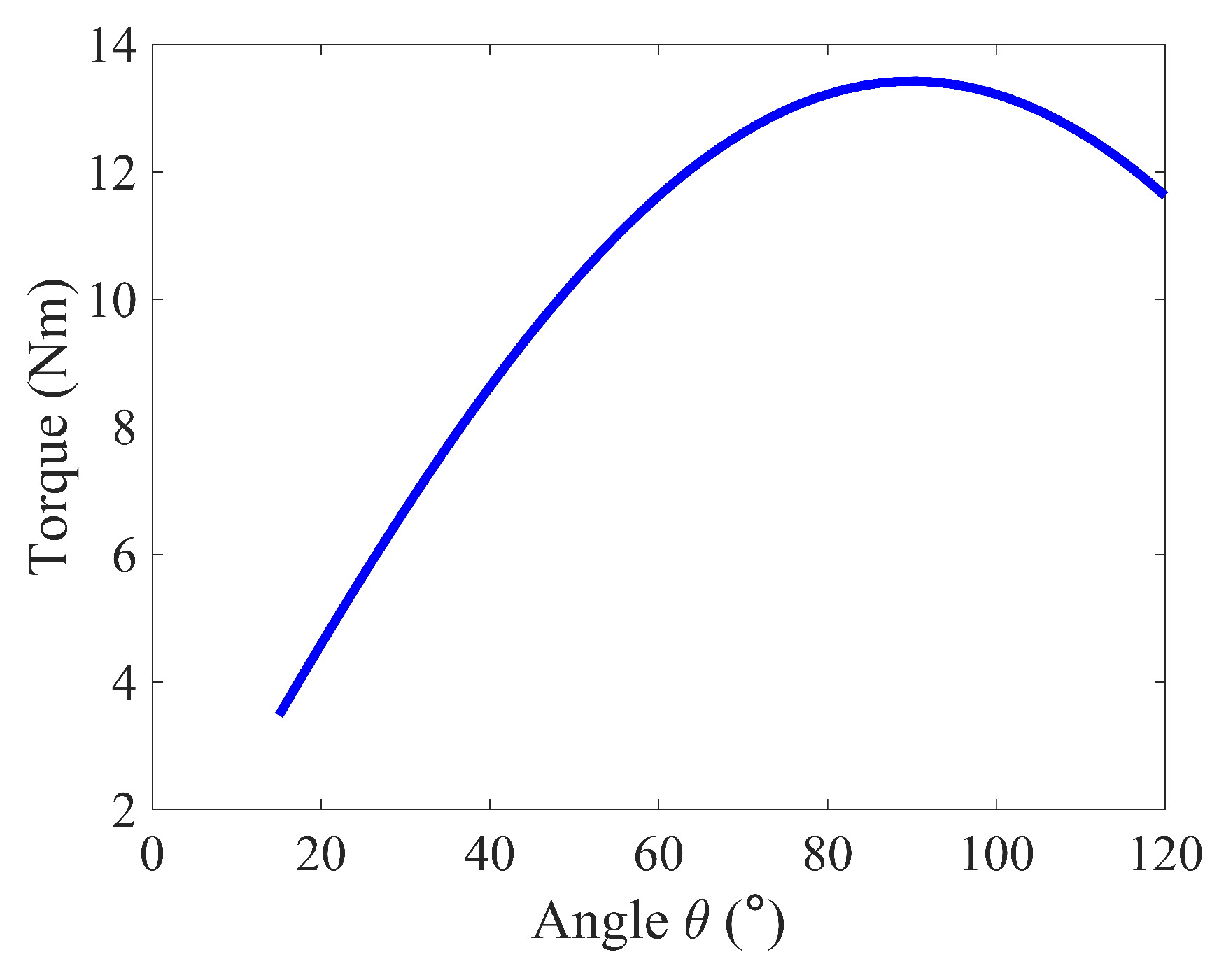
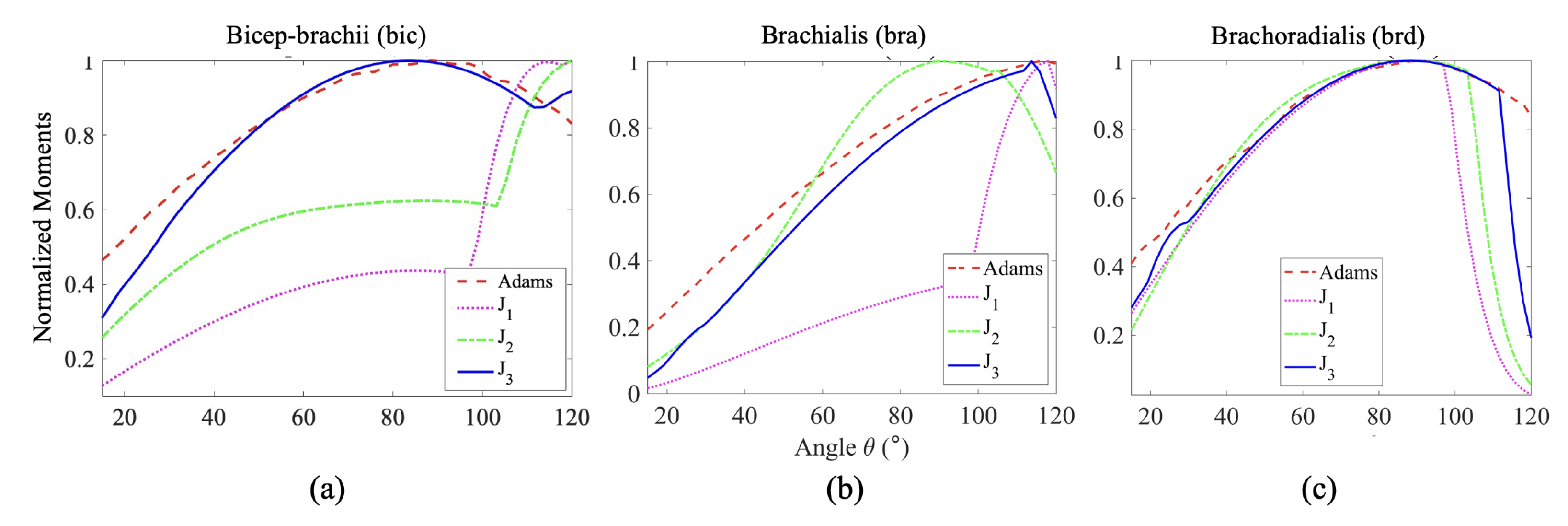
3.1. Joint Torque
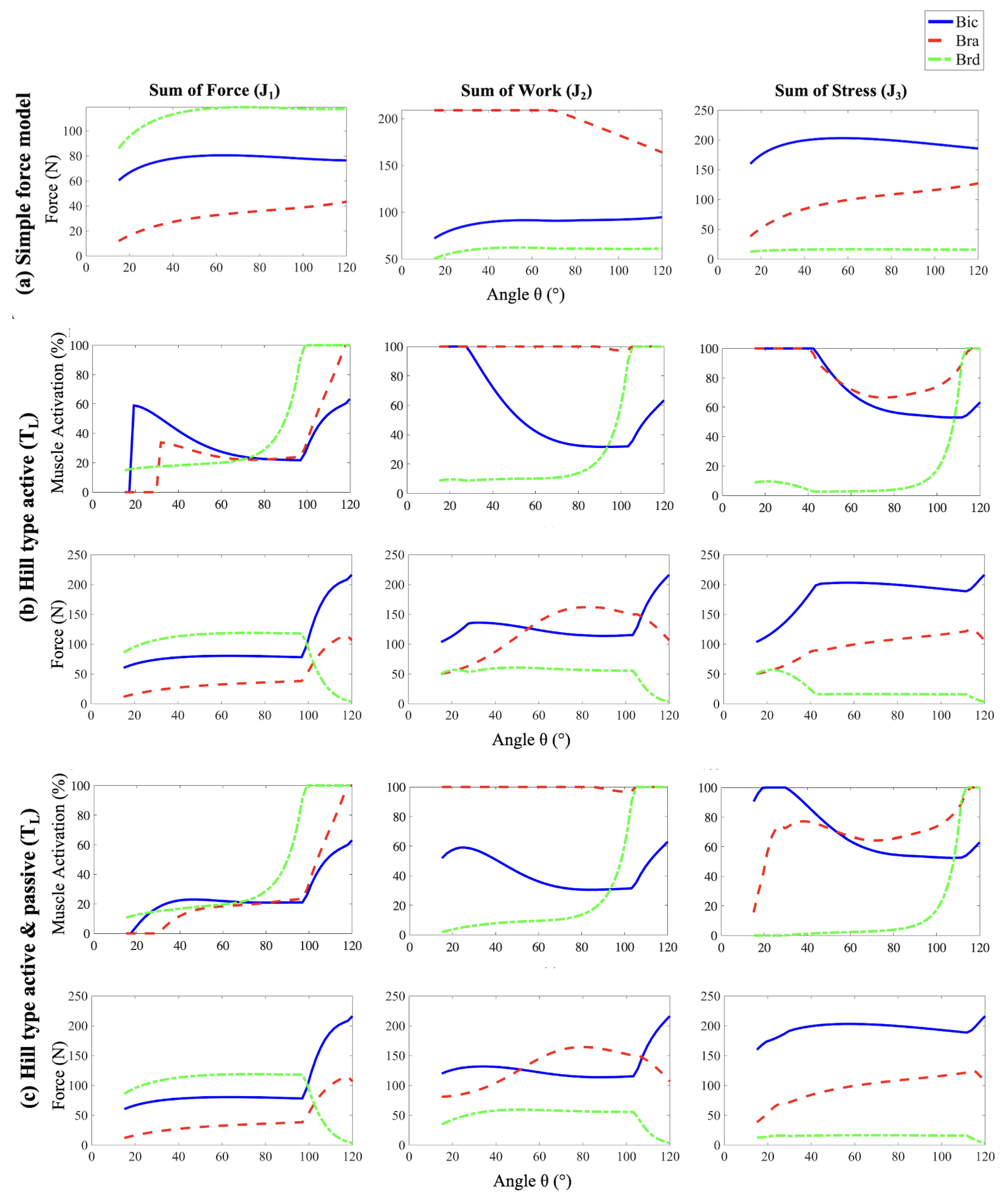
3.2. Muscle Forces
3.2.1. Simple Force Model
3.2.2. Hill-Type Active
3.2.3. Hill-Type Active and Passive
3.3. Model Validation
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| bic | biceps brachii |
| bra | brachialis |
| brd | brachoradialis |
| DoF | degrees of freedom |
| EMG | electromyography |
| PCSA | physiological cross-sectional area |
References
- Serrancolí, G.; Font-Llagunes, J.M.; Barjau, A. A weighted cost function to deal with the muscle force sharing problem in injured subjects: A single case study. J. Multi-Body Dyn. 2014, 228, 241–251. [Google Scholar] [CrossRef]
- Silva, M.T.; Ambrósio, J.A. Solution of redundant muscle forces in human locomotion with multibody dynamics and optimization tools. Mech. Based Des. Struct. Mach. 2003, 31, 381–411. [Google Scholar] [CrossRef]
- Nikooyan, A.A.; Veeger, H.E.J.; Chadwick, E.K.J.; Praagman, M.; van der Helm, F.C.T. Development of a comprehensive musculoskeletal model of the shoulder and elbow. Med. Biol. Eng. Comput. 2011, 49, 1425–1435. [Google Scholar] [CrossRef] [PubMed]
- Paraschiv, C.; Paraschiv, P.; Cimpoeşu, R. Determination of the elbow joint resulting torque and obtaining customized numerical results. Procedia 2014, 117, 522–528. [Google Scholar] [CrossRef]
- Robertson, G.; Caldwell, G.; Hamill, J.; Kamen, G.; Whittlesey, S. Research Methods in Biomechanics, 2nd ed.; Human Kinetics: Champaign, IL, USA, 2013. [Google Scholar]
- Erdemir, A.; McLean, S.; Herzog, W.; van den Bogert, A.J. Model-based estimation of muscle forces exerted during movements. Clin. Biomech. 2007, 22, 131–154. [Google Scholar] [CrossRef]
- Tsirakos, D.; Baltzopoulos, V.; Bartlett, R. Inverse optimization: Functional and physiological considerations related to the force-sharing problem. Crit. Rev. Biomed. Eng. 1997, 25, 371–407. [Google Scholar] [CrossRef]
- Zonnino, A.; Sergi, F. Model-based estimation of individual muscle force based on measurements of muscle activity in forearm muscles during isometric tasks. IEEE Trans. Biomed. Eng. 2019, 1, 171. [Google Scholar] [CrossRef]
- Shourijeh, M.S.; Fregly, B.J. Muscle synergies modify optimization estimates of joint stiffness during walking. J. Biomech. Eng. 2020, 142, 310. [Google Scholar] [CrossRef]
- Ao, D.; Shourijeh, M.S.; Patten, C.; Fregly, B.J. Evaluation of Synergy Extrapolation for Predicting Unmeasured Muscle Excitations from Measured Muscle Synergies. Front. Comput. Neurosci. 2020, 14, 588943. [Google Scholar] [CrossRef]
- Hardt, D. Determining muscle forces in the leg during normal human walking—An application and evaluation of optimization methods. J. Biomech. Eng. 1978, 100, 72–78. [Google Scholar] [CrossRef]
- Crowninshield, R.D.; Brand, R.A. A physiologically based criterion of muscle force prediction in locomotion. J. Biomech. 1981, 14, 793–801. [Google Scholar] [CrossRef] [PubMed]
- Heintz, S.; Gutierrez-Farewik, E.M. Static optimization of muscle forces during gait in comparison to EMG-to-force processing approach. Gait Posture 2007, 26, 279–288. [Google Scholar] [CrossRef] [PubMed]
- Zajac, F. Muscle and tendon: Properties, models, scaling, and application to biomechanics and motor control. Crit. Rev. Biomed. Eng. 1989, 17, 359–411. [Google Scholar] [PubMed]
- Lai, A.K.M.; Biewener, A.A.; Wakeling, J.M. Metabolic cost underlies task-dependent variations in motor unit recruitment. J. R. Soc. Interface 2018, 15, 20180541. [Google Scholar] [CrossRef] [PubMed]
- Ting, L.H.; Chvatal, S.A.; Safavynia, S.A.; McKay, J.L. Review and perspective: Neuromechanical considerations for predicting muscle activation patterns for movement. Int. J. Numer. Methods Biomed. Eng. 2012, 28, 1003–1014. [Google Scholar] [CrossRef]
- Michaud, F.; Lamas, M.; Lugrís, U.; Cuadrado, J. A fair and EMG-validated comparison of recruitment criteria, musculotendon models and muscle coordination strategies, for the inverse-dynamics based optimization of muscle forces during gait. J. Neuroeng. Rehabil. 2020, 18, 17. [Google Scholar] [CrossRef]
- Wena, J.; Raison, M.; Achiche, S. Using a cost function based on kinematics and electromyographic data to quantify muscle forces. J. Biomech. 2018, 80, 151–158. [Google Scholar] [CrossRef]
- Seireg, A.; Arvikar, R. A mathematical model for evaluation of forces in lower extremeties of the musculo-skeletal system. J. Biomech. 1973, 6, 313–326. [Google Scholar] [CrossRef]
- Penrod, D.; Davy, D.; Singh, D. An optimization approach to tendon force analysis. J. Biomech. 1974, 7, 123–129. [Google Scholar] [CrossRef]
- Crowninshield, R.D.; Johnston, R.C.; Andrews, J.G.; Brand, R.A. A biomechanical investigation of the human hip. J. Biomech. 1978, 11, 75–85. [Google Scholar] [CrossRef]
- Dul, J.; Johnson, G.; Shiavi, R.; Townsend, M. Muscular synergism—II. A minimum-fatigue criterion for load sharing between synergistic muscles. J. Biomech. 1984, 17, 675–684. [Google Scholar] [CrossRef] [PubMed]
- Racinais, S.; Maffiuletti, N.A.; Girard, O. M-wave, H- and V-reflex recruitment curves during maximal voluntary contraction. J. Clin. Neurophysiol. 2013, 30, 415–421. [Google Scholar] [CrossRef] [PubMed]
- Sartori, M.; Farina, D.; Lloyd, D.G. Hybrid neuromusculoskeletal modeling to best track joint moments using a balance between muscle excitations derived from electromyograms and optimization. J. Biomech. 2014, 47, 3613–3621. [Google Scholar] [CrossRef] [PubMed]
- Buchanan, T.S.; Lloyd, D.G.; Manal, K.; Besier, T.F. Neuromusculoskeletal modeling: Estimation of muscle forces and joint moments and movements from measurements of neural command. J. Appl. Biomech. 2004, 20, 367–395. [Google Scholar] [CrossRef]
- Praagmana, M.; Chadwickb, E.; van der Helmb, F.; Veegera, H. The relationship between two different mechanical cost functions and muscle oxygen consumption. J. Biomech. 2006, 39, 758–765. [Google Scholar] [CrossRef]
- Veerkamp, K.; Waterval, N.; Geijtenbeek, T.; Carty, C.; Lloyd, D.; Harlaar, J.; van der Krogt, M. Evaluating cost function criteria in predicting healthy gait. J. Biomech. 2021, 123, 110530. [Google Scholar] [CrossRef]
- Islam, S.U.; Glover, A.; MacFarlane, R.J.; Mehta, N.; Waseem, M. The Anatomy and Biomechanics of the Elbow. Open Orthop. J. 2020, 14, 95–99. [Google Scholar] [CrossRef]
- Malagelada, F.; Dalmau-Pastor, M.; Vega, J.; Golanó, P. Elbow Anatomy. Sport. Inj. 2014, 2, 527–553. [Google Scholar]
- Ettema, G.; Styles, G.; Kippers, V. The moment arms of 23 muscle segments of the upper limb with varying elbow and forearm positions: Implications for motor control. Hum. Mov. Sci. 1998, 17, 201–220. [Google Scholar] [CrossRef]
- Mitiguy, P. Dynamics of Mechanical, Aerospace, & Bio/Robotic Systems. Available online: http://www.motiongenesis.com (accessed on 13 September 2024).
- Nikravesh, P.E. Computer-Aided Analysis of Mechanical Systems; Prentice-Hall, Inc.: Hoboken, NJ, USA, 1988. [Google Scholar]
- Wendlova, J. Why is so important to balance the muscular dysbalance in mm. coxae area in osteoporotic patients? Bratisl. Lekárske Listy 2008, 109, 502–507. [Google Scholar]
- Maso, F.D.; Begon, M.; Raison, M. Methodology to customize maximal isometric forces for hill-type muscle models. J. Appl. Biomech. 2016, 33, 80–86. [Google Scholar] [CrossRef] [PubMed]
- Jo, S. A computational neuromusculoskeletal model of human arm movements. Int. J. Control. Autom. Syst. 2011, 9, 913–923. [Google Scholar] [CrossRef]
- Chang, Y.W.; Su, F.C.; Wu, H.W.; An, K.N. Optimum length of muscle contraction. Clin. Biomech. 1999, 14, 537–542. [Google Scholar] [CrossRef] [PubMed]
- Lemay, M.A.; Crago, P.E. A Dynamic Model for Simulating Movements of the Elbow, Forearm, and Wrist. J. Biomech. 1990, 29, 1319–1330. [Google Scholar] [CrossRef]
- Murray, W.M.; Buchanan, T.S.; Delp, S.L. The isometric functional capacity of muscles that cross the elbow. J. Biomech. 2000, 33, 943–952. [Google Scholar] [CrossRef]
- MathWorks. lsqlin—Solve Linear Least-Squares Problems with Linear Constraints. Available online: https://www.mathworks.com/help/optim/ug/lsqlin.html (accessed on 13 September 2024).
- MathWorks. fmincon—Find the Minimum of a Constrained Nonlinear Multivariable Function. Available online: https://www.mathworks.com/help/optim/ug/fmincon.html (accessed on 13 September 2024).
- Murray, W.M.; Delp, S.L.; Buchanan, T.S. Variation of muscle moment arms with elbow and forearm position. J. Biomech. 1995, 28, 513–525. [Google Scholar] [CrossRef]
- Ilbeigi, S.; Ramezani, H. Assessment and modelling of elbow joint for analysing of muscle moment and reaction force during flexion movement with ADAMS Software. Eur. J. Sport. Exerc. Sci. 2014, 3, 18–26. [Google Scholar]
| Humerus Length (), | 29 | |
| Forearm Length (), | 36 | |
| Forearm Center of Mass (), (Distance from the Elbow Joint) | ||
| Forearm Mass, | 1.53 | |
| Forearm Moment of Inertia, (About its Center of Mass) | ||
| Origin (at Humerus) | Insertion (at Forearm) | |
| bic | ||
| bra | ||
| brd |
| Cost Function | Description |
|---|---|
| Sum of Force criterion | |
| Sum of Work criterion | |
| Sum of Stress criterion |
| Mean Squared Error | |||
|---|---|---|---|
| Muscle | |||
| Bicep | 0.1926 | 0.0792 | 0.0029 |
| Brachialis | 0.1612 | 0.0147 | 0.0089 |
| Brachoradialis | 0.0989 | 0.0645 | 0.0207 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ahmed, M.H.; N’Guessan, J.-E.; Das, R.; Leineweber, M.; Goyal, S. Simplified Cost Functions Meet Advanced Muscle Models to Streamline Muscle Force Estimation. BioMed 2024, 4, 350-365. https://doi.org/10.3390/biomed4030028
Ahmed MH, N’Guessan J-E, Das R, Leineweber M, Goyal S. Simplified Cost Functions Meet Advanced Muscle Models to Streamline Muscle Force Estimation. BioMed. 2024; 4(3):350-365. https://doi.org/10.3390/biomed4030028
Chicago/Turabian StyleAhmed, Muhammad Hassaan, Jacques-Ezechiel N’Guessan, Ranjan Das, Matthew Leineweber, and Sachin Goyal. 2024. "Simplified Cost Functions Meet Advanced Muscle Models to Streamline Muscle Force Estimation" BioMed 4, no. 3: 350-365. https://doi.org/10.3390/biomed4030028
APA StyleAhmed, M. H., N’Guessan, J.-E., Das, R., Leineweber, M., & Goyal, S. (2024). Simplified Cost Functions Meet Advanced Muscle Models to Streamline Muscle Force Estimation. BioMed, 4(3), 350-365. https://doi.org/10.3390/biomed4030028






