Solvent Polarity/Polarizability Parameters: A Study of Catalan’s SPP^N, Using Computationally Derived Molecular Properties, and Comparison with π* and E_T(30)

Abstract

Catalan’s SPP^N, a measure of solvent polarity/polarizability has been analysed in terms of molecular properties derived from computational chemistry. The results show that SPP^N correlates positively with the molecular dipole moment and quadrupolar amplitude and negatively with the molecular polarizability. These correlations are shared with Kamet and Taft’s π* and Reichardt and Dimroth’s E_T(30). Thus, one can associate the solvent polarity with non-specific interactions involving the permanent charges on solvent molecules. It is also noted that the opposite correlations, all three parameters increasing with increasing solvent polarity but decreasing with increasing solvent polarizability, creates an ambiguity in their use, for example, in linear free energy relationships.

1. Introduction

Understanding and predicting the effects of changes in solvent on chemical processes are among the classical problems of solution chemistry. Thus, virtually all thermodynamic, kinetic and spectroscopic properties of chemical systems are sensitive to changes in the solvent, some changing by orders of magnitude.

The use of linear free energy relationships to explore or predict the effect of changes in solvent on chemical processes is well established. The principle is straightforward and one writes:

$$SP={\displaystyle \sum c_i}{P}_i$$

(1)

where SP is some property, such as the log of the solubility of a solute, the P_i are experimental parameters reflecting properties of the solvent (or solute) and that c_i are coefficients reflecting the response of the solute (or solvent). In effect each c_iP_i term represents the effect of a different intermolecular interaction on SP.

Generally the parameters P_i are derived from experiments that are designed to isolate a particular interaction. In general P_i values represent specific, acid–base interactions: (1) the basicity of the solvent (Kamlet and Taft’s β [1], Catalan’s SB [2], Gutmann’s DN [3]); (2) the acidity of the solvent (Kamlet and Taft’s α [4], Catalan’s SA [5], Gutmann’s AN [3]) or non-specific interactions that are collected together in parameters that are measures of the polarity/polarizability of the solvent (Kamlet and Taft’s π* [6], Catalan’s SPP [7], Reichardt and Dimroth’s E_T(30) [8]).

The range of experimental parameters raises several interesting questions, including (1) the extent to which these parameters simply reflect properties of the solvent molecules, (2) which molecular properties contribute to the experimental parameters and (3) whether different parameters, for basicity say, reflect the same molecular properties.

These have been explored in a series of papers where experimental parameters have been correlated with a set of molecular properties derived from computational chemistry. These have shown that measures of solvent basicity (β, DN [9] and SB [10]) reflect the partial charge on the most negative atom of the solvent molecule and the energy of the donor orbital, while measures of the solvent acidity (α [11], AN and SA [10]) reflect only the partial charge on the most positive hydrogen atom of the solvent molecule.

In the present paper, a similar analysis of Catalan’s SPP^N, a measure of solvent polarity and polarizability, is reported and the results compared to those reported previously for Kamlet and Tafts’s π* and Reichardt and Dimroth’s E_T(30) [12,13].

2. Methods and Materials

2.1. Computational Details

The computational methods have been discussed in some detail previously [9,11,12,13]. Since the calculated molecular properties can depend on the method used, calculations were carried out using both the Hartree–Fock and density functional (B3LYP functional) methods using the 6-311+g(3df,2p) basis set. For the molecular dipole moments, quadrupolar amplitudes and polarizabilities, plots of the values calculated using the two methods against each other are linear and so the choice of method is immaterial [9]. This isn’t the case for orbital energies, for which such plots show scatter around a straight line [9]. The situation for partial atomic charges is more complex, since these are not quantum mechanical observables and require a model that ascribes the electronic charge density to the individual atoms. Partial charges based on Mullican’s model are highly dependent on the basis set and appear not to converge as the basis set is increased. Those based on the Hirshfeld [14], Natural bond order [15] and the CM5 [16] model do converge as the basis set is improved [9] and all have been used previously. It was fond that the Hirshfeld and NBO models gave very similar results [12] and, in a recent paper considering Abraham parameters it was recognized that the CM5 model overestimates the partial charges on nitrogen atoms, for amides at least [17]. Thus the analyses reported here use partial charges derived using Hirshfeld’s model.

All calculations were carried out using the Gaussian 9 software [18].

2.2. Analyses of Parameters

As in previous work, the normalized parameter, SPP^N in this case, has been considered. The use of normalized scales allows relatively direct comparison of the results of the analyses. Values of SPP^N were taken from [7].

The approach used is a simple [9], multivariable regression of the experimental parameter, P, against molecular descriptors, Q_i, representing different molecular properties. Thus:

$$P=P^0+{\displaystyle \sum a_i{Q}_i}$$

(2)

where P⁰ is the value of P when all of the a_iQ_i terms are zero and the a_i are the coefficients recovered from the regression. In essence each a_iQ_i term represents the contribution of a particular interaction to P.

The molecular descriptors are normalized and calculated from the calculated molecular properties, q_i, as:

$$Q_i=\frac{q_i-q_i^{\min }}{q_i^{\max }-q_i^{\min }}$$

(3)

where $q_i^{\min }$ and $q_i^{\max }$ are the minimum and maximum values of $q_i$. With the exception of the orbital energies, the values of $q_i^{\min }$ are taken to be zero.

Seven molecular properties: the partial charges on the most negative atom and on the most positive hydrogen atom, the molecular dipole moments, quadrupolar amplitudes (see Appendix A) and polarizabilities and the energies of electron donor and acceptor orbitals are considered. In general, the orbital energies are taken to be those of the highest occupied and lowest unoccupied molecular orbitals; the exception to this is the case of solvents with aromatic groups. This was discussed previously [9] but, briefly, solvents with a common functional group have, for example, similar basicity parameters, whether they are aliphatic or aromatic, although for aromatic solvents, the high energy π-bonds of the aromatic ring are clearly the HOMOs, while for aliphatic solvents the HOMO is associated with the basic functional group. The fact that the basicity parameters are similar argues for the use of the energies of the orbitals at the interacting functional group rather than those of the aromatic ring.

In applying Equation (1), the experimental parameter, P, was initially correlated with all seven molecular descriptors; subsequently, descriptors making negligible contributions (typically a_i ≤ 0.03) or with standard deviations comparable to the value of the coefficient, were excluded and the correlation repeated with the remaining descriptors. Since both the calculated properties and experimental parameters for solvents with a common functional group are similar, the distributions of these can’t be represented by statistics based on the normal distribution. Thus, the decision as to whether a descriptor was making a significant contribution to P was decided on the basis of the increase in the standard deviation between the calculated and experimental values of P, when the descriptor was excluded from the correlation.

3. Results

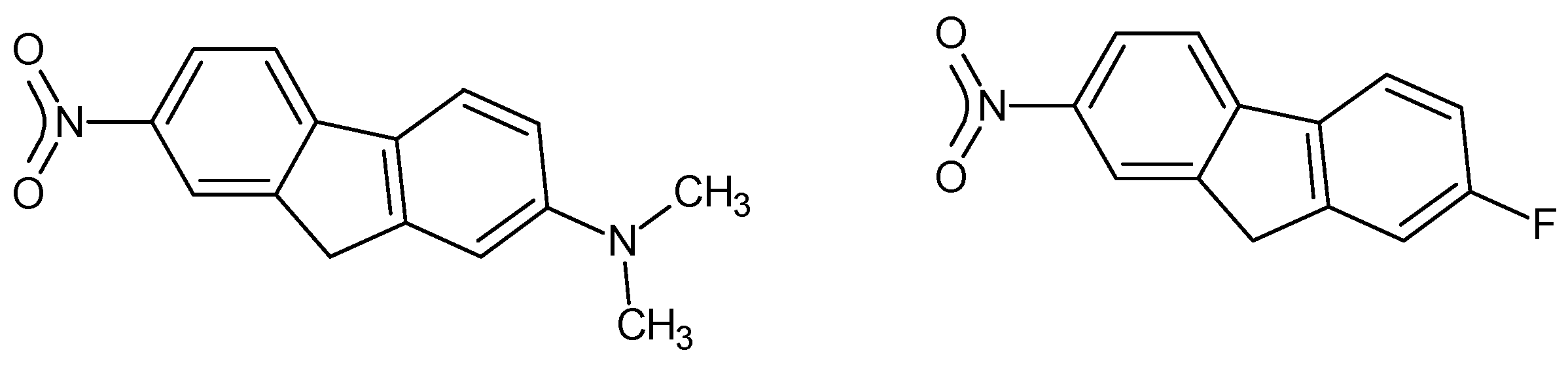
Catalan’s SPP^N is based on the differences in the solvatochromism of 2-(dimethylamino)-7-nitrofluorine and its homomorph, 2-fuoro-7-nitrofluorine (Figure 1), which show similar solvatochromism with that of 2-(dimethylamino)-7-nitrofluorine being substantially larger. This assumes that specific interactions, such as hydrogen bonding to the NO₂ moiety will be removed by the subtraction, leaving only effects from solvent polarity and polarizability.

In the analysis of SPP^N polychlorinated alkanes, flexible esters and pyridine were outliers and were excluded from the analysis. The analyses showed that only three solvent molecular properties: the dipole moment, quadrupolar amplitude and polarizability, showed significant correlations with SPP^N. The coefficients recovered from the analyses are listed in

Table 1. Comparison of the a_i values for Catalan SPP, Kamlet, Abboud and Taft’s π* and Reichardt and Dimroth’s $E_{\mathrm{T}}^{\mathrm{N}}\left(30\right)$.

	SPPN		π* b		${\mathit{E}}_{\mathbf{T}}^{\mathbf{N}}\left(30\right)$ c
	DF a	HF	DF	HF	DF	HF
Intercept	0.63 ± 0.02	0.63 ± 0.02	0.03 ± 0.04	0.01 ± 0.05	0.05 ± 0.02	0.05 ± 0.03
$a_{\mathrm{pol}}$	−0.14 ± 0.04	−0.18 ± 0.03	−0.51 ± 0.07	−0.58 ± 0.07	−0.24 ± 0.04	−0.32 ± 0.05
$a_{\mathrm{d}-\mathrm{pole}}$	0.35 ± 0.02	0.34 ± 0.03	0.63 ± 0.04	0.63 ± 0.04	0.19 ± 0.02	0.18 ± 0.03
$a_{\mathrm{q}-\mathrm{pole}}$	0.39 ± 0.05	0.49 ± 0.06	0.68 ± 0.07	0.74 ± 0.07	0.19 ± 0.04	0.21 ± 0.04
$a_{E_{\mathrm{Donor}}}$			0.40 ± 0.05	0.45 ± 0.05
$a_{q+}$ d					0.58 ± 0.02	0.61 ± 0.03
σ b	0.05	0.05	0.11	0.11	0.07	0.07

^a DF and HF indicate analyses based on properties calculated using density functional and Hartree–Fock calculation methods, respectively; uncertainties are standard deviations. ^b Ref. [12]; ^c Ref. [13]; ^d Charges calculated using Hirshfeld’s model [14].

. Also listed in Table 1 are the corresponding coefficients for Kamlet, Abboud and Taft’s π and Reichardt and Dimroth’s normalized $E_{\mathrm{T}}^{\mathrm{N}}\left(30\right)$. The SPP^N values calculated using the coefficients in Table 1 and solvent descriptors, Q_i, recovered from properties calculated using the density functional method are compared with experimental values in Figure 2 (the dashed lines are one standard deviation above and below the line representing perfect agreement); the plot using properties recovered from Hartree–Fock calculations is similar. The values for pyridine (brown circle below the line) and the polychloroalkanes (grey triangles) are included in Figure 2.

The values for the esters provide a cautionary example for the use of computational methods. For rigid molecules the molecular properties recovered from computational calculations are essentially determined by the method and basis set but for flexible molecules the properties may also vary depending on the assumed molecular structure. This was discussed previously [12] with regard to the variation of calculated properties of carboxylic acids with rotation around the C−OH bond of the acid group. It was found that the dipole moments and quadrupolar amplitudes of the acids depended strongly on the conformation but that the other properties did not. It was also found that all molecular properties were relatively insensitive to coiling of the alkyl chain of longer chain carboxylic acids.

In the case of the esters, the O=CR−O moiety will be planar while rotation around the C−O bond is relatively free. The variations in the calculated molecular properties with rotation around the C−O bond are shown, as normalized molecular descriptors, in

Table 2. Solvent descriptors ^a for esters with different molecular geometries.

	Propyl Formate–SPP = 0.815
O=C−O−R Dihedral Angle	Qd–pole	Qq–pole	Qpol	${\mathit{Q}}_{{\mathit{E}}_{\mathbf{Donor}}}$	${\mathit{Q}}_{{\mathit{E}}_{\mathbf{Acceptor}}}$	Qq−	Qq+	SPPcalc
0°	0.77	0.45	0.35	0.61	0.32	0.40	0.06	0.97
60° b	0.66	0.34	0.35	0.60	0.37	0.38	0.06	0.91
120° b	0.47	0.26	0.35	0.59	0.37	0.38	0.06	0.83
180° b	0.40	0.33	0.35	0.59	0.29	0.41	0.06	0.84
Average	0.58	0.35	0.35	0.60	0.34	0.39	0.06	0.89
	methyl acetate–SPP = 0.785
0°	0.78	0.20	0.27	0.66	0.29	0.42	0.08	0.91
60° b	0.67	0.20	0.27	0.66	0.32	0.40	0.08	0.88
120° b	0.46	0.32	0.27	0.67	0.32	0.41	0.08	0.86
180° b	0.33	0.34	0.27	0.64	0.25	0.42	0.08	0.84
Average	0.56	0.26	0.27	0.65	0.29	0.41	0.08	0.87
	ethyl acetate–SPP = 0.795
0°	0.79	0.30	0.35	0.67	0.29	0.43	0.08	0.93
180°	0.36	0.38	0.35	0.65	0.25	0.42	0.08	0.84
	propyl acetate–SPP = 0.795
0°	0.80	0.48	0.42	0.68	0.28	0.43	0.08	0.97
180°	0.38	0.40	0.42	0.66	0.25	0.42	0.08	0.84
	butyl acetate–SPP = 0.784
0°	0.81	0.61	0.50	0.68	0.28	0.43	0.08	1.00
180°	0.37	0.48	0.50	0.66	0.24	0.42	0.08	0.85
	methyl salicylate–SPP = 0.836
0°	0.19	0.70	0.65	0.86	0.47	0.40	0.22	0.85
60° c	0.27	0.61	0.64	0.85	0.42	0.40	0.24	0.84
120° c	0.34	0.53	0.64	0.85	0.42	0.40	0.25	0.83
180° c	0.44	0.51	0.65	0.87	0.48	0.37	0.19	0.84
Average	0.31	0.59	0.64	0.86	0.45	0.39	0.23	0.84

^a 0 ≤ Q_i ≤ 1; calculated from molecular properties using Equation (2); molecular properties from density functional calculations, Q_q− and Q_q+ are calculated using Hirshfeld’s model; ^b from single point calculations following rotation of the O=C−O−R dihedral angle of the optimized 0° structure; ^c 60° and 120° results are from single point calculations; angles are rotation of the benzene ring with respect to the O=CO−C plane; 0° corresponds to the OH group of the benzene ring being on the same side as the COC oxygen and 180° to the OH being on the side of the C=O.

; also shown are the corresponding SPP values calculated using the descriptors and the coefficients reported in Table 1.

Again, it can be seen that, while the variations are much smaller than those for the carboxylic acids, it is only the dipole moments and quadrupolar amplitudes that vary significantly with rotation around the C−O bond while the other molecular properties are relatively constant.

Results for methylsalicylate are included in Table 2, since rotation of the benzene ring progressively moves the O−H in the 2 position on the benzene ring away from the C−O−C oxygen to the C=O oxygen. In this case, the dipole moment essentially doubles between the 0° and 180° rotamers but, in the calculation of SPP^N, this is almost exactly compensated by the corresponding increase in the quadrupolar amplitude.

4. Discussion

Like SPP^N, both π* and $E_{\mathrm{T}}^{\mathrm{N}}\left(30\right)$ show positive dependences on the solvent dipole mements and quadrupolar amplitudes and a negative dependence on the solvent molecule’s polarizability. Each, however, shows an additional contribution.

In the case of $E_{\mathrm{T}}^{\mathrm{N}}\left(30\right)$, the additional contribution is from the charge on the most positive hydrogen atom on the solvent molecule and reflects hydrogen bonding at the pendant oxygen atom of the betaine dye used to define the scale. Of course, this is well known and was used by Kamlet and Taft to define their α scale of hydrogen bond acidity [4].

The reason for dependence of π* on the energy of the electron donor orbital of the solvent molecule is less clear. Both Catalan and Kamlet and Taft considered the possibility of hydrogen bonding to the NO₂ group on the aromatic probe molecules, but if this were the case one would expect a correlation with the charge on the most negative atom of the solvent molecule, which is the dominant contribution to measures of hydrogen bond basicity (Kamlet and Taft’s β [9,12], Gutmann’s donor number [9], Abraham’s B [17] and Catalan’s SB [10]).

Leaving these aside, it is interesting that all three parameters show positive correlations with the solvent’s molecular dipole moment and quadrupolar amplitude and a negative correlation with the solvent molecule’s polarizability.

Since these are intended as measures of a solvent’s polarity and polarizability, it is reasonable to associate the polarity with the dipole moment and quadrupolar amplitude. That is, the polarity is related to the intensity of the permanent charges imbedded in the solvent.

The basis of solvent parameters based on solvatochromism is that the equilibrium solvation of the ground state interacts with the excited state of the probe (By the Franck–Condon principle, the time scale of the electronic transition is vastly less than the time scale of molecular motions, so that, initially, the excited state interacts with the ground state solvation shells.) and so, if, for example. the excited state of the probe has greater charge separation than the ground state (This situation leads to an inverse relationship between the solvent parameter and the energy of the electronic transition; that is, the larger the energy gap the smaller the solvent parameter. The reverse situation, where the ground state has greater charge separation leads to a direct relationship; that is, to parameters that increase as the transition energy increases.), it will interact more strongly with the surrounding solvation shells, lowering the excitation energy.

It is implicit in the design of all based on solvatochromism, that all solute–solvent interactions will be stronger with the form of the solute with the greatest charge separation. This assumption is generally found to be correct; thus, basicity scales are positively correlated with the partial charge on the most negative atom and the energy of the donor orbital [9,10,12,17] and acidity parameters are positively correlated with the partial charge on the most positive hydrogen atom [10,11].

As is clear from Table 1, the polarity/polarizability parameters show positive correlations with the dipole moment and quadrupolar amplitude, measures of the intensity of charges imbedded in the surrounding solvent, but negative correlations with the molecular polarizability of the solvent. That is, increasing polarizability leads to decreases in SPP^N, π* and $E_{\mathrm{T}}^{\mathrm{N}}\left(30\right)$.

The expectation that polarizability should correlate positively with these experimental parameters essentially arises from the assumption that charge–induced dipole interactions will dominate. However, solvent molecules may also interact with the probe molecules by London forces, in which case the stronger interaction is likely to be with the form with the lower charge separation, the ground state in the above case, where the excited state has the greater dipole moment. The observed negative correlation between SPP^N, π* and $E_{\mathrm{T}}^{\mathrm{N}}\left(30\right)$ the polarizability of the solvent molecules, argues that this latter interaction dominates.

Whatever the explanation, SPP^N, π* and $E_{\mathrm{T}}^{\mathrm{N}}\left(30\right)$ all show the same negative correlation with the solvent molecular polarizability, which makes their use in linear free energy relationships problematic. Thus, solvents with equal values of SPP^N, for example, can have quite different polarizabilities, compensated by differences in the dipole moments and/or quadrupolar amplitudes.

5. Conclusions

The analysis of SPP^N shows that, like Kamlet and Taft’s π* and Reichardt’s $E_{\mathrm{T}}^{\mathrm{N}}\left(30\right)$, shows positive correlations with the molecular dipole moment and quadrupolar amplitude of solvent molecules and a negative correlation with the molecular polarizability.

Since these parameters are measures of the solvent polarity and polarizability it is reasonable to ascribe the solvent polarity to the permanent charges on the solvent molecules. In the approach used here, the dipole moment and quadrupolar amplitude are used as measures of the intensity of these charges. Thus, the solvent polarity is determined by the permanent charges in the solvent molecule and acts by non-specific interactions of these permanent charges with permanent charges on the solute. In contrast, solvent polarizability is determined by the polarizability of the individual solvent molecules and involves interactions with the non-polar parts of the solute through London forces.

It seems surprising that these experimental parameters reflect London rather than the expected charge/dipole–induced dipole interactions. However, the experimental parameters are based largely on probe molecules containing one or more aromatic rings, generally one for π* [6], two for SPP^N [7] and six for $E_{\mathrm{T}}^{\mathrm{N}}\left(30\right)$ [8], and so are likely to interact well through London forces.

The observation that the polarity and polarizability act on the experimental parameters in opposite directions, increasing polarity increasing the values of the parameter while increasing polarizability decreases the values creates an obvious difficulty in the use of these parameters in linear free energy relationships, for example.