The Relationship between Bedrock Depth and Site Fundamental Frequency in the Nakdonggang Delta Region, South Korea

Abstract

This paper describes the relationship between bedrock depth (D) and site fundamental frequency (f₀) in the Nakdonggang delta region in the southeastern part of the Korean peninsula. We collected borehole logs to confirm the thickness of the sediments and estimated the f₀ at over 200 locations across the delta using the horizontal-to-vertical spectral ratio (HVSR) method. We developed an f₀ map of the study area by spatially interpolating the f₀ values using the Ordinary Kriging method. The bedrock depth in the main delta showed a power-law dependence on the f₀. The derived f₀–D model predicted much shallower bedrock depths compared with similar studies from other parts of the world. This was attributed to the fact that the Nakdonggang delta region is composed of relatively low V_s Holocene sediments. With an f₀ map, the derived model could enable a quick estimation of the bedrock depth, which could help to determine the site class in the Nakdonggang delta region according to the Korean Seismic Design Standard (KDS 17 10 00).

1. Introduction

Ground motions can be amplified and lengthened due to the constructive interference and trapping of waves in sedimentary layers [1]. This phenomenon, known as site amplification effects, has been demonstrated by numerous case studies of historical earthquakes. Following the 1985 Mw 8.0 Mexico City earthquake, seismic stations installed on sedimentary sites recorded ground motions significantly larger than those installed on rocky sites [2]. Seed et al. [3] suggested that, during the Loma Prieta earthquake (Mw 6.9, 1989), the damage was concentrated in the San Francisco Bay area due to the site amplification effect in the deep sedimentary layers consisting of marine deposits. During the Kocaeli Earthquake (Mw 7.4, 1999), many buildings collapsed in Avcilar, which is located around 150 km away from the epicenter. Karagoz et al. [4] observed that the peak ground acceleration (PGA) was amplified by the deep sedimentary layer to four to five times larger than that of other stations installed on rock sites.

Observations from historical earthquakes have demonstrated that characterizing site amplification effects plays an important role in predicting the intensity of ground motions and that the design ground motions must account for the site effects. Ground motion amplification can be accounted for by either applying the correction factors specified in the seismic design codes or performing ground response analyses. Although code-based correction methods are generally more conservative, they are much more widely applied due to their simplicity in comparison with site-specific ground response analyses, which require a detailed characterization of the site’s dynamic properties and knowledge on the dynamic site response analysis [5].

In the Korean Seismic Design Standard [6], sites are classified based on the bedrock depth (D) and the average shear wave velocity of the soil layer (V_{s, soil}), as shown in

Table 1. Site classification criteria in KDS 17 10 00 [6].

Site Class	Type	Classification Criteria
		D [m]	Vs, soil [m/s]
S1	Rock	<1	-
S2	Shallow and Stiff	1~20	≥260
S3	Shallow and Soft		<260
S4	Deep and Stiff	>20	≥180
S5	Deep and Soft		<180
S6	Requires characterization of site-specific dynamic soil properties and ground response analysis to determine the design ground motion

. Different amplification factors are then applied to the design response spectrum according to the site classification. For example, the site class S1, corresponding to the rock site, only considers the amplification of the short-period ground motion. However, other classes consider the amplification of the long-period ground motion in the design response spectrum. Therefore, it is necessary to determine the bedrock depth and average shear wave velocity of each site to account for the site amplification effect according to the KDS 17 10 00 [6].

The D and V_{s, soil} for each site can be determined directly by performing a geotechnical investigation (i.e., drilling boreholes) and geophysical exploration. However, direct measurements are costly and time-consuming. The D and V_{s, soil} can also be determined from a pre-existing geotechnical investigation database, if a boring log exists near the target site. However, in the database, boring logs with stratigraphic data that reach the depth of the bedrock are scarce where it is deep, and the amount of geophysical data to confirm the V_{s, soil} are also insufficient. Therefore, it is necessary to develop an alternative approach that will enable a more convenient way of determining the site class.

For a uniform homogeneous soil layer, the relationship between the shear wave velocity (V_s), the thickness of the soil layer above the bedrock (D), and the natural frequency of the ground (f₀) can be expressed by the following equation [5]:

f₀ = V_s/4D,

(1)

which shows that f₀, D, and V_s are related to each other, and it is also possible to determine the remaining parameter if two of them are known. However, sedimentary soils are always heterogeneous, and the V_s of soils is usually dependent on the effective confining pressure. A direct application of Equation (1) is not always guaranteed.

Previous studies have shown that the relationship between f₀ and D can be expressed by a power-law model, which is shown in Equation (2) [7,8,9,10]:

D = af₀^b_,

(2)

where a and b are constants specific to the regional geologic characteristics. These models show that the constants a and b can be strongly dependent on the regional geologic characteristics, meaning that it is necessary to develop a region-specific model that considers the local geologic properties.

The influence of site amplification would be significant in the study area due to the presence of widely distributed loose Holocene sediments, which are as deep as eighty meters. The goal of this study was to establish a relationship between the f₀ and D in the Nakdonggang delta region, with which one can quickly estimate the bedrock depth and determine the seismic site class in accordance with KDS 17 10 00.

We estimated the f₀ at multiple sites in our previous study using the microtremor horizontal to vertical spectral ratio (HVSR) method [11]. Additionally, we collected bedrock depth data from the geotechnical investigations that had been conducted in the study area. We developed a power-law model of the bedrock depth in the study area using a log-log regression of the collected f₀ and D data. We then compared the model with similar studies conducted in other regions to investigate the difference in the f₀–D correlation model based on regional characteristics.

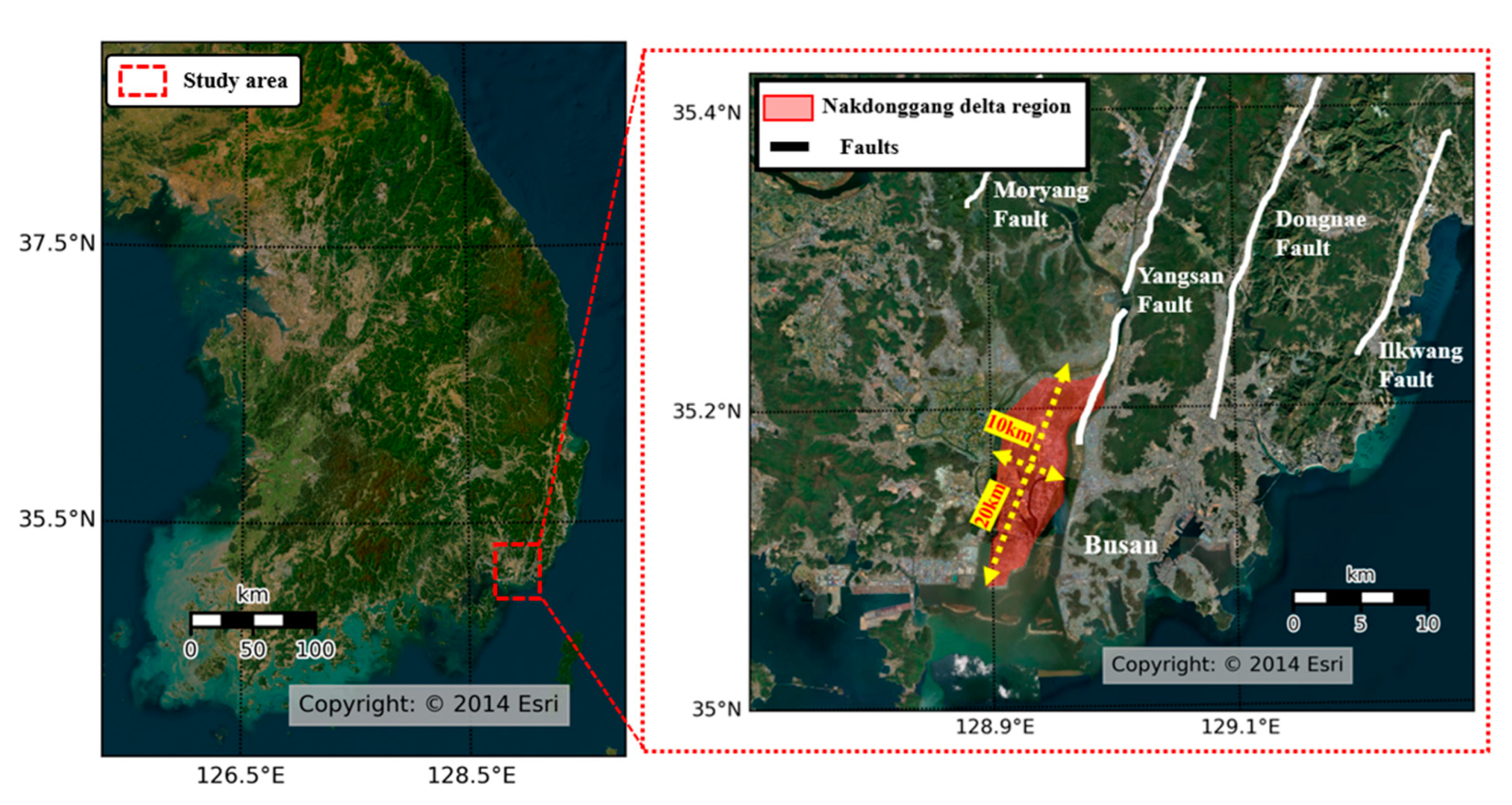
2. Study Area

The Nakdonggang delta region is in the southeastern part of the Korean peninsula, as shown in Figure 1. It is the largest delta area in the Korean peninsula and is surrounded by various faults and mountains. An extensional basin was formed by a half-graben structure bounded by the Yangsan fault, as shown in Figure 1. The main delta is approximately 10 km wide and 20 km long, with an exposed land area of around 136 km², a plains area, and several interdistributary islands [12]. The southeastern part of the Korean peninsula is known to have a higher seismic hazard compared with the rest of the country [13].

The Nakdonggang delta area is characterized by layers of deltaic, marine, and fluvial sediments that cover a wide area, as shown in Figure 1. The maximum thickness of the sediments overlying the bedrock is approximately 80 m. Holocene sediments consist of a sandy layer (deltaic sediments) up to around 15 m below the ground surface, overlying the remaining layer, consisting of thick marine clay [14]. According to Ham et al. [15], the study area was dominated by a fluvial environment during the late Pleistocene, due to low sea levels. Fluvial deposits, consisting of granular to fine sand and small amounts of gravel, were formed on top of the bedrock at a maximum observed depth of around 50 m. The sea level then gradually rose for a long period, leading to the formation of marine deposits containing a small amount of shells on top of the late Pleistocene fluvial deposits. The sea level then steadily decreased to its current level, resulting in the formation of the present sedimentary delta at the end of the Holocene. Therefore, it is expected that the ground dynamic characteristics at different depths may vary depending on the formative process, while it is also expected that layers that were deposited during the same era may have similar ground dynamic characteristics.

3. Methodology

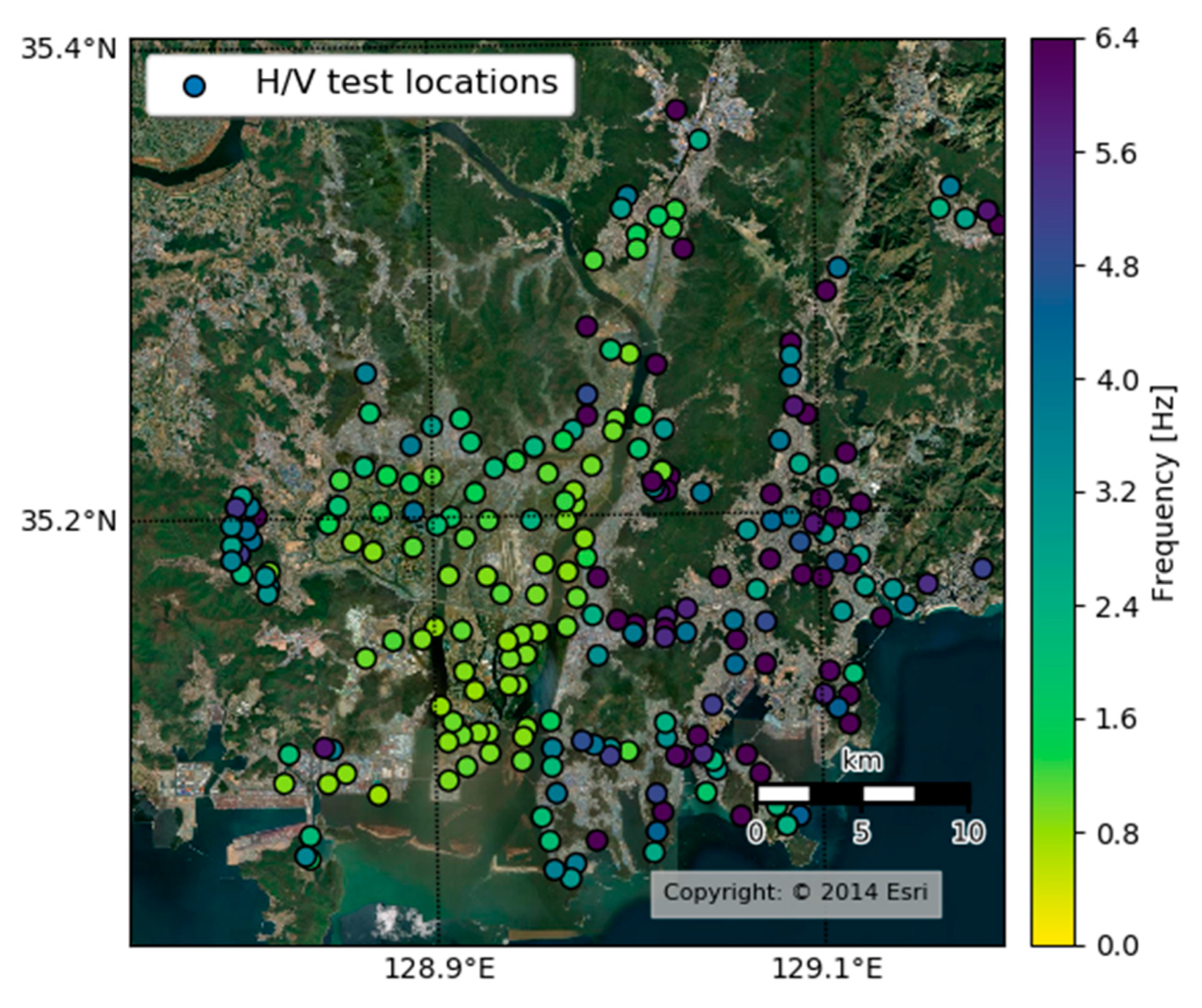
3.1. Characterization of f₀ Based on the HSVR Method

The HVSR method is a geophysical exploration method that provides a convenient way of estimating the fundamental vibration frequency (f₀) of sedimentary soils. It was first proposed by Nogoshi and Igarashi [16], and later, Nakamura [17] argued that the 1D transfer function of a site can be defined through the horizontal-to-vertical Fourier spectral ratio of the microtremor. It has become popular since the 1990s and many researchers have effectively used HVSR for the characterization of site amplification properties [18,19,20,21].

The origin of HVSR peaks and their relationship with site response characteristics are still controversial. For example, Nogoshi and Igarashi [16] suggested that the main contribution to ambient noise originates from Rayleigh waves and HVSR peaks represent the ellipticity of these Rayleigh waves. In contrast, Nakamura [17] argued that HVSR peaks are mainly due to S-wave resonance within the surficial sedimentary layer. Other studies have pointed out that the ambient noise on the ground surface is composed of different types of waves, including body, Rayleigh, and Love waves, and their proportions vary depending on the site conditions and source characteristics [22,23]. Some studies have attempted to isolate Rayleigh waves from Love waves within noise recordings for a more reliable identification of site amplification properties [24,25,26].

Nevertheless, many studies have confirmed that the peak frequency of microtremor HVSR generally corresponds to the fundamental frequency of the 1D transfer function of each site [18,19,20,21]. Bard [27] explained that HVSR peaks are very close to the site fundamental frequencies at sites with a strong impedance contrast, because the vertical components of Rayleigh waves tend to vanish near the S-wave resonance frequency. We used the microtremor HVSR method to estimate the f₀, and Figure 2 shows a typical example of an HVSR obtained in the study area.

To estimate the spatial distribution of the fundamental site frequencies in the study area, over 200 test locations were selected at spacings of about 1 km, as shown in Figure 3, and microtremor data were recorded for at least 1 hour at each location.

The microtremor data were recorded using Raspberry Shake 3D (Raspberry Shake, S.A., Panama) and Trillium Compact 20 s seismometers (Nanometrics Inc., Canada), shown in Figure 4, with a sampling rate of 100 samples per second. Raspberry Shake 3D is a geophone-based velocity sensor with a flat response in 2 s to 50 Hz. Trillium Compact 20 s is a force feedback velocity seismometer with a flat response in 20 s to 100 Hz. We used both types to expedite our field work. We conducted a huddle test before the field work to ensure all the sensors produced consistent outputs. To ensure a good coupling, we fixed the seismic sensors on the ground by combining spikes with an aluminum plate. The seismometers were aligned to the magnetic north using a compass and levelled using built-in bullseye bubbles.

We obtained the HVSR from the obtained microtremors using the Geopsy 2.10.1 software suite (https://www.geopsy.org (accessed on 7 July 2020)), applying the Konno-Ohmachi smoothing method [28] with a smoothing constant of b = 40. Within the analysis, the recorded data were divided into multiple time windows of 180 s long and the frequency sampling was done logarithmically from 0.1 to 50 Hz. The value of the f₀ was obtained from all time windows and, subsequently, the mean and standard deviation were calculated. Figure 3 also shows the f₀ at the studied sites, obtained using the HVSR method described above. Most sites located inside or near the Nakdonggang delta region showed a low f₀ of around 1 Hz, while others located near mountains showed higher values.

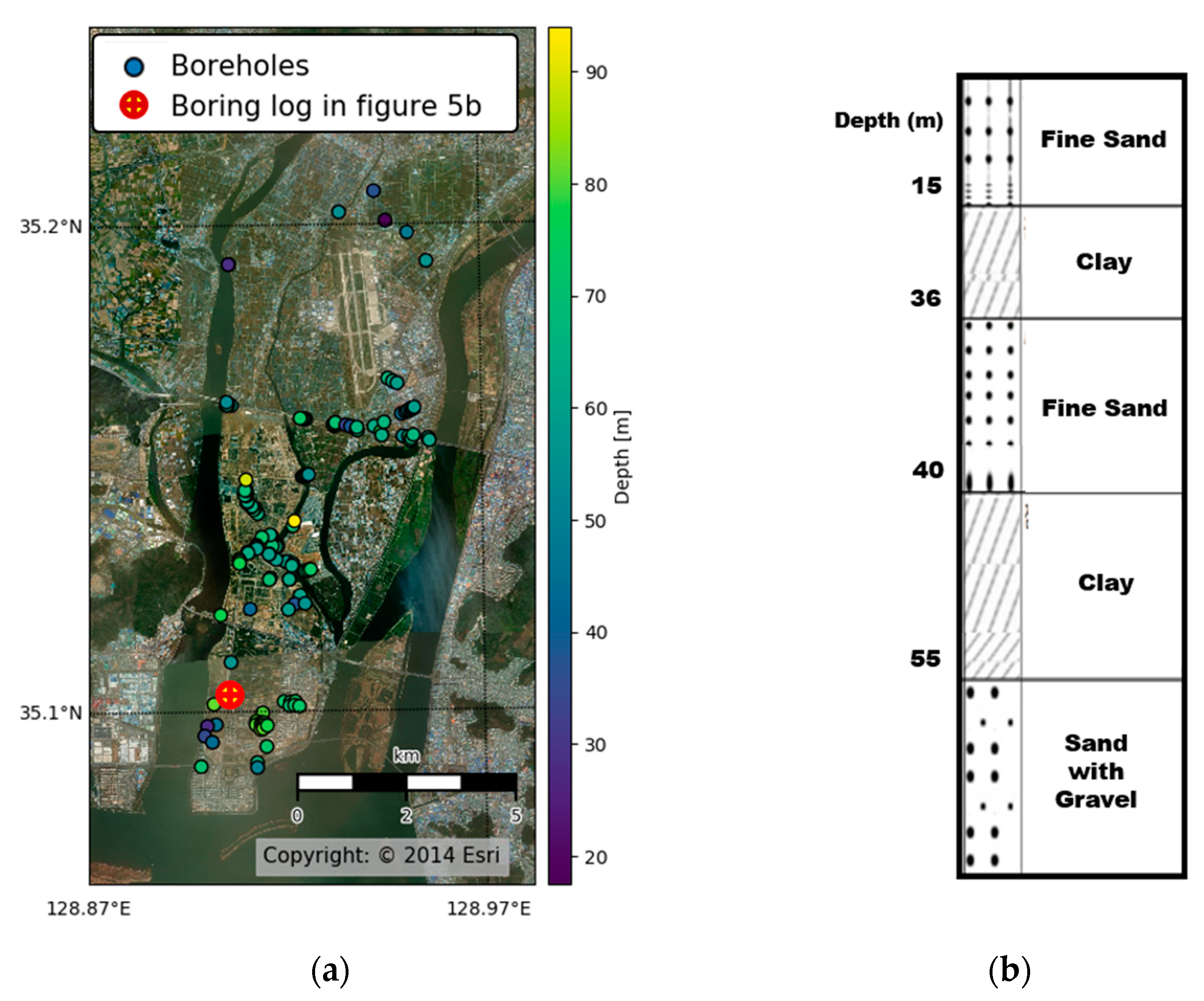
3.2. Bedrock Depth Obtained from Borehole Data

To derive the relationship between the f₀ and D, we collected boring logs from the Korean geotechnical investigation database system (www.geoinfo.or.kr (accessed on 6 March 2023)) in the study area. Figure 5a shows the bedrock depth logged at each location and Figure 5b provides a typical example of the stratigraphy in the study area, which is consistent with the known sedimentation history of the study area, as suggested by Ham et al. [15]. The boring logs showed that the bedrock depth in the study area was predominantly around 60 m, while at some sites towards the south, it reached up to 90 m. Overall, there was a relatively large variability in the bedrock depth in the study area. Based on Figure 5b, it was also expected that there would be no significant impedance contrast until the bedrock is reached.

3.3. Estimation of f₀ at Each Borehole by Spatial Interpolation

Based on the results of the HVSR, we created an f₀ map of the Nakdonggang delta region (Figure 6a) to confirm the spatial distribution of the f₀ in the study area. We used the Ordinary Kriging method to spatially interpolate the f₀ data of the study area with intervals of around 30 m. We applied a variogram with 3.82, 5.32, and 12,492 as “Sill”, “Nugget”, and “Range”, respectively. The spatial distribution of the variance is illustrated in Figure 6b. Based on the f₀ map of the Nakdonggang delta region, we can confirm that most of the study area had an f₀ around 1 Hz and showed a relatively small variability compared with other areas.

We then extracted the f₀ values from the interpolation results (Figure 7a) within the main delta that were closest to each borehole location, in order to investigate the relationship between the f₀ and bedrock depth at each site. Figure 7b shows the specific locations.

4. Result and Discussions

Ibs-von Seht and Wohlenberg [7] developed the correlation between the bedrock depth and f₀ in the western Lower Rhine Embayment of Germany. Parolai et al. [10] produced a similar model for the Cologne area (Germany). Jeong and Wotherspoon [8] applied the same technique in the Waikato region (New Zealand) and their results were overall consistent with those of the two previous studies. Moon et al. [9] derived the correlation between the f₀ and D in the Bukit Timah granite formation (Singapore) and found that their model showed a much smaller negative exponent compared with other studies. The specific model parameters and conditions of each study are summarized in

Table 2. Correlation coefficients in Equation (2) and conditions of previous studies.

Study	Location	a	b	Range of f0 [Hz]	Range of D [m]
Ibs-von Seht and Wohlenberg [7]	Western Lower Rhine Embayment, Germany	96	−1.388	0.1~5.0	15~1600
Parolai et al. [10]	Cologne, Germany	108	−1.551	0.5~10	0.5~400
Jeong and Wotherspoon [8]	Waikato Basin, New Zealand	101.6	−1.565	0.2~0.7	200~1100
Moon et al. [9]	Bukit Timah Granite Formation, Singapore	92.5	−1.06	2~9	10~50
This Study	Nakdonggang Delta Region, South Korea	55.5	−1.315	0.8~1.3	20~90

.

Using the bedrock depth from the borehole data and the interpolated f₀, we derived a f₀–D correlation model for the Nakdonggang delta region by performing a log-log regression analysis between the ln f₀ and ln D, as shown in Equation (3):

ln D = (4.017 ± 0.026) − (1.315 ± 0.232) ln f₀,

(3)

which can be converted back into a power function, as shown in Equation (4):

D = 55.5 f₀^−1.315_.

(4)

The coefficient of determination from the regression was 0.2, which is relatively low. Equation (3) shows that the standard error for coefficient b was relatively large. We attribute this to the relatively narrow range of fundamental frequencies combined with the inherently large variability in the bedrock depth from the boring logs.

Figure 8 shows the results of this study in comparison with similar studies conducted in other parts of the world. Some of the previous studies have shown a good agreement with each other, but the results of this study indicate much shallower bedrock depths given the same frequency in comparison with others. This suggests that the bedrock depth would be overestimated if the results of previous studies were used in the study area. Among the previous studies, the slope of the Ibs-von Seht and Wohlenberg [7] model was the closest to the result of this study; the slopes of other models, except for the Moon et al. [9] model, also fell within the standard error of this study.

However, coefficient a in Equation (4) from our study was much lower than that in all the other studies listed in Table 2. Coefficient a in Equation (4) gave the average estimate of the bedrock depth when f₀ = 1, and a lower value would suggest a lower average V_s. Our results summarized in Table 2 and Figure 8 suggest that the Holocene sedimentary soils considered in this study showed a much lower average V_s compared with the previous studies considered.

We calculated the residuals between the predicted bedrock depths, obtained from the power-law model defined in Equation (4), and the bedrock depths obtained from the borehole data. In Figure 9, the red line and filled area represent the moving average and 67% confidence interval, respectively, which were calculated by applying windows of 24 data points. By trialing the moving average with different binning schemes, we confirmed that using windows of 24 points effectively represented the variations in the means and standard deviations of the residuals. Figure 9 shows that the power-law model of the bedrock depth derived in this study was consistent with the observation and the standard deviations of the residuals were generally smaller than 0.3. This suggests that a measurement of the f₀ using the HVSR method could provide a quick estimate of the bedrock depth in the Nakdonggang delta region and valuable input for regional seismic hazard studies and regional-scale seismic performance assessments of structures [29,30,31,32]. However, the inconsistency between the empirical models demonstrated in Figure 8 suggests that the relationship between the f₀ and bedrock depth is region-specific.

5. Conclusions

We estimated the f₀ at over 200 locations using the HVSR method and collected geotechnical boring logs to determine the D in the Nakdonggang delta region in the southeastern part of the Korean Peninsula. We spatially interpolated the obtained f₀ using the Ordinary Kriging method and developed an f₀ map of the study area. We then derived an f₀–D correlation model for the Nakdonggang delta region as a power-law function and compared it with previous studies from other regions. The conclusions from this study can be summarized as follows:

• The HVSRs from the collected microtremor show that most sites located in or near the Nakdonggang delta region show a low f₀ of around 1 Hz, which corresponded to the fundamental mode vibration of the Holocene sediments. This suggests that the ground motions observed in this region would be rich in f ≥ 1 Hz due to the amplification in the fundamental and higher modes;
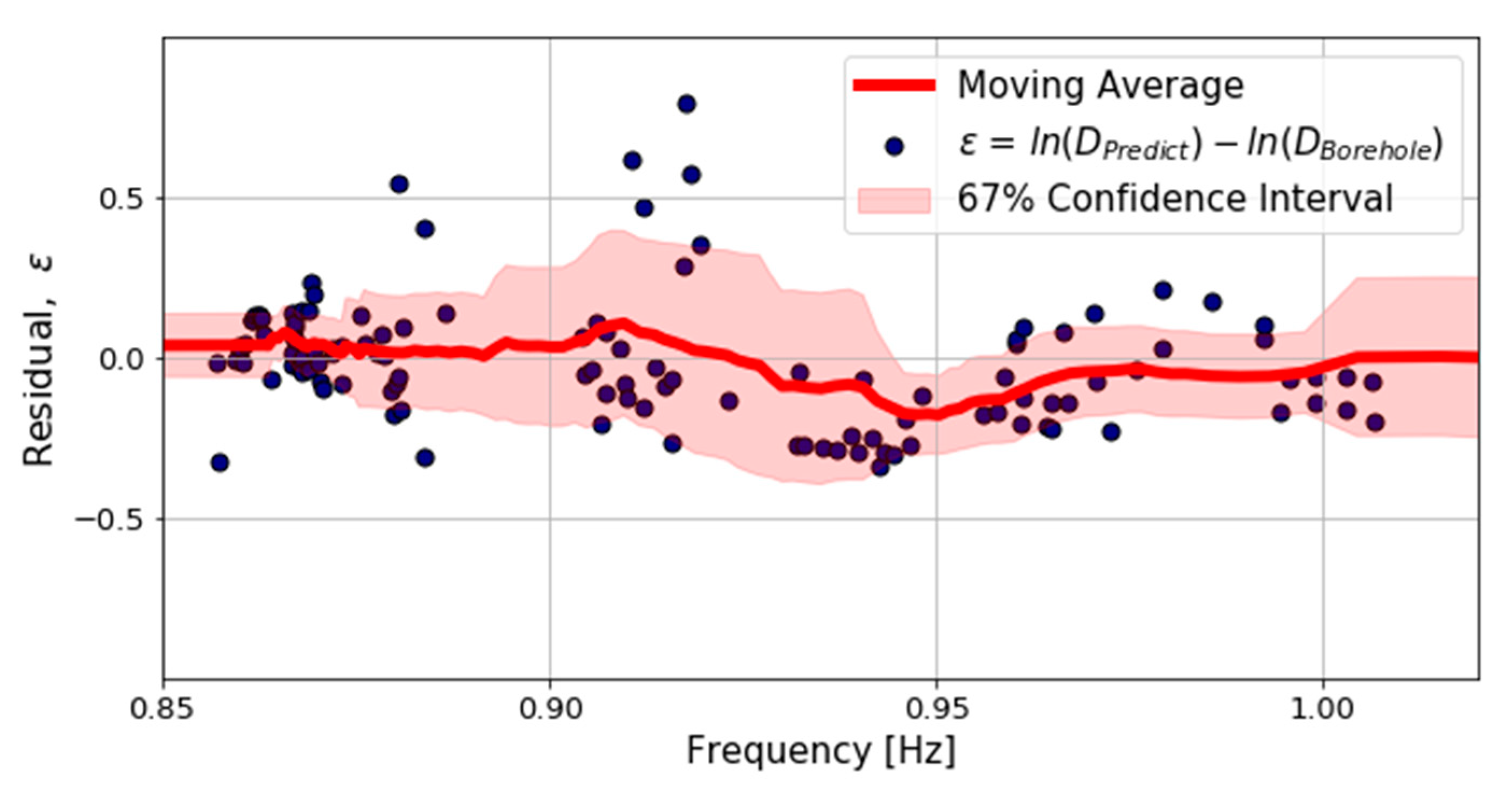
• Using the spatially interpolated f₀ and D from the boring logs, we developed an f₀–D correlation model. Our model predicted much shallower bedrock depths compared with those in previous studies, which was attributed to the relatively lower V_s of the Holocene sediments in the studied area. It also suggested that the relationship between the f₀ and bedrock depth is region-specific;
• The predicted bedrock depth from the f₀–D correlation model was in good agreement with the observations and the standard deviations of the residuals were generally smaller than 0.3. This suggests that a measurement of the f₀ using the HVSR method could provide a quick estimate of the bedrock depth for determination of the site class in the Nakdonggang delta region.