3-D Rock Mass Strength Criteria—A Review of the Current Status
Abstract
:1. Introduction
2. Physical Modeling Applications to Estimate Rock Mass Strength
2.1. Large Scale In-Situ Tests
2.2. Jointed Block Testing with a Significant Number of Fractures in the Laboratory
3. Empirical Rock Mass Strength Criteria
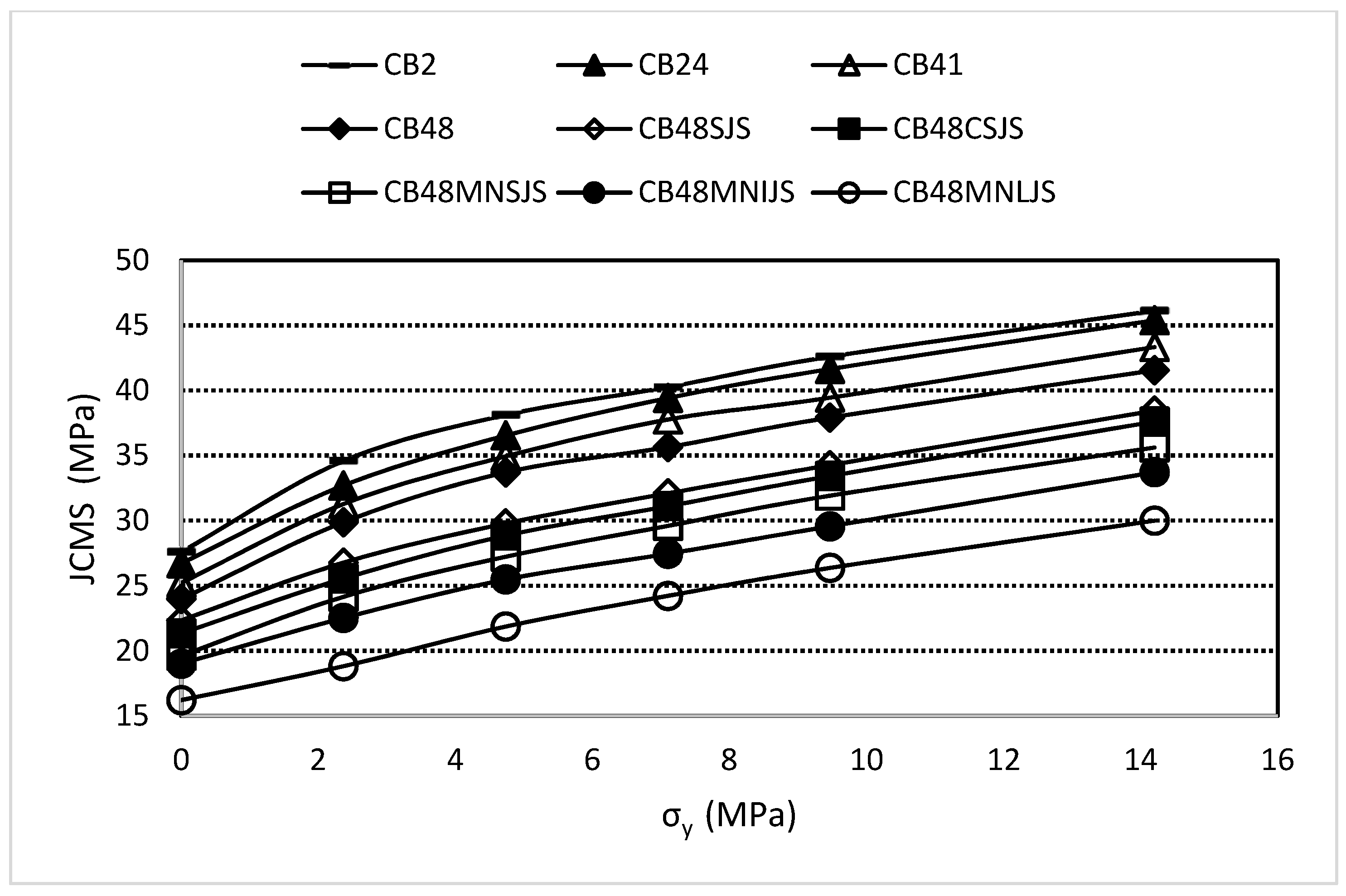
3.1. Mohr–Coulomb and Hoek–Brown Strength Criteria
- (a)
- For better quality rock masses (GSI > 25): for RMR76 > 18, GSI = RMR76 and for RMR89 > 23, GSI = RMR89 − 5.
- (b)
3.2. Yudhbir et al., Shoerey et al., and Ramamurthy Strength Criteria
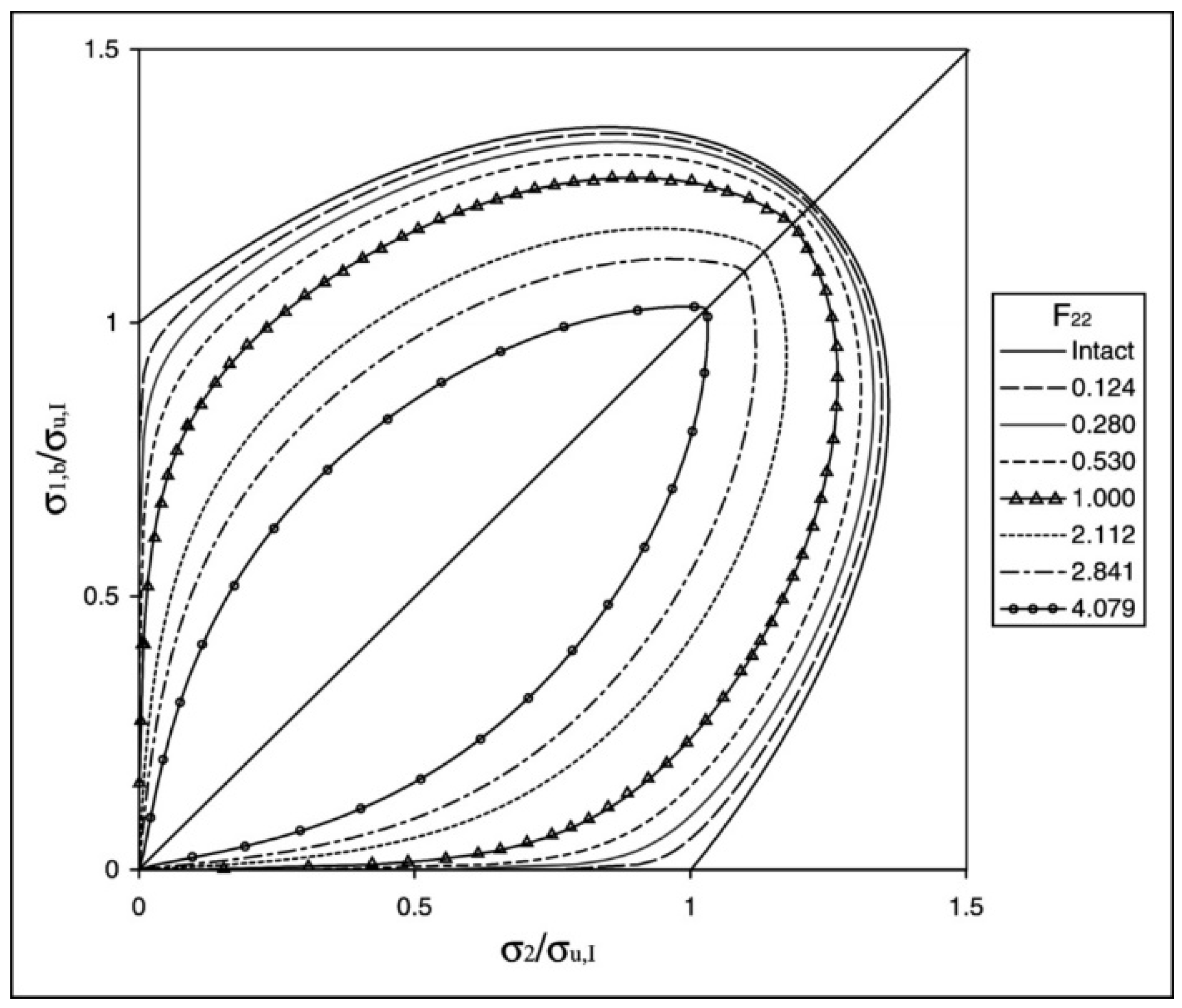
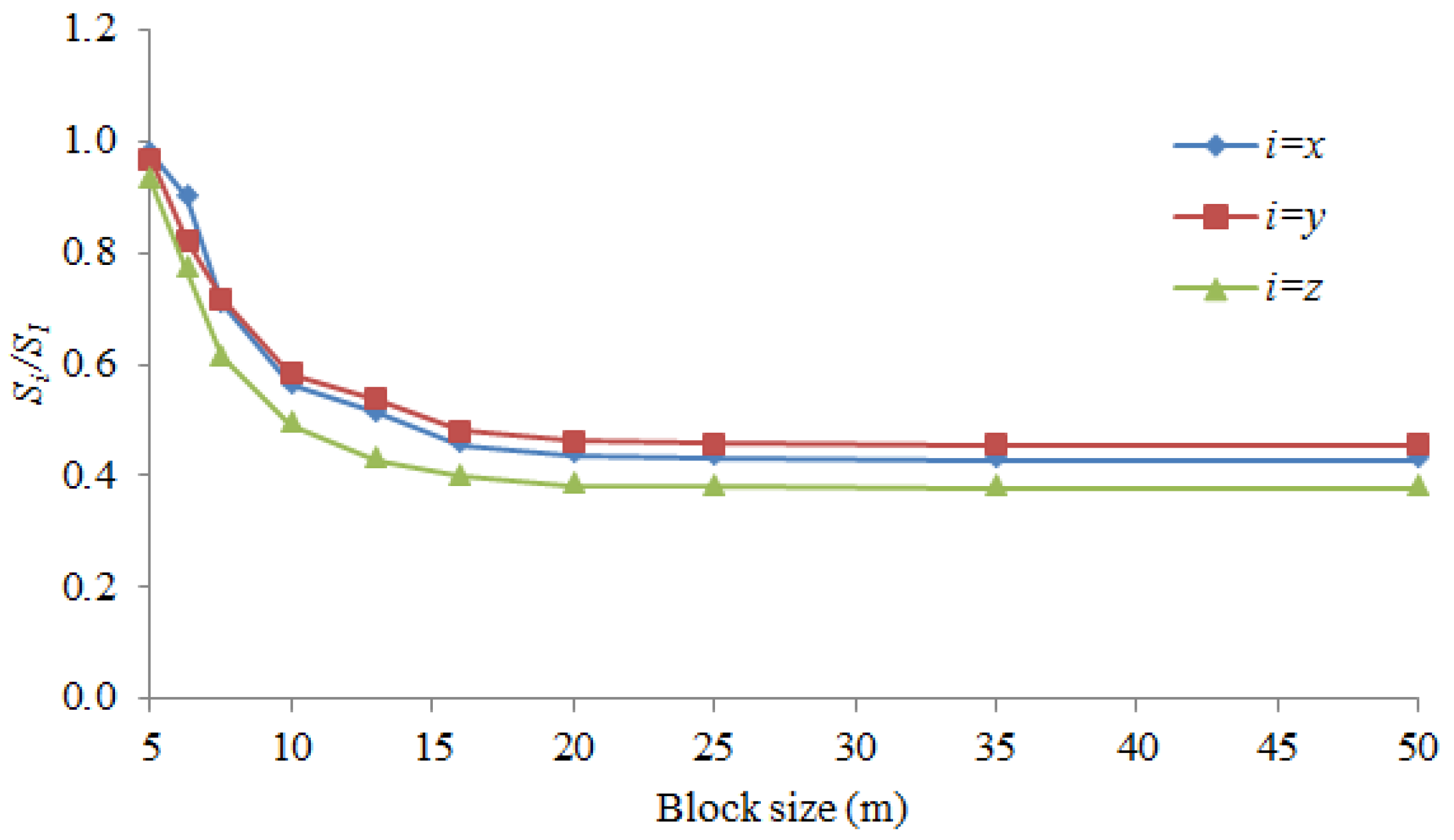
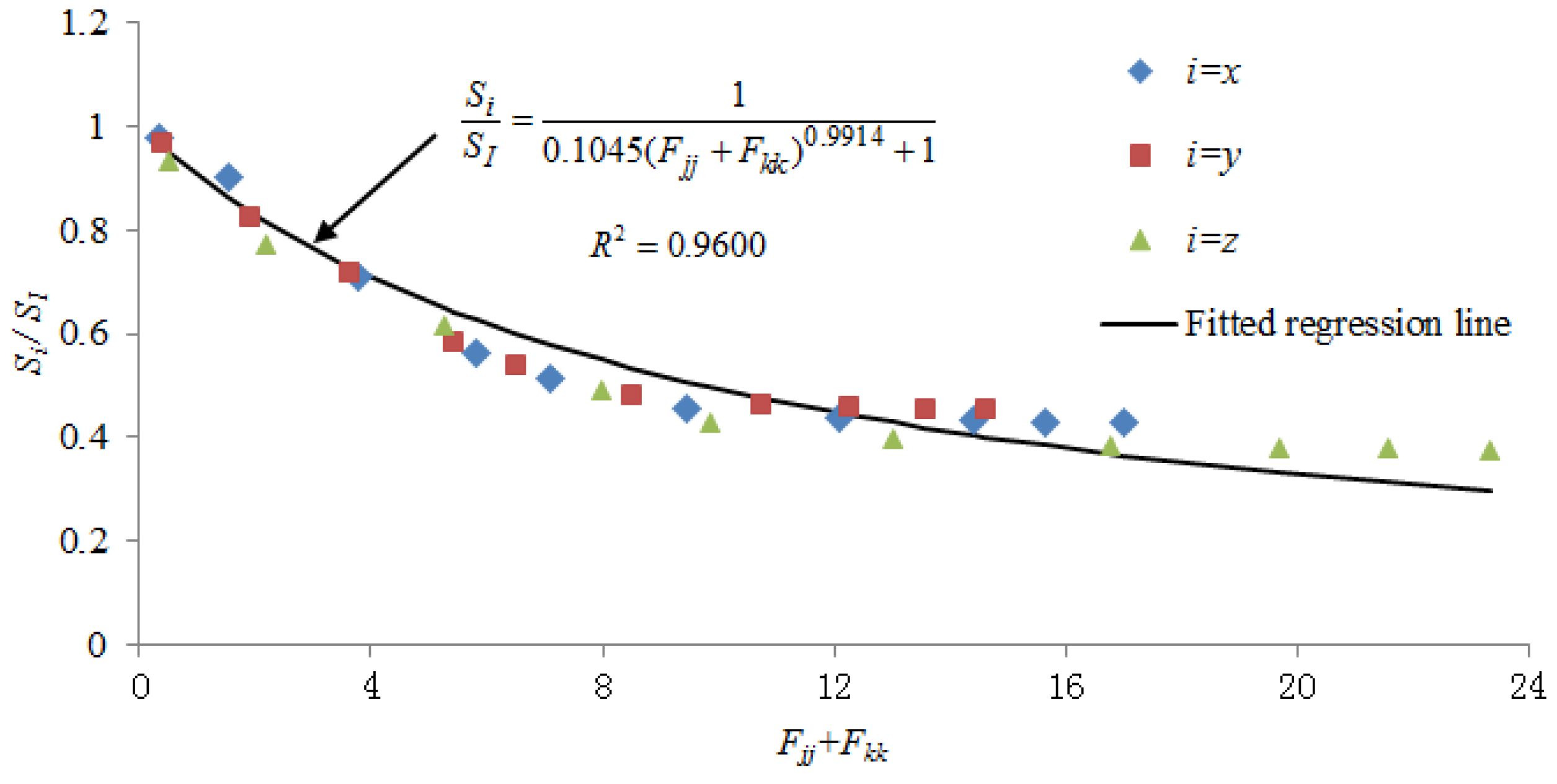
3.3. Kulatilake et al. Strength Criterion
4. Analytical Modeling Applications to Estimate Rock Mass Strength
5. Numerical Modeling Applications to Estimate Rock Mass Strength
5.1. FEM-Based Numerical Modeling Applications to Estimate Rock Mass Strength
5.2. Distinct Element Based Numerical Modeling Applications to Estimate Rock Mass Strength
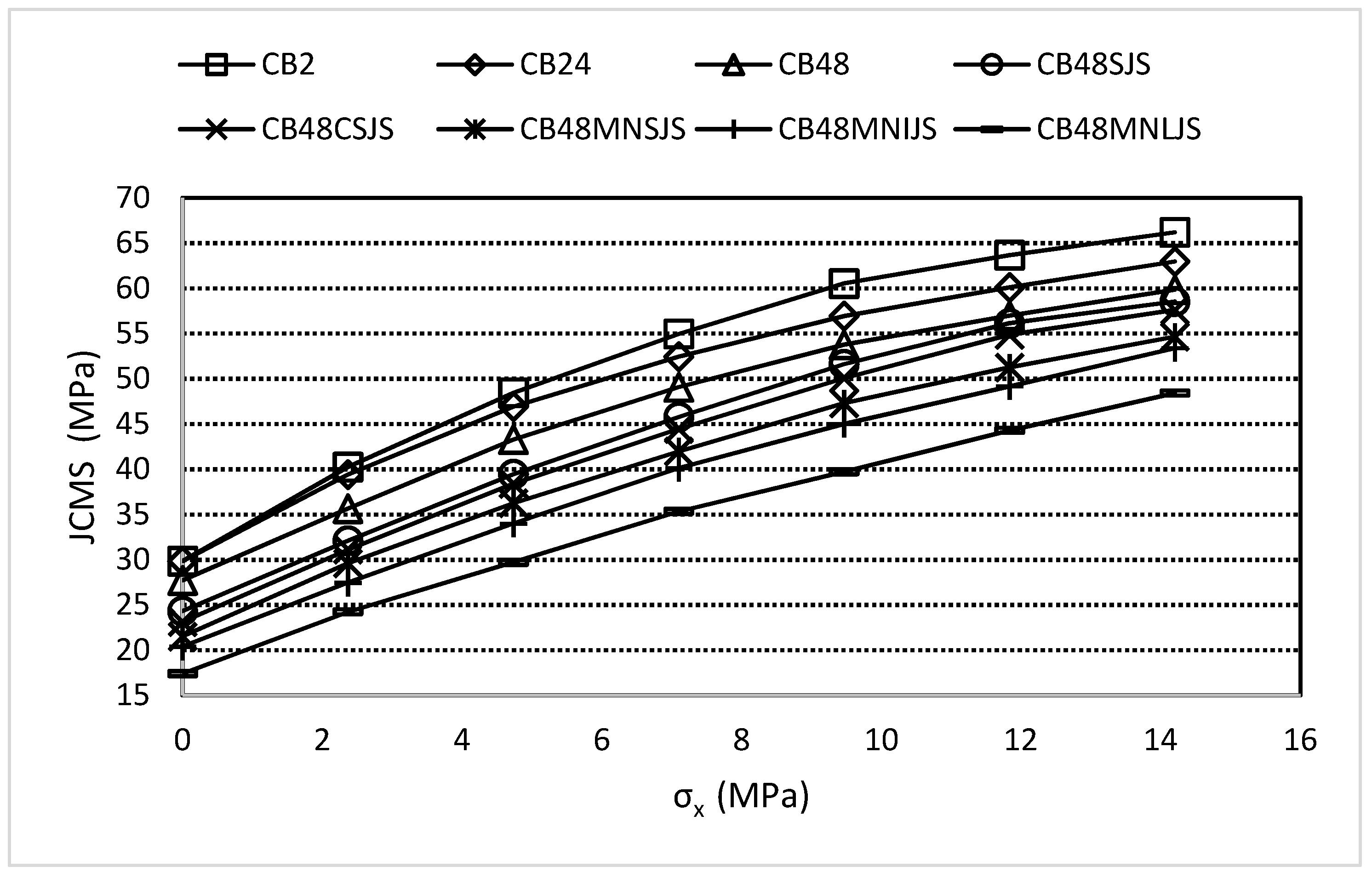
5.3. PFC Based Numerical Modeling Applications to Estimate Rock Mass Strength
6. Needed Improvements for the Currently Existing Rock Mass Strength Criteria
7. Conclusions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Kulatilake, P.H.S.W. Estimating Elastic Constants and Strength of Discontinuous Rock. J. Geotech. Eng. ASCE 1985, 111, 847–864. [Google Scholar] [CrossRef]
- Kulatilake, P.H.S.W.; Wang, S.; Stephansson, O. Effect of Finite Size Joints on Deformability of Jointed Rock at the Three-Dimensional Level. Int. J. Rock Mech. Min. Sci. 1993, 30, 479–501. [Google Scholar] [CrossRef]
- Wu, Q.; Kulatilake, P.H.S.W. REV and its properties on fracture system and mechanical properties, and an orthotropic constitutive model for a jointed rock mass in a dam site in China. Comput. Geotech. 2012, 43, 124–142. [Google Scholar] [CrossRef]
- Greenwald, H.P.; Howarth, H.C.; Hartman, I. Experiments on Strength of Small Pillars of Coal in the Pittsburgh Bed; Technical Report Number BM-TP-605; United States Government Printing Office: Washington, DC, USA, 1939. [Google Scholar]
- Greenwald, H.P.; Howarth, H.C.; Hartman, I. Progress Report: Experiments on Strength of Small Pillars of Coal in the Pittsburgh Bed; Report R.I. 3575; United States Department of the Interior, U.S. Bureau of Mines: Washington, DC, USA, 1941. [Google Scholar]
- Nose, M. Rock Tests in Situ. In Proceedings of the Transactions, 8th International Congress on Large Dams; ICOLD: Edinburgh, UK, 1964; pp. 219–252. [Google Scholar]
- Jahns, H. Measuring the strength of rock in situ at an increasing scale. In Proceedings of the 1st ISRM Congress, Lisbon, Portugal, 25 September–1 October 1966; Volume 1, pp. 477–482. [Google Scholar]
- Gimm, W.A.R.; Richter, E.; Rosetz, G.P.A. A study of the deformation and strength properties of rocks by block tests in situ in iron-ore mines. In Proceedings of the 1st ISRM Congress, Lisbon, Portugal, 25 September–1 October 1966; Volume 1, pp. 457–463. [Google Scholar]
- De Reeper, F. Design and execution of field pressure tests up to pressures of 200 kg/cm2. In Proceedings of the 1st ISRM Congress, Lisbon, Portugal, 25 September–1 October 1966; Volume 1, pp. 613–619. [Google Scholar]
- Bieniawski, Z.T. The effect of specimen size on compressive strength of coal. Int. J. Rock Mech. Min. Sci. Geomech. Abstr. 1968, 5, 325–335. [Google Scholar] [CrossRef]
- Georgi, F.; Hofer, K.H.; Knoll, P.; Menzel, W.; Thomas, K. Investigation about the fracture and deformation behavior of rock masses. In Proceedings of the 2nd ISRM Congress, Belgrade, Yugoslavia, 21 September 1970; Volume 2, pp. 295–305. [Google Scholar]
- Chaoui, A.; Mariotti, M.; Orliac, M. In situ calcareous marls strain and shear strength: Comparison between different test characteristics. In Proceedings of the 2nd ISRM Congress, Belgrade, Yugoslavia, 21 September 1970; Volume 2, pp. 3–50. [Google Scholar]
- Cook, N.G.W.; Hodgson, K.; Hojem, J.P.M.A. 100-MN jacking system for testing coal pillars underground. J. S. Afr. Inst. Min. Metall. 1971, 71, 215–224. [Google Scholar]
- Lama, R.D. In situ and Laboratory strength of coal. In Proceedings of the 12th US Symposium on Rock Mechanics (USRMS), Rolla, MO, USA, 16–18 November 1970; University of Missouri: Columbia, MO, USA; AIME: New York, NY, USA, 1971; pp. 265–300. [Google Scholar]
- Pratt, H.R.; Black, A.D.; Brown, W.S.; Brace, W.F. The effect of specimen size on the mechanical properties of unjointed diorite. Int. J. Rock Mech. Min. Sci. Geomech. Abstr. 1972, 9, 513–529. [Google Scholar] [CrossRef]
- Wagner, H. Determination of the complete load deformation characteristics of coal pillars. In Proceedings of the Third International Congress on Rock Mechanics, Denver, CO, USA, 25 June–6 July 1974; pp. 1076–1081. [Google Scholar]
- Bieniawski, Z.T.; Van Heerden, W.L. The significance of in situ tests on large rock specimens. Int. J. Rock Mech. Min. Sci. Geomech. Abstr. 1975, 12, 101–103. [Google Scholar] [CrossRef]
- Heuze, F.E. Scale effects in the determination of rock mass strength and deformability. Rock Mech. Rock Eng. 1980, 12, 167–192. [Google Scholar] [CrossRef]
- Ladanyi, B.; Archambault, G. Direct and indirect determination of shear strength of a jointed rock mass. In Proceedings of the AIME Annual Meeting, Las Vegas, NV, USA, 25–28 February 1980; Report number: 80–25. pp. 24–28. [Google Scholar]
- Brown, E.T. Strength of models of rock with intermittent joints. J. Soil Mech. Found. Div. ASCE 1970, 96, 1935–1949. [Google Scholar] [CrossRef]
- Einstein, H.H.; Hirschfeld, R.C. Model studies on mechanics of jointed rock. J. Soil Mech. Found. Div. ASCE 1973, 99, 229–248. [Google Scholar] [CrossRef]
- Chappel, B.A. Load distribution and deformational response in discontinua. Geotechnique 1974, 24, 641–654. [Google Scholar] [CrossRef]
- Yang, Z.Y.; Chen, J.M.; Huang, T.H. Effect of joint sets on the strength and deformation of rock mass models. Int. J. Rock Mech. Min. Sci. 1998, 35, 75–84. [Google Scholar] [CrossRef]
- Kulatilake, P.H.S.W.; Liang, J.; Gao, H. Experimental and numerical simulations of jointed rock block strength under uniaxial loading. J. Eng. Mech. ASCE 2001, 127, 1240–1247. [Google Scholar] [CrossRef]
- Kulatilake, P.H.S.W.; Malama, B.; Wang, J. Physical and particle flow modeling of jointed rock block behavior. Int. J. Rock Mech. 2001, 38, 641–657. [Google Scholar] [CrossRef]
- Kulatilake, P.H.S.W.; Park, J.; Malama, B. A new rock mass strength criterion for biaxial loading conditions. Int. J. Geotech. Geol. Eng. 2006, 24, 871–888. [Google Scholar] [CrossRef]
- Mehranpour, M.H.; Kulatilake, P.H.S.W.; Xingen, M.; He, M. Development of new three-dimensional rock mass strength criteria. Rock Mech. Rock Eng. 2018, 51, 3537–3561. [Google Scholar] [CrossRef] [Green Version]
- Mughieda, O.; Omar, M.T. Stress analysis for rock mass failure with offset joints. Geotech. Geol. Eng. 2008, 26, 543–552. [Google Scholar] [CrossRef]
- Prudencio, M.; Van Sint Jan, M. Strength and failure modes of rock mass models with non-persistent joints. Int. J. Rock Mech. Min. Sci. 2007, 44, 890–902. [Google Scholar] [CrossRef]
- Chen, X.; Liao, Z.H.; Li, D.J. Experimental study on the effect of joint orientation and persistence on the strength and deformation properties of rock masses under uniaxial compression. Chin. J. Rock Mech. Eng. 2011, 30, 781–789. [Google Scholar]
- Chen, X.; Liao, Z.H.; Peng, X. Deformability characteristics of jointed rock masses under uniaxial compression. Int. J. Min. Sci. Technol. 2012, 22, 213–221. [Google Scholar] [CrossRef]
- He, P.; Kulatilake, P.H.S.W.; Liu, D.; He, M. Development of a new 3-D coal mass strength criterion. Int. J. Geomech. 2017, 17, 04016067. [Google Scholar] [CrossRef]
- Edelbro, C.; Sjöberg, J.; Nordlund, E. A quantitative comparison of strength criteria for hard rock masses. Tunn. Undergr. Space Technol. 2006, 22, 57–68. [Google Scholar] [CrossRef]
- Hoek, E.; Brown, E.T. Empirical strength criterion for rock masses. J. Geotech. Eng. Div. ASCE 1980, 106, 1013–1035. [Google Scholar] [CrossRef]
- Griffith, A.A. The phenomena of rupture and flow in solids. Philos. Trans. R. Soc. Lond. Ser. A Math. Phys. Sci. 1920, 221, 163–198. [Google Scholar]
- Griffith, A.A. The theory of rupture. In Proceedings of the First International Congress for Applied Mechanics, Delft, The Netherlands, 22–26 April 1924; Biezeno, C.-B., Burgers, J.-M., Eds.; Delft, J. Waltman, jr.: Delft, The Netherlands, 1924; pp. 55–63. [Google Scholar]
- Hoek, E. Brittle failure of rock. In Rock Mechanics in Engineering Practice; Stagg, K.G., Zienkiewicz, O.C., Eds.; Wiley: New York, NY, USA, 1968; pp. 99–124. [Google Scholar]
- Bieniawski, Z.T. Engineering classification in rock engineering. In Proceedings of the Symposium on Exploration for Rock Engineering, Johannesburgh, South Africa, 1–5 November 1976; pp. 97–106. [Google Scholar]
- Hoek, E.; Wood, D.; Shah, S. A modified Hoek–Brown criterion for jointed rock masses. In Eurock 1992: Rock Characterization: ISRM Symposium, EUROCK; Hudson, J.-A., Ed.; Thomas Telford: Chester, UK; London, UK, 1992; pp. 209–213. [Google Scholar]
- Hoek, E.; Brown, E.T. Practical estimates of rock mass strength. Int. J. Rock Mech. Min. Sci. Geomech. Abstr. 1997, 34, 1165–1186. [Google Scholar] [CrossRef]
- Hoek, E.; Carranza-Torres, C.; Corkum, B. Hoek-Brown failure criterion-2002 Edition. In Proceedings of the NARMS-Tac, Toronto, ON, Canada, 7–10 July 2002; Volume 1, pp. 267–273. [Google Scholar]
- Hoek, E.; Brown, E.T. The Hoek-Brown failure criterion and GSI-2018 Edition. J. Rock Mech. Geotech. Eng. 2019, 11, 445–463. [Google Scholar] [CrossRef]
- Cai, M.; Kaiser, P.K.; Uno, H.; Tasaka, Y.; Minami, M. Estimation of rock mass deformation modulus and strength of jointed hard rock masses using the GSI system. Int. J. Rock Mech. Min. Sci. 2004, 41, 3–19. [Google Scholar] [CrossRef]
- Barton, N.R.; Lien, R.; Lunde, J. Engineering classification of rock masses for the design of tunnel support. Rock Mech. 1974, 6, 189–239. [Google Scholar] [CrossRef]
- Pan, X.D.; Hudson, J.A. A simplified three-dimensional Hoek–Brown yield criterion. In Rock Mechanics and Power Plants; Romana, M., Ed.; A.A. Balkema: Rotterdam, The Netherlands, 1988; pp. 95–103. [Google Scholar]
- Priest, S.D. Determination of shear strength and three-dimensional yield strength for the Hoek–Brown criterion. Rock Mech. Rock Eng. 2005, 38, 299–327. [Google Scholar] [CrossRef]
- Melkoumian, N.; Priest, S.D.; Hunt, S.P. Further development of the three-dimensional Hoek–Brown yield criterion. Rock Mech. Rock Eng. 2009, 42, 835–847. [Google Scholar] [CrossRef]
- Zhang, L.; Zhu, H. Three-dimensional Hoek–Brown strength criterion for rocks. J Geotech. Geoenviron. Engi. ASCE 2008, 133, 1128–1135. [Google Scholar] [CrossRef]
- Zhang, Q.; Zhu, H.; Zhang, L. Modification of a generalized three-dimensional Hoek–Brown strength criterion. Int. J. Rock Mech. Min. Sci. 2013, 59, 80–96. [Google Scholar] [CrossRef]
- Yudhbir, L.W.; Prinzl, F. An Empirical Failure Criterion for Rock Masses. In Proceedings of the 5th International Congress on Rock Mechanics, Melbourne, Australia, 10 April 1983; Balkema, Rotterdam: Melbourne, Australia, 1983; Volume 1, pp. B1–B8. [Google Scholar]
- Sheorey, P.R.; Biswas, A.K.; Choubey, V.D. An empirical failure criterion for rocks and jointed rock masses. Eng. Geol. 1989, 26, 141–159. [Google Scholar] [CrossRef]
- Ramamurthy, T. Shear strength response of some geological materials in triaxial compression. Int. J. Rock Mech. Min. 2001, 38, 683–697. [Google Scholar] [CrossRef]
- Kulatilake, P.H.S.W. Physical, empirical, and numerical modeling of jointed rock mass strength. In Rock Mechanics and Rock Engineering Multi-Volume Book; CRC Press, Balkema: Boca Raton, FL, USA, 2017; Chapter 12; Volume 2, p. 28. [Google Scholar] [CrossRef]
- Wiebols, G.; Cook, N. An energy criterion for the strength of rock in polyaxial compression. Int. J. Rock Mech. Min. Sci. Geomech. Abstr. 1968, 5, 529–549. [Google Scholar] [CrossRef]
- Drucker, D.; Prager, W. Soil mechanics and plastic analysis or limit design. Q. Appl. Math. 1952, 10, 157–165. [Google Scholar] [CrossRef] [Green Version]
- Mogi, K. Fracture and flow of rocks under high triaxial compression. J. Geophys. Res. 1971, 76, 1255–1269. [Google Scholar] [CrossRef]
- Jennings, E.B. A Mathematical Theory for the Calculation of the Stability of Slopes in Open Cast Mines. In Proceedings of the Planning Open Pit Mines; The South African Institute of Mining and Metallurgy: Johannesburg, South Africa, 1970; pp. 87–102. [Google Scholar]
- Amadei, B. Strength of a regularly jointed rock mass under biaxial and axisymmetric loading conditions. Int. J. Rock Mech. Min. Sci. Geomech. Abstr. 1988, 25, 3–13. [Google Scholar] [CrossRef]
- Bekaert, A.; Maghous, S. Three-dimensional yield strength properties of jointed rock mass as a homogenized medium. Mech Cohes-Frict Mat 1996, 1, 1–24. [Google Scholar] [CrossRef]
- Pouya, A.; Ghoreychi, M. Determination of rock mass strength properties by homogenization. Int. J. Numer. Anal Methods 2001, 25, 1285–1303. [Google Scholar] [CrossRef] [Green Version]
- Itasca. 3DEC User’s Guide; Itasca Consulting Group Inc.: Minneapolis, NU, USA, 2008. [Google Scholar]
- Itasca. PFC3D User’s Manual, Version 4.0; Itasca Consulting Group Inc.: Minneapolis, NU, USA, 2003. [Google Scholar]
- Ivars, D.M.; Pierce, M.E.; Darcel, C.; Montes, J.R.; Potyondy, D.O.; Young, R.P.; Cundall, P.A. The synthetic rock mass approach for jointed rock mass modelling. Int. J. Rock Mech. Min. Sci. 2011, 48, 219–244. [Google Scholar] [CrossRef]
- Goodman, R.E.; Taylor, R.L.; Brekke, T.L. A model for the mechanics of jointed rock. J. Soil Mech. Found. Div. 1968, 94, 637–659. [Google Scholar] [CrossRef]
- Chalhoub, M.; Pouya, A. Numerical homogenization of a fractured rock mass: A geometrical approach to determine the mechanical representative elementary volume. Electron J Geotech. Eng. 2008, 13, 1–12. [Google Scholar]
- Yang, J.P.; Chen, W.Z.; Yang, D.S.; Yang, J.Q. Numerical determination of strength and deformability of fractured rock mass by FEM modeling. Comput. Geotech. 2015, 64, 20–31. [Google Scholar]
- Cundall, P.A. A computer model for simulating progressive large-scale movements in blocky rock system. In Proceedings of the International Symposium on Rock Mechanic, Nancy, France, 4–6 October 1971; Volume 2. [Google Scholar]
- Lemos, J.V.; Hart, R.D.; Cundall, P.A. A generalized distinct element program for modelling jointed rock mass. In Proceedings of the Symposium on Foundamentals of Rock Joints, Bjorkliden, Sweden, 15–20 September 1985; pp. 335–343. [Google Scholar]
- Cundall, P.A. Formulation of a three-dimensional distinct element model-Part 1. A scheme to detect and represent contacts in a system composed of many polyhedral blocks. Int. J. Rock. Mech. Min. Sci. Geomech. Abstr. 1988, 25, 107–116. [Google Scholar] [CrossRef]
- Hart, R.; Cundall, P.A.; Lemos, J. Foundation of a three-dimensional distinct element model-Part II. Mechanical calculations for motion and interaction of a system composed of many polyhedral blocks. Int. J. Rock. Mech. Min. Sci. Geomech. Abstr 1988, 25, 117–126. [Google Scholar] [CrossRef]
- Kulatilake, P.H.S.W.; Ucpirti, H.; Wang, S.; Radberg, G.; Stephansson, O. Use of the Distinct Element Method to perform stress analysis in rock with non-persistent joints and to study the effect of joint geometry parameters on the strength and deformability of rock masses. Rock Mech. Rock Eng. 1992, 25, 253–274. [Google Scholar] [CrossRef]
- Wang, S.; Kulatilake, P.H.S.W. Linking between joint geometry models and a distinct element method in three dimensions to perform stress analyses in rock masses containing finite size joints. Jpn. Soc. Soil Mech. Found. Eng. 1993, 33, 88–98. [Google Scholar] [CrossRef] [Green Version]
- Kulatilake, P.H.S.W.; Ucpirti, H.; Stephansson, O. Effect of Finite Size Joints on the Deformability of Jointed Rock at the Two-Dimensional Level. Can. Geotech. J. 1994, 31, 364–374. [Google Scholar] [CrossRef]
- Kulatilake, P.H.S.W.; Park, J.; Um, J. Estimation of rock mass strength and deformability in 3-D for a 30m cube at a depth of 485m at Äspö Hard Rock Laboratory, Sweden. Int. J. Geotech. Geol. Eng. 2004, 22, 313–330. [Google Scholar] [CrossRef]
- Potyondy, D.O. Simulating stress corrosion with a bonded-particle model for rock. Int. J. Rock Mech. Min. Sciences 2007, 44, 677–691. [Google Scholar] [CrossRef]
- Koyama, T.; Jing, L. Effects of model scale and particle size on micro-mechanical properties and failure processes of rocks—A particle mechanics approach. Eng. Anal. Bound. Elem. 2007, 31, 458–472. [Google Scholar] [CrossRef]
- Lee, H.; Jeon, S. An experimental and numerical study of fracture coalescence in pre-cracked specimens under uniaxial compression. Int. J. Solids Struct. 2011, 48, 979–999. [Google Scholar] [CrossRef] [Green Version]
- Zhang, X.P.; Wong, L.N.Y. Cracking processes in rock-like material containing a single flaw under uniaxial compression: A numerical study based on parallel bonded-particle model approach. Rock Mech. Rock Eng. 2012, 45, 711–737. [Google Scholar] [CrossRef]
- Bahaaddini, M.; Sharrock, G.; Hebblewhite, B.K. Numerical investigation of the effect of joint geometrical parameters on the mechanical properties of a non-persistent jointed rock mass under uniaxial compression. Comput. Geotech. 2013, 49, 206–225. [Google Scholar] [CrossRef]
- Gao, F.Q.; Stead, D.; Kang, H.P. Numerical investigation of the scale effect and anisotropy in the strength and deformability of coal. Int. J. Coal Geol. 2014, 136, 25–37. [Google Scholar] [CrossRef]
- Zhang, Y.; Stead, D.; Elmo, D. Characterization of strength and damage of hard rock pillars using a synthetic rock mass method. Comput. Geotech. 2015, 65, 56–72. [Google Scholar] [CrossRef]
- Fan, X.; Kulatilake, P.H.S.W.; Chen, X. Mechanical behavior of rock-like jointed blocks with multi-non-persistent joints under uniaxial loading: A particle mechanics approach. Eng. Geol. 2015, 190, 17–32. [Google Scholar] [CrossRef]
- Kulatilake, P.H.S.W.; Chen, J.; Teng, J.; Shufang, X.; Pan, G. Discontinuity Geometry Characterization for the Rock Mass Around a Tunnel Close to the Permanent Shiplock Area of the Three Gorges Dam Site in China. Int. J. Rock Mech. Min. Sci. 1996, 33, 255–277. [Google Scholar] [CrossRef]
- Kulatilake, P.H.S.W.; Um, J.; Wang, M.; Escandon, R.F.; Narvaiz, J. Stochastic fracture Geometry modeling in 3-D including validations for a part of Arrowhead East Tunnel site, California, USA. Eng. Geol. 2003, 70, 131–155. [Google Scholar] [CrossRef]
- Kulatilake, P.H.S.W.; Fuenkajorn, K. Factor of Safety of Tetrahedral Wedges: A Probabilistic Study. Int. J. Surf. Min. 1987, 1, 147–154. [Google Scholar] [CrossRef]
- Kulatilake, P.H.S.W. Probabilistic Characterization of Shear Strength Parameters Using Triaxial Test Data. In ASTM STP on Advanced Triaxial Testing of Soil and Rock; ASTM International: West Conshohocken, PA, USA, 1988; pp. 553–566. [Google Scholar]
- Kulatilake, P.H.S.W. Minimum Rock Bolt Force and Minimum Static Acceleration in Tetrahedral Wedge Stability: A Probabilistic Study. Int. J. Surf. Min. 1988, 2, 19–26. [Google Scholar] [CrossRef]


Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kulatilake, P.H.S.W. 3-D Rock Mass Strength Criteria—A Review of the Current Status. Geotechnics 2021, 1, 128-146. https://doi.org/10.3390/geotechnics1010007
Kulatilake PHSW. 3-D Rock Mass Strength Criteria—A Review of the Current Status. Geotechnics. 2021; 1(1):128-146. https://doi.org/10.3390/geotechnics1010007
Chicago/Turabian StyleKulatilake, Pinnaduwa H. S. W. 2021. "3-D Rock Mass Strength Criteria—A Review of the Current Status" Geotechnics 1, no. 1: 128-146. https://doi.org/10.3390/geotechnics1010007
APA StyleKulatilake, P. H. S. W. (2021). 3-D Rock Mass Strength Criteria—A Review of the Current Status. Geotechnics, 1(1), 128-146. https://doi.org/10.3390/geotechnics1010007




