Next Article in Journal
Can We Identify Subgroups of Patients with Chronic Low Back Pain Based on Motor Variability? A Systematic Scoping Review
Previous Article in Journal
Association of Foot Sole Sensibility with Quiet and Dynamic Body Balance in Morbidly Obese Women
 
 
Search for Articles:
Title / Keyword
Author / Affiliation / Email
Journal
Article Type
 
 
Advanced Search
 
Section
Special Issue
Volume
Issue
Number
Page
 
Journals
Biomechanics
Volume 1
Issue 3
10.3390/biomechanics1030029
biomechanics-logo
Submit to this Journal Review for this Journal Propose a Special Issue
► Article Menu

Article Menu

share Share announcement Help format_quote Cite question_answer Discuss in SciProfiles thumb_up
...
Endorse textsms
...
Comment
first_page
settings
Order Article Reprints
Font Type:
Arial Georgia Verdana
Font Size:
Aa Aa Aa
Line Spacing:
Column Width:
Background:
Article

Prolonged Load Carriage Impacts Magnitude and Velocity of Knee Adduction Biomechanics

by
Gaervyn J. Salverda
,
Micah D. Drew
,
Samantha M. Krammer
and
Tyler N. Brown
*
Department of Kinesiology, Boise State University, Boise, ID 83702, USA
*
Author to whom correspondence should be addressed.
Biomechanics 2021, 1(3), 346-357; https://doi.org/10.3390/biomechanics1030029
Submission received: 1 October 2021 / Revised: 28 November 2021 / Accepted: 29 November 2021 / Published: 17 December 2021
(This article belongs to the Section Injury Biomechanics and Rehabilitation)
Browse Figures
Versions Notes

Abstract

:
Background: This study determined whether prolonged load carriage increased the magnitude and velocity of knee adduction biomechanics and whether increases were related to knee varus thrust or alignment. Methods: Seventeen participants (eight varus thrust and nine control) had knee adduction quantified during 60-min of walking (1.3 m/s) with three body-borne loads (0 kg, 15 kg, and 30 kg). Magnitude, average and maximum velocity, and time to peak of knee adduction biomechanics were submitted to a mixed model ANOVA. Results: With the 0 and 15 kg loads, varus thrust participants exhibited greater magnitude (p ≤ 0.037, 1.9–2.3°), and average (p ≤ 0.027, up to 60%) and maximum velocity (p ≤ 0.030, up to 44%) of varus thrust than control, but differences were not observed with the 30 kg load. The 15 and 30 kg loads led to significant increases in magnitude (p ≤ 0.017, 15–25%) and maximum velocity (p ≤ 0.017, 11–20%) of knee adduction moment, while participants increased magnitude (p ≤ 0.043, up to 0.3°) and maximum velocity (p ≤ 0.022, up to 5.9°/s and 6.7°/s) for knee adduction angle and varus thrust at minutes 30 and 60. Static alignment did not differ between groups (p = 0.412). Conclusion: During prolonged load carriage, all participants increased the magnitude and velocity of knee adduction biomechanics and the potential risk of knee OA.

1. Introduction

Osteoarthritis (OA) is a significant occupational burden for the military in general and service members specifically [1]. Every year over 10,000 service members are diagnosed with lower limb OA, costing upwards of $60 billion dollars to treat [2]. The knee joint is the most common location for OA in military populations, and reportedly 100% of service members who suffer occupational knee injury go on to develop OA at the joint [3]. Service members, in fact, are twice as likely to develop knee OA than the general population and the rate among service members steadily rose 45% between 2005 and 2014 [4]. Knee OA development typically causes loss of joint function and an increase of pain, leading to long term disability and medical discharge for service members [1]. Therefore, it is imperative researchers understand knee biomechanics that contributes to service members’ elevated rate of OA at the joint.
Service member knee OA development may be attributed to altered lower limb biomechanics when walking with heavy body borne loads [5], which routinely exceed 15 kg during training activities [6]. Locomoting with a body borne load leads to significant increases in peak vertical ground reaction forces (GRF) [7] and requires greater force production from lower limb musculature to prevent limb collapse [8]. Yet, the larger GRFs and muscle force coincide with a significant increase in limb stiffness [9]. The stiffer limb may transmit greater impact forces to the soft-tissue structures of the lower limb in general and the knee joint specifically [10], increasing the likelihood of soft-tissue injury [11]. In response to the heavy body borne loads service members reportedly adopt hazardous knee biomechanics [12,13], potentially further elevating the risk of soft-tissue damage and OA development [14].
Knee OA is characterized by the degeneration of the joint’s articular cartilage and may occur when abnormal joint forces damage the knee’s soft tissues [15]. Of particular importance, are knee adduction biomechanics. Specifically, the magnitude of knee adduction angle and moment, and varus thrust (rapid lateral knee motion, i.e., adduction, immediately following heel strike [16]) have been directly implicated in the pathogenesis of knee OA [17,18], and are reported to increase when walking with heavy body borne loads [12,13]. Individuals with visually confirmed varus thrust (>2.5 degrees [16]), for instance, are four times more likely to develop knee OA during unloaded walking [18]. While individuals with knee OA exhibit greater amounts of knee adduction moment than individuals without OA, and each 1% increase in knee adduction moment is purported to lead to a 6.5 times faster progression of disease in the knee [19]. In addition to magnitude, the velocity of knee adduction biomechanics, as it encompasses both direction and speed of motion [16], may provide greater insight on the transmission of forces to the medial knee joint compartment and the risk of OA development. Knee adduction velocity is reportedly larger for both knee OA individuals during unloaded walking [16] and young, otherwise healthy individuals that suffer military training-related knee overuse injury [20]. However, it is currently unknown whether walking with a heavy body borne load increases the velocity of the knee adduction biomechanics related to OA development.
Service members are often required to perform occupational-related locomotor tasks, such as walking or marching, for extended periods of time [5]. During prolonged bouts of walking (i.e., 60 min or longer) with a body borne load, individuals are reported to increase peak vertical GRF every 15 min [7]. This continual increase in GRF may require a concomitant rise in muscular effort to stabilize the knee joint [21] and leads to exercise induced muscular weakness, resulting in lower limb biomechanical alterations [7,22]. Specifically, during prolonged periods of walking with a body borne load, the magnitude of knee flexion and adduction joint angle and moment is reported to increase [13,23]. During a recent prolonged load carriage task, individuals exhibited a significant increase in the magnitude of knee adduction angle and moment after 30 min of walking and the addition of 15 kg and 30 kg body borne loads [13]; yet it is unknown whether walking for extended periods with heavy body borne load leads to increases in velocity of knee adduction biomechanics. Considering decreased knee extensor and flexor strength is purportedly associated with increased peak knee adduction velocity for individuals with knee OA [24], and exercise-induced muscular weakness may lead to similar increases in knee adduction velocity, particularly when walking with heavy body borne load.
Static knee malalignment has also been identified as a risk factor for knee OA development and may be a precursor to the adoption of hazardous knee adduction biomechanics, especially varus thrust [16]. Individuals that present greater varus alignment reportedly increased the risk of knee OA development two-fold [25]. Varus knee alignment is associated with larger peak knee adduction moments and greater magnitude of varus thrust during unloaded walking [26], but it is currently unknown whether static knee malalignment is associated with hazardous alterations in knee adduction biomechanics during prolonged load carriage. To build upon recent load carriage research [13], this study sought to determine whether body borne load and duration of walking impacted the magnitude and velocity of knee adduction biomechanics for individuals with and without varus thrust and whether static knee varus malalignment differed for individuals with and without varus thrust. We hypothesized that varus thrust participants would exhibit greater increases in magnitude and velocity of knee adduction biomechanics with the addition of body borne load and walk duration than the control participants, and static knee varus malalignment compared to individuals without varus thrust.

2. Materials and Methods

2.1. Participants

Seventeen (11 males/six females) recreationally active adults (between 18 and 40 years of age) were recruited to participate (Table 1). Potential participants were included if they self-reported the ability to safely walk with 75 pounds but were excluded if they reported: (1) history of surgery in the low back or lower extremities; (2) recent (within the last six months) pain and/or injury located in the back or lower extremity; (3) any known neurological disorder; and/or (4) currently pregnant. Prior to testing, research approval was obtained from the local Institutional Review Board, and all participants provided written informed consent.

2.2. Load Configurations

Each participant completed three test sessions, during which they performed a prolonged walk task with a different body borne load (0 kg, 15 kg, and 30 kg) relevant to military training (Figure 1) [6]. For all sessions, participants wore tight-fitting spandex shorts and a shirt. For testing of the 15 kg and 30 kg loads, participants also wore a weighted vest (V-MAX, WeightVest.com, Rexburg, ID, USA) adjusted to provide the necessary load (loads ±2% of the targeted weight were accepted). Before testing, a 3 × 3 Latin square was used to randomly assign the load test order to every participant. All test sessions were separated by at least 24 h to minimize injury risk from fatigue.

2.3. Prolonged Walk Task

The prolonged walk task required participants to walk continuously over-ground at a typical march speed (1.3 m/s) for 60 min [27]. During the 60-min, each participant completed 13 laps (i.e., one lap every 5 min) around a 390-m walk course (for further detail see Drew et al. [13]). Each lap required the participants to begin in the laboratory, and complete three walk trials through the motion capture volume. After completion of the walk trials, participants immediately proceeded outside of the laboratory, where they followed a marked route that traveled over asphalt and grass and returned the participant to the laboratory door. Throughout the walking task, participants were required to step to a metronome (Planet Waves PW-MT-01, D’Addario, Famingdale, NY, USA), set to their predetermined cadence, to ensure they continuously walked at 1.3 m/s both inside and outside the laboratory.

2.4. Biomechanical Collection and Analysis

For each walking trial, participants walked 1.3 m/s (±5%) through the motion capture volume, where they had three-dimensional (3D) lower limb biomechanics recorded. Specifically, eight high speed (240 Hz) optical cameras (MXF20, Vicon Motion Systems LTD, Oxford, UK) recorded lower limb motion data, while synchronous ground reaction force (GRF) data were collected with one in-ground force platform (2400 Hz, AMTI OR6 Series, Advanced Mechanical Technology Inc., Watertown, MA, USA). The speed of each walking trial was recorded with two sets of infrared timing gaits (TracTronix TF100, TracTonix Wireless Timing Systems, Lenexa, KS, USA), placed 4 m apart in the capture volume. A successful walk trial required participants to walk at the correct speed and only contact the force platform with their dominant limb, determined by asking the participant the foot they preferred to kick a ball with [28].
During each walking trial, lower limb biomechanical data was quantified from the 3D coordinates of 34 retroreflective markers and four virtual markers (Table 2). The reflective markers were attached to specific bony landmarks using double-sided tape and secured with elastic tape (Cover-Roll Stretch, BSN Medical, Charlotte, NC, USA) by a single experimenter (MDD). The virtual markers were digitized at specific bony landmarks in the global coordinate system using a Davis Digitizing Pointer (C-Motion, Inc., Germantown, MD, USA). After each marker was placed, participants stood in anatomical position for a static recording used to create a kinematic model in accordance with previous literature [13,29]. After fitting the kinematic model, the synchronous GRF and marker trajectory data were filtered using a fourth-order Butterworth filter (12Hz), and knee biomechanics were calculated in Visual3D (v6, C-Motion, Inc, Germantown, MD, USA). In Visual 3D, the filtered marker trajectories were processed to calculate knee joint rotations expressed with respect to each participants’ static pose using the joint coordinate system approach [30,31]. The filtered kinematic and GRF data were processed to obtain 3D knee forces and moments using standard inverse-dynamics analysis and segmental inertial properties according to Dempster [32]. To be consistent with existing load carriage literature, knee moments were expressed as external and normalized to the participant’s height (m) and weight (N) for analysis.
Custom MATLAB (MATLAB r2018a, Mathworks, Natick, MA, USA) code was used to calculate the average and maximum velocity of stance phase knee adduction biomechanics. The stance phase was identified as heel strike to toe-off, defined as the first instance the vertical GRF ascends and descends past 10 N, respectively. The average velocity of knee adduction angle and moment was calculated as the change in angle (or moment) from initial contact to peak value exhibited during the stance phase divided by the corresponding change in time from initial contact to the peak. Maximum knee adduction velocity was defined as the largest instantaneous velocity exhibited from initial contact to the peak of the stance angle (or moment). In addition, average and maximum velocity of varus thrust were also calculated, as the change in knee adduction angle exhibited during the first 16% of stance divided by the corresponding change in time, and largest instantaneous velocity of knee adduction angle during the first 16% of stance, respectively [16]. Each participant also had static knee alignment calculated from their static recording as the frontal plane knee projection angle using hip, knee, and ankle joint centers, according to Mizner et al. [33].

2.5. Statistical Analysis

For statistical analysis, participants who exhibited knee adduction equal to or greater than 2.5 degrees during the first 16% of stance at minute 0 when walking with the 0 kg load were assigned to the varus thrust group (VT; N = 8, range = 2.69 to 5.78 degrees) [16], whereas participants who exhibited less than 2.5 degrees of knee adduction were assigned to the control group (CON; N = 9, range 0.92 to 2.18 degrees) (Table 1).
Knee adduction biomechanics including, average and maximum velocity for knee adduction angle (KAA) and moment (KAM), and varus thrust, as well the magnitude of and time to peak for KAA, KAM, and varus thrust were submitted to statistical analysis. Each dependent variable was averaged across two walk trials recorded at minutes 0, 30, and 60 of the prolonged walk task. Then, each variable was submitted to a mixed model ANOVA to test the main effect and interaction between body borne load (0 kg, 15 kg, and 30 kg), time (minutes 0, 30, and 60) and group (VT and CON). Significant interactions were submitted to a simple effects analysis and a Bonferroni correction was used for significant pairwise comparisons. For all significant main effects and interactions, effect size was calculated using partial omega squared (ω2) and pairwise comparisons using Cohen’s d (d) [34,35]. An independent t-test was determined whether static alignment differed between groups (VT and CON). Alpha was set a priori p <.05. All statistical analysis was performed using SPSS software (v25 IMB, Armonk, NY, USA).

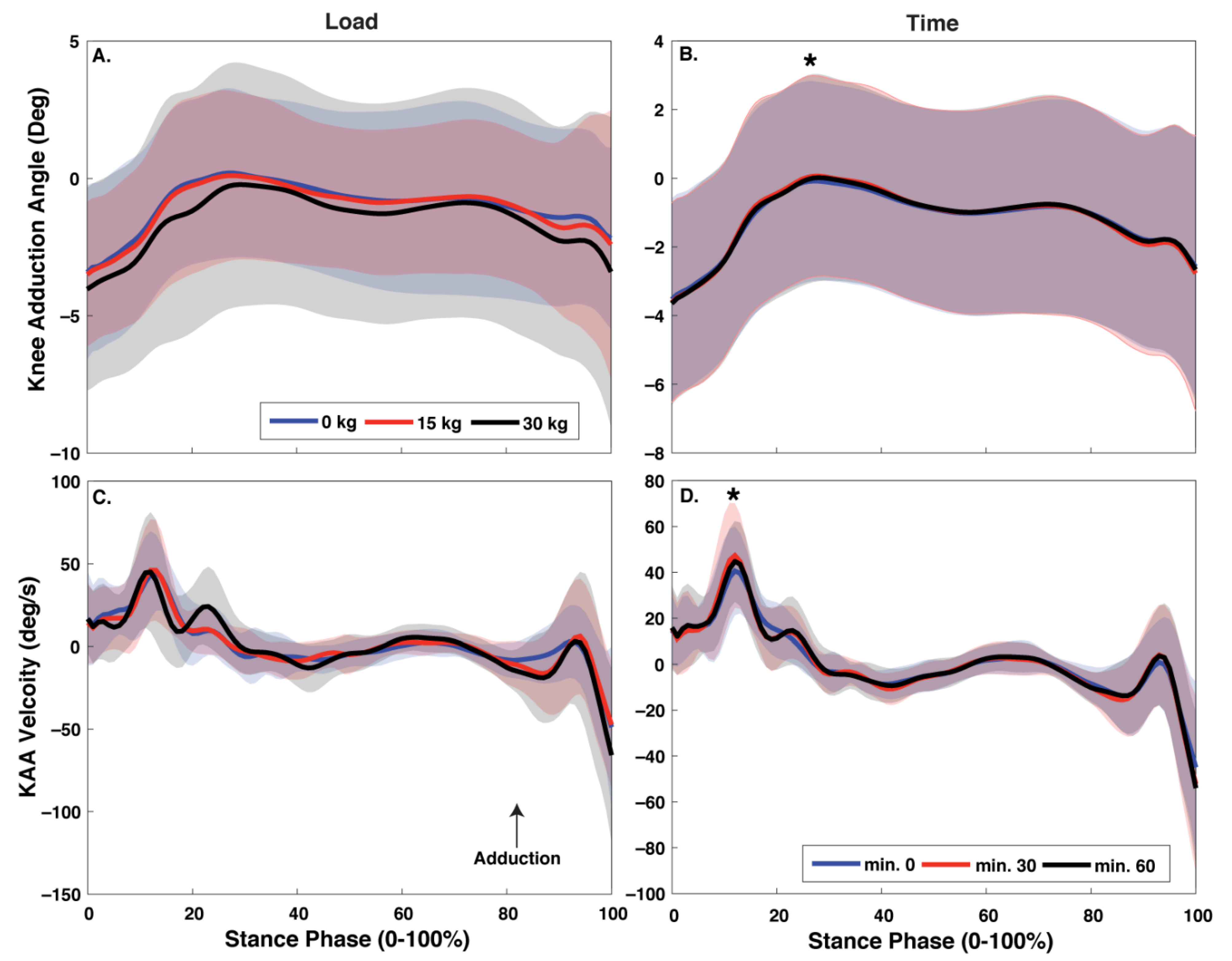
3. Results

A significant three-way interaction was observed for magnitude of varus thrust (p = 0.038, ω2 = 0.05) (Figure 2 and Table 3). With the 0 kg and 15 kg loads, the VT group exhibited a greater magnitude of varus thrust at minutes 0, 30, and 60 compared to CON participants (all: p ≤ 0.037, d = 1.17–3.05), but no group differences were observed at any time point with the 30 kg load (p > 0.05). The VT participants increased varus thrust magnitude at minute 60 compared to minute 0 with the 15 kg load (p = 0.013, d = 0.15), but their varus thrust magnitude did not differ between any time point with either the 0 kg or 30 kg load (p > 0.05); whereas CON exhibited no significant difference in varus thrust magnitude between any time point with any of the loads (p > 0.05). Additionally, neither VT nor CON exhibited a significant difference in varus thrust magnitude between any load at minutes 0, 30 or 60 (p > 0.05).
A significant three-way interaction was observed for average varus thrust velocity (p = 0.003, ω2 = 0.05) (Figure 2 and Table 3). With the 0 kg and 15 kg loads, the VT group exhibited greater average varus thrust velocity at minutes 0, 30, and 60 compared to CON (all: p ≤ 0.027, d = 1.26–3.41). However, no group differences in average velocity were observed with the 30 kg load (p > 0.05). The VT participants increased average varus thrust velocity at minute 60 compared to minute 0, with the 15 kg load (p = 0.031, d = 0.52), but average velocity did not differ between any time point with either the 0 kg or 30 kg load (p > 0.05). CON, however, exhibited no change in average varus thrust velocity between the time points with any of the loads (p > 0.05). In addition, VT decreased average varus thrust velocity with the 30 kg compared to 0 kg load at minute 60, but similar load differences were observed at the other time points (p > 0.05); while CON exhibited no difference in average velocity between any load at minutes 0, 30 or 60 (p > 0.05).
The ANOVA also revealed a three-way interaction for time to peak KAA (p = 0.018, ω2 = 0.06) (Figure 2 and Table 4). The VT participants increased time to peak KAA at minute 60 compared to minute 0 with the 30 kg load (p = 0.039, d = 1.63), but exhibited no differences with either 0 kg or 15 kg loads (p > 0.05); whereas CON exhibited no difference in time to peak KAA between time points with any load (p > 0.05). Additionally, no group differences in time to peak KAA were observed at any time point or load (p > 0.05), and neither VT, nor CON exhibited a significant difference in time to peak KAA between time points with the 0 kg, 15 kg or 30 kg loads (p > 0.05).
The ANOVA also revealed a significant load by group for maximum varus thrust velocity (p = 0.015, ω2 = 0.19) (Figure 2 and Table 3). Specifically, VT participants exhibited greater maximum varus thrust velocity than the CON participants with the 0 kg (p = 0.002, d = 1.87) and 15 kg (p = 0.030, d = 1.23), but not 30 kg load (p > 0.05).
Load impacted magnitude and maximum velocity for KAM (p = 0.002 and p = 0.003, ω2 = 0.29 and ω2 = 0.26) (Figure 3 and Table 5). Both magnitude and maximum KAM velocity were greater with the 15 kg (p = 0.001 and p = 0.017, d = 0.62 and d = 0.49) and 30 kg (p = 0.017 and p = 0.014, d = 0.85 and d = 0.91) compared to the 0 kg load, but no difference was observed between the 15 kg and 30 kg loads (p > 0.367). Load had no significant effect on time to peak or average KAM velocity, or any KAA and varus thrust measure (p > 0.05).
Time had a significant effect on magnitude of and maximum velocity for both KAA (p = 0.006 and p = 0.012, ω2 = 0.24 and ω2 = 0.20) and varus thrust (p = 0.009 and p = 0.012, ω2 = 0.21 and ω2 = 0.20), as well as average varus thrust velocity (p = 0.019, ω2 = 0.18) (Figure 2 and Table 3 and Table 4). KAA magnitude was greater at minute 30 (p = 0.003, d = 0.31) compared to 0, while varus thrust magnitude was greater at minutes 30 (p = 0.031, d = 0.32) and 60 (p = 0.043, d = 0.35) compared to minute 0. Maximum KAA and varus thrust velocity was greater at minute 60 compared to 0 (p = 0.009 and p = 0.022, d = 0.35 and d = 0.39). After correcting for Type I error, no significant difference in average varus thrust velocity was observed between any time point (p > 0.05). Time had no impact on any KAM measure (p > 0.05).
The VT participants had greater magnitude of varus thrust (p = 0.005, ω2 = 0.23), average and maximum velocity for both KAA (p = 0.004 and p = 0.043, ω2 = 0.24 and ω2 = 0.11) and varus thrust (p = 0.003 and p = 0.045, ω2 = 0.27 and ω2 = 0.10), and faster time to peak KAA (p = 0.001, ω2 = 0.02) than the CON participants (Table 2, Table 3 and Table 4). No group differences were observed for any of the KAM measure (p > 0.05).
Static alignment was not different between VT and CON (p = 0.412).

4. Discussion

This study sought to examine whether individuals that present varus thrust exhibit greater magnitude and velocity of knee adduction biomechanics during prolonged walking with a body borne load. Although the addition of load increased the magnitude and velocity of knee adduction moment and walk duration increased the magnitude and velocity of knee adduction motions, our hypotheses were only partially supported, as VT participants only exhibited greater knee adduction biomechanics than the CON participants with the lighter 0 kg and 15 kg loads.
The VT participants exhibited larger, faster knee adduction motion than the CON participants. Compared to CON, the VT participants exhibited 2.3° and 1.9° greater varus thrust with the 0 kg and 15 kg loads. Varus thrust is reportedly indicative of dynamic knee instability and may coincide with larger forces transmitted through the joint [36], requiring greater contribution from the knee’s passive soft-tissue structures for joint stabilization [37]. The larger varus thrust may lead to greater tissue damage at the knee joint, and in fact, individuals that present varus thrust during unloaded walking are four times more likely to develop knee OA [18]. With the light body borne loads, the current VT participants also adopted faster knee adduction motions than the CON participants. When walking with the 0 kg and 15 kg loads, VT participants exhibited up to 60% and 44% faster average and maximum varus thrust velocity than the CON participants, respectively. Considering knee adduction velocity encompasses both speed and direction of the movement and is presented by individuals with radiographically confirmed knee OA, as well as knee overuse injury [16,20], the larger and faster knee adduction adopted by VT participants may elevate their risk for knee OA development, as increasing movement velocity is reported to strain the joint’s soft-tissues more [38]. Although the velocity of loading produces greater tension in soft-tissues and may be an important etiologic factor for knee musculoskeletal injury [39,40], further research is needed to determine if faster knee adduction does, indeed, place more force on the knee’s passive soft-tissue structures and elevate knee OA risk when walking with a body borne load.
Contrary to our hypothesis, the VT participants did not exhibit larger, faster knee adduction motion than the CON group with the 30 kg body borne load. In agreement with previous experimental evidence, VT participants decreased magnitude and velocity of knee adduction 38% and 40% with the addition of the heavy, 30 kg load, whereas the CON participants exhibited a non-significant 44% and 40% increase magnitude and velocity of knee adduction with the 30 kg load [41]. The VT participants’ decrease in knee adduction, in particular velocity, may be attributed to the concurrent 70% increase in time to peak knee adduction they exhibited with 30 kg load. Increasing the time to peak knee adduction may afford the VT participants musculature more time to stabilize the joint and result in the smaller and slower knee adduction currently evident. While the reason only CON participants exhibited large, but non-significant increases in knee adduction motion with heavy body borne load is not immediately evident, future research may be warranted to focus on neuromuscular control, or strength and activation of the surrounding knee musculature, rather than lower limb alignment, as no significant between-group difference in static alignment was currently observed and strength of the knee musculature reportedly exhibits a significant relation to knee adduction velocity [24].
The addition of body borne load led to larger and faster KAM, but not adduction motions. In agreement with previous experimental evidence, the addition of the 15 kg and 30 kg loads led to significant 15% and 25% increases in KAM [42]. Considering KAM is reportedly a correlate for medial knee joint compartment loading, long periods of walking with a body borne load may result in the tissue damage that characterizes knee OA [19,43]—particularly considering the additional load may also coincide with faster loading of the knee’s soft tissues. The current participants also exhibited a significant 11% and 20% increase in maximum velocity of KAM when donning the 15 kg and 30 kg loads during the prolonged walk task. A significant increase in maximum KAM velocity or rate the external adduction moment was applied to the musculoskeletal system, which may require greater muscular effort to stabilize the knee and prevent excessive lateral motion of the joint [8]. Moreover, faster transmission force to the knee joint and associated passive soft-tissue structures may increase the risk for tissue damage, as faster movements are reported to increase both stress and strain of the knee’s soft-tissues [38], potentially increasing the risk of lower limb soft-tissue injury [22,44].
Longer walk duration led to larger, faster knee adduction motions. In particular, both maximum KAA and varus thrust velocity increased 5.9°/s and 6.7°/s after walking for 60 min. The physiological demands of long durations of walking [45], particularly with heavy body borne load, reportedly lead to exercise induced muscular weakness [46]. Exercise induced weakness of the knee’s musculature may prevent it from providing active joint stabilization and result in the significant increases in the magnitude and velocity of knee adduction motions currently evident towards the end of the walking task. The larger, faster knee adduction motions may increase the reliance on the joint’s passive soft-tissue structures to safely dissipate the impact forces of walking and elevate the risk for a knee injury and OA development. However, considering the current observed increases in the magnitude of both KAA and varus thrust are not likely to be clinically meaningful (0.3° after 30 min of walking), future research is warranted to determine the specific increases in knee adduction that result in greater loading of the knee’s passive soft-tissue structures.
Static knee alignment, particularly varus malalignment, is purportedly a knee OA risk factor and may increase the odds of developing the disease by two-fold [47]. Considering varus malalignment is reportedly related to larger peak KAM [26], and larger, faster varus thrust during unloaded walking [16], we hypothesized that individuals with static varus alignment would exhibit greater knee adduction biomechanics when walking with a load. Yet, contrary to our hypothesis, static knee varus alignment did not differ between groups. Although the current VT participants exhibited a small, insignificant 1.5° difference in static knee alignment compared to CON, the current sample may not be powered appropriately to detect small differences in knee alignment between groups. Future research that tests a larger sample is warranted, as it may be needed to detect differences in static knee alignment between groups and/or to determine whether static alignment impacts knee adduction biomechanics during prolonged load carriage.
This study may also be limited by the current static knee alignment calculation. Currently, static knee alignment was determined using frontal plane knee projection angle. Although using a radiograph may provide less variable knee alignment values than the chosen method, calculating static alignment with the frontal plane projection angle provides alignment values comparable to those quantified using a radiograph [48], and previously exhibited a significant relation with knee biomechanics during dynamic unloaded locomotor tasks [15]. As such, we are confident that the current method of determining static knee alignment was appropriate. Further study of static knee alignment’s role in knee adduction biomechanics during prolonged walking with a body borne load is warranted.

5. Conclusions

In conclusion, prolonged load carriage may elevate the risk of knee OA development because it led to increases in magnitude and velocity of knee adduction biomechanics. The VT group exhibited larger and faster knee adduction motions, and potentially greater OA risk, when walking with the lighter 0 kg and 15 kg loads. Yet, all participants, regardless of whether they exhibit varus thrust or not, may increase knee adduction biomechanics during prolonged walking with a body borne load, as the addition of load increased magnitude and velocity of KAM, and walk duration increased the magnitude and velocity of knee adduction motion.

Author Contributions

Conceptualization, T.N.B.; methodology, G.J.S., M.D.D., S.M.K. and T.N.B.; validation, G.J.S., M.D.D., S.M.K. and T.N.B.; formal analysis, G.J.S., M.D.D., S.M.K. and T.N.B.; investigation, G.J.S., M.D.D. and S.M.K.; resources, T.N.B.; data curation, M.D.D., S.M.K. and T.N.B.; writing—original draft preparation, G.J.S. and T.N.B.; writing—review and editing, G.J.S. and T.N.B.; visualization, G.J.S. and T.N.B.; supervision, T.N.B.; project administration, T.N.B.; funding acquisition, T.N.B. All authors have read and agreed to the published version of the manuscript.

Funding

Project funding was provided by MW CTR-IN/NIGMS (Award #2U54GM104944). The funding source had no involvement in any part of the study.

Institutional Review Board Statement

The study was conducted according to the guidelines of the Declaration of Helsinki and approved by the Institutional Review Board of Boise State University (protocol number: 186-MED18-003 and date of approval: 5/4/2018).

Informed Consent Statement

Informed consent was obtained from all subjects involved in the study.

Acknowledgments

We would like thank Alexis Flock and Kayla Seymore for their assistance with testing.

Conflicts of Interest

None of the authors demonstrate any conflict of interest regarding this submission.

References

  1. Cross, J.D.; Ficke, J.R.; Hsu, J.R.; Masini, B.D.; Wenke, J.C. Battlefield Orthopaedic Injuries Cause the Majority of Long-term Disabilities. Am. Acad. Orthop. Surg. 2011, 19, S1–S7. [Google Scholar] [CrossRef]
  2. Cameron, K.L.; Hsiao, M.S.; Owens, B.D.; Burks, R.; Svoboda, S.J. Incidence of physician-diagnosed osteoarthritis among active duty United States military service members. Arthritis Rheum. 2011, 63, 2974–2982. [Google Scholar] [CrossRef] [PubMed] [Green Version]
  3. Rivera, J.C.; Wenke, J.C.; Buckwalter, J.A.; Ficke, J.R.; Johnson, A.E. Posttraumatic Osteoarthritis Caused by Battlefield Injuries. J. Am. Acad. Orthop. Surg. 2012, 20, S64–S69. [Google Scholar] [CrossRef] [Green Version]
  4. Showery, J.E.; Kusnezov, N.A.; Dunn, J.C.; Bader, J.O.; Belmont, P.J.; Waterman, B.R. The Rising Incidence of Degenerative and Posttraumatic Osteoarthritis of the Knee in the United States Military. J. Arthroplast. 2016, 31, 2108–2114. [Google Scholar] [CrossRef]
  5. Andersen, K.A.; Grimshaw, P.N.; Kelso, R.M.; Bentley, D.J. Musculoskeletal Lower Limb Injury Risk in Army Populations. Sports Med.-Open 2016, 2, 22. [Google Scholar] [CrossRef] [Green Version]
  6. Orr, R.M.; Johnston, V.; Coyle, J.; Pope, R. Reported load carriage injuries of the Australian army soldier. J. Occup. Rehabil. 2015, 25, 316–322. [Google Scholar] [CrossRef] [PubMed] [Green Version]
  7. Lidstone, D.E.; Stewart, J.A.; Gurchiek, R.; Needle, A.R.; van Werkhoven, H.; McBride, J.M. Physiological and Biomechanical Responses to Prolonged Heavy Load Carriage During Level Treadmill Walking in Females. J. Appl. Biomech. 2017, 33, 248–255. [Google Scholar] [CrossRef]
  8. Seay, J.F.; Fellin, R.E.; Sauer, S.G.; Frykman, P.N.; Bensel, C.K. Lower extremity biomechanical changes associated with symmetrical torso loading during simulated marching. Mil. Med. 2014, 179, 85–91. [Google Scholar] [CrossRef] [PubMed] [Green Version]
  9. Silder, A.; Besier, T.; Delp, S.L. Running with a load increases leg stiffness. J. Biomech. 2015, 48, 1003–1008. [Google Scholar] [CrossRef]
  10. Majumdar, D.; Pal, M.S.; Majumdar, D. Effects of military load carriage on kinematics of gait. Ergonomics 2010, 53, 782–791. [Google Scholar] [CrossRef]
  11. Chu, M.L.; Yazdani-Ardakani, S.; Gradisar, I.A.; Askew, M.J. An in vitro simulation study of impulsive force transmission along the lower skeletal extremity. J. Biomech. 1986, 19, 979–987. [Google Scholar] [CrossRef]
  12. Brown, T.; O’Donovan, M.; Hasselquist, L.; Corner, B.; Schiffman, J. Body borne loads impact walk-to-run and running biomechanics. Gait Posture 2014, 40, 237–242. [Google Scholar] [CrossRef]
  13. Drew, M.D.; Krammer, S.M.; Brown, T.N. Effects of prolonged walking with body borne load on knee adduction biomechanics. Gait Posture 2021, 84, 192–197. [Google Scholar] [CrossRef]
  14. Knapik, J.J.; Reynolds, K.L.; Harman, E. Soldier load carriage: Historical, physiological, biomechanical, and medical aspects. Mil. Med. 2004, 169, 45–56. [Google Scholar] [CrossRef] [Green Version]
  15. Wilson, D.R.; McWalter, E.J.; Johnston, J.D. The Measurement of Joint Mechanics and their Role in Osteoarthritis Genesis and Progression. Rheum. Dis. Clin. N. Am. 2008, 34, 605–622. [Google Scholar] [CrossRef] [PubMed] [Green Version]
  16. Chang, A.H.; Chmiel, J.S.; Moisio, K.C.; Almagor, O.; Zhang, Y.; Cahue, S.; Sharma, L. Varus thrust and knee frontal plane dynamic motion in persons with knee osteoarthritis. Osteoarthr. Cartil. 2013, 21, 1668–1673. [Google Scholar] [CrossRef] [Green Version]
  17. Mahmoudian, A.; van Dieen, J.H.; Bruijn, S.M.; Baert, I.A.; Faber, G.S.; Luyten, F.P.; Verschueren, S.M. Varus thrust in women with early medial knee osteoarthritis and its relation with the external knee adduction moment. Clin. Biomech. 2016, 39, 109–114. [Google Scholar] [CrossRef] [Green Version]
  18. Chang, A.; Hayes, K.; Dunlop, D.; Hurwitz, D.; Song, J.; Cahue, S.; Genge, R.; Sharma, L. Thrust during ambulation and the progression of knee osteoarthritis. Arthritis Rheum. 2004, 50, 3897–3903. [Google Scholar] [CrossRef] [PubMed]
  19. Miyazaki, T.; Wada, M.; Kawahara, H.; Sato, M.; Baba, H.; Shimada, S. Dynamic load at baseline can predict radiographic disease progression in medial compartment knee osteoarthritis. Ann. Rheum. Dis. 2002, 61, 617–622. [Google Scholar] [CrossRef]
  20. Stickley, C.D.; Presuto, M.M.; Radzak, K.N.; Bourbeau, C.M.; Hetzler, R.K. Dynamic Varus and the Development of Iliotibial Band Syndrome. J. Athl. Train. 2018, 53, 128–134. [Google Scholar] [CrossRef] [PubMed] [Green Version]
  21. Wang, H.; Frame, J.; Ozimek, E.; Leib, D.; Dugan, E.L. Influence of Fatigue and Load Carriage on Mechanical Loading during Walking. Mil. Med. 2012, 177, 152–156. [Google Scholar] [CrossRef] [PubMed] [Green Version]
  22. Milner, C.E.; Ferber, R.; Pollard, C.D.; Hamill, J.; Davis, I.S. Biomechanical Factors Associated with Tibial Stress Fracture in Female Runners. Med. Sci. Sports Exerc. 2006, 38, 323–328. [Google Scholar] [CrossRef] [Green Version]
  23. Simpson, K.M.; Munro, B.J.; Steele, J.R. Effects of prolonged load carriage on ground reaction forces, lower limb kinematics and spatio-temporal parameters in female recreational hikers. Ergonomics 2012, 55, 316–326. [Google Scholar] [CrossRef]
  24. Espinosa, S.E.; Costello, K.E.; Souza, R.B.; Kumar, D. Lower knee extensor and flexor strength is associated with varus thrust in people with knee osteoarthritis. J. Biomech. 2020, 107, 109865. [Google Scholar] [CrossRef] [PubMed]
  25. Brouwer, G.M.; Van Tol, A.W.; Bergink, A.P.; Belo, J.N.; Bernsen, R.M.D.; Reijman, M.; Pols, H.A.P.; Bierma-Zeinstra, S.M.A. Association between valgus and varus alignment and the development and progression of radiographic osteoarthritis of the knee. Arthritis Rheum. 2007, 56, 1204–1211. [Google Scholar] [CrossRef]
  26. Kuroyanagi, Y.; Nagura, T.; Kiriyama, Y.; Matsumoto, H.; Otani, T.; Toyama, Y.; Suda, Y. A quantitative assessment of varus thrust in patients with medial knee osteoarthritis. Knee 2012, 19, 130–134. [Google Scholar] [CrossRef]
  27. Department of the Army. Field Manual No. 21-18: Foot Marches; Department of the Army: Washington, DC, USA, 1990. [Google Scholar]
  28. Van Melick, N.; Meddeler, B.M.; Hoogeboom, T.J.; Der Sanden, M.W.G.N.-V.; Van Cingel, R.E.H. How to determine leg dominance: The agreement between self-reported and observed performance in healthy adults. PLoS ONE 2017, 12, e0189876. [Google Scholar] [CrossRef] [Green Version]
  29. Lobb, N.J.; Fain, A.L.C.; Seymore, K.D.; Brown, T.N. Sex and stride length impact leg stiffness and ground reaction forces when running with body borne load. J. Biomech. 2019, 86, 96–101. [Google Scholar] [CrossRef] [PubMed]
  30. Grood, E.S.; Suntay, W.J. A joint coordinate system for the clinical description of three-dimensional motions: Application to the Knee. J. Biomech. Eng. 1983, 105, 136–144. [Google Scholar] [CrossRef]
  31. Wu, G.; Siegler, S.; Allard, P.; Kirtley, C.; Leardini, A.; Rosenbaum, D.; Whittle, M.; D’Lima, D.D.; Cristofolini, L.; Witte, H.; et al. ISB recommendation on definitions of joint coordinate system of various joints for the reporting of human joint motion—Part I: Ankle, hip, and spine. J. Biomech. 2002, 35, 543–548. [Google Scholar] [CrossRef]
  32. Dempster, W.T. Space Requirements of the Seated Operator, Geometrical, Kinematic, and Mechanical Aspects of the Body with Special Reference to the Limbs; Michigan State University East Lansing: East Lansing, MI, USA, 1955. [Google Scholar]
  33. Mizner, R.L.; Chmielewski, T.L.; Toepke, J.J.; Tofte, K.B. Comparison of 2-Dimensional Measurement Techniques for Predicting Knee Angle and Moment During a Drop Vertical Jump. Clin. J. Sport Med. 2012, 22, 221–227. [Google Scholar] [CrossRef] [PubMed]
  34. Cohen, J. Statistical Power for the Behavioral Sciences, 2nd ed.; Lawrence Erlbaum Associates Inc.: Hillsdale, NJ, USA, 1988. [Google Scholar]
  35. Keren, G.; Lewis, C. Partial Omega Squared for Anova Designs. Educ. Psychol. Meas. 1979, 39, 119–128. [Google Scholar] [CrossRef]
  36. Chang, A.; Hochberg, M.; Song, J.; Dunlop, D.; Chmiel, J.S.; Nevitt, M.; Hayes, K.; Eaton, C.; Bathon, J.; Jackson, R.; et al. Frequency of varus and valgus thrust and factors associated with thrust presence in persons with or at higher risk of developing knee osteoarthritis. Arthritis Rheum. 2010, 62, 1403–1411. [Google Scholar] [CrossRef] [PubMed] [Green Version]
  37. Schipplein, O.D.; Andriacchi, T.P. Interaction between active and passive knee stabilizers during level walking. J. Orthop. Res. 1991, 9, 113–119. [Google Scholar] [CrossRef]
  38. Earp, J.E.; Newton, R.U.; Cormie, P.; Blazevich, A.J. Faster movement speed results in greater tendon strain during the loaded squat exercise. Front. Physiol. 2016, 7, 366. [Google Scholar] [CrossRef] [PubMed] [Green Version]
  39. Noyes, F.R.; Delucas, J.L.; Torvik, P.J. Biomechanics of Anterior Cruciate Ligament Failure. J. Bone Jt. Surg. 1974, 56, 236–253. [Google Scholar] [CrossRef]
  40. Hamill, J.; Miller, R.; Noehren, B.; Davis, I. A prospective study of iliotibial band strain in runners. Clin. Biomech. 2008, 23, 1018–1025. [Google Scholar] [CrossRef] [PubMed]
  41. Brown, T.N.; Kaplan, J.T.; Cameron, S.E.; Seymore, K.D.; Ramsay, J.W. Individuals with varus thrust do not increase knee adduction when running with body borne load. J. Biomech. 2018, 69, 97–102. [Google Scholar] [CrossRef] [PubMed]
  42. Loverro, K.L.; Hasselquist, L.; Lewis, C.L. Females and males use different hip and knee mechanics in response to symmetric military-relevant loads. J. Biomech. 2019, 95, 109280. [Google Scholar] [CrossRef]
  43. Foroughi, N.; Smith, R.; Vanwanseele, B. The association of external knee adduction moment with biomechanical variables in osteoarthritis: A systematic review. Knee 2009, 16, 303–309. [Google Scholar] [CrossRef]
  44. Gabbett, T.J.; Ullah, S. Relationship Between Running Loads and Soft-Tissue Injury in Elite Team Sport Athletes. J. Strength Cond. Res. 2012, 26, 953–960. [Google Scholar] [CrossRef]
  45. Mullins, A.K.; Annett, L.E.; Drain, J.R.; Kemp, J.G.; Clark, R.A.; Whyte, D.G. Lower limb kinematics and physiological responses to prolonged load carriage in untrained individuals. Ergonomics. 2015, 58, 770–780. [Google Scholar] [CrossRef] [PubMed]
  46. Blacker, S.D.; Fallowfield, J.L.; Bilzon, J.L.J.; Willems, M.E.T. Neuromuscular function following prolonged load carriage on level and downhill gradients. Aviat. Space Environ. Med. 2010, 81, 745–753. [Google Scholar] [CrossRef]
  47. Sharma, L.; Song, J.; Dunlop, D.; Felson, D.; Lewis, C.E.; Segal, N.; Torner, J.; Cooke, T.D.V.; Hietpas, J.; Lynch, J.; et al. Varus and valgus alignment and incident and progressive knee osteoarthritis. Ann. Rheum. Dis. 2010, 69, 1940–1945. [Google Scholar] [CrossRef]
  48. Vanwanseele, B.; Parker, D.; Coolican, M. Frontal Knee Alignment: Three-dimensional Marker Positions and Clinical Assessment. Clin. Orthop. Relat. Res. 2009, 467, 504–509. [Google Scholar] [CrossRef] [Green Version]
Biomechanics 01 00029 g001 550
Figure 1. Depicts the weighted vest worn for the 15 kg and 30 kg load conditions, which was systematically adjusted to apply the necessary load for both conditions.
Figure 1. Depicts the weighted vest worn for the 15 kg and 30 kg load conditions, which was systematically adjusted to apply the necessary load for both conditions.
Biomechanics 01 00029 g001
Biomechanics 01 00029 g002 550
Figure 2. Mean ± SD stance phase (0–100%) for knee adduction angle (A,C) and velocity (B,D) with each body borne load (0 kg, 15 kg, and 30 kg) and time point (minute 0, 30, and 60). Time had a significant effect on magnitude and maximum velocity for KAA (p < 0.05 indicated with *).
Figure 2. Mean ± SD stance phase (0–100%) for knee adduction angle (A,C) and velocity (B,D) with each body borne load (0 kg, 15 kg, and 30 kg) and time point (minute 0, 30, and 60). Time had a significant effect on magnitude and maximum velocity for KAA (p < 0.05 indicated with *).
Biomechanics 01 00029 g002
Biomechanics 01 00029 g003 550
Figure 3. Mean ± SD stance phase (0–100%) for knee adduction moment (A,C) and velocity (B,D) with each body borne load (0 kg, 15 kg, and 30 kg) and time point (minute 0, 30, and 60). The load had a significant effect on the magnitude and maximum velocity for KAM (p < 0.05 indicated with *).
Figure 3. Mean ± SD stance phase (0–100%) for knee adduction moment (A,C) and velocity (B,D) with each body borne load (0 kg, 15 kg, and 30 kg) and time point (minute 0, 30, and 60). The load had a significant effect on the magnitude and maximum velocity for KAM (p < 0.05 indicated with *).
Biomechanics 01 00029 g003
Table
Table 1. Mean ± SD demographics for both varus thrust (VT) and control (CON) participants.
Table 1. Mean ± SD demographics for both varus thrust (VT) and control (CON) participants.
NAgeHeight (m)Weight (kg)
VT823 ± 1.91.79 ± 0.173.1 ± 14.5
CON923 ± 4.11.73 ± 0.169.3 ± 9.6
Table
Table 2. Placement of each retroreflective and virtual marker.
Table 2. Placement of each retroreflective and virtual marker.
Marker Placement
Trunkxiphoid process, clavicular notch, c7 vertebrae, bottom of the scapula, right and left acromion process
Pelvisanterior-superior iliac spines, posterior-superior iliac spines, iliac crests
Thighgreater trochanter, lateral epicondyles, medial epicondyles, distal thigh
Shanktibial tuberosity, lateral fibula, distal tibia, lateral malleoli, medial malleoli
Footfirst metatarsal head, fifth metatarsal head, heel, midpoint of first and fifth metatarsals
Note: Bold indicates calibration markers, italics indicate virtual markers, and the rest are tracking markers.
Table
Table 3. Mean ± SD for the varus thrust measures for both VT and CON participants at each time point (minutes 0, 30, and 60) with each body borne load (0 kg, 15 kg, and 30 kg).
Table 3. Mean ± SD for the varus thrust measures for both VT and CON participants at each time point (minutes 0, 30, and 60) with each body borne load (0 kg, 15 kg, and 30 kg).
Varus Thrust 0 Kg 15 Kg30 Kg
VT CONVT CONVT CON
Magnitude (deg) a,c,dMin. 03.63 ± 0.281.29 ± 0.293.20 ± 0.431.77 ± 0.462.62 ± 0.462.28 ± 0.49
Min. 303.93 ± 0.271.59 ± 0.293.61 ± 0.481.74 ± 0.512.93 ± 0.512.74 ± 0.55
Min. 603.83 v 0.311.64 ± 0.33 3.94 ± 0.481.69 ± 0.512.42 ± 0.452.93 ± 0.48
Average Velocity (deg/s) a,c,dMin. 025.76 ± 1.999.98 ± 2.1123.55 ± 2.9912.81 ± 3.1819.37 ± 3.0615.74 ± 3.25
Min. 3028.43 ± 1.7711.37 ± 1.8725.21 ± 3.2312.08 ± 3.4321.09 ± 3.4218.34 ± 3.62
Min. 6028.07 ± 2.2111.31 ± 2.3428.01 ± 3.1111.61 ± 3.3018.91 ± 3.1721.13 ± 3.37
Maximum Velocity (deg/s) b,c,dMin. 063.31 ± 4.0232.57 ± 4.2761.54 ± 7.1137.30 ± 7.5455.65 ± 7.8744.79 ± 8.34
Min. 3065.93 ± 5.6937.45 ± 6.0364.82 ± 8.3539.04 ± 8.8565.89 ± 10.4853.68 ± 11.11
Min. 6067.24 ± 7.2240.26 ±7.6669.06 ± 7.7840.99 ± 8.2553.06 ± 8.5164.42 ± 9.02
a Denotes a significant (p < 0.05) 3-way interaction. b Denotes a significant (p < 0.05) load by group interaction. c Denotes a significant (p < 0.05) main effect of time. d Denotes a significant (p < 0.05) group difference.
Table
Table 4. Mean ± SD for the knee adduction angle measures for both VT and CON participants at each time point (minutes 0, 30, and 60) with each body borne load (0 kg, 15 kg, and 30 kg).
Table 4. Mean ± SD for the knee adduction angle measures for both VT and CON participants at each time point (minutes 0, 30, and 60) with each body borne load (0 kg, 15 kg, and 30 kg).
Knee Adduction Angle 0 Kg 15 Kg30 Kg
VT CONVT CONVT CON
Magnitude (deg) bMin. 04.78 ± 0.362.36 ± 0.384.95 ± 0.413.34 ± 0.434.84 ± 0.593.72 ± 0.63
Min. 305.15 ± 0.332.59 ± 0.355.35 ± 0.483.07 ± 0.505.08 ± 0.624.26 ± 0.66
Min. 605.09 ± 0.342.90 ± 0.375.28 ± 0.443.17 ± 0.473.83 ± 0.494.42 ± 0.52
Average Velocity (deg/s) cMin. 026.39 ± 3.4310.17 ± 3.6422.94 ± 3.7910.81 ± 4.0218.69 ± 2.6910.83 ± 2.86
Min. 3028.51 ± 2.5012.35 ± 2.6622.93 ± 4.3510.96 ± 4.6120.79 ± 3.4513.431 ± 3.66
Min. 6029.29 ± 3.5913.52 ± 3.8124.67 ± 3.0810.47 ± 3.2715.25 ± 2.8317.61 ± 3.00
Maximum Velocity
(deg/s) b,c		Min. 064.14 ± 4.1035.66 ± 4.3564.79 ± 6.7840.67 ± 7.1859.03 ± 8.5849.18 ± 9.09
Min. 3066.46 ± 5.6438.23 ± 5.9968.86 ± 7.6243.04 ± 8.0970.84 ± 11.5054.47 ± 12.20
Min. 6068.15 ± 6.7841.67 ± 7.7570.03 ± 7.1145.06 ± 7.5459.52 ± 8.6264.42 ± 9.14
Time to peak (s) a,cMin. 00.24 ± 0.050.29 ± 0.050.30 ± 0.060.42 ± 0.070.33 ± 0.07 0.42 ± 0.02
Min. 300.21 ± 0.040.29 ± 0.040.34 ± 0.070.39 ± 0.070.34 ± 0.070.39 ± 0.07
Min. 600.20 ± 0.040.27 ± 0.040.30 ± 0.060.43 ± 0.070.43 ± 0.070.30 ± 0.07
a Denotes significant (p < 0.05) 3-way interaction. b Denotes a significant (p < 0.05) main effect of time. c Denotes a significant (p < 0.05) group difference.
Table
Table 5. Mean ± SD for the knee adduction moment measures for both VT and CON participants at each time point (minutes 0, 30, and 60) with each body borne load (0 kg, 15 kg, and 30 kg).
Table 5. Mean ± SD for the knee adduction moment measures for both VT and CON participants at each time point (minutes 0, 30, and 60) with each body borne load (0 kg, 15 kg, and 30 kg).
Knee Adduction Moment 0 kg15 kg30 kg
VTCONVTCONVTCON
Magnitude
(Nm/kgm) a		Min. 0−0.37 ± 0.03−0.37 ± 0.03−0.43 ± 0.03−0.43 ± 0.03−0.47 ± 0.06−0.48 ± 0.07
Min. 30−0.39 ± 0.03−0.37 ± 0.03−0.45 ± 0.03−0.42 ± 0.03−0.53 ± 0.05−0.47 ± 0.06
Min. 60−0.38 ± 0.03−0.37 ± 0.03−0.43 ± 0.04−0.43 ± 0.04−0.46 ± 0.07−0.47 ± 0.07
Average Velocity (Nm/kgm/s)Min. 0−2.03 ± 0.38−2.15 ± 0.40−2.45 ± 0.39−2.15 ± 0.41−2.52 ± 0.56−2.41 ± 0.59
Min. 30−2.08 ± 0.39−1.90 ± 0.42−2.44 ± 0.43−1.91 ± 0.46−3.20 ± 0.51−2.29 ± 0.54
Min. 60−2.13 ± 0.37−2.17 ± 0.39−2.15 ± 0.38−2.15 ± 0.41−2.44 ± 0.48−2.35 ± 0.51
Maximum Velocity
(Nm/kgm/s) a		Min. 0−5.89 ± 0.44−6.00 ± 0.47−6.79 ± 0.61−6.24 ± 0.65−6.89 ± 0.89−6.97 ± 0.95
Min. 30−5.87 ± 0.52−5.83 ± 0.55−6.91 ± 0.58−6.40 ± 0.62−8.68 ± 0.87−7.19 ± 0.92
Min. 60−6.05 ± 0.51−5.75 ± 0.54−6.56 ± 0.52−6.65 ± 0.54−6.81 ± 0.90−7.78 ± 0.96
Time to peak (s)Min. 00.27 ± 0.060.23 ± 0.060.23 ± 0.060.28 ± 0.060.29 ± 0.060.29 ± 0.07
Min. 300.27 ± 0.060.27 ± 0.060.26 ± 0.060.31 ± 0.070.23 ± 0.050.28 ± 0.06
Min. 600.26 ± 0.050.20 ± 0.060.28 ± 0.050.28 ± 0.060.22 ± 0.050.26 ± 0.06
a Denotes a significant (p < 0.05) main effect of load.
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Share and Cite

MDPI and ACS Style

Salverda, G.J.; Drew, M.D.; Krammer, S.M.; Brown, T.N. Prolonged Load Carriage Impacts Magnitude and Velocity of Knee Adduction Biomechanics. Biomechanics 2021, 1, 346-357. https://doi.org/10.3390/biomechanics1030029

AMA Style

Salverda GJ, Drew MD, Krammer SM, Brown TN. Prolonged Load Carriage Impacts Magnitude and Velocity of Knee Adduction Biomechanics. Biomechanics. 2021; 1(3):346-357. https://doi.org/10.3390/biomechanics1030029

Chicago/Turabian Style

Salverda, Gaervyn J., Micah D. Drew, Samantha M. Krammer, and Tyler N. Brown. 2021. "Prolonged Load Carriage Impacts Magnitude and Velocity of Knee Adduction Biomechanics" Biomechanics 1, no. 3: 346-357. https://doi.org/10.3390/biomechanics1030029

APA Style

Salverda, G. J., Drew, M. D., Krammer, S. M., & Brown, T. N. (2021). Prolonged Load Carriage Impacts Magnitude and Velocity of Knee Adduction Biomechanics. Biomechanics, 1(3), 346-357. https://doi.org/10.3390/biomechanics1030029

Article Metrics

Back to TopTop