Lithium on CH Divacancy Self-Healed Graphane: A First-Principles Study
Abstract
1. Introduction
2. Computational Details
3. Results and Discussion
3.1. Proposed Structures Studied
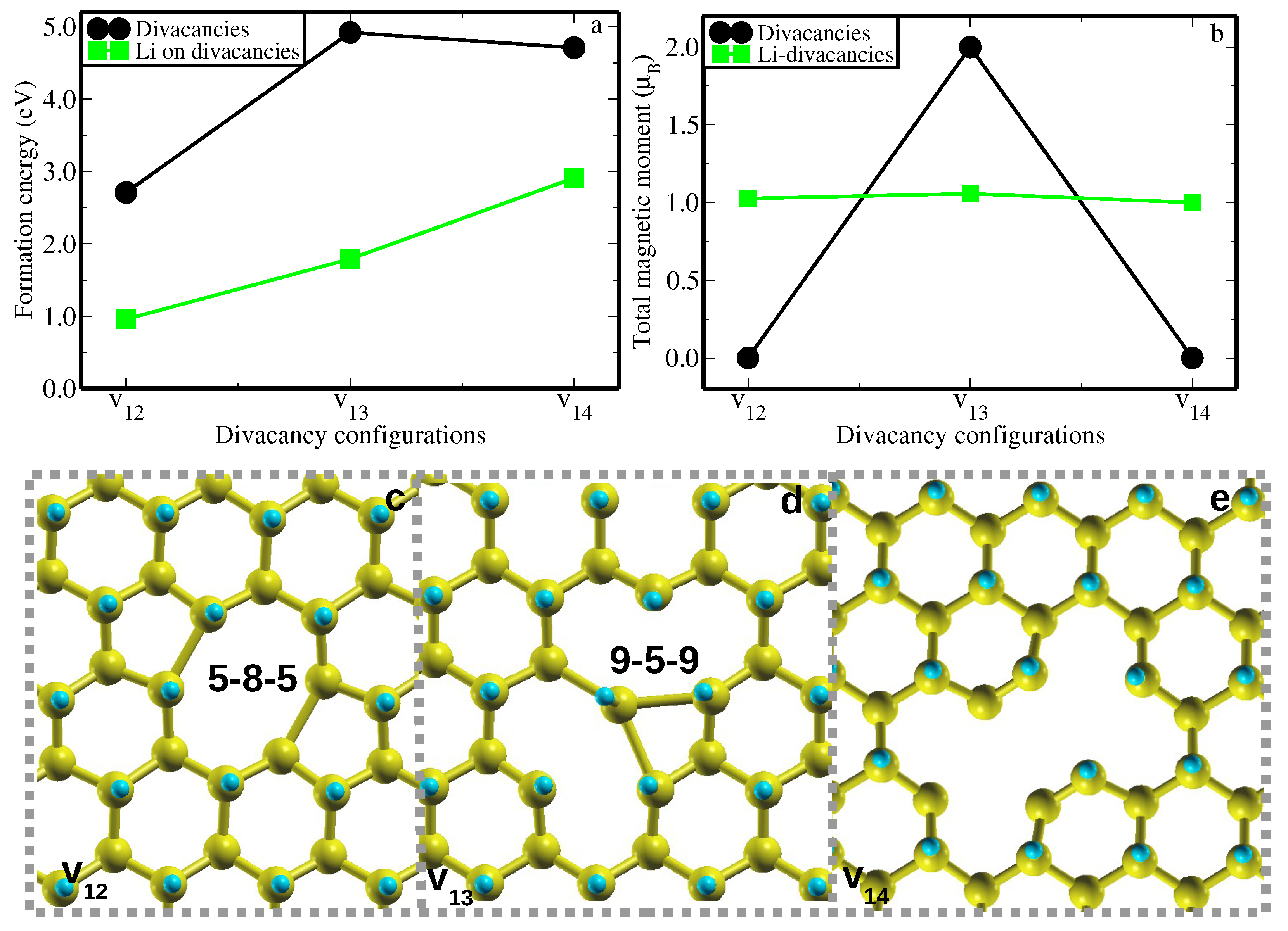
3.2. Thermodynamic Stability and Structural Properties of Different CH Vacancy Configurations
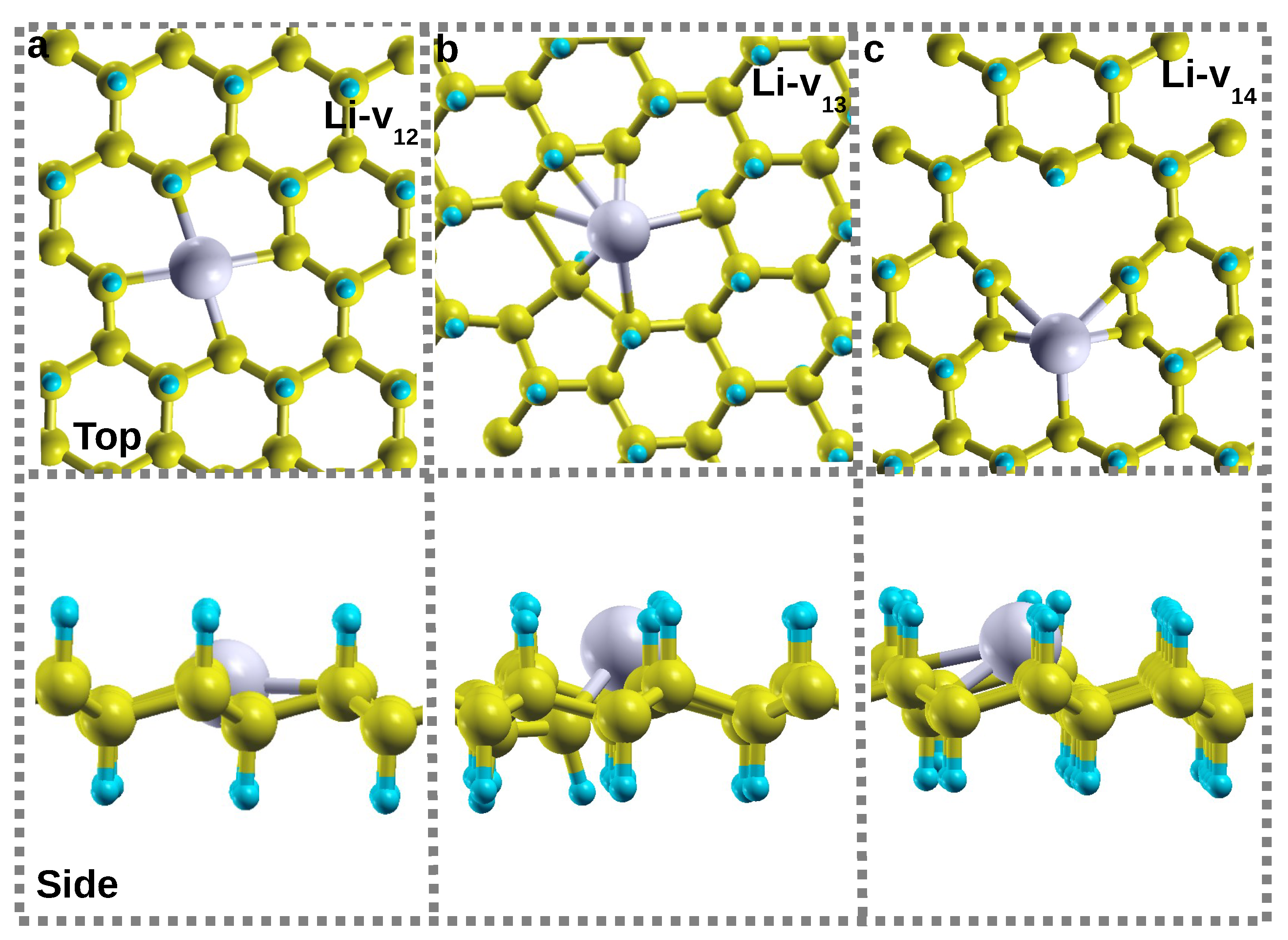
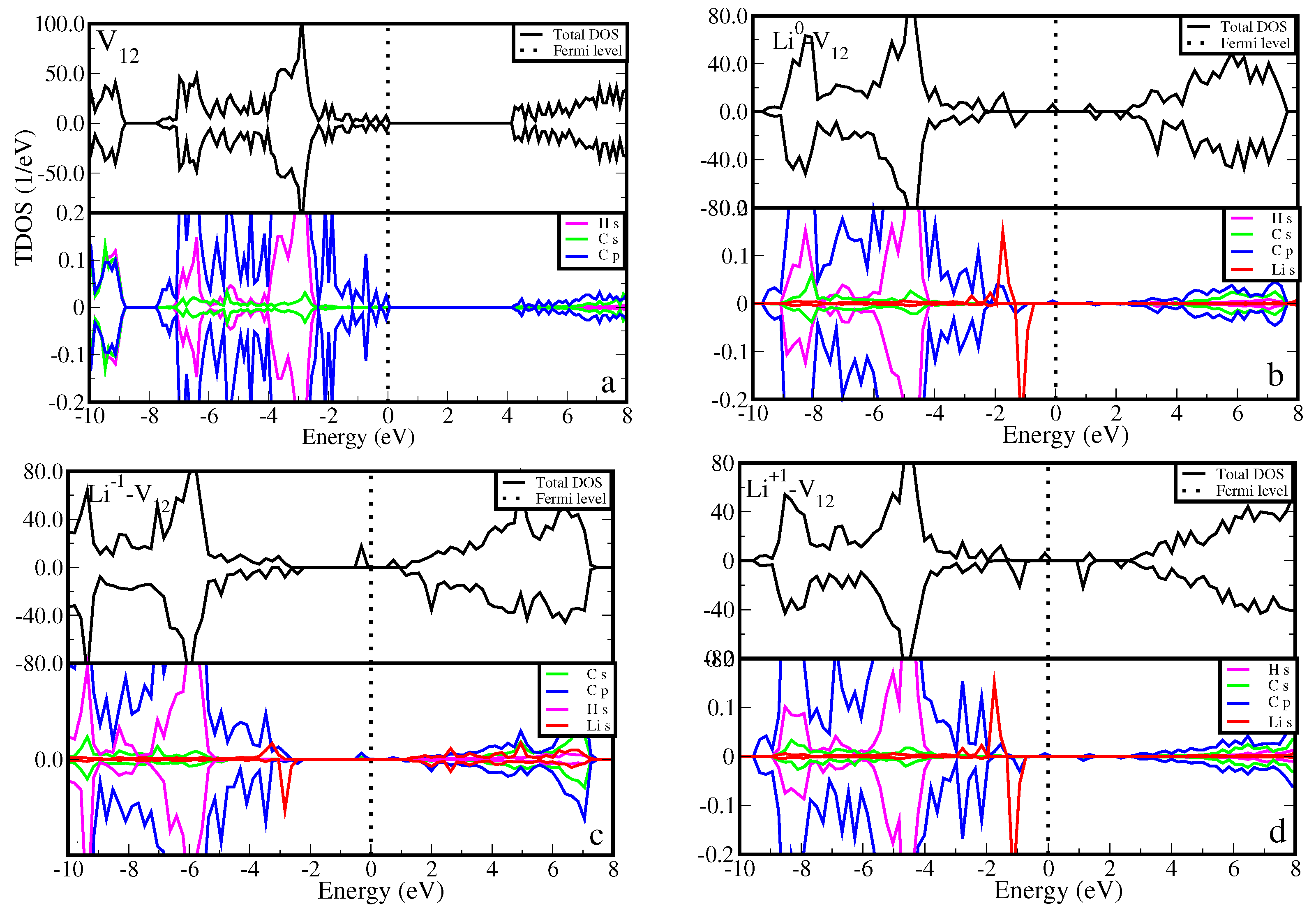
3.3. Effect of Li on the Energetic Stability and Structural Properties of Different CH Vacancy Configurations
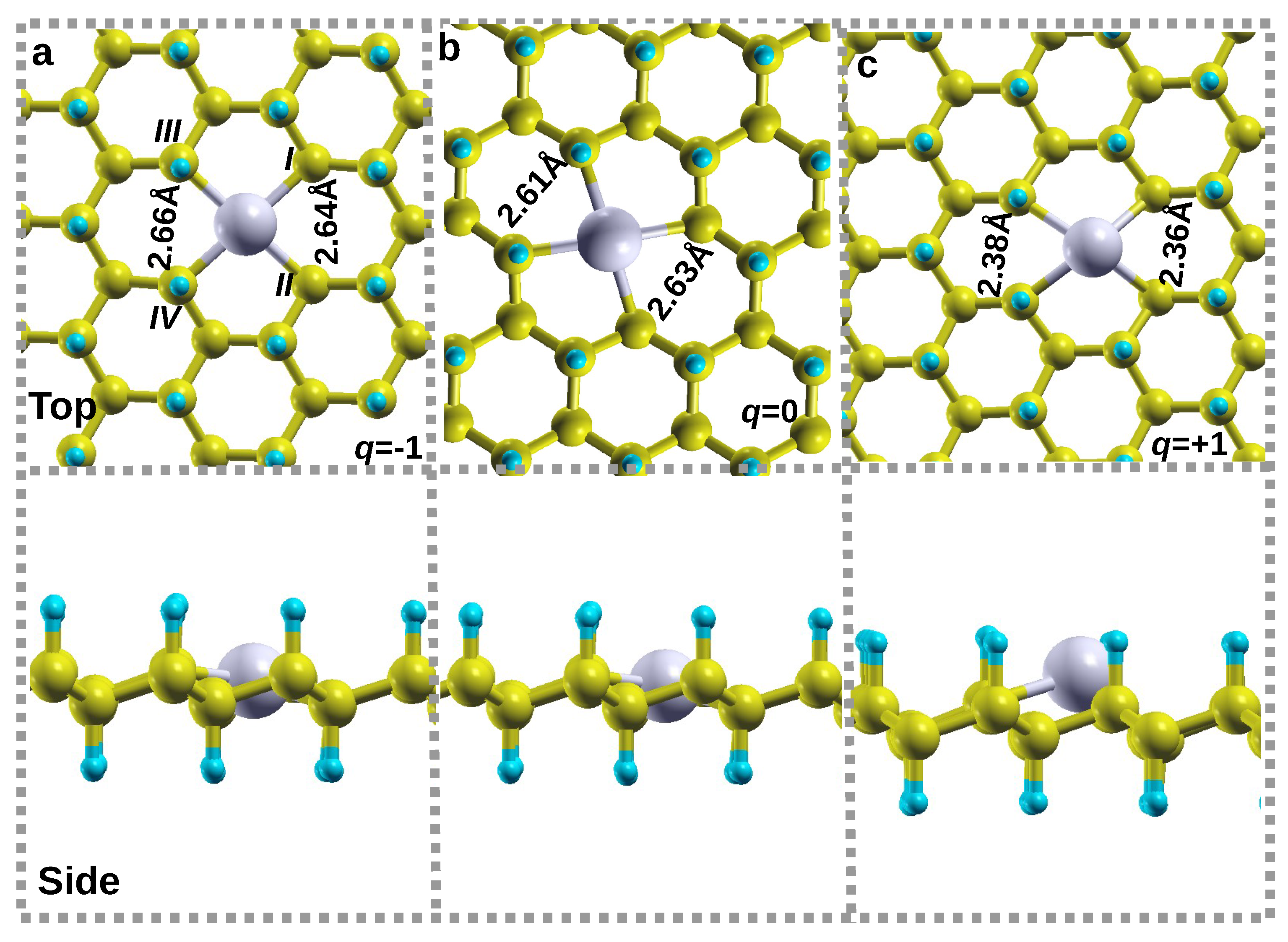
3.4. Influence of Different Charge States (−1 and +1) on the Energetic Stability, Structural, and Electronic Properties of Li-v12
| Configurations | Eb | dLi | Vacancy Reconstruction |
|---|---|---|---|
| Li-v12 | 3.25 | 0.00 | 5-8-5 changes to divacancy symmetry |
| Li-v13 | 2.07 | 0.62 | 9-4-9 translates to penta ring and vCH |
| Li-v14 | 1.99 | 1.14 | None |
| Li-vCH | 1.72 a | 1.54 a | None |
| Li- | 1.096 b, 1.29 c | 1.71 b 1.69 c | None |
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Tarascon, J.M.; Armand, M. Issues and challenges facing rechargeable lithium batteries. Nature 2001, 414, 359. [Google Scholar] [CrossRef] [PubMed]
- Oyama, N.; Tatsuma, T.; Sato, T.; Sotomura, T. Dimercaptan–polyaniline composite electrodes for lithium batteries with high energy density. Nature 1995, 374, 196. [Google Scholar] [CrossRef]
- Dubal, D.P.; Ayyad, O.; Ruiz, V.; Gómezromero, P. Hybrid energy storage: The merging of battery and supercapacitor chemistries. Chem. Soc. Rev. 2015, 44, 1777. [Google Scholar] [CrossRef] [PubMed]
- Wang, H.; Cui, L.F.; Yang, Y.; Sanchez, C.H.; Robinson, J.T. Mn3O4-graphene hybrid as a high-capacity anode material for lithium ion batteries. J. Am. Chem. Soc. 2010, 132, 13978. [Google Scholar] [CrossRef] [PubMed]
- Zou, F.; Hu, X.; Li, Z.; Long, Q.; Hu, C. MOF-derived porous ZnO/ZnFe2O4/C octahedra with hollow interiors for high-rate lithium-ion batteries. Adv. Mater. 2014, 26, 6622. [Google Scholar] [CrossRef] [PubMed]
- Sun, X.; Si, W.; Liu, X.; Deng, J.; Xi, L. Multifunctional Ni/NiO hybrid nanomembranes as anode materials for high-rate Li-ion batteries. Nano Energy 2014, 9, 168. [Google Scholar] [CrossRef]
- Chen, K.-S.; Balla, I.; Luu, N.S.; Hersam, M.C. Emerging opportunities for two- dimensional materials in lithium-ion batteries. ACS Energy Lett. 2017, 2, 2026–2034. [Google Scholar] [CrossRef]
- Peng, L.; Zhu, Y.; Chen, D.; Ruoff, R.S.; Yu, G. Two-dimensional materials for beyond- lithium-ion batteries. Adv. Energ Mater. 2016, 6, 1600025. [Google Scholar] [CrossRef]
- Li, H.; Shi, Y.; Chiu, M.-H.; Li, L.-J. Emerging energy applications of two-dimensional layered transition metal dichalcogenides. Nano Energy 2015, 18, 293–305. [Google Scholar] [CrossRef]
- Sofo, J.O.; Chaudhari, A.S.; Barber, G.D. Graphane: A two-dimensional hydrocarbon. Phys. Rev. B 2007, 75, 153401. [Google Scholar] [CrossRef]
- Sluiter, M.H.F.; Kawazoe, Y. Cluster expansion method for adsorption: Application to hydrogen chemisorption on graphene. Phys. Rev. B 2003, 68, 085410. [Google Scholar] [CrossRef]
- Hohenberg, P.; Kohn, W. Density functional theory (DFT). Phys. Rev. 1964, B864, 136. [Google Scholar]
- Elias, D.C.; Nair, R.R.; Mohiuddin, T.M.G.; Morozov, S.V.; Blake, P.; Ferrari, M.P.H.A.C.; Boukhvalov, D.W.; Katsnelson, M.I.; Geim, A.K.; Novoselov, K.S. Control of graphene’s properties by reversible hydrogenation: Evidence for graphane. Science 2009, 323, 610. [Google Scholar] [CrossRef]
- Zhou, C.; Chen, S.; Lou, J.; Wang, J.; Yang, Q.; Liu, C.; Huang, D.; Zhu, T. Graphene’s cousin: The present and future of graphane. Nanoscale Res. Lett. 2014, 9, 26. [Google Scholar] [CrossRef] [PubMed]
- Shavelkina, M.B.; Amirov, R.K.; Alikhanov, N.R.; Vakhitov, I.R.; Shatalova, T.B. Continuous Synthesis of Hydrogenated Graphene in Thermal Plasma. J. Struct. Chem. 2018, 59, 773–779. [Google Scholar] [CrossRef]
- Son, J.; Lee, S.; Kim, S.J.; Park, B.C.; Lee, H.; Kim, S.; Kim, J.H.; Hong, B.H.; Hong, J. Hydrogenated monolayer graphene with reversible and tunable wide band gap and its field-effect transistor. Nat. Comm. 2016, 7, 13261. [Google Scholar] [CrossRef] [PubMed]
- Betti, M.G.; Placidi, E.; Izzo, C.; Blundo, E.; Polimeni, A.; Sbroscia, M.; Avila, J.; Dudin, P.; Hu, K.; Ito, Y.; et al. Gap Opening in Double-Sided Highly Hydrogenated Free-Standing Graphene. Nano Lett. 2022, 22, 2971–2977. [Google Scholar] [CrossRef] [PubMed]
- Lebègue, S.; Klintenberg, M.; Eriksson, O.; Katsnelson, M.I. Accurate electronic band gap of pure and functionalized graphane from GW calculations. Phys. Rev. B. 2009, 79, 245117. [Google Scholar] [CrossRef]
- Mapasha, R.E.; Molepo, M.P.; Chetty, N. Li states on a C–H vacancy in graphane: A first-principles study. RSC Adv. 2017, 7, 39748–39757. [Google Scholar] [CrossRef]
- Sahin, H.; Leenaerts, O.; Singh, S.K.; Peeters, F.M. Graphane. Wires Comput. Mol. Sci. 2015, 5, 255–272. [Google Scholar] [CrossRef]
- Keith, E.; Whitener, J. Review Article: Hydrogenated graphene: A user’s guide. J. Vac. Sci. Technol. A 2018, 36, 05G401. [Google Scholar] [CrossRef]
- Watcharinyanon, S.; Johansson, L.; Zakharov, A.; Virojanadara, C. Studies of Li intercalation of hydrogenated graphene on SiC(0001). Surf. Scie 2012, 606, 401–406. [Google Scholar] [CrossRef]
- Yang, Y.E.; Xiao, Y.; Yan, X.H. Charge distribution of lithium-doped graphane/graphene hybrid system: Role of nearly-free electronic states. Sol. Stat. Commun. 2016, 229, 43–48. [Google Scholar] [CrossRef]
- Sahin, H.; Ataca, C.; Ciraci, S. Electronic and magnetic properties of graphane nanoribbons. Phys. Rev. B 2010, 81, 205417. [Google Scholar] [CrossRef]
- Sahin, H.; Ataca, C.; Ciraci, S. Magnetization of graphane by dehydrogenation. Appl. Phys. Lett. 2009, 95, 222510. [Google Scholar] [CrossRef]
- Berashevich, J.; Chakraborty, T. Sustained ferromagnetism induced by H-vacancies in graphane. Nanotechnology 2010, 21, 355201. [Google Scholar] [CrossRef] [PubMed][Green Version]
- Mapasha, R.E.; Molepo, M.P.; Chetty, N. Ab initio studies of isolated hydrogen vacancies in graphane. Phys. E 2016, 79, 52–58. [Google Scholar] [CrossRef]
- Pujari, B.S.; Kanhere, D.G. Density functional investigations of defect-induced mid-gap states in graphane. J. Phys. Chem. C 2009, 113, 21063. [Google Scholar] [CrossRef]
- Hedin, L. New method for calculating the one-particle Green’s function with application to the electron-gas problem. Phys. Rev. 1965, 139, A796. [Google Scholar] [CrossRef]
- Heyd, J.; Scuseria, G.E.; Ernzerhof, M. Hybrid functionals based on a screened Coulomb potential. J. Chem. Phys. 2003, 118, 8207. [Google Scholar] [CrossRef]
- Kresse, G.; Hafner, J. Ab initio molecular dynamics for liquid metals. Phys. Rev. B 1993, 47, 558. [Google Scholar] [CrossRef] [PubMed]
- Blochl, P.E. Projector augmented-wave method. Phys. Rev. B 1994, 50, 17953. [Google Scholar] [CrossRef] [PubMed]
- Monkhorst, H.J.; Pack, J.D. Special points for Brillouin-zone integrations. Phys. Rev. B 1976, 13, 5188. [Google Scholar] [CrossRef]
- Zhang, S.B.; Northrup, J.E. Chemical potential dependence of defect formation energies in GaAs: Application to Ga self-diffusion. Phys. Rev. Lett. 1991, 67, 2339. [Google Scholar] [CrossRef] [PubMed]
- Chan, K.T.; Neaton, J.B.; Cohen, M.L. First-principles study of metal adatom adsorption on graphene. Phys. Rev. 2008, 77, 235430. [Google Scholar] [CrossRef]
- Jin, C.; Lan, H.; Peng, L.; Suenaga, K.; Iijima, S. Deriving carbon atomic chains from graphene. Phys. Rev. Lett. 2009, 102, 205501. [Google Scholar] [CrossRef]
- Garay-Tapia, A.M.; Romero, A.H.; Barone, V. Lithium adsorption on graphene: From isolated adatoms to metallic sheets. J. Chem. Theory Comput. 2012, 8, 1064–1071. [Google Scholar] [CrossRef]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mapasha, R.E.; Kgalema, S.P.; Mapingire, H.; Igumbor, E. Lithium on CH Divacancy Self-Healed Graphane: A First-Principles Study. Nanoenergy Adv. 2024, 4, 122-132. https://doi.org/10.3390/nanoenergyadv4010007
Mapasha RE, Kgalema SP, Mapingire H, Igumbor E. Lithium on CH Divacancy Self-Healed Graphane: A First-Principles Study. Nanoenergy Advances. 2024; 4(1):122-132. https://doi.org/10.3390/nanoenergyadv4010007
Chicago/Turabian StyleMapasha, Refilwe Edwin, Sentserere Phodisho Kgalema, Hezekia Mapingire, and Emmanuel Igumbor. 2024. "Lithium on CH Divacancy Self-Healed Graphane: A First-Principles Study" Nanoenergy Advances 4, no. 1: 122-132. https://doi.org/10.3390/nanoenergyadv4010007
APA StyleMapasha, R. E., Kgalema, S. P., Mapingire, H., & Igumbor, E. (2024). Lithium on CH Divacancy Self-Healed Graphane: A First-Principles Study. Nanoenergy Advances, 4(1), 122-132. https://doi.org/10.3390/nanoenergyadv4010007





