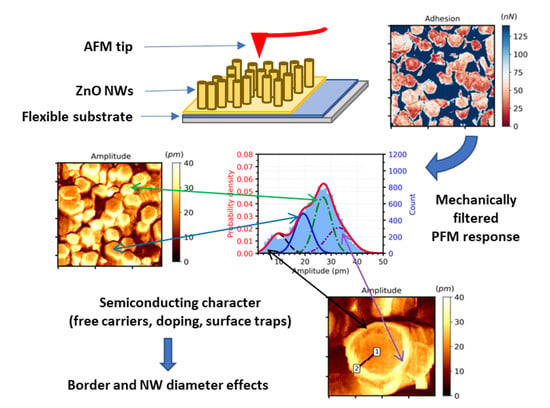
Size and Semiconducting Effects on the Piezoelectric Performances of ZnO Nanowires Grown onto Gravure-Printed Seed Layers on Flexible Substrates
Abstract
:1. Introduction
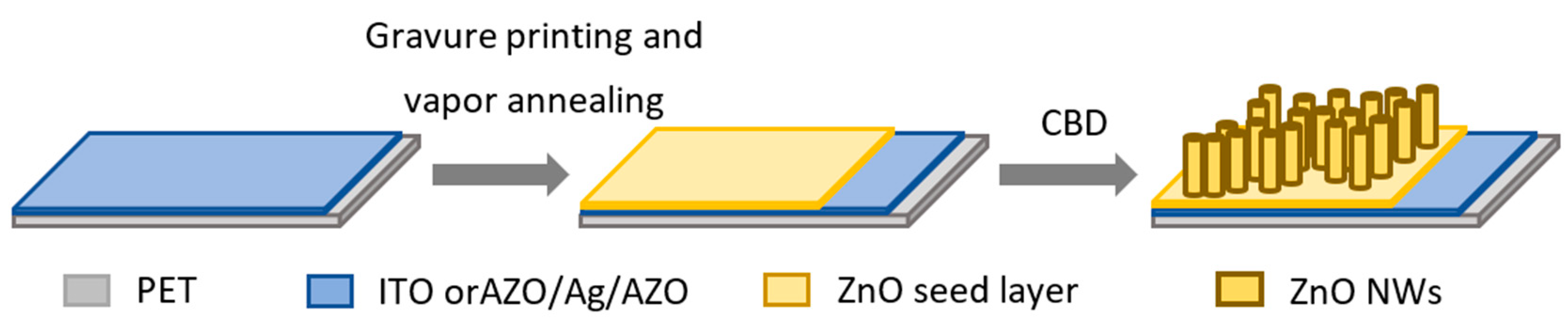
2. Experimental and Simulation Details
3. Results and Discussion
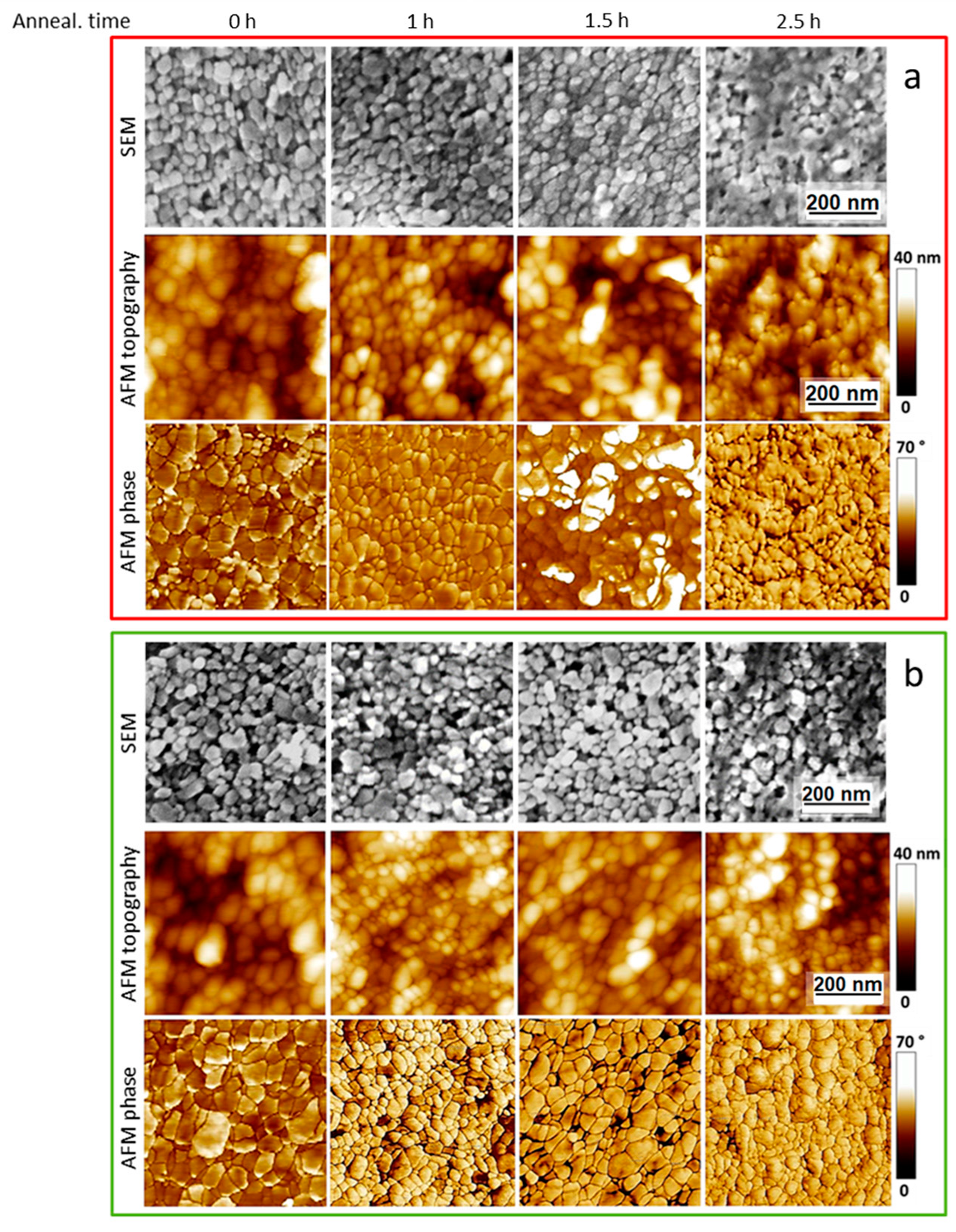
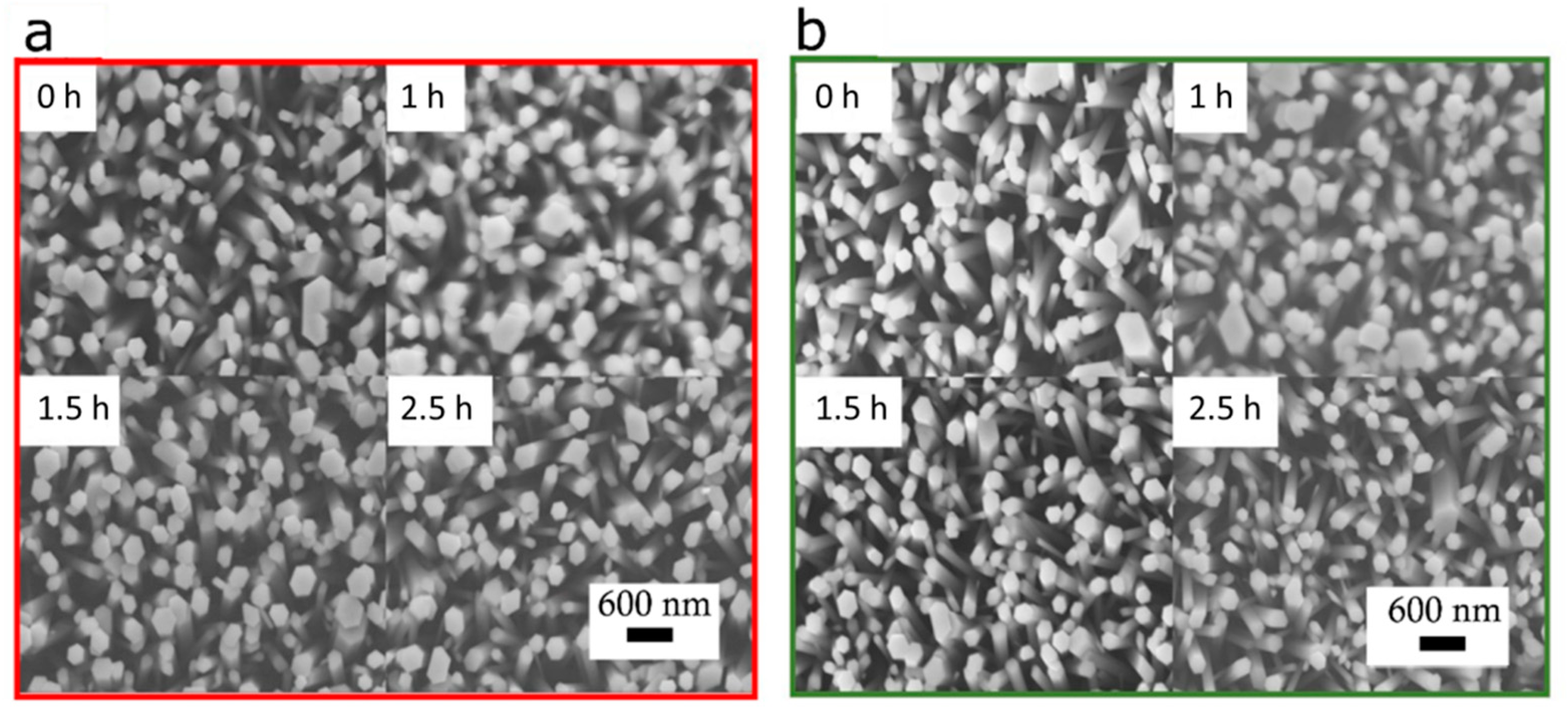
3.1. Structural Characterization
3.2. Piezoelectric Measurements
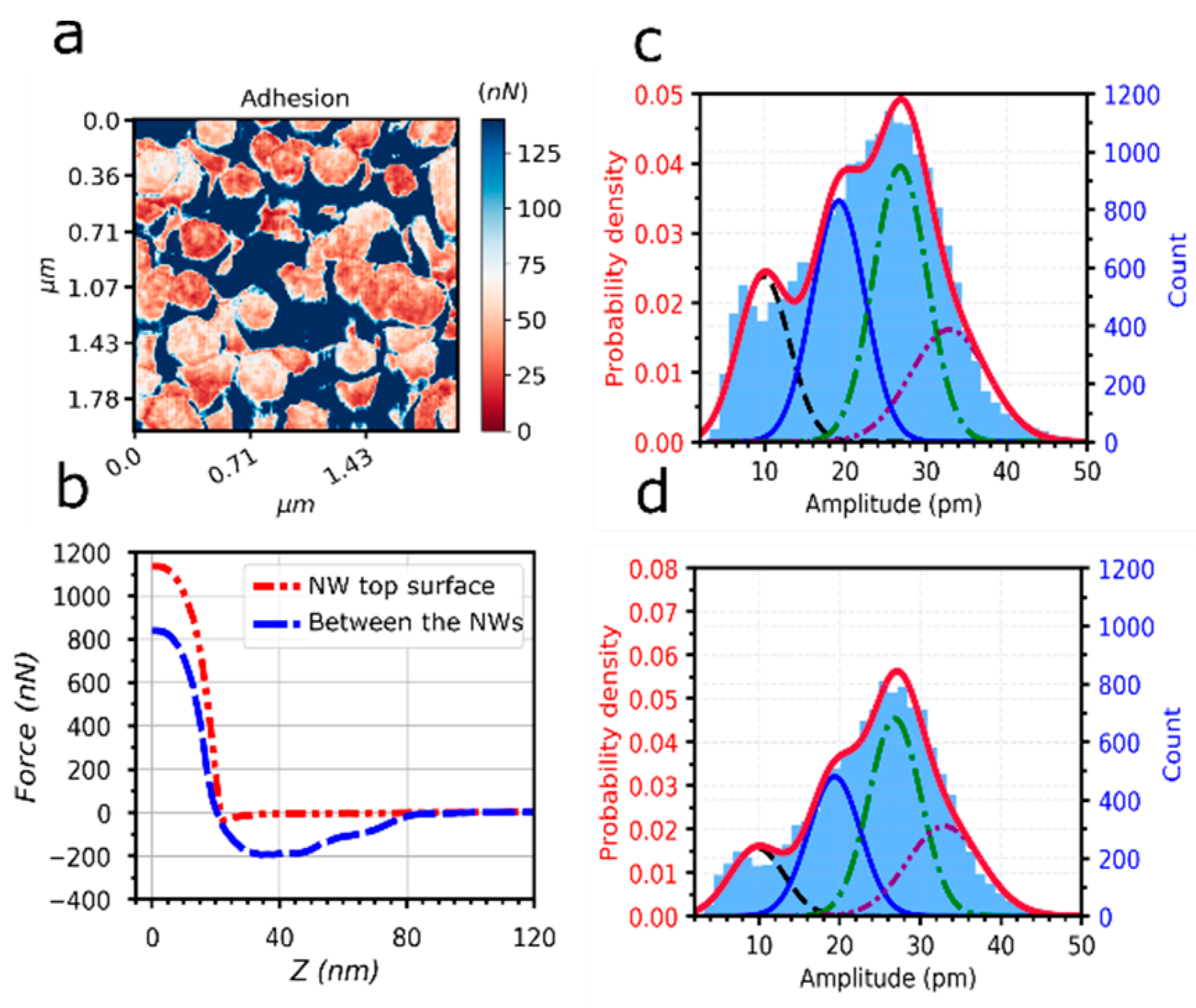
3.2.1. Extracting the Piezoelectric Properties of the NWs’ Top Surface
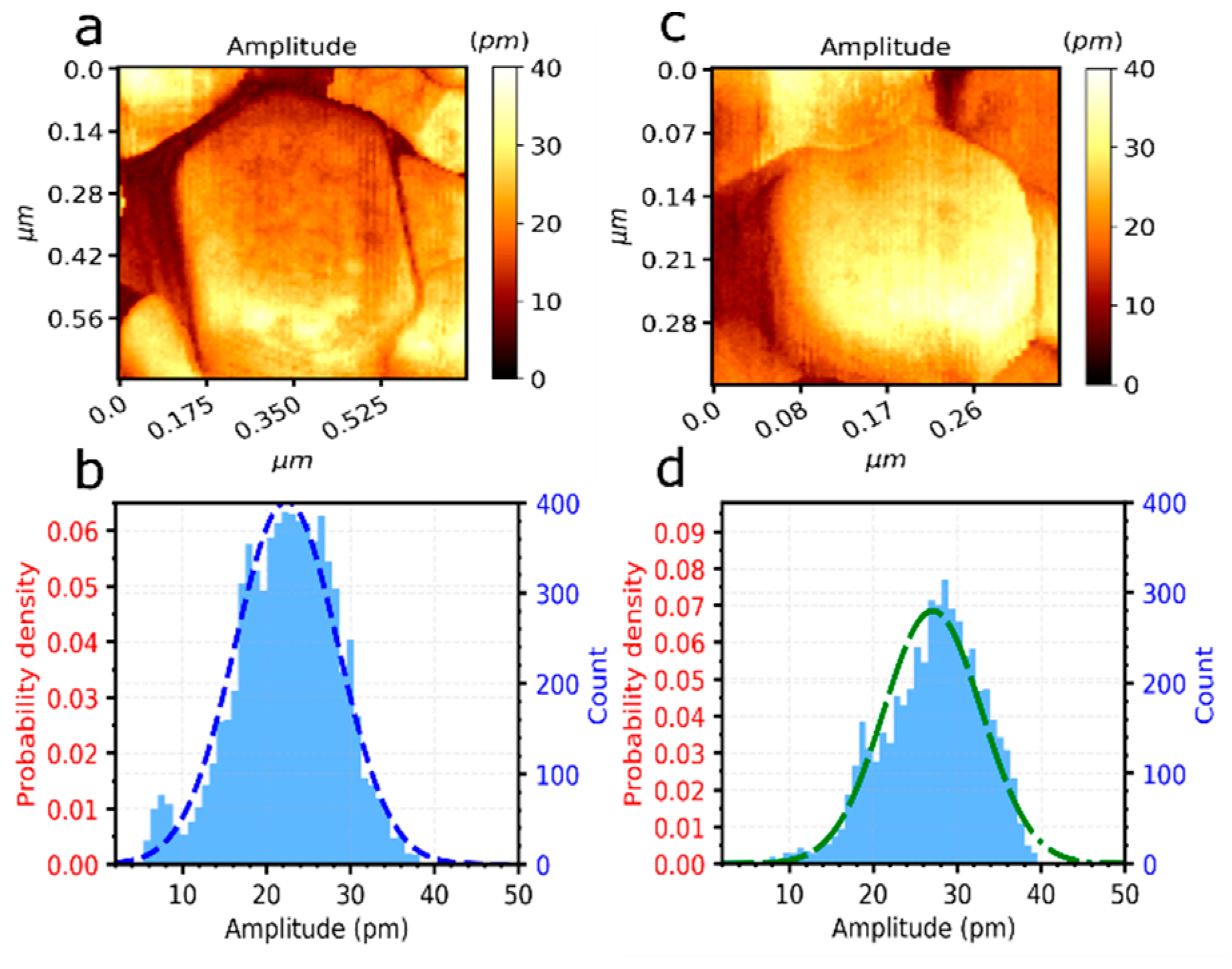
3.2.2. Effect of NW Radius
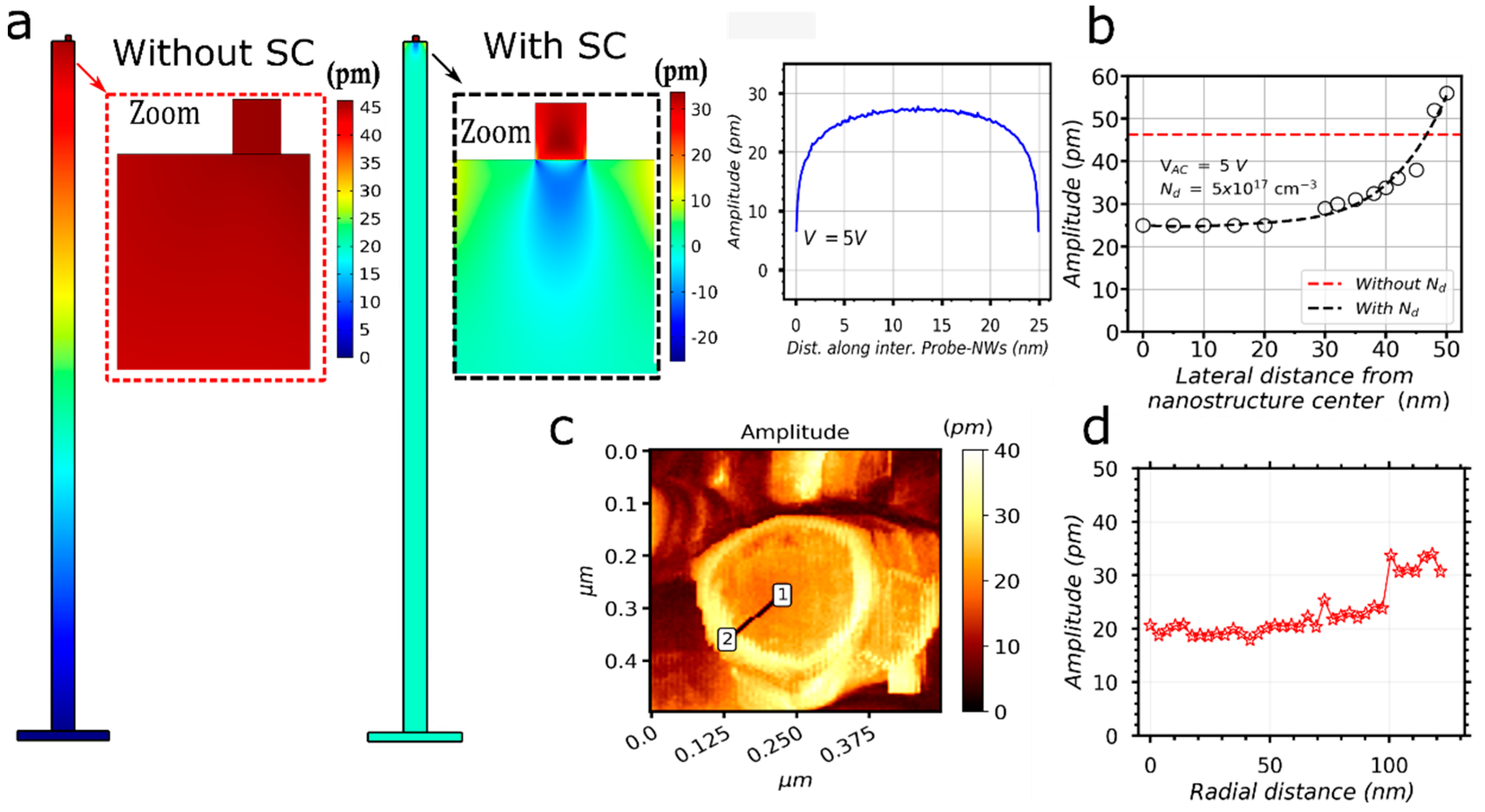
3.2.3. Border Effects
3.2.4. Comparison with Other Studies
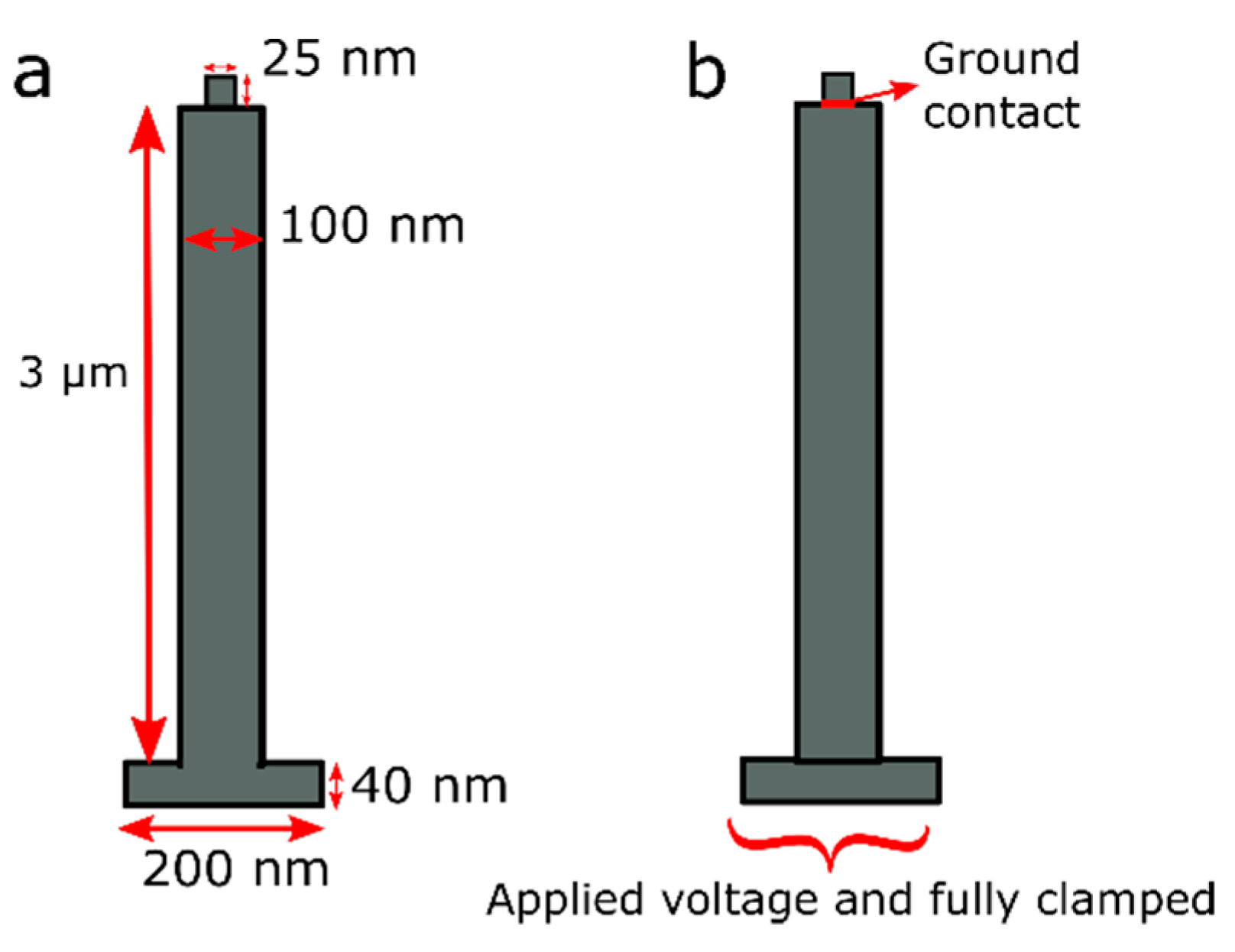
3.3. Simulation Results
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Wang, Z.L.; Song, J. Piezoelectric Nanogenerators Based on Zinc Oxide Nanowire Arrays. Science 2006, 312, 242–246. [Google Scholar] [CrossRef] [PubMed]
- Wang, Z.L. Towards Self-Powered Nanosystems: From Nanogenerators to Nanopiezotronics. Adv. Funct. Mater. 2008, 18, 3553–3567. [Google Scholar] [CrossRef]
- Bairagi, S.; Ghosh, S.; Ali, S.W. A Fully Sustainable, Self-Poled, Bio-Waste Based Piezoelectric Nanogenerator: Electricity Generation from Pomelo Fruit Membrane. Sci. Rep. 2020, 10, 12121. [Google Scholar] [CrossRef] [PubMed]
- Xie, L.; Li, X.; Cai, S.; Huang, L.; Li, J. Increased Energy Harvesting from Backpack to Serve as Self-Sustainable Power Source via a Tube-like Harvester. Mech. Syst. Signal Process. 2017, 96, 215–225. [Google Scholar] [CrossRef]
- Wen, B.; Sader, J.E.; Boland, J.J. Mechanical Properties of ZnO Nanowires. Phys. Rev. Lett. 2008, 101, 175502. [Google Scholar] [CrossRef]
- Gordon, M.J.; Baron, T.; Dhalluin, F.; Gentile, P.; Ferret, P. Size Effects in Mechanical Deformation and Fracture of Cantilevered Silicon Nanowires. Nano Lett. 2009, 9, 525–529. [Google Scholar] [CrossRef]
- Garcia, A.J.L.; Sico, G.; Montanino, M.; Defoor, V.; Pusty, M.; Mescot, X.; Loffredo, F.; Villani, F.; Nenna, G.; Ardila, G. Low-Temperature Growth of ZnO Nanowires from Gravure-Printed ZnO Nanoparticle Seed Layers for Flexible Piezoelectric Devices. Nanomaterials 2021, 11, 1430. [Google Scholar] [CrossRef]
- Lin, L.; Hu, Y.; Xu, C.; Zhang, Y.; Zhang, R.; Wen, X.; Lin Wang, Z. Transparent Flexible Nanogenerator as Self-Powered Sensor for Transportation Monitoring. Nano Energy 2013, 2, 75–81. [Google Scholar] [CrossRef]
- Kammel, R.S.; Sabry, R.S. Effects of the Aspect Ratio of ZnO Nanorods on the Performance of Piezoelectric Nanogenerators. J. Sci. Adv. Mater. Devices 2019, 4, 420–424. [Google Scholar] [CrossRef]
- He, Q.; Li, X.; Zhang, J.; Zhang, H.; Briscoe, J. P–N Junction-Based ZnO Wearable Textile Nanogenerator for Biomechanical Energy Harvesting. Nano Energy 2021, 85, 105938. [Google Scholar] [CrossRef]
- Zhu, G.; Wang, A.C.; Liu, Y.; Zhou, Y.; Wang, Z.L. Functional Electrical Stimulation by Nanogenerator with 58 V Output Voltage. Nano Lett. 2012, 12, 3086–3090. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Dahiya, A.S.; Morini, F.; Boubenia, S.; Nadaud, K.; Alquier, D.; Poulin-Vittrant, G. Organic/Inorganic Hybrid Stretchable Piezoelectric Nanogenerators for Self-Powered Wearable Electronics. Adv. Mater. Technol. 2018, 3, 1700249. [Google Scholar] [CrossRef] [Green Version]
- Hu, Y.; Lin, L.; Zhang, Y.; Wang, Z.L. Replacing a Battery by a Nanogenerator with 20 V Output. Adv. Mater. 2012, 24, 110–114. [Google Scholar] [CrossRef] [PubMed]
- Romano, G.; Mantini, G.; Carlo, A.D.; D’Amico, A.; Falconi, C.; Wang, Z.L. Piezoelectric Potential in Vertically Aligned Nanowires for High Output Nanogenerators. Nanotechnology 2011, 22, 465401. [Google Scholar] [CrossRef]
- Tao, R.; Mouis, M.; Ardila, G. Unveiling the Influence of Surface Fermi Level Pinning on the Piezoelectric Response of Semiconducting Nanowires. Adv. Electron. Mater. 2018, 4, 1700299. [Google Scholar] [CrossRef]
- Kim, S.; Seol, D.; Lu, X.; Alexe, M.; Kim, Y. Electrostatic-Free Piezoresponse Force Microscopy. Sci. Rep. 2017, 7, 41657. [Google Scholar] [CrossRef]
- Jaloustre, L.; Le Denmat, S.; Auzelle, T.; Azadmand, M.; Geelhaar, L.; Dahlem, F.; Songmuang, R. Toward Quantitative Measurements of Piezoelectricity in III-N Semiconductor Nanowires. ACS Appl. Nano Mater. 2021, 4, 43–52. [Google Scholar] [CrossRef]
- Agrawal, R.; Espinosa, H.D. Giant Piezoelectric Size Effects in Zinc Oxide and Gallium Nitride Nanowires. A First Principles Investigation. Nano Lett. 2011, 11, 786–790. [Google Scholar] [CrossRef]
- Lopez Garcia, A.J.; Tao, R.; Mouis, M.; Ardila, G. A New Approach to Calculate the Piezoelectric Coefficient of Piezo-Semiconductor Nanowires Integrated in Nanocomposites: Experiment and Simulation. In Proceedings of the 2021 21st International Conference on Solid-State Sensors, Actuators and Microsystems (Transducers), online, 20–24 June 2021; pp. 1056–1059. [Google Scholar]
- Sico, G.; Montanino, M.; Ventre, M.; Mollo, V.; Prontera, C.T.; Minarini, C.; Magnani, G. Pressureless Sintering of ZnO Thin Film on Plastic Substrate via Vapor Annealing Process at Near-Room Temperature. Scr. Mater. 2019, 164, 48–51. [Google Scholar] [CrossRef]
- Bui, Q.C.; Ardila, G.; Sarigiannidou, E.; Roussel, H.; Jiménez, C.; Chaix-Pluchery, O.; Guerfi, Y.; Bassani, F.; Donatini, F.; Mescot, X.; et al. Morphology Transition of ZnO from Thin Film to Nanowires on Silicon and Its Correlated Enhanced Zinc Polarity Uniformity and Piezoelectric Responses. ACS Appl. Mater. Interfaces 2020, 12, 29583–29593. [Google Scholar] [CrossRef]
- Rohrer, G.S. Grain Boundary Energy Anisotropy: A Review. J. Mater. Sci. 2011, 46, 5881–5895. [Google Scholar] [CrossRef] [Green Version]
- Lee, J.-S.; Wiederhorn, S.M. Effects of Polarity on Grain-Boundary Migration in ZnO. J. Am. Ceram. Soc. 2004, 87, 1319–1323. [Google Scholar] [CrossRef]
- German, R.M. Coarsening in Sintering: Grain Shape Distribution, Grain Size Distribution, and Grain Growth Kinetics in Solid-Pore Systems. Crit. Rev. Solid State Mater. Sci. 2010, 35, 263–305. [Google Scholar] [CrossRef]
- Sui, M.; Pandey, P.; Li, M.-Y.; Zhang, Q.; Kunwar, S.; Lee, J. Tuning the Configuration of Au Nanostructures: From Vermiform-like, Rod-like, Triangular, Hexagonal, to Polyhedral Nanostructures on c-Plane GaN. J. Mater. Sci. 2017, 52, 391–407. [Google Scholar] [CrossRef]
- Kuang, Q.; Wang, X.; Jiang, Z.; Xie, Z.; Zheng, L. High-Energy-Surface Engineered Metal Oxide Micro- and Nanocrystallites and Their Applications. Acc. Chem. Res. 2014, 47, 308–318. [Google Scholar] [CrossRef]
- Wu, W.-Y.; Yeh, C.-C.; Ting, J.-M. Effects of Seed Layer Characteristics on the Synthesis of ZnO Nanowires. J. Am. Ceram. Soc. 2009, 92, 2718–2723. [Google Scholar] [CrossRef]
- Nirmal Peiris, T.A.; Alessa, H.; Sagu, J.S.; Ahmad Bhatti, I.; Isherwood, P.; Upul Wijayantha, K.G. Effect of ZnO Seed Layer Thickness on Hierarchical ZnO Nanorod Growth on Flexible Substrates for Application in Dye-Sensitised Solar Cells. J. Nanopart. Res. 2013, 15, 2115. [Google Scholar] [CrossRef]
- Syrrokostas, G.; Govatsi, K.; Yannopoulos, S.N. High-Quality, Reproducible ZnO Nanowire Arrays Obtained by a Multiparameter Optimization of Chemical Bath Deposition Growth. Cryst. Growth Des. 2016, 16, 2140–2150. [Google Scholar] [CrossRef]
- Lopez Garcia, A.J.; Mouis, M.; Consonni, V.; Ardila, G. Dimensional Roadmap for Maximizing the Piezoelectrical Response of ZnO Nanowire-Based Transducers: Impact of Growth Method. Nanomaterials 2021, 11, 941. [Google Scholar] [CrossRef]
- Tian, G.; Xiong, D.; Su, Y.; Yang, T.; Gao, Y.; Yan, C.; Deng, W.; Jin, L.; Zhang, H.; Fan, X.; et al. Understanding the Potential Screening Effect through the Discretely Structured ZnO Nanorods Piezo Array. Nano Lett. 2020, 20, 4270–4277. [Google Scholar] [CrossRef]
- Tamvakos, D.; Lepadatu, S.; Antohe, V.-A.; Tamvakos, A.; Weaver, P.M.; Piraux, L.; Cain, M.G.; Pullini, D. Piezoelectric Properties of Template-Free Electrochemically Grown ZnO Nanorod Arrays. Appl. Surf. Sci. 2015, 356, 1214–1220. [Google Scholar] [CrossRef]
- Fan, H.J.; Lee, W.; Hauschild, R.; Alexe, M.; Le Rhun, G.; Scholz, R.; Dadgar, A.; Nielsch, K.; Kalt, H.; Krost, A.; et al. Template-Assisted Large-Scale Ordered Arrays of ZnO Pillars for Optical and Piezoelectric Applications. Small 2006, 2, 561–568. [Google Scholar] [CrossRef] [PubMed]
- Scrymgeour, D.A.; Sounart, T.L.; Simmons, N.C.; Hsu, J.W.P. Polarity and Piezoelectric Response of Solution Grown Zinc Oxide Nanocrystals on Silver. J. Appl. Phys. 2007, 101, 014316. [Google Scholar] [CrossRef]
- Cavallini, D.; Fortunato, M.; Bellis, G.D.; Sarto, M.S. PFM Characterization of Piezoelectric PVDF/ZnONanorod Thin Films. In Proceedings of the 2018 IEEE 18th International Conference on Nanotechnology (IEEE-NANO), Cork, Ireland, 23–26 July 2018; pp. 1–3. [Google Scholar]
- Broitman, E.; Soomro, M.Y.; Lu, J.; Willander, M.; Hultman, L. Nanoscale Piezoelectric Response of ZnO Nanowires Measured Using a Nanoindentation Technique. Phys. Chem. Chem. Phys. 2013, 15, 11113–11118. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Chelu, M.; Stroescu, H.; Anastasescu, M.; Calderon-Moreno, J.M.; Preda, S.; Stoica, M.; Fogarassy, Z.; Petrik, P.; Gheorghe, M.; Parvulescu, C.; et al. High-Quality PMMA/ZnO NWs Piezoelectric Coating on Rigid and Flexible Metallic Substrates. Appl. Surf. Sci. 2020, 529, 147135. [Google Scholar] [CrossRef]
- Novak, N.; Keil, P.; Frömling, T.; Schader, F.H.; Martin, A.; Webber, K.G.; Rödel, J. Influence of Metal/Semiconductor Interface on Attainable Piezoelectric and Energy Harvesting Properties of ZnO. Acta Mater. 2019, 162, 277–283. [Google Scholar] [CrossRef]
- Tao, R.; Ardila, G.; Hinchet, R.; Michard, A.; Montès, L.; Mouis, M. Will Composite Nanomaterials Replace Piezoelectric Thin Films for Energy Transduction Applications? In Future Trends in Microelectronics: Journey into the Unknown; Wiley, John & Sons: Hoboken, NJ, USA; IEEE Press: Piscataway, NJ, USA, 2016; pp. 291–307. [Google Scholar]
- Bui, Q.C.; Salem, B.; Roussel, H.; Mescot, X.; Guerfi, Y.; Jiménez, C.; Consonni, V.; Ardila, G. Effects of Thermal Annealing on the Structural and Electrical Properties of ZnO Thin Films for Boosting Their Piezoelectric Response. J. Alloys Compd. 2021, 870, 159512. [Google Scholar] [CrossRef]
- Zhao, M.-H.; Wang, Z.-L.; Mao, S.X. Piezoelectric Characterization of Individual Zinc Oxide Nanobelt Probed by Piezoresponse Force Microscope. Nano Lett. 2004, 4, 587–590. [Google Scholar] [CrossRef]
- Abu Ali, T.; Pilz, J.; Schäffner, P.; Kratzer, M.; Teichert, C.; Stadlober, B.; Coclite, A.M. Piezoelectric Properties of Zinc Oxide Thin Films Grown by Plasma-Enhanced Atomic Layer Deposition. Phys. Status Solidi A 2020, 217, 2000319. [Google Scholar] [CrossRef]
- Zhou, Z.-Q.; Taylor, K.; Gjonaj, E.; Frömling, T.; Xu, B.-X. Finite Element Simulations on Piezoelectric Modulation of ZnO Grain Boundary Barrier Height. J. Appl. Phys. 2019, 126, 205101. [Google Scholar] [CrossRef]
- Bateman, T.B. Elastic Moduli of Single-Crystal Zinc Oxide. J. Appl. Phys. 1962, 33, 3309–3312. [Google Scholar] [CrossRef]
- Carlotti, G.; Socino, G.; Petri, A.; Verona, E. Acoustic Investigation of the Elastic Properties of ZnO Films. Appl. Phys. Lett. 1987, 51, 1889–1891. [Google Scholar] [CrossRef]
- Ashkenov, N.; Mbenkum, B.N.; Bundesmann, C.; Riede, V.; Lorenz, M.; Spemann, D.; Kaidashev, E.M.; Kasic, A.; Schubert, M.; Grundmann, M.; et al. Infrared Dielectric Functions and Phonon Modes of High-Quality ZnO Films. J. Appl. Phys. 2003, 93, 126–133. [Google Scholar] [CrossRef]
- Look, D.C.; Reynolds, D.C.; Sizelove, J.R.; Jones, R.L.; Litton, C.W.; Cantwell, G.; Harsch, W.C. Electrical Properties of Bulk ZnO. Solid State Commun. 1998, 105, 399–401. [Google Scholar] [CrossRef]
- Canet-Ferrer, J.; Coronado, E.; Forment-Aliaga, A.; Pinilla-Cienfuegos, E. Correction of the Tip Convolution Effects in the Imaging of Nanostructures Studied through Scanning Force Microscopy. Nanotechnology 2014, 25, 395703. [Google Scholar] [CrossRef] [PubMed]

| ZnO Structure | Diameter (nm), Length (µm), Thickness (nm) | Substrate | Tip Stiffness (N/m)/Frequency (Hz) | Polarity | Method | |
|---|---|---|---|---|---|---|
| NW [32] | 150, 1.5, NC | Si/Au | 8.8/40k | 11.8 | + | PFM |
| NW [36] | 150, 2.3, NC | paper/PEDOT:PSS | NC/NC | 9.8 | NC | Nanoindentation |
| NW [33] | 300, 2, NC | GaN | 3/33k | 7.5 | + | PFM |
| NW [34] | 150, 600, NC | Ag | 40/15k | 4.41 | + | PFM |
| NW [35] | 30, NC, NC | Glass/ITO, PET/ITO | 5/15k | 2.13 and 5.2 | + | PFM |
| NW/PMMA composite [37] | 80–160, 1.3–1.7 | Pt, Au, Ti | NC/30–110 | 3, 3, 7–26 | + | Piezometer |
| Bulk [41] | NC, NC, NC | NC | 40/30–300k | 9.93 | + | PFM |
| Thin film [42] | NC, NC, 70 | Glass/ITO | NC/NC | 3–20 | + | Current/charge |
| Thin film [40] | NC, NC, 1100 | Si | 45/7k | 1.8–2.6 | +/− | PFM |
| Single crystal [38] | 5 × 5 × 5 mm3 | ITO, Ag | NC/NC | 0, 11 | + | Impedance and direct piezoelectric measurements |
| NW (this work) | 100–200, 3, NC | PET-ITO, PET-AZO | 43–50/204–497k | 4–5 | + | PFM |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Garcia, A.J.L.; Jalabert, T.; Pusty, M.; Defoor, V.; Mescot, X.; Montanino, M.; Sico, G.; Loffredo, F.; Villani, F.; Nenna, G.; et al. Size and Semiconducting Effects on the Piezoelectric Performances of ZnO Nanowires Grown onto Gravure-Printed Seed Layers on Flexible Substrates. Nanoenergy Adv. 2022, 2, 197-209. https://doi.org/10.3390/nanoenergyadv2020008
Garcia AJL, Jalabert T, Pusty M, Defoor V, Mescot X, Montanino M, Sico G, Loffredo F, Villani F, Nenna G, et al. Size and Semiconducting Effects on the Piezoelectric Performances of ZnO Nanowires Grown onto Gravure-Printed Seed Layers on Flexible Substrates. Nanoenergy Advances. 2022; 2(2):197-209. https://doi.org/10.3390/nanoenergyadv2020008
Chicago/Turabian StyleGarcia, Andrés Jenaro Lopez, Thomas Jalabert, Manojit Pusty, Viktor Defoor, Xavier Mescot, Maria Montanino, Giuliano Sico, Fausta Loffredo, Fulvia Villani, Giuseppe Nenna, and et al. 2022. "Size and Semiconducting Effects on the Piezoelectric Performances of ZnO Nanowires Grown onto Gravure-Printed Seed Layers on Flexible Substrates" Nanoenergy Advances 2, no. 2: 197-209. https://doi.org/10.3390/nanoenergyadv2020008
APA StyleGarcia, A. J. L., Jalabert, T., Pusty, M., Defoor, V., Mescot, X., Montanino, M., Sico, G., Loffredo, F., Villani, F., Nenna, G., & Ardila, G. (2022). Size and Semiconducting Effects on the Piezoelectric Performances of ZnO Nanowires Grown onto Gravure-Printed Seed Layers on Flexible Substrates. Nanoenergy Advances, 2(2), 197-209. https://doi.org/10.3390/nanoenergyadv2020008