Uncovering the Possibilities of Ceramic Ba(1−x)CoxTiO3 Nanocrystals: Heightened Electrical and Dielectric Attributes
Abstract
1. Introduction
2. Experimental
2.1. Materials and Methods
2.2. Characterizations
2.2.1. Structural Analysis
2.2.2. Complex Impedance Spectroscopy (CIS)
3. Results
3.1. X-ray Diffraction Analysis
3.1.1. Qualitative XRD Analysis
3.1.2. Quantitative XRD Analysis
3.2. Impedance Spectrosopy
3.2.1. Electrical Conductivity
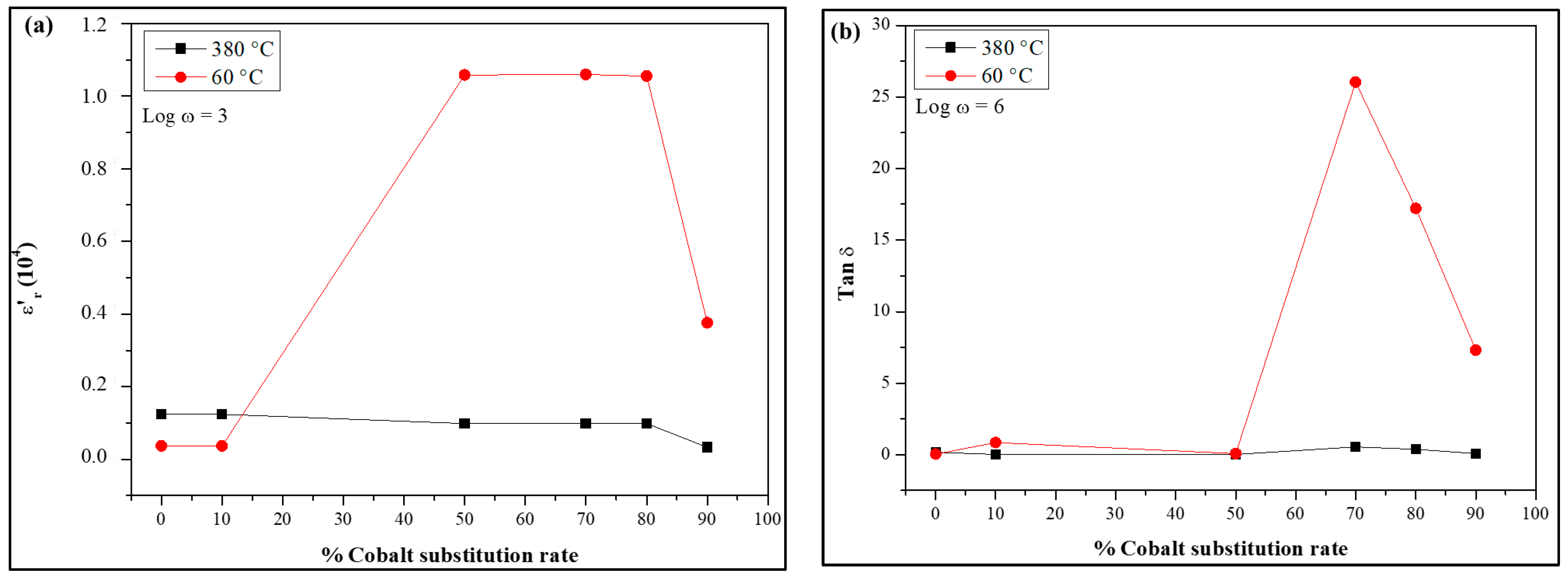
3.2.2. Dielectric Study
3.2.3. Modulus Mechanism
4. Discussion
4.1. Boundary between Doping and Cobalt Substitution within the BaTiO3 Matrix
4.2. Enhancing Electrical Properties as a Function of Cobalt Substitution Rate
5. Conclusions
- XRD analysis identified and confirmed the crystal phases in BCT nanocrystals, providing details on structural parameters.
- Electrical conductivity investigations revealed semiconducting behavior, emphasizing potential applications in electronics and solid-state technology, with conductivity increasing at higher temperatures and with greater cobalt concentrations.
- The dielectric constant (εr′) exhibited intriguing frequency-dependent behavior, decreasing with increasing frequency but stabilizing at higher frequencies. Elevated temperatures and cobalt substitution further enhanced εr′, making the material suitable for various technological applications.
- The loss angle demonstrated frequency-dependent behavior, gradually decreasing and stabilizing with frequency. Elevated temperatures and increased cobalt concentration reduced tan(δ), indicating minimal energy dissipation during energy storage and release processes.
- The electrical modulus real part (M′) and imaginary part (M″) showed insights into charge carrier mobility. At low frequencies, M′ remained low, highlighting other dominant conduction mechanisms. As frequency increased, M′ increased, signifying long-range charge carrier mobility. At higher frequencies, M′ stabilized, reflecting short-range mobility.
- Overall, cobalt substitution in BCT nanocrystals significantly improved their electrical and dielectric properties, showcasing their potential for cutting-edge energy storage applications.
Supplementary Materials
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Gupta, S.; Kumar, M. Computational Fluid Dynamics: Innovations in Numerical Techniques, Multi-Phase Flow Modeling, and Prospects for Sustainable Energy Applications. J. Sustain. Urban Futures 2023, 13, 1–20. [Google Scholar]
- Müller, C.; Ouyang, L.; Lund, A.; Moth-Poulsen, K.; Hamedi, M.M. From Single Molecules to Thin Film Electronics, Nanofibers, e-Textiles and Power Cables: Bridging Length Scales with Organic Semiconductors. Adv. Mater. 2019, 31, 1807286. [Google Scholar] [CrossRef] [PubMed]
- Janicke, M.; Jacob, K. A third industrial revolution. In Long-Term Governance for Social-Ecological Change; Routledge: Abingdon, UK, 2013; pp. 47–71. [Google Scholar]
- Misra, A.; Kozma, K.; Streb, C.; Nyman, M. Beyond charge balance: Counter-cations in polyoxometalate chemistry. Angew. Chem. Int. Ed. 2020, 59, 596–612. [Google Scholar] [CrossRef] [PubMed]
- Cox, P.A. Transition Metal Oxides: An Introduction to Their Electronic Structure and Properties; Oxford University Press: Oxford, UK, 2010; Volume 27. [Google Scholar]
- Nkele, A.C.; Ezugwu, S.; Suguyima, M.; Ezema, F.I. Structural and electronic properties of metal oxides and their applications in solar cells. In Chemically Deposited Nanocrystalline Metal Oxide Thin Films: Synthesis, Characterizations, and Applications; Springer International Publishing: Cham, Switzerland, 2021; pp. 147–163. [Google Scholar]
- Yuan, C.; Wu, H.B.; Xie, Y.; Lou, X.W. Mixed transition-metal oxides: Design, synthesis, and energy-related applications. Angew. Chem. Int. Ed. 2014, 53, 1488–1504. [Google Scholar] [CrossRef]
- Larcher, D.; Tarascon, J.M. Towards greener and more sustainable batteries for electrical energy storage. Nat. Chem. 2015, 7, 19–29. [Google Scholar] [CrossRef]
- Kanwade, A.; Shirage, P.M. A review on synergy of transition metal oxide nanostructured materials: Effective and coherent choice for supercapacitor electrodes. J. Energy Storage 2022, 55, 105692. [Google Scholar]
- Jebali, S.; Meftah, M.; Mejri, C.; Ben Haj Amara, A.; Oueslati, W. Enhancement of Photocatalytic Activity and Microstructural Growth of Cobalt-Substituted Ba1−xCoxTiO3 {x = 0,…, 1} Heterostructure. ChemEngineering 2023, 7, 43. [Google Scholar] [CrossRef]
- Butt, M.K.; Saleem, S.; Al-Harbi, F.F.; Atta, S.; Ishfaq, M.; Al Juman, F.S.; Yaseen, M. Optical and magnetic characteristics of BaTi1−xCoxO3: A first-principles study. Chalcogenide Lett. 2023, 20, 459–467. [Google Scholar] [CrossRef]
- Mohanty, H.S.; Puhan, T.S.; Padhi, S.; Panda, S.; Samantaray, S.S.; Rana, G.; Bhoi, K.; Sahu, U.K.; Mohapatra, S.; Raut, S.; et al. Insight of the Influence of Cobalt Substitution on the Structural, Microstructural, Optical and Electrical Properties of Tetragonal Barium Titanate Ceramics. ECS J. Solid State Sci. Technol. 2023, 12, 093001. [Google Scholar] [CrossRef]
- Padilla-Campos, L.; Diaz-Droguett, D.E.; Lavín, R.; Fuentes, S. Synthesis and structural analysis of Co-doped BaTiO3. J. Mol. Struct. 2015, 1099, 502–509. [Google Scholar] [CrossRef]
- Gupta, P.; Mahapatra, P.K.; Choudhary RN, P. Investigation on structural and electrical properties of Co and W modified BaTiO3. Ceram. Int. 2019, 45, 22862–22871. [Google Scholar] [CrossRef]
- Chu, B.J.; Chen, D.R.; Li, G.R.; Yin, Q.R. Electrical properties of Na1/2Bi1/2TiO3–BaTiO3 ceramics. J. Eur. Ceram. Soc. 2002, 22, 2115–2121. [Google Scholar] [CrossRef]
- Ghayour, H.; Abdellahi, M. A brief review of the effect of grain size variation on the electrical properties of BaTiO3-based ceramics. Powder Technol. 2016, 292, 84–93. [Google Scholar] [CrossRef]
- Morrison, F.D.; Sinclair, D.C.; West, A.R. Doping mechanisms and electrical properties of La-doped BaTiO3 ceramics. Int. J. Inorg. Mater. 2001, 3, 1205–1210. [Google Scholar] [CrossRef]
- Arshad, M.; Du, H.; Javed, M.S.; Maqsood, A.; Ashraf, I.; Hussain, S.; Ma, W.; Ran, H. Fabrication, structure, and frequency-dependent electrical and dielectric properties of Sr-doped BaTiO3 ceramics. Ceram. Int. 2020, 46, 2238–2246. [Google Scholar] [CrossRef]
- Slimani, Y.; Selmi, A.; Hannachi, E.; Almessiere, M.; AlFalah, G.; AlOusi, L.F.; Yasin, G.; Iqbal, M. Study on the addition of SiO2 nanowires to BaTiO3: Structure, morphology, electrical and dielectric properties. J. Phys. Chem. Solids 2021, 156, 110183. [Google Scholar] [CrossRef]
- Slimani, Y.; Shirsath, S.E.; Hannachi, E.; Almessiere, M.A.; Aouna, M.M.; Aldossary, N.E.; Yasin, G.; Baykal, A.; Ozçelik, B.; Ercan, I. (BaTiO3)1−x + (Co0.5Ni0.5Nb0.06Fe1.94O4)x nanocomposites: Structure, morphology, magnetic and dielectric properties. J. Am. Ceram. Soc. 2021, 104, 5648–5658. [Google Scholar] [CrossRef]
- Gargar, Z.; Tachafine, A.; Fasquelle, D.; Zegzouti, A.; Elaatmani, M.; Daoud, M. Grain size effects on dielectric properties of yttrium doped BaTiO3 ceramics. Phase Transit. 2023, 97, 338–349. [Google Scholar] [CrossRef]
- Hu, X.; Zhang, H.; Wu, D.; Yin, D.; Zhu, N.; Guo, K.; Lu, C. PVDF-based matrix with covalent bonded BaTiO3 nanowires enabled ultrahigh energy density and dielectric properties. Chem. Eng. J. 2023, 451, 138391. [Google Scholar] [CrossRef]
- Martínez-López, R.; Pérez-Labra, M.; Romero-Serrano, J.; Barrientos-Hernández, F.; Reyes-Pérez, M.; Valenzuela-Carrillo, M.; Urbano-Reyes, G.; Reyes-Cruz, V.; Dávila-Pulido, G. BaTiO3 solid solutions co-doped with Gd3+ and Eu3+: Synthesis, structural evolution and dielectric properties. J. Rare Earths 2023. [Google Scholar] [CrossRef]
- Sidaoui, S.; Ghoudi, A.; Oueslati, A.; Raduca, M.; Badea, M.; Andruh, M.; Nasr, S.; Naïli, H.; Rekik, W. Crystal structure, thermal behavior and electric properties of a new semiconductor cobalt-based hybrid material. J. Mol. Struct. 2023, 1294, 136394. [Google Scholar] [CrossRef]
- Fontaine, B. Optimisation des Propriétés de Céramiques Piézoélectriques sans Plomb du Type BCTZ par Modification de la Composition et par Dopage. Ph.D. Thesis, Université Polytechnique Hauts-de-France, Institut National des Sciences Appliquées Hauts-de-France, Valenciennes, France, 2022. [Google Scholar]
- Bottala-Gambetta, I. Elaboration et Caractérisation de Couches Ferroélectriques pour la Fabrication de Transistors FeFET. Ph.D. Thesis, Université Grenoble Alpes, Grenoble, France, 2023. [Google Scholar]
- Borderon, C.; Ginestar, S.; Renoud, R.; Gundel, H.; Muzzupapa, V.; Huitema, L.; Crunteanu, A. Couches Minces Ferroelectriques de Ba1−xSrxTiO3 Accordables pour une Antenne Patch Millimétrique Reconfigurable en Fréquence. 2023. Available online: https://hal.science/hal-04030162 (accessed on 19 June 2024).
- Chatta, W. Etude des Propriétés Diélectriques des Céramiques a Base de Titanate de Baryum BaTiO3. Ph.D. Thesis, Université de Laghouat-Amar Telidji, Laghouat, Algeria, 2013. [Google Scholar]
- Laasri, H.A. Étude et Elaboration de Matériaux Ferroélectriques sans Plomb pour le Stockage de l’Energie Electrique. Ph.D. Thesis, Université du Littoral Côte d’Opale, Dunkirk, France, Université Cadi Ayyad, Faculté des Sciences Semlalia, Marrakech, Morocco, 2018. [Google Scholar]
- Lahcen, P.B.; Said, P.B.; Mohamed, P.E.O.; Mustapha, P.H.; Amar, P.B.; Abdeslam, P.E.B.; Bouchaib, P.M. Verres et Vitrocéramiques Diélectriques Titano-Phosphatés pour le Stockage Electrostatique de l’Energie. Ph.D. Thesis, Univerisité Moulay Ismail, Meknes, Morocco, 2020. [Google Scholar]
- Lataoui, R.; Triki, A.; Hcini, S.; Oueslati, A.; Zemni, S.; Kanoun, O. Conduction mechanisms and complex impedance analysis in La0.6Sr0.4FeO3 ceramic. J. Electroceram. 2023, 50, 121–138. [Google Scholar] [CrossRef]
- Syaizwadi, S.M.; Ghazali, M.S.M.; Ikhmal, W.M.K.W.M.; Hazim, M.A.M.S.; Rafizah, W.A.W.; Kassim, S.; Lee, O.J.; Aini, I.N.Q.; Kamilah, S.N.; Zaid, M.H.M.; et al. Nonlinearity characteristics of low-voltage barium titanate based zinc oxide varistor ceramics modified by cobalt dopants. J. Integr. Circuits Syst. 2021, 16, 1–7. [Google Scholar] [CrossRef]
- Liu, P.; Ng, V.M.H.; Yao, Z.; Zhou, J.; Lei, Y.; Yang, Z.; Lv, H.; Kong, L.B. Facile synthesis and hierarchical assembly of flowerlike NiO structures with enhanced dielectric and microwave absorption properties. ACS Appl. Mater. Interfaces 2017, 9, 16404–16416. [Google Scholar] [CrossRef] [PubMed]
- Pinto, A.H.; Nogueira, A.E.; Dalmaschio, C.J.; Frigini, I.N.; de Almeida, J.C.; Ferrer, M.M.; Berengue, O.M.; Gonçalves, R.A.; de Mendonça, V.R. Doped Tin Dioxide (d-SnO2) and Its Nanostructures: Review of the Theoretical Aspects, Photocatalytic and Biomedical Applications. Solids 2022, 3, 327–360. [Google Scholar] [CrossRef]
- Joshi, M.J. Importance of impedance spectroscopy technique in materials characterization: A brief review. Mech. Mater. Sci. Eng. MMSE J. 2017, 9, hal-01504661. [Google Scholar]
- Patterson, A.L. The Scherrer formula for X-ray particle size determination. Phys. Rev. 1939, 56, 978. [Google Scholar] [CrossRef]
- Le, O.K.T.; Pham, A.T.T.; Pham, N.K.; Pham, T.H.C.; Nguyen, T.H.; Van Hoang, D.; Ta, H.K.T.; Truong, D.C.; Lai, H.T.; Ung, T.D.T.; et al. Compensation of Zn substitution and secondary phase controls effective mass and weighted mobility in In and Ga co-doped ZnO material. J. Mater. 2021, 7, 742–755. [Google Scholar]
- Phan, T.L.; Thanh, T.D.; Ho, T.A.; Manh, T.V.; Tran, Q.T.; Lampen, P.; Phan, M.H.; Yu, S.C. An Effective Route to Control the Magnetic-Phase Transition and Magnetocaloric Effect of La0.7Ca0.3MnO3 Nanoparticles. IEEE Trans. Magn. 2014, 50, 2302604. [Google Scholar] [CrossRef]
- Williamson, G.K.; Hall, W.H. X-ray line broadening from filed aluminium and wolfram. Acta Metall. 1953, 1, 22–31. [Google Scholar] [CrossRef]
- de Pauli, M.; Gomes, A.M.; Cavalcante, R.L.; Serpa, R.B.; Reis, C.P.; Reis, F.T.; Sartorelli, M.L. Capacitance spectra extracted from EIS by a model-free generalized phase element analysis. Electrochim. Acta 2019, 320, 134366. [Google Scholar] [CrossRef]
- Mane, S.M.; Pawar, S.A.; Patil, D.S.; Kulkarni, S.B.; Tayade, N.T.; Shin, J.C. Magnetoelectric, magnetodielectric effect and dielectric, magnetic properties of microwave-sintered lead-free x(Co0.9Ni0.1Fe2O4)-(1−x)[0.5(Ba0.7Ca0.3TiO3)-0.5(BaZr0.2Ti0.8O3)] particulate multiferroic composite. Ceram. Int. 2020, 46, 3311–3323. [Google Scholar]
- Bora, D.J.; Chowdary, V.A.; Chandana, P.; Chaithanya, P. Modeling and Simulation of Electrical Circuits to Determine Human Body Impedance. In Proceedings of the 2023 Fifth International Conference on Electrical, Computer and Communication Technologies (ICECCT), Erode, India, 22–24 February 2023; IEEE: Piscataway, NJ, USA, 2023; pp. 1–4. [Google Scholar]
- Sassi, M.; Bettaibi, A.; Oueslati, A.; Khirouni, K.; Gargouri, M.J.J.O.A. Electrical conduction mechanism and transport properties of LiCrP2O7 compound. J. Alloys Compd. 2015, 649, 642–648. [Google Scholar] [CrossRef]
- Cui, X.; Hu, T.; Wu, H.; Zhang, J.; Yang, L.; Zhong, X.; Wu, X.; Wang, J.; Li, X.; Yang, J.; et al. Charge Carrier Transport Behavior and Dielectric Properties of BaF2:Tb3+ Nanocrystals. Nanomaterials 2020, 10, 155. [Google Scholar] [CrossRef]
- Puente-Córdova, J.G.; Luna-Martínez, J.F.; Mohamed-Noriega, N.; Miranda-Valdez, I.Y. Electrical Conduction Mechanisms in Ethyl Cellulose Films under DC and AC Electric Fields. Polymers 2024, 16, 628. [Google Scholar] [CrossRef]
- Zhang, X.; Pu, Y.; Ning, Y.; Zhang, L.; Wang, B.; Chen, Z. Ultrahigh energy storage with superfast charge-discharge capability achieved in linear dielectric ceramic. J. Mater. Sci. Technol. 2024, 177, 59–67. [Google Scholar] [CrossRef]
- Shekhar, M.; Pradhan, L.K.; Kumar, L.; Kumar, P. Dielectric Relaxation Behavior and Conduction Mechanism of Ca and Ti Co-Doped Multiferroic Bismuth Ferrite. J. Electron. Mater. 2023, 52, 6182–6202. [Google Scholar] [CrossRef]
- Smith, C.S. Multiscale Characterization of Ferroelastic Deformation in Ceramic Materials. Ph.D. Thesis, University of Illinois at Urbana-Champaign, Champaign, IL, USA, 2020. [Google Scholar]
- Jebli, M.; Rayssi, C.; Dhahri, J.; Henda, M.B.; Belmabrouk, H.; Bajahzar, A. Structural and morphological studies, and temperature/frequency dependence of electrical conductivity of Ba0.97La0.02Ti1−xNb4x/5O3 perovskite ceramics. RSC Adv. 2021, 11, 23664–23678. [Google Scholar] [CrossRef] [PubMed]
- Husenkhan, D.B. Studies of Physical Properties of Low Melt Oxide Glasses. Ph.D. Thesis, Gulbarga University, Gulbarga, India, 2022. [Google Scholar]
- Barsoukov, E.; Macdonald, J.R. (Eds.) Impedance Spectroscopy: Theory, Experiment, and Applications; John Wiley & Sons: Hoboken, NJ, USA, 2018. [Google Scholar]
- Saha, S.; Chanda, S.; Dutta, A.; Sinha, T.P. Dielectric relaxation of PrFeO3 nanoparticles. Solid State Sci. 2016, 58, 55–63. [Google Scholar] [CrossRef]
- Singha, S.; Thomas, M.J.; Kulkarni, A. Complex permittivity characteristics of epoxy nanocomposites at low frequencies. IEEE Trans. Dielectr. Electr. Insul. 2010, 17, 1249–1258. [Google Scholar] [CrossRef]
- Fan, J.; Chen, Y.; Long, Z.; Tong, L.; He, G.; Hu, Z. Giant dielectric response and relaxation behavior in (Tm+ Ta) co-doped TiO2 ceramics. Phys. Chem. Chem. Phys. 2022, 24, 4759–4768. [Google Scholar] [CrossRef] [PubMed]
- Das, A.; Pamu, D. A comprehensive review on electrical properties of hydroxyapatite based ceramic composites. Mater. Sci. Eng. C 2019, 101, 539–563. [Google Scholar] [CrossRef] [PubMed]
- Bandaru, P.R. Electrical properties and applications of carbon nanotube structures. J. Nanosci. Nanotechnol. 2007, 7, 1239–1267. [Google Scholar] [CrossRef] [PubMed]
- Remya, K.P.; Prabhu, D.; Joseyphus, R.J.; Bose, A.C.; Viswanathan, C.; Ponpandian, N. Tailoring the morphology and size of perovskite BiFeO3 nanostructures for enhanced magnetic and electrical properties. Mater. Des. 2020, 192, 108694. [Google Scholar] [CrossRef]
- Kumar, S.; Supriya, S.; Kar, M. Enhancement of dielectric constant in polymer-ceramic nanocomposite for flexible electronics and energy storage applications. Compos. Sci. Technol. 2018, 157, 48–56. [Google Scholar] [CrossRef]
- Rogti, F.; Ferhat, M. Maxwell–Wagner polarization and interfacial charge at the multi-layers of thermoplastic polymers. J. Electrost. 2014, 72, 91–97. [Google Scholar] [CrossRef]
- Vu TT, N.; Teyssedre, G.; Le Roy, S.; Laurent, C. Maxwell–Wagner effect in multi-layered dielectrics: Interfacial charge measurement and modelling. Technologies 2017, 5, 27. [Google Scholar] [CrossRef]
- BinAhmed, S.; Hozalski, R.M.; Romero-Vargas Castrillón, S. Feed temperature effects on organic fouling of reverse osmosis membranes: Competition of interfacial and transport properties. ACS ES&T Eng. 2021, 1, 591–602. [Google Scholar]
- Hodge, I.M.; Ngai, K.L.; Moynihan, C.T. Comments on the electric modulus function. J. Non-Cryst. Solids 2005, 351, 104–115. [Google Scholar] [CrossRef]
- Lemziouka, H.; Nekkach, F.; Boutahar, A.; Moubah, R.; Omari, L.H.; Filali, M.; Rjeb, A.; Lassri, H.; Abid, M.; Hlil, E.K.; et al. Effect of Cobalt Doping on the Structural, Linear, and Nonlinear Optical Properties in Ba1−xCoxTiO3 Perovskites. J. Electron. Mater. 2023, 52, 3420–3430. [Google Scholar] [CrossRef]
- Tihtih, M.; Ibrahim JE, F.; Basyooni, M.A.; Kurovics, E.; Belaid, W.; Hussainova, I.; Kocserha, I. Role of A-site (Sr), B-site (Y), and A, B sites (Sr, Y) substitution in lead-free BaTiO3 ceramic compounds: Structural, optical, microstructure, mechanical, and thermal conductivity properties. Ceram. Int. 2023, 49, 1947–1959. [Google Scholar] [CrossRef]
- Ramay, S.M.; Kassim, H.; Mahmood, A.; Siddig, A.A.; Al Zayed, N.S. Tunable bandgap of bismuth-modified barium titanate by cobalt substitution for photovoltaic application. J. Mater. Sci. Mater. Electron. 2022, 33, 14343–14355. [Google Scholar] [CrossRef]
- Bujakiewicz-Koronska, R.; Gondek, Ł.; Vasylechko, L.; Balanda, M.; Juszynska-Galazka, E.; Galazka, M.; Majda, D.; Piekarczyk, W.; Zywczak, A.; Cizman, A.; et al. Magnetoelectric, spectroscopic, optical and elastic properties of Co-doped BaTiO3 ceramics. J. Alloys Compd. 2023, 946, 169344. [Google Scholar] [CrossRef]
- Niu, M.M.; Liu, J.; Sun, T.L.; Zu Jiang, R.; Hou, D.H.; Xu, D. Effect of transition metal element substitution on magnetoelectric properties of BiFeO3-BaTiO3 ceramics. J. Alloys Compd. 2021, 859, 158224. [Google Scholar] [CrossRef]
- Zhang, L.; Pu, Y.; Chen, M. Complex impedance spectroscopy for capacitive energy-storage ceramics: A review and prospects. Mater. Today Chem. 2023, 28, 101353. [Google Scholar] [CrossRef]
- Ku Muhsen KN, D.; Osman RA, M.; Idris, M.S.; Muhammad Nadzri, N.I.; Ruiz León, D.A. Structural Analysis and Dielectric Properties of Oxygen Non-Stoichiometry 5% Fe-Doped BaTiO3 Ceramic. Adv. Sci. Technol. 2022, 118, 47–52. [Google Scholar]
- Gent, W.E.; Busse, G.M.; House, K.Z. The predicted persistence of cobalt in lithium-ion batteries. Nat. Energy 2022, 7, 1132–1143. [Google Scholar] [CrossRef]
- Saadi, H.; Benzarti, Z.; Sanguino, P.; Pina, J.; Abdelmoula, N.; de Melo, J.S.S. Enhancing the electrical conductivity and the dielectric features of ZnO nanoparticles through Co doping effect for energy storage applications. J. Mater. Sci. Mater. Electron. 2023, 34, 116. [Google Scholar] [CrossRef]
- Cao, M.; Yan, X.J.; Li, L.; Wu, S.Y.; Chen, X.M. Obtaining Greatly Improved Dielectric Constant in BaTiO3–Epoxy Composites with Low Ceramic Volume Fraction by Enhancing the Connectivity of Ceramic Phase. ACS Appl. Mater. Interfaces 2022, 14, 7039–7051. [Google Scholar] [CrossRef]
- Akter, S.; Ahad, A.; Das, M.K.; Taher, M.A.; Barik, A.; Alam, F.; Khan, M.N.I. Study of structural, dielectric, and impedance properties of lead-free Zr and Y Co-doped BaTiO3. Mater. Chem. Phys. 2022, 275, 125241. [Google Scholar] [CrossRef]
- Kumari, A.; Singh, O.; Arya, E.; Chauhan, M.; Vermani, V.; Sanghi, S.; Agarwal, A. Crystal structure, dielectric and magnetic properties of (1−x)BaTiO3-(x) BaFe12O19 (x = 0.50, 0.60, 0.70) multiferroic composites. Appl. Phys. A 2023, 129, 334. [Google Scholar] [CrossRef]
- Sandeep, Y.; Rambabu, T.; Vinod, G.; Narayana, M.V.; Aravind, G.; Nathanial, V. Investigation of structural and dielectric properties of Gd3+ substituted nickel-zinc ferrite prepared via low-temperature citrate gel self-ignited process. Mater. Today Proc. 2022, 59, 1211–1220. [Google Scholar] [CrossRef]
- Samuvel, K.; Ramachandran, K.; Vinodkumar, R.; Kumaran, M.; Laksmishri, S. Equal-molar ratio of barium titanate doped with cobalt oxides, electrical parameter study of under high calcination of low milling hours. Int. J. Curr. Sci. 2023, 13, IJCSP23A1218. [Google Scholar]
- Dhahri, A.; Belkahla, A.; Laifi, J.; Gouadria, S.; Elhadi, M.; Dhahri, J.; Dhahri, E. Crystal structure and dielectric properties of the Ca/Y co-substituted BaTiO3. Inorg. Chem. Commun. 2022, 141, 109570. [Google Scholar] [CrossRef]
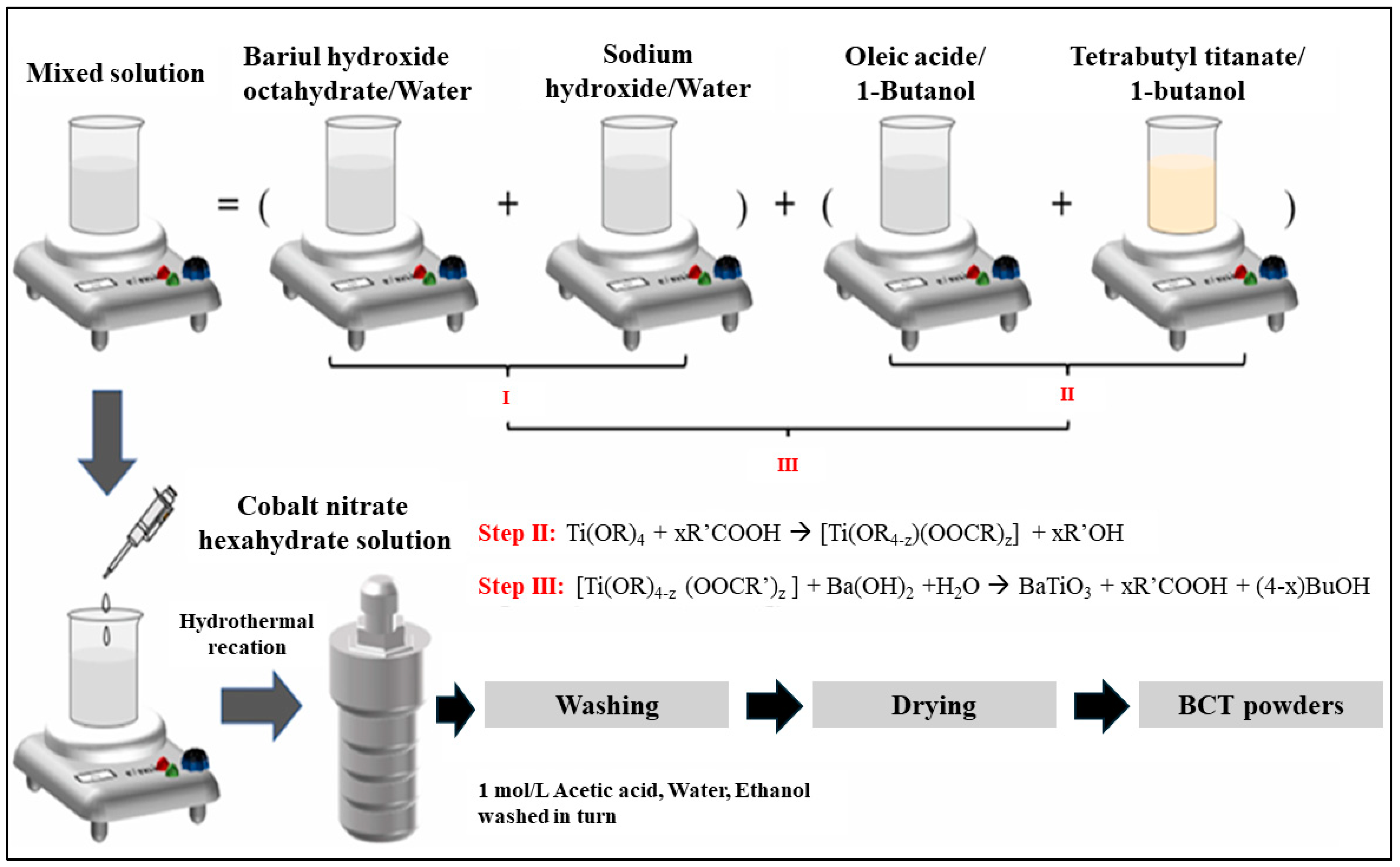
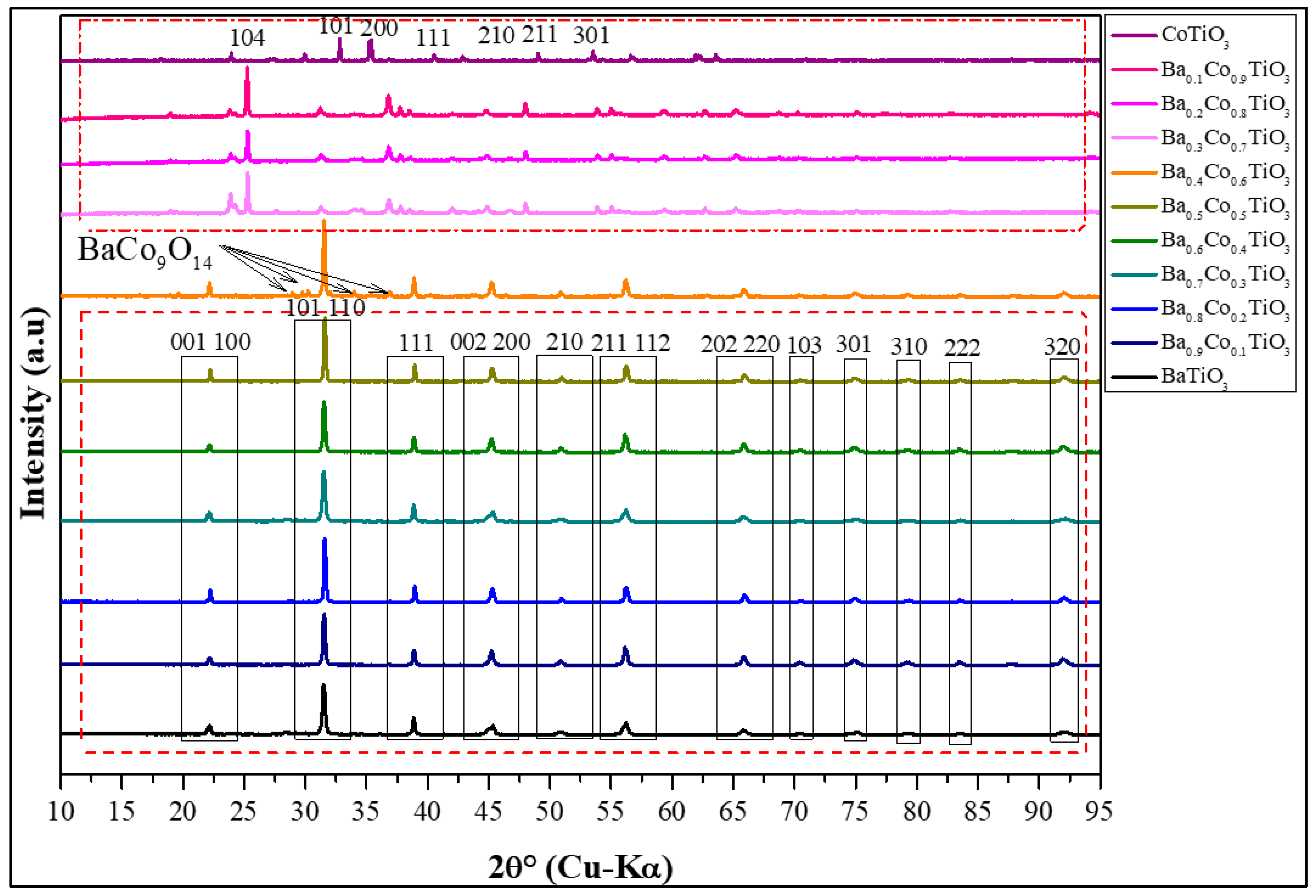
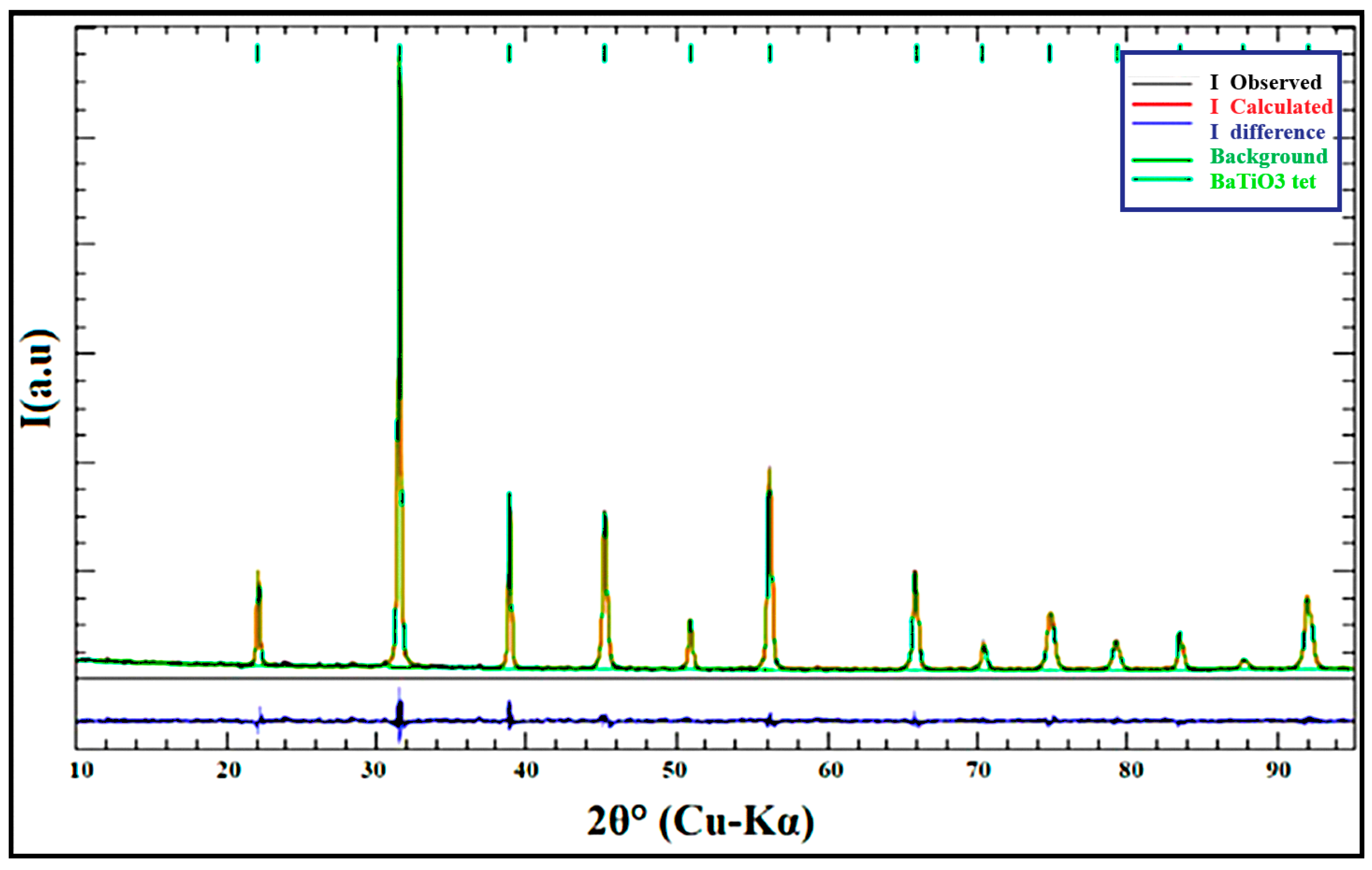




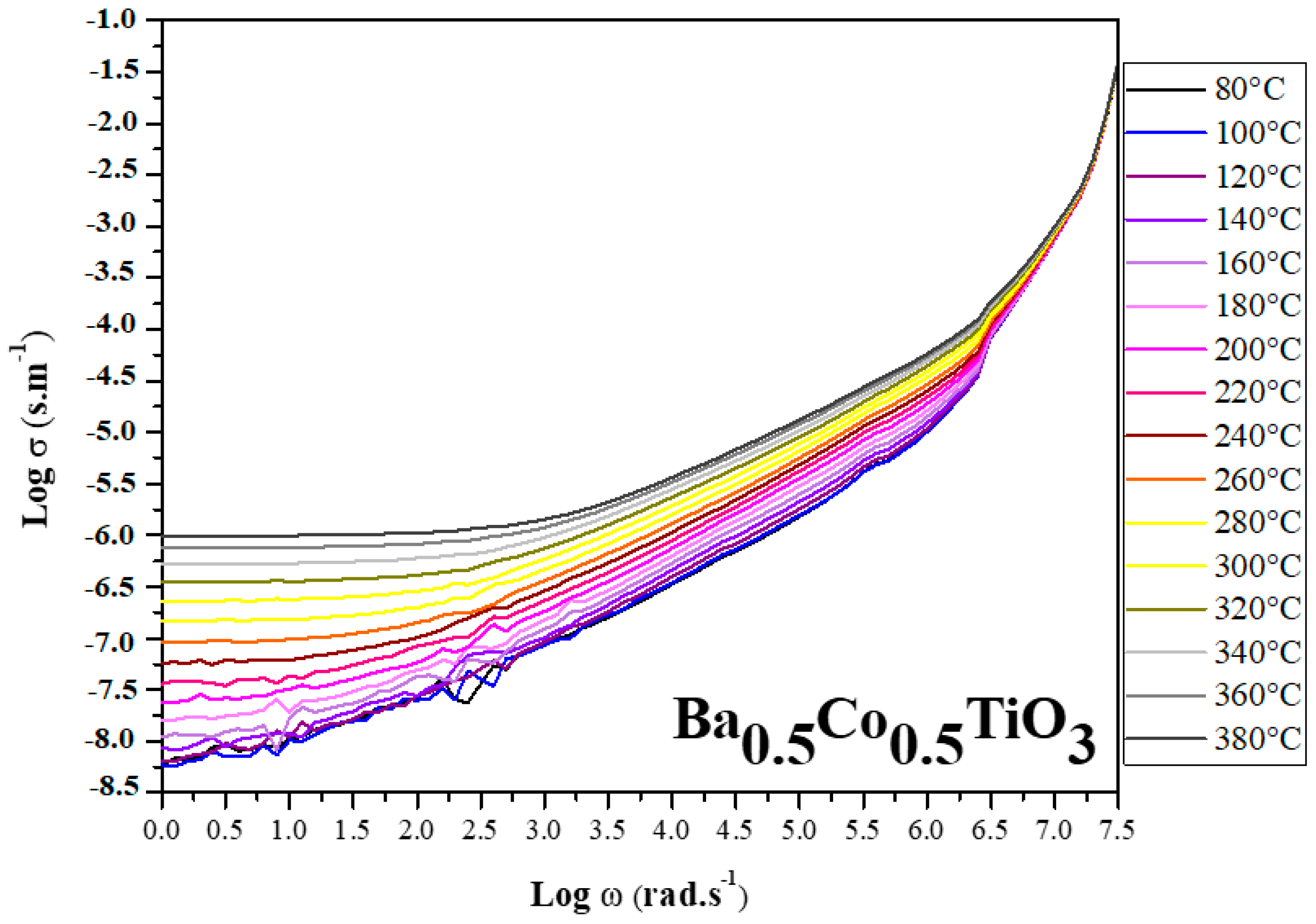





| Nanocrystals | Symmetries | Crystallographic Structures |
|---|---|---|
| BT | Tetragonal | Perovskite |
| BCT (x = 0.1) | Tetragonal | Perovskite |
| BCT (x = 0.2) | Tetragonal | Perovskite |
| BCT (x = 0.3) | Tetragonal | Perovskite |
| BCT (x = 0.4) | Tetragonal | Perovskite |
| BCT (x = 0.5) | Tetragonal | Perovskite |
| BCT (x = 0.6) | Tetragonal | Perovskite |
| BCT (x = 0.7) | Rhombohedral | Ilmenite |
| BCT (x = 0.8) | Rhombohedral | Ilmenite |
| BCT (x = 0.9) | Rhombohedral | Ilmenite |
| CT (x = 1) | Rhombohedral | Ilmenite |
| Nanocrystals | Crystallite Sizes (nm) |
|---|---|
| BT | 78.2 |
| BCT (x = 0.1) | 73.2 |
| BCT (x = 0.2) | 66.9 |
| BCT (x = 0.3) | 58.3 |
| BCT (x = 0.4) | 56.6 |
| BCT (x = 0.5) | 51.1 |
| BCT (x = 0.6) | 50.1 |
| BCT (x = 0.7) | 48.8 |
| BCT (x = 0.8) | 44.3 |
| BCT (x = 0.9) | 42.7 |
| CT (x = 1) | 39.1 |
| % Co | Unit Cell | Reliability Factors | Space Group | |||||||
|---|---|---|---|---|---|---|---|---|---|---|
| a (Å) | b (Å) | c (Å) | α (°) | β (°) | ɣ (°) | RP | RWP | GoF | ||
| 0 | 3.9886 | 3.9886 | 4.00060 | 90 | 90 | 90 | 5.57 | 06.99 | 1.25 | P4mm |
| 10 | 3.9980 | 3.9980 | 4.01800 | 5.62 | 11.11 | 1.97 | P4mm | |||
| 20 | 3.9980 | 3.9980 | 4.02200 | 5.29 | 14.72 | 2.78 | P4mm | |||
| 30 | 3.99000 | 3.9900 | 12.2114 | 120 | 6.17 | 14.11 | 1.73 | P63/mmc | ||
| 40 | 3.6983 | 3.6983 | 12.6023 | 5.28 | 07.04 | 1.66 | P63/mmc | |||
| 50 | 2.7240 | 2.7240 | 11.9640 | 5.70 | 10.87 | 1.32 | P63/mmc | |||
| 60 | 2.9301 | 2.9301 | 11.9980 | 2.83 | 09.98 | 3.52 | R-3 | |||
| 70 | 5.4860 | 5.4860 | 7.03200 | 1.78 | 04.74 | 2.66 | R-3 | |||
| 80 | 5.4860 | 5.4860 | 7.03200 | 1.78 | 05.29 | 2.97 | R-3 | |||
| 90 | 5.0760 | 5.0760 | 5.48600 | 54.83 | 54.83 | 54.83 | 1.53 | 04.87 | 3.18 | R-3 |
| 100 | 5.0760 | 5.0760 | 5.48600 | 54.83 | 54.83 | 54.83 | 1.78 | 04.74 | 2.66 | R-3 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jebali, S.; Mejri, C.; Albouchi, W.; Meftah, M.; Oueslati, A.; Oueslati, W. Uncovering the Possibilities of Ceramic Ba(1−x)CoxTiO3 Nanocrystals: Heightened Electrical and Dielectric Attributes. Solids 2024, 5, 460-484. https://doi.org/10.3390/solids5030031
Jebali S, Mejri C, Albouchi W, Meftah M, Oueslati A, Oueslati W. Uncovering the Possibilities of Ceramic Ba(1−x)CoxTiO3 Nanocrystals: Heightened Electrical and Dielectric Attributes. Solids. 2024; 5(3):460-484. https://doi.org/10.3390/solids5030031
Chicago/Turabian StyleJebali, Sana, Chadha Mejri, Wael Albouchi, Mahdi Meftah, Abderrazek Oueslati, and Walid Oueslati. 2024. "Uncovering the Possibilities of Ceramic Ba(1−x)CoxTiO3 Nanocrystals: Heightened Electrical and Dielectric Attributes" Solids 5, no. 3: 460-484. https://doi.org/10.3390/solids5030031
APA StyleJebali, S., Mejri, C., Albouchi, W., Meftah, M., Oueslati, A., & Oueslati, W. (2024). Uncovering the Possibilities of Ceramic Ba(1−x)CoxTiO3 Nanocrystals: Heightened Electrical and Dielectric Attributes. Solids, 5(3), 460-484. https://doi.org/10.3390/solids5030031







