A Methodology to Determine Undercutting Height in Block/Panel Caving
Abstract
1. Introduction
2. Methodology
Undercut Conceptual Model
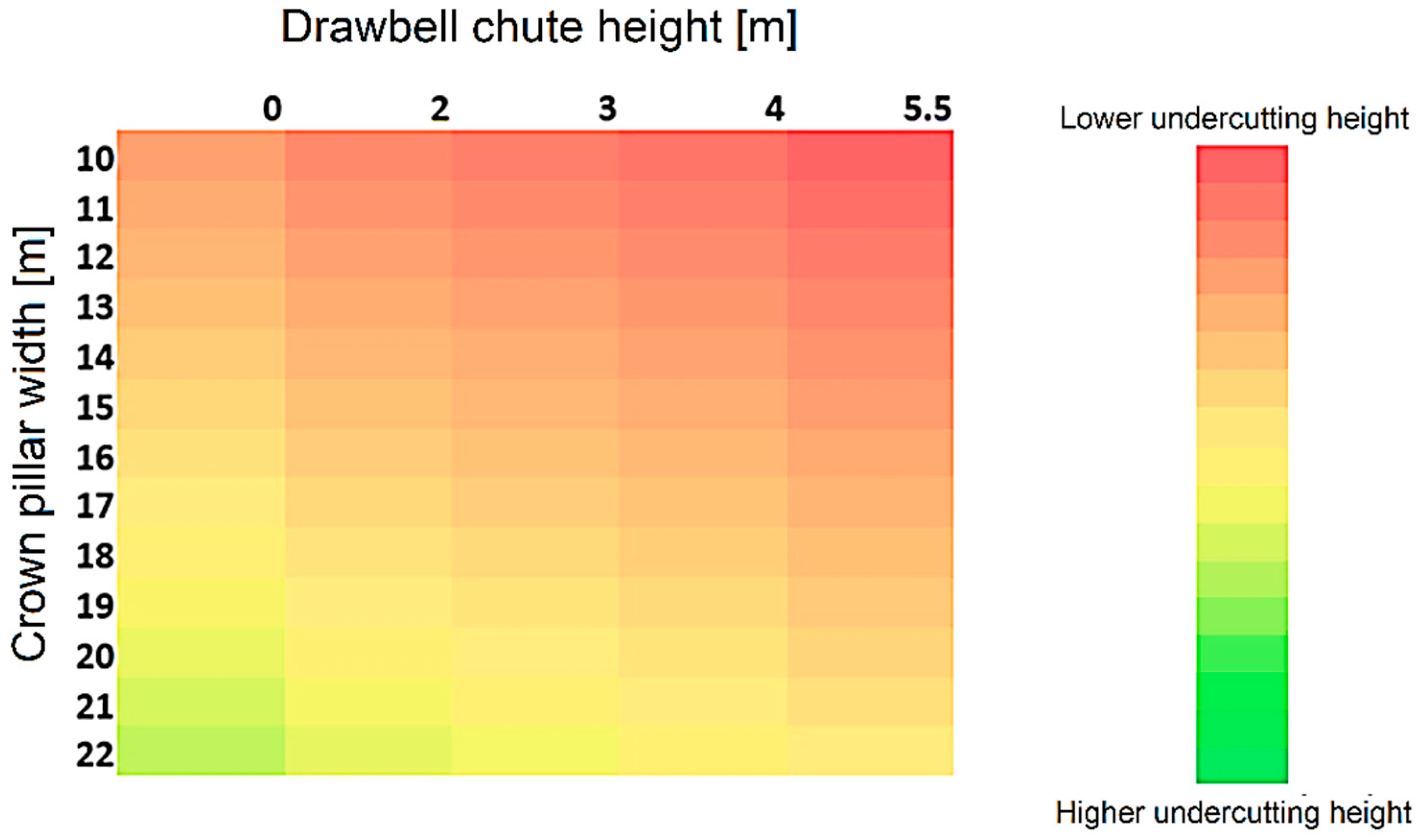
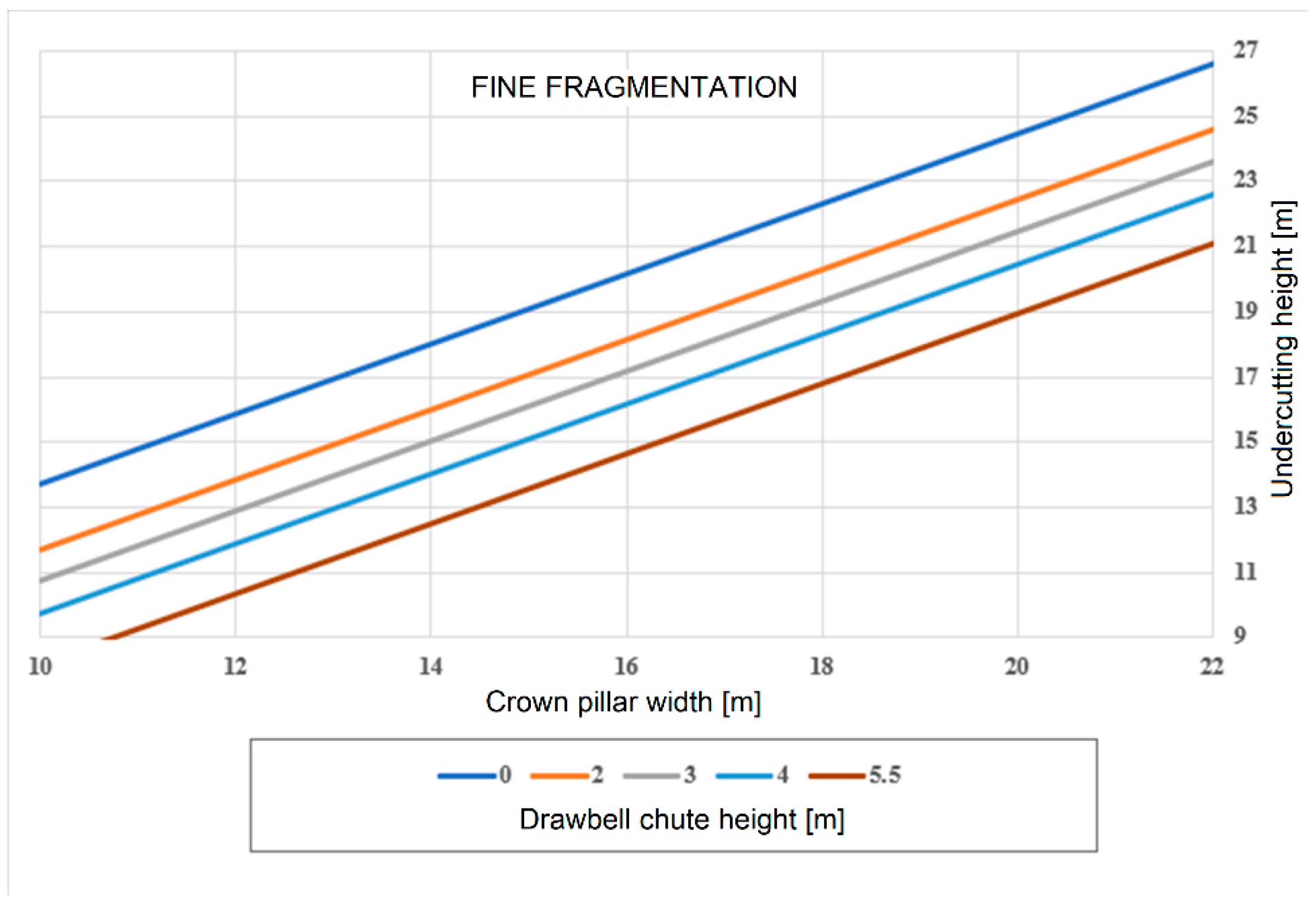
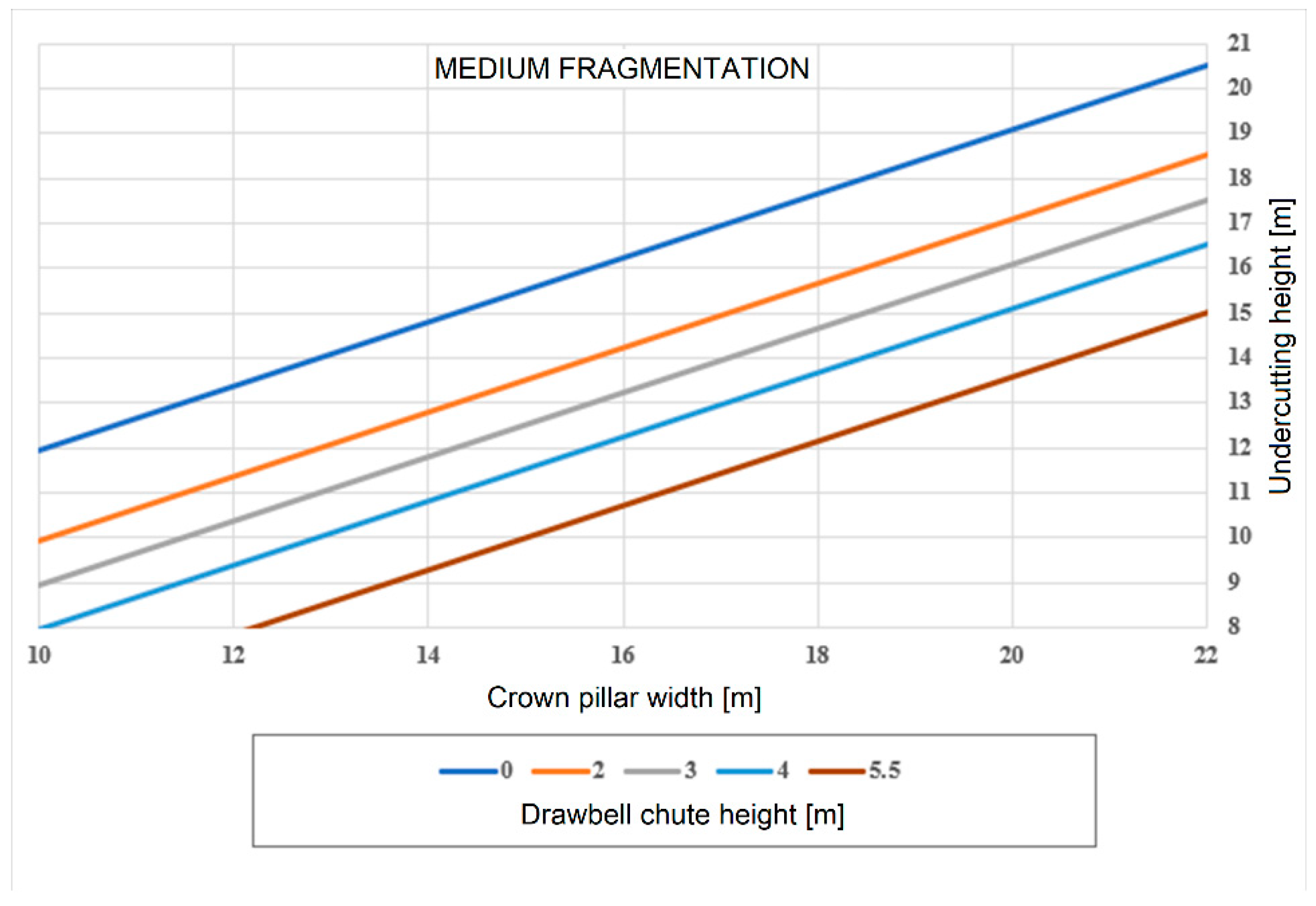
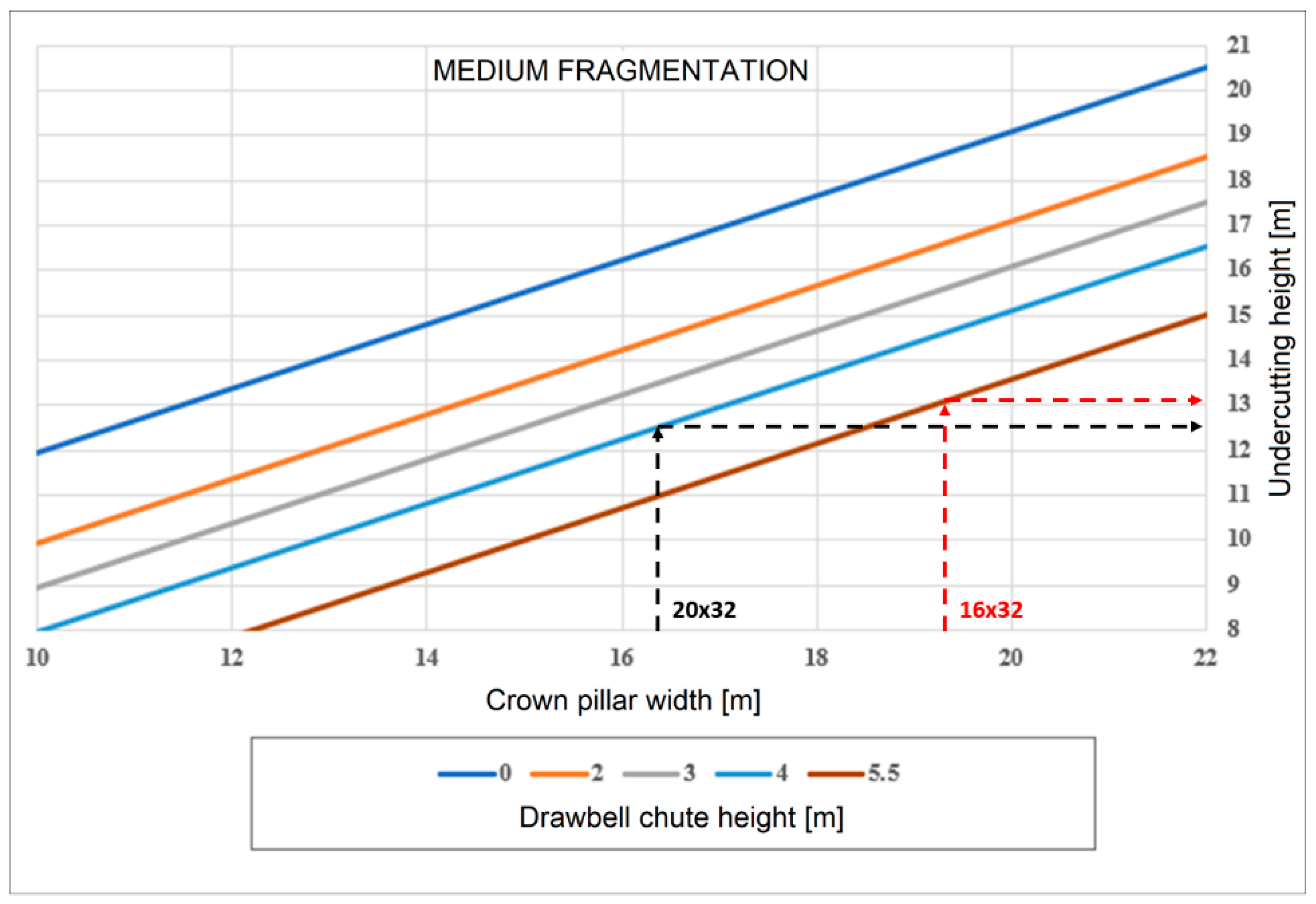
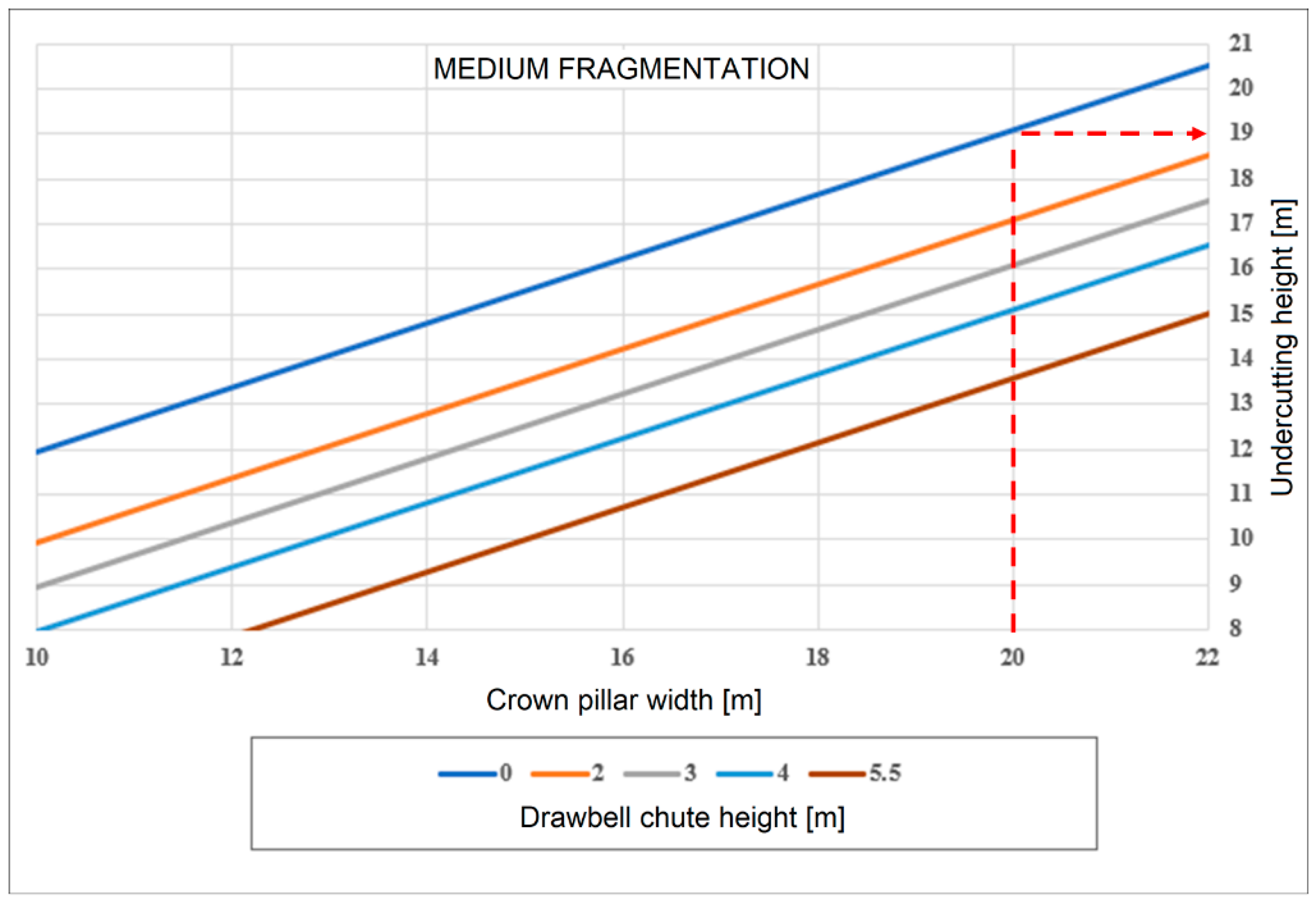
3. Undercutting Height Design Chart
4. Application
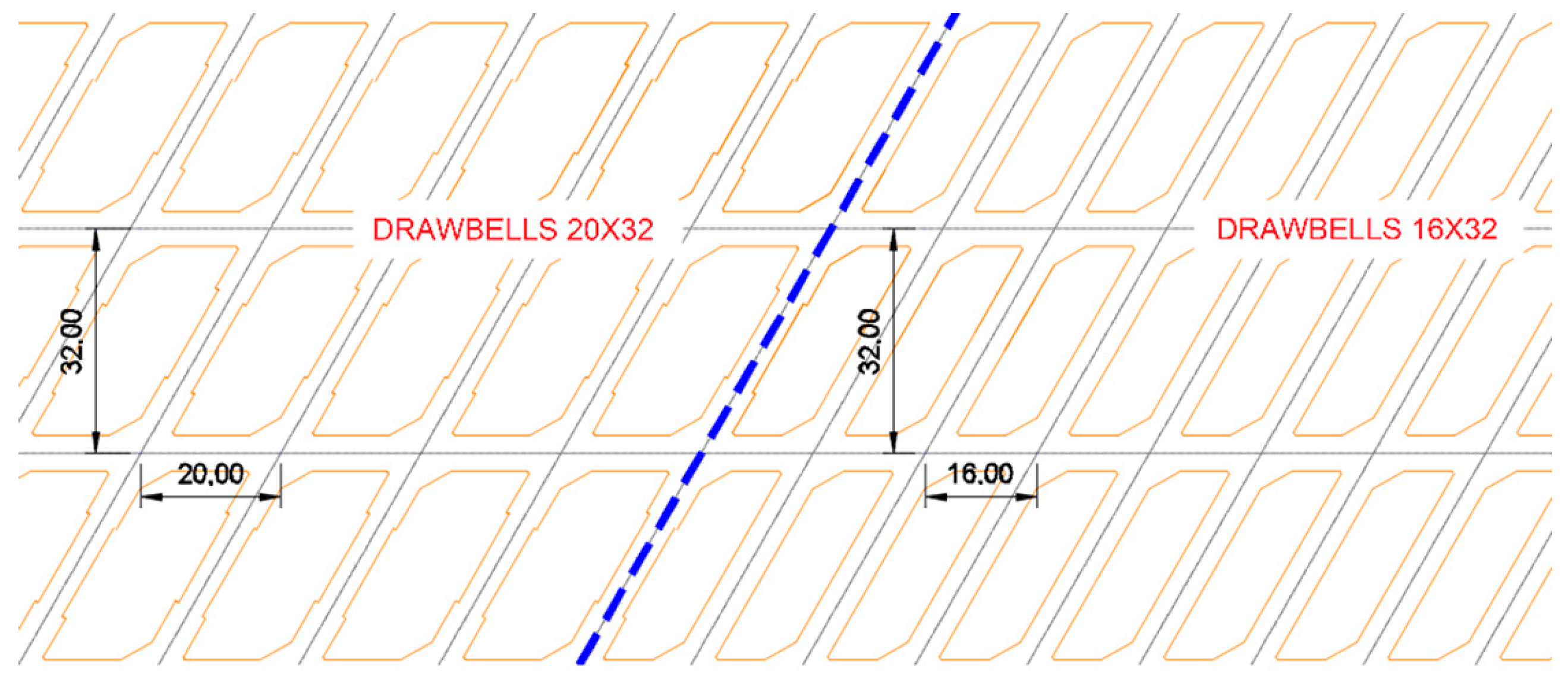
4.1. Example of Application 1
4.2. Example of Application 2
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Laubscher, D. Block Cave Manual; Julius Kruttschnitt Mineral Research Centre: Brisbane, Australia, 2000. [Google Scholar]
- Brown, E.T. Cave initation by undercutting. In Block Caving Geomechanics; Julius Kruttschnitt Mineral Research Centre: Brisbane, Australia, 2007; pp. 229–287. [Google Scholar]
- Trueman, R.; Pierce, M.; Wattimena, R. Quantifying stresses and support requirements in the undercut and production level drifts of block and panel caving mines. Int. J. Rock Mech. Min. Sci. 2002, 39, 617–632. [Google Scholar] [CrossRef]
- Fernandez, F.; Evans, P.; Gelson, R. Design and implementation of a damage assessment system at Argyle Diamond’s block cave project. In Proceedings of the Second International Symposium on Block and Sublevel Caving, Perth, Australia, 20–22 April 2010; pp. 65–81. [Google Scholar]
- Lett, J.L.; Brunton, I.; Capes, G.W.; Jager, A.; Mobilio, B.; Rachocki, J.; Sharrock, G.B.; Secheny, M. Undercutting to surface breakthrough—Cadia East panel cave (stage 1). In Proceedings of the Seventh International Conference & Exhibition on Mass Mining, Massmin 2016, Sydney, Australia, 9–11 May 2016; AusIMM: Sydney, Australia, 2016; pp. 65–82. [Google Scholar]
- Snyman, L.; Webster, S.; Samosir, J. E48 cave extension at Northparkes. In Proceedings of the Seventh International Conference & Exhibition on Mass Mining, Massmin 2016, Sydney, Australia, 9–11 May 2016; AusIMM: Sydney, Australia, 2016; pp. 111–117. [Google Scholar]
- Rojas, E.; Molina, R.; Bonani, A.; Constanzo, H. The pre-undercut caving method at the El Teniente mine, Codelco Chile. In Massmin 2000 Proceedings; The Australasian Institute of Mining and Metallurgy: Brisbane, Australia, 2000; pp. 261–266. [Google Scholar]
- Vasquez, P.; Díaz-Salas, J.; Barindelli, G. Lecciones aprendidas, colapsos MB N01S02, Chuquicamata Subterránea. In 1o Congreso Chileno Mecánica de Rocas; Universidad de Santiago de Chile: Santiago, Chile, 2023; pp. 940–947. [Google Scholar]
- Gómez, R.; Labbé, E. An option for the change to massive underground mining: The current Chilean methodology of block caving. Bol. Geol. Min. 2019, 130, 181–198. [Google Scholar] [CrossRef]
- Pardo, C.; Rojas, E. Selection of the explotation method based on the experience of hydraulic fracture techniques at the El Teniente mine. In Proceedings of the Seventh International Conference & Exhibition on Mass Mining, Massmin 2016, Sydney, Australia, 9–11 May 2016; AusIMM: Sydney, Australia, 2016; pp. 97–104. [Google Scholar]
- Navarrete, R.; Soto, C.; Henriquez, F.; Godoy, H. Hydraulic fracturing in the construction of Andes Norte project. In Caving 2022, Proceedings of the Fifth International Conference on Block and Sublevel Caving, Adelaide, Australia, 30 August–1 September 2022; Potvin, Y., Ed.; Australian Centre for Geomechanics: Adelaide, Australia, 2022; pp. 1227–1240. [Google Scholar]
- Paredes, P.; Lopa, I.; Kline, P.; Sykes, M. Undercutless caving at Newcrest: Towards the next generation of cave mining. In Proceedings of the Eighth International Conference & Exhibition on Mass Mining, Massmin 2020, Santiago, Chile, 9–11 December 2020; Castro, R., Baez, F., Suzuki, K., Eds.; ACG: Santiago, Chile, 2020; pp. 166–181. [Google Scholar] [CrossRef]
- Rivera, N.; Music, A.; Quiroz, R.; Díaz, M.; Vergara, C. Undercutting confined blasting in Esmeralda and Dacita case study at El Teniente. In UMining 2016, 1er Congreso Internacional en Minería Subterránea; Castro, R., Valencia, M.E., Eds.; Universidad Chile: Santiago, Chile, 2016; pp. 403–424. [Google Scholar]
- Butcher, R.J. Block Cave Undercutting—Aims, Strategies, Methods and Management. In Massmin 2000 Proceedings; AusIMM: Brisbane, Australia, 2000; pp. 423–428. [Google Scholar]
- Henriquez, J.P. Evaluación de la Altura de Socavación en la Productividad de la Minería de Block/Panel Caving; University of Chile: Santiago, Chile, 2018. [Google Scholar]
- Hormazabal, E.; Alvarez, R.; Russo, A.; Acevedo, D. Influence of the undercut height on the behaviour of pillars at the extraction level in block and panel caving operations. In Caving 2018; Potvin, Y., Jakubec, J., Eds.; Australian Centre for Geomechanics: Vancouber, BC, Canada, 2018; pp. 351–362. [Google Scholar]
- Galilea, B. Estimación de la Tasa de Incorporación de Área Socavada en Block Caving Mediante Técnicas de Simulación; University of Chile: Santiago, Chile, 2017. [Google Scholar]
- Fernandez, R. Impacto del Cambio de Diámetro de la Perforación de Socavación de División Andina; Codelco Chile, University of Chile: Santiago, Chile, 2020. [Google Scholar]
- Brunton, I.; Sharrock, G.; Lett, G. Full Scale Near Field Flow Behaviour at the Ridgeway Deeps Block Cave Mine. In Proceedings of the 6th International Conference & Exhibition on Mass Mining, Massmin 2012, Sudbury, ON, Canada, 10–14 June 2012; Canadian Institute of Mining, Metallurgy and Petroleum: Sudbury, MA, USA, 2012. [Google Scholar]
- Flores, G.; Karzulovic, A. Benchmarking Report; International Caving Study Stage 2 (ICS-II): Brisbane, Australia, 2002. [Google Scholar]
- Laubscher, D.H. Cave mining-the state of the art. J. S. Afr. Inst. Min. Metall. 1994, 94, 279–293. [Google Scholar]
- Laubscher, D.H. Cavability, in A Practical Manual on Block Caving; JKMRC and Itasca Consulting Group, Inc.: Brisbane, Australia, 2000; Volume 27. [Google Scholar]
- Castro, R.; Gómez, R.; Hekmat, A. Experimental quantification of hang-up for block caving applications. Int. J. Rock Mech. Min. Sci. 2016, 85, 1–9. [Google Scholar] [CrossRef]
- Castro, R.; López, S.; Gómez, R.; Ortiz, S.; Carreño, N. Experimental Study of the Influence of Drawbell Geometry on Hang-Ups in Cave Mine Applications. Rock Mech. Rock Eng. 2020, 54, 1–10. [Google Scholar] [CrossRef]
- Gómez, R.; Sanhueza, V.; Fustos, R.; Nelis, G. A new approach for hang-ups prediction in block caving mines based on drawbell geometry and vertical stress. Min. Metall. Explor. 2022, 39, 2393–2400. [Google Scholar] [CrossRef]
- Castro, R.L.; Vargas, R.; De La Huerta, F. Determination of drawpoint spacing in panel caving: A case study at the el teniente mine. J. S. Afr. Inst. Min. Metall. 2012, 112, 871–876. [Google Scholar]





| Undercut Shape | Advantages | Disadvantages |
|---|---|---|
| High undercutting | - High productivity at the beginning of caving due to fine fragmentation and few hang-ups [15]. - Large undercutting heights (greater than 10 m) reduce induced stress on the floor of the undercut level, favoring the propagation of caving [16]. | - The deflection of drill holes and misfires, which can result in poor blasting and require re-drilling [17]. - More drilling required and therefore higher drilling and blasting costs [15]. - Narrower flow ellipsoids due to both finer fragmentation and greater flow angle, which increase the height at which interactive flow occurs and, therefore, result in a greater amount of remaining ore [15]. |
| Narrow-horizontal undercutting | - Higher opening rates due to less drilling and blasting time [17,18]. - Lower costs of drilling and blasting [17]. | - A risk of remnant pillars due to failure to achieve a complete break because of remaining shots, deviation of drill holes, or confined blasting [15]. - The presence of coarse fragmentation at the beginning of the caving process [2]. |
| Narrow-inclined undercutting | - The ore flow of blasted material moves more easily through the inclined section. Complete breakage of the rock on the major pillar is ensured [2]. | - The presence of coarse fragmentation at the beginning of the caving process [2]. - Stability problems in the interaction between horizontal and inclined drill holes [2]. - Possible disturbances in the ore flow and generation of hang-ups [19]. |
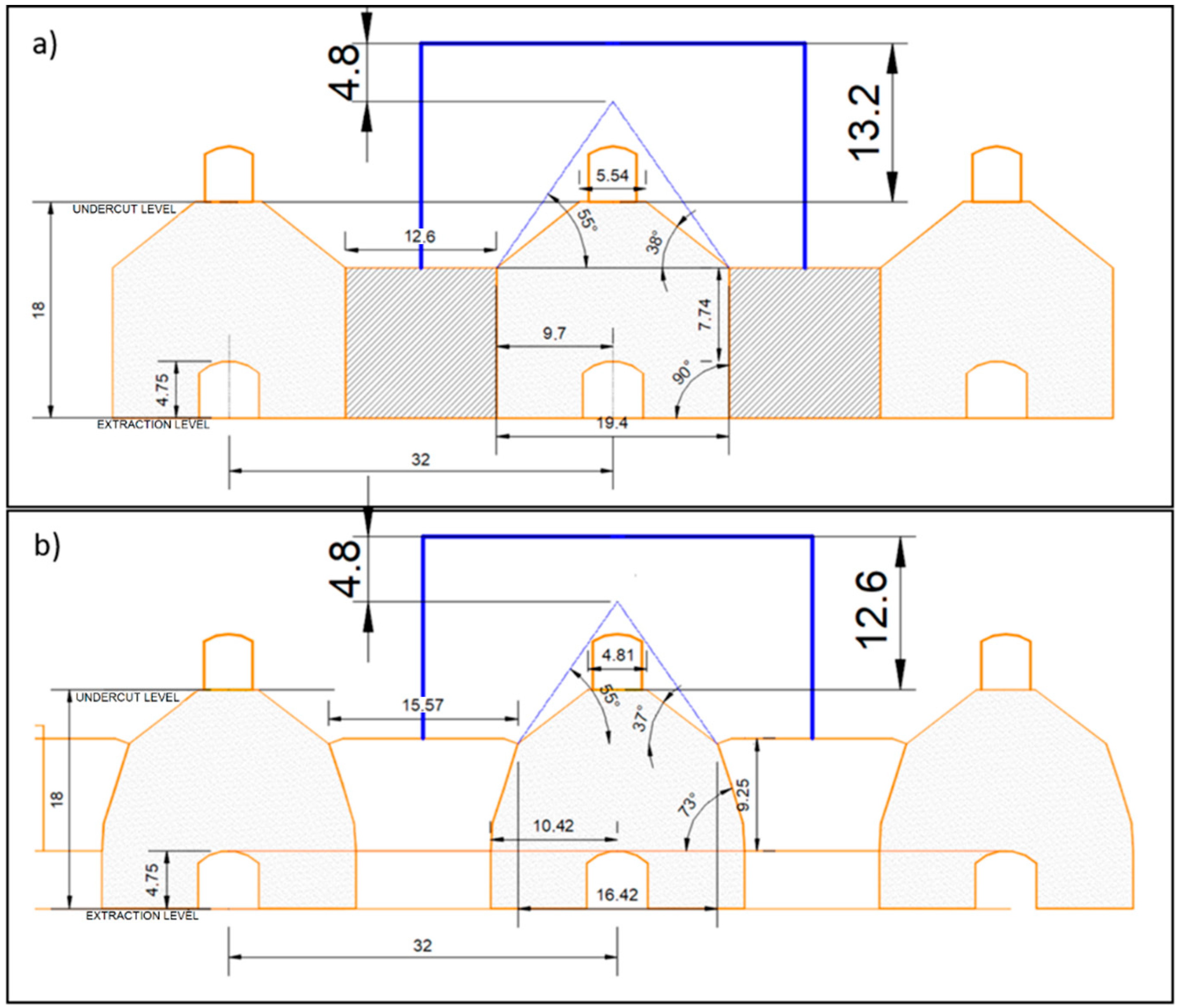
| Parameters | Symbol | Layout 16 × 32 | Layout 20 × 32 |
|---|---|---|---|
| Distance between drifts [m] | 32 | 32 | |
| Drawbell length (upper) [m] | 12.6 | 15.57 | |
| Undercut apex [m] | 5.5 | 4.81 | |
| Distance drift/brow [m] | 9.7 | 10.42 | |
| Angle of brow [°] | 90 | 73 | |
| Angle of drawbell wall [°] | 38 | 37 | |
| Distance between production and undercut levels [m] | 18 | 18 | |
| Production drift height [m] | 4.75 | 4.75 | |
| Height of brow [m] | 7.7 | 9.25 |
| Design Parameters | Symbol | Layout 16 × 32 | Layout 20 × 32 |
|---|---|---|---|
| Crown pillar width [m] | 19.4 | 16.43 | |
| Height of drawbell chute [m] | 5.5 | 4.0 | |
| Undercutting height [m] | 13.2 | 12.6 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Morales, D.; Castro, R.; Gómez, R. A Methodology to Determine Undercutting Height in Block/Panel Caving. Mining 2024, 4, 417-428. https://doi.org/10.3390/mining4020024
Morales D, Castro R, Gómez R. A Methodology to Determine Undercutting Height in Block/Panel Caving. Mining. 2024; 4(2):417-428. https://doi.org/10.3390/mining4020024
Chicago/Turabian StyleMorales, Danko, Raúl Castro, and René Gómez. 2024. "A Methodology to Determine Undercutting Height in Block/Panel Caving" Mining 4, no. 2: 417-428. https://doi.org/10.3390/mining4020024
APA StyleMorales, D., Castro, R., & Gómez, R. (2024). A Methodology to Determine Undercutting Height in Block/Panel Caving. Mining, 4(2), 417-428. https://doi.org/10.3390/mining4020024







