Development and Validation of a Practical Model for Transient Biofilter Performance
Abstract
1. Introduction
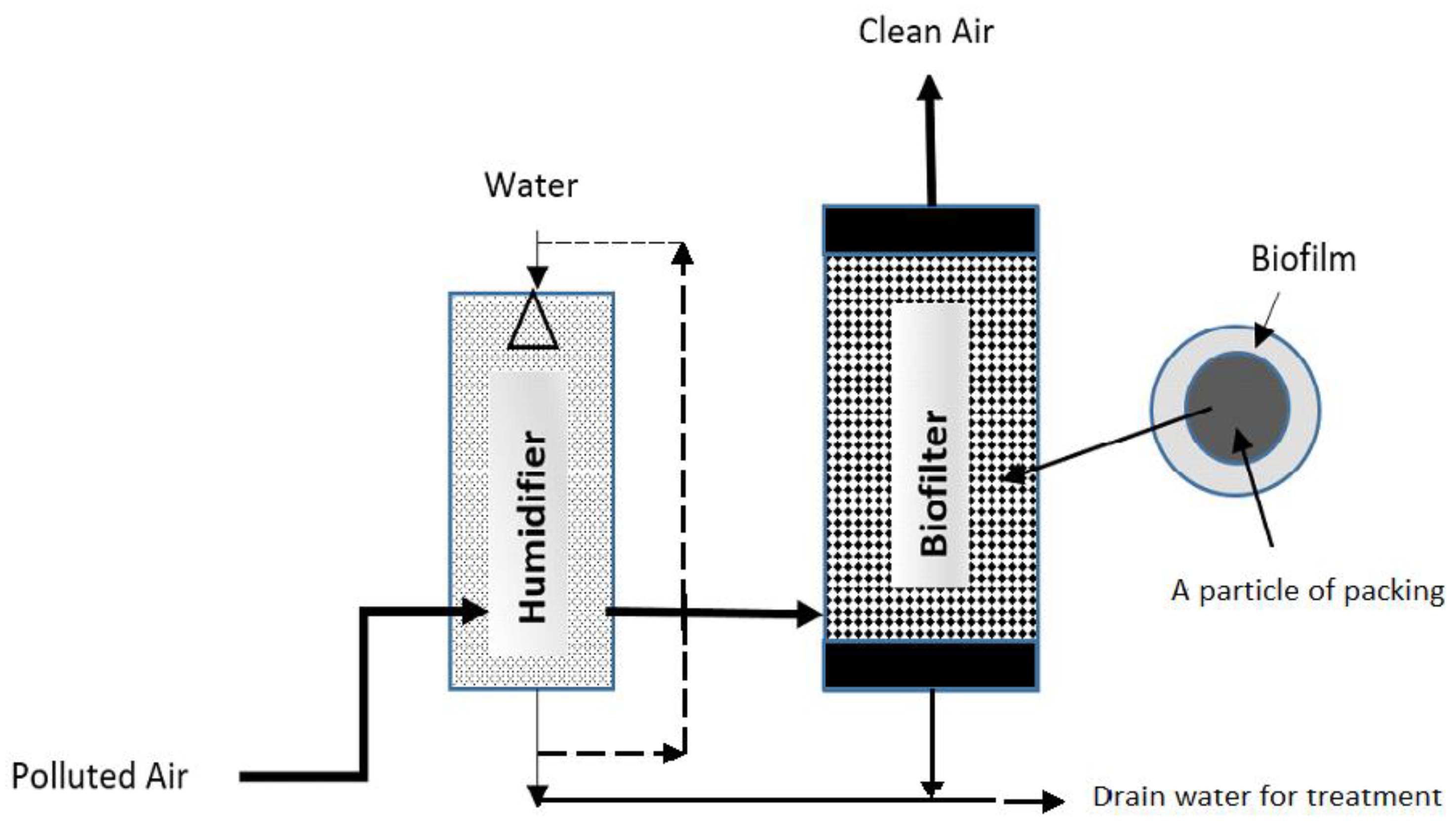
2. Theory and Model Development
2.1. Model Development
2.2. Model Parameters
2.3. Comments on the Model Parameters
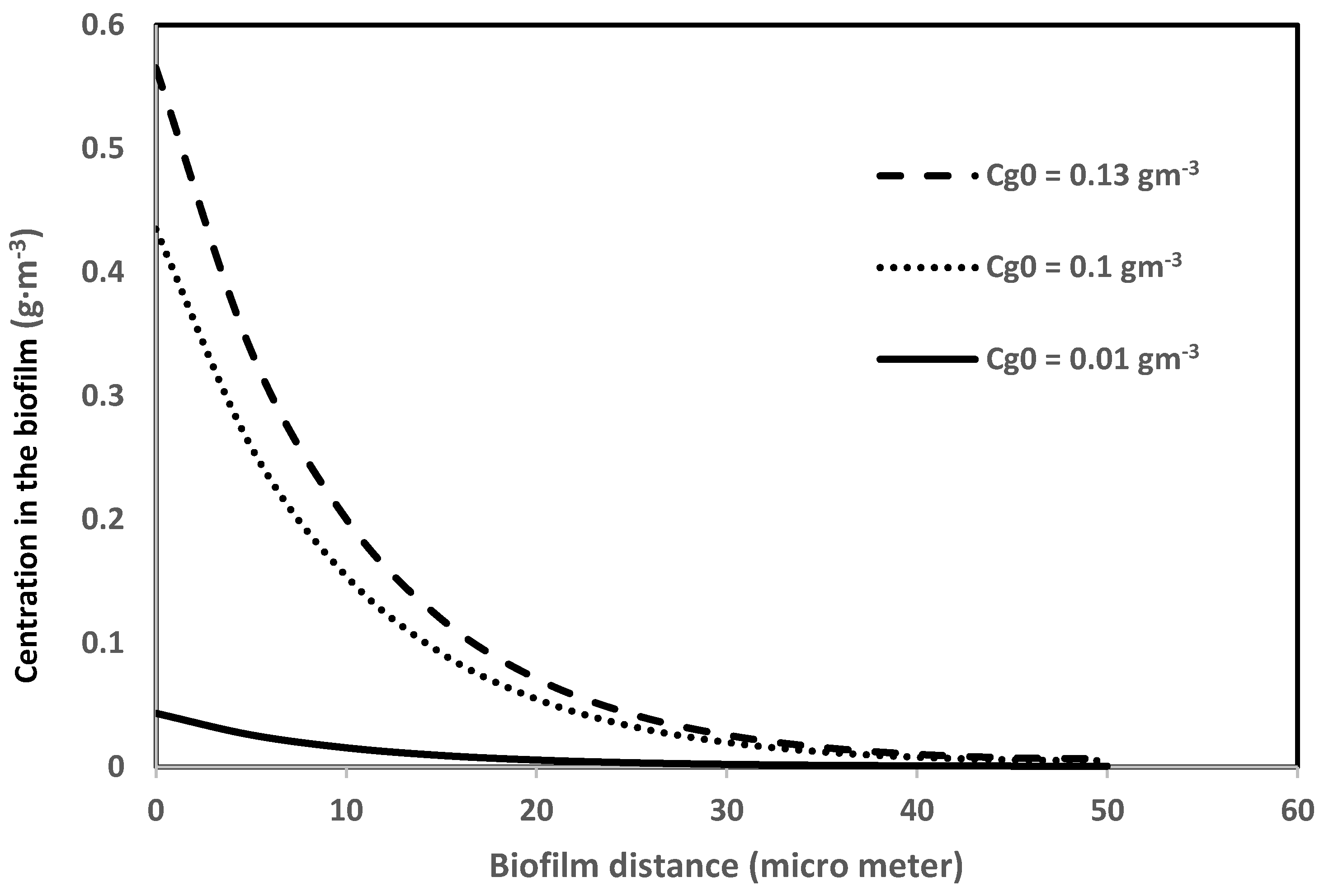
3. Results and Discussion
4. Conclusions
Funding
Acknowledgments
Conflicts of Interest
References
- Ottengraf, S.P.P.; Van Den Oever, A.H.C. Kinetics of organic compound removal from waste gases with a biological filter. Biotechnol. Bioeng. 1983, 25, 3089–3102. [Google Scholar] [CrossRef]
- Shareefdeen, Z.; Baltzis, B.C.; Oh, Y.S.; Bartha, R. Biofiltration of methanol vapor. Biotechnol. Bioeng. 1993, 41, 512–524. [Google Scholar] [CrossRef] [PubMed]
- Shareefdeen, Z.; Baltzis, B.C. Biofiltration of toluene vapor under steady state and transient conditions: Theory ad experimental results. Chem. Eng. Sci. 1994, 49, 4347–4360. [Google Scholar] [CrossRef]
- Zarook, S.M.; Shaikh, A.A.; Ansar, Z. Development, experimental validation, and dynamic analysis of a general transient biofilter model. Chem. Eng. Sci. 1994, 52, 759–773. [Google Scholar] [CrossRef]
- Zarook, S.; Shaikh, A.; Ansar, Z.; Baltzis, B. Biofiltration of volatile organic compound (VOC) mixtures under transient conditions. Chem. Eng. Sci. 1997, 52, 4135–4142. [Google Scholar] [CrossRef]
- Baltzis, B.C.; Wojdyla, S.M.; Zarook, S.M. Modeling Biofiltration of VOC Mixtures under Steady-State Conditions. J. Environ. Eng. 1997, 123, 599–605. [Google Scholar] [CrossRef]
- Shareefdeen, Z.; Shaikh, A.A. Analysis and comparison of biofilter models. Chem. Eng. J. 1997, 65, 55–61. [Google Scholar]
- Zarook, S.; Shaikh, A.; Azam, S. Axial dispersion in biofilters. Biochem. Eng. J. 1998, 1, 77–84. [Google Scholar] [CrossRef]
- Elmrini, H.; Bredin, N.; Shareefdeen, Z.; Heitz, M. Biofiltration of xylene emissions: Bioreactor response to variations in the pollutant inlet concentration and gas flow rate. Chem. Eng. J. 2004, 100, 149–158. [Google Scholar] [CrossRef]
- Malakar, S.; Saha, P.D.; Baskaran, D.; Rajamanickam, R. Microbial biofilter for toluene removal: Performance evaluation, transient operation and theoretical prediction of elimination capacity. Sustain. Environ. Res. 2018, 28, 121–127. [Google Scholar] [CrossRef]
- Salih, M.; Shareefdeen, Z.; Khouri, S. A novel approach to the solution of a steady state biofilter model. Environ. Eng. Res. 2019, 25, 779–787. [Google Scholar] [CrossRef]
- Rajamanickam, R.; Baskaran, D.; Kaliyamoorthi, K.; Baskaran, V.; Krishnan, J. Steady State, transient behavior and kinetic modeling of benzene removal in an aerobic biofilter. J. Environ. Chem. Eng. 2020, 8, 103657. [Google Scholar] [CrossRef]
- Jawad, J.; Khalil, M.J.; Sengar, A.K.; Zaidi, S.J. Experimental analysis and modeling of the methane degradation in a three stage biofilter using composted sawdust as packing media. J. Environ. Manag. 2021, 286, 112214. [Google Scholar] [CrossRef] [PubMed]
- Azlah, N.; Shareefdeen, Z.; Elkamel, A. Dispersion of volatile organic compounds (VOCs) emissions from a biofilter at an electronic manufacturing facility. Environ. Prog. Sustain. Energy 2017, 36, 1100–1107. [Google Scholar] [CrossRef]
- Shareefdeen, Z. Industrial Biofilter Case Studies. In From Biofiltration to Featured Options in Gaseous Fluxes Biotreatment: Recent Developments, New Trends, Advances, and Opportunities; Soreanu, G., Dumont, E., Eds.; Elsevier: Amsterdam, The Netherlands, 2020; ISBN 9780128190647. [Google Scholar] [CrossRef]
- Shareefdeen, Z. High-Performance Biofilters for Air Treatment Applications. In From Biofiltration to Featured Options in Gaseous Fluxes Biotreatment: Recent Developments, New Trends, Advances, and Opportunities; Soreanu, G., Dumont, E., Eds.; Elsevier: Amsterdam, The Netherlands, 2020; ISBN 9780128190647. [Google Scholar] [CrossRef]
- Ceder, N. The Quick Python Book, 3rd ed.; Manning: Shelter Island, NY, USA, 2018; ISBN 9781617294037. [Google Scholar]



| Parameter | Description | Values | Units | Remarks |
|---|---|---|---|---|
| (A) The parameters in this section are compound-dependent | ||||
| H′ | Dimensionless Henry’s constant | 0.23 | -- | Specific to benzene |
| µm | Specific growth rate (in Monod expression) | 0.68 | h−1 | “ |
| K | Kinetic constant (in Monod expression) | 12.22 | g.m−3 | “ |
| Y | Yield coefficient | 0.708 | -- | “ |
| Dw | Diffusivity of benzene in water | 1.04 × 10−9 | m2.s−1 | “ |
| De | Diffusivity of benzene in biofilm | * | m2.s−1 | * Calculated using the values Dw, Xv, and Equation (2). |
| (B) The parameters in this section are related to the biofilm and biofilter | ||||
| Xv | Biofilm density | 100 | Kg.m−3 | Specific to biofilm |
| As | Biofilm surface area | 40 | m−1 | “ |
| ẟ | Biofilm thickness | 5.0 × 10−5 | m | “ |
| (C) The parameters in this section are related to the biofilter operation | ||||
| Cg0 | Inlet concentration to the biofilter | 0.13 | g.m−3 | Specific to biofilter operation |
| H | Height of the biofilter | 1.5 | m | |
| ∆h | A section of a biofilter (if n = 10) | 0.15 | m | |
| ug | Superficial velocity | 0.025 | m.s−1 | |
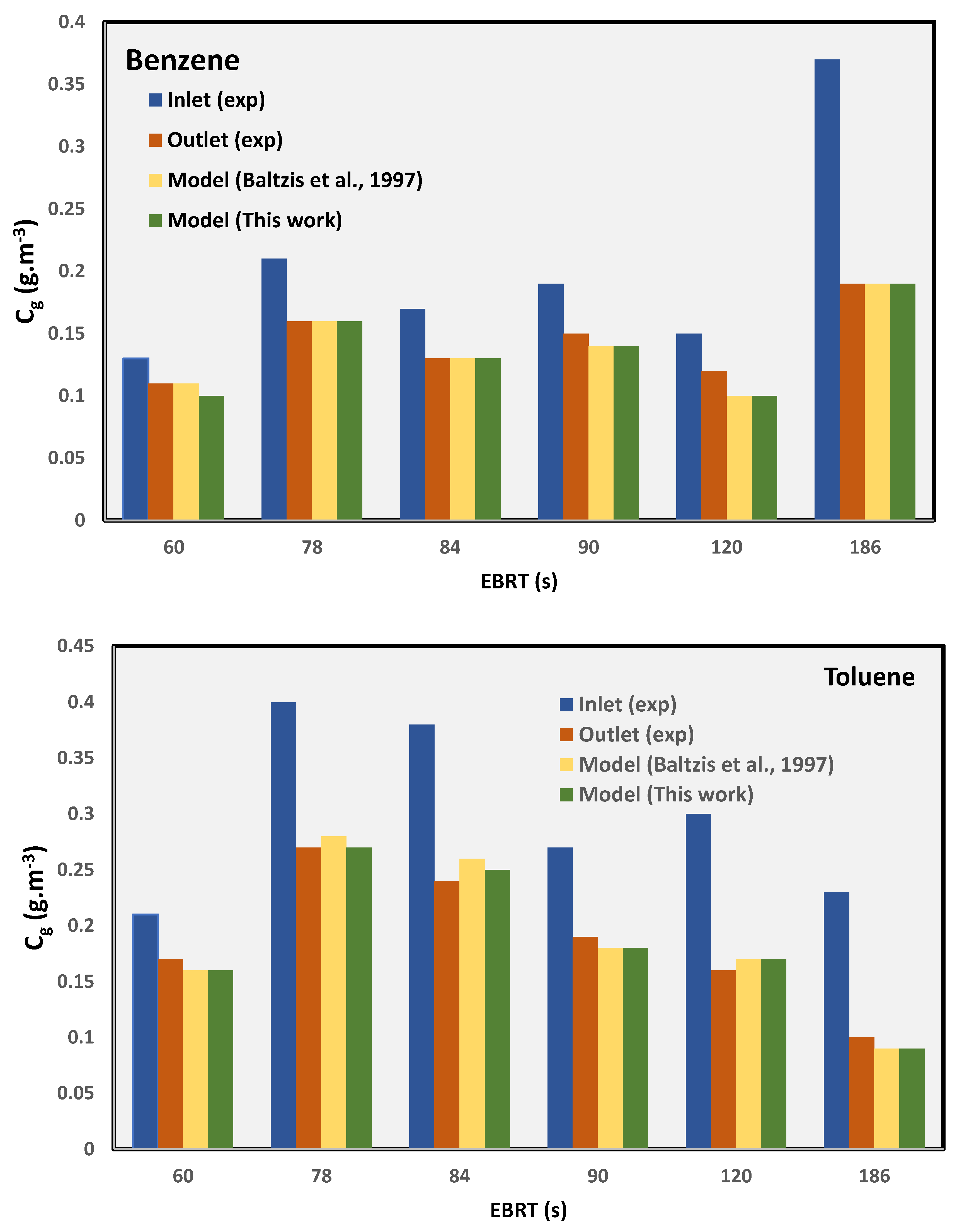
| EBRT (s) | Inlet Cgo (g·m−3) | Outlet Cge (g·m−3) Experimental | Outlet Cge (g·m−3) Baltzis et al. [6] | Error (%) Compared to Exp. | Outlet Cge (g·m−3) This Work | Error (%) Compared to Exp. |
|---|---|---|---|---|---|---|
| Benzene | ||||||
| 60 | 0.13 | 0.11 | 0.11 | 0 | 0.1 | −9.1 |
| 78 | 0.21 | 0.16 | 0.16 | 0 | 0.16 | 0 |
| 84 | 0.17 | 0.13 | 0.13 | 0 | 0.13 | 0 |
| 90 | 0.19 | 0.15 | 0.14 | −6.2 | 0.14 | −6.2 |
| 120 | 0.15 | 0.12 | 0.1 | −16.7 | 0.1 | −16.7 |
| 186 | 0.37 | 0.19 | 0.19 | 0 | 0.19 | 0 |
| Toluene | ||||||
| 60 | 0.21 | 0.17 | 0.16 | −5.9 | 0.16 | −5.9 |
| 78 | 0.4 | 0.27 | 0.28 | 3.7 | 0.27 | 0 |
| 84 | 0.38 | 0.24 | 0.26 | 8.3 | 0.25 | 4.2 |
| 90 | 0.27 | 0.19 | 0.18 | −5.3 | 0.18 | −5.3 |
| 120 | 0.3 | 0.16 | 0.17 | 6.2 | 0.17 | 6.2 |
| 186 | 0.23 | 0.1 | 0.09 | −10 | 0.09 | −10 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Shareefdeen, Z. Development and Validation of a Practical Model for Transient Biofilter Performance. BioTech 2022, 11, 51. https://doi.org/10.3390/biotech11040051
Shareefdeen Z. Development and Validation of a Practical Model for Transient Biofilter Performance. BioTech. 2022; 11(4):51. https://doi.org/10.3390/biotech11040051
Chicago/Turabian StyleShareefdeen, Zarook. 2022. "Development and Validation of a Practical Model for Transient Biofilter Performance" BioTech 11, no. 4: 51. https://doi.org/10.3390/biotech11040051
APA StyleShareefdeen, Z. (2022). Development and Validation of a Practical Model for Transient Biofilter Performance. BioTech, 11(4), 51. https://doi.org/10.3390/biotech11040051







