Numerical Simulation of Non-Darcy Flow in Naturally Fractured Tight Gas Reservoirs for Enhanced Gas Recovery
Abstract
1. Introduction
1.1. Brief Review: Shale and Tight Gas Reservoirs
1.2. Carbon Sequestration
2. Non-Darcy Flow
3. Two-Component Single-Phase Flow Model
4. Finite Volume Formulation
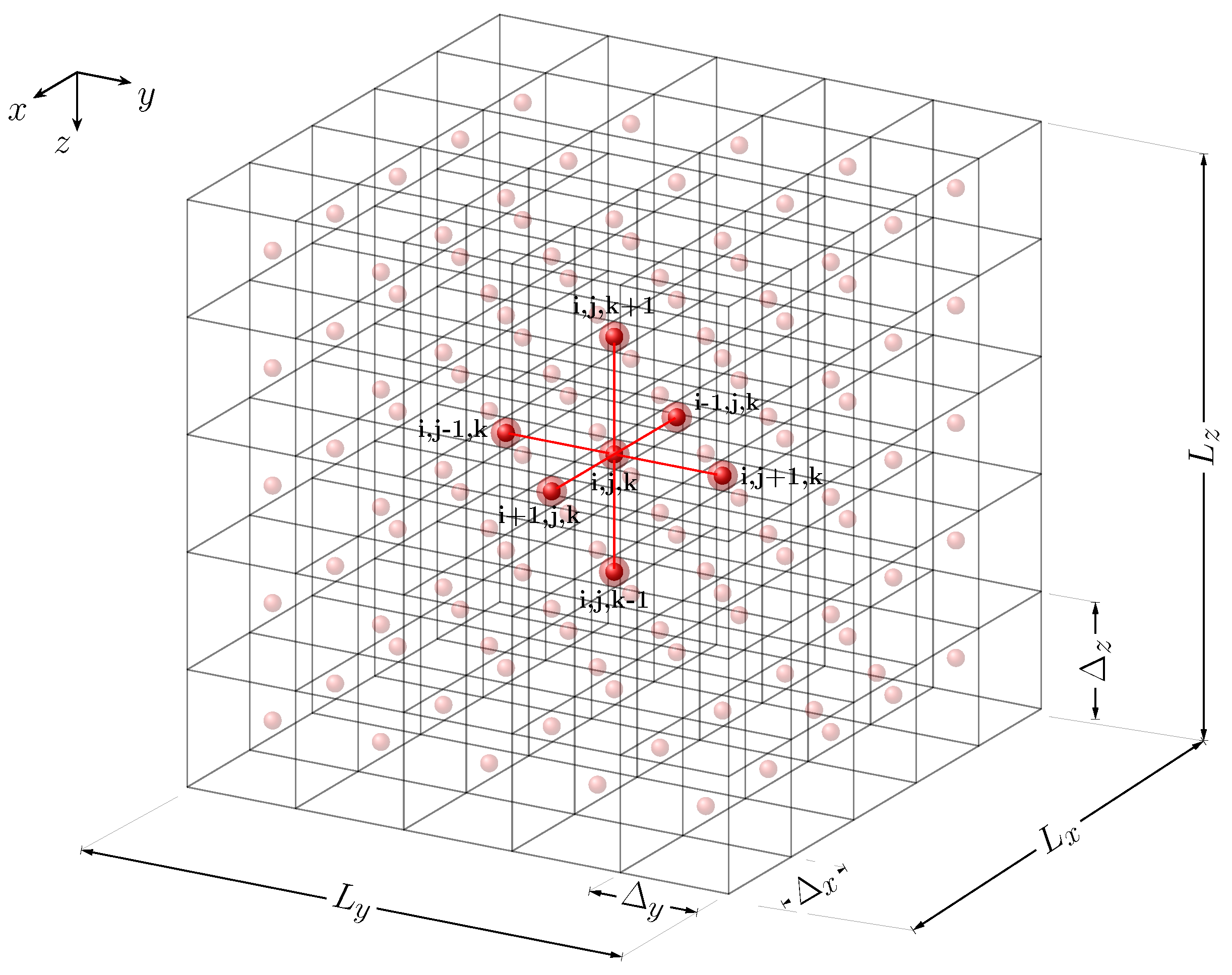
5. Material and Methods
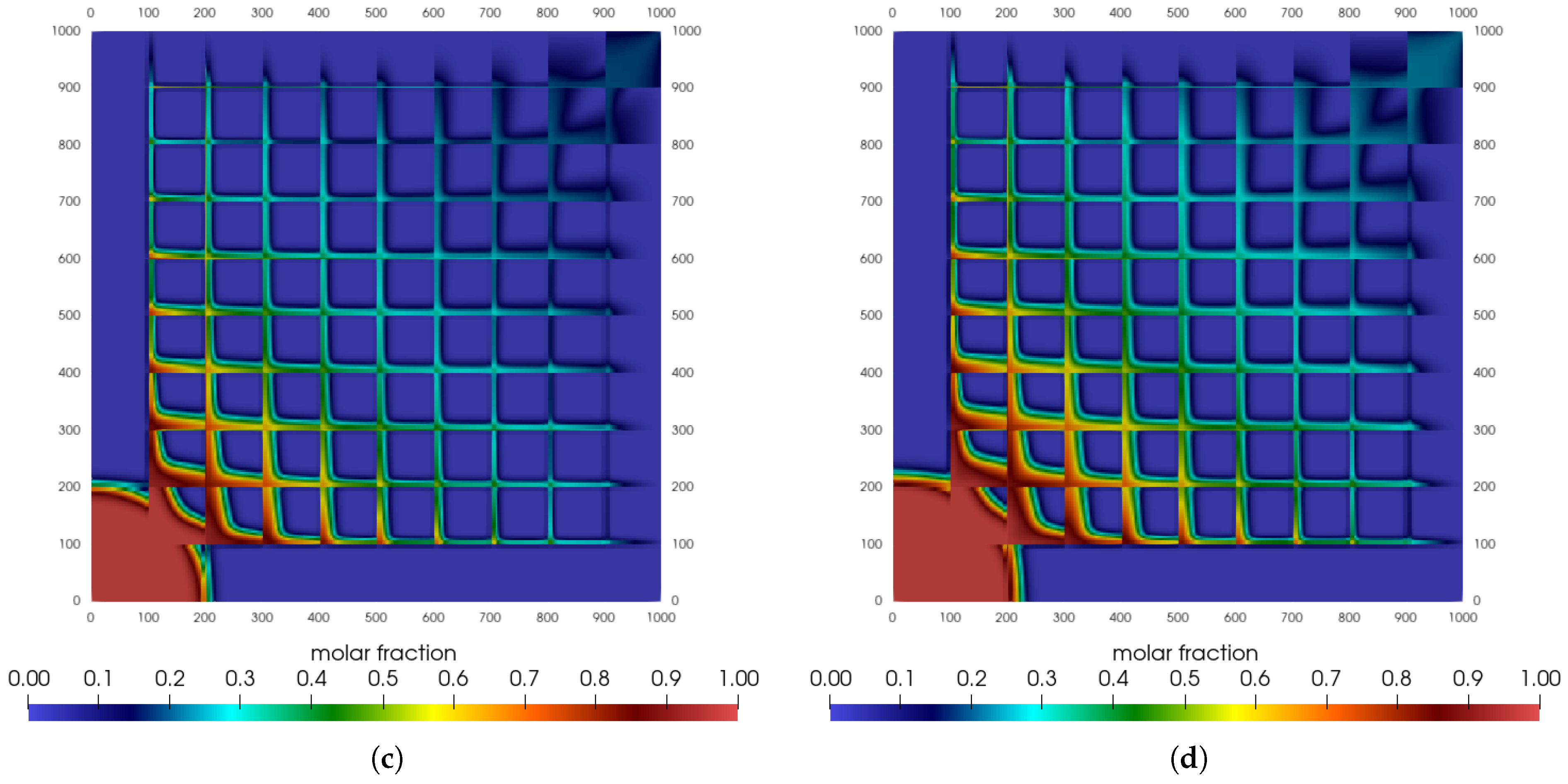
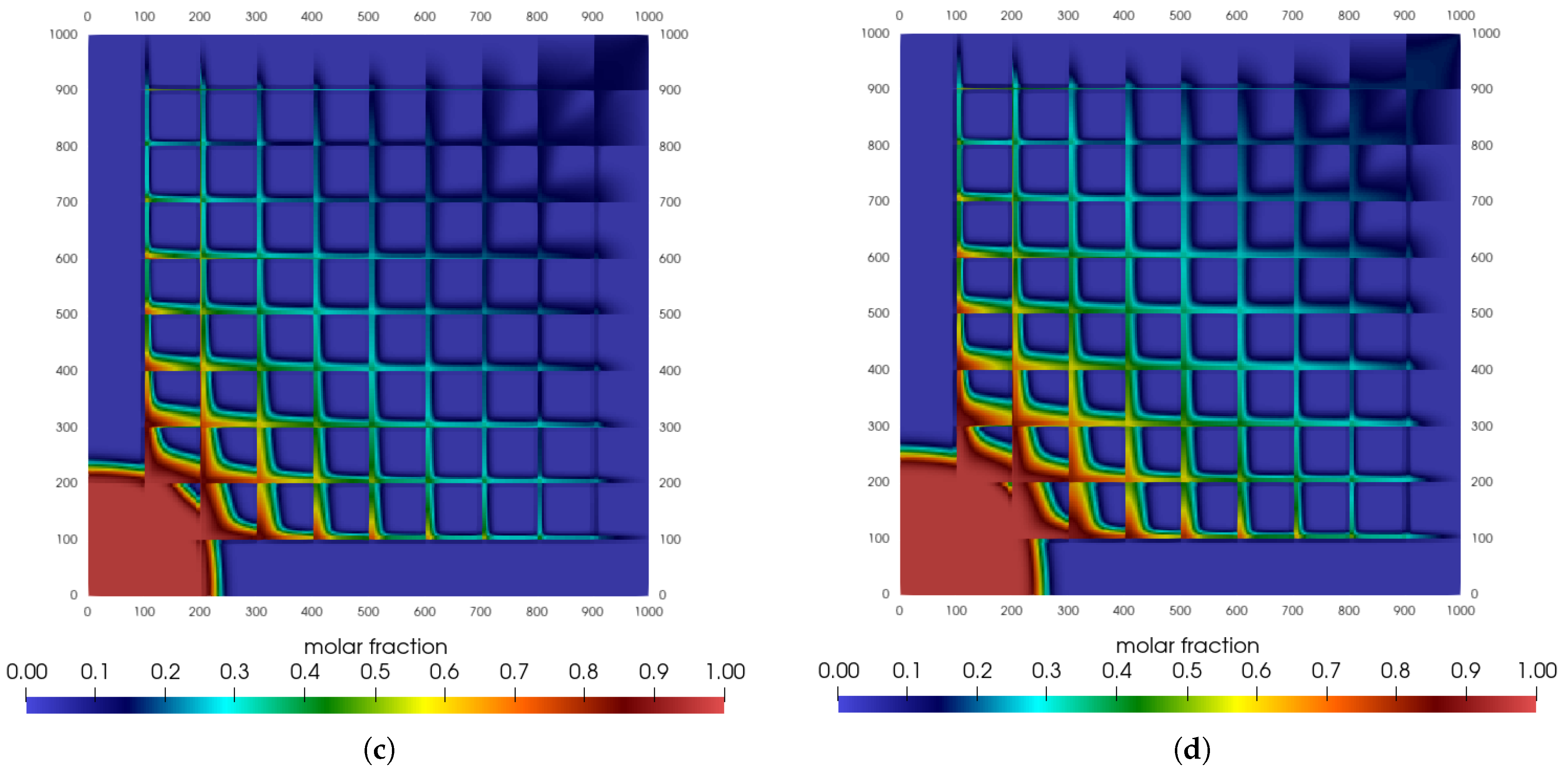
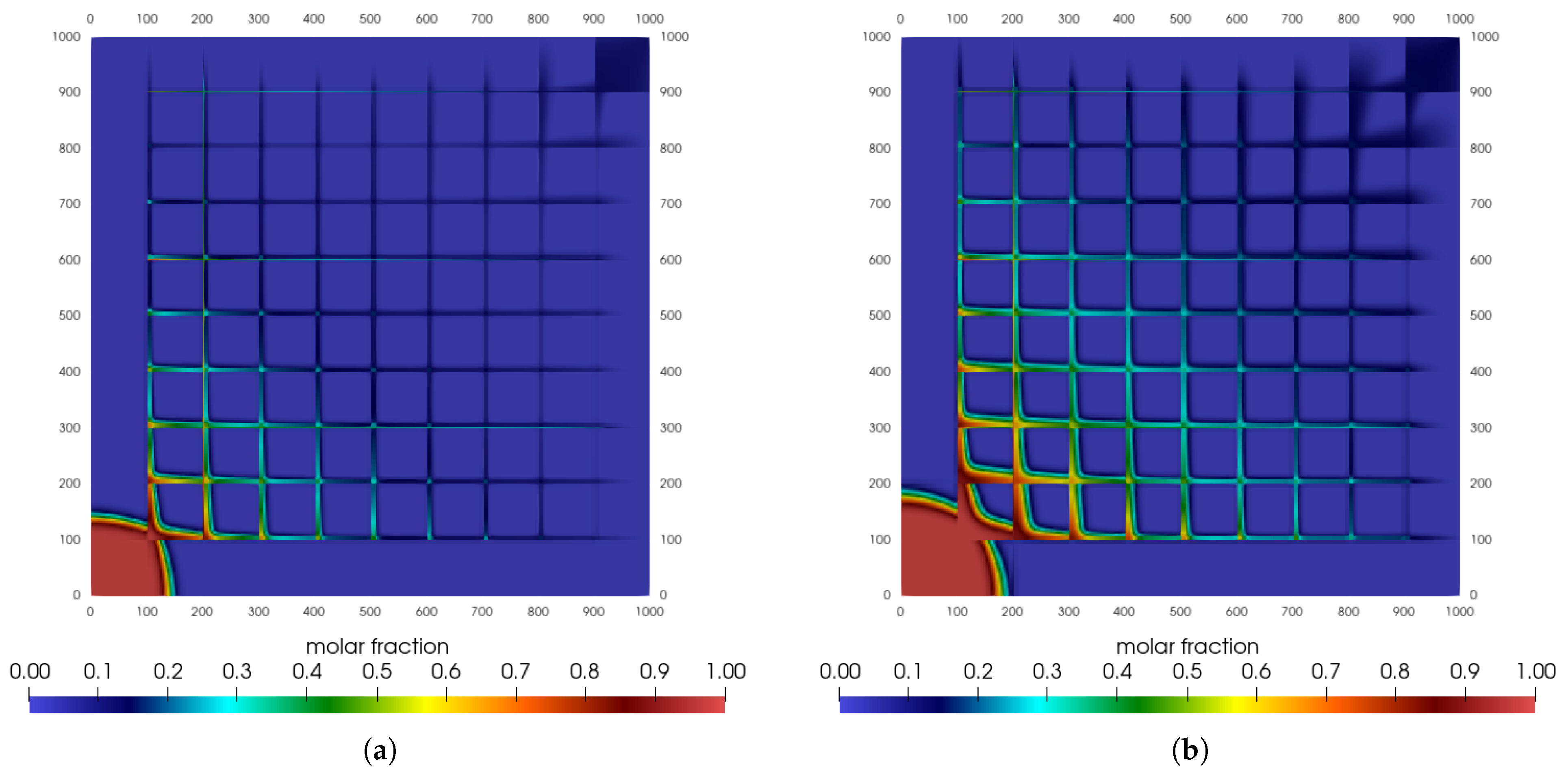
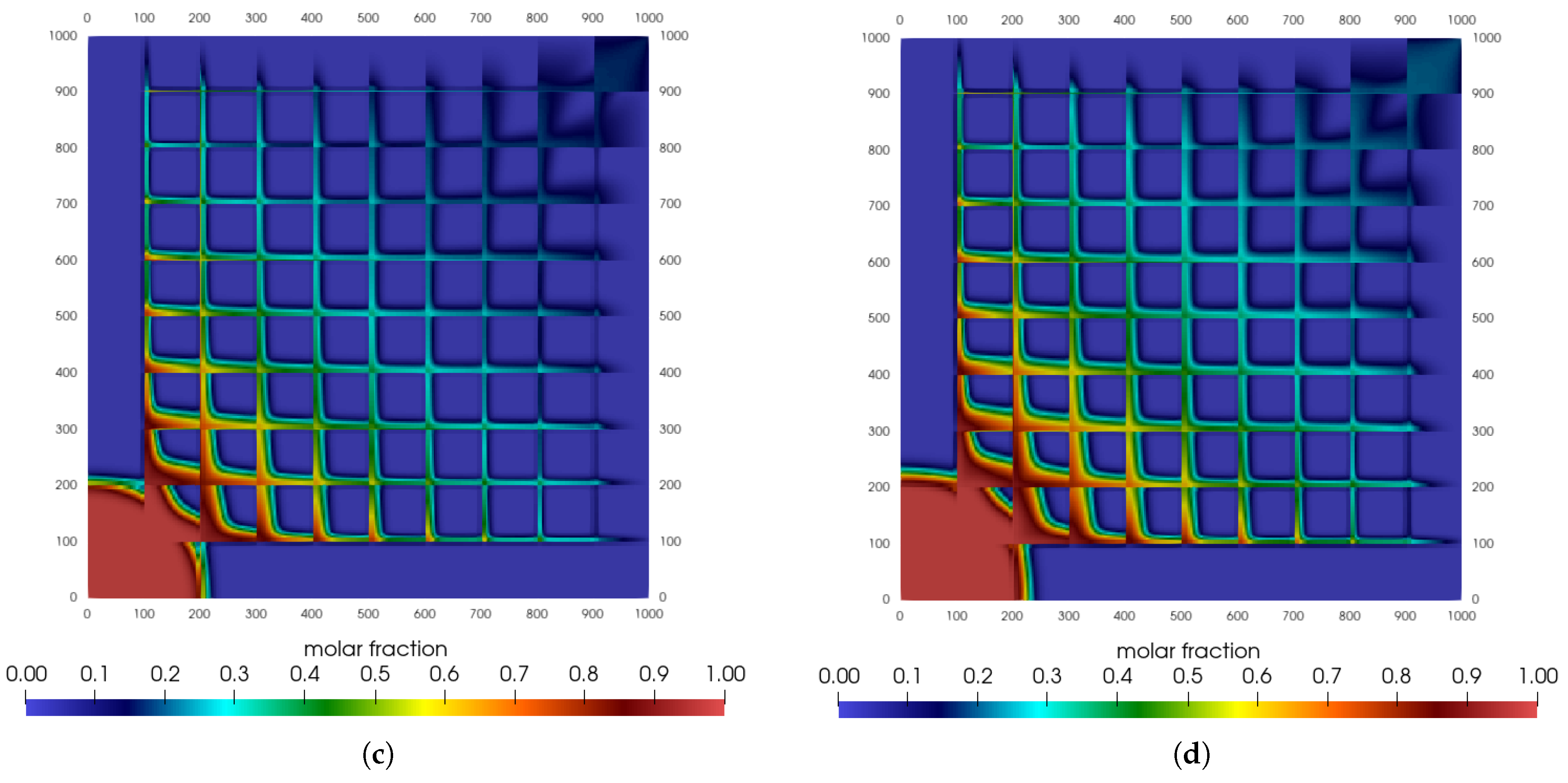
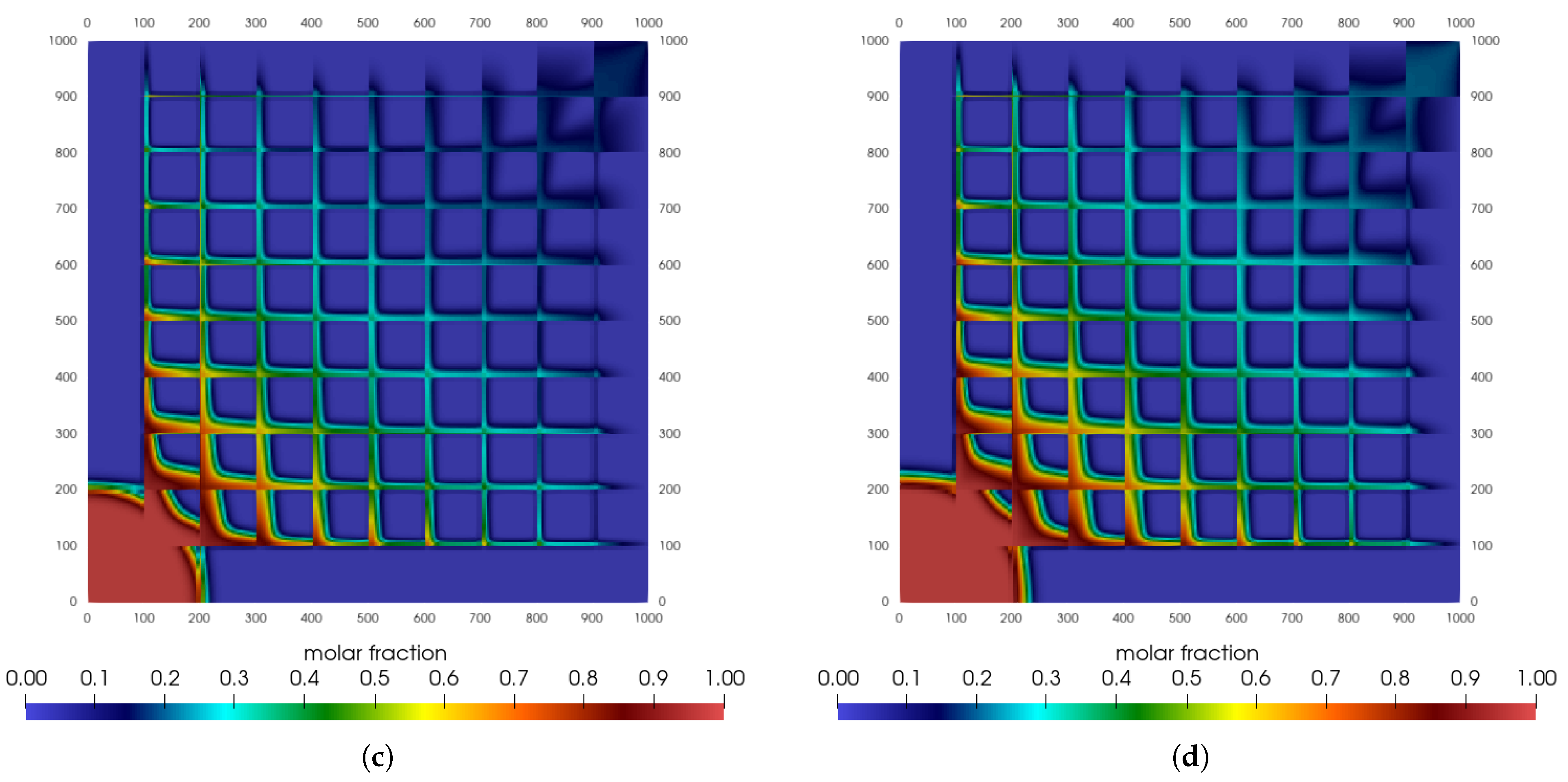
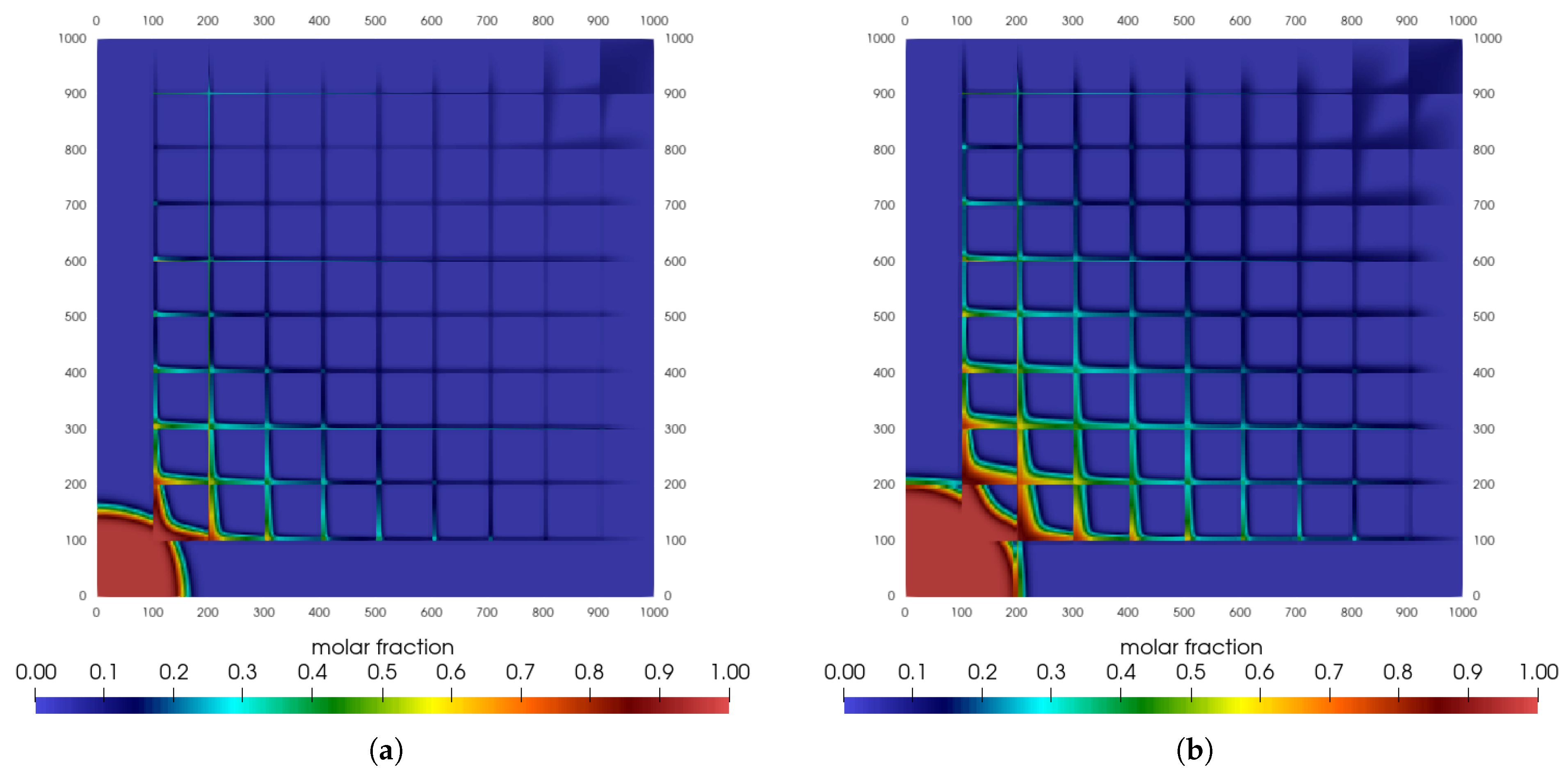
6. Numerical Results
7. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| EGR | Enhanced Gas Recovery |
| CG | Conjugate Gradient Method |
| CSEGR | CO2 Storage with Enhanced Gas Recovery |
| BiCGSTAB | Biconjugate Gradient Stabilized Method |
| DFN | Discrete Fracture Networks |
| FVM | Finite Volume Method |
References
- Bear, J. Modeling Phenomena of Flow and Transport in Porous Media; Springer: Cham, Switzerland, 2018. [Google Scholar]
- Halsey, T.C. Computational sciences in the upstream oil and gas industry. Philos. Trans. R. Soc. 2016, 374, 1–12. [Google Scholar] [CrossRef] [PubMed][Green Version]
- Boudet, H.; Clarke, C.; Bugden, D.; Maibach, E.; Roser-Renouf, C.; Leiserowitz, A. Fracking controversy and communication: Using national survey data to understand public perceptions of hydraulic fracturing. Energy Policy 2014, 65, 57–67. [Google Scholar] [CrossRef]
- Zhao, Y.; Zhang, L.; Shan, B. Mathematical model of fractured horizontal well in shale gas reservoir with rectangular stimulated reservoir volume. J. Nat. Gas Sci. Eng. 2018, 59, 67–79. [Google Scholar] [CrossRef]
- Sheng, G.; Javadpour, F.; Su, Y. Effect of microscale compressibility on apparent porosity and permeability in shale gas reservoirs. Int. J. Heat Mass Transf. 2018, 120, 56–65. [Google Scholar] [CrossRef]
- Shi, J.; Li, X.; Li, Q.; Wang, F.; Sepehrnoori, K. Gas permeability model considering rock deformation and slippage in low permeability water-bearing gas reservoirs. J. Pet. Sci. Eng. 2014, 120, 61–72. [Google Scholar] [CrossRef]
- Moghaddam, R.N.; Jamiolahmady, M. Fluid transport in shale gas reservoirs: Simultaneous effects of stress and slippage on matrix permeability. Int. J. Coal Geol. 2016, 163, 87–99. [Google Scholar] [CrossRef]
- Afagwu, C.; Abubakar, I.; Kalam, S.; Al-Afnan, S.F.; Awotunde, A.A. Pressure-transient analysis in shale gas reservoirs: A review. J. Nat. Gas Sci. Eng. 2020, 78, 103319. [Google Scholar] [CrossRef]
- Miao, Y.; Li, X.; Zhou, Y.; Lee, J.; Sun, Z.; Chang, Y.; Wang, S.; Hou, C. A new rate-transient analysis model for shale gas reservoirs coupled the effect of slip flow and surface diffusion. Int. J. Heat Mass Transf. 2018, 124, 1–10. [Google Scholar] [CrossRef]
- Li, B.; Zhou, F.; Fan, W.; Ren, D.; Li, H.; Li, M. Experimental investigation and theoretical modeling of stress-dependent permeability in naturally fractured tight gas reservoir. J. Pet. Sci. Eng. 2020, 188, 106949. [Google Scholar] [CrossRef]
- Wang, L.; Wang, S.; Zhang, R.; Wang, C.; Xiong, Y.; Zheng, X.; Li, S.; Jin, K.; Rui, Z. Review of multi-scale and multi-physical simulation technologies for shale and tight gas reservoirs. J. Nat. Gas Sci. Eng. 2017, 37, 560–578. [Google Scholar] [CrossRef]
- Fu, J.; Su, Y.; Li, L.; Wang, W.; Wang, C.; Li, D. Productivity model with mechanisms of multiple seepage in tight gas reservoir. J. Pet. Sci. Eng. 2022, 209, 109825. [Google Scholar] [CrossRef]
- Zhang, Y.; Jin, Y.; Yang, D. Semi-analytical modeling of transient pressure behaviour for a multifractured horizontal well in a gas reservoir with a complex fracture network by considering effects of slippage, stress-sensitivity, and gas adsorption/desorption. J. Pet. Sci. Eng. 2022, 214, 110504. [Google Scholar] [CrossRef]
- Song, H.; Cao, Y.; Yu, M.; Wang, Y.; Killough, J.E.; Leung, J. Impact of permeability heterogeneity on production characteristics in water-bearing tight gas reservoirs with threshold pressure gradient. J. Nat. Gas Sci. Eng. 2015, 22, 172–181. [Google Scholar] [CrossRef]
- Guria, C. Pressure- and temperature-dependent Klinkenberg slippage effect in porous media to non-ideal gases. Geoenergy Sci. Eng. 2023, 224, 211629. [Google Scholar] [CrossRef]
- Rubin, C.; Zamirian, M.; Takbiri-Borujeni, A.; Gu, M. Investigation of gas slippage effect and matrix compaction effect on shale gas production evaluation and hydraulic fracturing design based on experiment and reservoir simulation. Fuel 2019, 241, 12–24. [Google Scholar] [CrossRef]
- Ding, J.; Yang, S.; Nie, X.; Wang, Z. Dynamic threshold pressure gradient in tight gas reservoir. J. Nat. Gas Sci. Eng. 2014, 20, 155–160. [Google Scholar] [CrossRef]
- Friedel, T.; Voigt, H.D. Investigation of non-Darcy flow in tight-gas reservoirs with fractured wells. J. Pet. Sci. Eng. 2006, 54, 112–128. [Google Scholar] [CrossRef]
- Clarkson, C.; Jensen, J.; Chipperfield, S. Unconventional gas reservoir evaluation: What do we have to consider? J. Nat. Gas Sci. Eng. 2012, 8, 9–33. [Google Scholar] [CrossRef]
- Ye, Z.; Chen, D.; Wang, J. Evaluation of the non-Darcy effect in coalbed methane production. Fuel 2014, 121, 1–10. [Google Scholar] [CrossRef]
- Wang, C.; Li, Z.P.; Li, H.; Wei, Q.; Bai, R.T. A new method to calculate the productivity of fractured horizontal gas wells considering non-Darcy flow in the fractures. J. Nat. Gas Sci. Eng. 2015, 26, 981–991. [Google Scholar] [CrossRef]
- Farahani, M.; Saki, M.; Ghafouri, A.; Khaz’ali, A.R. Laboratory measurements of slippage and inertial factors in carbonate porous media: A case study. J. Pet. Sci. Eng. 2018, 162, 666–673. [Google Scholar] [CrossRef]
- Feng, R.; Chen, S.; Bryant, S.; Liu, J. Stress-dependent permeability measurement techniques for unconventional gas reservoirs: Review, evaluation, and application. Fuel 2019, 256, 115987. [Google Scholar] [CrossRef]
- Wang, J.; Yu, L.; Yuan, Q. Experimental study on permeability in tight porous media considering gas adsorption and slippage effect. Fuel 2019, 253, 561–570. [Google Scholar] [CrossRef]
- Luo, H.; Li, H.; Zhou, X.; Li, Y.; Li, Y.; Zhu, X. Modeling temperature behavior of multistage fractured horizontal well with two-phase flow in low-permeability gas reservoirs. J. Pet. Sci. Eng. 2019, 173, 1187–1209. [Google Scholar] [CrossRef]
- Liu, G.; Xie, S.; Tian, W.; Wang, J.; Li, S.; Wang, Y.; Yang, D. Effect of pore-throat structure on gas-water seepage behaviour in a tight sandstone gas reservoir. Fuel 2022, 310, 121901. [Google Scholar] [CrossRef]
- Sun, H.; Ouyang, W.; Zhu, S.; Wan, Y.; Tang, Y.; Cao, W. A new numerical well test method of multi-scale discrete fractured tight sandstone gas reservoirs and its application in the Kelasu Gas Field of the Tarim Basin. Nat. Gas Ind. B 2023, 10, 103–113. [Google Scholar] [CrossRef]
- Tapia, J.F.D.; Lee, J.Y.; Ooi, R.E.; Foo, D.C.; Tan, R.R. A review of optimization and decision-making models for the planning of CO2 capture, utilization and storage (CCUS) systems. Sustain. Prod. Consum. 2018, 13, 1–15. [Google Scholar] [CrossRef]
- Zhao, X.; Chen, Z.; Wang, B.; Liao, X.; Li, D.; Zhou, B. A Multi-medium and Multi-mechanism model for CO2 injection and storage in fractured shale gas reservoirs. Fuel 2023, 345, 128167. [Google Scholar] [CrossRef]
- Liu, S.Y.; Ren, B.; Li, H.Y.; Yang, Y.Z.; Wang, Z.Q.; Wang, B.; Xu, J.; Agarwal, R. CO2 storage with enhanced gas recovery (CSEGR): A review of experimental and numerical studies. Pet. Sci. 2022, 19, 594–607. [Google Scholar] [CrossRef]
- Haq, B.; Salahu Muhammed, N.; Liu, J.; Tong Chua, H. Enhanced natural gas production using CO2 injection: Application to sustainable hydrogen production. Fuel 2023, 347, 128474. [Google Scholar] [CrossRef]
- Tang, C.; Zhou, W.; Chen, Z.; Wei, J. Numerical simulation of CO2 sequestration in shale gas reservoirs at reservoir scale coupled with enhanced gas recovery. Energy 2023, 277, 127657. [Google Scholar] [CrossRef]
- Aminu, M.D.; Manovic, V. A modelling study to evaluate the effect of impure CO2 on reservoir performance in a sandstone saline aquifer. Heliyon 2020, 6, E04597. [Google Scholar] [CrossRef] [PubMed]
- Wang, Y.; Vuik, C.; Hajibeygi, H. CO2 Storage in deep saline aquifers: Impacts of fractures on hydrodynamic trapping. Int. J. Greenh. Gas Control. 2022, 113, 103552. [Google Scholar] [CrossRef]
- Parvin, S.; Masoudi, M.; Sundal, A.; Miri, R. Continuum scale modelling of salt precipitation in the context of CO2 storage in saline aquifers with MRST compositional. Int. J. Greenh. Gas Control. 2020, 99, 103075. [Google Scholar] [CrossRef]
- Zeng, F.; Zhao, G. Semianalytical Model for Reservoirs with Forchheimer’s Non-Darcy Flow. Soc. Pet. Eng. Reserv. Eval. Eng. 2008, 11, 280–291. [Google Scholar] [CrossRef]
- Amao, A.M. Mathematical Model for Darcy-Forchheimer Flow with Applications to Well Performance Analysis. Master’s Thesis, Texas Tech University, Lubbock, TX, USA, 2007. [Google Scholar]
- Barree, R.D.; Conway, M.W. Beyond Beta Factors: A Complete Model for Darcy, Forchheimer, and Trans-Forchheimer Flow in Porous Media. In Proceedings of the Society of Petroleum Engineers Annual Technical Conference and Exhibition, Houston, TX, USA, 26–29 September 2004. [Google Scholar] [CrossRef]
- Lai, B.; Miskimins, J.L.; Wu, Y.S. Non-Darcy Porous Media Flow According to the Barree and Conway’s Model: Laboratory and Numerical Modeling Studies. In Proceedings of the Society of Petroleum Engineers Rocky Mountain Petroleum Technology Conference, Denver, CO, USA, 14–16 April 2009. [Google Scholar] [CrossRef]
- Souza, G.; Amaral Souto, H.P. A comparative study of non-Darcy flows in naturally fractured gas reservoirs. J. Braz. Soc. Mech. Sci. Eng. 2016, 38, 1701–1715. [Google Scholar] [CrossRef]
- Zhang, Y.; Yang, D. Modeling two-phase flow behaviour in a shale gas reservoir with complex fracture networks and flow dynamics. Gas Sci. Eng. 2023, 119, 205112. [Google Scholar] [CrossRef]
- Civan, F.; Rai, C.S.; Sondergeld, C.H. Shale-Gas Permeability and Diffusivity Inferred by Improved Formulation of Relevant Retention and Transport Mechanisms. Transp. Porous Media 2011, 86, 925–944. [Google Scholar] [CrossRef]
- Florence, F.A.; Rushing, J.A.; Newsham, K.E.; Blasingame, T.A. Improved Permeability Prediction Relations for Low-Permeability Sands. In Proceedings of the SPE Rocky Mountain Petroleum Technology Conference/Low Permeability Reservoirs Symposium 2007, Denver, CO, USA, 16–18 April 2007. [Google Scholar] [CrossRef]
- Wang, S.; Lukyanov, A.A.; Wu, Y.S. Second-order gas slippage model for the Klinkenberg effect of multicomponent gas at finite Knudsen numbers up to 1. Fuel 2019, 235, 1275–1286. [Google Scholar] [CrossRef]
- de Queiroz, R.Z.H.G.; do Rosário, R.C.D.; de Souza, G.; Amaral Souto, H.P. On the numerical simulation of wellbore pressure in gas reservoirs incorporating the phenomena of slippage, formation damage and wellbore storage. Int. J. Adv. Eng. Res. Sci. 2020, 7, 224–239. [Google Scholar] [CrossRef]
- Secchi, S.; Schrefler, B.A. A method for 3-D hydraulic fracturing simulation. Int. J. Fract. 2012, 178, 245–258. [Google Scholar] [CrossRef]
- Chen, Z. Reservoir Simulation—Mathematical Techniques in Oil Recovery; Society of Industrial and Applied Mathematics: Philadelphia, PA, USA, 2007. [Google Scholar]
- Debossam, J.G.S.; dos Santos Heringer, F.D.; de Souza, G.; Amaral Souto, H.P. Numerical simulation of single-phase two-components flow in naturally fractured oil reservoirs. Coupled Syst. Mech. 2019, 8, 129–146. [Google Scholar] [CrossRef]
- Debossam, J.G.S.; de Souza, G.; Amaral Souto, H.P.; Pires, A.P. Numerical simulation of single-phase two-component non-Darcy flow in naturally fractured reservoirs for enhanced gas recovery and carbon dioxide storage. Braz. J. Chem. Eng. 2023, 41, 197–219. [Google Scholar] [CrossRef]
- Böttcher, N.; Singh, A.K.; Kolditz, O.; Liedl, R. Non-isothermal, compressible gas flow for the simulation of an enhanced gas recovery application. J. Comput. Appl. Math. 2012, 236, 4933–4943. [Google Scholar] [CrossRef]
- Soave, G. Equilibrium constants from a modified Redlich-Kwong equation of state. Chem. Eng. Sci. 1972, 27, 1197–1203. [Google Scholar] [CrossRef]
- Lohrenz, J.; Bray, B.G.; Clark, C.R. Calculating viscosities of reservoir fluids from their compositions. J. Pet. Technol. 1964, 16, 1171–1176. [Google Scholar] [CrossRef]
- Versteeg, H.K.; Malalasekera, W. An Introduction to Computational Fluid Dynamics: The Finite Volume Method; Pearson: London, UK, 2007. [Google Scholar]
- Ertekin, T.; Abou-Kassem, J.; King, G. Basic Applied Reservoir Simulation; Society of Petroleum Engineers: Richardson, TX, USA, 2001. [Google Scholar]
- Biryukov, D.; Kuchuk, F.J. Transient Pressure Behavior of Reservoirs with Discrete Conductive Faults and Fractures. Transp. Porous Media 2012, 95, 239–268. [Google Scholar] [CrossRef]
- Vennemo, S. Multiscale Simulation of Thermal Flow in Porous Media. Master’s Thesis, Norwegian University of Science and Technology, Trondheim, Norway, 2016. [Google Scholar]
- Saad, Y. Iterative Methods for Sparse Linear Systems, 2nd ed.; Society of Industrial and Applied Mathematics: Philadelphia, PA, USA, 2003. [Google Scholar]
- Sandve, T.; Berre, I.; Nordbotten, J. An efficient multi-point flux approximation method for Discrete Fracture—Matrix simulations. J. Comput. Phys. 2012, 231, 3784–3800. [Google Scholar] [CrossRef]
- Warren, J.E.; Root, P.J. The behavior of naturally fractured reservoirs. Soc. Pet. Eng. J. 1963, 3, 245–255. [Google Scholar] [CrossRef]
- Barenblatt, G.; Zheltov, I.; Kochina, I. Basic concepts in the theory of seepage of homogeneous liquids in fissured rocks [strata]. J. Appl. Math. Mech. 1960, 24, 1286–1303. [Google Scholar] [CrossRef]
- do Rosário, R.C.D.; de Souza, G.; Amaral Souto, H.P. A comparative study of some well-reservoir coupling models in the numerical simulation of oil reservoirs. Int. J. Adv. Eng. Res. Sci. 2020, 7, 126–148. [Google Scholar] [CrossRef]




| Parameter | Unit | Value |
|---|---|---|
| rock compressibility () | kPa−1 | 3 × 10−11 |
| molecular diffusion coefficient () | m2/s | 1 × 10−8 |
| Barree and Conway model parameter () | – | 0.013 |
| reservoir permeability () | mDarcy | 0.1 |
| characteristic length () | m | 1000 |
| width and length of the reservoir () | m | 1000 |
| reservoir depth () | m | 10 |
| reference pressure () | MPa | 30 |
| prescribed flow rate () | mol/day | 50 × 103 |
| reference temperature () | K | 394 |
| permeability modulus () | kPa−1 | 3 × 10−5 |
| hydrodynamic dispersion model parameter () | – | 1.2 |
| hydrodynamic dispersion model parameter () | – | 2.958 |
| tortuosity () | m−1 | |
| inverse of characteristic length () | m−1 | 5000 |
| reservoir porosity () | – | 0.1 |
| Parameter | Unit | CO2 | CH4 |
|---|---|---|---|
| critical pressure () | MPa | 7.38 | 4.61 |
| critical temperature () | K | 304.1 | 190.6 |
| acentric factor () | – | 0.239 | 0.0116 |
| Parameter | Unit | Value |
|---|---|---|
| fracture length () | m | 1000 |
| fracture thickness (w) | m | 0.01 |
| fracture permeability () | mDarcy | 7.0 × 104 |
| fracture porosity () | – | 0.75 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Debossam, J.G.S.; de Freitas, M.M.; de Souza, G.; Amaral Souto, H.P.; Pires, A.P. Numerical Simulation of Non-Darcy Flow in Naturally Fractured Tight Gas Reservoirs for Enhanced Gas Recovery. Gases 2024, 4, 253-272. https://doi.org/10.3390/gases4030015
Debossam JGS, de Freitas MM, de Souza G, Amaral Souto HP, Pires AP. Numerical Simulation of Non-Darcy Flow in Naturally Fractured Tight Gas Reservoirs for Enhanced Gas Recovery. Gases. 2024; 4(3):253-272. https://doi.org/10.3390/gases4030015
Chicago/Turabian StyleDebossam, João Gabriel Souza, Mayksoel Medeiros de Freitas, Grazione de Souza, Helio Pedro Amaral Souto, and Adolfo Puime Pires. 2024. "Numerical Simulation of Non-Darcy Flow in Naturally Fractured Tight Gas Reservoirs for Enhanced Gas Recovery" Gases 4, no. 3: 253-272. https://doi.org/10.3390/gases4030015
APA StyleDebossam, J. G. S., de Freitas, M. M., de Souza, G., Amaral Souto, H. P., & Pires, A. P. (2024). Numerical Simulation of Non-Darcy Flow in Naturally Fractured Tight Gas Reservoirs for Enhanced Gas Recovery. Gases, 4(3), 253-272. https://doi.org/10.3390/gases4030015







