Numerical Modeling on Fate and Transport of Pollutants in the Vadose Zone †
Abstract
:1. Introduction
2. Theoretical Framework
3. Materials and Methods
3.1. Setup of the Numerical Model
3.2. Comparison Methodology
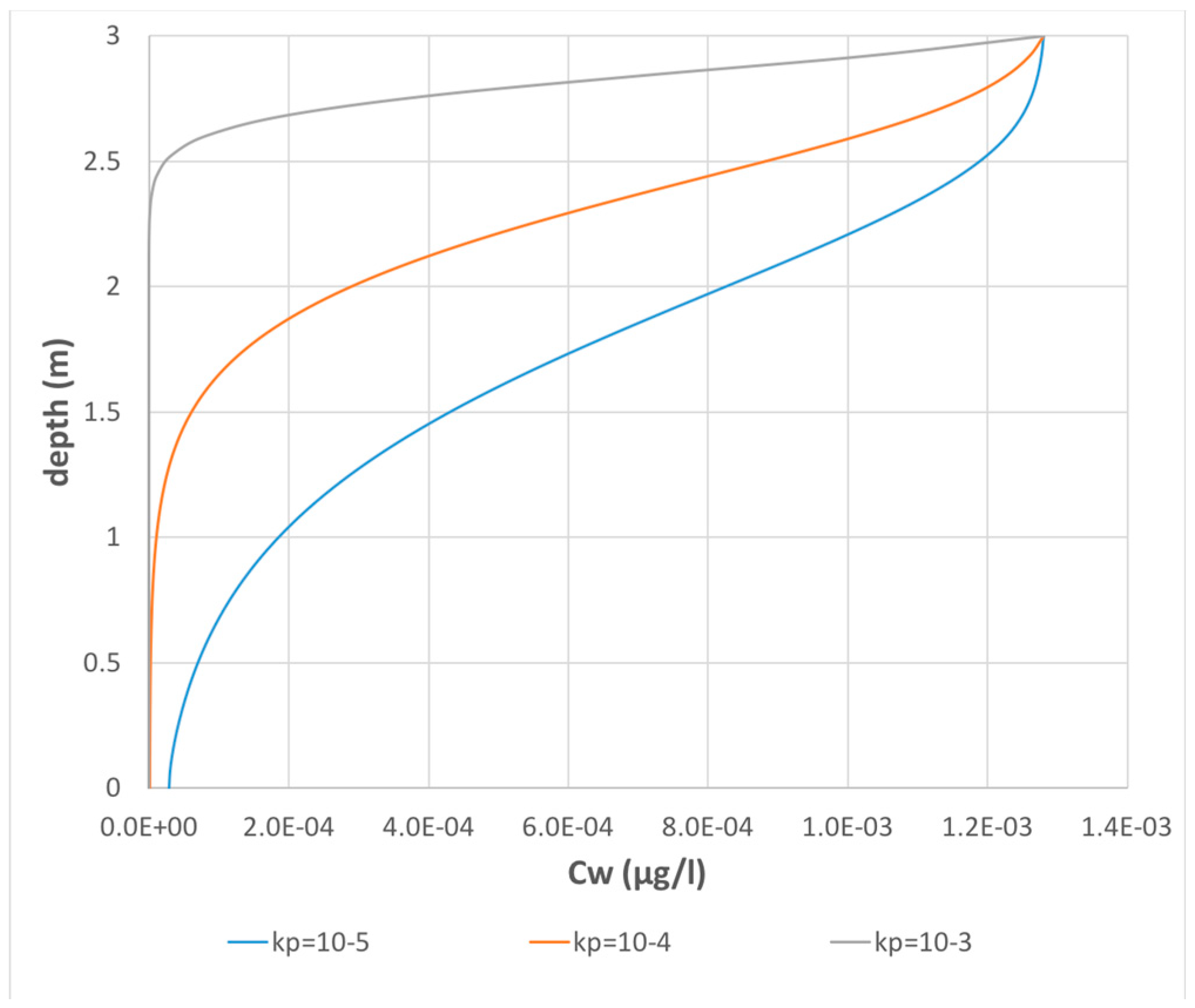
4. Results and Discussion
- Three families of four curves each, the first one relating to the depth of 2 m in shades of blue, the second one relating to the depth of 1 m in shades of orange, and the third one relating to the depth of 0.5 m in shades of green. The i-th family (i = 1, …, 4) is related to the constant precipitation and the transient precipitation with a 3 months’ step, 5 months’ step, and 8 months’ step;
- Each family of curves has the same inflexion point;
- Curves follow a similar trend, with an initial concavity upwards and a final concavity downwards;
- The effects of the different precipitation scenario are not predominant, compared to the remaining parameters being investigated.
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Foster, S.; Hirata, R.; Gomes, D.; D’Elia, M.; Paris, M. Groundwater Quality Protection: A Guide for Water Service Companies, Municipal Authorities and Environment Agencies; The World Bank: Washington, DC, USA, 2002. [Google Scholar] [CrossRef]
- Withers, P.J.; Jordan, P.; May, L.; Jarvie, H.P.; Deal, N.E. Do septic tank systems pose a hidden threat to water quality? Front. Ecol. Environ. 2014, 12, 123–130. [Google Scholar] [CrossRef]
- Šimůnek, J.; van Genuchten, M.T. Contaminant transport in the unsaturated zone: Theory and modeling. In The Handbook of Groundwater Engineering, 3rd ed.; CRC Press: Boca Raton, FL, USA, 2016; pp. 221–254. ISBN 9781498703055. [Google Scholar]
- Rivett, M.O.; Wealthall, G.P.; Dearden, R.A.; McAlary, T.A. Review of unsaturated-zone transport and attenuation of volatile organic compound (VOC) plumes leached from shallow source zones. J. Contam. Hydrol. 2011, 123, 130–156. [Google Scholar] [CrossRef] [PubMed]
- Berlin, M.; Vasudevan, M.; Kumar, G.S.; Nambi, I.M. Numerical modelling on fate and transport of petroleum hydrocarbons in an unsaturated subsurface system for varying source scenario. J. Earth Syst. Sci. 2015, 124, 655–674. [Google Scholar] [CrossRef]
- Zhang, P.; Hu, L. Contaminant Transport in Soils Considering Preferential Flowpaths. Geoenviron. Eng. 2014, 50–59. [Google Scholar] [CrossRef]
- Pang, L.; Lafogler, M.; Knorr, B.; McGill, E.; Saunders, D.; Baumann, T.; Abraham, P.; Close, M. Influence of colloids on the attenuation and transport of phosphorus in alluvial gravel aquifer and vadose zone media. Sci. Total Environ. 2016, 550, 60–68. [Google Scholar] [CrossRef] [PubMed]
- Mulligan, C.N.; Yong, R.N. Natural attenuation of contaminated soils. Environ. Int. 2004, 30, 587–601. [Google Scholar] [CrossRef] [PubMed]
- Flury, M.; Wai, N.N. Dyes as tracers for vadose zone hydrology. Rev. Geophys. 2003, 41. [Google Scholar] [CrossRef]
- Vanderborght, J.; Vereecken, H. Review of dispersivities for transport modeling in soils. Vadose Zone J. 2007, 6, 29–52. [Google Scholar] [CrossRef]
- Goyne, K.W.; Jun, H.J.; Anderson, S.H.; Motavalli, P.P. Phosphorus and nitrogen sorption to soils in the presence of poultry litter-derived dissolved organic matter. J. Environ. Qual. 2008, 37, 154–163. [Google Scholar] [CrossRef] [PubMed]
- Kuntz, D.; Grathwohl, P. Comparison of steady-state and transient flow conditions on reactive transport of contaminants in the vadose soil zone. J. Hydrol. 2009, 369, 225–233. [Google Scholar] [CrossRef]
- Marshall, J.D.; Shimada, B.W.; Jaffe, P.R. Effect of temporal variability in infiltration on contaminant transport in the unsaturated zone. J. Contam. Hydrol. 2000, 46, 151–161. [Google Scholar] [CrossRef]
- Wang, P.; Quinlan, P.; Tartakovsky, D.M. Effects of spatio-temporal variability of precipitation on contaminant migration in the vadose zone. Geophys. Res. Lett. 2009, 36. [Google Scholar] [CrossRef]
- COMSOL Multiphysics®. v. 5.3. www.comsol.com; COMSOL AB: Stockholm, Sweden, 2020. [Google Scholar]
- Richards, L.A. Capillary conduction of liquids through porous mediums. Physics 1931, 1, 318–333. [Google Scholar] [CrossRef]
- Bear, J. Dynamics of Fluids in Porous Media; Courier Corporation: Chelmsford, MA, USA, 2013; ISBN 978-0486131801. [Google Scholar]
- Kosugi, K.; Hopmans, J.W.; Dane, J.H. Water retention and storage—parametric models. In Methods of Soil Analysis. Part. 4. Physical Methods; Dane, J.H., Topp, G.C., Eds.; Soil Science Society of America: Madison, WI, USA, 2002; pp. 739–758, ISBN 13 978-0891188414. [Google Scholar]
- Van Genuchten, M.T. A closed-form equation for predicting the hydraulic conductivity of unsaturated soils. Soil Sci. Soc. Am. J. 1980, 44, 892–898. [Google Scholar] [CrossRef]
- Song, X.; Yan, M.; Li, H. The development of a one-parameter model for the soil-water characteristic curve in the loess gully region. J. Food Agric. Environ. 2013, 11, 1546–1549. [Google Scholar] [CrossRef]
- Vanclooster, M.; Javaux, M.; Vanderborght, J. Solute transport in soil at the core and field scale. Encycl. Hydrol. Sci. 2006. [Google Scholar] [CrossRef]
- Bear, J.; Cheng, A.H.D. Modeling Groundwater Flow and Contaminant Transport (Volume 23); Springer Science and Business Media: Berlin, Germany, 2010; ISBN 978-1402066818. [Google Scholar]
- Foo, K.Y.; Hameed, B.H. Insights into the modeling of adsorption isotherm systems. Chem. Eng. J. 2010, 156, 2–10. [Google Scholar] [CrossRef]
- Brusseau, M.L.; Chorover, J. Chemical processes affecting contaminant transport and fate. In Environmental Pollution Science, 3rd ed.; Academic Press: Cambridge, MA, USA, 2019; Volume 8, pp. 113–130. [Google Scholar] [CrossRef]
- Allen-King, R.M.; Grathwohl, P.; Ball, W.P. New modeling paradigms for the sorption of hydrophobic organic chemicals to heterogeneous carbonaceous matter in soils, sediments, and rocks. Adv. Water Resour. 2002, 25, 985–1016. [Google Scholar] [CrossRef]
- Thornthwaite, C.W. An approach toward a rational classification of climate. Geogr. Rev. 1948, 38, 55–94. [Google Scholar] [CrossRef]
- ISS-ISPESL. Database of the Physico-Chemical and Toxicological Properties of Contamainants. Available online: http://old.iss.it/suol/?lang=1&id=96&tipo=13 (accessed on 22 May 2020). (In Italian).
- Gelhar, L.W. A Review of Field-Scale Physical Solute Transport Processes in Saturated and Unsaturated Porous Media; EA-4190, Research Project 2485-5; Electronic Power Research Institute: Palo Alto, CA, USA, 1985; 116p. [Google Scholar]
- Connor, J.A.; Newell, C.J.; Malander, M.W. Parameter estimation guidelines for risk-based corrective action (RBCA) modeling. In Proceedings of the Petroleum Hydrocarbons and Organic Chemicals in Groundwater Conference; National Ground Water Association: Houston, TX, USA; 1996; pp. 13–15. [Google Scholar]
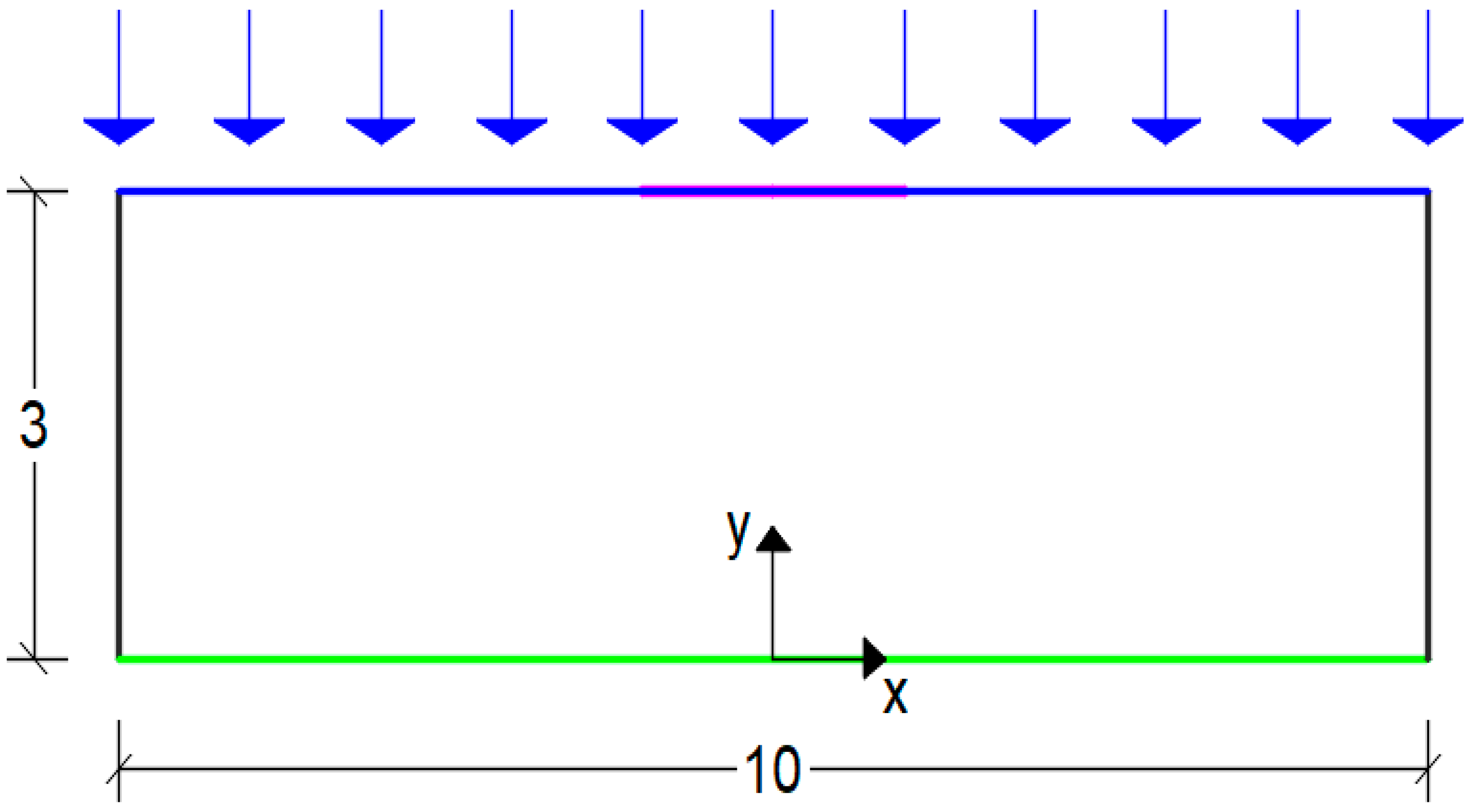


| Parameter | Measurement Unit | Values | |
|---|---|---|---|
| Benzene | Tetrachloroethylene | ||
| Molecular weight | g/mol | 78.1 | 165.8 |
| Solubility (S) | mg/L | 1750 | 200 |
| Diffusion coefficient in water (Dw) | cm2/s | 9.80 × 10−6 | 8.20 × 10−6 |
| Parameter | Measurement Unit | Values |
|---|---|---|
| Soil bulk density (ρb) | kg/m3 | 1700 |
| Saturated water content (θs) | - | 0.41 |
| Residual water content (θr) | - | 0.065 |
| Van Genuchten parameter (α) | - | 0.075 |
| Van Genuchten parameter (n) | - | 1.89 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Viccione, G.; Stoppiello, M.G.; Lauria, S.; Cascini, L. Numerical Modeling on Fate and Transport of Pollutants in the Vadose Zone. Environ. Sci. Proc. 2020, 2, 34. https://doi.org/10.3390/environsciproc2020002034
Viccione G, Stoppiello MG, Lauria S, Cascini L. Numerical Modeling on Fate and Transport of Pollutants in the Vadose Zone. Environmental Sciences Proceedings. 2020; 2(1):34. https://doi.org/10.3390/environsciproc2020002034
Chicago/Turabian StyleViccione, Giacomo, Maria Grazia Stoppiello, Silvia Lauria, and Leonardo Cascini. 2020. "Numerical Modeling on Fate and Transport of Pollutants in the Vadose Zone" Environmental Sciences Proceedings 2, no. 1: 34. https://doi.org/10.3390/environsciproc2020002034
APA StyleViccione, G., Stoppiello, M. G., Lauria, S., & Cascini, L. (2020). Numerical Modeling on Fate and Transport of Pollutants in the Vadose Zone. Environmental Sciences Proceedings, 2(1), 34. https://doi.org/10.3390/environsciproc2020002034






