Strong Light–Matter Interaction of a Quantum Emitter near a Graphene Nanodisk †
Abstract
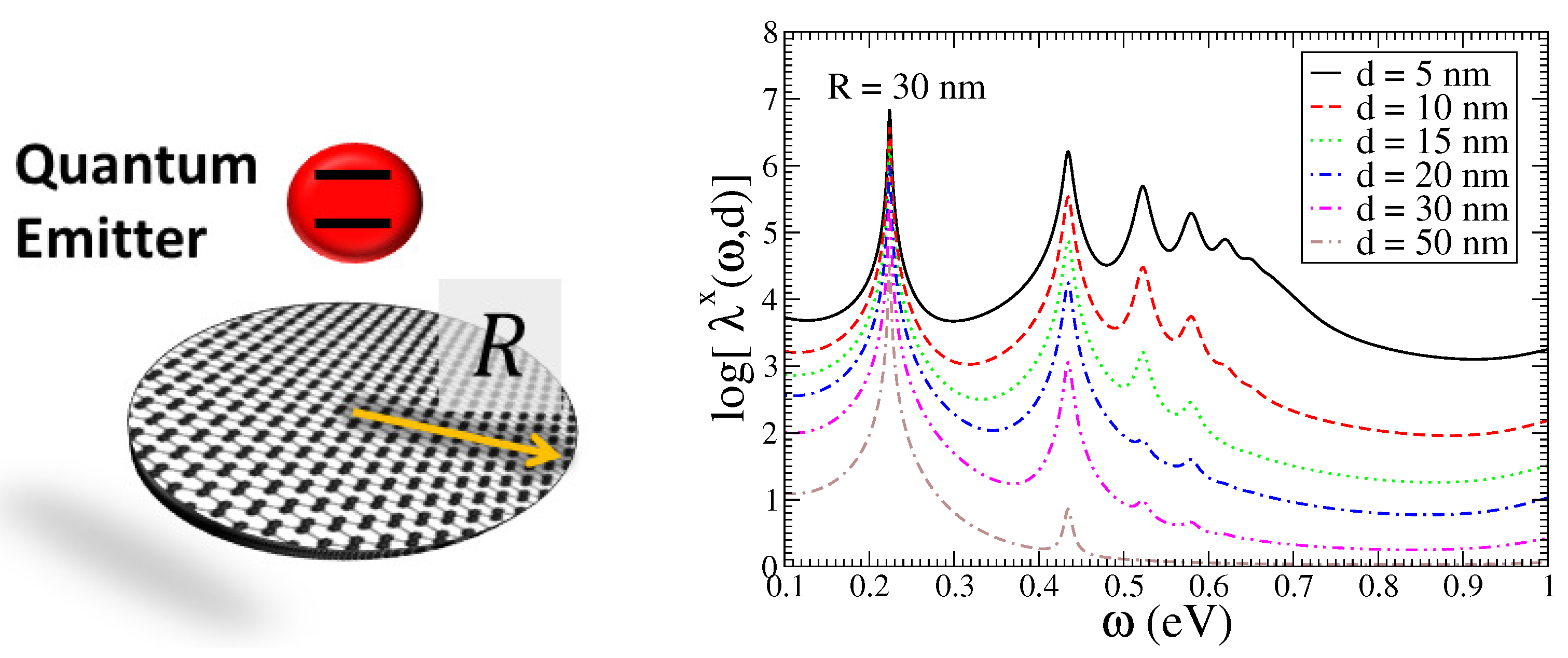
:1. Introduction
2. Theory
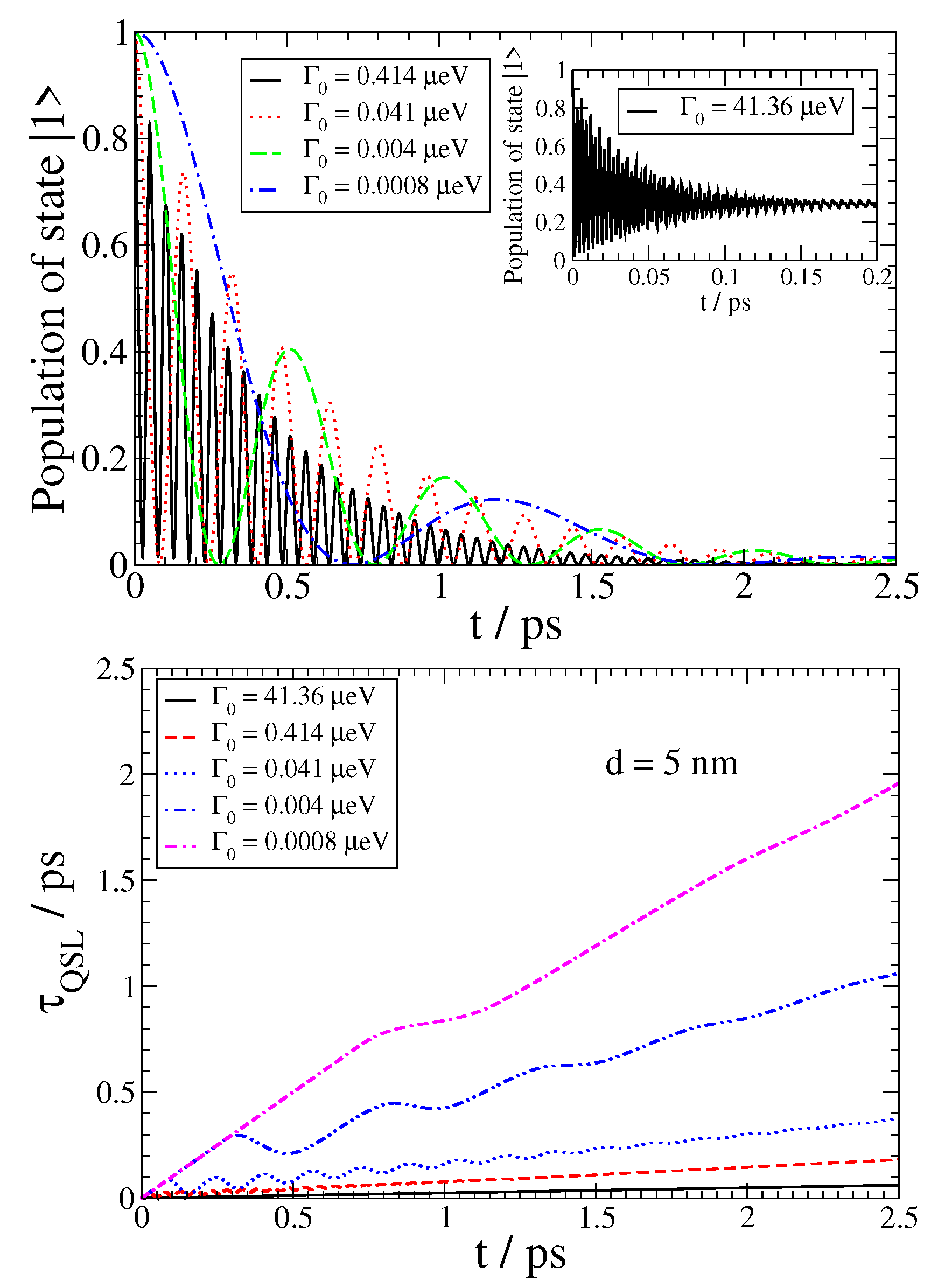
3. Results and Discussion
4. Summary
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Abbreviations
| QE | quantum emitter |
| SE | spontaneous emission |
References
- Baranov, D.G.; Wersäll, M.; Cuadra, J.; Antosiewicz, T.J.; Shegai, T. Novel nanostructures and materials for strong light-matter interactions. ACS Photonics 2018, 5, 24–42. [Google Scholar] [CrossRef]
- Low, T.; Avouris, P. Graphene plasmonics for terahertz to mid-infrared applications. ACS Nano 2014, 8, 1086–1101. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- García de Abajo, F.J. Graphene plasmonics: Challenges and opportunities. ACS Photonics 2014, 1, 135–152. [Google Scholar] [CrossRef] [Green Version]
- Koppens, F.H.L.; Chang, D.E.; García de Abajo, F.J. Graphene plasmonics: A platform for strong light–matter interactions. Nano Lett. 2011, 11, 3370–3377. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Karanikolas, V.D.; Marocico, C.A.; Bradley, A.L. Tunable and long-range energy transfer efficiency through a graphene nanodisk. Phys. Rev. B 2016, 93, 035426. [Google Scholar] [CrossRef] [Green Version]
- Sanders, S.; May, A.; Alabastri, A.; Manjavacas, A. Extraordinary enhancement of quadrupolar transitions using nanostructured graphene. ACS Photonics 2018, 5, 3282–3290. [Google Scholar] [CrossRef]
- Cox, J.D.; García de Abajo, F.J. Nonlinear atom-plasmon interactions enabled by nanostructured graphene. Phys. Rev. Lett. 2018, 121, 257403. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Dung, H.T.; Knöll, L.; Welsch, D.-G. Spontaneous decay in the presence of dispersing and absorbing bodies: General theory and application to a spherical cavity. Phys. Rev. A 2000, 62, 053804. [Google Scholar] [CrossRef] [Green Version]
- Breuer, H.-P.; Laine, E.-M.; Piilo, J. Measure for the degree of non-Markovian behaviour of quantum processes in open systems. Phys. Rev. Lett. 2009, 103, 210401. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Rivas, A.; Huelga, S.F.; Plenio, M.B. Entanglement and Non-Markovianity of quantum evolutions. Phys. Rev. Lett. 2010, 105, 050403. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Deffner, S.; Lutz, E. Quantum speed limit for non-Markovian dynamics. Phys. Rev. Lett. 2013, 111, 010402. [Google Scholar] [CrossRef] [Green Version]
- Thanopulos, I.; Karanikolas, V.; Paspalakis, E. Non-Markovian spontaneous emission dynamics of a quantum emitter near a transition-metal dichalcogenide layer. IEEE J. Select. Top. Quant. Electron. 2021, 27, 6700108. [Google Scholar] [CrossRef]
- Thanopulos, I.; Blekos, K.; Kalozoumis, P.; Karanikolas, V.; Paspalakis, E. Memory effects and quantum speedup for a quantum emitter near a molybdenum disulfide nanodisk. Phys. E Low-Dimens. Syst. Nanostruct. 2021, 133, 114780. [Google Scholar] [CrossRef]
- Yang, C.-J.; An, J.-H. Suppressed dissipation of a quantum emitter coupled to surface plasmon polaritons. Phys. Rev. B 2017, 95, 161408(R). [Google Scholar] [CrossRef] [Green Version]
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Thanopulos, I.; Karanikolas, V.; Paspalakis, E. Strong Light–Matter Interaction of a Quantum Emitter near a Graphene Nanodisk. Mater. Proc. 2022, 9, 17. https://doi.org/10.3390/materproc2022009017
Thanopulos I, Karanikolas V, Paspalakis E. Strong Light–Matter Interaction of a Quantum Emitter near a Graphene Nanodisk. Materials Proceedings. 2022; 9(1):17. https://doi.org/10.3390/materproc2022009017
Chicago/Turabian StyleThanopulos, Ioannis, Vasilios Karanikolas, and Emmanuel Paspalakis. 2022. "Strong Light–Matter Interaction of a Quantum Emitter near a Graphene Nanodisk" Materials Proceedings 9, no. 1: 17. https://doi.org/10.3390/materproc2022009017
APA StyleThanopulos, I., Karanikolas, V., & Paspalakis, E. (2022). Strong Light–Matter Interaction of a Quantum Emitter near a Graphene Nanodisk. Materials Proceedings, 9(1), 17. https://doi.org/10.3390/materproc2022009017








