Long-Term Slope Stability of Abandoned Mine Lake—Numerical Modelling and Risk Assessment †
Abstract
:1. Introduction and Objective
2. Long-Term Slope Stability
2.1. Geometric and Geologic Data
2.2. Geotechnical Data
2.3. Hydraulic Data
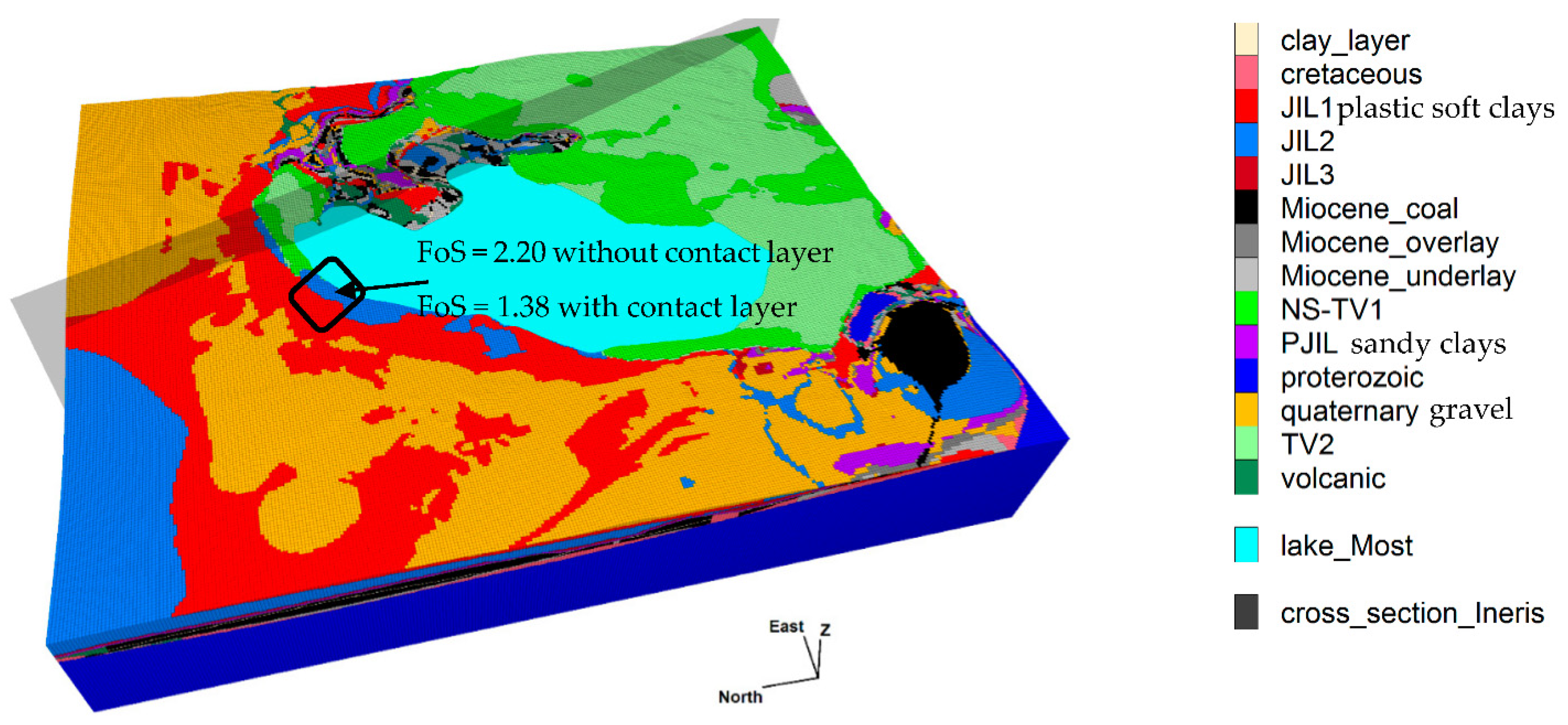
3. Results
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Szafranski, C.; Stachowski, P.; Kozaczyk, P. Influence of meteorological conditions on water balance of soils created from post-mining grounds. In Proceedings of the 5th National Polish Scientific Conference on Complex and Detailed Problems of Environmental Engineering, Warsaw, Poland, 24–27 May 2001. [Google Scholar]
- Vinzelberg, G.; Dahmen, D. The Inden Residual Lake and the Federal Autobahn A44n—Geotechnical Requirements to Be Met by Large-Scale Structures in the Rhenish Lignite Mining Area. In Proceedings of the 12th International Symposium Continuous Surface Mining—Aachen 2014; Springer: Cham, Switzerland, 2014; pp. 91–100. [Google Scholar] [CrossRef]
- Žižka, L.; Burda, J. Post Exploitation Lakes. In Zpravodaj Hnědé Uhlí; Brown Coal Research Institute: Budovatelů, Czech Republic, 2021; pp. 11–46. [Google Scholar]
- Robertson, P.K.; Campanella, R.G. Interpretation of cone penetration tests—Part I (sand). Can. Geotech. J. 1983, 20, 718–733. [Google Scholar] [CrossRef]
- Kulhawy, F.H.; Mayne, P.W. Manual on Estimating Soil Properties for Foundation Design; Report EL-6800; Electric Power Research Institute, EPRI: Washington, DC, USA, 1990. [Google Scholar]
- Mayne, P.W. In situ test calibrations for evaluating soil parameters. In Proceedings of the Characterization and Engineering Properties of Natural Soils II, Singapore, 29 November–1 December 2006. [Google Scholar]
- Motaghedi, H.; Eslami, A. Analytical Approach for determination of soil shear strength parameters from CPT and CPTu data. Arab. J. Sci. Eng. 2014, 39, 4363–4376. [Google Scholar] [CrossRef]
- Zienkiewicz, O.C.; Humpheson, C.; Lewis, R.W. Associated and non-associated visco-plasticity and plasticity in soil mechanics. Géotechnique 1975, 25, 671–689. [Google Scholar] [CrossRef]
- Itasca. FLAC3D (Fast Lagrangian Analysis of Continua in Three Dimensions) Version 7.0, User’s Guide and Command Reference; Itasca Consulting Group Inc.: Minneapolis, MI, USA, 2019. [Google Scholar]


| Dump Unit/Property | NS + TV1 | TV2 | Contact Layer |
|---|---|---|---|
| cohesion: C (kPa) | mean: 4.59 d + 46.15 min: 3.5 d + 10 LN dist ∊ [0.6, 650]: µLN = 0.0324 d + 4.034 σLN = −0.0063 d + 0.598 | mean: 247.4 min: 4 d − 60 LN dist ∊ [6, 1098]: μLN = 5.31 & σLN = 0.626 | 6.0 |
| friction: ϕ ( ) | mean: −0.323 d + 30.69 min: −0.13 d + 20 LN dist ∊ [7, 44]: μLN = −0.241 d + 28.73 σLN = −0.071 d + 6.074 | mean: 22.7 min: 16.8 BS dist ∊ [8.2, 38.6]: β = 22.019 & γ = 0.244 | 6.0 |
| Young’s modulus: E (MPa) | mean: 3.67 d + 18.51 min: 2.96 d + 5 LN dist ∊ [1, 354]: μLN = 0.037 d + 3.577 σLN = −0.004 d + 0.377 | mean: 193.9 min: 3.6 d − 52 LN dist ∊ [29.6, 636.5]: μLN = 3.855 d + 32.046 σLN = 0.6195 d + 28.295 | 70 |
| saturated density: ρ (t/m3) | mean: 0.0124 d + 1.739 (d ≤ 12.5 m) 0.0035 d + 1.869 (d > 12.5 m) min: 0.0035 d + 2.0 N dist ∊ [1.3, 2.2]: μ = 0.0053 d + 1.813 σ = −0.0008 d + 0.0919 | mean: 2.023 min: 0.0017 d + 2.079 N dist ∊ [1.55, 2.285]: μ = 2.023 & σ = 0.098 | 2.0 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Renaud, V.; Al Heib, M.; Muller, R.; Burda, J. Long-Term Slope Stability of Abandoned Mine Lake—Numerical Modelling and Risk Assessment. Mater. Proc. 2021, 5, 88. https://doi.org/10.3390/materproc2021005088
Renaud V, Al Heib M, Muller R, Burda J. Long-Term Slope Stability of Abandoned Mine Lake—Numerical Modelling and Risk Assessment. Materials Proceedings. 2021; 5(1):88. https://doi.org/10.3390/materproc2021005088
Chicago/Turabian StyleRenaud, Vincent, Marwan Al Heib, Roxane Muller, and Jan Burda. 2021. "Long-Term Slope Stability of Abandoned Mine Lake—Numerical Modelling and Risk Assessment" Materials Proceedings 5, no. 1: 88. https://doi.org/10.3390/materproc2021005088
APA StyleRenaud, V., Al Heib, M., Muller, R., & Burda, J. (2021). Long-Term Slope Stability of Abandoned Mine Lake—Numerical Modelling and Risk Assessment. Materials Proceedings, 5(1), 88. https://doi.org/10.3390/materproc2021005088







