A Mathematical and Software Tool to Estimate the Cell Voltage Distribution and Energy Consumption in Aluminium Electrolysis Cells †
Abstract
:1. Introduction
1.1. Overview
1.2. Electrolysis Cell Principles
2. Electrolysis Cell Voltage Model
2.1. Components of the Cell Voltage
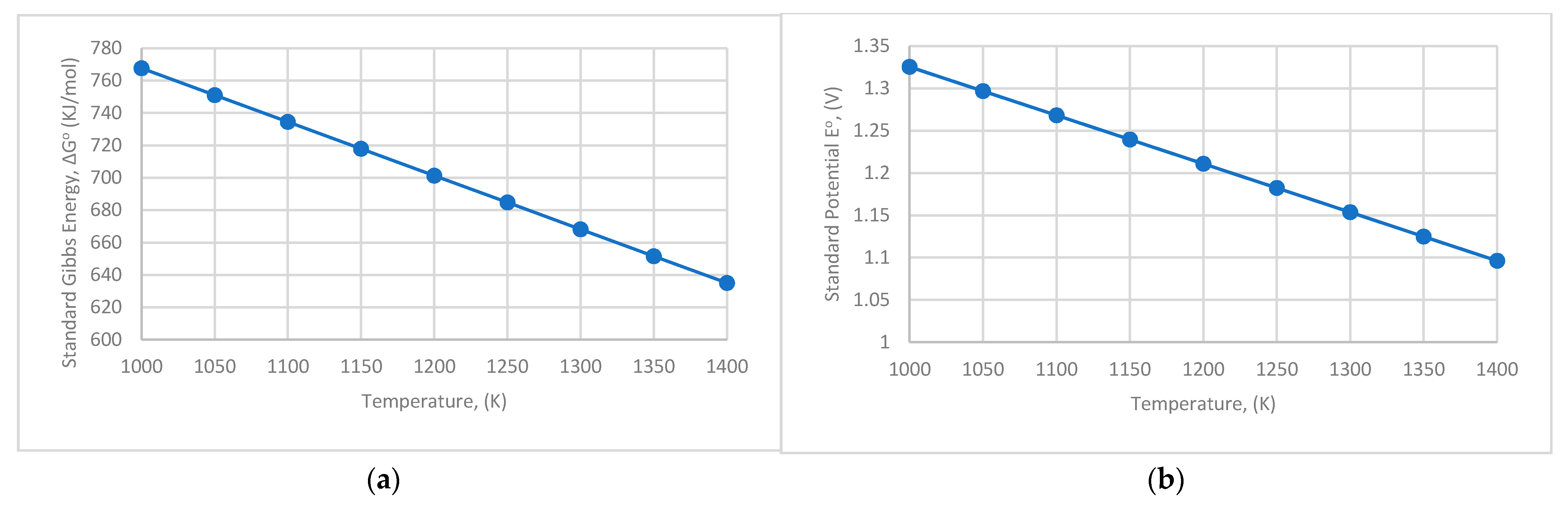
2.2. Ecell the Reversible (Equilibrium) Voltage
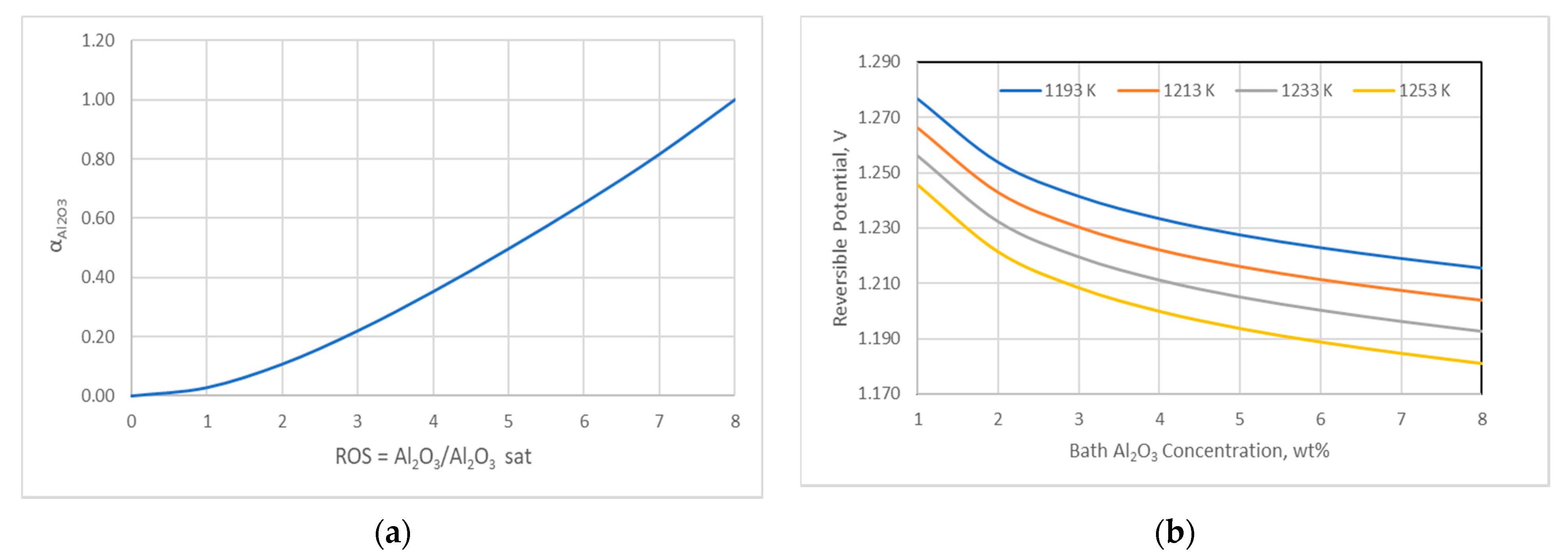
2.3. Concentration Overvoltage
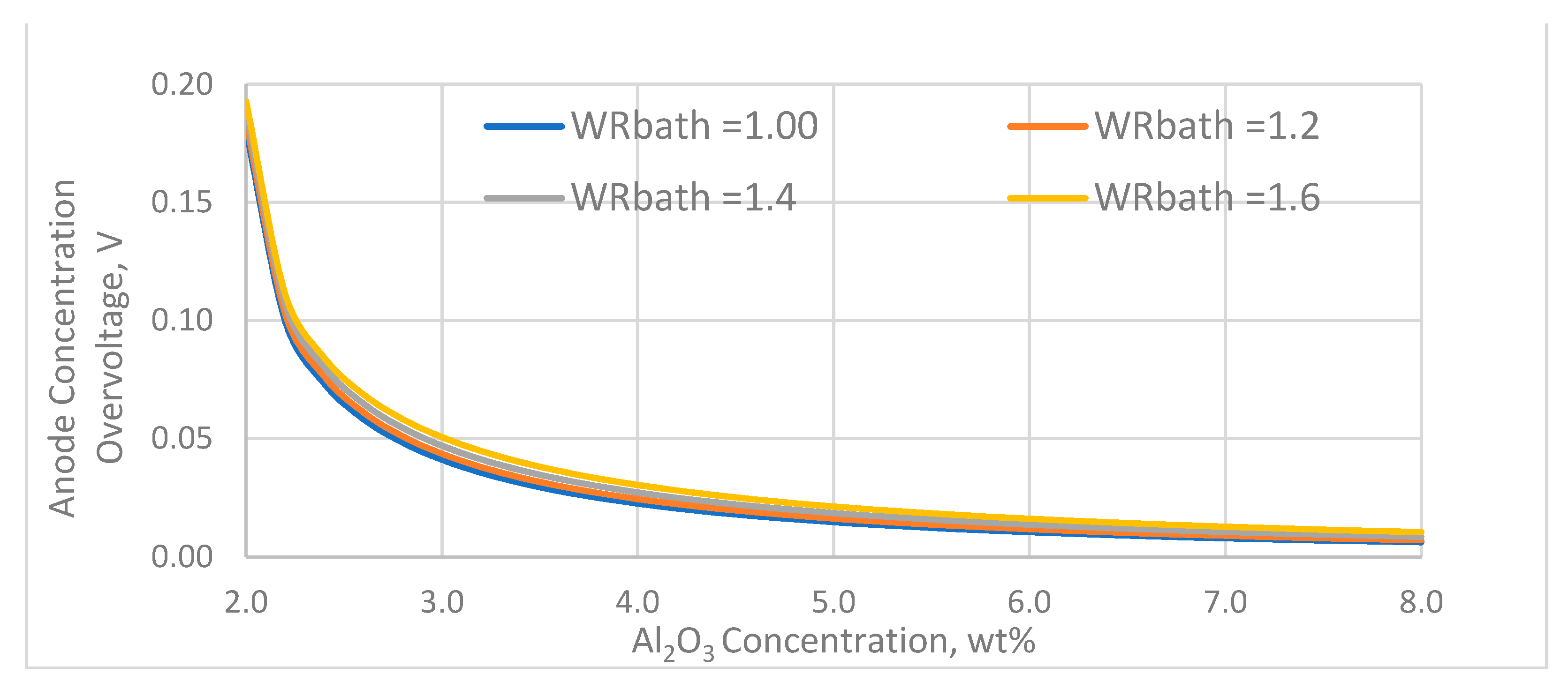
2.3.1. Anode Concentration Overvoltage
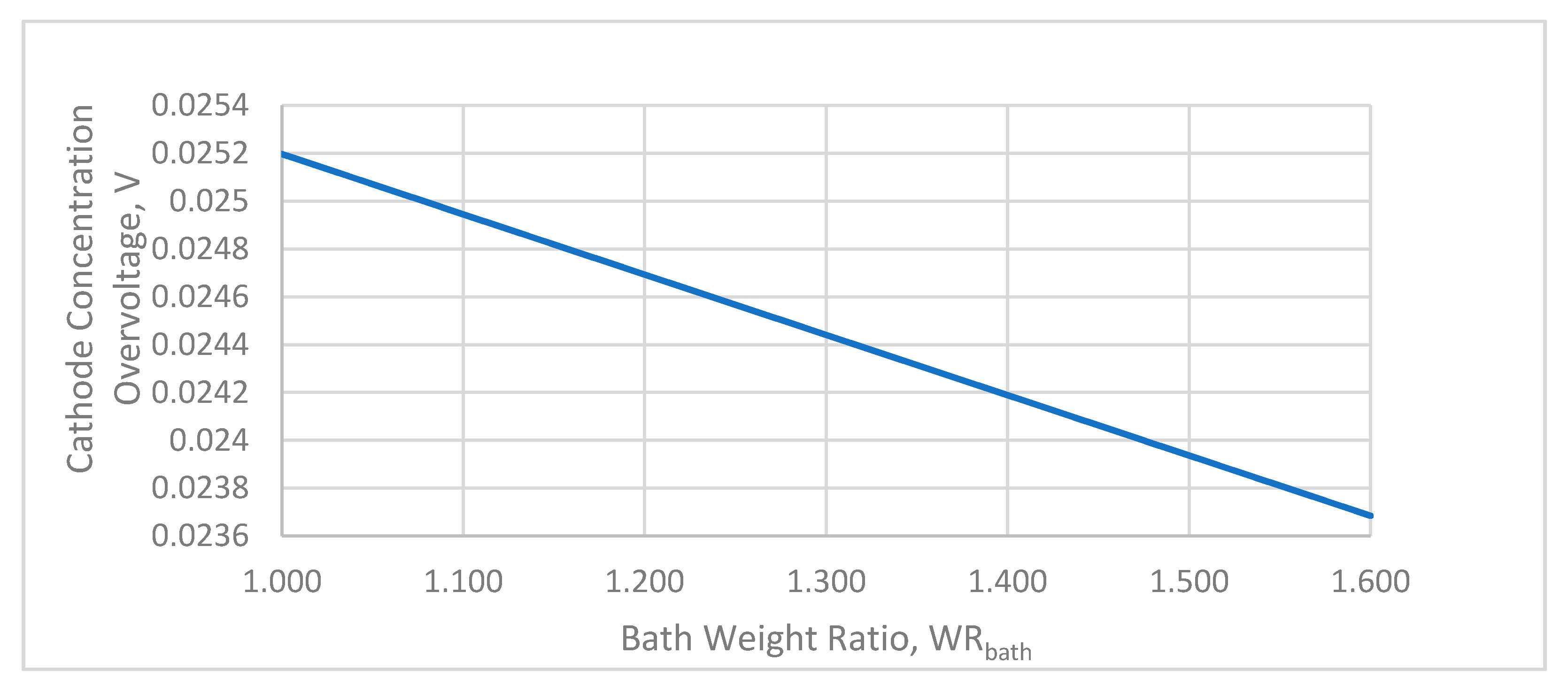
2.3.2. Cathode Concentration Overvoltage
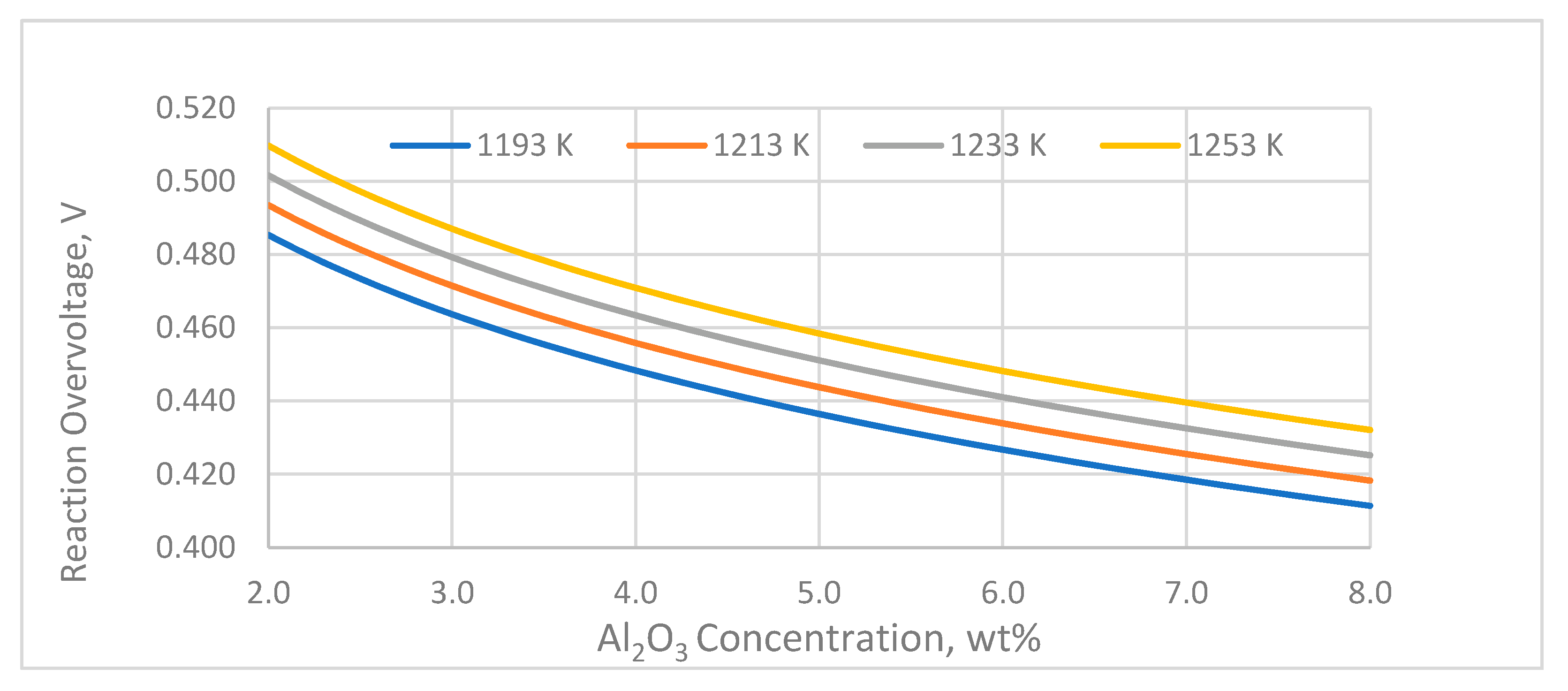
2.3.3. Anode Reaction Overvoltage
2.4. Ohmic Voltage Drops
2.4.1. Electrolyte Voltage Drop
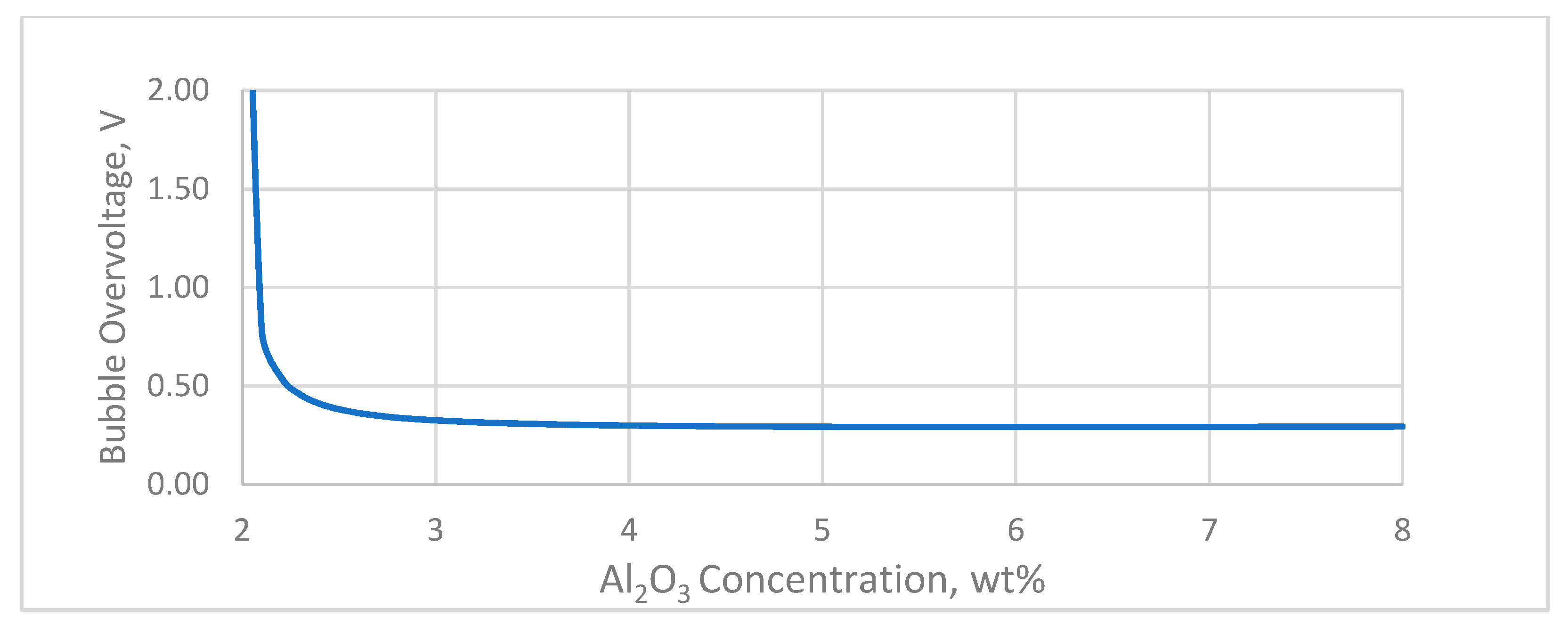
2.4.2. Bubble Voltage Drop
2.5. Cell Element Voltage Drop
2.6. Electrolysis Cell Energy Consumption
2.6.1. Theoretical Energy Consumption
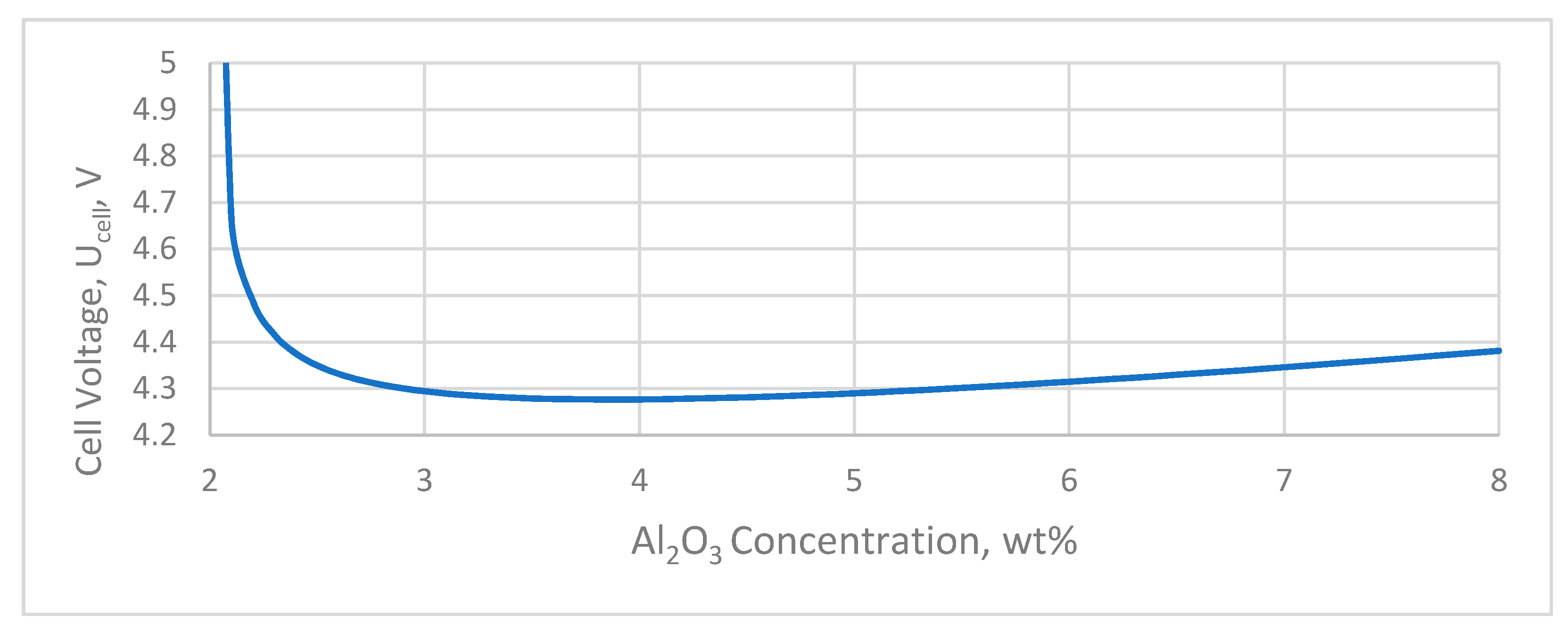
2.6.2. Energy Consumption of an Electrolysis Cell
3. Electrolysis Cell Energy Balance Software
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Abbreviation
| ACD | Anode–cathode distance (cm) |
| CEcel | Current Efficiency |
| MB | the molecular weight of the Chemical species |
| nα | 3 the number of exchange electrons per mole of aluminium produced, |
| F | Faraday constant (96,485 A s mol−1) |
| Icell | Cell current |
| mAl gen | mass of aluminium produced (kg/h) |
| mCO2 gen | mass of carbon dioxide produced (kg/h) |
| mAl2O3 cons | mass of alumina consumed (kg/h) |
| mC cons | mass of carbon consumed (kg/h) |
| Ucell | Cell voltage (V) |
| Rp | Cell pseudoresistance (μOhm) |
| SEC | Specific energy consumption (kWh/kg Al) |
| Ecell | Reversible (equilibrium) voltage (V) |
| ncc | Concentration overvoltage at the cathode (V) |
| naa | Reaction overvoltage at the anode (V) |
| nac | Concentration overvoltage at the anode (V) |
| Ub | Ohmic voltage drop in the electrolyte (V) |
| Ue | Sum of cathode–anode, and other external voltage drops (V) |
| ROS | Saturation degree of the bath in alumina |
| [Al2O3] | Alumina concentration in the electrolysis bath (%) |
| [Al2O3 sat] | Maximum solubility of alumina in the bath (%) |
| κ | Electrical conductivity of the electrolyte (S/cm) |
| CAl2O3 AE | Alumina concentration at which the anode effect occurs |
References
- Drengstig, T.; Ljungquist, D.; Foss, B.A. On the AlF/sub 3/ and temperature control of an aluminum electrolysis cell. IEEE Trans. Control Syst. Technol. 1998, 6, 157–171. [Google Scholar] [CrossRef]
- Grjotheim, K.; Welch, B.J. Aluminium Smelter Technology, 2nd ed.; Aluminium-Verlag: Düsseldorf, Germany, 1988. [Google Scholar]
- Grjotheim, K.; Kvande, H. Introduction to Aluminum Electrolysis, Understanding the Hall-Héroult Process, 2nd ed.; Aluminium-Verlag: Düsseldorf, Germany, 1993. [Google Scholar]
- Statistics. International Aluminium Institute, London, United Kingdom. Available online: https://www.world-aluminium.org/statistics (accessed on 16 March 2021).
- Totten, G.E.; MacKenzie, D.S. (Eds.) Handbook of Aluminum: Volume 2: Alloy Production and Materials Manufacturing; CRC Press: Boca Raton, FL, USA, 2003. [Google Scholar]
- Pearson, T.G.; Waddington, J. Electrode Reaction in the Aluminium Reduction Cell. Discuss. Faraday Soc. 1947, 1, 307–320. [Google Scholar] [CrossRef]
- Kvande, H.; Haupin, W. Cell voltage in aluminum electrolysis: A practical approach. JOM 2000, 52, 31–37. [Google Scholar] [CrossRef]
- Vassiliadou, V.; Paspaliaris, I.; Stefanidis, D.; Georgantonis, D. Energy Savings in Aluminium Electrolysis by Continual Monitoring and Control of the AlF3 Content of the Cryolitic Melt. In Energy Efficiency in Process Technology; Pilavachi, P.A., Ed.; Springer: Dordrecht, The Netherlands, 1993; Volume 52. [Google Scholar]
- Haupin, W.E. Principles of Aluminum Electrolysis. In Essential Readings in Light Metals; Bearne, G., Dupuis, M., Tarcy, G., Eds.; Springer: Cham, Switzerland, 2013. [Google Scholar] [CrossRef]
- Haupin, W.E. Interpreting the Components of Cell Voltage. In Light Metals; The Minerals, Metals & Materials Society: Warrendale, PA, USA, 1998; pp. 531–537. [Google Scholar]
- Bearne, G.; Dupuis, M.; Tarcy, G. Essential Readings in Light Metals: Volume 2, 1st ed.; Springer: Cham, Switzerland, 2016; ISBN 10-331948575X. Available online: http://books.scholarsportal.info/viewdoc.html?id=/ebooks/ebooks3/springer/2017-08-17/3/9783319481562 (accessed on 10 April 2021).
- Hives, J.; Thonstad, J.; Sterten, Å.; Fellner, P. Electrical Conductivity of Molten Cryolite-based Mixtures Obtained with a Tube-type Cell made of Pyrolitic Boron Nitride. In Light Metals; The Minerals, Metals & Materials Society: Warrendale, PA, USA, 1994; pp. 187–194. [Google Scholar]
- Hyde, T.M.; Welch, B.J. The Gas under Anodes in Aluminium Smelting Cells Part I: Measuring and Modelling Bubble Resistance under Horizontally Oriented Electrodes. In Light Metals; The Minerals, Metals & Materials Society: Warrendale, PA, USA, 1997; pp. 333–340. [Google Scholar]
- Drengstig, T. On Process Model Representation and AlF3 Dynamics of Aluminum Electrolysis Cells. Ph.D. Thesis, Norwegian University of Science and Technology, Trondheim, Norway, 1997. [Google Scholar]

| Input Parameters | |||
|---|---|---|---|
| Parameter | Symbol | Value | Units |
| Temperature | |||
| Bath Temperature | TK | 1243.000 | K |
| TC | 970.000 | °C | |
| Pressure Barometric | Pb atm | 1.000 | atm |
| Pb kPa | 101.325 | kPa | |
| Bath Superheat | ΔTKs | 20.000 | K |
| ΔTCs | 20.000 | °C | |
| Cell Operation Parameters | |||
| Cell Current | Icell | 180,700 | A |
| Cell Current Efficiency | CEcell | 0.9200 | |
| Anodic current density | ia | 0.48 | A/cm2 |
| Cathodic current density | ic | 0.49 | A/cm2 |
| Anode Cathode Distance | |||
| ACD | 3.9 | ||
| Electrolysis Bath Composition | |||
| Alumina | Cbath Al2O3 | 2.46 | wt% |
| Excess Aluminium Fluoride | Cxs bath AlF3 | 10 | wt% |
| Calcium Fluoride | Cbath CaF2 | 4.5 | wt% |
| Magnesium Fluoride | Cbath MgF2 | 0.3 | wt% |
| Potassium Fluoride | Cbath KF | 0.1 | wt% |
| Lithium Fluoride | Cbath LiF | 0.5 | wt% |
| Cell Calculated Voltage Distribution | |||
|---|---|---|---|
| Parameter | Symbol | Value | Units |
| Cell Voltage | Ucell | 4.182 | V |
| Cell Standard Potential at T | E°T | 1.187 | V |
| Cell Reversible Potential | Ecell | 1.224 | V |
| Reaction Overvoltage at Anode | ηaa | 0.516 | V |
| Anode Concentration overvoltage | ηac | 0.0747 | V |
| Bubble Voltage Drop | Ububble | 0.259 | V |
| Ohmic Voltage Drop of Electrolyte | Uohmic | 1.335 | V |
| Cathode Concentration overvoltage | ηcc | 0.033 | V |
| External Voltage | Uext | 0.741 | V |
| Cell Power Consumption | |||
| Parameter | Symbol | Value | Units |
| Cell Voltage | PEcell | 756 | kW |
| Cell Standard Potential at T | PE°T | 214 | kW |
| Cell Reversible Potential | PEcell | 221 | kW |
| Reaction Overvoltage at Anode | Paa | 93 | kW |
| Anode Concentration overvoltage | Pac | 13 | kW |
| Bubble Voltage Drop | Pbubble | 47 | kW |
| Ohmic Voltage Drop of Electrolyte | Pohmic | 241 | kW |
| Cathode Concentration overvoltage | Pcc | 6 | kW |
| External Voltage | Pext | 134 | kW |
| Cell Overall Energy Consumption | |||
| Parameter | Symbol | Value | Units |
| Aluminium Cell Production Theoretical | mAlth | 1455.4 | kg/d |
| Aluminium Cell Production Actual | mAlactual | 1339.0 | kg/d |
| Energy Consumption Theoretical | Wal Gibbs | 3.65 | kWh/kg Al |
| Energy Consumption isothermal | Wal iso | kWh/kg Al | |
| Energy Consumption Actual | Wal actual | 13.546 | kWh/kg Al |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Vassiliadou, V.; Peppas, A.; Taxiarchou, M. A Mathematical and Software Tool to Estimate the Cell Voltage Distribution and Energy Consumption in Aluminium Electrolysis Cells. Mater. Proc. 2021, 5, 116. https://doi.org/10.3390/materproc2021005116
Vassiliadou V, Peppas A, Taxiarchou M. A Mathematical and Software Tool to Estimate the Cell Voltage Distribution and Energy Consumption in Aluminium Electrolysis Cells. Materials Proceedings. 2021; 5(1):116. https://doi.org/10.3390/materproc2021005116
Chicago/Turabian StyleVassiliadou, Vicky, Antonis Peppas, and Maria Taxiarchou. 2021. "A Mathematical and Software Tool to Estimate the Cell Voltage Distribution and Energy Consumption in Aluminium Electrolysis Cells" Materials Proceedings 5, no. 1: 116. https://doi.org/10.3390/materproc2021005116
APA StyleVassiliadou, V., Peppas, A., & Taxiarchou, M. (2021). A Mathematical and Software Tool to Estimate the Cell Voltage Distribution and Energy Consumption in Aluminium Electrolysis Cells. Materials Proceedings, 5(1), 116. https://doi.org/10.3390/materproc2021005116







