Design and Simulation of a Microfluidic Platform for the Encapsulation and Separation of Yeasts Expressing Translocating Peptides †
Abstract
:1. Introduction
2. Materials and Methods
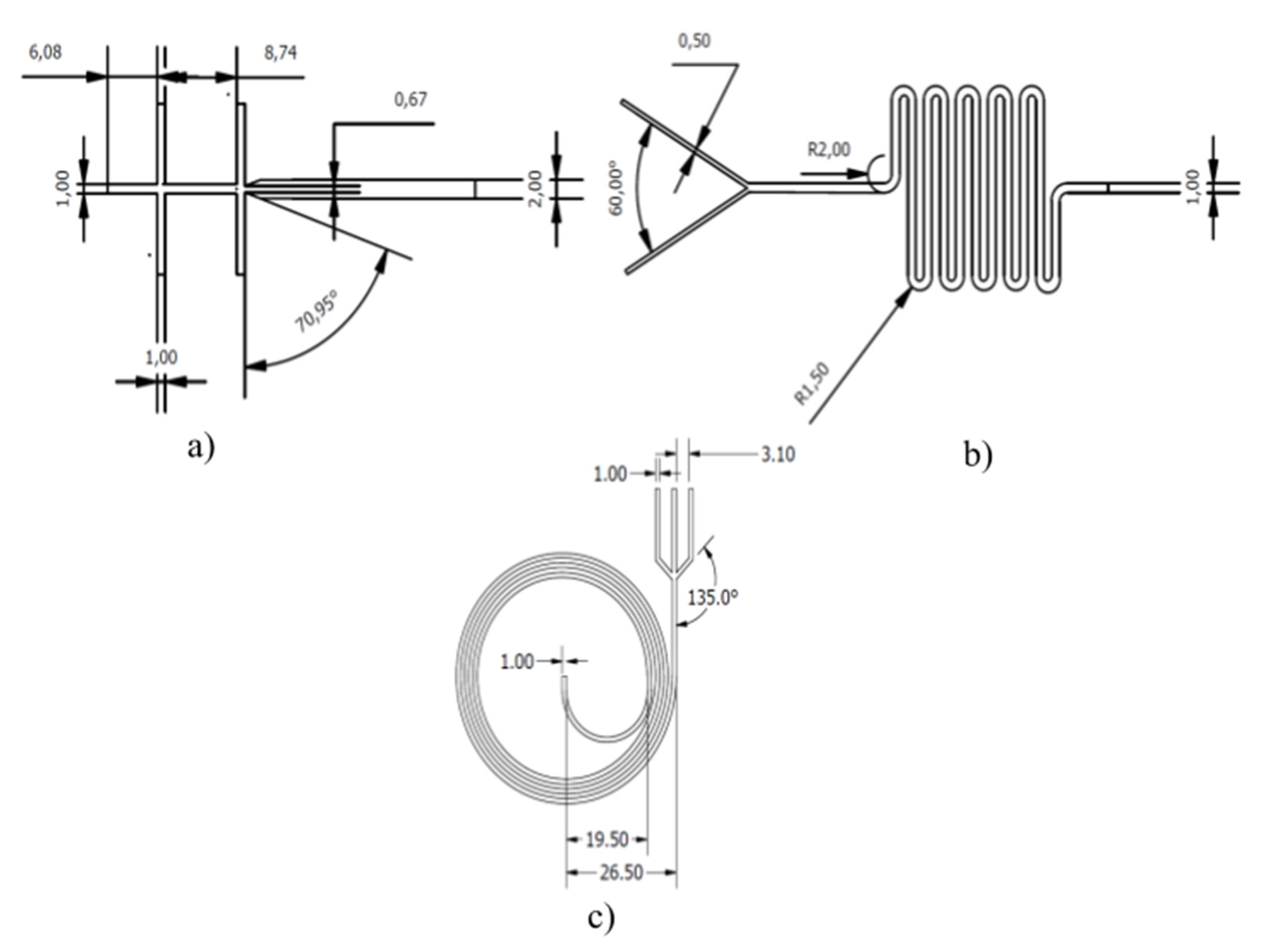
2.1. Microfluidic System Design
2.2. Simulation
3. Results
4. Discussion
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Paulo, F.; Santos, L. Design of experiments for microencapsulation applications: A review. Mater. Sci. Eng. C 2017, 77, 327–1340. [Google Scholar] [CrossRef] [PubMed]
- Hendrawati, T.Y.; Sari, A.M.; Rahman, M.I.S.; Nugrahani, R.A.; Siswahyu, A. Microencapsulation Techniques of Herbal Compounds for Raw Materials in Food Industry, Cosmetics and Pharmaceuticals. Microencapsul. Process. Technol. Ind. Appl. 2019, 1–15. [Google Scholar] [CrossRef]
- Ozkan, G.; Franco, P.; de Marco, I.; Xiao, J.; Capanoglu, E. A review of microencapsulation methods for food antioxidants: Principles, advantages, drawbacks and applications. Food Chem. 2019, 272, 494–506. [Google Scholar] [CrossRef] [PubMed]
- Ye, Q.; Georges, N.; Selomulya, C. Microencapsulation of active ingredients in functional foods: From research stage to commercial food products. Trends Food Sci. Technol. 2018, 78, 167–179. [Google Scholar] [CrossRef]
- Shishir, M.R.I.; Xie, L.; Sun, C.; Zheng, X.; Chen, W. Advances in micro and nano-encapsulation of bioactive compounds using biopolymer and lipid-based transporters. Trends Food Sci. Technol. 2018, 78, 34–60. [Google Scholar] [CrossRef]
- De Barros Fernandes, R.V.; Botrel, D.A.; Silva, E.K.; Borges, S.V.; de Oliveira, C.R.; Yoshida, M.I.; de Andrade Feitosa, J.P.; de Paula, R.C.M. Cashew gum and inulin: New alternative for ginger essential oil microencapsulation. Carbohydr. Polym. 2016, 153, 133–142. [Google Scholar] [CrossRef]
- Hoyos-Leyva, J.D.; Bello-Pérez, L.A.; Alvarez-Ramirez, J.; Garcia, H.S. Microencapsulation using starch as wall material: A review. Food Rev. Int. 2018, 34, 148–161. [Google Scholar] [CrossRef]
- Pieczykolan, E.; Kurek, M.A. Use of guar gum, gum arabic, pectin, beta-glucan and inulin for microencapsulation of anthocyanins from chokeberry. Int. J. Biol. Macromol. 2019, 129, 665–671. [Google Scholar] [CrossRef]
- Suganya, V.; Anuradha, V. Microencapsulation and Nanoencapsulation: A Review. Int. J. Pharm. Clin. Res. 2017, 9, 233–239. [Google Scholar] [CrossRef]
- Mu, R.J.; Yuan, Y.; Wang, L.; Ni, Y.; Li, M.; Chen, H.; Pang, J. Microencapsulation of Lactobacillus acidophilus with konjac glucomannan hydrogel. Food Hydrocoll. 2018, 76, 42–48. [Google Scholar] [CrossRef]
- Sharif, N.; Khoshnoudi-Nia, S.; Jafari, S.M. Nano/microencapsulation of anthocyanins; a systematic review and meta-analysis. Food Res. Int. 2020, 132, 109077. [Google Scholar] [CrossRef] [PubMed]
- Meng, F.; Wang, S.; Wang, Y.; Liu, H.; Huo, X.; Ma, H.; Ma, Z.; Xiong, H. Microencapsulation of oxalic acid via oil-in-oil (O/O) emulsion solvent evaporation. Powder Technol. 2017, 320, 405–411. [Google Scholar] [CrossRef]
- Otálora, M.C.; Carriazo, J.G.; Iturriaga, L.; Osorio, C.; Nazareno, M.A. Encapsulating betalains from Opuntia ficus-indica fruits by ionic gelation: Pigment chemical stability during storage of beads. Food Chem. 2016, 202, 373–382. [Google Scholar] [CrossRef] [PubMed]
- Timilsena, Y.P.; Akanbi, T.O.; Khalid, N.; Adhikari, B.; Barrow, C.J. Complex coacervation: Principles, mechanisms and applications in microencapsulation. Int. J. Biol. Macromol. 2019, 121, 1276–1286. [Google Scholar] [CrossRef] [PubMed]
- Ma, L.; Shang, Y.; Zhu, Y.; Zhang, X.; Jingjing, E.; Zhao, L.; Wang, J. Study on microencapsulation of Lactobacillus plantarum LIP-1 by emulsification method. J. Food Process Eng. 2020, 43. [Google Scholar] [CrossRef]
- Zhang, Y.; Rochefort, D. Characterisation and applications of microcapsules obtained by interfacial polycondensation. J. Microencapsul. 2012, 29, 636–649. [Google Scholar] [CrossRef]
- Campaña, A.L.; Sotelo, D.C.; Oliva, H.A.; Aranguren, A.; Ornelas-Soto, N.; Cruz, J.C.; Osma, J.F. Fabrication and characterization of a low-cost microfluidic system for the manufacture of alginate-lacasse microcapsules. Polymers 2020, 12, 1158. [Google Scholar] [CrossRef]
- Lee, S.; Sundararajan, N. Microfabrication and Microfluidics; Artech House: Boston, MA, USA, 2010. [Google Scholar]
- Kastner, E.; Kaur, R.; Lowry, D.; Moghaddam, B.; Wilkinson, A.; Perrie, Y. High-throughput manufacturing of size-tuned liposomes by a new microfluidics method using enhanced statistical tools for characterization. Int. J. Pharm. 2014, 477, 361–368. [Google Scholar] [CrossRef]
- Trantidou, T.; Friddin, M.S.; Salehi-Reyhani, A.; Ces, O.; Elani, Y. Droplet microfluidics for the construction of compartmentalised model membranes. Lab Chip 2018, 18, 2488–2509. [Google Scholar] [CrossRef]
- Fang, A.; Cathala, B. Smart swelling biopolymer microparticles by a microfluidic approach: Synthesis, in situ encapsulation and controlled release. Colloids Surf. B Biointerfaces 2011, 82, 81–86. [Google Scholar] [CrossRef]
- Matalanis, A.; Jones, O.G.; McClements, D.J. Structured biopolymer-based delivery systems for encapsulation, protection, and release of lipophilic compounds. Food Hydrocoll. 2011, 25, 1865–1880. [Google Scholar] [CrossRef]
- Tan, Y.C.; Hettiarachchi, K.; Siu, M.; Pan, Y.R.; Lee, A.P. Controlled microfluidic encapsulation of cells, proteins, and microbeads in lipid vesicles. J. Am. Chem. Soc. 2006, 128, 5656–5658. [Google Scholar] [CrossRef] [PubMed]
- Matosevic, S. Synthesizing artificial cells from giant unilamellar vesicles: State-of-the art in the development of microfluidic technology. BioEssays 2012, 34, 992–1001. [Google Scholar] [CrossRef]
- Kitson, P.J.; Rosnes, M.H.; Sans, V.; Dragone, V.; Cronin, L. Configurable 3D-Printed millifluidic and microfluidic ‘lab on a chip’ reactionware devices. Lab Chip 2012, 12, 3267–3271. [Google Scholar] [CrossRef] [PubMed]
- Garcia, M.; Pennathur, S. A model for inertial particles in curvilinear flows. Microfluid. Nanofluid. 2019, 23, 1–9. [Google Scholar] [CrossRef]

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gómez, S.C.; Quezada, V.; Osma, J.F.; Reyes, L.H.; Cruz, J.C. Design and Simulation of a Microfluidic Platform for the Encapsulation and Separation of Yeasts Expressing Translocating Peptides. Mater. Proc. 2021, 4, 64. https://doi.org/10.3390/IOCN2020-07799
Gómez SC, Quezada V, Osma JF, Reyes LH, Cruz JC. Design and Simulation of a Microfluidic Platform for the Encapsulation and Separation of Yeasts Expressing Translocating Peptides. Materials Proceedings. 2021; 4(1):64. https://doi.org/10.3390/IOCN2020-07799
Chicago/Turabian StyleGómez, Saúl C., Valentina Quezada, Johann F. Osma, Luis H. Reyes, and Juan C. Cruz. 2021. "Design and Simulation of a Microfluidic Platform for the Encapsulation and Separation of Yeasts Expressing Translocating Peptides" Materials Proceedings 4, no. 1: 64. https://doi.org/10.3390/IOCN2020-07799
APA StyleGómez, S. C., Quezada, V., Osma, J. F., Reyes, L. H., & Cruz, J. C. (2021). Design and Simulation of a Microfluidic Platform for the Encapsulation and Separation of Yeasts Expressing Translocating Peptides. Materials Proceedings, 4(1), 64. https://doi.org/10.3390/IOCN2020-07799








