Predicting the Flexural Modulus of Variable Pitch Angle, Porous Bouligand Structured 3D Printed Polymer †
Abstract
1. Introduction
2. Materials and Methods
2.1. Three-Point Bending
2.2. Models to Predict the Elastic Modulus
3. Results and Discussion
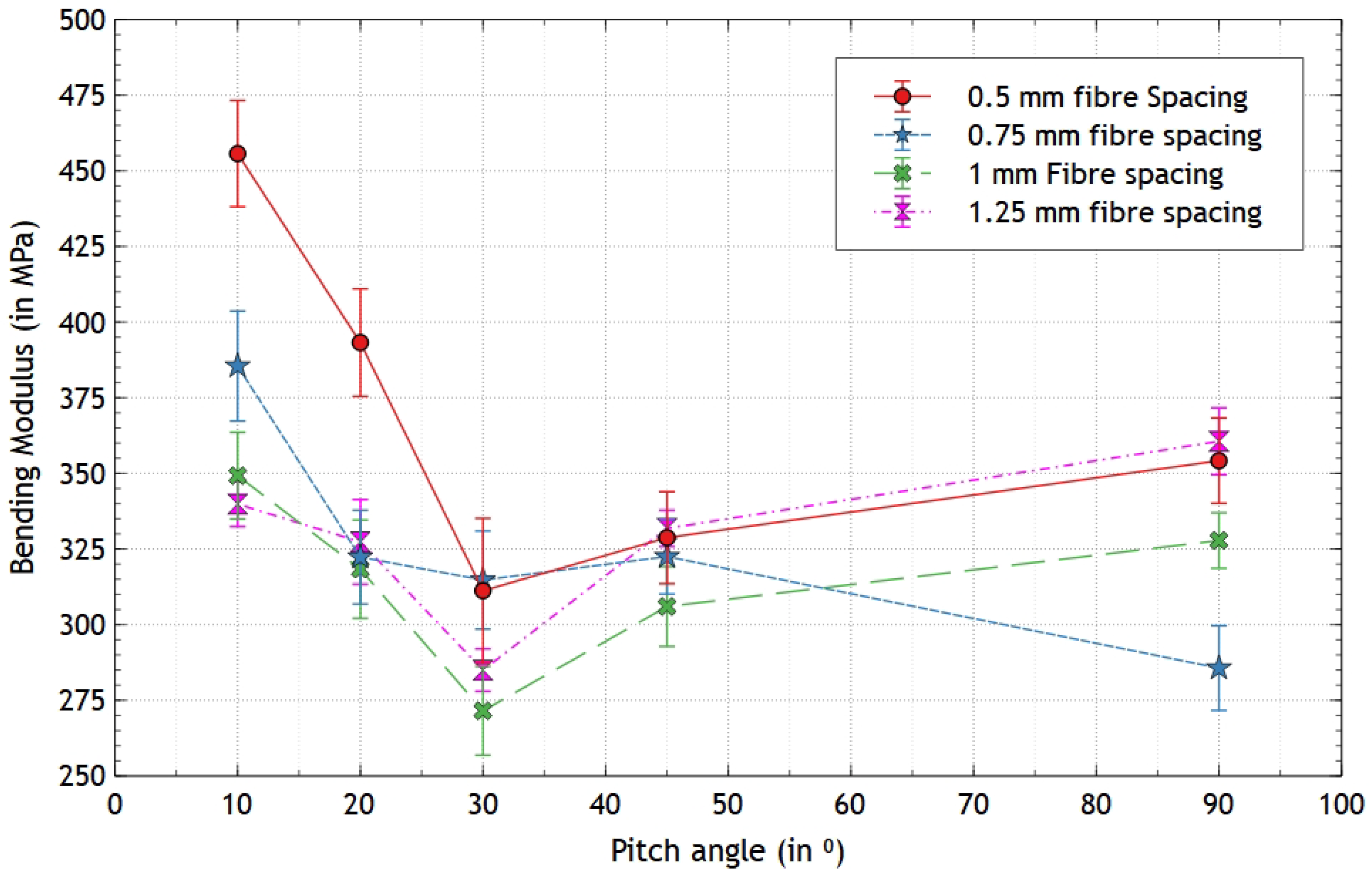
3.1. Flexural Properties
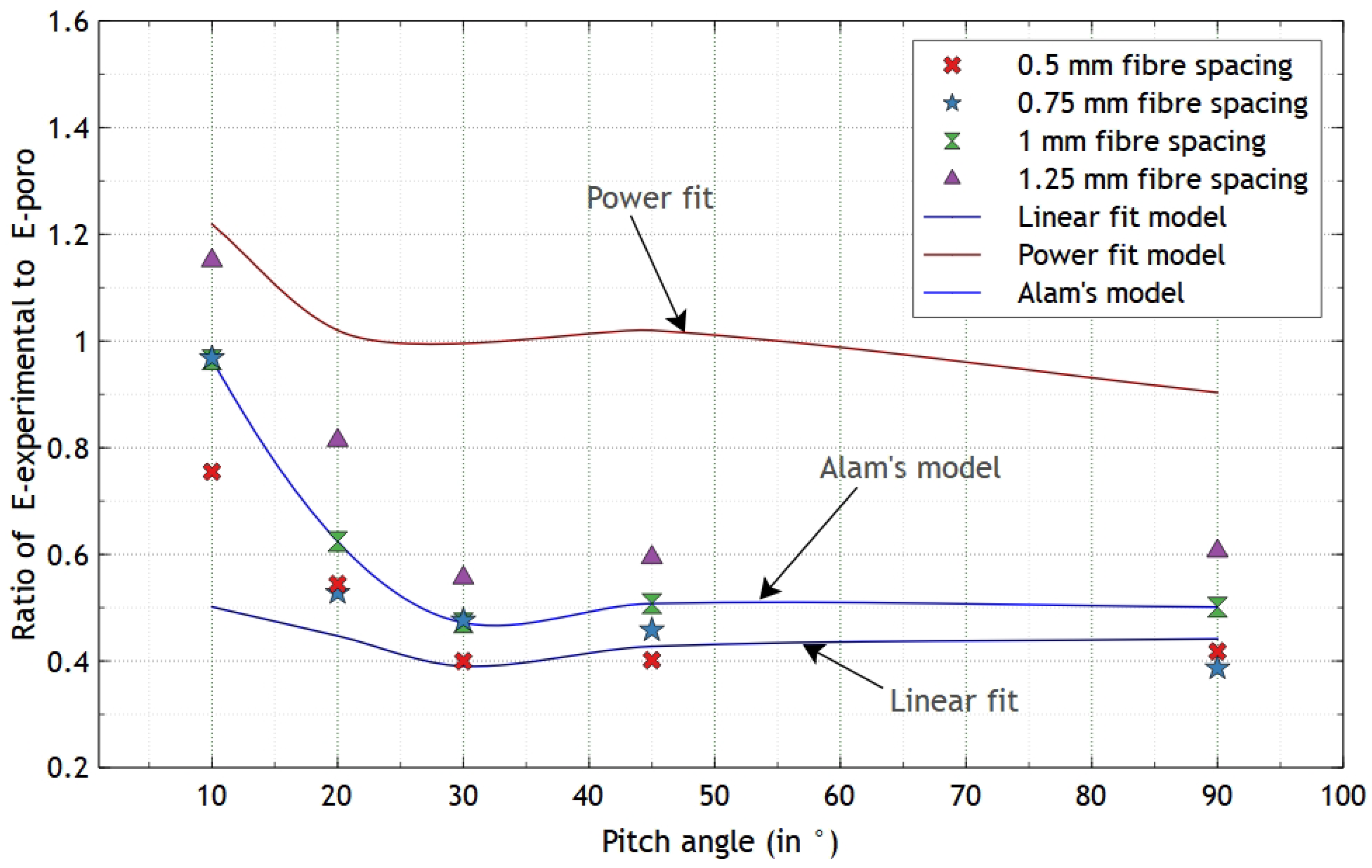
3.2. Elastic Modulus Prediction
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Sanchez, C.; Arribart, H.; Guille, M.M. Biomimetism and bioinspiration as tools for the design of innovative materials and systems. Nat. Mater. 2005, 4, 277–288. [Google Scholar] [CrossRef] [PubMed]
- Fratzl, P. Biomimetic materials research: What can we really learn from nature’s structural materials? J. R. Soc. Interface 2007, 4, 637–642. [Google Scholar] [CrossRef]
- Jeronimidis, G. Wood, one of nature’s challenging composites. Symp. Soc. Exp. Biol. 1980, 34, 169–182. [Google Scholar] [PubMed]
- Kemp, M. Structural intuitions and metamorphic thinking in art, architecture and science. In Proceedings of the Metamorph—9th International Architecture Exhibition, Venice, Italy, 12 September–7 November 2004; pp. 30–43. [Google Scholar]
- Milwich, M.; Speck, T.; Speck, O.; Stegmaier, T.; Planck, H. Biomimetics and technical textiles: Solving engineering problems with the help of nature’s wisdom. Am. J. Bot. 2006, 93, 1455–1465. [Google Scholar] [CrossRef] [PubMed]
- Natarajan, B.; Gilman, J.W. Bioinspired Bouligand cellulose nanocrystal composites: A review of mechanical properties. Philos. Trans. R. Soc. Math. Phys. Eng. Sci. 2018, 376, 20170050. [Google Scholar] [CrossRef] [PubMed]
- Lu, T.; Shen, H.S.; Chen, H.W.X.; Feng, M. Optimization Design and Nonlinear Bending of Bio-Inspired Helicoidal Composite Laminated Plates. Materials 2023, 16, 4550. [Google Scholar] [CrossRef]
- Alam, P.; Sanka, I.; Alam, L.P.; Wijaya, S.; Sintya, E.; Handayani, N.S.N.; ller, A. The snapping shrimp dactyl plunger: A thermomechanical damage-tolerant sandwich composite. Zoology 2018, 126, 1–10. [Google Scholar] [CrossRef]
- Alam, P. Porous Particle-Polymer Composites. In Advances in Composite Materials; Tesinova, P., Ed.; IntechOpen: Rijeka, Croatia, 2011; Chapter 2. [Google Scholar] [CrossRef]
- Yu, Z.; Du, X.; Liu, R.; Xie, Q.; Zhang, X.; Zhu, Q. Increasing the Compressive Strength of Helicoidal Laminates after Low-Velocity Impact upon Mixing with 0 Orientation Plies and Its Analysis. Matrerials 2023, 16, 4599. [Google Scholar] [CrossRef] [PubMed]
- Yang, Y.; Chen, Z.; Song, X.; Zhang, Z.; Zhang, J.; Shung, K.K.; Zhou, Q.; Chen, Y. Biomimetic Anisotropic Reinforcement Architectures by Electrically Assisted Nanocomposite 3D Printing. Adv. Mater. 2017, 29, 1–8. [Google Scholar] [CrossRef] [PubMed]
- Danfeng, W.; Chen, D.D.; Chen, Z. Recent Progress in 3D Printing of Bioinspired Structures. Front. Mater. 2020, 7, 1–10. [Google Scholar] [CrossRef]
- Ko, K.; Jin, S.; Lee, S.E.; Hong, J.W. Impact resistance of nacre-like composites diversely patterned by 3D printing. Compos. Struct. 2020, 238, 111951. [Google Scholar] [CrossRef]
- Ko, K.; Jin, S.; Lee, S.E.; Lee, I.; Hong, J.W. Bio-inspired bimaterial composites patterned using three-dimensional printing. Compos. Part B Eng. 2019, 165, 594–603. [Google Scholar] [CrossRef]
- Guan, L.; Peng, W.; Wen, R.N.; Fan, J.; Le Ferrand, H. Izod impact resistance of 3D printed discontinuous fibrous composites with Bouligand structure. Npg Asia Matrerials 2023, 15, 60. [Google Scholar] [CrossRef]
- Sun, Y.; Tian, W.; Zhang, T.; Chen, P.; Li, M. Strength and toughness enhancement in 3d printing via bioinspired tool path. Mater. Des. 2020, 185, 108239. [Google Scholar] [CrossRef]
- ASTM D790-17; Standard Test Method for Flexural Properties of Unidirectional and Reinforced Plastics and Electric Insulating Materials. ASTM: West Conshohochen, PA, USA, 2017.
- Alam, P. Composites Engineering: An A–Z Guide; IOP Publishing: Bristol, UK, 2021; pp. 2053–2563. [Google Scholar] [CrossRef]
- Brown, S.D.; Biddulph, R.B.; Wilcox, P.D. A Strength–Porosity Relation Involving Different Pore Geometry and Orientation. J. Am. Ceram. Soc. 1964, 47, 320–322. [Google Scholar] [CrossRef]
- Maitra, A.K.; Phani, K.K. Ultrasonic evaluation of elastic parameters of sintered powder compacts. J. Mater. Sci. 1994, 29, 4415–4419. [Google Scholar] [CrossRef]
- Wagh, A.; Singh, J.; Poeppel, R. Dependence of ceramic fracture properties on porosity. J. Mater. Sci. 1993, 28, 3589–3593. [Google Scholar] [CrossRef]
- Hull, D.; Clyne, T. An Introduction to Composite Materials; Cambridge University Press: Cambridge, UK, 1996. [Google Scholar]
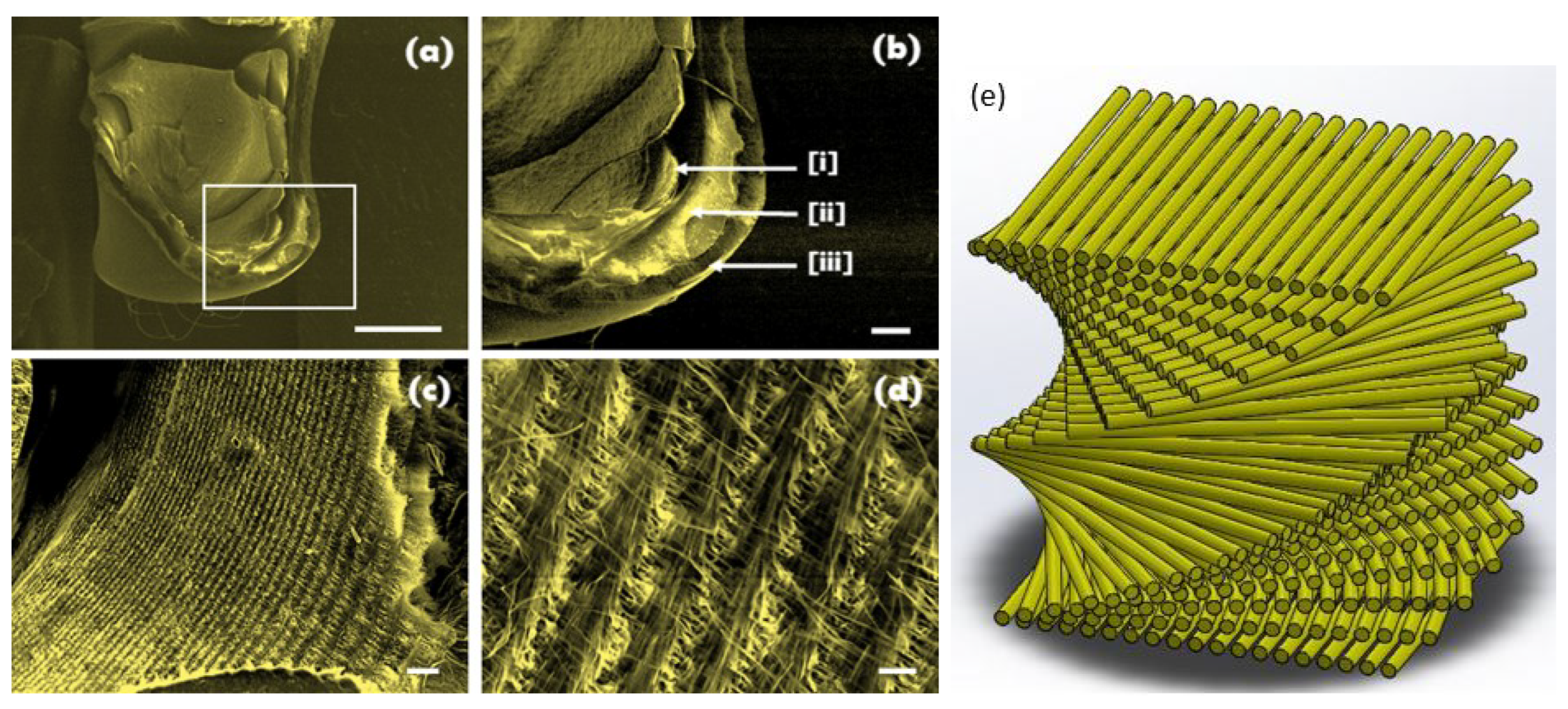
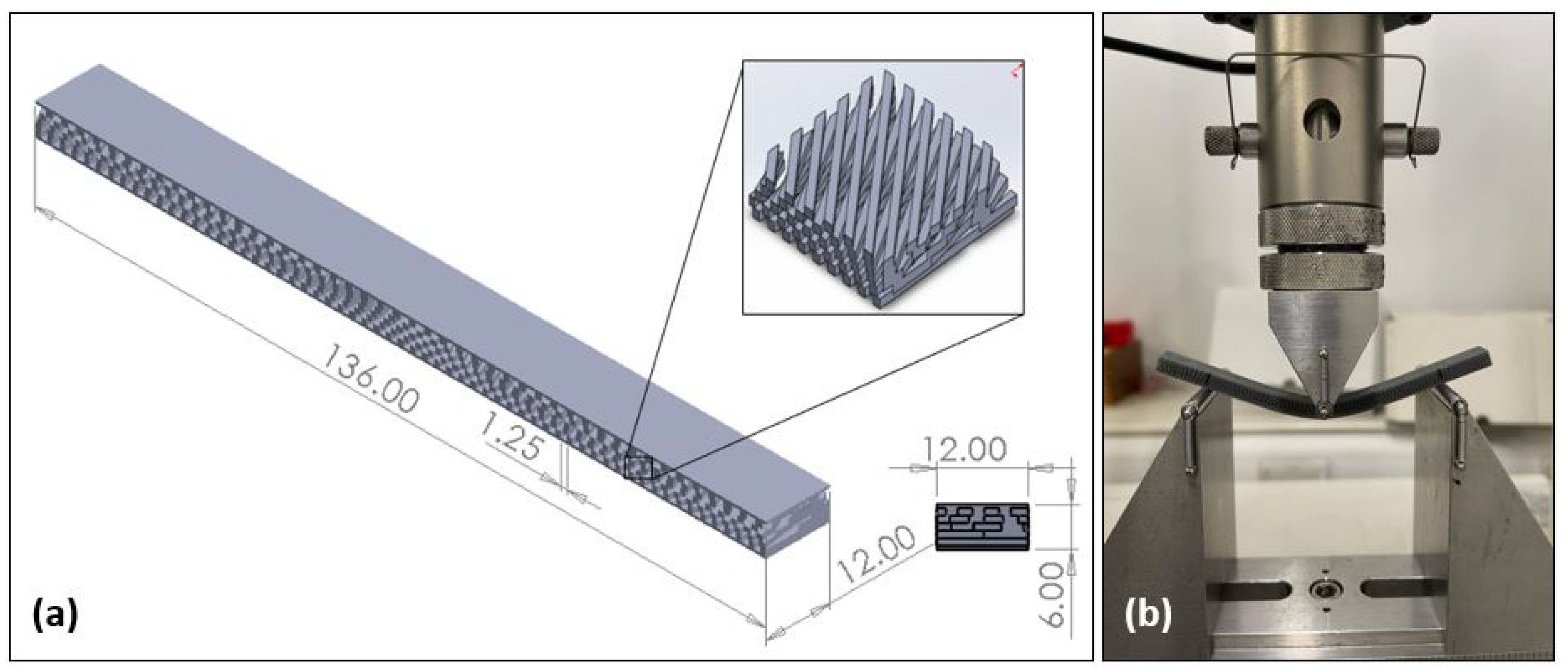
| Description | Printing Direction | Fibre Spacing (in mm) |
|---|---|---|
| Helicoidal, 10° ply angle | 0°, 10°, 20°, 30°, 40° | 0.5, 0.75, 1, 1.25 |
| Helicoidal, 20° ply angle | 0°, 20°, 40°, 60°, 80° | 0.5, 0.75, 1, 1.25 |
| Helicoidal, 30° ply angle | 0°, 30°, 60°, 90°, 120° | 0.5, 0.75, 1, 1.25 |
| Quasi-isotropic | 0°, 45°, 90°, 135°, 180° | 0.5, 0.75, 1, 1.25 |
| Cross-ply | 0°, 90°, 180°, 270°, 360° | 0.5, 0.75, 1, 1.25 |
| Properties | Value | Remark |
|---|---|---|
| Young’s Modulus | 1.2 GPa | ASTM 638-14 |
| Ultimate Tensile strength | 46 MPa | ASTM 638-14 |
| Density | 1.11 g/mm3 | |
| Poisson’s Ratio | 0.3 |
| Fibre Spacing (mm) | Linear Fit | Power Fit | Modified Alam Fit |
|---|---|---|---|
| 0.5 | 0.7940 | 0.4172 | 1.1055 |
| 0.75 | 0.8743 | 0.7503 | 0.9567 |
| 1 | 1.0704 | 1.1518 | 0.923 |
| 1.25 | 1.1587 | 1.1382 | 1.1693 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Patil, P.S.; McCarthy, E.D.; Alam, P. Predicting the Flexural Modulus of Variable Pitch Angle, Porous Bouligand Structured 3D Printed Polymer. Mater. Proc. 2025, 20, 1. https://doi.org/10.3390/materproc2025020001
Patil PS, McCarthy ED, Alam P. Predicting the Flexural Modulus of Variable Pitch Angle, Porous Bouligand Structured 3D Printed Polymer. Materials Proceedings. 2025; 20(1):1. https://doi.org/10.3390/materproc2025020001
Chicago/Turabian StylePatil, Praveenkumar S., Edward D. McCarthy, and Parvez Alam. 2025. "Predicting the Flexural Modulus of Variable Pitch Angle, Porous Bouligand Structured 3D Printed Polymer" Materials Proceedings 20, no. 1: 1. https://doi.org/10.3390/materproc2025020001
APA StylePatil, P. S., McCarthy, E. D., & Alam, P. (2025). Predicting the Flexural Modulus of Variable Pitch Angle, Porous Bouligand Structured 3D Printed Polymer. Materials Proceedings, 20(1), 1. https://doi.org/10.3390/materproc2025020001







