First Principles Investigation of the Optoelectronic Properties of Molybdenum Dinitride for Optical Sensing Applications †
Abstract
:1. Introduction
2. Calculation Details
3. Results and Discussion
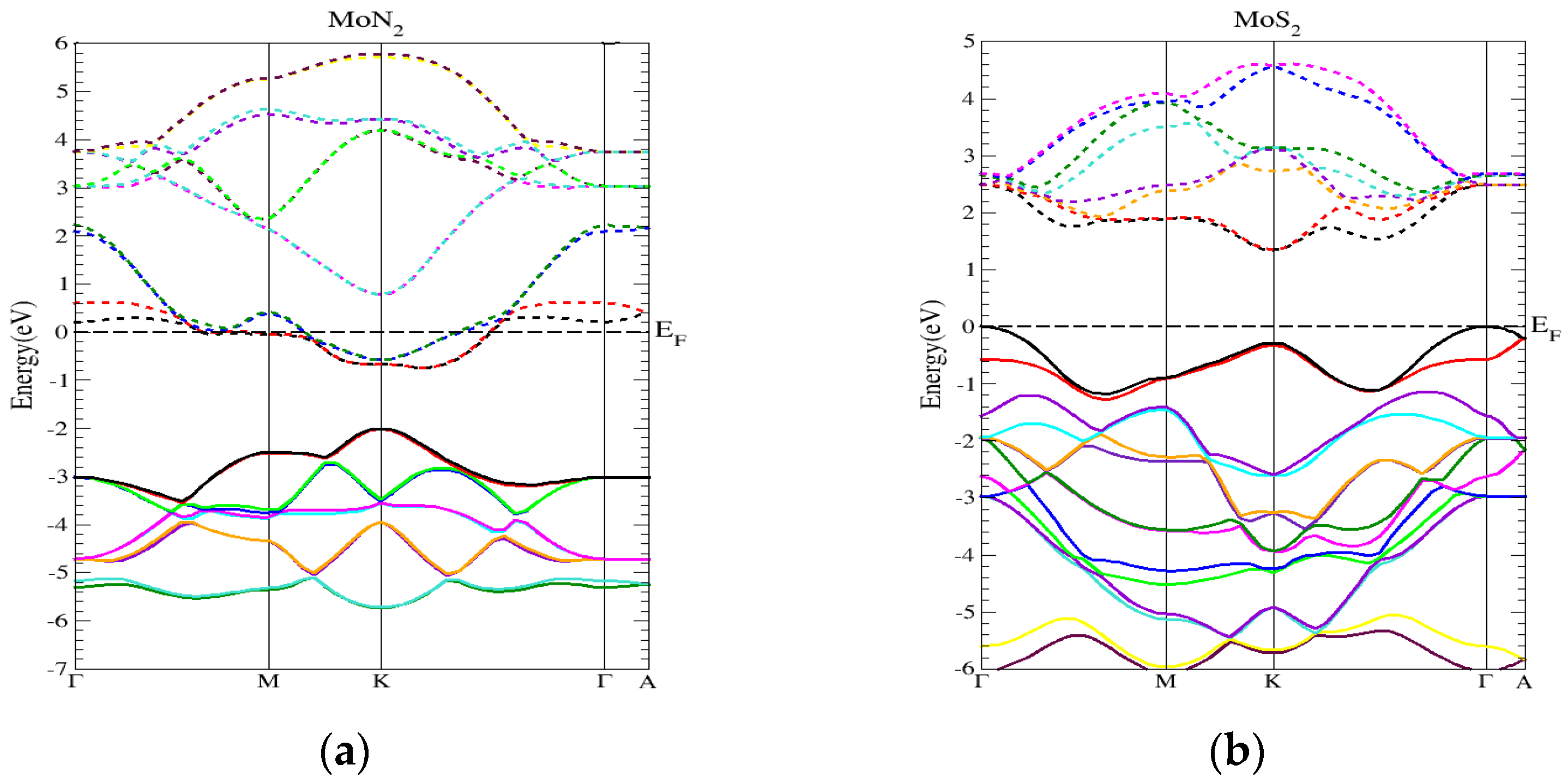
3.1. Structural and Electronic
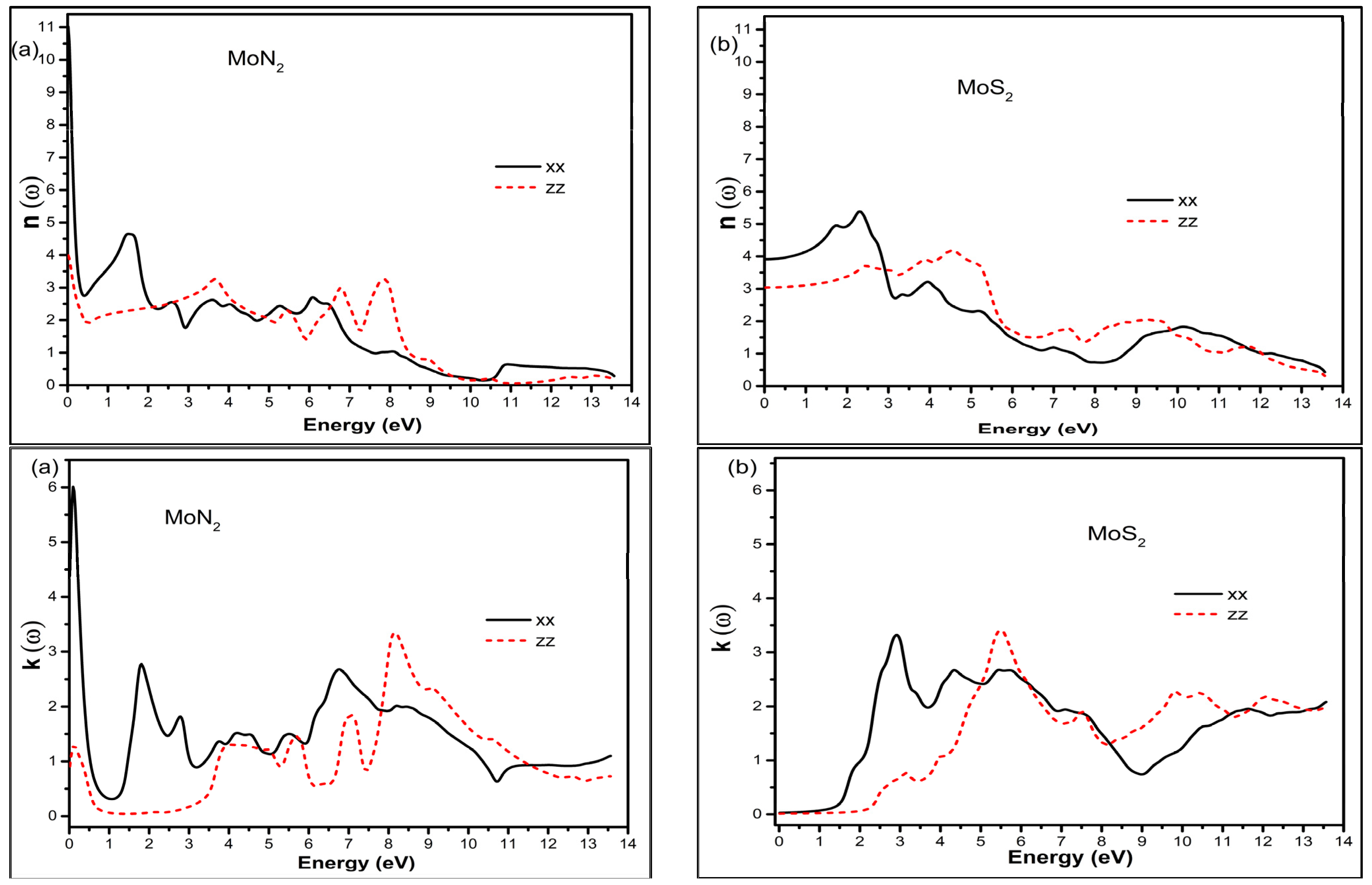
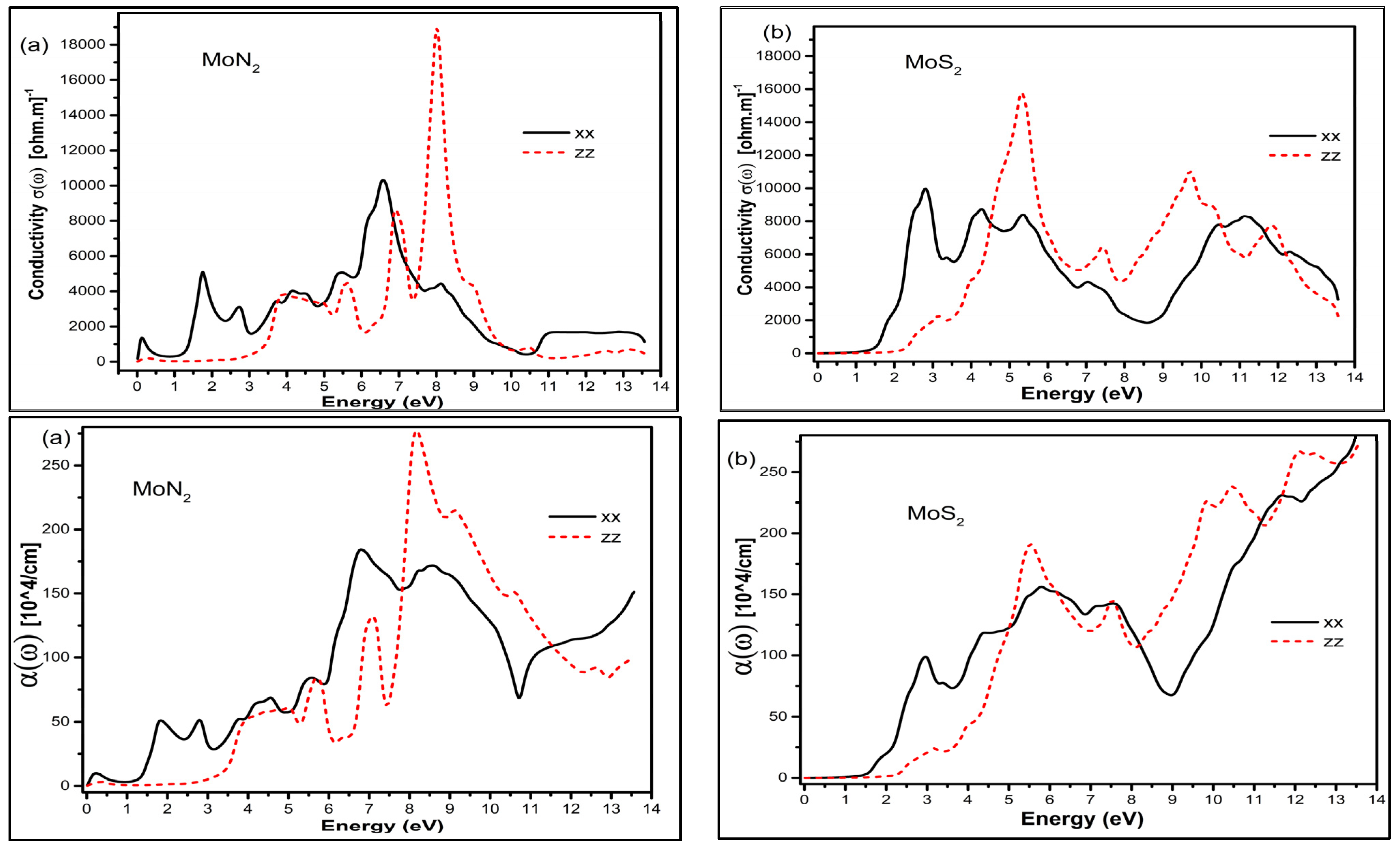
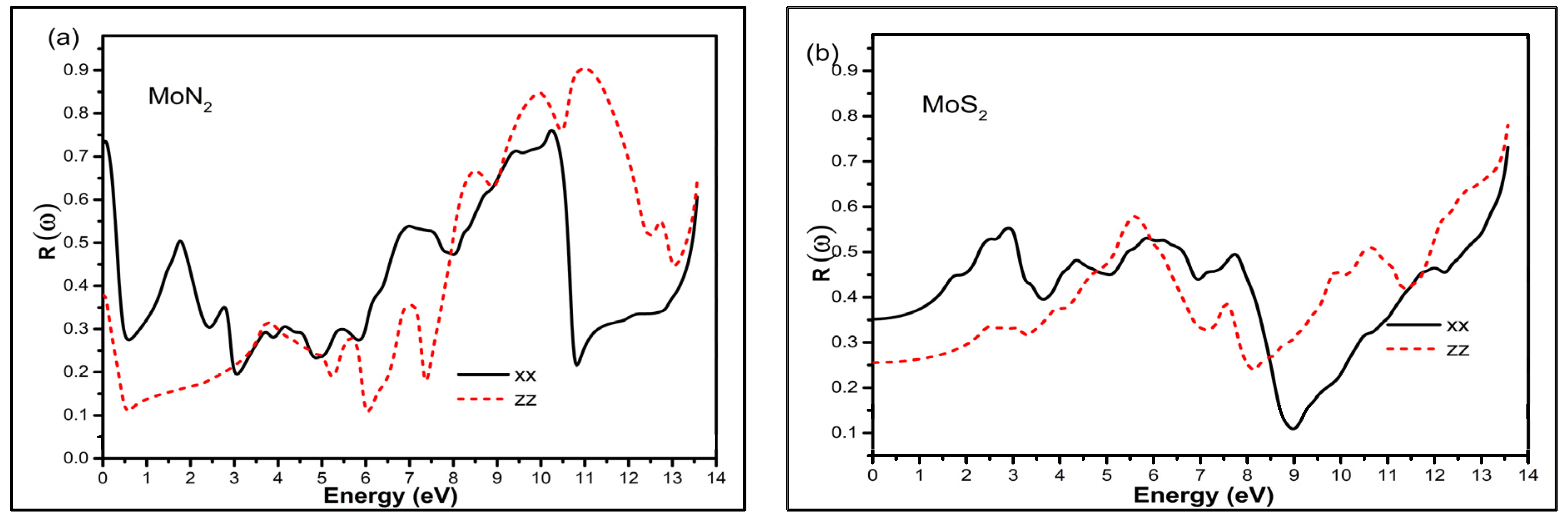
3.2. Optical Properties
4. Conclusions
Supplementary Materials
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Onofrio, N.; Guzman, D.M.; Strachan, A. Novel doping alternatives for transition metal dichalcogenides from high-throughput DFT calculations. J. Appl. Phys. 2017, 122, 185102. [Google Scholar] [CrossRef] [Green Version]
- Wang, Y.H.; Huang, K.J.; Wu, X. Recent advances in transition-metal dichalcogenides based electrochemical biosensors: A review. Biosens. Bioelectron. 2017, 97, 305. [Google Scholar] [CrossRef]
- Vikraman, D.; Akbar, K.; Hussain, S.; Yoo, G.; Jang, J.-Y.; Chun, S.-H.; Jung, J.; Park, H.J. Direct synthesis of thickness-tunable MoS2 quantum dot thin layers: Optical, structural and electrical properties and their application to hydrogen evolution. Nano Energy 2017, 35, 101. [Google Scholar] [CrossRef]
- Ramanathan, A.A. Defect Functionalization of MoS2 nanostructures as toxic gas sensors. IOP Conf. Ser. Mater. Sci. Eng. 2018, 305, 012001. [Google Scholar] [CrossRef] [Green Version]
- Zhang, H.; Lu, S.B.; Zheng, J.; Du, J.; Wen, S.C.; Tang, D.Y.; Loh, K.P. Molybdenum disulfide (MoS2) as a broadband saturable absorber for ultra-fast photonics. Opt. Express 2014, 22, 7249–7260. [Google Scholar] [CrossRef]
- Ramanathan, A.A.; Khalifeh, J.M. Enhanced thermoelectric properties of suspended mono and bilayer of MoS2 from first principles. IEEE Trans. Nanotechnol. 2018, 17, 974. [Google Scholar] [CrossRef]
- Chen, W.-F.; Muckerman, J.T.; Fujita, E. Recent developments in transition metal carbides and nitrides as hydrogen evolution electrocatalysts. Chem. Commun. 2013, 49, 8896–8909. [Google Scholar] [CrossRef] [PubMed]
- Tabata, M.; Maeda, K.; Higashi, M.; Lu, D.; Takata, T.; Abe, R.; Domen, K. Modified Ta3N5 powder as a photocatalyst for O2 evolution in a two-step water splitting system with an iodate/iodide shuttle redox mediator under visible light. Langmuir 2010, 26, 9161–9165. [Google Scholar] [CrossRef] [PubMed]
- Wang, S.M.; Ge, H.; Sun, S.L.; Zhang, J.Z.; Liu, F.M.; Wen, X.D.; Yu, X.H.; Wang, L.P.; Zhang, Y.; Xu, H.W.; et al. A New Molybdenum Nitride Catalyst with Rhombohedral MoS2 Structure for Hydrogenation Applications. J. Am. Chem. Soc. 2015, 137, 4815. [Google Scholar] [CrossRef] [PubMed]
- Zhang, X.; Yao, Y.; Yu, Z.; Wang, S.-S.; Guan, S.; Yang, H.Y.; Yang, S.A. Theoretical prediction of MoN2 monolayer as a high capacity electrode material for metal ion batteries. J. Mater. Chem. A 2016, 4, 15224. [Google Scholar] [CrossRef] [Green Version]
- Ramanathan, A.A.; Khalfeh, J.M. Thermoelectrics of MoS2(1−x)N2x Compounds. Phys. Sci. Biophys. J. 2021, 5, 000167. [Google Scholar] [CrossRef]
- Ramanathan, A.A. A DFT calculation of Nb and Ta (001) Surface Properties. J. Mod. Phys. 2013, 4, 432–437. [Google Scholar] [CrossRef] [Green Version]
- Gonze, X.; Jollet, F.; Araujo, F.A.; Adams, D.; Amadon, B.; Applencourt, T.; Audouze, C.; Beuken, J.M.; Bieder, J.; Bokhanchuk, A. Recent developments in the ABINIT software package. Comput. Phys. Commun. 2016, 205, 106. [Google Scholar] [CrossRef] [Green Version]
- Perdew, J.P.; Burke, K.; Ernzerhof, M. Generalized Gradient Approximation Made Simple. Phys. Rev. Lett. 1996, 77, 3865. [Google Scholar] [CrossRef] [Green Version]
- Ramanathan, A.A.; Khalifeh, J.M. Substrate matters: Magnetic tuning of the Fe monolayer. J. Magn. Magn. Mater. 2017, 426, 450–453. [Google Scholar] [CrossRef]
- Ramanathan, A.A.; Khalifeh, J.M.; Hamad, B.A. Evidence of surface magnetism in the V/Nb(0 0 1) system: A total energy pseudopotential calculation. Surf. Sci. 2008, 602, 607–613. [Google Scholar] [CrossRef]
- Blaha, P.; Schwarz, K.; Tran, F.; Laskowski, R.; Madsen, G.K.H.; Marks, L.D. WIEN2k: An APW+lo program for calculating the properties of solids. J. Chem. Phys. 2020, 152, 074101. [Google Scholar] [CrossRef]
- Tran, F.; Blaha, P. Accurate band gaps of semiconductors and insulators with a semilocal exchange-correlation potential. Phys. Rev. Lett. 2009, 102, 226401. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Coehoorn, R.; Haas, C.; Dijkstra, J.; Flipse, C.J.F.; de Groot, R.A.; Wold, A. Electronic structure of MoSe2, MoS2, and WSe2. I. Band-structure calculations and photoelectron spectroscopy. Phys. Rev. B 1987, 35, 6195. [Google Scholar] [CrossRef] [Green Version]
- Hulthén, R. Kramers–Kronig relations generalized: On dispersion relations for finite frequency intervals. A spectrum-restoring filter. J. Opt. Soc. Am. 1982, 72, 794–803. [Google Scholar] [CrossRef]
- Ramanathan, A.A.; Khalifeh, J.M. Electronic, magnetic and optical properties of XScO3 (X = Mo, W) perovskites. PeerJ Mater. Sci. 2021, 3, e15. [Google Scholar] [CrossRef]

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ramanathan, A.A. First Principles Investigation of the Optoelectronic Properties of Molybdenum Dinitride for Optical Sensing Applications. Chem. Proc. 2021, 5, 27. https://doi.org/10.3390/CSAC2021-10429
Ramanathan AA. First Principles Investigation of the Optoelectronic Properties of Molybdenum Dinitride for Optical Sensing Applications. Chemistry Proceedings. 2021; 5(1):27. https://doi.org/10.3390/CSAC2021-10429
Chicago/Turabian StyleRamanathan, Amall A. 2021. "First Principles Investigation of the Optoelectronic Properties of Molybdenum Dinitride for Optical Sensing Applications" Chemistry Proceedings 5, no. 1: 27. https://doi.org/10.3390/CSAC2021-10429
APA StyleRamanathan, A. A. (2021). First Principles Investigation of the Optoelectronic Properties of Molybdenum Dinitride for Optical Sensing Applications. Chemistry Proceedings, 5(1), 27. https://doi.org/10.3390/CSAC2021-10429





