Using Frozen Beads from a Mixture of Mesitylene and Meta-Xylene with Rupert’s Drop Properties in Cryogenic Neutron Moderators
Abstract
1. Introduction
2. Materials and Methods
2.1. Formation of a Solid Frozen Bead from Mesitylene with the Properties of a Rupert’s Drop
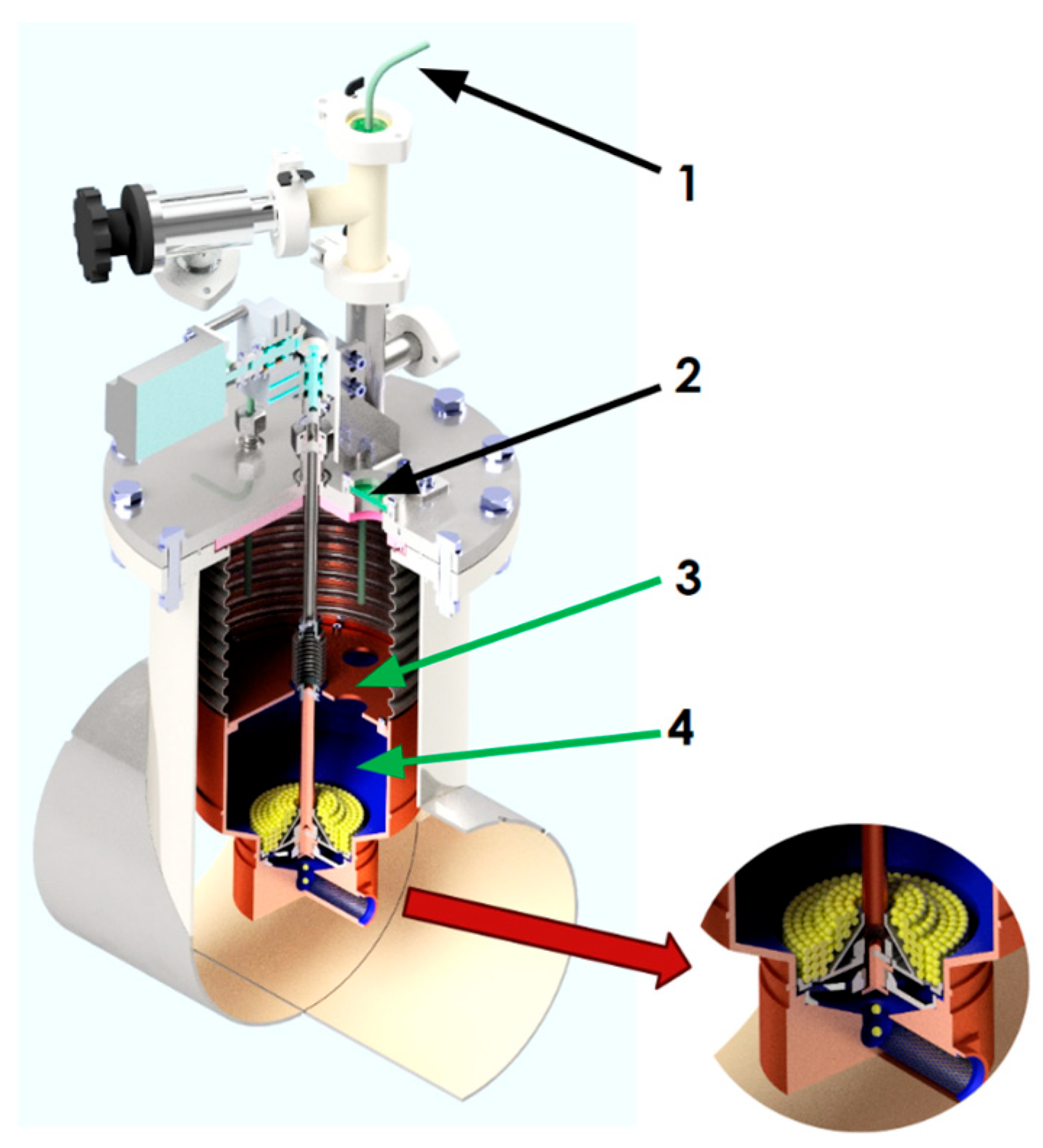
2.2. Experimental Setup for Loading the Moderator Chamber with Frozen Beads Exhibiting Rupert’s Drop Properties, Using a Modified Dosing Device with a High Unloading Rate
- The time for fully loading the moderator chamber of the test stand using the modified dosing device with high unloading speed was 1.2 h, which is 5 times faster compared to the standard dosing device. No differences were found in the temperature, mass flow rate, or helium flow rate in the pneumatic transport system compared to the standard dosing device;
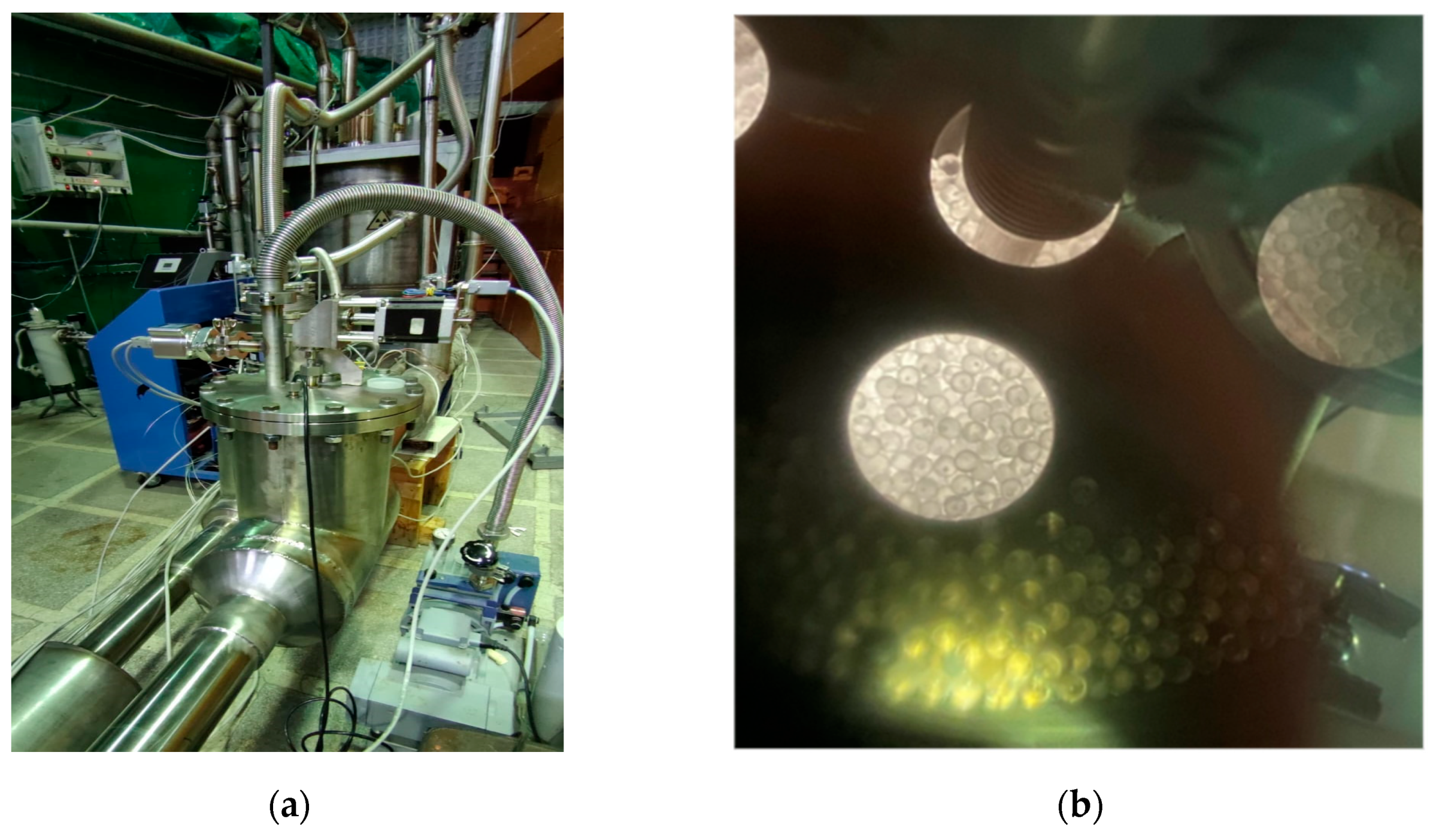
- Using standard pneumatic transport parameters, a total of ~6.7 L or approximately 180,000 frozen beads made from a mixture of mesitylene and meta-xylene with Rupert’s drop properties were successfully loaded into the moderator chambers. No fragments of frozen beads were found during the experiment.
3. Results
- During pneumatic transportation of the beads with properties of Rupert’s drops, the effect of spontaneous destruction was not observed. Thus, such beads can be subjected to more intensive mechanical impact, which allows for faster loading times by increasing the gas velocity or the mass flow rate of helium in the pneumatic transport pipeline.
- The use of a modified dosing device for loading frozen beads with properties resembling Rupert’s drops into the chamber of a pelletized cryogenic moderator reduces the loading time by a factor of five.
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Appendix A
Appendix B
| Physical Quantity | Notation | Value |
|---|---|---|
| Surface tension of liquid nitrogen with nitrogen vapor at 77.4 K | , | 8.85 mN/m [16] |
| Surface tension of mesitylene at 230 K | 30 mN/m [17] | |
| Density of liquid nitrogen at 77.4 K | 806.08 kg/m3 [18] | |
| Density of mesitylene drop at 300 K | 861.12 kg/m3 [17] | |
| Density of nitrogen vapor at 77.4 K | 4.6121 kg/m3 [18] | |
| Viscosity of nitrogen vapor at 77.4 K | 54.440 10−7 Pa · s [18] | |
| Thermal conductivity coefficient of nitrogen vapor over liquid nitrogen at 77.4 K | 7.1876 mW/m/K [18] | |
| Specific heat capacity of the drop at 300 K | 1.75 kJ/kg/K [17] | |
| Specific heat capacity of the crystallized drop at 230 K | 1.21 kJ/kg/K [19] | |
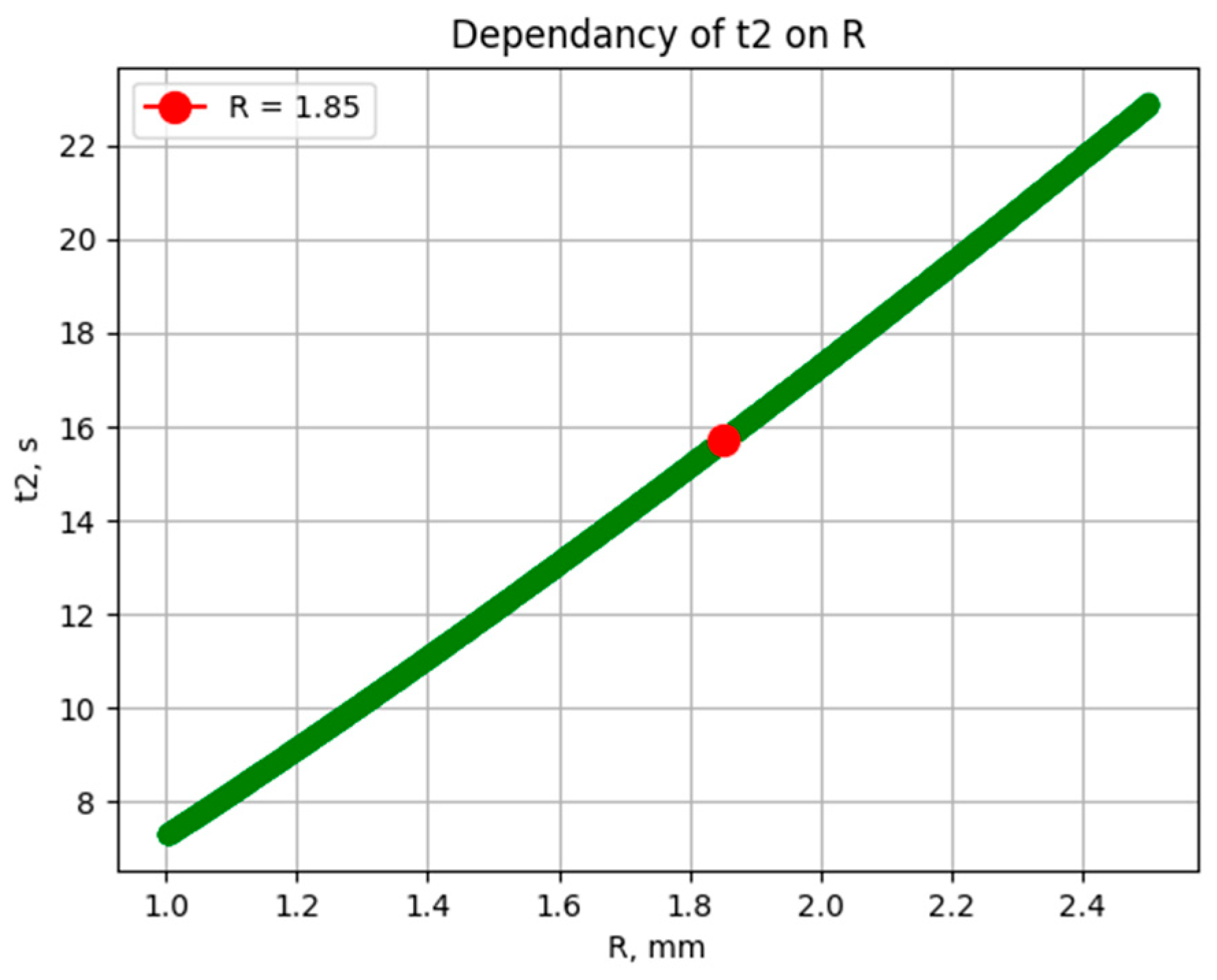
| Drop radius | R | 1.75–2.5 mm |
| Fraction of the drop’s surface in contact with the vapor layer | 0.5 | |
| Gravitational acceleration | 9.81 | |
| Latent heat of vaporization of liquid nitrogen | 200 kJ/kg [20] | |
| Latent heat of fusion of mesitylene | 80.12 [21] | |
| Initial temperature of the drop | 300 K | |
| Temperature of liquid nitrogen | 77.4 | |
| Melting temperature of mesitylene | 228.43 [22] | |
| Leidenfrost temperature of liquid nitrogen | 126 [23] | |
| Temperature outside the cryostat | 300 K |
Appendix C
References
- Shabalin, E.; Kulikov, S.; Bulavin, M.; Verhoglyadov, A. The World’s First Pelletized Cold Neutron Moderator Began its Operation. Neutron News 2013, 24, 27. [Google Scholar]
- Ananiev, V.; Belyakov, A.; Bulavin, M.; Kulagin, E.; Kulikov, S.; Mukhin, K.; Petukhova, T.; Sirotin, A.; Shabalin, D.; Shabalin, E.; et al. The world’s first pelletized cold neutron moderator at a neutron scattering facility. Nucl. Instrum. Methods Phys. Res. Sect. B 2014, 320, 70–74. [Google Scholar] [CrossRef]
- Ananiev, V.; Beliakov, A.; Bulavin, M.; Verkhogliadov, A.; Kulagin, E.; Kulikov, S.; Mukhin, K.; Shabalin, E.; Loktaev, K. Pelletized cold moderator of the IBR-2 reactor: Current status and future development. J. Phys. Conf. Ser. 2016, 746, 012031. [Google Scholar]
- Bulavin, M.V.; Belyakov, A.A.; Verkhoglyadov, A.E.; Kulikov, S.A.; Mukhin, K.A. Gain Factor of the Pelletized Cold Neutron Moderator at 22 K. J. Surf. Investig. X-Ray Synchrotron Neutron Tech. 2020, 14, 434–436. [Google Scholar]
- Bulavin, M.V.; Mukhin, K.A.; Yskakov, A.; Rogov, A.D.; Galushko, A.V.; Skuratov, V.A.; Smelyansky, I.A. Some Features of the Operation of Pelletized Cryogenic Mesitylene-Based Moderators at the IBR-2 Pulsed Fast Reactor. J. Surf. Investig. X-Ray Synchrotron Neutron Tech. 2022, 16, 1–6. [Google Scholar]
- Belyakov, A.A.; Bulavin, M.V.; Verkhoglyadov, A.E.; Skuratov, V.A.; Smelyansky, I.A.; Kulikov, S.A.; Kustov, A.A.; Mukhin, K.A.; Lyubimtsev, A.A.; Sirotin, A.P.; et al. Possibility of loading the chamber of the “central” pelletized cold moderator for IBR-2 reactor beams 1, 4–6, and 9. Phys. Part. Nucl. Lett. 2016, 13, 747–754. [Google Scholar]
- Bulavin, M.V.; Kazakov, A.V.; Shabalin, E.P. On the theory of pneumotransport of beads in cold neutron moderator of the IBR-2 reactor. Phys. Part. Nucl. Lett. 2017, 14, 520–532. [Google Scholar] [CrossRef]
- Bulavin, M.V. Spherical Cold Moderator of the IBR-2 Reactor: Certain Aspects of Creation and Application. Ph.D. Thesis, Joint Institute for Nuclear Research (JINR), Dubna, Russia, 2017; p. 149. [Google Scholar]
- Hooke, R. Micrographia, or, Some Physiological Descriptions of Minute Bodies Made by Magnifying Glasses: With Observations and Inquiries Thereupon; Printed by Jo. Marten, and Ja. Allestry, printers to the Royal Society, and are to be sold at their shop at the Bell in S. Paul’s Church-yard; MDCLXV: London, UK, 1665. [Google Scholar]
- Snart, J. Physico-mechanical experiments and discussions of the phenomena observable in that casual product of art the hand grenade, Prince Rupert drop, or glass tear. Philos. Mag. 1805, 22, 334–339. [Google Scholar] [CrossRef][Green Version]
- Johnson, W.; Chandrasekar, S. Rupert’s glass drops: Residual-stress measurements and calculations and hypotheses for explaining disintegrating fracture. J. Mater. Process. Technol. 1992, 31, 413–440. [Google Scholar]
- Gauthier, A.; Diddens, C.R.; Lohse, D.; van der Meer, D. Self-propulsion of inverse Leidenfrost drops on a cryogenic bath. Proc. Natl. Acad. Sci. USA 2019, 116, 1174–1179. [Google Scholar] [CrossRef] [PubMed]
- Adda-Bedia, M.; Kumar, S.; Lechenault, F.; Moulinet, S.; Schillaci, M.; Vella, D. Inverse Leidenfrost Effect: Levitating Drops on Liquid Nitrogen. Langmuir 2016, 32, 4179–4188. [Google Scholar] [CrossRef] [PubMed]
- Cashman, K.V.; Liu, E.J.; Rust, A.C. Rust. Prince Rupert’s Drops: An analysis of fragmentation by thermal stresses and quench granulation of glass and bubbly glass. Proc. Natl. Acad. Sci. USA 2022, 119, e2202856119. [Google Scholar] [CrossRef] [PubMed]
- Erasov, V.S.; Oreshko, E.I.; Lutsenko, A.N. Area of a free surface as criterion of brittle fracture. Aviat. Mater. Technol. 2017, 2, 69–79. [Google Scholar]
- Jasper, J.J. The Surface Tension of Pure Liquid Compounds. J. Phys. Chem. Ref. Data 1972, 1, 841–1010. [Google Scholar]
- He, Y.; Jiang, R.; Zhu, F.; Luan, T.; Huang, Z.; Ouyang, G. Excess Molar Volumes and Surface Tensions of 1,2,4-Trimethylbenzene and 1,3,5-Trimethylbenzene with Isopropyl Acetate and Isobutyl Acetate at (298.15, 308.15, and 313.15) K. J. Chem. Eng. Data 2008, 53, 1186–1191. [Google Scholar]
- NIST Chemistry WebBook. Available online: https://webbook.nist.gov/cgi/fluid.cgi?ID=C7727379&Action=Page (accessed on 20 March 2025).
- Taylor, R.D.; Kilpatrick, J.E. Entropy, heat capacity, and heats of transition of 1, 3, 5-trimethylbenzene. J. Chem. Phys. 1955, 23, 1232–1235. [Google Scholar]
- Dana, L.I. The latent heat of vaporization of liquid oxygen-nitrogen mixtures. Proc. Am. Acad. Arts Sci. 1925, 60, 241–267. [Google Scholar]
- NIST Chemistry WebBook. Available online: https://webbook.nist.gov/cgi/cbook.cgi?ID=C108678&Mask=1EFF (accessed on 10 June 2024).
- Griesbaum, K.; Behr, A.; Biedenkapp, D.; Voges, H.-W.; Garbe, D.; Paetz, C.; Collin, G.; Mayer, D.; Höke, H. Hydrocarbons. In Ullmann’s Encyclopedia of Industrial Chemistry; Wiley-VCH: Weinheim, Germany, 2000. [Google Scholar]
- Kim, H. Floating phenomenon of a water drop on the surface of liquid nitrogen. J. Korean Phys. Soc. 2006, 49, L1335–L1338. [Google Scholar]
- Shabalin, E.P.; Kulikov, S.A. Aromatic Hydrocarbons Based Cold Neutron Moderator; P13-2004-73 Communication of the Joint Institute for Nuclear Research: Dubna, Russia, 2004. [Google Scholar]
- Easteal, A.J.; Woolf, L.A. Freezing pressures, p, V, T, and self-diffusion data at 298 and 313 K and pressures up to 300 MPa for 1,3,5-trimethylbenzene. Int. J. Thermophys. 1987, 8, 71–79. [Google Scholar]




| Standard Method | Optimized Method | ||
|---|---|---|---|
| 1 Bead in 10 s | 1 Bead in 7 s | ||
| Production time, h | 6 | 3 | 2 |
| Frequency of liquid nitrogen addition, times/h | 1 | 3 | 3 |
| Defective beads with a diameter greater than 3.9 mm | <2.5% | <8.5% | <12.5% |
| Defective beads with a diameter less than 3.6 mm | <2.5% | <1.5% | <0.5% |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bulavin, M.V.; Litvak, I.L. Using Frozen Beads from a Mixture of Mesitylene and Meta-Xylene with Rupert’s Drop Properties in Cryogenic Neutron Moderators. J. Nucl. Eng. 2025, 6, 9. https://doi.org/10.3390/jne6020009
Bulavin MV, Litvak IL. Using Frozen Beads from a Mixture of Mesitylene and Meta-Xylene with Rupert’s Drop Properties in Cryogenic Neutron Moderators. Journal of Nuclear Engineering. 2025; 6(2):9. https://doi.org/10.3390/jne6020009
Chicago/Turabian StyleBulavin, Maksim V., and Ivan L. Litvak. 2025. "Using Frozen Beads from a Mixture of Mesitylene and Meta-Xylene with Rupert’s Drop Properties in Cryogenic Neutron Moderators" Journal of Nuclear Engineering 6, no. 2: 9. https://doi.org/10.3390/jne6020009
APA StyleBulavin, M. V., & Litvak, I. L. (2025). Using Frozen Beads from a Mixture of Mesitylene and Meta-Xylene with Rupert’s Drop Properties in Cryogenic Neutron Moderators. Journal of Nuclear Engineering, 6(2), 9. https://doi.org/10.3390/jne6020009





