Sensitivity-Analysis-Driven Surrogate Model for Molten Salt Reactors Control
Abstract
1. Introduction
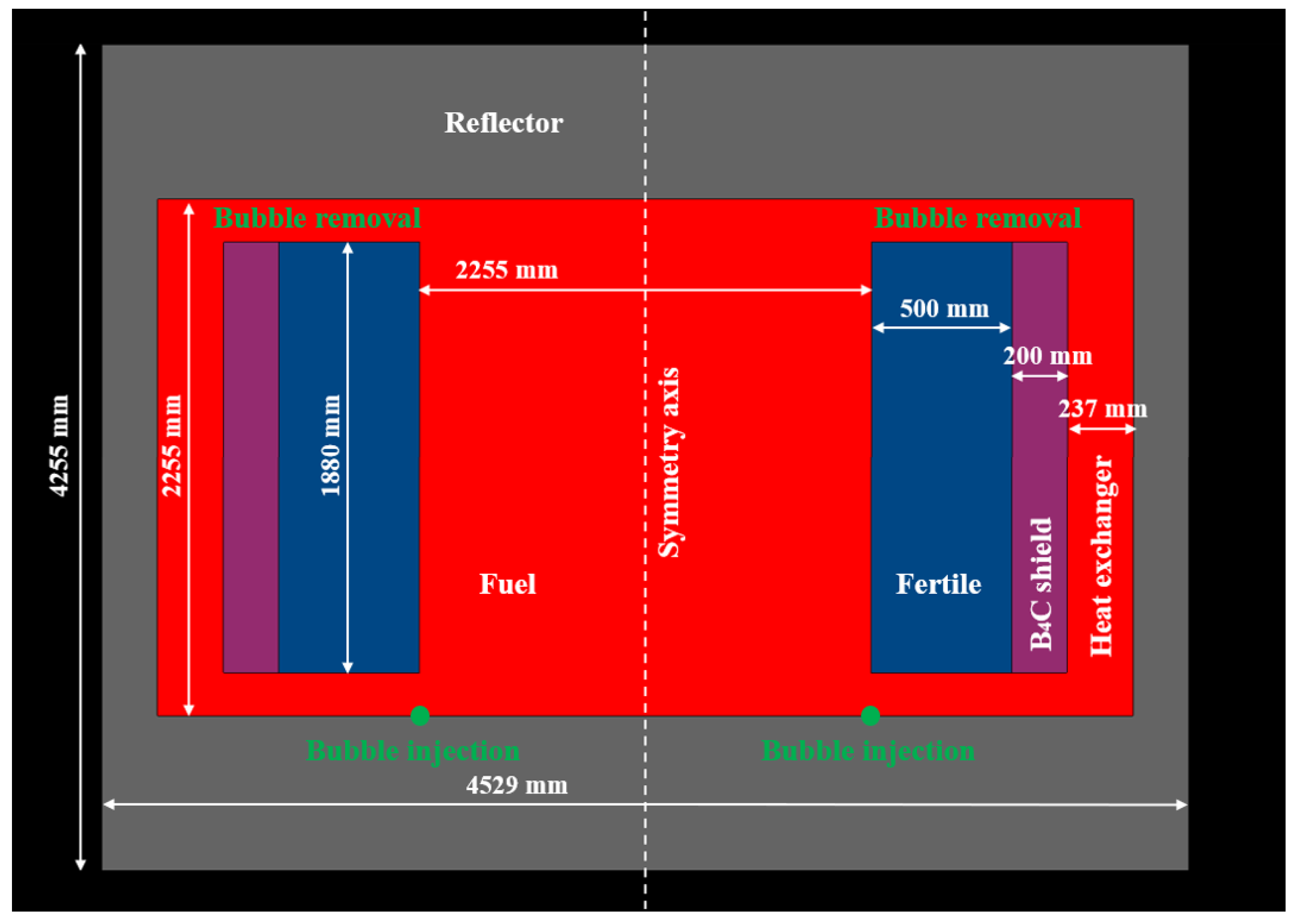
2. MSRs
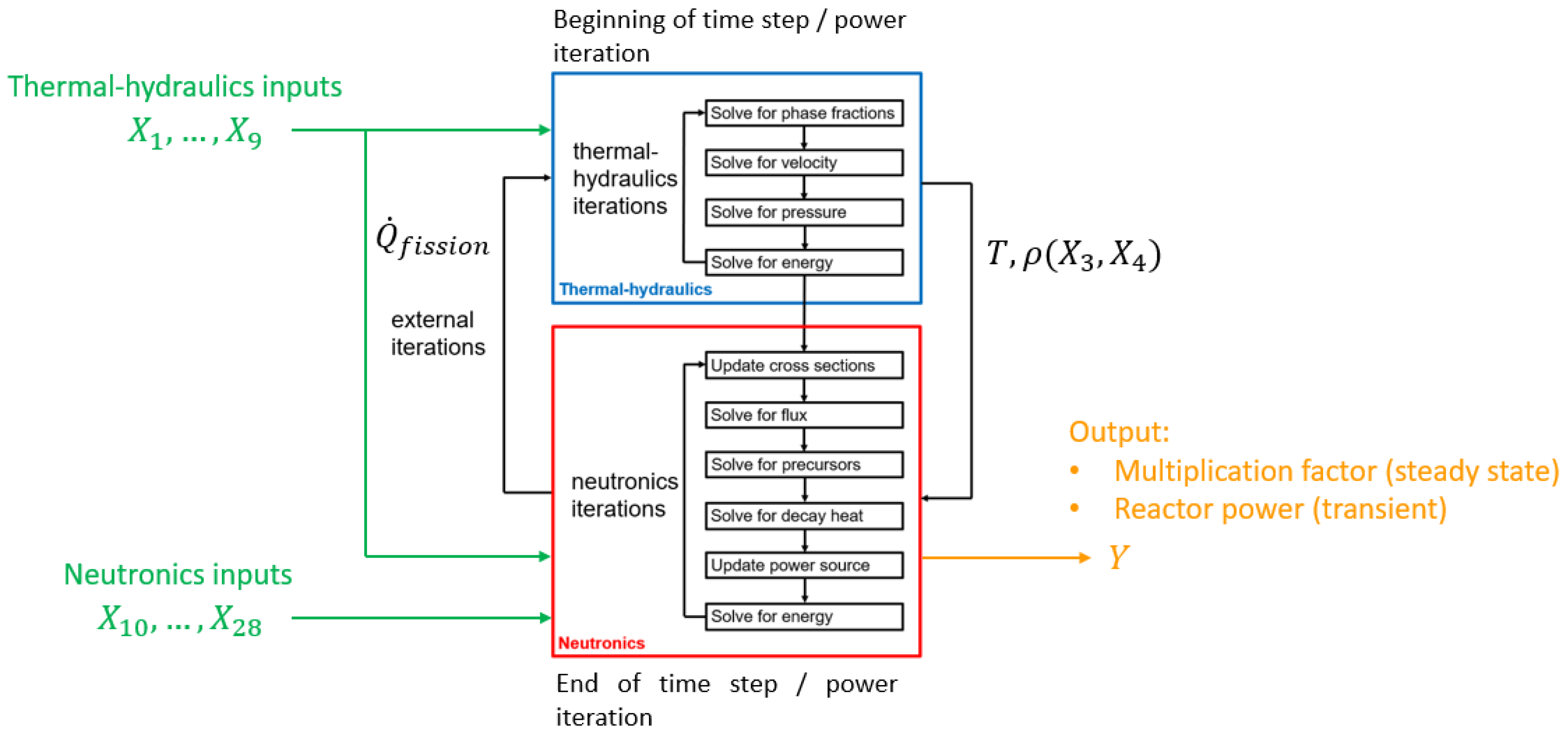
3. The Multiphysics High-Fidelity Model
- A time-independent, criticality mode, in which the system multiplication factor is evaluated at steady-state conditions. To this aim, a power iteration routine, based on the k-eigenvalue method [48] is implemented into the neutronics module. In this case, the main output is represented by the multiplication factor.
- A time-dependent mode, for the analysis of operational as well as accidental transients. The main output provided by the transient mode is the reactor thermal power.
3.1. The Thermal-Hydraulics Model
3.2. The Neutronics Model
4. Global SA Methods
5. SA Results
6. The Surrogate Model
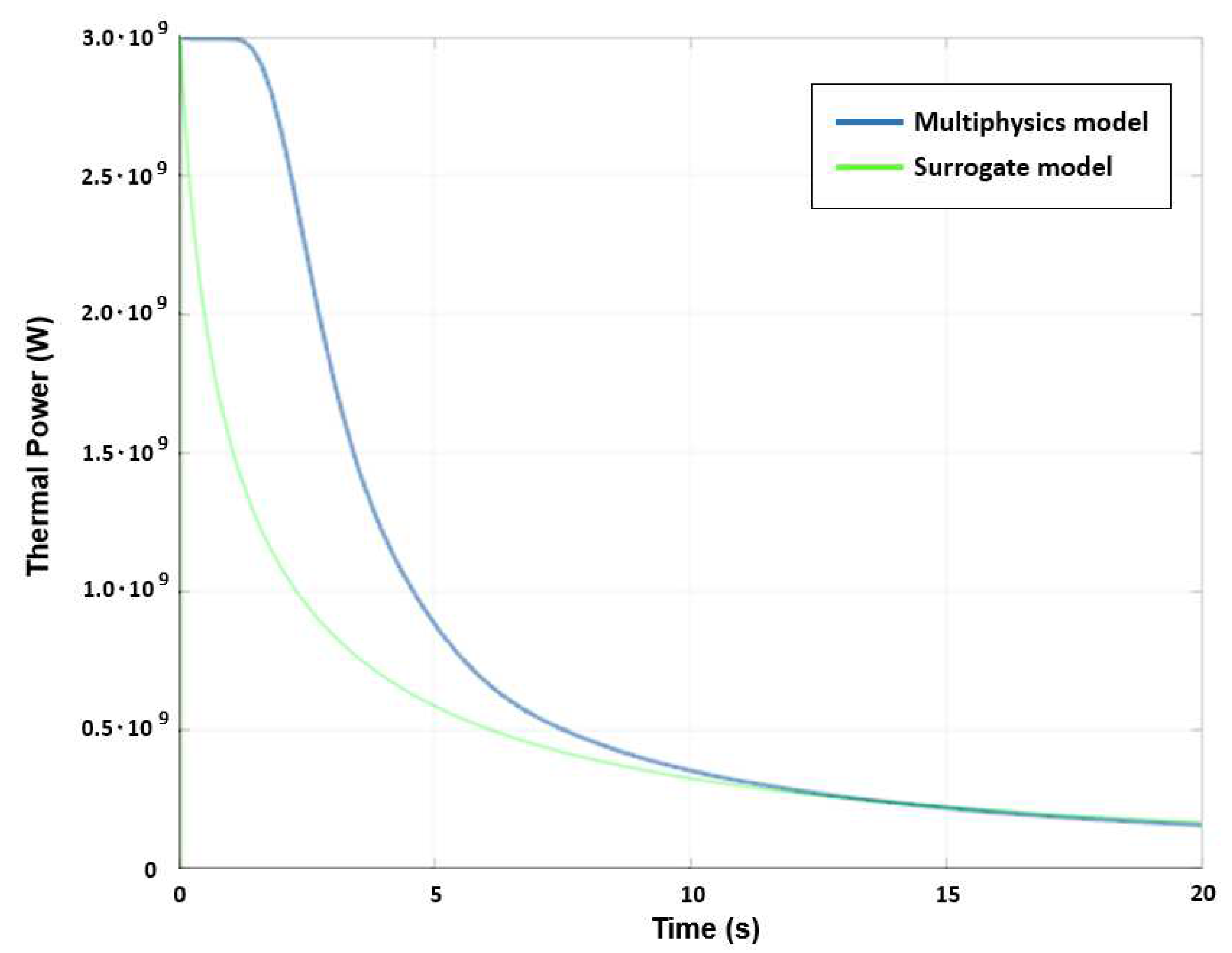
7. Comparison between the Surrogate and High-Fidelity Models
7.1. Super-Prompt-Critical Reactivity Insertion
7.2. Loss of Heat Sink
8. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| Latin symbols | |
| Delayed neutron precursor density, m−3 | |
| Neutron diffusion coefficient, m | |
| Decay heat precursor density, W m−3 | |
| Specific enthalpy, J kg−1 | |
| Modified thermal diffusivity, J m−1 s−1 K−1 | |
| Specific kinetic energy, J kg−1 | |
| Effective multiplication factor, − | |
| Inter-phase heat transfer coefficient, W m−3 K | |
| Inter-phase momentum transfer, kg m−2 s−2 | |
| Pressure, Pa | |
| per cent mille (=105) | |
| Power source density, W m−3 | |
| Mass source, kg m−3 s−1 | |
| Time, s | |
| Velocity, m s−1 | |
| Neutron velocity, m s−1 | |
| Greek symbols | |
| Gas fraction, − | |
| Delayed neutron precursor fraction, − | |
| Decay heat energy fraction, − | |
| Inter-phase temperature difference, K | |
| Delayed neutron precursor decay constant, s−1 | |
| Decay heat precursor decay constant, s−1 | |
| Dynamic viscosity, Pa s | |
| Kinematic viscosity, m2 s-1 | |
| Mean neutrons per fission, − | |
| Density, kg m−3 | |
| Macroscopic cross section, m−2 | |
| Neutron flux (diffusion equation), m−2 s−1 | |
| Subscripts–superscripts | |
| Absorption | |
| Bubble | |
| Delayed | |
| Fission | |
| Decay heat | |
| Neutron energy group | |
| Phase | |
| Delayed neutron precursor group | |
| Liquid | |
| Decay heat precursor group | |
References
- Gerardin, D.; Allibert, M.; Heuer, D.; Laureau, A.; Merle-Lucotte, E.; Seuvre, C. Design evolutions of Molten Salt Fast Reactor. In Proceedings of the International conference on Fast Reactors and Related Fuel Cycles: Next Generation Nuclear Systems for Sustainable Development (FR17), Yekaterinburg, Russia, 26–29 June 2017. [Google Scholar]
- Fiorina, C. The Molten Salt Fast Reactor as a Fast-Spectrum Candidate for Thorium Implementation. PhD Thesis, Politecnico di Milano, Milan, Italy, 2013. [Google Scholar]
- Serp, J.; Allibert, M.; Beneš, O.; Delpech, S.; Feynberg, O.; Ghetta, V.; Heuer, D.; Holcomb, D.; Ignatiev, V.; Kloosterman, J.L.; et al. The molten salt reactor (MSR) in generation IV: Overview and perspectives. Prog. Nucl. Energy 2014, 77, 308–319. [Google Scholar] [CrossRef]
- Dolan, T.J. Molten Salt Reactors and Thorium Energy; Woodhead Publishing Series in Energy; Elsevier Ltd.: Cambridge, MA, USA, 2017. [Google Scholar]
- Ragusa, J.C.; Mahadevan, V.S. Consistent and accurate schemes for coupled neutronics thermal-hydraulics reactor analysis. Nucl. Eng. Des. 2009, 239, 566–579. [Google Scholar] [CrossRef]
- Cammi, A.; Di Marcello, V.; Luzzi, L.; Memoli, V.; Ricotti, M.E. A Multi-Physics Modelling Approach to the Dynamics of Molten Salt Reactors. Ann. Nucl. Energy 2011, 38, 1356–1372. [Google Scholar] [CrossRef]
- Cammi, A.; Fiorina, C.; Guerrieri, C.; Luzzi, L. Dimensional Effects in the Modelling of MSR Dynamics: Moving on from Simplified Schemes of Analysis to a Multi-Physics Modelling Approach. Nucl. Eng. Des. 2012, 246, 12–26. [Google Scholar] [CrossRef]
- Aufiero, M.; Brovchenko, M.; Cammi, A.; Clifford, I.; Geoffroy, O.; Heuer, D.; Laureau, A.; Losa, M.; Luzzi, L.; Merle, E.; et al. Calculating the effective delayed neutron fraction in the Molten Salt Fast Reactor: Analytical, deterministic and Monte Carlo approaches. Ann. Nucl. Energy 2014, 65, 390–401. [Google Scholar] [CrossRef]
- Aufiero, M.; Cammi, A.; Geoffroy, O.; Losa, M.; Luzzi, L.; Ricotti, M.E.; Rouch, H. Development of an OpenFOAM model for the Molten Salt Fast Reactor transient analysis. Chem. Eng. Sci. 2014, 111, 78–90. [Google Scholar] [CrossRef]
- Fiorina, C.; Aufiero, M.; Cammi, A.; Franceschini, F.; Krepel, J.; Luzzi, L.; Mikityuk, K.; Ricotti, M.E. Investigation of the MSFR core physics and fuel cycle characteristics. Prog. Nucl. Energy 2013, 68, 153–158. [Google Scholar] [CrossRef]
- Fiorina, C.; Lathouwers, D.; Aufiero, M.; Cammi, A.; Guerrieri, C.; Kloosterman, J.L.; Luzzi, L.; Ricotti, M.E. Modelling and analysis of the MSFR transient behaviour. Ann. Nucl. Energy 2014, 64, 485–498. [Google Scholar] [CrossRef]
- Tiberga, M.; Lathouwers, D.; Kloosterman, J.L. A discontinuous Galerkin FEM multi-physics solver for the molten salt fast reactor. In Proceedings of the International Conference on Mathematics and Computation Methods Applied to Nuclear Science and Engineering, M&C 2019, Portland, OR, USA, 25–29 August 2019. [Google Scholar]
- Alsayyari, F.; Tiberga, M.; Perkó, Z.; Lathouwers, D.; Kloosterman, J.L. A nonintrusive adaptive reduced order modeling approach for a molten salt reactor system. Ann. Nucl. Energy 2020, 141, 107321. [Google Scholar] [CrossRef]
- Tiberga, M.; Hennik, A.; Kloosterman, J.L.; Lathouwers, D. A high-order discontinuous Galerkin solver for the incompressible RANS equations coupled to the k-ε turbulence model. Comput. Fluids 2020, 212, 104710. [Google Scholar] [CrossRef]
- De Oliveira, R.G.G.; Mikityuk, K. Analytical solutions to a coupled fluid dynamics and neutron transport problem with application to GeN-Foam verification. Ann. Nucl. Energy 2018, 121, 446–451. [Google Scholar] [CrossRef]
- Tano, M.; Rubiolo, P.; Doche, O. Progress in modeling solidification in molten salt coolants. Model. Simul. Mater. Sci. Eng. 2017, 25, 074001. [Google Scholar] [CrossRef]
- Tano, M.; Rubiolom, P.; Giraus, J.; Ghetta, V. Multiphysics study of the draining transient in the molten salt fast reactor. In Proceedings of the 2018 International Congress on Advances in Nuclear Power Plants (ICAPP 18), Charlotte, NC, USA, 8–11 April 2018. [Google Scholar]
- Cervi, E.; Lorenzi, S.; Cammi, A.; Luzzi, L. Development of a multiphysics model for the study of fuel compressibility effects in the Molten Salt Fast Reactor. Chem. Eng. Sci. 2019, 193, 379–393. [Google Scholar] [CrossRef]
- Cervi, E.; Lorenzi, S.; Cammi, A.; Luzzi, L. Development of an SP3 neutron transport solver for the analysis of the Molten Salt Fast Reactor. Nucl. Eng. Des. 2019, 346, 209–219. [Google Scholar]
- Cervi, E.; Lorenzi, S.; Luzzi, L.; Cammi, A. Multiphysics analysis of the MSFR helium bubbling system: A comparison between neutron diffusion, SP3 neutron transport and Monte Carlo approaches. Ann. Nucl. Energy 2019, 132, 227–235. [Google Scholar] [CrossRef]
- Rusche, H. Computational Fluid Dynamics of Dispersed Two-Phase Flows at High Phase Fractions. PhD Thesis, Imperial College London, London, UK, December 2002. Available online: https://spiral.imperial.ac.uk/handle/10044/1/8110 (accessed on 9 October 2022).
- Howard, R.A. Uncertainty about Probability: A Decision Analysis Perspective. Risk Anal. 1988, 8, 91–98. [Google Scholar] [CrossRef]
- Sobol’, I.M. Sensitivity analysis for non-linear mathematical models. Math. Model. Comput. Exp. 1993, 1, 407–414. [Google Scholar]
- Borgonovo, E.; Plischke, E. Sensitivity Analysis: A Review of Recent Advances. Eur. J. Oper. Res. 2016, 3, 869–887. [Google Scholar] [CrossRef]
- Di Maio, F.; Nicola, G.; Borgonovo, E.; Zio, E. Invariant methods for an ensemble-based sensitivity analysis of a passive containment cooling system of an AP1000 nuclear power plant. Reliab. Eng. Syst. Saf. 2016, 161, 12–19. [Google Scholar] [CrossRef][Green Version]
- Borgonovo, E.; Hazen, G.; Plischke, E. A Common Rationale for Global Sensitivity Measures and their Estimation. Risk Anal. 2016, 36, 1871–1895. [Google Scholar] [CrossRef]
- Efron, B.; Gong, G. A leisurely look at the bootstrap, the jackknife, and cross-validation. Am. Stat. 1983, 37, 36–48. [Google Scholar]
- Plischke, E. An effective algorithm for computing global sensitivity indices (EASI). Reliab. Eng. Syst. Saf. 2010, 94, 354–360. [Google Scholar] [CrossRef]
- Borgonovo, E.; Lu, X.; Plischke, E.; Rakovec, O.; Hill, M.C. Making the most out of a hydrological model data set: Sensitivity analyses to open the model black-box. Water Resour. Res. 2017, 53, 7933–7950. [Google Scholar] [CrossRef]
- Jeong, Y.S.; Cervi, E.; Cammi, A.; Ninokata, H.; Bang, I.C. Adjoint-based sensitivity analysis of circulating liquid fuel system for the multiphysics model of molten salt reactor. Int. J. Energy Res. 2020, 44, 1–16. [Google Scholar] [CrossRef]
- Zhang, D.; Qiu, S.; Su, G. Development of a safety analysis code for molten salt reactors. Nucl. Eng. Des. 2009, 239, 2778–2785. [Google Scholar] [CrossRef]
- Zhang, D.; Qiu, S.; Su, G.; Liu, C.; Qian, L. Analysis of the neutron kinetics for a molten salt reactor. Prog. Nucl. Energy 2009, 51, 624–636. [Google Scholar] [CrossRef]
- Guo, Z.; Zhang, D.; Xiao, Y.; Tian, W.; Su, G.; Qiu, S. Simulation of unprotected loss of heat sink and combination of events accidents for a molten salt reactor. Ann. Nucl. Energy 2013, 53, 309–319. [Google Scholar] [CrossRef]
- Suzuki, N.; Shimazu, Y. Reactivity-Initiated-Accident Analysis without Scram of a Molten Salt Reactor. J. Nucl. Sci. Technol. 2007, 45, 575–581. [Google Scholar] [CrossRef]
- Cammi, A.; Di Marcello, V.; Guerrieri, C.; Luzzi, L. Transfer Function Modelling of Zero-Power Dynamics of Circulating Fuel Reactors. J. Eng. Gas Turbines Power 2011, 133, 1–8. [Google Scholar] [CrossRef]
- Stempiewicz, M.M.; Roelofs, F. Molten salt modelling in SPECTRA applied to MSRE. Nucl. Eng. Design 2021, 384, 111483. [Google Scholar] [CrossRef]
- OpenFOAM, 2013. OpenFOAM Documentation. Available online: http://www.openfoam.org/docs/ (accessed on 9 October 2022).
- Descamps, M.N.; Oliemans, R.V.A.; Omms, G.; Mudde, R.F. Air-water flow in a vertical pipe: Experimental study of air bubbles in the vicinity of the wall. Exp. Fluids 2008, 45, 357–370. [Google Scholar] [CrossRef][Green Version]
- Lage, P.L.C.; Esposito, R.O. Experimental determination of bubble size distributions in bubble columns: Prediction of mean bubble diameter and gas hold up. Powder Technol. 1999, 101, 142–150. [Google Scholar] [CrossRef]
- Lathouwers, D. Modelling and Simulation of Turbulent Bubbly Flow. PhD Thesis, Delft University of Technology, Delft, The Netherlands, December 1999. [Google Scholar]
- Engel, J.R.; Steffy, R.C. Xenon Behavior in the Molten Salt Reactor Experiment; ORNL-TM-3464; Oak Ridge National Laboratory: Oak Ridge, TN, USA, 1971. [Google Scholar]
- Cervi, E. An Innovative Multiphysics Modelling Approach for the Analysis and the Development of the Generation IV Molten Salt Fast Reactor. PhD Thesis, Politecnico Di Milano, Milano, Italy, July 2020. [Google Scholar]
- Sohal, M.; Ebner, M.; Sabharwall, P.; Sharpe, P. Engineering Database of Liquid Salt Thermophysical and Thermochemical Properties; INL-EXT-10-18297; Idaho National Laboratory: Idaho Falls, ID, USA, 2013. [Google Scholar]
- Ignatiev, V.V.; Feynberg, O.; Merzlyakov, A.; Toropov, A. Progress in development of MOSART concept with Th support. In Proceedings of the ICAPP ’12, Chicago, IL, USA, 24–28 June 2012. [Google Scholar]
- Lorenzi, S.; Cammi, A.; Luzzi, L.; Pini, A.; Allibert, M.; Gérardin, D.; Heuer, D.; Laureau, A.; Merle, E.; Lathouwers, D.; et al. TEC1.1 Plant Database for the System and Safety Analysis of the MSFR, SAMOFAR Safety Assessment of the Molten Salt Fast Reactor—MSFR; Internal Report, Euratom Grant Agreement 661891—Samofar; Euratom: Rome, Italy, 2016. [Google Scholar]
- Leppänen, J.; Pusa, M.; Viitanen, T.; Valtavirta, V.; Kaltiaisenaho, T. The Serpent Monte Carlo code: Status, development and application in 2013. Ann. Nucl. Energy 2015, 82, 142–150. [Google Scholar] [CrossRef]
- Santamarina, A.; Bernard, D.; Blaise, P.; Coste, M.; Courcelle, A.; Huynh, T.D.; Jouanne, C.; Leconte, P.; Litaize, O.; Ruggiéri, J.-M.; et al. The JEFF-3.1.1 Nuclear Data Library, JEFF Report 22, OECD/NEA. 2009. Available online: https://www.oecd-nea.org/jcms/pl_14470/the-jeff-3-1-1-nuclear-data-library?details=true (accessed on 9 October 2022).
- Bell, G.I.; Glasstone, S. Nuclear Reactor Theory; Van Nostrand Reinhold Company: New York, NY, USA, 1970. [Google Scholar]
- Gidaspow, D. Multiphase Flow and Fluidization; Academic Press: New York, NY, USA, 1994. [Google Scholar]
- Enwald, H.; Peirano, E.; Almstedt, A.E. Eulerian Two-Phase Flow Theory Applied to Fluidization. Int. J. Multiph. Flow 1996, 22, 21–66. [Google Scholar] [CrossRef]
- Ranz, W.E.; Marshall, W.R. Evaporation from droplets. Chem. Eng. Prog. 1952, 48, 173–180. [Google Scholar]
- Lahey, R.T. The simulation of multidimensional multiphase flows. Nucl. Eng. Des. 2005, 235, 1043–1060. [Google Scholar] [CrossRef]
- Cervi, E.; Cammi, A. An Arbitrary Lagrangian-Eulerian, coupled neutronics-shock physics model for the analysis of shockwave compression of solid fissile materials. Ann. Nucl. Energy 2020, 141, 107322. [Google Scholar] [CrossRef]
- Fiorina, C.; Hursin, M.; Pautz, A. Extension of the GeN-Foam neutronic solver to SP3 analysis and application to the CROCUS experimental reactor. Ann. Nucl. Energy 2017, 101, 419–428. [Google Scholar] [CrossRef]
- Pearson, K. On the General Theory of Skew Correlation and Non-linear Regression; Dulau & Co.: London, UK, 1905. [Google Scholar]
- Lamarsh, J.R. Introduction to Nuclear Reactor Theory; American Nuclear Society: LaGrange Park, IL, USA, 2002. [Google Scholar]
- Cervi, E.; Cammi, A.; Di Ronco, A. Stability analysis of the Generation-IV nuclear reactors by means of the root locus criterion. Prog. Nucl. Energy 2018, 106, 316–334. [Google Scholar] [CrossRef]



| Parameter | Value |
|---|---|
| Nominal power | 3000 MWth |
| Fuel inlet temperature | 923 K |
| Fuel outlet temperature | 1023 K |
| Total salt volume | 18 m3 |
| Fuel composition (mol. %) | LiF (77.5)—ThF4 (20.0)—233UF4 (2.5) |
| Injector diameter | 3 mm |
| Input | Parameter | Description | Ref. |
|---|---|---|---|
| Bubble diameter | A log-normal distribution is adopted in this work as well, as commonly done in literature. With regards to the support of such distribution, the bubble diameter is typically assumed to lay in between 1 and 5 mm with most probable values around 3 mm (i.e., for air and water [38,39,40]) and, in the case of the Molten Salt Reactor Experiment (MSRE), in between 0.127 and 0.508 mm [41]. For the MSR considered in this work, the diameter of the helium bubbles is taken equal to 3 mm, i.e., the most probable diameter, since it can be determined by the helium injector diameter (of 3 mm, see Table 1), as supported by analyses carried out in [42], where different bubble diameter models are compared. In more detail, [42] points out that bubbles injected with 3 mm diameter remain the same size in the whole reactor, without being significantly affected by bubble coalescence and break-up. In this respect, it is worth mentioning that, up to now, a helium bubbling system has never been designed for a fast-spectrum MSR and, therefore, no evidence is available to support different hypotheses on the actual helium bubbles diameter. Distribution (*): | [38,39,40] | |
| Surface tension | The surface tension of the salt adopted in this work (77.5% LiF 20.0% ThF4 2.5% 233UF4) has not been measured yet. A uniform distribution is assumed, that accounts for the uncertainties of correlations for other fluorides. For 46.5% LiF, 11.5% NaF, 42% KF (FLiNaK): ±2% of uncertainty. Valid for the temperature range T = (770–1040) K. For 33% LiF, 67% BeF2 (FLiBe): ±3% of uncertainty. Valid for the temperature range T = (773.15–1073.15) K. Distribution: | [43] | |
| Fuel density | where: ±0.9% of error. Distribution (**): | [44,45] | |
| Fuel specific heat | where: ±10% of error. Distribution (**): | [44,45] | |
| Fuel thermal conductivity | where: ±5% of experimental error. Distribution (**): | [44,45] | |
| Fuel kinematic viscosity | ±5% of experimental error. Distribution (**): | [44,45] | |
| All neutronics parameters | Normally distributed. Mean and standard deviation evaluated with Serpent (***) | See box below for details |
| Sensitivity Measure/Rank | 1st | 2nd | 3rd | 4th |
|---|---|---|---|---|
| 3 | 4 | 11 | 1 | |
| 4 | 3 | 20 | 1 | |
| 4 | 3 | 20 | 15 | |
| 4 | 3 | 20 | 15 | |
| 4 | 3 | 20 | 1 or 15 |
| Parameter | Symbol | Value | Unit |
|---|---|---|---|
| Effective delayed neutron fraction | 146 | Pcm | |
| Precursor decay constant | 0.317 | s−1 | |
| Mean neutron generation time | 1.147 | μs | |
| Density reactivity coefficient | 7.85 | pcm m3 kg−1 | |
| Fuel inlet temperature | 923 (steady state) | K | |
| Reactor volume | 9 | m3 | |
| Fuel specific heat (at T = 900 K) | 1391 | J kg−1 K−1 | |
| Initial power | 3000 | MW | |
| Nominal volumetric flow rate | 4.5 | m3 s−1 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Cervi, E.; Lu, X.; Cammi, A.; Di Maio, F.; Zio, E. Sensitivity-Analysis-Driven Surrogate Model for Molten Salt Reactors Control. J. Nucl. Eng. 2022, 3, 277-294. https://doi.org/10.3390/jne3040016
Cervi E, Lu X, Cammi A, Di Maio F, Zio E. Sensitivity-Analysis-Driven Surrogate Model for Molten Salt Reactors Control. Journal of Nuclear Engineering. 2022; 3(4):277-294. https://doi.org/10.3390/jne3040016
Chicago/Turabian StyleCervi, Eric, Xuefei Lu, Antonio Cammi, Francesco Di Maio, and Enrico Zio. 2022. "Sensitivity-Analysis-Driven Surrogate Model for Molten Salt Reactors Control" Journal of Nuclear Engineering 3, no. 4: 277-294. https://doi.org/10.3390/jne3040016
APA StyleCervi, E., Lu, X., Cammi, A., Di Maio, F., & Zio, E. (2022). Sensitivity-Analysis-Driven Surrogate Model for Molten Salt Reactors Control. Journal of Nuclear Engineering, 3(4), 277-294. https://doi.org/10.3390/jne3040016









