Multiphysics Studies of 3D Plate Fin Heat Exchanger Filled with Ortho-Para-Hydrogen Conversion Catalyst for Hydrogen Liquefaction
Abstract
1. Introduction
2. Three-Dimensional Modelling
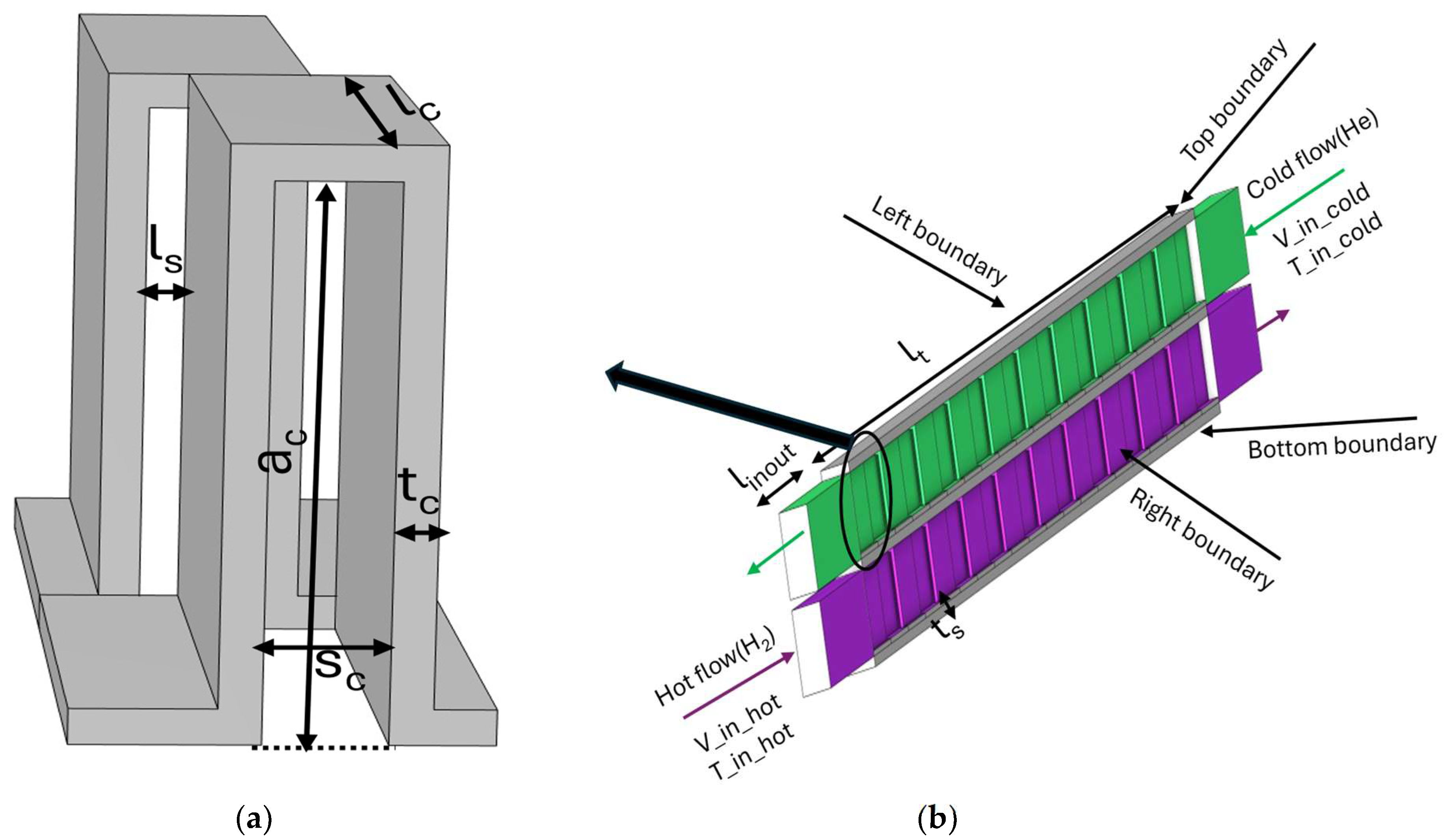
2.1. PFHE Geometry
2.2. Model Description
- (1)
- The cold channel operates with helium as the working fluid, while hydrogen is used on the hot channel. The hydrogen is a mixture of ortho- and para-form with the given concentration.
- (2)
- The catalyst particles are uniformly filled exclusively in the hot side of fin channel, maintaining fixed porosity and permeability in the catalyst bed.
- (3)
- Both the flow dynamics and heat transfer in the cold and hot channels, as well as the OPC kinetics in the hot channel, are assumed to be at steady state.
- (1)
- Mass and momentum conservation equations: These equations describe the flow behaviour in both the cold and hot channels separately, accounting for their distinct flow characteristics.
- (2)
- Species transport equation: This equation governs the transport of ortho- and para-hydrogen species in the hot channel with consideration of the OPC kinetics.
- (3)
- Heat transfer equation: This equation governs the transfer of heat between the cold and hot channels and the parting sheet, taking into account the enthalpy changes due to the OPC reaction.
2.2.1. Flow Characteristics
2.2.2. Species Transportation
2.2.3. Heat Transfer
2.2.4. Multiphysics Coupling
2.3. Material Property
2.4. Boundary Conditions and Solver Setting
- Inlet Boundary: Normal inflow velocity is specified. The inlet velocity for the cold flow is denoted as V_in_cold and for the hot flow as V_in_hot.
- Outlet Boundary: Specified as a pressure boundary with suppressed backflow.
- Wall Boundary: Non-slip wall boundary condition is applied to all walls.
- Asymmetric Boundaries: Left and right boundaries are set as asymmetric to accommodate the flow dynamics.
- Inlet Boundary: Specified as normal hydrogen consisting of 75% ortho-hydrogen and 25% para-hydrogen.
- Reaction Source: Includes a reaction source term considering the production of para-hydrogen from the OPC.
- Asymmetric Boundaries: Left and right boundaries are set as asymmetric to capture concentration gradients effectively.
- Inlet Boundary: Fixed temperature is specified. The inlet temperature for the cold flow is T_in_cold and for the hot flow is T_in_hot.
- Heat Source: Includes a heat source term accounting for the heat generated by the OPC reaction.
- Boundary Conditions: Left and right boundaries are set as asymmetric, while the top and bottom boundaries are set as periodic to simulate continuous heat exchange.
- Dependent variable: A segregated solver is employed to solve for eight dependent variables, which includes pressure (P1), velocity (v1), turbulent dissipation rate (ɛ) and turbulent kinetic energy (k) for the cold channel, pressure (P2) and velocity (v2) for the hot channel, temperature (T) for the entire heat exchanger, and parahydrogen mass fraction (ω) in the hot channel.
- Relative Tolerance: A relative tolerance of 1E-3 is set for each variable to ensure numerical stability and accuracy.
3. Results and Discussion
3.1. Model Verification
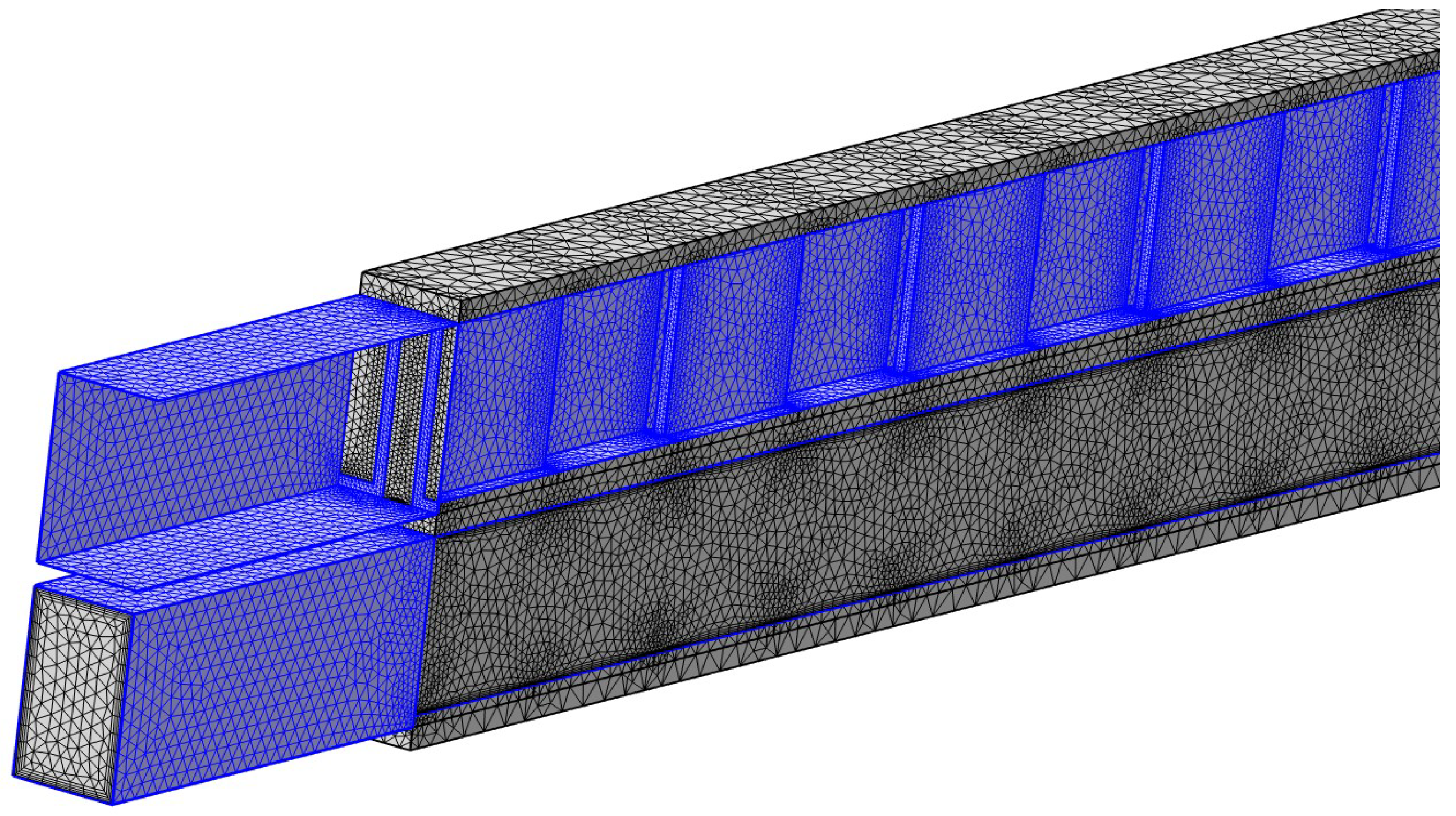
3.1.1. Mesh Size Setting
- Both hot and cold flow inlet velocity set to V_in_cold = V_in_hot = 5 m/s;
- Hot flow inlet temperature, T_in_hot = 77 K and cold flow inlet temperature, T_in_cold = 60 K.
- Coarse mesh with 890,000 elements;
- Normal mesh of 2.21 million elements;
- Fine mesh of 4.46 million elements;
- Finer mesh of 8.87 million elements.
3.1.2. Verification of Heat Transfer
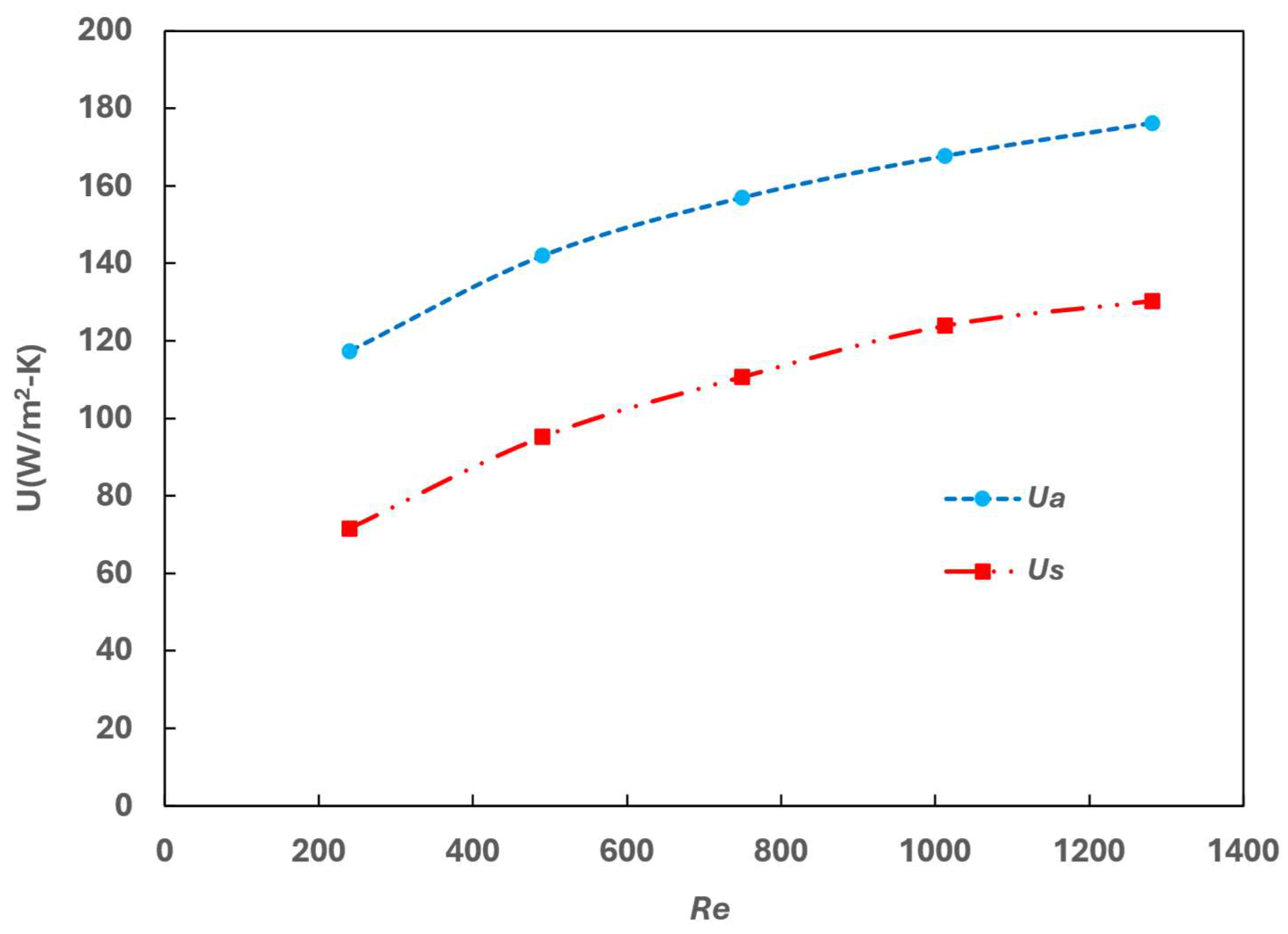
3.1.3. Verification of Pressure Drop
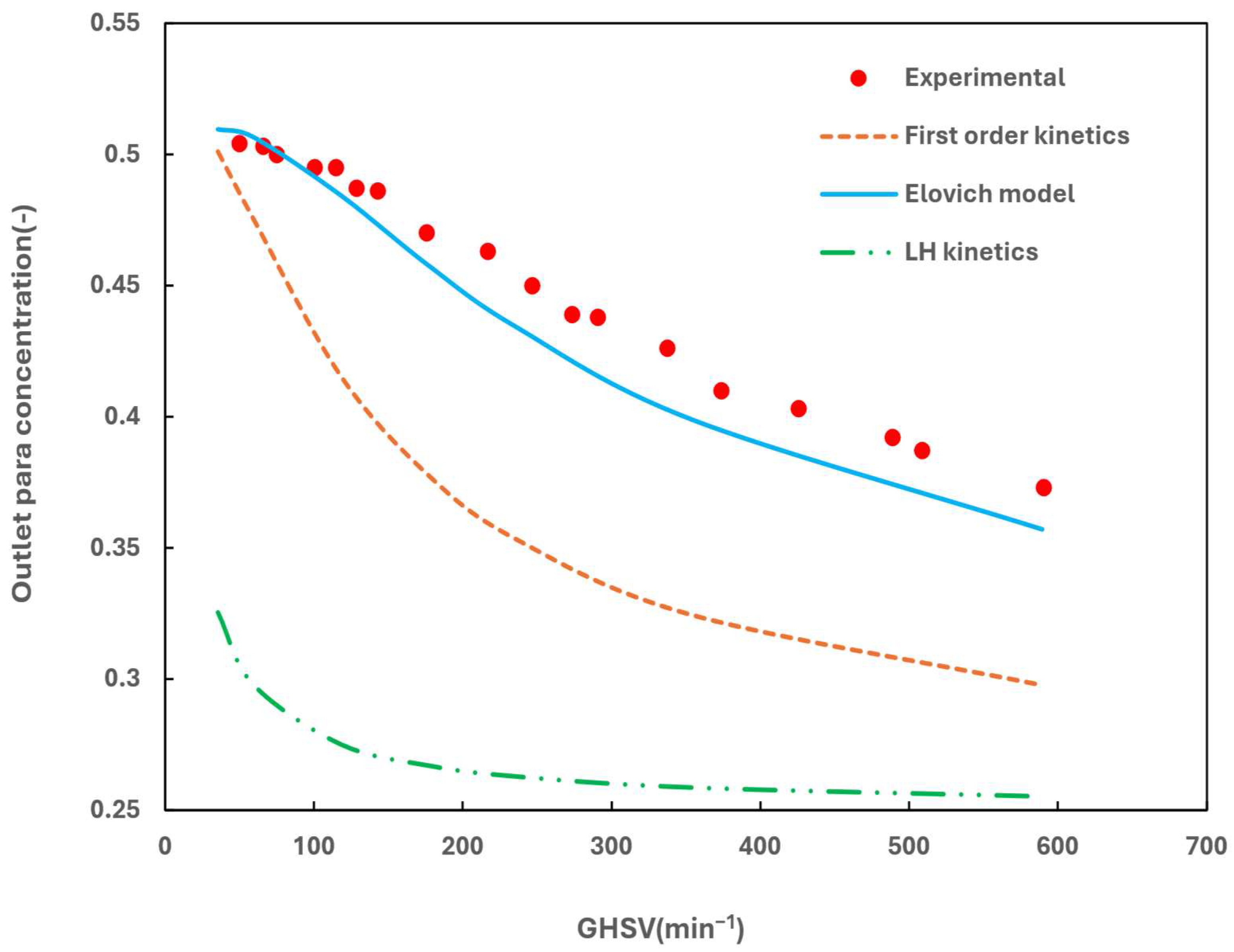
3.1.4. Verification of Kinetic Models
3.2. General Description of the Features of CPFHE
3.3. Sensitivity Analysis of CPFHE Model
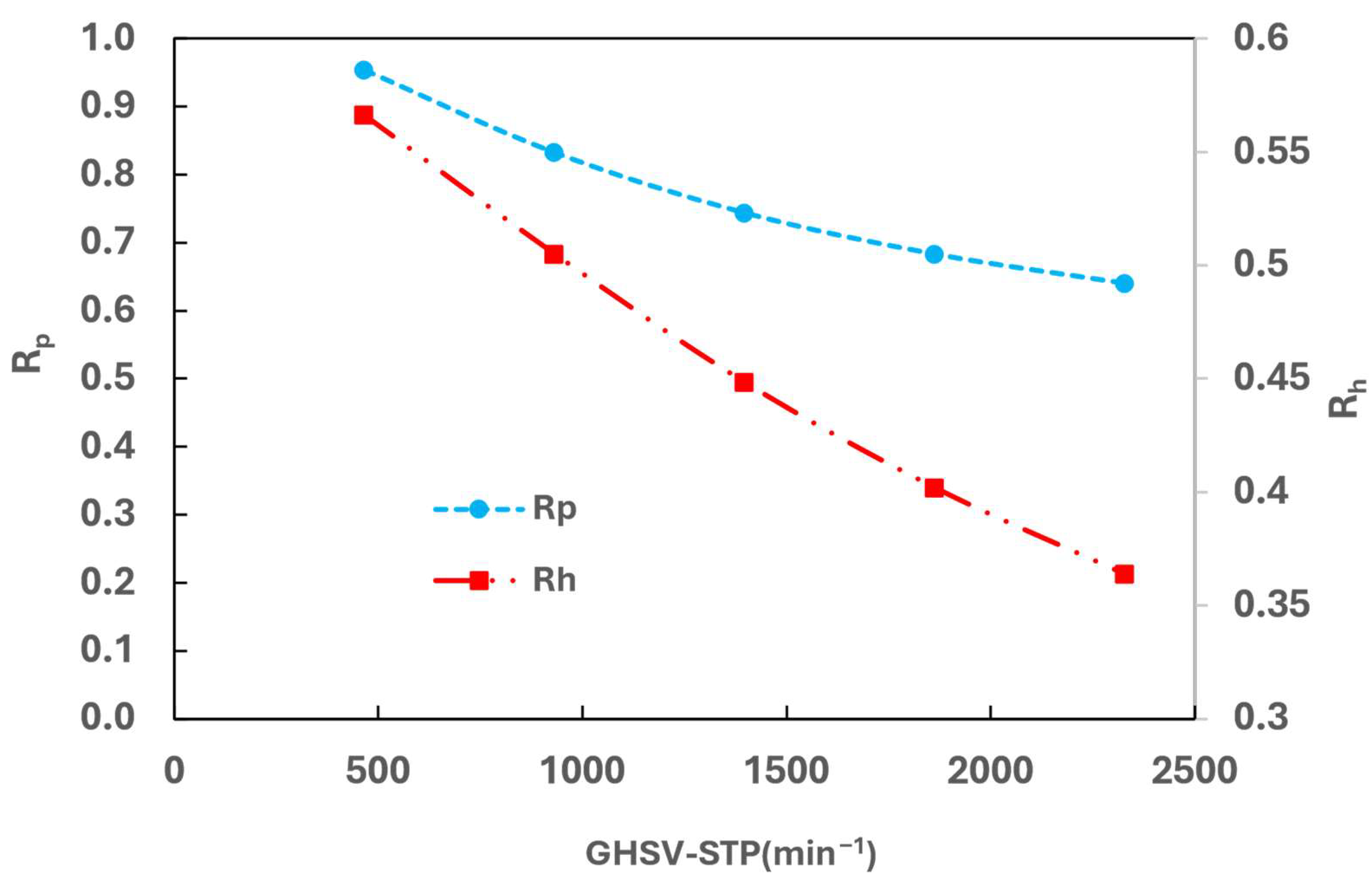
3.3.1. GHSV Effect
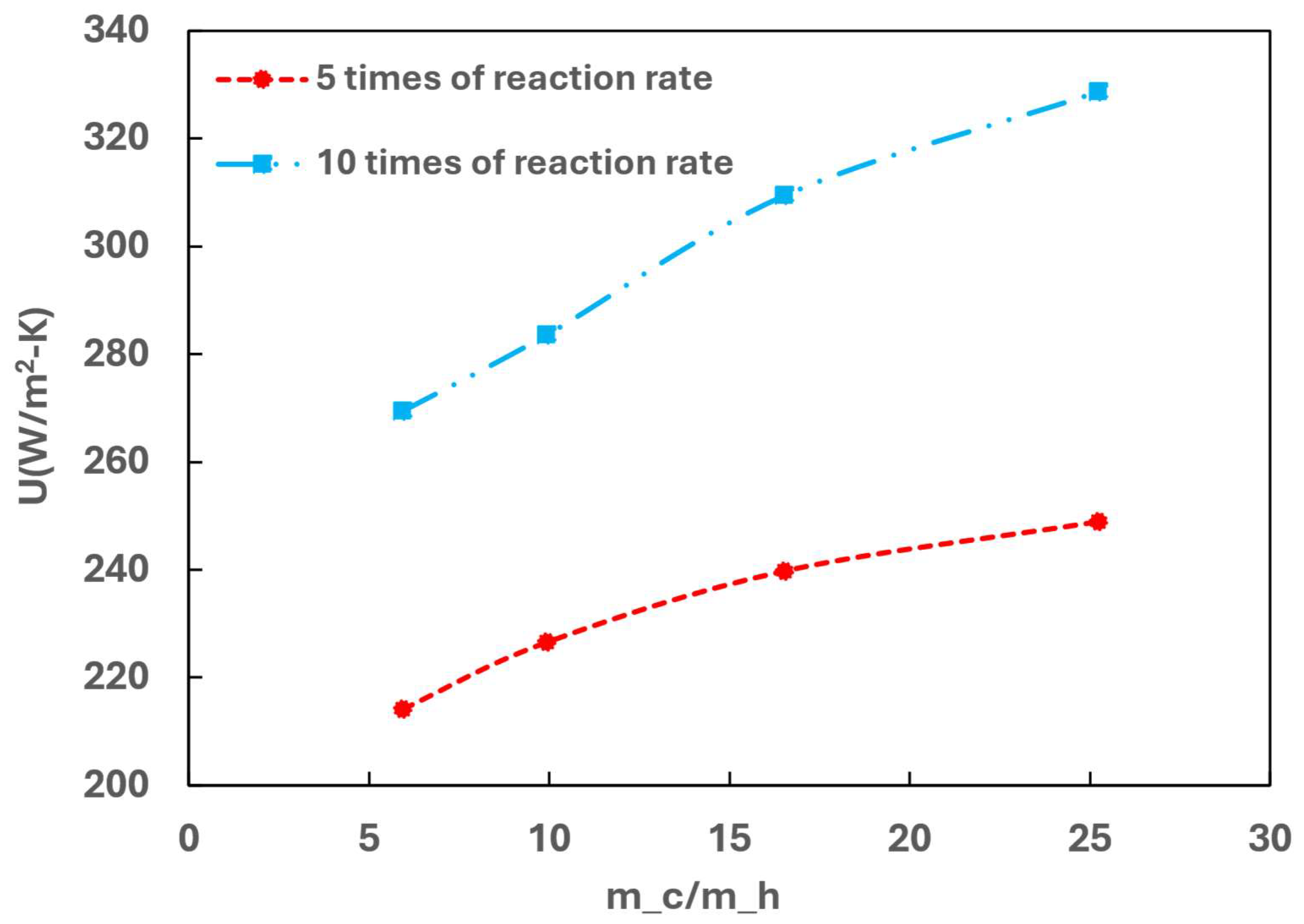
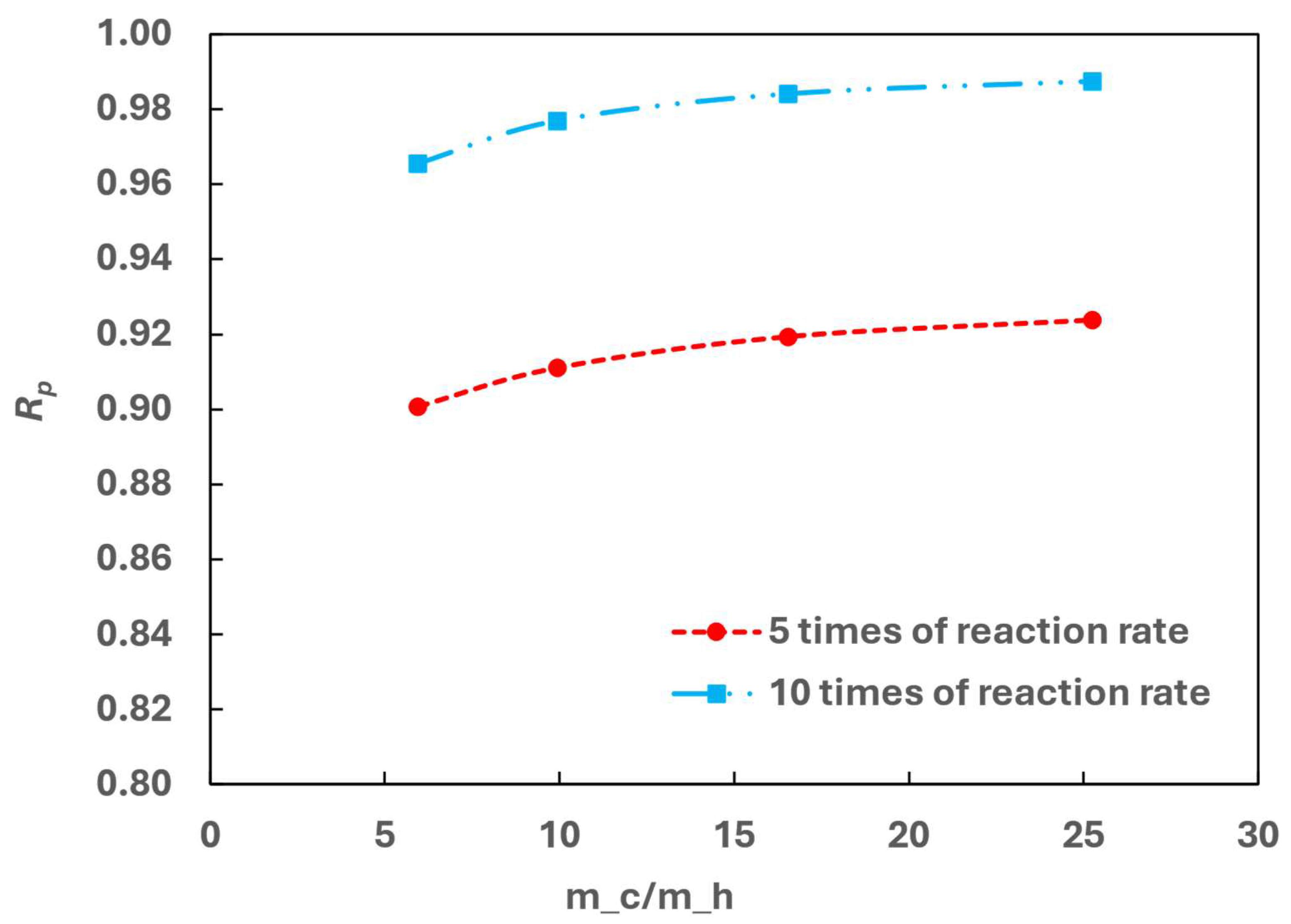
3.3.2. Cooling Effects
3.3.3. OPC Kinetic Effects
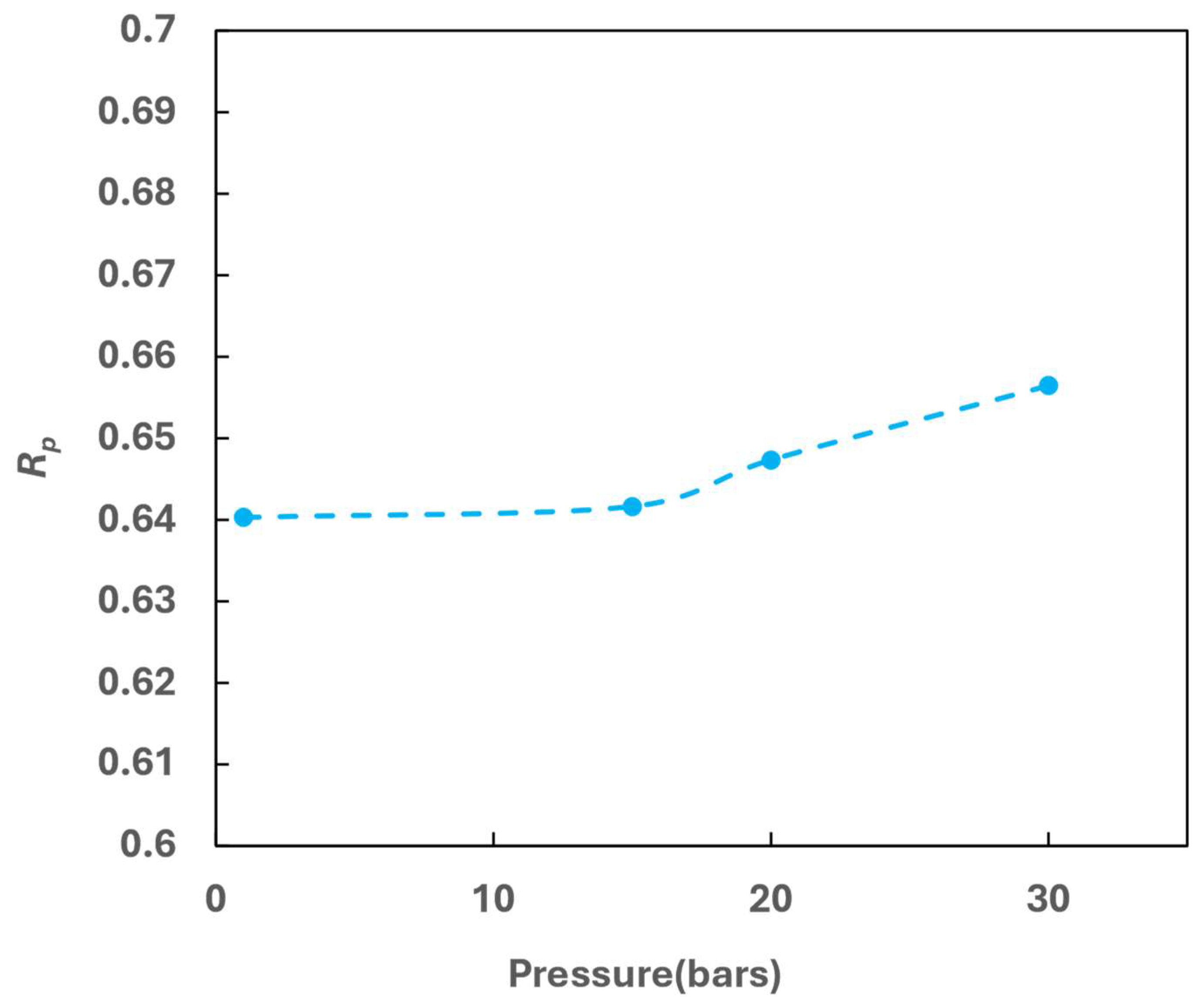
3.3.4. Effect of Operating Pressure
3.3.5. Pressure Drop
3.4. Discussion
4. Conclusions
- (1)
- Despite the compact nature of the PFHE with large heat transfer area and millimetre-scale fin spacing, simulations reveal non-uniform temperature distribution within the fin channels. The addition of a catalyst improved the temperature uniformity, with the catalyst possessing high thermal conductivity and further enhancing this uniformity.
- (2)
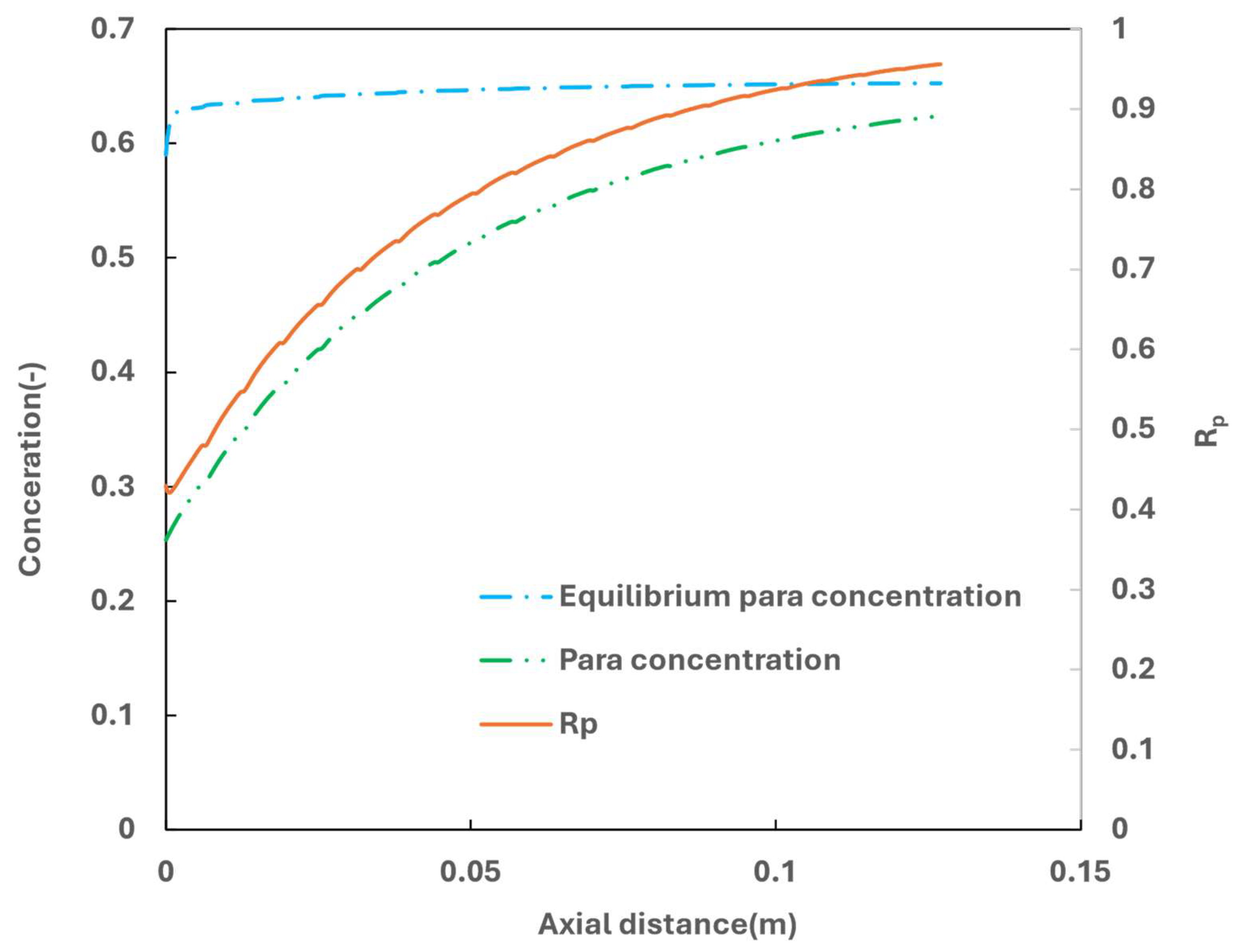
- Increasing the gas velocities of both the hot and cold media in the CPFHE channels can enhance the overall heat transfer coefficient. However, higher gas velocities in the hot channel results in a large GHSV, thus compromising the OPC performance. At a GHSV-STP of 2300 min−1, the ratio of actual para concertation to the equilibrium para concentration can only reach 0.65, irrespective of the heat transfer performance.
- (3)
- In large-scale hydrogen liquefaction systems requiring high GHSVs, OPC kinetics predominately dictate CPFHE performance. Improving heat transfer alone does not enhance the OPC performance; instead, developing catalysts with enhanced activity is crucial. A catalyst with kinetics 5 to 10 times more active than the current commercial catalyst can help para concertation reaching equilibrium at a GHSV-STP of 2300 min −1.
- (4)
- Incorporating a catalyst into fin channels increases the pressure drop significantly, which can reach approximately 400 kPa/m at a GHSV of 2300 min−1. However, the increased operating pressure will mitigate this, which also positively impacts the OPC performance marginally.
- (5)
- These findings underscore the complex interplay between heat transfer, OPC catalytic activity, and pressure drops in the CPFHE design for hydrogen liquefaction. Optimizing the CPFHE operating parameters necessitates minimizing exergy destruction due to heat transfer, pressure loss, and OPC reaction.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Nomenclature
| A | total heat transfer area, m2 |
| A(h,c,w) | heat transfer area of hot/cold channel/parting sheet, m2 |
| Cp | specific heat capacity, J/kg-K |
| CH2 | molar concentration of hydrogen, mol/m3 |
| Dh | hydraulic diameter of the fin, m |
| Ea | activation energy of OPC reaction, J/mol |
| Fanning friction factor from simulation/analytical correlation, - | |
| F | volume force vector, N/m3 |
| FF | viscous force due to Forchheimer and Ergun option, N/m3 |
| ha,(h,c) | heat transfer coefficient for cold/hot channel, W/m2-K |
| I | identity matrix, - |
| transport of chemical species (i) due to diffusion, mol/m2-s | |
| j(a) | Colburn number, - |
| effective thermal conductivity, W/m-K | |
| kp | permeability of catalyst bed, m2 |
| K | viscous stress tensor, Pa |
| and M | molar mass of species i and mixture, g/mol |
| Pk | production of turbulent kinetic energy, m2/s3 |
| Pc | critical pressure of hydrogen, MPa |
| P | pressure, Pa |
| Δp | pressure loss, Pa |
| q | heat flux by conduction, W/m2 |
| mass source, kg/m3-s | |
| Q | total heat transferred, W |
| Qconv | heat of conversion, J/mol. |
| reaction rate expression for the species i, mol/m3-s. | |
| Re | Reynolds number, calculated as , - |
| Tc | critical temperature of hydrogen, K |
| T | temperature, K |
| ΔT(A,B) | the temperature difference between the two fluids at end A/B, K |
| u | gas velocity, m/s |
| U(s,a) | the overall heat transfer coefficient calculated from simulation or analytical correlation, W/m2-K |
| V | velocity vector, m/s |
| mole fraction of species i and can be calculated as , - | |
| Greek symbols | |
| k | turbulent kinetic energy, m2/s2 |
| ɛ | turbulent dissipation, m2/s3 |
| porosity of catalyst bed, - | |
| fin temperature effectiveness of the cold/hot channel, - | |
| µ | fluid dynamic viscosity, Pa-s |
| eddy viscosity and is given as , m2/s | |
| mass fraction of species (i), - | |
| ρ | density, kg/m3 |
| λ(h,c,w) | thermal conductivity of fluid or hot/cold channel/parting sheet, W/m-K, |
| Subscript | |
| a | from analytic correlation |
| c | cold side |
| h | hot side |
| s | from numerical simulation |
| w | parting sheet |
References
- Al Ghafri, S.Z.S.; Munro, S.; Cardella, U.; Funke, T.; Notardonato, W.; Trusler, J.P.M.; Leachman, J.; Span, R.; Kamiya, S.; Pearce, G.; et al. Hydrogen liquefaction: A review of the fundamental physics, engineering practice and future opportunities. Energy Environ. Sci. 2022, 15, 2690–2731. [Google Scholar] [CrossRef]
- Aziz, M. Liquid Hydrogen: A Review on Liquefaction, Storage, Transportation, and Safety. Energies 2021, 14, 5917. [Google Scholar] [CrossRef]
- Lipman, M.S.; Cheung, H.; Roberts, O.P. Continuous conversion hydrogen liquefaction. Chem. Eng. Prog. 1963, 59, 49–54. [Google Scholar]
- Teng, J.J.; Wang, K.; Zhu, S.L.; Bao, S.R.; Zhi, X.Q.; Zhang, X.B.; Qiu, L.M. Comparative study on thermodynamic performance of hydrogen liquefaction processes with various ortho-para hydrogen conversion methods. Energy 2023, 271, 127016. [Google Scholar] [CrossRef]
- Krasae-in, S.; Stang, J.H.; Neksa, P. Development of large-scale hydrogen liquefaction processes from 1898 to 2009. Int. J. Hydrog. Energy 2010, 35, 4524–4533. [Google Scholar] [CrossRef]
- Xu, P.; Wen, J.; Li, K.; Wang, S.M.; Li, Y.Z. Review of the continuous catalytic ortho-para hydrogen conversion technology for hydrogen liquefaction. Int. J. Hydrog. Energy 2024, 62, 473–487. [Google Scholar] [CrossRef]
- Popov, D.; Fikiin, K.; Stankov, B.; Alvarez, G.; Youbi-Idrissi, M.; Damas, A.; Evans, J.; Brown, T. Cryogenic heat exchangers for process cooling and renewable energy storage: A review. Appl. Therm. Eng. 2019, 153, 275–290. [Google Scholar] [CrossRef]
- London, A.L.; Shah, R.K. Offset Rectangular Plate-Fin Surfaces—Heat Transfer and Flow Friction Characteristics. J. Eng. Gas Turbines Power 1968, 90, 218–228. [Google Scholar] [CrossRef]
- Wang, Z.; Li, Y.Z. Layer pattern thermal design and optimization for multistream plate-fin heat exchangers—A review. Renew. Sustain. Energy Rev. 2016, 53, 500–514. [Google Scholar] [CrossRef]
- Meagher, J.P. Modelling of Hydrogen Liquefiers with Kinetic Conversion of Ortho to Para Hydrogen in Plate-Fin Heat Excahngers. Master’s Thesis, Department of Chemical and Biological Engineering, University at Buffalo, State University of New York, Buffalo, NY, USA, 2009. [Google Scholar]
- Hutchinson, H.L. Analysis of Catalytic Ortho-Parahydrogen Reaction Mechanisms. Ph.D. Dissertation, Department of Chemical Engineering, University of Colorado, Boulder, CO, USA, 1966. [Google Scholar]
- Wilhelmsen, Ø.; Berstad, D.; Aasen, A.; Neksa, P.; Skaugen, G. Reducing the exergy destruction in the cryogenic heat exchangers of hydrogen liquefaction processes. Int. J. Hydrog. Energy 2018, 43, 5033–5047. [Google Scholar] [CrossRef]
- Donaubauer, P.J.; Cardella, U.; Decker, L.; Klein, H. Kinetics and Heat Exchanger Design for Catalytic Ortho-Para Hydrogen Conversion during Liquefaction. Chem. Eng. Technol. 2019, 42, 669–679. [Google Scholar] [CrossRef]
- Park, J.; Lim, H.; Rhee, G.H.; Karng, S.W. Catalyst filled heat exchanger for hydrogen liquefaction. Int. J. Heat Mass Transf. 2021, 170, 121007. [Google Scholar] [CrossRef]
- O’Neill, K.T.; Al Ghafri, S.; da Silva Falc, B.; Tang, L.G.; Kozielski, K.; Johns, M.L. Hydrogen ortho-para conversion: Process sensitivities and optimisation. Chem. Eng. Process. Process Intensif. 2023, 184, 109272. [Google Scholar] [CrossRef]
- Xu, P.; Lei, G.; Xu, Y.Y.; Wen, J.; Wang, S.M.; Li, Y.Z. Study on continuous cooling process coupled with ortho-para hydrogen conversion in plate-fin heat exchanger filled with catalyst. Int. J. Hydrog. Energy 2022, 47, 4690–4703. [Google Scholar] [CrossRef]
- Zhu, S.L.; Teng, J.J.; Zhi, X.Q.; Bao, S.R.; Qiu, L.M.; Wang, K. Numerical study on comprehensive performance of flow and heat transfer coupled with ortho-para hydrogen conversion. Int. J. Heat Mass Transf. 2023, 201, 123653. [Google Scholar] [CrossRef]
- COMSOL. Multiphysics Reference Manual, version 6.2; COMSOL, Inc.: Stockholm, Sweden, 2023. Available online: www.comsol.com (accessed on 25 July 2024).
- Molecular Products Inc. Ionex® Type OP Catalyst. 2024. Available online: https://www.molecularproducts.com/products/ionex-type-op-catalyst (accessed on 15 January 2024).
- Leachman, J.W.; Jacobsen, R.T.; Penoncello, S.G.; Lemmon, E.W. Fundamental Equations of State for Parahydrogen, Normal Hydrogen, and Orthohydrogen. J. Phys. Chem. Ref. Data 2009, 38, 721–748. [Google Scholar] [CrossRef]
- Lemmon, E.W.; Bell, I.H.; Huber, M.L.; McLinden, M.O. NIST Standard Reference Database 23, NIST Reference Fluid Thermodynamic and Transport Properties, Version 10.0. 2018. Available online: https://www.nist.gov/srd/refprop (accessed on 20 March 2024).
- van Baten, J. REFPROP CAPE-OPEN Property Package Manager. 2024. Available online: https://www.amsterchem.com/refpropco.html (accessed on 20 March 2024).
- Manglik, R.M.; Bergles, A.E. Heat Transfer and Pressure Drop Correlations for the Rectangular Offset Strip Fin Compact Heat Exchanger. Exp. Therm. Fluid Sci. 1995, 10, 171–180. [Google Scholar] [CrossRef]
- Kays, W.M.; London, A.L. Compact Heat Exchangers; Vinod Kumar Jain, Scientific International (Pvt.) Ltd.: New Delhi, Inida, 2018. [Google Scholar]
- Webb, R.L.; Kim, N.H. Principles of Enhanced Heat Transfer, 2nd ed.; Taylor & Francis Group: New York, NY, USA, 2005. [Google Scholar]
- Schmauch, G.E.; Singleton, A.H. Technical aspects of ortho-parahydrogen conversion. Ind. Eng. Chem. 1964, 56, 20–31. [Google Scholar] [CrossRef]
- Weitzel, D.H.; Van Valin, C.C.; Draper, J.W. Design data for ortho-parahydrogen converters. In Proceedings of the 1957 Cryogenic Engineering Conference, National Bureau of Standards, Boulder, CO, USA, 19–21 August 1957; Springer: Boston, MA, USA, 1960. [Google Scholar]
- Wakao, N.; Smith, J.M.; Selwood, P.W. The low-temperature orthohydrogen conversion over supported oxides and metals. J. Catal. 1962, 1, 62–73. [Google Scholar] [CrossRef]
- Wieting, A.R. Empirical Correlations for Heat Transfer and Flow Friction Characteristics of Rectangular Offset-Fin Plate-Fin Heat Exchangers. Trans. ASME 1975, 97, 488–490. [Google Scholar] [CrossRef]
- Yang, H.Z.; Li, Y.Y.; Yang, Y.; Zhu, Y.G.; Wen, J. Effect of surface efficiency on the thermal design of plate-fin heat exchangers with passages stack arrangement. Int. J. Heat Mass Transf. 2019, 143, 118494. [Google Scholar] [CrossRef]
- Pérez-Ramírez, J.; Berger, R.J.; Mul, G.; Kapteijn, F.; Moulijn, J.A. The six-flow reactor technology: A review on fast catalyst screening and kinetic studies. Catal Today 2000, 60, 93–109. [Google Scholar] [CrossRef]
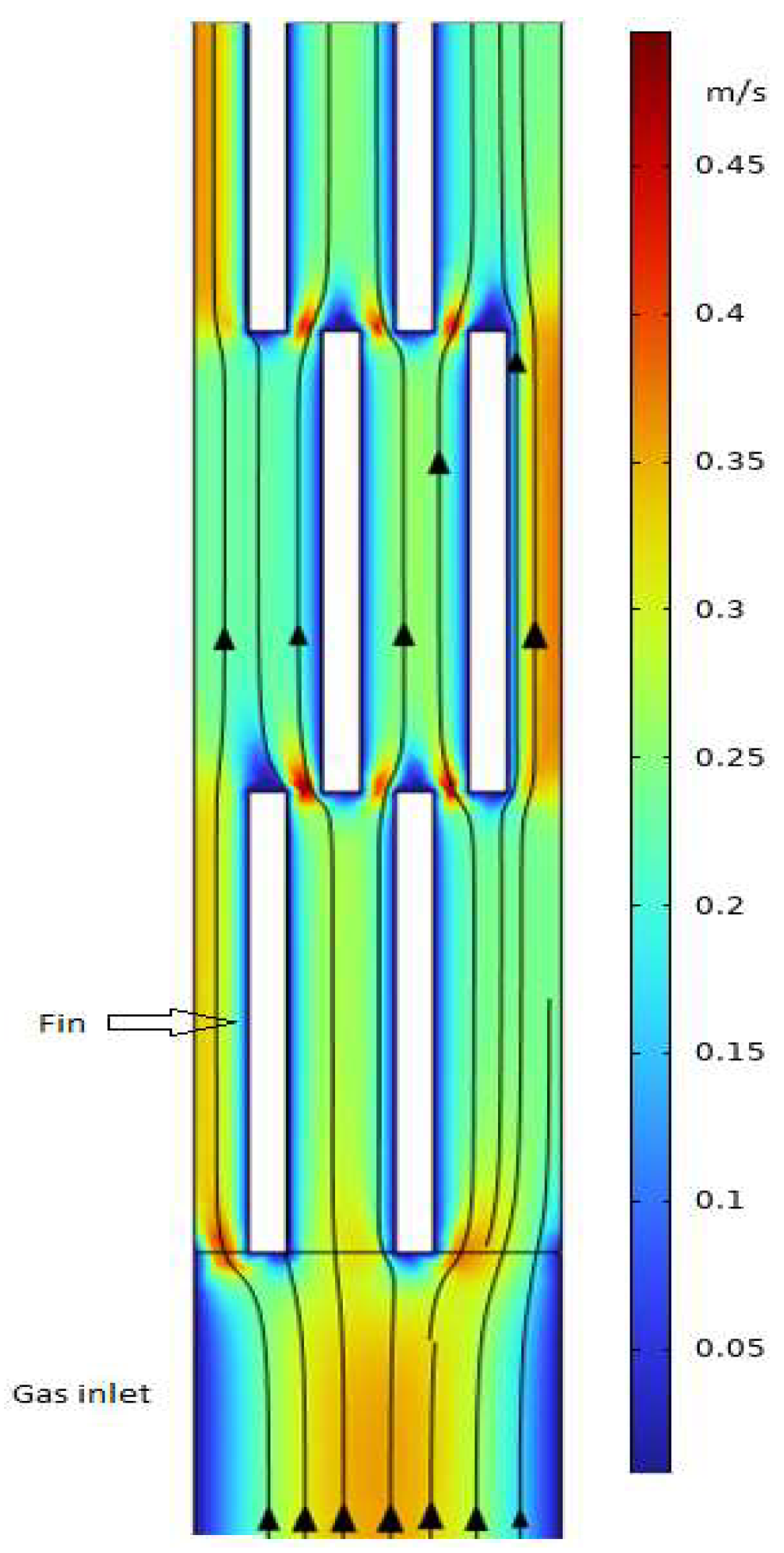
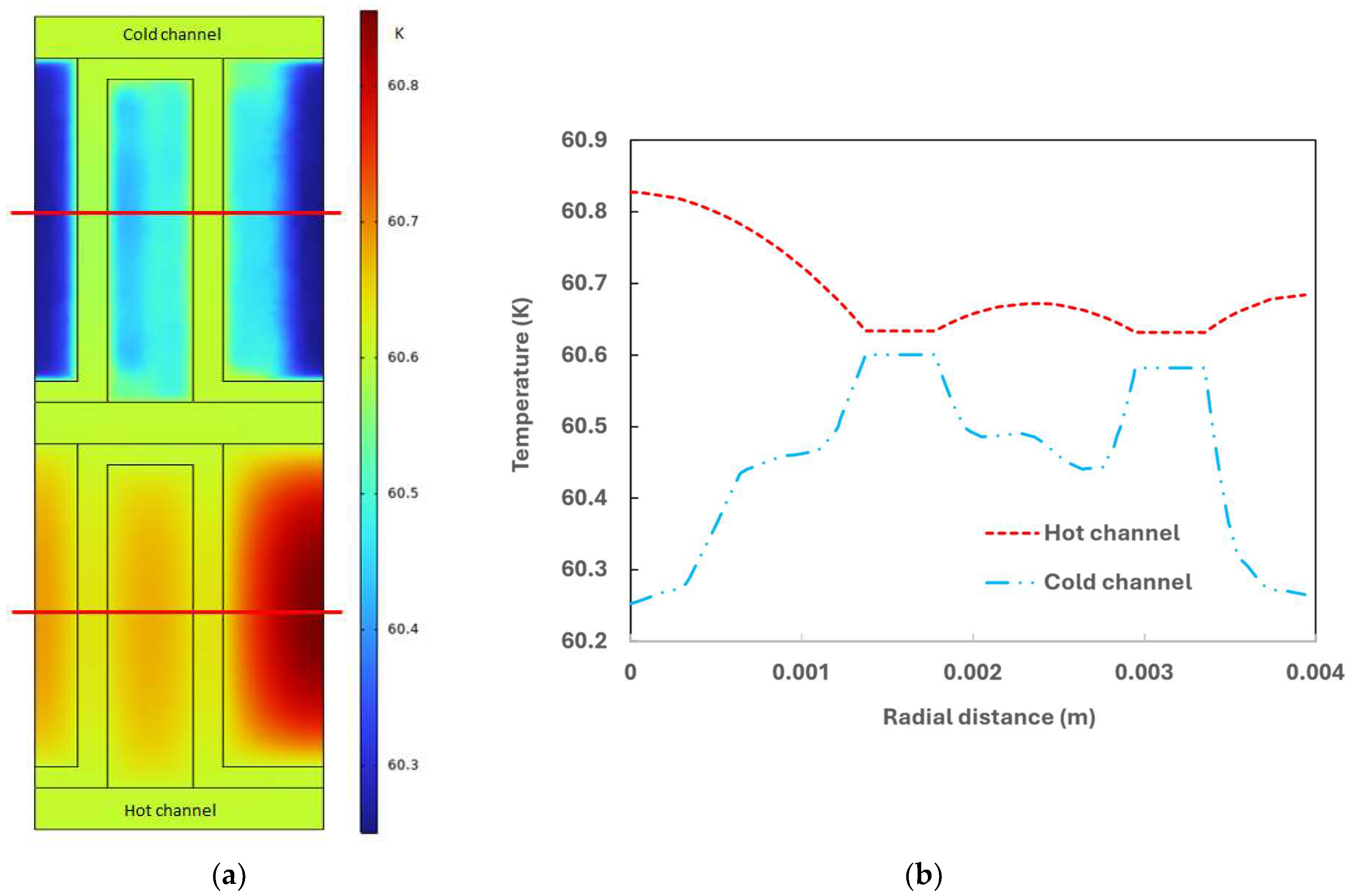
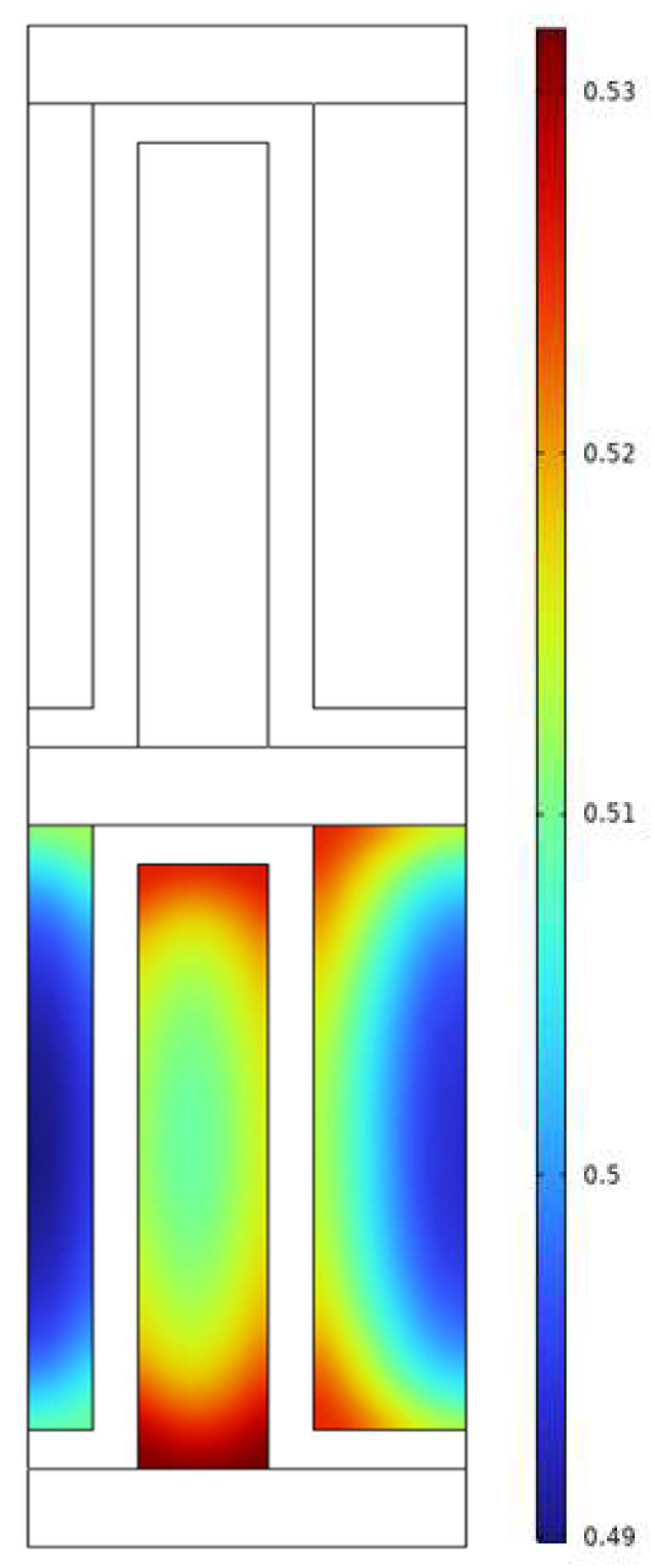
 ) and cold (
) and cold ( ) channels derived from fine mesh; (b) Effect of the mesh size on the logarithmic mean temperature difference.
) channels derived from fine mesh; (b) Effect of the mesh size on the logarithmic mean temperature difference.
 ) and cold (
) and cold ( ) channels derived from fine mesh; (b) Effect of the mesh size on the logarithmic mean temperature difference.
) channels derived from fine mesh; (b) Effect of the mesh size on the logarithmic mean temperature difference.







| Symbol | Item | Length (mm) | Comments |
|---|---|---|---|
| ac | Fin altitude | 6.7056 | 0.264 inch |
| tc | Fin thickness | 0.4064 | 0.016 inch |
| sc | Fin spacing | 1.1684 | 0.046 inch |
| lc | Fin serration length | 6.35 | 0.25 inch |
| ls | Stagged length | 0.5842 | 0.023 inch |
| ts | Parting sheet thickness | 0.8128 | 0.032 inch |
| lt | PFHE length | 127 | 20 × lc |
| linout | Free flow path length | 15.875 | 2.5 × lc |
| Material | Density (kg/m3) | Thermal Conductivity (W/m·K) | Specific Heat (J/kg·K) | Porosity (-) | Permeability (m2) |
|---|---|---|---|---|---|
| Aluminium | 2700 | 138 | 334 | ||
| Ionex® | 5240 | 0.58 | 700 | ||
| Catalyst bed formed by Ionex® particles | 0.5 | 1.8 × 10−11 |
| Parameter | Unit | Value | Parameter | Unit | Value |
|---|---|---|---|---|---|
| J/mol | −586.28 | J/mol | 20.99 | ||
| a | ×103 m3s/mol | 9.87 | a′ | −1.15 | |
| b | ×103 s | −144.5 | b′ | mol/m3 | 132.28 |
| Parameter | Unit | Value |
|---|---|---|
| J/mol | −336.45 | |
| afirst | ×103 m3s/mol | 2.2 |
| bfirst | ×103 s | −35.11 |
| Parameters | Unit | Value |
|---|---|---|
| 1.0924 | ||
| b | 0.0597 | |
| c | −0.2539 | |
| d | −0.0116 | |
| Tc | K | 32.937 |
| Pc | MPa | 1.28377 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tang, L.; Yamaguchi, D.; Orellana, J.; Tian, W. Multiphysics Studies of 3D Plate Fin Heat Exchanger Filled with Ortho-Para-Hydrogen Conversion Catalyst for Hydrogen Liquefaction. Hydrogen 2024, 5, 682-709. https://doi.org/10.3390/hydrogen5040036
Tang L, Yamaguchi D, Orellana J, Tian W. Multiphysics Studies of 3D Plate Fin Heat Exchanger Filled with Ortho-Para-Hydrogen Conversion Catalyst for Hydrogen Liquefaction. Hydrogen. 2024; 5(4):682-709. https://doi.org/10.3390/hydrogen5040036
Chicago/Turabian StyleTang, Liangguang, Doki Yamaguchi, Jose Orellana, and Wendy Tian. 2024. "Multiphysics Studies of 3D Plate Fin Heat Exchanger Filled with Ortho-Para-Hydrogen Conversion Catalyst for Hydrogen Liquefaction" Hydrogen 5, no. 4: 682-709. https://doi.org/10.3390/hydrogen5040036
APA StyleTang, L., Yamaguchi, D., Orellana, J., & Tian, W. (2024). Multiphysics Studies of 3D Plate Fin Heat Exchanger Filled with Ortho-Para-Hydrogen Conversion Catalyst for Hydrogen Liquefaction. Hydrogen, 5(4), 682-709. https://doi.org/10.3390/hydrogen5040036






