Is There No Need to Consider the Influence of Ion Adsorption and the Hofmeister Effect for the Precise Evaluation of Membrane Potential?

Abstract

Within the field of physiology, it is widely recognized that the constant flow of mobile ions across the plasma membrane generates membrane potential in living cells. This understanding is a part of the membrane theory. Despite this, membrane theory does not account for the role of ion adsorption (or desorption) processes in generating membrane potential, even though ion adsorption is a key concept in basic thermodynamics. Presently, the study of physiology lacks integration with thermodynamic principles. The membrane theory posits that living cells can differentiate between Na⁺ and K⁺ by means of channels and pumps. Thus, Na⁺ and K⁺ differentially impact the membrane potential. On the other hand, the Hofmeister effect, an older and less prominent thermodynamic theory, proposes that Na⁺ and K⁺ have varying adsorption levels to biomolecules, potentially accounting for their distinct effects on membrane potential even without the involvement of channels and pumps. This concept, distinct from the traditional membrane theory and grounded in ion adsorption (desorption) alongside the Hofmeister effect, might elucidate the process of membrane potential formation. This ion adsorption (desorption) and Hofmeister effect-based idea relates to the previously overlooked Association-Induction Hypothesis (AIH). Our experimental measurements of membrane potentials using artificial cell models highlight that ion adsorption activity and the Hofmeister effect have a comparable impact on the generation of membrane potential as ion flow in the conventional physiological model, assisted by channels and pumps.

1. Introduction

In the last ten years, we have rigorously assessed the shortcomings of the Goldman–Hodgkin–Katz (GHK) equation and explored the Association-Induction Hypothesis (AIH) as an alternative mechanism for generating membrane potential [1,2,3,4,5,6].

Initially introduced by Goldman, the physiological interpretation of the GHK equation was later expanded by Hodgkin and Katz [2]. Due to the extensive use of the GHK equation and the pivotal contributions of these three scientists, it continues to be recognized within the field [2,7]. The GHK equation emerges from membrane theory, which posits that ion channels and pumps embedded in the plasma membrane facilitate ongoing ion transport. This equation assumes that such ion transport is a continuous process in living cells [2,3,4].

The Association-Induction Hypothesis (AIH), a relatively obscure theory introduced by Gilbert Ling [2,3,4], offers an alternative explanation for the membrane potential generation mechanism. Despite its fundamental differences from the GHK equation, AIH suggests that the membrane potential arises from a non-uniform distribution of charges because of the adsorption of mobile ions. In contrast to the GHK equation, the AIH mechanism does not necessitate the continuous transport of ions across the plasma membrane for membrane potential generation. As a result, this AIH-based explanation is applicable to both living and nonliving systems

Tamagawa and Ikeda made an intriguing discovery that a formula for membrane potential, identical to the GHK equation, can be derived by employing the AIH mechanism [8]. For instance, if the GHK equation relevant to the system is described by Equation (1), then the AIH-based potential formula is represented by Equation (2). The distinction between them lies solely in the definitions of $P_i$ and $K_i$. Here, $P_i$ is the membrane permeability coefficient for the mobile ion i, whereas $K_i$ denotes the binding constant for the interaction between the mobile ion i and its adsorption site. Nevertheless, the expressions in Equations (1) and (2) remain identical.

$$\begin{array}{c}\hfill {\psi }^{GHK}=-{\displaystyle \frac{kT}{e}}ln{\displaystyle \frac{P_K{\left[K^{+}\right]}_{in}+P_{Cl}{\left[C{l}^{-}\right]}_{out}}{P_K{\left[K^{+}\right]}_{out}+P_{Cl}{\left[C{l}^{-}\right]}_{in}}}\end{array}$$

(1)

$$\begin{array}{c}\hfill {\psi }^{AIH}=-{\displaystyle \frac{kT}{e}}ln{\displaystyle \frac{K_K{\left[K^{+}\right]}_{in}+K_{Cl}{\left[C{l}^{-}\right]}_{out}}{K_K{\left[K^{+}\right]}_{out}+K_{Cl}{\left[C{l}^{-}\right]}_{in}}}\end{array}$$

(2)

The GHK equation precisely replicates the membrane potential observed experimentally. Researchers often endeavor to determine the appropriate numerical values for $P_i$ so that the GHK equation aligns with the empirical potential data. Consequently, $P_i$ is not measured directly experimentally but instead is estimated to make the GHK equation align with the data [9,10,11,12]. Our repeated confirmations show that the potential produced across an impermeable membrane separating two electrolytic solutions can still be modeled using the GHK equation with hypothetical non-zero values for $P_i$, even though logically, $P_i$ should be zero given the membrane’s impermeability [8,13]. Therefore, adjusting $P_i$ by trial and error until the calculated potential of the GHK equation matches the experimental results lacks scientific validity. In contrast, the AIH-based equation (Equation (2)), taking into account ion adsorption, is valid regardless of membrane permeability.

In this study, we assess the membrane potential of our synthetic cell models. Subsequently, we conduct a theoretical analysis of the potential data. Through this theoretical analysis, we arrived at an old and less well-known thermodynamic theory called the Hofmeister effect [14,15,16,17,18,19]. We found that the Hofmeister effect aligns with the AIH mechanism and concluded that the membrane potential characteristics can be explained by the Hofmeister effect. Consequently, we reached the conclusion that the membrane potential must be non-negligibly influenced by the AIH mechanism, even though this finding does not necessarily require abandoning the conventional membrane potential generation mechanism.

2. Materials and Measurements

This section describes the processing procedure of the specimens and the experimental procedure. They are basically the same as those described in the ref. [20], and some of the experimental data (experimentally measured potential data) are adopted from the ref. [20].

2.1. Speciment Preparation

Two distinct triple-layered artificial membranes were manufactured. The first type was assembled by bonding the Selemion CMVN, a cation-exchange membrane produced by Asahi Glass Co., Ltd. (Tokyo, Japan) with a polypropylene film (PP), using a 16 wt% PVA aqueous solution as a linking agent. This configuration is designated as C-memb and is depicted in Figure 1a. The second type was constructed by substituting the Selemion CMVN with the Selemion AMVN, an anion exchange membrane also manufactured by Asahi Glass Co., Ltd. (Tokyo). This configuration is termed A-memb and is illustrated in Figure 1b.

The following electrolytic solutions were prepared: 10⁻⁵ M∼1 M KCl solutions, 10⁻⁵ M∼1 M NaCl solutions, 10⁻⁵ M∼10⁻¹ M SDS solutions and 10⁻⁵ M∼1 M LiCl solutions.

2.2. Measurements

Two electrolytic solutions were divided by the C-memb (A-memb), and the membrane potential across the C-memb (A-memb) was recorded. Figure 2 illustrates a standard experimental arrangement. The Selemion CMVN, a cation exchange membrane for C-memb, interfaces with the electrolytic solution on the left (sol-L), while the polypropylene (PP) of C-memb interfaces with the right-side electrolytic solution (sol-R). The reference zero membrane potential is defined as the bulk phase potential of sol-R.

3. Results and Discussion

3.1. Membrane Potential in GHK Equation Perspective and in AIH Perspective

Displayed here are the membrane potentials experimentally determined by using different combinations of electrolytic solutions for sol-L and sol-R.

3.1.1. sol-L = KCl Solution, sol-R = KCl Solution

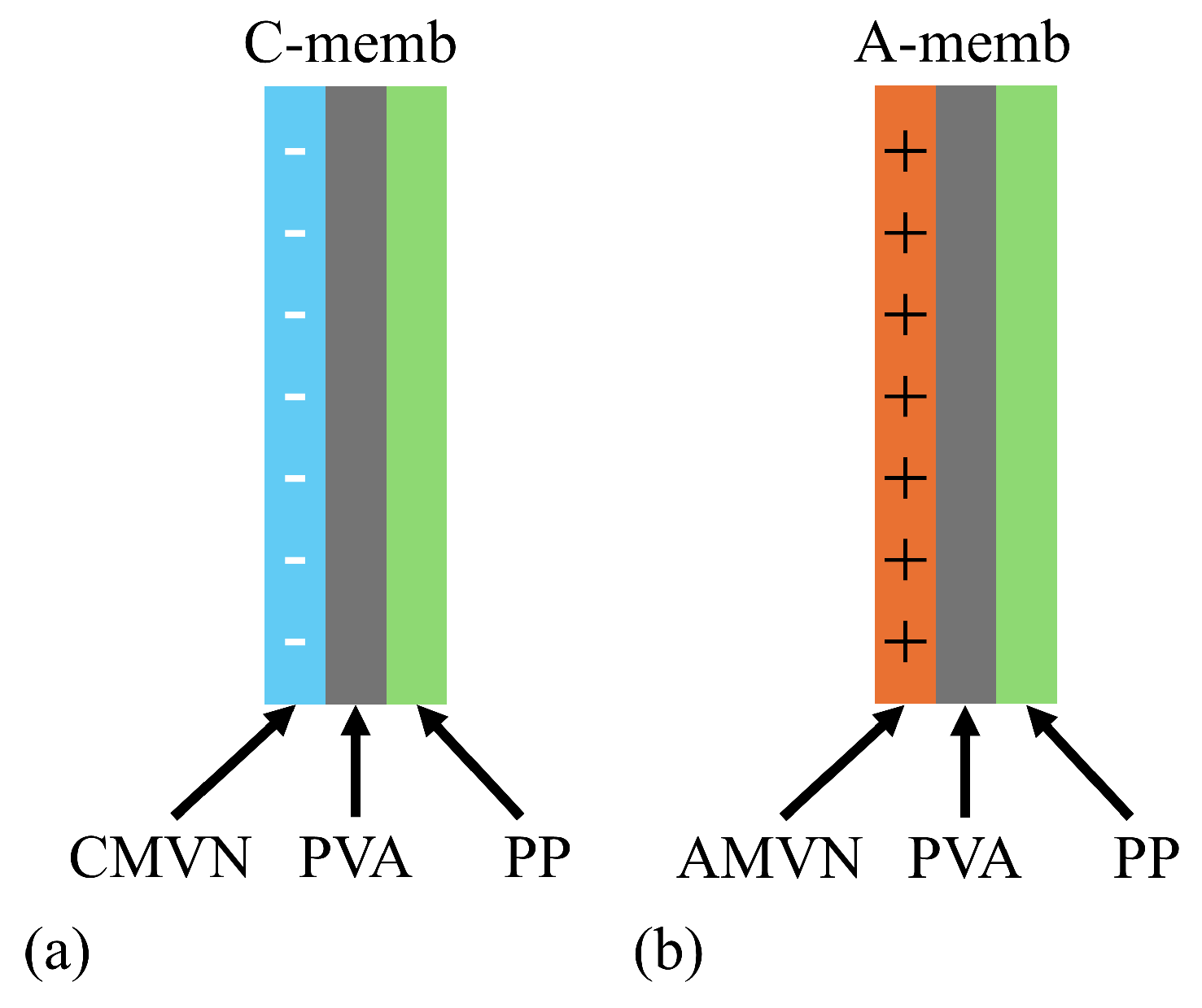
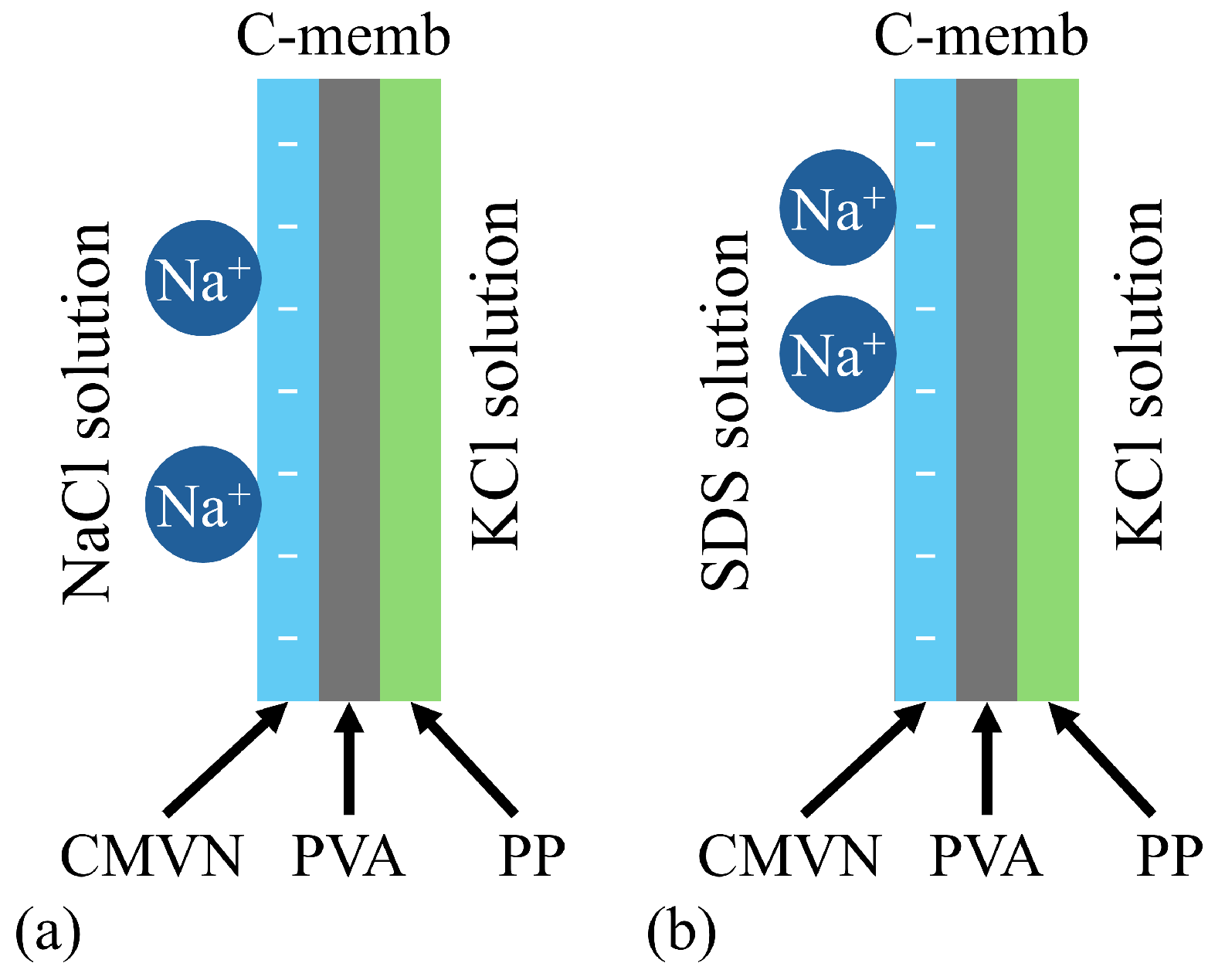
Initially, we outline the method for determining the membrane potential. For instance, a C-memb serves as a barrier between sol-L and sol-R, as illustrated in Figure 2. The experimental conditions are summarized in

Table 1. sol-L = KCl solution; sol-R = KCl solution; membrane = C-memb.

sol-L/M	${10}^{-5}$	${10}^{-4}$	${10}^{-3}$	${10}^{-2}$	${10}^{-1}$	1
C_KK-5	sol-R = 10−5 M
C_KK-4	sol-R = 10−4 M
C_KK-3	sol-R = 10−3 M
C_KK-2	sol-R = 10−2 M
C_KK-1	sol-R = 10−1 M
C_KK0	sol-R = 100 (=1) M

, and the experimental procedure under condition C_KK-5 is detailed step by step below as an example:

• The C-memb is placed in the center of the container (see Figure 2).
• A 10⁻⁵ M KCl solution is poured into the right phase (sol-R).
• A 10⁻⁵ M KCl solution is poured into the left phase (sol-L).
• The membrane potential measurement is conducted.
• Next, another potential measurement is performed after replacing sol-L with a 10⁻⁴ KCl solution.
• The same process as the previous step is repeated, progressively increasing the sol-L concentration from 10⁻⁵ M KCl to 1 M KCl.
• Subsequently, sol-R is changed from a 10⁻⁵ M KCl solution to a 10⁻⁴ M KCl solution, where this condtion (sol-R = 10⁻⁴ M KCl solution) is denoted by C_KK-4, as shown in Table 1.
• The sol-L KCl concentration is adjusted from 10⁻⁵ M to 1 M while monitoring the membrane potential.
• This entire procedure is iterated to systematically vary sol-R’s KCl concentration from 10⁻⁵ M to 1 M.

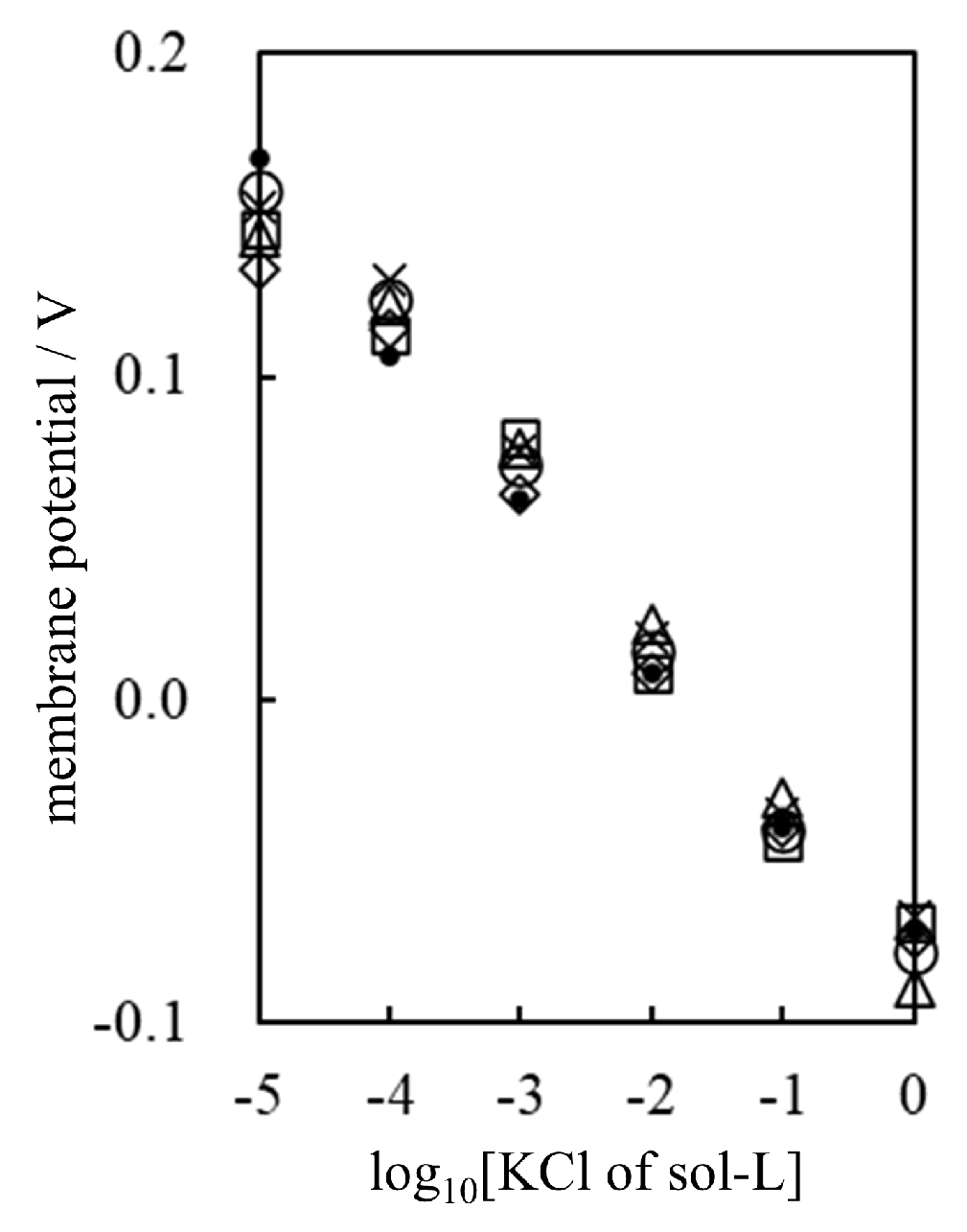
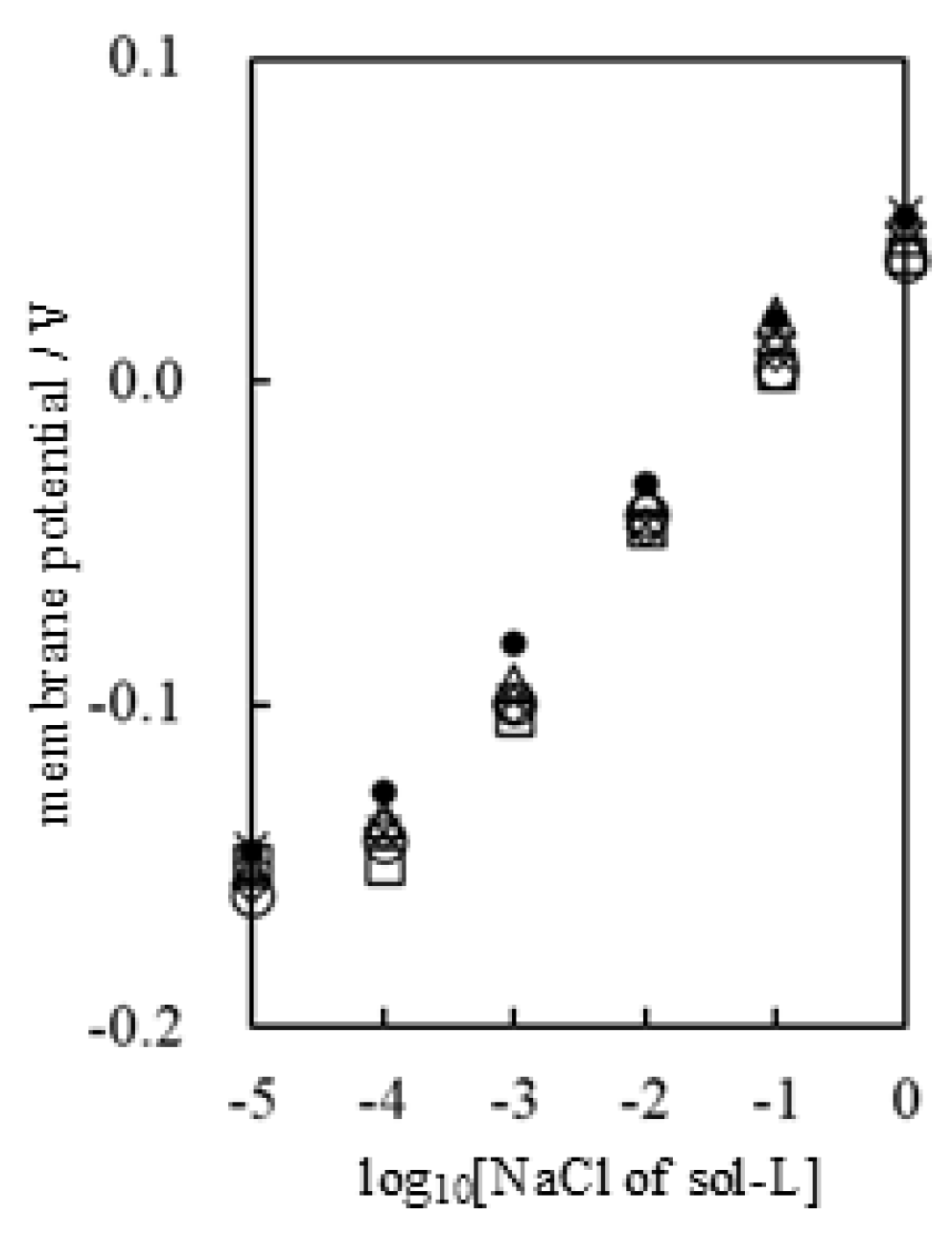
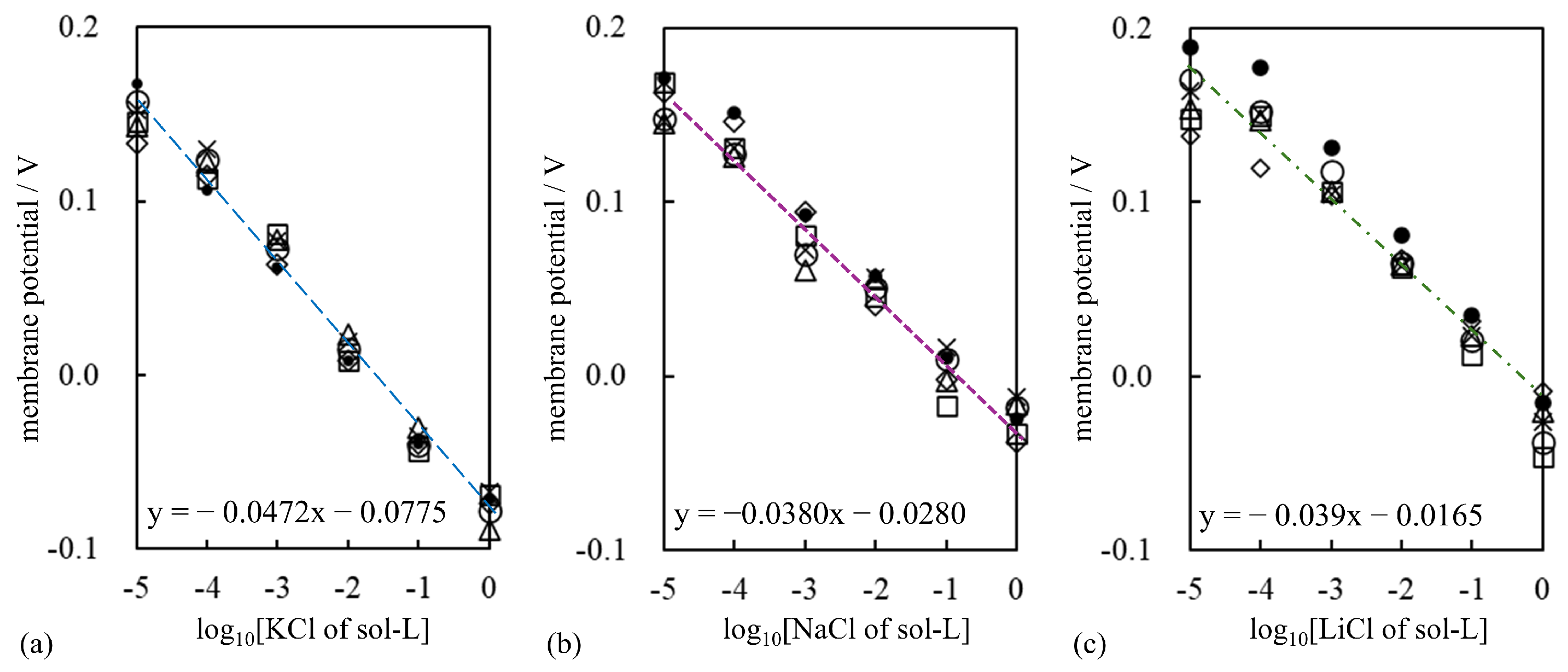
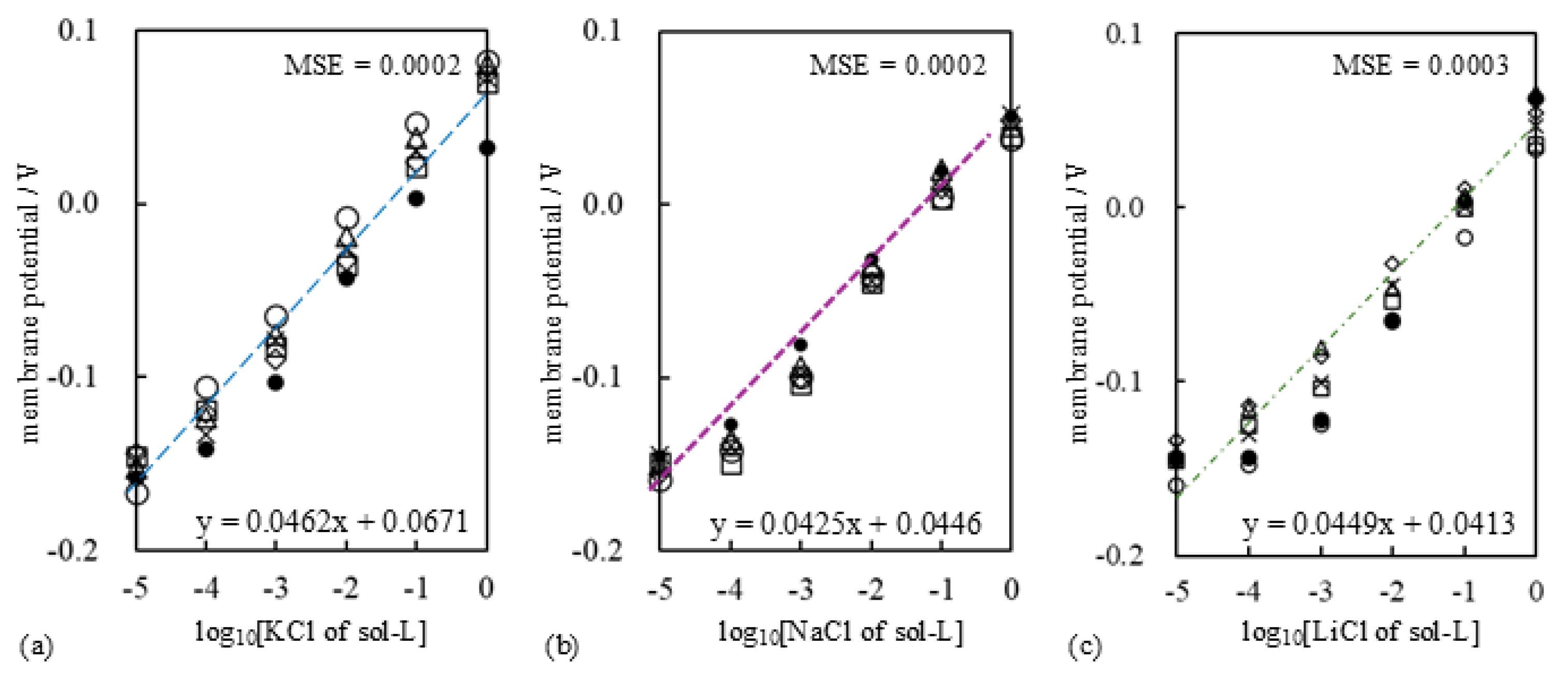
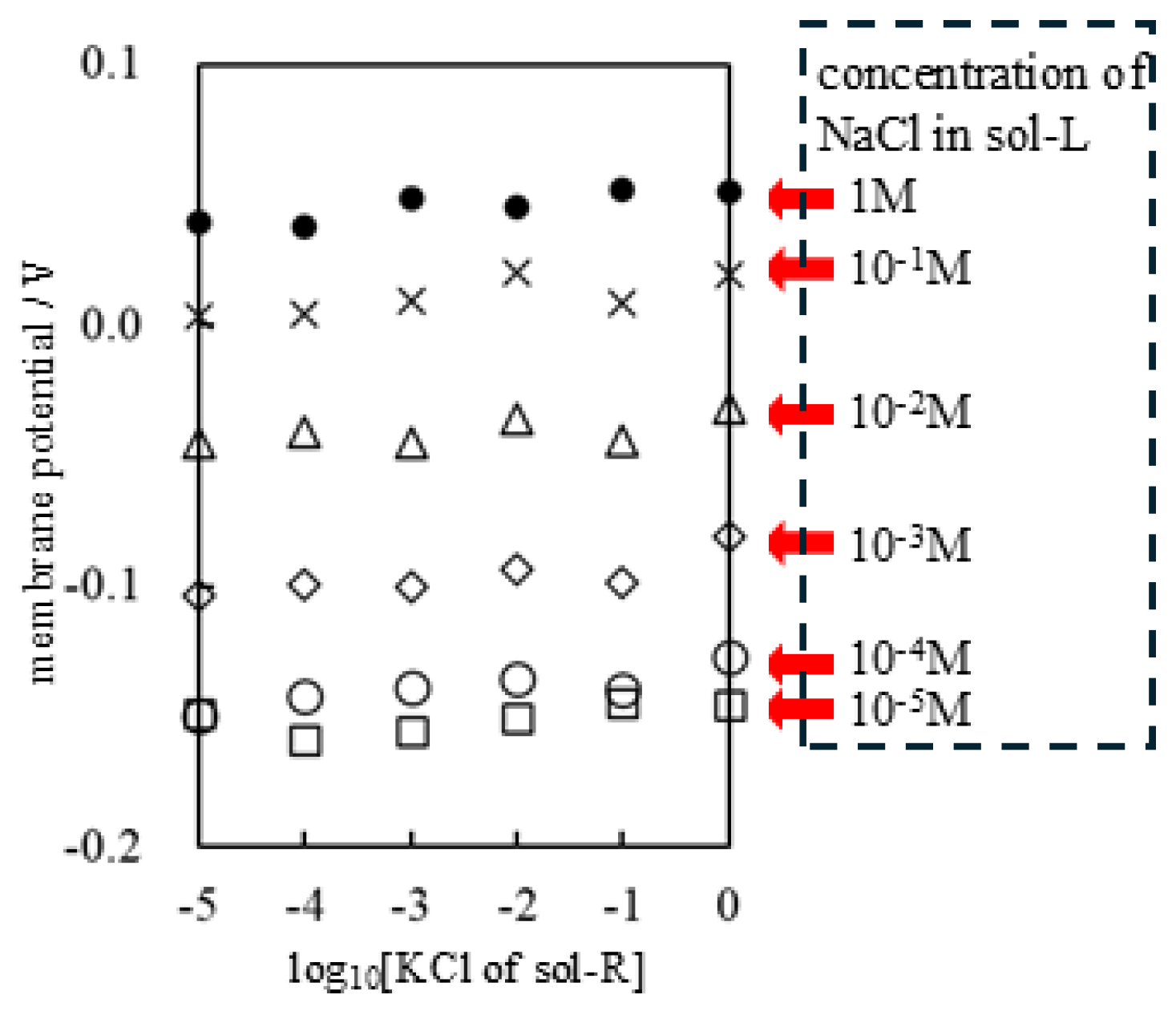
The membrane potential measured experimentally for condition C_KK-5 is depicted in Figure 3a. Similarly, data from condition C_KK-4 are illustrated in Figure 3b. Figure 3c–f display membrane potential data for conditions ranging from C_KK-3 to C_KK0. Interestingly, all these plots can be approximated by a single straight line, as shown in Figure 4. The data portrayed in Figure 4 have been reorganized in Figure 5. It is important to note that although identical symbols are used in both Figure 4 and Figure 5, they do not necessarily indicate the same potential datasets. For instance, the data indicated by □ in Figure 3a do not correspond to the data indicated by □ in Figure 5. Figure 3 implies that the membrane potential is influenced by the sol-L KCl concentration, while Figure 5 implies that the membrane potential is not affected by the sol-R KCl concentration. This potential behavior can be elucidated by AIH, as consistently reported in previous studies, including our works [2,3,4,8,13].

According to the AIH, the membrane potential is the sum of the potentials created in sol-L and sol-R, although these potentials are generated independently. For sol-L, the potential is influenced by the ion charge distribution, which is heavily affected by the mobile cation adsorption on the left surface of the C-memb since this surface is a cation-exchange membrane (Selemion CMVN). Regardless of the experimental conditions C_KK-5 through C_KK0, the conditions in the sol-L phase are consistent. Thus, the potential for sol-L can be described using a single potential function $\psi \left(Q_L\right)$, where $Q_L$ denotes the ion concentration in sol-L. In the sol-R phase, ion adsorption does not occur because the left surface of the C-memb is a PP lacking ion adsorption sites. Therefore, the potential in sol-R remains constant, regardless of ion concentration $Q_R$, and can be defined by a single function $\varphi \left(Q_R\right)$. Consequently, the membrane potential, measured under the conditions described in Table 1, is represented by the single formula $\mathrm{\Phi }(Q_L,Q_R)$ as shown in Equation (3).

$$\begin{array}{c}\hfill \mathrm{\Phi }(Q_L,Q_R)=\psi \left(Q_L\right)-\varphi \left(Q_R\right)\end{array}$$

(3)

The C-memb is an impermeable membrane, rendering the GHK equation inapplicable for analyzing the membrane potential depicted in Figure 3. However, our objective here was to see if the GHK equation could replicate the observed potential data using hypothetical values for $P_i$. Note: $P_i$ does not hold any physiological meaning for this analysis, and it is merely a hypothetical quantity. The GHK equation is expressed by Equation (4). To test this, we attempted to match the experimental membrane potential data illustrated in Figure 3d by plugging the hypothetical values $P_i$ (described in Equations (5) and (6)) into the GHK equation (Equation (4)). 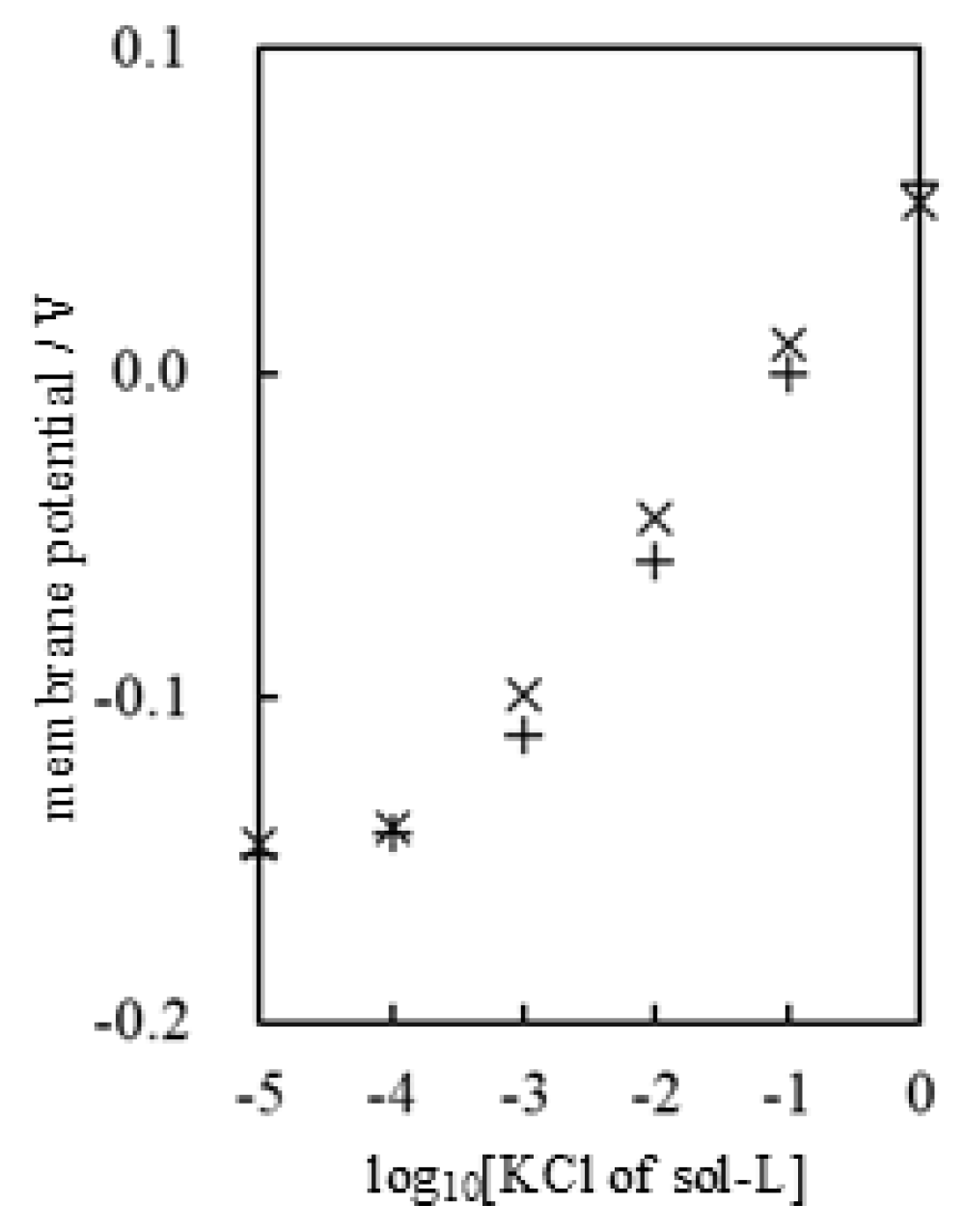Figure 6 indicates that the GHK equation can reproduce the experimental potential data fairly accurately. However, this finding lacks physiological or scientific relevance, as the GHK equation is unsuitable for systems with impermeable membranes. In particular, incorrect numerical values for $P_i$ can still match the experimental membrane potential results when using the GHK equation. Consequently, using the GHK equation with hypothetical $P_i$ values to mirror the experimental membrane potential is an incorrect strategy from a physiological point of view [8,21]. An earlier study by Aono and Ohki [10] indicated that the axon potential they observed could not be replicated by the GHK equation; essentially, they could not even identify the correct $P_i$ values for the equation. They concluded that considering surface charge contributions is necessary for accurately predicting the membrane potential theoretically. This conclusion aligns with the fundamental AIH concept that immobile charges contribute to membrane potential generation.

$$\begin{array}{c}\hfill {\psi }^{GHK}=-{\displaystyle \frac{kT}{e}}ln{\displaystyle \frac{P_K{Q}_L^K+P_{Cl}{Q}_R^{Cl}}{P_K{Q}_R^K+P_{Cl}{Q}_L^{Cl}}}\end{array}$$

(4)

$$\begin{array}{ccc}\hfill P_K& =& 1\hfill \end{array}$$

(5)

$$\begin{array}{ccc}\hfill P_{Cl}& =& 0.003\hfill \end{array}$$

(6)

Subsequently, the identical membrane potential measurement was repeated with the A-memb substituting the C-memb. The experimental parameters are described in

Table 2. sol-L = KCl solution; sol-R = KCl solution; membrane = A-memb.

sol-L/M	${10}^{-5}$	${10}^{-4}$	${10}^{-3}$	${10}^{-2}$	${10}^{-1}$	1
A_KK-5	sol-R = 10−5 M
A_KK-4	sol-R = 10−4 M
A_KK-3	sol-R = 10−3 M
A_KK-2	sol-R = 10−2 M
A_KK-1	sol-R = 10−1 M
A_KK0	sol-R = 100 (=1) M

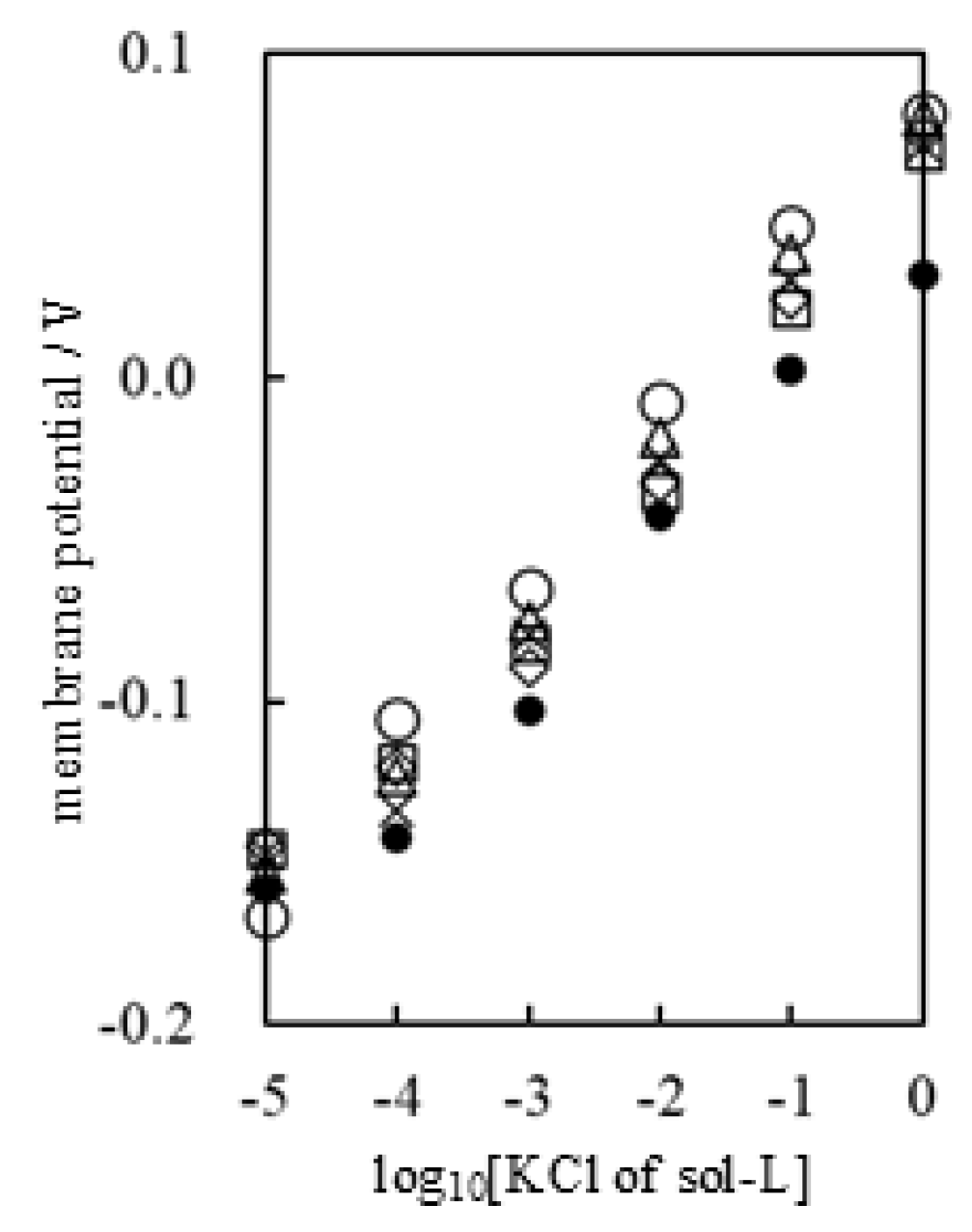
. The outcome is depicted in Figure 7. Exactly like in Figure 4, all potential data align closely with a single line. In particular, the slope of this line is the inverse of the slope observed in Figure 4.

The membrane potential depicted in Figure 7 is restructured as membrane potential versus ${log}_{10}$[KCl in sol-R] as shon in Figure 8. Like the membrane potential across the C-memb, the membrane potential across the A-memb is influenced by the KCl concentration in sol-L and remains unaffected by the KCl concentration in sol-R. The behavior of this potential across the A-memb can be explained similarly with the AIH, just as the potential across the C-memb.

The potential difference across the A-memb under condition A_KK-2 can be replicated using the GHK equation in accordance with the conditions outlined in Equations (7) and (8). However, this potential calculated through the GHK equation lacks scientific and physiological significance because the A-memb is an impermeable barrier. Thus, it is important to reiterate that the reproducibility of potential data using the GHK equation does not imply that the system operates according to the principles of the GHK equation.

$$\begin{array}{ccc}\hfill P_K& =& 0.003\hfill \end{array}$$

(7)

$$\begin{array}{ccc}\hfill P_{Cl}& =& 1\hfill \end{array}$$

(8)

Let us revisit Figure 6. This figure indicates that the GHK equation is capable of replicating the experimental membrane potential across the C-memb with C_KK-2 conditions by using hypothetical values $P_i$, as defined in Equations (5) and (6). Previously, these $P_i$ values were observed to lack physiological or scientific significance due to the membrane’s impermeability. However, the GHK equation effectively reconstructs the experimental membrane potential. As shown in Figure 3, the membrane potential data collected under conditions C_KK-5 through C_KK-3, C_KK-1, and C_KK0 are quantitatively identical to that under C_KK-2. However, determining the exact numerical values for $P_i$’s under all these conditions, except for C_KK-2, which can replicate the observed membrane potentials, proved to be unfeasible. What makes the C_KK-2 condition distinct from the other conditions? This question is also addressed in Figure 9. The illustration indicates that the membrane potential across the A-memb in the A_KK-2 condition can be modeled using the GHK equation using the hypothetical permeability constants $P_i$ provided by the Equations (7) and (8). Despite this, it is not feasible to determine the exact numerical values of the $P_i$ that match the experimental membrane potentials except for the A_KK-2 condition. Therefore, the experimental condition A_KK-2 appears to be particularly significant compared to the other conditions, which include A_KK-5 through A_KK-3, A_KK-1, and A_KK0. Consequently, being able to replicate the experimental membrane potential using the GHK equation with $P_i$’s determined by trial and error is physiologically meaningless. Therefore, we need to consider the possibility that there may be an omission in the GHK equation.

3.1.2. sol-L = NaCl Solution, sol-R = KCl Solution

The same membrane potential measurement as in Section 3.1.1 was performed under the conditions specified in

Table 3. sol-L = NaCl solution; sol-R = KCl solution; membrane = C-memb.

sol-L/M	${10}^{-5}$	${10}^{-4}$	${10}^{-3}$	${10}^{-2}$	${10}^{-1}$	1
C_NaK-5	sol-R = 10−5 M
C_NaK-4	sol-R = 10−4 M
C_NaK-3	sol-R = 10−3 M
C_NaK-2	sol-R = 10−2 M
C_NaK-1	sol-R = 10−1 M
C_NaK0	sol-R = 100 (=1) M

and

Table 4. sol-L = NaCl solution; sol-R = KCl solution; membrane = A-memb.

sol-L/M	${10}^{-5}$	${10}^{-4}$	${10}^{-3}$	${10}^{-2}$	${10}^{-1}$	1
A_NaK-5	sol-R = 10−5 M
A_NaK-4	sol-R = 10−4 M
A_NaK-3	sol-R = 10−3 M
A_NaK-2	sol-R = 10−2 M
A_NaK-1	sol-R = 10−1 M
A_NaK0	sol-R = 100 (=1) M

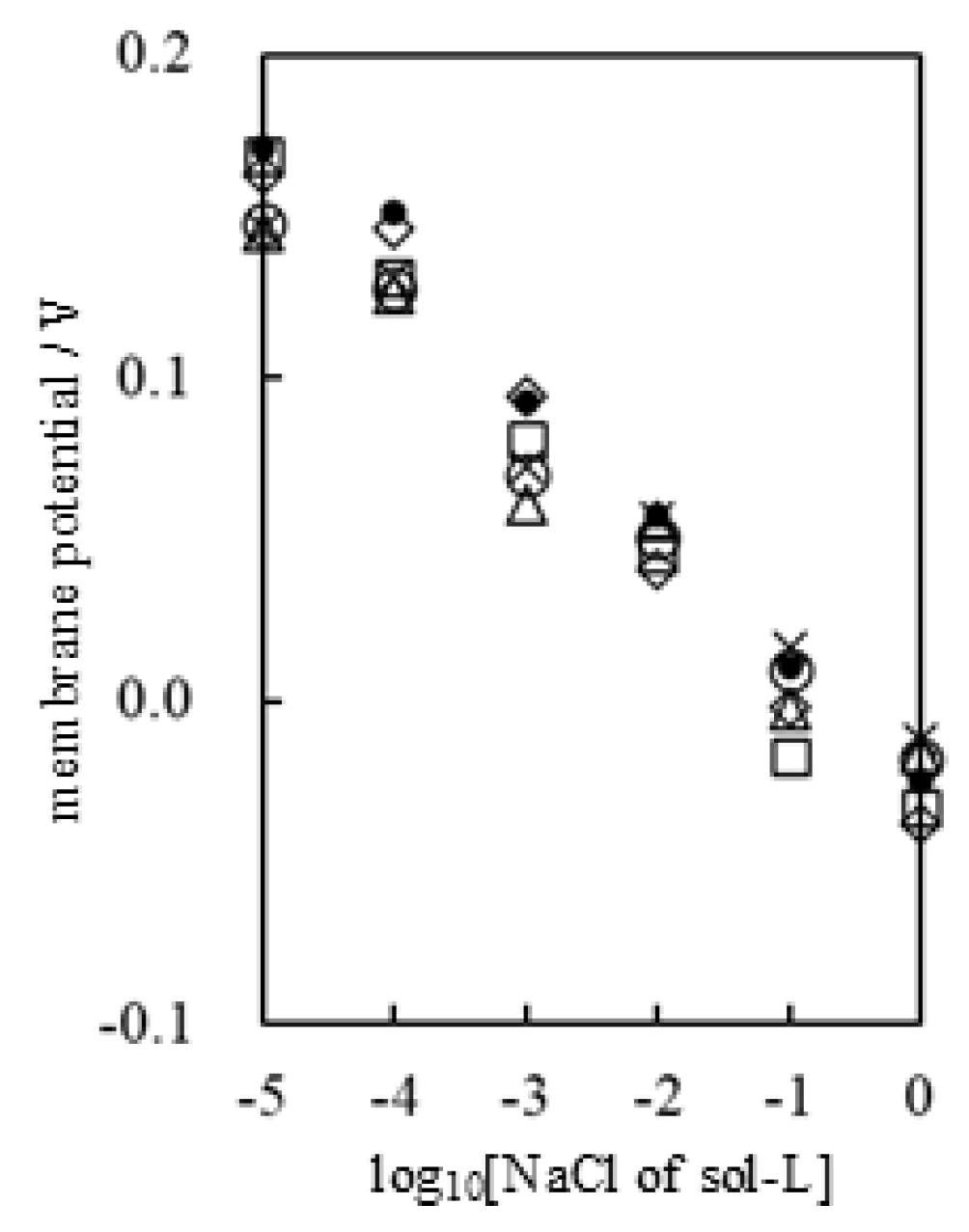
. The difference between the experiments described in the Section 3.1.1 and those in Section 3.1.2 lies in the difference in species of ion in sol-L. NaCl solution is used in the experiments described in this section while KCl solution is used in the previous section. The potential profiles in Figure 10 and Figure 11 are quite similar to those in Figure 4 and Figure 7, respectively. Similarly, the potential profiles in Figure 12 and Figure 13 are similar to those of Figure 5 and Figure 8, respectively. Thus, the membrane potentials depend solely on the ion concentration of sol-L and are unaffected by the ion concentration of sol-R, even when sol-L is a NaCl aqueous solution instead of a KCl aqueous solution.

We now explore if the GHK equation can replicate the membrane potentials observed under the conditions listed in Table 3 and Table 4. Interestingly, the membrane potential for the C_NaK-1 condition was found to be reproducible using the assumed values $P_i$ mentioned in Equations (9)–(11). The findings are depicted in Figure 14.

$$\begin{array}{ccc}\hfill P_{Na}& =& 1.05\hfill \end{array}$$

(9)

$$\begin{array}{ccc}\hfill P_K& =& 1\hfill \end{array}$$

(10)

$$\begin{array}{ccc}\hfill P_{Cl}& =& 0.004\hfill \end{array}$$

(11)

$$\begin{array}{c}\hfill {\psi }^{GHK}=-{\displaystyle \frac{kT}{e}}ln{\displaystyle \frac{P_{Na}{Q}_L^{Na}+P_K{Q}_L^K+P_{Cl}{Q}_R^{Cl}}{P_{Na}{Q}_R^{Na}+P_K{Q}_R^K+P_{Cl}{Q}_L^{Cl}}}\end{array}$$

(12)

Similarly, the membrane potential under condition A_NaK-1 was found to be reproducible using the hypothetical $P_i$ values provided in Equations (13)–(15). The result is shown in Figure 15.

$$\begin{array}{ccc}\hfill P_{Na}& =& 0.003\hfill \end{array}$$

(13)

$$\begin{array}{ccc}\hfill P_K& =& 0.003\hfill \end{array}$$

(14)

$$\begin{array}{ccc}\hfill P_{Cl}& =& 1\hfill \end{array}$$

(15)

However, the GHK equation could not reproduce the membrane potentials obtained under the conditions specified in Table 3 and Table 4, except for C_NaK-1 and A_NaK-1. These results suggest that the GHK equation may lack a critical factor necessary to fully explain the observed membrane potentials.

3.2. Hofmeister Effect and AIH

The experimental outcomes presented thus far indicate that the membrane potential is controlled by the ion concentration in sol-L, which interfaces with the ion exchange membrane, whether it is C-memb or A-memb. This suggests that ion adsorption on the surface of the left membrane is the primary influence in generating the membrane potential. These results support the AIH prediction as detailed: Under the circumstances described in Table 1, the $K^{+}$ ions adsorb onto the left side of C-memb due to its role as a cation exchange membrane. This adsorption on the left side of the C-memb establishes the heterogeneous spatial ion charge distribution shown in Figure 16, resulting in a nonzero potential in sol-L.

In contrast, no such heterogeneous charge distribution occurs in sol-R, resulting in a constant potential profile in sol-R, regardless of the ion concentration in sol-R. Therefore, the membrane potentials under the conditions shown in Table 1 depend solely on the $K^{+}$ concentration in sol-L.

Upon measuring the membrane potentials under the conditions outlined in Table 2, $C{l}^{-}$ ions were also found to adhere to the left side of the A-memb. Thus, the membrane potentials in these scenarios are determined solely by the concentration of $C{l}^{-}$ in sol-L. This indicates that the presence of either $K^{+}$ or $N{a}^{+}$ ions as counterions for $C{l}^{-}$ in sol-L makes no difference, as these cations do not attach to the left surface of the A-memb. The $C{l}^{-}$ ions, being the mobile anions, remain consistent regardless of whether sol-L comprises KCl or NaCl.

Therefore, according to the AIH, the membrane potentials outlined in Table 4 should match those specified in Table 2. In Figure 17a, the potential profiles from Figure 7 are displayed along with a regression line defined by Equation (16), which shows an MSE (Mean Squared Error) of 0.0002 [22]. Likewise, Figure 17b includes the potential profiles from Figure 11, with a dashed line representing Equation (16). Even though Equation (16) is not the regression line for Figure 17b, the MSE for the potential data in Figure 17b with respect to Equation (16) is 0.0004. This result suggests that Equation (16) can effectively serve as the regression line for both diagrams, Figure 17a,b. Consequently, the potential profiles in these two diagrams are fundamentally identical, as predicted by the AIH.

$$\begin{array}{c}\hfill y=0.0462x+0.0671\end{array}$$

(16)

A similar analysis was performed on the membrane potentials measured under the conditions shown in Table 1 and Table 3. Figure 18 shows the corresponding potential profiles.

In Figure 18a, the potential profiles seen in Figure 4 are shown alongside the regression line described by Equation (17). Similarly, Figure 18b illustrates the potential profiles from Figure 10 with the same regression line expressed by Equation (17). The MSE for the potential data in Figure 18a is 0.0001. The dashed line in Figure 18b corresponds to Equation (17), but it should be noted that Equation (17) does not represent the regression line for Figure 18b; rather, it corresponds to the regression line for Figure 18a. The MSE of the potential data in Figure 18b relative to Equation (17) is 0.0011, which is notably different from the MSE of 0.0001 in Figure 18a. This suggests that the potential characteristics in Figure 18a differ from those in Figure 18b. What accounts for this distinct difference? According to the AIH, the binding characteristics between $K^{+}$ and the left surface of C-memb must differ from those between $N{a}^{+}$ and the left surface of C-memb, since the left surface of C-memb is a cation exchange membrane (Selemion CMVN).

$$\begin{array}{c}\hfill y=-0.0472x-0.0775\end{array}$$

(17)

It is natural to question whether such a difference in binding characteristics exists. The Hofmeister effect may offer an explanation for this phenomenon [14,15,16,17,18,19]. The Hofmeister effect refers to the ability of individual ions to induce protein precipitation (or salting-out of proteins). This phenomenon describes how specific ions influence protein behavior and the effectiveness of typical cations, such as K⁺, Na⁺, and Li⁺, in salting out proteins is often ranked according to Equation (18). (Note: it should be noted that this order is not always so clear-cut [18,19]). Therefore, even though the basic characteristics of $K^{+}$, such as the atomic radius and its valency, are quite similar to those of $N{a}^{+}$, there is a certain difference in characteristics between $K^{+}$ and $N{a}^{+}$.

$$\begin{array}{c}\hfill ...>K^{+}>N{a}^{+}>L{i}^{+}>...\end{array}$$

(18)

Nostro and Ninham strongly assert that the Hofmeister effect is indeed real and that numerous scientific studies have explored it due to its long history. Although both $K^{+}$ and $N{a}^{+}$ share the valency of +1, they are not identical ions and produce different impacts on biological systems. The same differentiation applies to, for example, Ca²⁺ and Zn²⁺. Despite this, the Hofmeister effect is often overlooked in biological contexts [14]. Ling, the originator of AIH, and Pollack, the developer of the AIH, also discuss the essential role of the Hofmeister effect in biological systems [2,23]. The existence of the Hofmeister effect provides evidence supporting the significant role of ion adsorption-desorption in generating membrane potential.

3.3. Membrane Potential in Hofmeister Effect Perspective

In light of the previous discussion, the key factor is the binding between mobile ions and the left surface of the membrane (specifically, the ion-exchange membranes of C-memb and A-memb). This implies that mobile ions that do not bind to the left surface do not influence the membrane potential. Based on this, we conducted membrane potential measurements under the conditions specified in

Table 5. sol-L = SDS solution; sol-R = KCl solution; membrane = C-memb.

sol-L/M	${10}^{-5}$	${10}^{-4}$	${10}^{-3}$	${10}^{-2}$	${10}^{-1}$	1
C_SK-5	sol-R = 10−5 M
C_SK-4	sol-R = 10−4 M
C_SK-3	sol-R = 10−3 M
C_SK-2	sol-R = 10−2 M
C_SK-1	sol-R = 10−1 M
C_SK0	sol-R = 100 (=1) M

and

Table 6. sol-L = KCl solution; sol-R = SDS solution; membrane = C-memb.

sol-L/M	${10}^{-5}$	${10}^{-4}$	${10}^{-3}$	${10}^{-2}$	${10}^{-1}$	1
C_KS-5	sol-R = 10−5 M
C_KS-4	sol-R = 10−4 M
C_KS-3	sol-R = 10−3 M
C_KS-2	sol-R = 10−2 M
C_KS-1	sol-R = 10−1 M
C_KS0 †	sol-R = 100 (=1) M

^† It is impossible to prepare 1 M SDS due to its high concentration.

. We then compared these measured potentials with the membrane potentials previously discussed in Table 4.

Figure 19 shows the membrane potential profiles obtained under the conditions summarized as follows. Figure 19a: Table 3 (the potential profiles are the same as those in Figure 10 and Figure 18b), Figure 19b: Table 5, Figure 19c: Table 6. The dotted lines in Figure 19a–c represent the regression line given by Equation (19) for the diagram in Figure 19a (but not for (b) or (c)). We will discuss these in relation to Figure 18, as follows.

$$\begin{array}{c}\hfill y=-0.0380x-0.0280\end{array}$$

(19)

Let us revisit Figure 18a,b. The regression line in Equation (17), which corresponds to the diagram in Figure 18a, cannot serve as the appropriate regression line for the diagram in Figure 18b. As we hypothesize, this discrepancy is due to the difference in the degree of adsorption between K⁺ and Na⁺ on the left surface of C-memb, as shown in Figure 20. This difference is likely a manifestation of the Hofmeister effect. Consequently, this result is consistent with the AIH, which suggests that ion adsorption is the predominant factor in generating the membrane potential.

Then, let us revisit Figure 19a,b. The regression line in Equation (19), which applies to the diagram in Figure 19a, also serves as the appropriate regression line for the diagram in Figure 19b. In Figure 19a, sol-L is SDS, while in Figure 19b, it is NaCl. Although the characteristics of SDS, such as its dimensions and mass, differ significantly from those of NaCl, Equation (19) still fits well as the regression line for both diagrams. The MSE for Figure 19b is 0.0002, which is the same as the MSE for Figure 19a, even though Equation (19) is not the regression line for Figure 19b. This suggests that the degree of adsorption of Na⁺ in NaCl and Na⁺ in SDS on the left surface of C-memb is similar, as illustrated in Figure 21. This result is consistent with the Hofmeister effect and the AIH [2,14,15,16,17,18,19,23].

Figure 19c shows the membrane potential when sol-L and sol-R from Figure 19b are swapped. In this case, the ion adsorbed on the left surface of the C-memb is K⁺ instead of Na⁺. As a result, Equation (19) cannot serve as the appropriate regression line for the diagram in Figure 19c (MSE = 0.0023), as predicted by the AIH and the Hofmeister effect. Based on the previous discussion, the regression line in Equation (17) for the diagram in Figure 18a is related to the adsorption of K⁺ on the left surface of the C-memb (see Figure 22a). The diagram in Figure 19c also concerns the adsorption of K⁺ on the left surface of the C-memb, as shown in Figure 22b. Therefore, Equation (17) is expected to serve as a good regression line for the diagram in Figure 19c, despite the fact that the R-sol in Figure 22b corresponding to Figure 19c is different from that in Figure 22a corresponding to Figure 18a. When Equation (17) is assumed as the regression line for the diagram in Figure 19c, the MSE is calculated to be 0.0005. This demonstrates that Equation (17) can still serve as a good regression line for the diagram in Figure 19c. This result provides strong supporting evidence for validating the AIH from the perspective of the Hofmeister effect.

3.4. Prediction of Membrane Potential Characeritics by Hofmeiter Effect and AIH

We have posited that the Hofmeister effect [14,15,16,17,18,19] aligns with the membrane potential generation mechanism based on the AIH [2,3,4]. From the perspective of the Hofmeister effect, we predict that the membrane potential profile (membrane potential vs. ${log}_{10}$[LiCl in sol-L]) obtained under the conditions summarized in

Table 7. sol-L = LiCl solution; sol-R = KCl solution; membrane = C-memb.

sol-L/M	${10}^{-5}$	${10}^{-4}$	${10}^{-3}$	${10}^{-2}$	${10}^{-1}$	1
C_LK-5	sol-R = 10−5 M
C_LK-4	sol-R = 10−4 M
C_LK-3	sol-R = 10−3 M
C_LK-2	sol-R = 10−2 M
C_LK-1	sol-R = 10−1 M
C_LK0	sol-R = 100 (=1) M

will differ somewhat from the profiles shown in Figure 4 (which were obtained under the conditions in Table 1) and Figure 10 (which were obtained under the conditions in Table 3). This difference arises because the adsorbed cations on the left surface of C-memb differ (K⁺, Na⁺, or Li⁺) across these diagrams. Specifically, the degree and amount of cation adsorption on the membrane must differ, as depicted in Figure 23, and this difference must be reflected in the variations in membrane potential characteristics. The experimentally measured membrane potential under the condition in Table 7 is shown in Figure 24c, along with the other profiles obtained under the conditions in Table 1 and Table 3.

The membrane potential profiles obtained under the three different conditions (Table 1, Table 3 and Table 7) are not entirely distinct from one another. The formulas for the regression lines in the diagrams of Figure 24a–c are provided within these diagrams and are also presented in Equations (20)–(22), respectively. Contrary to our expectations based on the Hofmeister effect, the potential profiles in Figure 24b,c are virtually identical, as clearly demonstrated by the similarity between the regression line expressions in Equations (21) and (22), while Equation (20) is significantly different from the others.

$$\begin{array}{ccc}\hfill y& =& -0.0472x-0.0775\hfill \end{array}$$

(20)

$$\begin{array}{ccc}\hfill y& =& -0.0380x-0.0280\hfill \end{array}$$

(21)

$$\begin{array}{ccc}\hfill y& =& -0.0390x-0.0165\hfill \end{array}$$

(22)

Next, we need to examine the diagrams of the membrane potential generated across the A-memb under the conditions specified in Table 2, Table 4 and

Table 8. sol-L = LiCl solution; sol-R = KCl solution; membrane = A-memb.

sol-L/M	${10}^{-5}$	${10}^{-4}$	${10}^{-3}$	${10}^{-2}$	${10}^{-1}$	1
A_LK-5	sol-R = 10−5 M
A_LK-4	sol-R = 10−4 M
A_LK-3	sol-R = 10−3 M
A_LK-2	sol-R = 10−2 M
A_LK-1	sol-R = 10−1 M
A_LK0	sol-R = 100 (=1) M

. The membrane potentials under these conditions should be identical, as long as the Hofmeister effect and the AIH are the predominant factors governing the membrane potential characteristics. This is because the anion adsorbed on the left surface of the A-memb is Cl⁻ in all cases, as illustrated in Figure 25.

Figure 7 and Figure 11 present the membrane potentials obtained under the conditions outlined in Table 4 and Table 8, respectively. These two membrane potential diagrams are shown in Figure 26a,b, respectively, along with their regression lines and formulas (see also Equations (23) and (24)). Figure 26c displays the membrane potential obtained under the condition specified in Table 8, along with its regression line formula (see also Equation (25)). The regression lines for Figure 26a–c fit the data well, as the MSE values indicated in the individual diagrams are only 0.0002 or 0.0003.

$$\begin{array}{ccc}\hfill y& =& 0.0462x+0.0671\hfill \end{array}$$

(23)

$$\begin{array}{ccc}\hfill y& =& 0.0425x+0.0446\hfill \end{array}$$

(24)

$$\begin{array}{ccc}\hfill y& =& 0.0449x+0.0413\hfill \end{array}$$

(25)

These three regression lines, given by Equation (23) through Equation (25), are quite similar to one another, which aligns with the Hofmeister effect and the AIH. We computed the MSE values for Figure 26b,c, assuming that their regression lines follow Equation (23). We found that the MSE values for Figure 26b,c were 0.0004 and 0.0006, respectively. These values are not significantly different from the MSE values obtained using their own regression lines, Equations (24) and (25), which were 0.0002 and 0.0003, respectively. Therefore, these three diagrams, Figure 26a–c, quantitatively represent the same potential characteristics, as expected from the Hofmeister effect and AIH [2,3,4,14,15,16,17,18,19].

4. Transition from the Membrane Theory to AIH and Hofmeister Effect

In this section, we would like to present our view on the significance of adopting AIH and the Hofmeister effect as causes of membrane potential generation. All three theories—membrane theory, AIH, and the Hofmeister effect—were proposed a long time ago [1,2,3,4,5,6,14,15,16,17,18,19]. The recognition of the Hofmeister effect remains obscure among scientists, whereas membrane theory has survived and become the central physiological concept in modern times.

On the other hand, AIH completely faded from the field of physiology many years ago. Nonetheless, AIH is still scientifically attractive since it is fundamentally rooted in one of the core scientific disciplines, thermodynamics. At the same time, the Hofmeister effect is a thermodynamic concept that describes the effectiveness of ions in salting out proteins [18]. In contrast, membrane theory has developed independently over time. That is, membrane theory has progressed separately from thermodynamics. As a result, current physiological concepts are not well supported by thermodynamics or physics, which could lead to the misdirection of physiology as some researchers such as Schneider, Bagatolli et al., and Jaeken warn [24,25,26]. As a matter of fact, Ling devoted his entire scientific career to integrating physiology and thermodynamics, which had long been considered separate fields [1,2,3,4]. In a sense, he achieved this goal by proposing AIH. However, AIH has not gained much attention from physiologists, and it has even been regarded as a sort of pseudoscience [27].

The significant difference between membrane theory and AIH regarding the mechanism of membrane potential generation is described here again as follows: membrane theory attributes membrane potential generation to transmembrane ion transport, while AIH attributes it to ion adsorption. This difference may sound trivial. However, AIH is deeply rooted in thermodynamics. To be precise, AIH is supported by thermodynamics for real systems rather than ideal systems. All phenomena, including biological systems, are governed by the laws of thermodynamics, but biological systems are far from the thermodynamically ideal state. They exist in the thermodynamically real state. Therefore, adopting AIH leads to a shift in our perspective on biological systems. Such a transition will change how we view biological systems. For example, Ling denies the occurrence of channel- and pump-assisted ion transport across the plasma membrane, which is believed to be the primary cause of membrane potential generation in purview of membrane theory. Then, he proposed an alternative theory called the Association-Induction Hypothesis (AIH), which relies on ion adsorption as the cause of membrane potential generation. Ling never denied the occurrence of transmembrane ion movement, the existence of proteins called channels, the existence of substances called pumps, the generation of membrane potential, or other biological phenomena that have been experimentally confirmed. The authors of this paper believe that what Ling had emphasized throughout his scientific career can be summarized as follows: modern physiological interpretations of biological phenomena are far from thermodynamic principles and should be reinterpreted using thermodynamics for real systems in the correct manner. This view is shared by some other scientists like Pollack, Bagatolli, and his colleagues, Schneider, Jaeken, and Edelmann [23,24,25,26,27].

So, many contemporary researchers still continue to use membrane theory in their studies. Some of these studies are not solely for basic scientific purposes but also for practical applications. For example, Lucia et al. investigated the cause of inflammation from the perspective of cell potential characteristics [28]. This is a unique study, as their research demonstrates how a cell’s deviation from normal conditions is reflected in its membrane potential characteristics. They then attempted to identify therapeutic methods to restore the abnormal cell condition. Despite the usefulness of membrane potential, we believe that its generation mechanism has not been correctly interpreted. However, even some researchers who support membrane theory seem to inadvertently acknowledge the correlation between membrane potential and ion adsorption. The work of Benarroch and Asally is particularly interesting [29]. While they do not reject membrane theory, their findings undoubtedly suggest that ion adsorption plays a role in membrane potential generation. Although they do not explicitly discuss the mechanism of membrane potential, their work implicitly acknowledges the existence of a factor beyond transmembrane ion transport. They never use the term “ion adsorption”, yet their discussion clearly considers its influence on membrane potential generation. Sun and Son’s study on membrane potential generation is also quite fascinating [30]. Their work provides a theoretical framework for determining membrane potential, rigorously based on mathematics and physics. Although they do not consider ion adsorption as AIH does, they account for the localized charge effect in membrane potential generation. This approach is one of the foundational principles of AIH, which asserts that spatially localized charge due to ion adsorption contributes to membrane potential generation. Unfortunately, however, they rely on the well-known GHK equation without deriving it from their own theoretical framework. Although neither Benarroch and Asally nor Sun and Son explicitly discuss the relationship between membrane potential generation and ion adsorption, it is evident that some researchers who have utilized membrane theory have inadvertently incorporated the concept of ion adsorption—the fundamental principle of AIH—into their work.

So far, the authors have discussed that ion adsorption-desorption is essential for membrane potential generation. However, from the perspective of researchers who support the conventional physiological view, it is true that the actual plasma membrane is permeable not only to ions but also to some small molecules [3]. Hence, it may be inappropriate to abandon the whole body of accomplishments in conventional physiology. Instead, a reform or shift from the full membrane theory to AIH and the Hofmeister effect, integrated with the fundamental achievements made by numerous physiologists, is necessary in order to gain a more accurate understanding of real electrophysiological systems. Ultimately, this transition could even lead to a more effective utilization of membrane potential, beyond the scope of basic physiological science, for promoting human health, such as the accomplishment by Lucia et al. [28].

5. Conclusions

It is widely recognized that membrane potential arises from life processes such as the function of the Na⁺/K⁺ pump. The necessity of ion pumping underscores the importance of transmembrane ion transport for sustaining life, leading to the generation of membrane potential.

The reproducibility of experimental membrane potential data using the GHK equation is often regarded as evidence that transmembrane ion transport is responsible for membrane potential generation. However, our findings suggest that membrane potential analysis based on the GHK equation is not as reliable as we have believed and its physiological significance is questionable in some cases.

Our research indicates that transmembrane potential can form even across impermeable membranes. Certain thermodynamic principles, such as ion adsorption-desorption and the Hofmeister effect, offer explanations for potential generation across these impermeable membranes. Although these concepts have primarily been studied in colloidal and solution chemistry rather than physiology [2,14,15,16,31], they are also applicable to biological systems. These ideas align well with the largely overlooked physiological theory known as the Association-Induction Hypothesis (AIH). Complete denial of conventional physiology may not be the right approach for the progress of physiology, but this work suggests that we should not ignore AIH and the Hofmeister effect for the elucidation of the genuine mechanism of the electrical characteristics of living cells.