The Forces behind Directed Cell Migration
Abstract
1. Introduction
2. The Molecular Clutch Framework
3. Forces during Gradient Sensing
4. Forces during the Integration of Gradient Sensing Information
5. Establishing Polarity
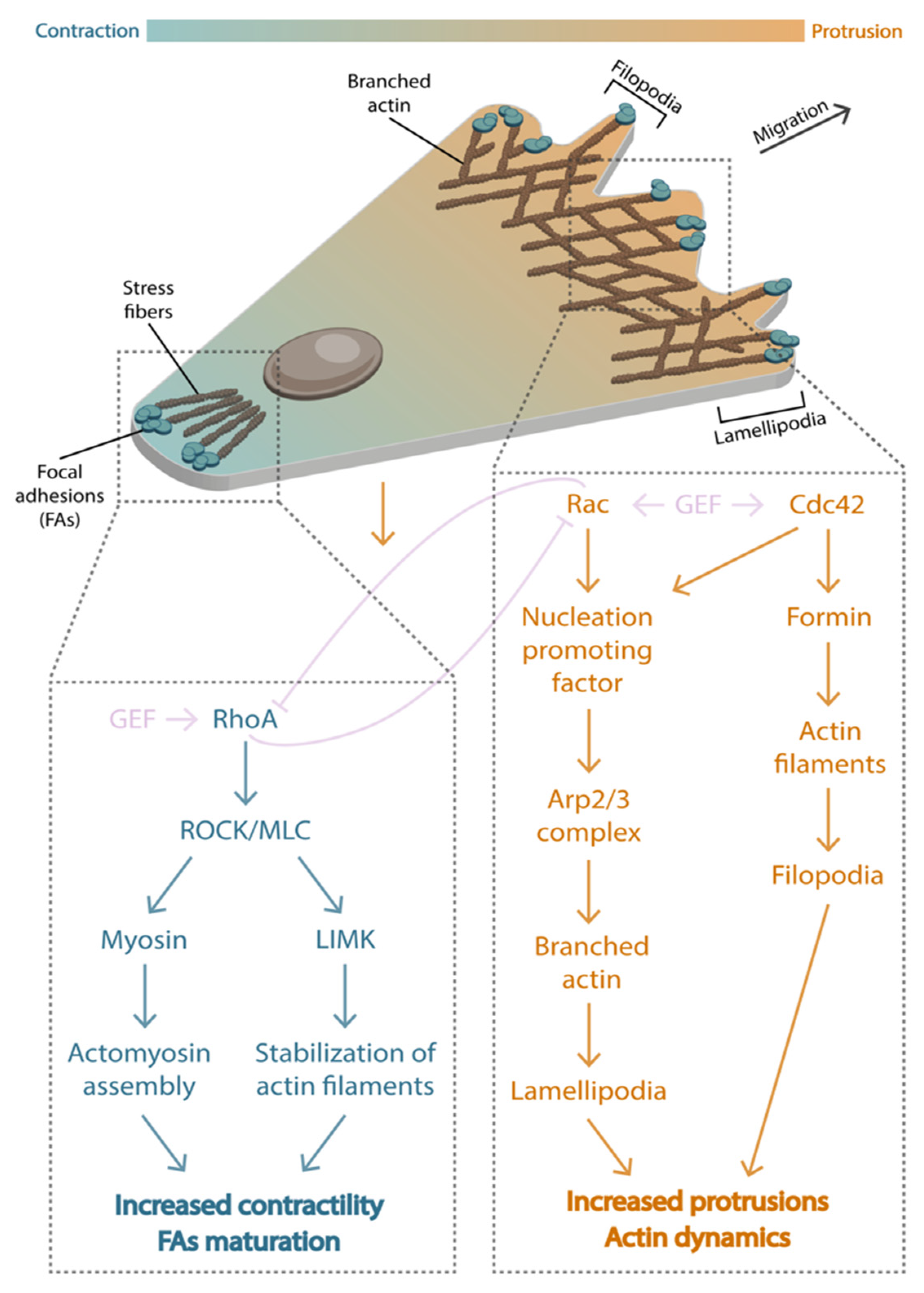
6. Forces during Migration
7. Forces beyond the Clutch Model
8. Concluding Remarks
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Reid, C.R.; Lutz, M.J.; Powell, S.; Kao, A.B.; Couzin, I.D.; Garnier, S. Army ants dynamically adjust living bridges in response to a cost–benefit trade-off. Proc. Natl. Acad. Sci. USA 2015, 112, 15113–15118. [Google Scholar] [CrossRef]
- Ginelli, F.; Peruani, F.; Pillot, M.-H.; Chaté, H.; Theraulaz, G.; Bon, R. Intermittent collective dynamics emerge from conflicting imperatives in sheep herds. Proc. Natl. Acad. Sci. USA 2015, 112, 12729–12734. [Google Scholar] [CrossRef]
- Zitterbart, D.P.; Wienecke, B.; Butler, J.P.; Fabry, B. Coordinated Movements Prevent Jamming in an Emperor Penguin Huddle. PLoS ONE 2011, 6, e20260. [Google Scholar] [CrossRef]
- Ayala, R.; Shu, T.; Tsai, L.-H. Trekking across the Brain: The Journey of Neuronal Migration. Cell 2007, 128, 29–43. [Google Scholar] [CrossRef]
- Kriegstein, A.R.; Noctor, S.C. Patterns of neuronal migration in the embryonic cortex. Trends Neurosci. 2004, 27, 392–399. [Google Scholar] [CrossRef]
- Weber, M.; Hauschild, R.; Schwarz, J.; Moussion, C.; de Vries, I.; Legler, D.F.; Luther, S.A.; Bollenbach, T.; Sixt, M. Interstitial Dendritic Cell Guidance by Haptotactic Chemokine Gradients. Science 2013, 339, 328–332. [Google Scholar] [CrossRef]
- Eming, S.A.; Martin, P.; Tomic-Canic, M. Wound repair and regeneration: Mechanisms, signaling, and translation. Sci. Transl. Med. 2014, 6, 265sr6. [Google Scholar] [CrossRef]
- Watt, K.E.N.; Trainor, P.A. Chapter 17—Neurocristopathies: The Etiology and Pathogenesis of Disorders Arising from Defects in Neural Crest Cell Development. In Neural Crest Cells; Trainor, P.A., Ed.; Academic Press: Boston, MA, USA, 2014; pp. 361–394. ISBN 978-0-12-401730-6. [Google Scholar]
- Roussos, E.T.; Condeelis, J.S.; Patsialou, A. Chemotaxis in cancer. Nat. Rev. Cancer 2011, 11, 573–587. [Google Scholar] [CrossRef]
- Bravo-Cordero, J.J.; Hodgson, L.; Condeelis, J. Directed Cell Invasion and Migration during Metastasis. Curr. Opin. Cell Biol. 2012, 24, 277–283. [Google Scholar] [CrossRef]
- Petrie, R.J.; Doyle, A.D.; Yamada, K.M. Random versus directionally persistent cell migration. Nat. Publ. Group 2009, 10, 538–549. [Google Scholar] [CrossRef]
- Friedl, P.; Gilmour, D. Collective cell migration in morphogenesis, regeneration and cancer. Nat. Rev. Mol. Cell Biol. 2009, 10, 445–457. [Google Scholar] [CrossRef] [PubMed]
- Haeger, A.; Wolf, K.; Zegers, M.M.; Friedl, P. Collective cell migration: Guidance principles and hierarchies. Trends Cell Biol. 2015, 25, 556–566. [Google Scholar] [CrossRef] [PubMed]
- Shellard, A.; Mayor, R. All Roads Lead to Directional Cell Migration. Trends Cell Biol. 2020, 30, 852–868. [Google Scholar] [CrossRef] [PubMed]
- SenGupta, S.; Parent, C.A.; Bear, J.E. The principles of directed cell migration. Nat. Rev. Mol. Cell Biol. 2021, 22, 529–547. [Google Scholar] [CrossRef] [PubMed]
- Espina, J.A.; Marchant, C.L.; Barriga, E.H. Durotaxis: The mechanical control of directed cell migration. FEBS J. 2022, 289, 2736–2754. [Google Scholar] [CrossRef]
- Abercrombie, M. The Croonian Lecture, 1978—The crawling movement of metazoan cells. Proc. R. Soc. Lond. B Biol. Sci. 1980, 207, 129–147. [Google Scholar] [CrossRef]
- Mitchison, T.; Kirschner, M. Cytoskeletal dynamics and nerve growth. Neuron 1988, 1, 761–772. [Google Scholar] [CrossRef]
- Chan, C.E.; Odde, D.J. Traction Dynamics of Filopodia on Compliant Substrates. Science 2008, 322, 1687–1691. [Google Scholar] [CrossRef]
- Elosegui-Artola, A.; Bazellières, E.; Allen, M.D.; Andreu, I.; Oria, R.; Sunyer, R.; Gomm, J.J.; Marshall, J.F.; Jones, J.L.; Trepat, X.; et al. Rigidity sensing and adaptation through regulation of integrin types. Nat. Mater. 2014, 13, 631–637. [Google Scholar] [CrossRef]
- Elosegui-Artola, A.; Oria, R.; Chen, Y.; Kosmalska, A.; Pérez-González, C.; Castro, N.; Zhu, C.; Trepat, X.; Roca-Cusachs, P. Mechanical regulation of a molecular clutch defines force transmission and transduction in response to matrix rigidity. Nat. Cell Biol. 2016, 18, 540–548. [Google Scholar] [CrossRef]
- Sunyer, R.; Conte, V.; Escribano, J.; Elosegui-Artola, A.; Labernadie, A.; Valon, L.; Navajas, D.; García-Aznar, J.M.; Muñoz, J.J.; Roca-Cusachs, P.; et al. Collective cell durotaxis emerges from long-range intercellular force transmission. Science 2016, 353, 1157–1161. [Google Scholar] [CrossRef]
- Bangasser, B.L.; Shamsan, G.A.; Chan, C.E.; Opoku, K.N.; Tüzel, E.; Schlichtmann, B.W.; Kasim, J.A.; Fuller, B.J.; McCullough, B.R.; Rosenfeld, S.S.; et al. Shifting the optimal stiffness for cell migration. Nat. Commun. 2017, 8, 15313. [Google Scholar] [CrossRef]
- Elosegui-Artola, A.; Trepat, X.; Roca-Cusachs, P. Control of Mechanotransduction by Molecular Clutch Dynamics. Trends Cell Biol. 2018, 28, 356–367. [Google Scholar] [CrossRef]
- Bangasser, B.L.; Rosenfeld, S.S.; Odde, D.J. Determinants of Maximal Force Transmission in a Motor-Clutch Model of Cell Traction in a Compliant Microenvironment. Biophys. J. 2013, 105, 581–592. [Google Scholar] [CrossRef]
- Kechagia, J.Z.; Ivaska, J.; Roca-Cusachs, P. Integrins as biomechanical sensors of the microenvironment. Nat. Rev. Mol. Cell Biol. 2019, 20, 457–473. [Google Scholar] [CrossRef]
- Bagorda, A.; Parent, C.A. Eukaryotic chemotaxis at a glance. J. Cell Sci. 2008, 121, 2621–2624. [Google Scholar] [CrossRef]
- DesMarais, V.; Yamaguchi, H.; Oser, M.; Soon, L.; Mouneimne, G.; Sarmiento, C.; Eddy, R.; Condeelis, J. N-WASP and cortactin are involved in invadopodium-dependent chemotaxis to EGF in breast tumor cells. Cell Motil. Cytoskelet. 2009, 66, 303–316. [Google Scholar] [CrossRef]
- Lo, C.-M.; Wang, H.-B.; Dembo, M.; Wang, Y. Cell Movement Is Guided by the Rigidity of the Substrate. Biophys. J. 2000, 79, 144–152. [Google Scholar] [CrossRef] [PubMed]
- Raab, M.; Swift, J.; Dingal, P.C.D.P.; Shah, P.; Shin, J.W.; Discher, D.E. Crawling from soft to stiff matrix polarizes the cytoskeleton and phosphoregulates myosin-II heavy chain. J. Cell Biol. 2012, 199, 669–683. [Google Scholar] [CrossRef]
- Wang, H.B.; Dembo, M.; Hanks, S.K.; Wang, Y. Focal adhesion kinase is involved in mechanosensing during fibroblast migration. Proc. Natl. Acad. Sci. USA 2001, 98, 11295. [Google Scholar] [CrossRef]
- Vincent, L.G.; Choi, Y.S.; Alonso-Latorre, B.; del Álamo, J.C.; Engler, A.J. Mesenchymal stem cell durotaxis depends on substrate stiffness gradient strength. Biotechnol. J. 2013, 8, 472–484. [Google Scholar] [CrossRef] [PubMed]
- DuChez, B. The Response of Cancer Cells to Local Changes in Extracellular Stiffness—ProQuest; Georgetown University: Washington, DC, USA, 2017. [Google Scholar]
- Rong, Y.; Yang, W.; Hao, H.; Wang, W.; Lin, S.; Shi, P.; Huang, Y.; Li, B.; Sun, Y.; Liu, Z.; et al. The Golgi microtubules regulate single cell durotaxis. EMBO Rep. 2021, 22, e51094. [Google Scholar] [CrossRef] [PubMed]
- Hakeem, R.M.; Subramanian, B.C.; Hockenberry, M.A.; King, Z.T.; Butler, M.T.; Legant, W.R.; Bear, J.E. Zyxin and non-muscle myosin are required for single fibroblast durotaxis, but Rho-kinase activity and the Arp2/3 complex are dispensable. bioRxiv 2022, 2022.06.03.494725. [Google Scholar] [CrossRef]
- Yip, A.K.; Zhang, S.; Chong, L.H.; Cheruba, E.; Woon, J.Y.X.; Chua, T.X.; Goh, C.J.H.; Yang, H.; Tay, C.Y.; Koh, C.-G.; et al. Zyxin Is Involved in Fibroblast Rigidity Sensing and Durotaxis. Front. Cell Dev. Biol. 2021, 9, 735298. [Google Scholar] [CrossRef]
- Isomursu, A.; Park, K.-Y.; Hou, J.; Cheng, B.; Mathieu, M.; Shamsan, G.A.; Fuller, B.; Kasim, J.; Mahmoodi, M.M.; Lu, T.J.; et al. Directed cell migration towards softer environments. Nat. Mater. 2022, 21, 1081–1090. [Google Scholar] [CrossRef]
- Sunyer, R.; Trepat, X. Durotaxis. Curr. Biol. 2020, 30, R383–R387. [Google Scholar] [CrossRef]
- Beedle, A.E.M.; Roca-Cusachs, P. In search of a softer environment. Nat. Mater. 2022, 21, 995–996. [Google Scholar] [CrossRef]
- Carter, S.B. Haptotaxis and the Mechanism of Cell Motility. Nature 1967, 213, 256. [Google Scholar] [CrossRef]
- Wen, J.H.; Choi, O.; Taylor-Weiner, H.; Fuhrmann, A.; Karpiak, J.V.; Almutairi, A.; Engler, A.J. Haptotaxis is Cell Type Specific and Limited by Substrate Adhesiveness. Cell. Mol. Bioeng. 2015, 8, 530–542. [Google Scholar] [CrossRef]
- King, S.J.; Asokan, S.B.; Haynes, E.M.; Zimmerman, S.P.; Rotty, J.D.; Alb, J.G.; Tagliatela, A.; Blake, D.R.; Lebedeva, I.P.; Marston, D.; et al. Lamellipodia are crucial for haptotactic sensing and response. J. Cell Sci. 2016, 129, 2329–2342. [Google Scholar] [CrossRef]
- Leclech, C.; Villard, C. Cellular and Subcellular Contact Guidance on Microfabricated Substrates. Front. Bioeng. Biotechnol. 2020, 8, 1198. [Google Scholar] [CrossRef] [PubMed]
- Fischer, R.S.; Sun, X.; Baird, M.A.; Hourwitz, M.J.; Seo, B.R.; Pasapera, A.M.; Mehta, S.B.; Losert, W.; Fischbach, C.; Fourkas, J.T.; et al. Contractility, focal adhesion orientation, and stress fiber orientation drive cancer cell polarity and migration along wavy ECM substrates. Proc. Natl. Acad. Sci. USA 2021, 118, e2021135118. [Google Scholar] [CrossRef]
- Kubow, K.E.; Shuklis, V.D.; Sales, D.J.; Horwitz, A.R. Contact guidance persists under myosin inhibition due to the local alignment of adhesions and individual protrusions. Sci. Rep. 2017, 7, 14380. [Google Scholar] [CrossRef]
- Juan, G.R.R.-S.; Oakes, P.W.; Gardel, M.L. Contact guidance requires spatial control of leading-edge protrusion. Mol. Biol. Cell 2017, 28, 1043–1053. [Google Scholar] [CrossRef]
- Tabdanov, E.D.; Rodríguez-Merced, N.J.; Cartagena-Rivera, A.X.; Puram, V.V.; Callaway, M.K.; Ensminger, E.A.; Pomeroy, E.J.; Yamamoto, K.; Lahr, W.S.; Webber, B.R.; et al. Engineering T cells to enhance 3D migration through structurally and mechanically complex tumor microenvironments. Nat. Commun. 2021, 12, 2815. [Google Scholar] [CrossRef]
- Comelles, J.; Fernández-Majada, V.; Acevedo, V.; Rebollo-Calderon, B.; Martínez, E. Soft topographical patterns trigger a stiffness-dependent cellular response to contact guidance. bioRxiv 2022, 2022.01.25.477731. [Google Scholar] [CrossRef]
- Park, J.Y.; Lee, D.H.; Lee, E.J.; Lee, S.-H. Study of cellular behaviors on concave and convex microstructures fabricated from elastic PDMS membranes. Lab. Chip 2009, 9, 2043–2049. [Google Scholar] [CrossRef]
- Song, K.H.; Park, S.J.; Kim, D.S.; Doh, J. Sinusoidal wavy surfaces for curvature-guided migration of T lymphocytes. Biomaterials 2015, 51, 151–160. [Google Scholar] [CrossRef]
- Pieuchot, L.; Marteau, J.; Guignandon, A.; Dos Santos, T.; Brigaud, I.; Chauvy, P.-F.; Cloatre, T.; Ponche, A.; Petithory, T.; Rougerie, P.; et al. Curvotaxis directs cell migration through cell-scale curvature landscapes. Nat. Commun. 2018, 9, 3995. [Google Scholar] [CrossRef]
- Baptista, D.; Teixeira, L.; van Blitterswijk, C.; Giselbrecht, S.; Truckenmüller, R. Overlooked? Underestimated? Effects of Substrate Curvature on Cell Behavior. Trends Biotechnol. 2019, 37, 838–854. [Google Scholar] [CrossRef]
- Vassaux, M.; Pieuchot, L.; Anselme, K.; Bigerelle, M.; Milan, J.-L. Designing Optimal Scaffold Topographies to Promote Nucleus-Guided Mechanosensitive Cell Migration Using In Silico Models. In Developments and Novel Approaches in Biomechanics and Metamaterials; Abali, B.E., Giorgio, I., Eds.; Advanced Structured Materials; Springer International Publishing: Cham, Switzerland, 2020; pp. 199–216. ISBN 978-3-030-50464-9. [Google Scholar]
- Winkler, B.; Aranson, I.S.; Ziebert, F. Confinement and substrate topography control cell migration in a 3D computational model. Commun. Phys. 2019, 2, 82. [Google Scholar] [CrossRef]
- Vassaux, M.; Pieuchot, L.; Anselme, K.; Bigerelle, M.; Milan, J.-L. A Biophysical Model for Curvature-Guided Cell Migration. Biophys. J. 2019, 117, 1136–1144. [Google Scholar] [CrossRef]
- Tambe, D.T. Collective cell guidance by cooperative intercellular forces. Nat. Mater. 2011, 10, 469–475. [Google Scholar] [CrossRef]
- Trepat, X.; Fredberg, J.J. Plithotaxis and emergent dynamics in collective cellular migration. Trends Cell Biol. 2011, 21, 638–646. [Google Scholar] [CrossRef]
- Malet-Engra, G.; Yu, W.; Oldani, A.; Rey-Barroso, J.; Gov, N.S.; Scita, G.; Dupré, L. Collective Cell Motility Promotes Chemotactic Prowess and Resistance to Chemorepulsion. Curr. Biol. 2015, 25, 242–250. [Google Scholar] [CrossRef]
- Camley, B.A. Collective gradient sensing and chemotaxis: Modeling and recent developments. J. Phys. Condens. Matter Inst. Phys. J. 2018, 30, 223001. [Google Scholar] [CrossRef]
- Wang, N.; Tytell, J.D.; Ingber, D.E. Mechanotransduction at a distance: Mechanically coupling the extracellular matrix with the nucleus. Nat. Rev. Mol. Cell Biol. 2009, 10, 75–82. [Google Scholar] [CrossRef]
- Ghassemi, S.; Meacci, G.; Liu, S.; Gondarenko, A.A.; Mathur, A.; Roca-Cusachs, P.; Sheetz, M.P.; Hone, J. Cells test substrate rigidity by local contractions on submicrometer pillars. Proc. Natl. Acad. Sci. USA 2012, 109, 5328–5333. [Google Scholar] [CrossRef]
- Plotnikov, S.V.; Pasapera, A.M.; Sabass, B.; Waterman, C.M. Force Fluctuations within Focal Adhesions Mediate ECM-Rigidity Sensing to Guide Directed Cell Migration. Cell 2012, 151, 1513–1527. [Google Scholar] [CrossRef]
- Trichet, L.; le Digabel, J.; Hawkins, R.J.; Vedula, S.R.K.; Gupta, M.; Ribrault, C.; Hersen, P.; Voituriez, R.; Ladoux, B. Evidence of a large-scale mechanosensing mechanism for cellular adaptation to substrate stiffness. Proc. Natl. Acad. Sci. USA 2012, 109, 6933–6938. [Google Scholar] [CrossRef]
- Hoffecker, I.T.; Guo, W.; Wang, Y. Assessing the spatial resolution of cellular rigidity sensing using a micropatterned hydrogel–photoresist composite. Lab Chip 2011, 11, 3538–3544. [Google Scholar] [CrossRef]
- Hu, S.; Chen, J.; Fabry, B.; Numaguchi, Y.; Gouldstone, A.; Ingber, D.E.; Fredberg, J.J.; Butler, J.P.; Wang, N. Intracellular stress tomography reveals stress focusing and structural anisotropy in cytoskeleton of living cells. Am. J. Physiol.-Cell Physiol. 2003, 285, C1082–C1090. [Google Scholar] [CrossRef] [PubMed]
- Autenrieth, T.J.; Frank, S.C.; Greiner, A.M.; Klumpp, D.; Richter, B.; Hauser, M.; Lee, S.; Levine, J.; Bastmeyer, M. Actomyosin contractility and RhoGTPases affect cell-polarity and directional migration during haptotaxis. Integr. Biol. 2016, 8, 1067–1078. [Google Scholar] [CrossRef] [PubMed]
- Koser, D.E.; Thompson, A.J.; Foster, S.K.; Dwivedy, A.; Pillai, E.K.; Sheridan, G.K.; Svoboda, H.; Viana, M.; da Costa, L.F.; Guck, J.; et al. Mechanosensing is critical for axon growth in the developing brain. Nat. Neurosci. 2016, 19, 1592–1598. [Google Scholar] [CrossRef]
- Yeoman, B.; Shatkin, G.; Beri, P.; Banisadr, A.; Katira, P.; Engler, A.J. Adhesion strength and contractility enable metastatic cells to become adurotactic. Cell Rep. 2021, 34, 108816. [Google Scholar] [CrossRef]
- Rappel, W.-J.; Edelstein-Keshet, L. Mechanisms of Cell Polarization. Curr. Opin. Syst. Biol. 2017, 3, 43–53. [Google Scholar] [CrossRef]
- Ridley, A.J.; Hall, A. The small GTP-binding protein rho regulates the assembly of focal adhesions and actin stress fibers in response to growth factors. Cell 1992, 70, 389–399. [Google Scholar] [CrossRef]
- Rohatgi, R.; Nollau, P.; Ho, H.Y.; Kirschner, M.W.; Mayer, B.J. Nck and phosphatidylinositol 4,5-bisphosphate synergistically activate actin polymerization through the N-WASP-Arp2/3 pathway. J. Biol. Chem. 2001, 276, 26448–26452. [Google Scholar] [CrossRef]
- Burridge, K.; Wennerberg, K. Rho and Rac take center stage. Cell 2004, 116, 167–179. [Google Scholar] [CrossRef]
- Rottner, K.; Schaks, M. Assembling actin filaments for protrusion. Curr. Opin. Cell Biol. 2019, 56, 53–63. [Google Scholar] [CrossRef]
- Møller, L.L.V.; Klip, A.; Sylow, L. Rho GTPases-Emerging Regulators of Glucose Homeostasis and Metabolic Health. Cells 2019, 8, E434. [Google Scholar] [CrossRef] [PubMed]
- Ridley, A.J. Life at the leading edge. Cell 2011, 145, 1012–1022. [Google Scholar] [CrossRef] [PubMed]
- Chrzanowska-Wodnicka, M.; Burridge, K. Rho-stimulated contractility drives the formation of stress fibers and focal adhesions. J. Cell Biol. 1996, 133, 1403–1415. [Google Scholar] [CrossRef] [PubMed]
- Worthylake, R.A.; Burridge, K. RhoA and ROCK promote migration by limiting membrane protrusions. J. Biol. Chem. 2003, 278, 13578–13584. [Google Scholar] [CrossRef]
- Mayor, R.; Carmona-Fontaine, C. Keeping in touch with contact inhibition of locomotion. Trends Cell Biol. 2010, 20, 319–328. [Google Scholar] [CrossRef]
- Li, Z.; Dong, X.; Dong, X.; Wang, Z.; Liu, W.; Deng, N.; Ding, Y.; Tang, L.; Hla, T.; Zeng, R.; et al. Regulation of PTEN by Rho small GTPases. Nat. Cell Biol. 2005, 7, 399–404. [Google Scholar] [CrossRef]
- Vemula, S.; Shi, J.; Hanneman, P.; Wei, L.; Kapur, R. ROCK1 functions as a suppressor of inflammatory cell migration by regulating PTEN phosphorylation and stability. Blood 2010, 115, 1785–1796. [Google Scholar] [CrossRef]
- Yang, S.; Kim, H.-M. The RhoA-ROCK-PTEN pathway as a molecular switch for anchorage dependent cell behavior. Biomaterials 2012, 33, 2902–2915. [Google Scholar] [CrossRef] [PubMed]
- Lawson, C.D.; Ridley, A.J. Rho GTPase signaling complexes in cell migration and invasion. J. Cell Biol. 2018, 217, 447–457. [Google Scholar] [CrossRef]
- Parent, C.A.; Devreotes, P.N. A Cell’s Sense of Direction. Science 1999, 284, 765–770. [Google Scholar] [CrossRef]
- Turing, A.M. The chemical basis of morphogenesis. Philos. Trans. R. Soc. Lond. B Biol. Sci. 1952, 237, 37–72. [Google Scholar] [CrossRef]
- Bailles, A.; Gehrels, E.W.; Lecuit, T. Mechanochemical Principles of Spatial and Temporal Patterns in Cells and Tissues. Annu. Rev. Cell Dev. Biol. 2022, 38, 321–347. [Google Scholar] [CrossRef] [PubMed]
- Houk, A.R.; Jilkine, A.; Mejean, C.O.; Boltyanskiy, R.; Dufresne, E.R.; Angenent, S.B.; Altschuler, S.J.; Wu, L.F.; Weiner, O.D. Membrane Tension Maintains Cell Polarity by Confining Signals to the Leading Edge during Neutrophil Migration. Cell 2012, 148, 175–188. [Google Scholar] [CrossRef] [PubMed]
- Yang, Y.; Xie, K.; Jiang, H. Durotaxis Index of 3T3 Fibroblast Cells Scales with Stiff-to-Soft Membrane Tension Polarity. Biophys. J. 2020, 119, 1427–1438. [Google Scholar] [CrossRef] [PubMed]
- Tsujita, K.; Takenawa, T.; Itoh, T. Feedback regulation between plasma membrane tension and membrane-bending proteins organizes cell polarity during leading edge formation. Nat. Cell Biol. 2015, 17, 749–758. [Google Scholar] [CrossRef] [PubMed]
- Keren, K.; Pincus, Z.; Allen, G.M.; Barnhart, E.L.; Marriott, G.; Mogilner, A.; Theriot, J.A. Mechanism of shape determination in motile cells. Nature 2008, 453, 475–480. [Google Scholar] [CrossRef]
- Meili, R.; Alonso-Latorre, B.; del Álamo, J.C.; Firtel, R.A.; Lasheras, J.C. Myosin II Is Essential for the Spatiotemporal Organization of Traction Forces during Cell Motility. Mol. Biol. Cell 2010, 21, 405–417. [Google Scholar] [CrossRef]
- Escribano, J.; Sánchez, M.T.; García-Aznar, J.M. Modeling the formation of cell-matrix adhesions on a single 3D matrix fiber. J. Theor. Biol. 2015, 384, 84–94. [Google Scholar] [CrossRef]
- Maiuri, P.; Rupprecht, J.-F.; Wieser, S.; Ruprecht, V.; Bénichou, O.; Carpi, N.; Coppey, M.; De Beco, S.; Gov, N.; Heisenberg, C.-P.; et al. Actin Flows Mediate a Universal Coupling between Cell Speed and Cell Persistence. Cell 2015, 161, 374–386. [Google Scholar] [CrossRef]
- Ron, J.E.; Monzo, P.; Gauthier, N.C.; Voituriez, R.; Gov, N.S. One-dimensional cell motility patterns. Phys. Rev. Res. 2020, 2, 033237. [Google Scholar] [CrossRef]
- Vedula, S.R.K.; Ravasio, A.; Lim, C.T.; Ladoux, B. Collective Cell Migration: A Mechanistic Perspective. Physiology 2013, 28, 370–379. [Google Scholar] [CrossRef] [PubMed]
- Sheetz, M.P.; Felsenfeld, D.; Galbraith, C.G.; Choquet, D. Cell migration as a five-step cycle. Biochem. Soc. Symp. 1999, 65, 233–243. [Google Scholar]
- Ulrich, T.A.; de Juan Pardo, E.M.; Kumar, S. The Mechanical Rigidity of the Extracellular Matrix Regulates the Structure, Motility, and Proliferation of Glioma Cells. Cancer Res. 2009, 69, 4167–4174. [Google Scholar] [CrossRef]
- Discher, D.E. Tissue Cells Feel and Respond to the Stiffness of Their Substrate. Science 2005, 310, 1139–1143. [Google Scholar] [CrossRef] [PubMed]
- Shi, Z.; Graber, Z.T.; Baumgart, T.; Stone, H.A.; Cohen, A.E. Cell membranes resist flow. bioRxiv 2018, 175, 1769–1779. [Google Scholar] [CrossRef] [PubMed]
- Belly, H.D.; Yan, S.; da Rocha, H.B.; Ichbiah, S.; Town, J.P.; Turlier, H.; Bustamante, C.J.; Weiner, O.D. Actin-driven protrusions generate rapid long-range membrane tension propagation in cells. bioRxiv 2022, 2022.09.07.507005. [Google Scholar] [CrossRef]
- Canales Coutiño, B.; Mayor, R. Mechanosensitive ion channels in cell migration. Cells Dev. 2021, 166, 203683. [Google Scholar] [CrossRef]
- Becker, D.; Bereiter-Hahn, J.; Jendrach, M. Functional interaction of the cation channel transient receptor potential vanilloid 4 (TRPV4) and actin in volume regulation. Eur. J. Cell Biol. 2009, 88, 141–152. [Google Scholar] [CrossRef]
- Fiorio Pla, A.; Ong, H.L.; Cheng, K.T.; Brossa, A.; Bussolati, B.; Lockwich, T.; Paria, B.; Munaron, L.; Ambudkar, I.S. TRPV4 mediates tumor-derived endothelial cell migration via arachidonic acid-activated actin remodeling. Oncogene 2012, 31, 200–212. [Google Scholar] [CrossRef]
- Fabian, A.; Bertrand, J.; Lindemann, O.; Pap, T.; Schwab, A. Transient receptor potential canonical channel 1 impacts on mechanosignaling during cell migration. Pflugers Arch. 2012, 464, 623–630. [Google Scholar] [CrossRef]
- Damann, N.; Owsianik, G.; Li, S.; Poll, C.; Nilius, B. The calcium-conducting ion channel transient receptor potential canonical 6 is involved in macrophage inflammatory protein-2-induced migration of mouse neutrophils. Acta Physiol. Oxf. Engl. 2009, 195, 3–11. [Google Scholar] [CrossRef]
- Lepannetier, S.; Zanou, N.; Yerna, X.; Emeriau, N.; Dufour, I.; Masquelier, J.; Muccioli, G.; Tajeddine, N.; Gailly, P. Sphingosine-1-phosphate-activated TRPC1 channel controls chemotaxis of glioblastoma cells. Cell Calcium 2016, 60, 373–383. [Google Scholar] [CrossRef] [PubMed]
- Legant, W.R.; Choi, C.K.; Miller, J.S.; Shao, L.; Gao, L.; Betzig, E.; Chen, C.S. Multidimensional traction force microscopy reveals out-of-plane rotational moments about focal adhesions. Proc. Natl. Acad. Sci. USA 2013, 110, 881–886. [Google Scholar] [CrossRef] [PubMed]
- Dalaka, E.; Kronenberg, N.M.; Liehm, P.; Segall, J.E.; Prystowsky, M.B.; Gather, M.C. Direct measurement of vertical forces shows correlation between mechanical activity and proteolytic ability of invadopodia. Sci. Adv. 2020, 6, eaax6912. [Google Scholar] [CrossRef] [PubMed]
- Li, D.; Colin-York, H.; Barbieri, L.; Javanmardi, Y.; Guo, Y.; Korobchevskaya, K.; Moeendarbary, E.; Li, D.; Fritzsche, M. Astigmatic traction force microscopy (aTFM). Nat. Commun. 2021, 12, 2168. [Google Scholar] [CrossRef]
- Owen, L.M.; Adhikari, A.S.; Patel, M.; Grimmer, P.; Leijnse, N.; Kim, M.C.; Notbohm, J.; Franck, C.; Dunn, A.R. A cytoskeletal clutch mediates cellular force transmission in a soft, three-dimensional extracellular matrix. Mol. Biol. Cell 2017, 28, 1959–1974. [Google Scholar] [CrossRef]
- Garcin, C.; Straube, A. Microtubules in cell migration. Essays Biochem. 2019, 63, 509–520. [Google Scholar] [CrossRef]
- Guo, M.; Ehrlicher, A.J.; Mahammad, S.; Fabich, H.; Jensen, M.H.; Moore, J.R.; Fredberg, J.J.; Goldman, R.D.; Weitz, D.A. The Role of Vimentin Intermediate Filaments in Cortical and Cytoplasmic Mechanics. Biophys. J. 2013, 105, 1562–1568. [Google Scholar] [CrossRef]
- Kechagia, Z.; Sáez, P.; Gómez-González, M.; Zamarbide, M.; Andreu, I.; Koorman, T.; Beedle, A.E.M.; Derksen, P.W.B.; Trepat, X.; Arroyo, M.; et al. The laminin-keratin link shields the nucleus from mechanical deformation and signalling. bioRxiv 2022, 2022.03.01.482474. [Google Scholar] [CrossRef]
- Shellard, A.; Mayor, R. Collective durotaxis along a self-generated stiffness gradient in vivo. Nature 2021, 600, 690–694. [Google Scholar] [CrossRef]
- Roca-Cusachs, P.; Sunyer, R.; Trepat, X. Mechanical guidance of cell migration: Lessons from chemotaxis. Curr. Opin. Cell Biol. 2013, 25, 543–549. [Google Scholar] [CrossRef] [PubMed]



Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Fortunato, I.C.; Sunyer, R. The Forces behind Directed Cell Migration. Biophysica 2022, 2, 548-563. https://doi.org/10.3390/biophysica2040046
Fortunato IC, Sunyer R. The Forces behind Directed Cell Migration. Biophysica. 2022; 2(4):548-563. https://doi.org/10.3390/biophysica2040046
Chicago/Turabian StyleFortunato, Isabela C., and Raimon Sunyer. 2022. "The Forces behind Directed Cell Migration" Biophysica 2, no. 4: 548-563. https://doi.org/10.3390/biophysica2040046
APA StyleFortunato, I. C., & Sunyer, R. (2022). The Forces behind Directed Cell Migration. Biophysica, 2(4), 548-563. https://doi.org/10.3390/biophysica2040046





