The Dynamics of Subunit Rotation in a Eukaryotic Ribosome

Abstract

Protein synthesis by the ribosome is coordinated by an intricate series of large-scale conformational rearrangements. Structural studies can provide information about long-lived states, however biological kinetics are controlled by the intervening free-energy barriers. While there has been progress describing the energy landscapes of bacterial ribosomes, very little is known about the energetics of large-scale rearrangements in eukaryotic systems. To address this topic, we constructed an all-atom model with simplified energetics and performed simulations of subunit rotation in the yeast ribosome. In these simulations, the small subunit (SSU; ∼1 MDa) undergoes spontaneous and reversible rotation events (∼8${}^{\circ }$). By enabling the simulation of this rearrangement under equilibrium conditions, these calculations provide initial insights into the molecular factors that control dynamics in eukaryotic ribosomes. Through this, we are able to identify specific inter-subunit interactions that have a pronounced influence on the rate-limiting free-energy barrier. We also show that, as a result of changes in molecular flexibility, the thermodynamic balance between the rotated and unrotated states is temperature-dependent. This effect may be interpreted in terms of differential molecular flexibility within the rotated and unrotated states. Together, these calculations provide a foundation, upon which the field may begin to dissect the energetics of these complex molecular machines.

1. Introduction

The ribosome is a massive molecular assembly that undergoes a wide range conformational rearrangements in order to accurately synthesize proteins [1,2,3,4]. The precise composition of a ribosome is organism-specific, though it generally contains two large RNA (rRNA) chains (thousands of nucleotides in length, each), in addition to a variable number of smaller RNA and protein molecules (∼50–100, in total). The overall architecture of the ribosome is commonly described in terms of the large subunit (LSU) and small subunit (SSU), where each has three distinct tRNA binding sites (Figure 1). During protein synthesis, aminoacyl-transfer RNA (aa-tRNA) molecules must decode messenger RNA (mRNA). Upon recognition of an mRNA codon, the incoming aa-tRNA molecule binds the ribosomal A site. The nascent protein chain that is attached to the P-site tRNA is then passed to the A-site tRNA through the formation of a peptide bond. After peptide bond formation, the A-site and P-site tRNA molecules are displaced to the P and E sites, respectively. This process, called translocation, leads to a vacant A site, which allows the ribosome to decode the next mRNA codon.

The process of tRNA translocation involves large-scale displacements of tRNA molecules (∼40 Å), which are facilitated by an elaborate sequence of collective rearrangements within the ribosome. In bacteria, countless cryo-EM and crystallographic structures have been resolved in which the SSU is rotated relative to the LSU (i.e., body rotation [5,6]), or the SSU head is rotated relative to the SSU body (head swivel [7,8] and tilting [9]). To complement these structural snapshots, biochemical measurements [10] and single-molecule studies [11,12,13,14,15] have identified coupling between SSU rearrangements and tRNA dynamics. Single-molecule measurements have also suggested aspects of hybrid-state formation are similar in eukaryotic ribosomes [16,17]. Similar to bacterial ribosomes, structural models of eukaryotic cytoplasmic [18,19] and mitochondrial [20] ribosomes have illustrated the broad range of orientations that are accessible to the SSU. In addition to rotary-like rearrangements, cryo-EM structures have also revealed that the SSU body may undergo tilt-like rearrangements in eukaryotic systems (called “rolling” [19]). These more recent insights into eukaryotic structure raise new questions into the relationship between SSU motion and tRNA dynamics. For example, how do differences in eukaryotic and bacterial ribosome structure give rise to differential dynamics? What is the relationship between SSU rotation and eukaryotic-specific tilting/rolling? While there is significant interest in understanding these dynamic properties, the biophysical features that govern eukaryotic translation are largely unexplored.

For nearly two decades, advances in structure determination have fueled the development and application of theoretical models to study subunit rotation in bacteria. In the earliest theoretical efforts, coarse-grained models were utilized to perform normal mode analysis [21] and principal component analysis [22], which illustrated how the architecture of the ribosome predisposes it to rotation-like fluctuations of the SSU. Later, highly-detailed explicit-solvent simulations (100 ns–1 μs) were applied to study small-scale structural fluctuations [23,24], which reinforced the predicted energetic accessibility of rotary-like fluctuations. Explicit-solvent simulations have also been applied to characterize how SSU-LSU bridge interactions may facilitate large-scale rotation [25]. Complementary to explicit-solvent methods, recently-developed coarse-grained models have allowed for spontaneous rotation events to be simulated [26]. With those models, it has been shown how specific subunit bridges can “hand off” the SSU during rotation. While each effort has provided insights into distinct aspects of rotation in bacterial ribosomes, simulations of spontaneous and reversible SSU rotation in a eukaryotic ribosome have not been reported previously.

In the current study, we provide a physicochemical foundation for understanding the dynamics of SSU rotation in eukaryotic ribosomes. Specifically, we developed and applied an all-atom structure-based (SMOG) model [27,28] to study the dynamics of subunit rotation in the yeast ribosome. This model includes all non-hydrogen atoms (206 k atoms, in total), and the energetics are defined to explicitly stabilize the rotated and unrotated conformations. Using this simplified model, we were able to simulate 25 reversible rotation events, where the SSU spontaneously rotated/back-rotated by ∼8${}^{\circ }$. With this data set, we provide an initial description of the free-energy landscape associated with SSU rotation. This analysis reveals a distinct sequence of rearrangements at the SSU-LSU interface, as well as a pronounced temperature dependence of the free-energy landscape. Together, these calculations establish a technical and conceptual framework for rigorously characterizing eukaryotic ribosome dynamics, both through theoretical and experimental approaches.

2. Materials and Methods

2.1. Multi-Basin Structure-Based Model

In the current study, we developed a multi-basin structure-based model, where knowledge of the rotated and unrotated conformations (PDB: 3J77 and 3J78 [18]) were used to define the potential energy function. For this, we applied a SMOG-AMBER variant [29] of the SMOG class of structure-based models [28], where the potential energy is given by:

$$\begin{array}{cc}\hfill V& =\sum _{bonds}\frac{{ϵ}_r}{2}{\left(r_{ij}-r_{ij,0}\right)}^2+\sum _{angles}\frac{{ϵ}_{\theta }}{2}{\left({\theta }_{ijk}-{\theta }_{ijk,0}\right)}^2+\hfill \\ \hfill & +\sum _{impropers}\frac{{ϵ}_{{\chi }_{imp}}}{2}{\left({\chi }_{ijkl}-{\chi }_{ijkl,0}\right)}^2+\sum _{planar}\frac{{ϵ}_{{\chi }_{planar}}}{2}{F}_P\left({\phi }_{ijkl}\right)+\hfill \\ \hfill & +\sum _{backbone}{ϵ}_{bb}{F}_D{\left({\varphi }_{ijkl}-{\varphi }_{ijkl,0}\right)}^{}+\sum _{sidechains}{ϵ}_{sc}{F}_D{\left({\varphi }_{ijkl}-{\varphi }_{ijkl,0}\right)}^{}+\hfill \\ \hfill & +\sum _{contacts}{ϵ}_C\left[{\left(\frac{{\sigma }_{ij}}{r_{ij}}\right)}^{12}-2{\left(\frac{{\sigma }_{ij}}{r_{ij}}\right)}^6\right]\hfill \\ \hfill & +\sum _{non-contacts}{ϵ}_{nc}{\left(\frac{{\sigma }_{nc}}{r_{ij}}\right)}^{12}.\hfill \end{array}$$

(1)

Here, $F_D\left(\varphi \right)=[1-cos\left(\varphi \right)]+\frac{1}{2}[1-cos\left(3\varphi \right)]$ and $F_P\left(\phi \right)=[1-cos\left(2\phi \right)]$. The bonded parameters ($r_{ij,0}$ and ${\theta }_{ijk,0}$) were obtained from the AMBER03 force field [30]. The planar ring dihedrals were maintained by cosine potentials of periodicity 2. The position of the minimum of each dihedral angle ${\varphi }_{ijkl,0}$ was defined as the mean value adopted in the rotated and unrotated structures. This ensures that the dihedral energies in the two structures are isoenergetic. Combined with the AMBER-based bonded geometry, these terms ensure there is no intrasubunit bias toward either endpoint configuration. Dihedral energies were assigned as described previously [28]. For completeness, we will summarize the details, here. To define the dihedral interaction weights (${ϵ}_{bb}$ and ${ϵ}_{sc}$), dihedrals were first grouped based on the composition of the middle bond. Each dihedral group was given a summed weight of ${ϵ}_{bb}$ or ${ϵ}_{sc}$. The ratio $R_{bb/sc}=\frac{{ϵ}_{bb}}{{ϵ}_{sc}}$ was set to 1 (nucleic acids) or 2 (proteins). ${ϵ}_{bb}$ was defined to be equal for protein and nucleic acids. As a note, it was previously shown that folding mechanisms and free-energy barriers are largely robust for $1\le R_{bb/sc}\le 4$ [27]. Contact and dihedral strengths were scaled, such that

$$R_{C/D}=\frac{\sum {ϵ}_C}{\sum {ϵ}_{bb}+\sum {ϵ}_{sc}}=2$$

and

$$\sum {ϵ}_C+\sum {ϵ}_{bb}+\sum {ϵ}_{sc}=Nϵ,$$

where N is the number of atoms in the system and $ϵ$ is the reduced energy unit, which is equal to $2k_{B}T$. Contact pairs were defined using the Shadow Contact Map algorithm with default values [31]. ${\sigma }_{ij}$ was set to 0.96 ${\sigma }_{ij}^{{}^{\prime }}$ where ${\sigma }_{ij}^{{}^{\prime }}$ is the interatomic distance between atoms that are in contact in the rotated (or unrotated) configuration. This scaling of contacts was introduced to avoid the artificial expansion of the ribosome that can arise from configurational entropy [29,32].

To construct the multi-basin force field, any inter-subunit contact pair that is common to both structures was assigned a single 6–12 interaction. The position of the minimum was defined for each atom pair, such that each common contact is isoenergetic (with value ${ϵ}_{\mathrm{iso}}$), with respect to the rotated and unrotated conformations (Figure S1). If ${ϵ}_{\mathrm{iso}}/{ϵ}_C>1/2$, the contacting pair of atoms was classified as a “common” contact. Applying this criterion, all inter-subunit pairs whose distances are similar in both structures were assigned an isoenergetic distance. All intra-subunit contact pairs were also considered common contacts. Consistent with the dihedral parameters, this isoenergetic assignment of contacts ensures that common interactions do not favor either rotation state. The weights of common contacts (inter-subunit and intra-subunit) followed the scaling rules described above [28]. For the remaining interface contacts (i.e., unique contacts), ${\sigma }_{ij}$ was assigned the value found in the conformation for which the contact is defined: (un)rotated contacts are given the distances found in the (un)rotated conformation. Contacts unique to the rotated configuration were then given an energetic weight of 0.21 and contacts unique to the unrotated configuration were given weights 0.19. An initial parameter sweep was performed to identify weights for which the rotated and unrotated conformations represent pronounced free-energy minima of comparable depths.

Since the available cryo-EM reconstructions resolved different numbers of atoms, minor components of the structure had to be structurally modeled. Consistent with previous efforts [32], position-restraint-based techniques were applied to model the missing regions, where the completed region in the alternate structure was aligned to the missing region. With this approach, residues 65–135 of eL24 were reconstructed in the unrotated structure (3J78), and residues 2061–2075 of the 25S rRNA were reconstructed in the rotated structure. After these steps, both models contained 206,389 non-hydrogen atoms. See Supplementary Materials for details.

2.2. Simulation Details

Simulations were performed using the GROMACS software package (v5.1.4) [33,34]. Force field files were generated using SMOG v2.3 [28] with the SBM_AA-amber-bonds force field templates [29], which are available through the smog-server.org force field repository. The contacts and dihedrals were then subsequently modified by custom scripts. Two simulations were initiated from the unrotated structure (PDB: 3J78 [18]). To ensure robustness of the results, an additional simulation was initiated from the rotated configuration (PDB: 3J77 [18]). The temperature was maintained via Langevin Dynamics protocols, with a value of 60 in GROMACS units, or a reduced value of 0.49887, for all simulations. Each simulation was continued for at least $7·{10}^9$ time steps. Using the estimate of Yang et al. [35], each simulation represents an effective timescale of approximately 15 ms. To allow for equilibration, the first ${10}^7$ time steps were excluded from analysis. The time step was 0.002 reduced units and configurations were saved every 5000 time steps.

2.3. SSU Rotation Measures

To describe the orientation of the SSU, we extended the definition of rotation angles that were originally introduced for bacterial ribosomes [32,36]. Consistent with previous descriptions [32], the overall strategy is to identify sets of residues that are structurally conserved between the rRNA of the LSU and SSU body in E. coli and yeast. Using these structurally-conserved residues, a reference E. coli structure is separately aligned to the LSU and SSU body of the yeast ribosome. This allows one to approximate the position of the SSU body and head in terms of rigid-body rotations, relative to E. coli. The reference E. coli model is PDB entry 4V6D [6], where the axis of pure rotation is defined by the structures of the classical and rotated body. With this definition, we decompose the rotation in terms of Euler angles ($\varphi ,\theta ,\psi$). Here, we report the net body rotation as ${\varphi }_{\mathrm{body}}=\varphi +\psi$, body tilting/rolling as ${\theta }_{\mathrm{body}}=\theta$ and the tilt direction as ${\psi }_{\mathrm{body}}=\varphi +C$. C is an arbitrary constant that ensures ${\psi }_{\mathrm{body}}=0$ describes tilting motions that are roughly about the long axis of helix 44 in the SSU body.

In the current study, we extended our protocol for defining angles, in order to apply Euler Angle decomposition to the yeast ribosome, while still allowing for direct comparisons with SSU orientations in other organisms. First, we performed STAMP alignment [37] to determine the corresponding E. coli numbering of the yeast rRNA residues. STAMP was applied separately to the LSU and SSU body. Then, for the LSU and SSU body, we applied a second, more stringent, condition to define which residues in the rotated yeast structure are structurally conserved with the reference E. coli structure. Specifically, we performed least-squares alignment of the STAMP-aligned residues and calculated the spatial deviation of each P atom. Any P atom that deviated by more than 1 Å was then excluded in a second round of fitting. After the second round of alignment, all P atoms (including those that were not used for fitting) that are within (above) 1 Å are included in (excluded from) the fitting group. This process was repeated until the set of included residues converged. These sets of residues, which we will call the “core” groups, were then used for all subsequent angle calculations in the study. All angle calculations were performed using in-house scripts written for use with VMD [38].

3. Results

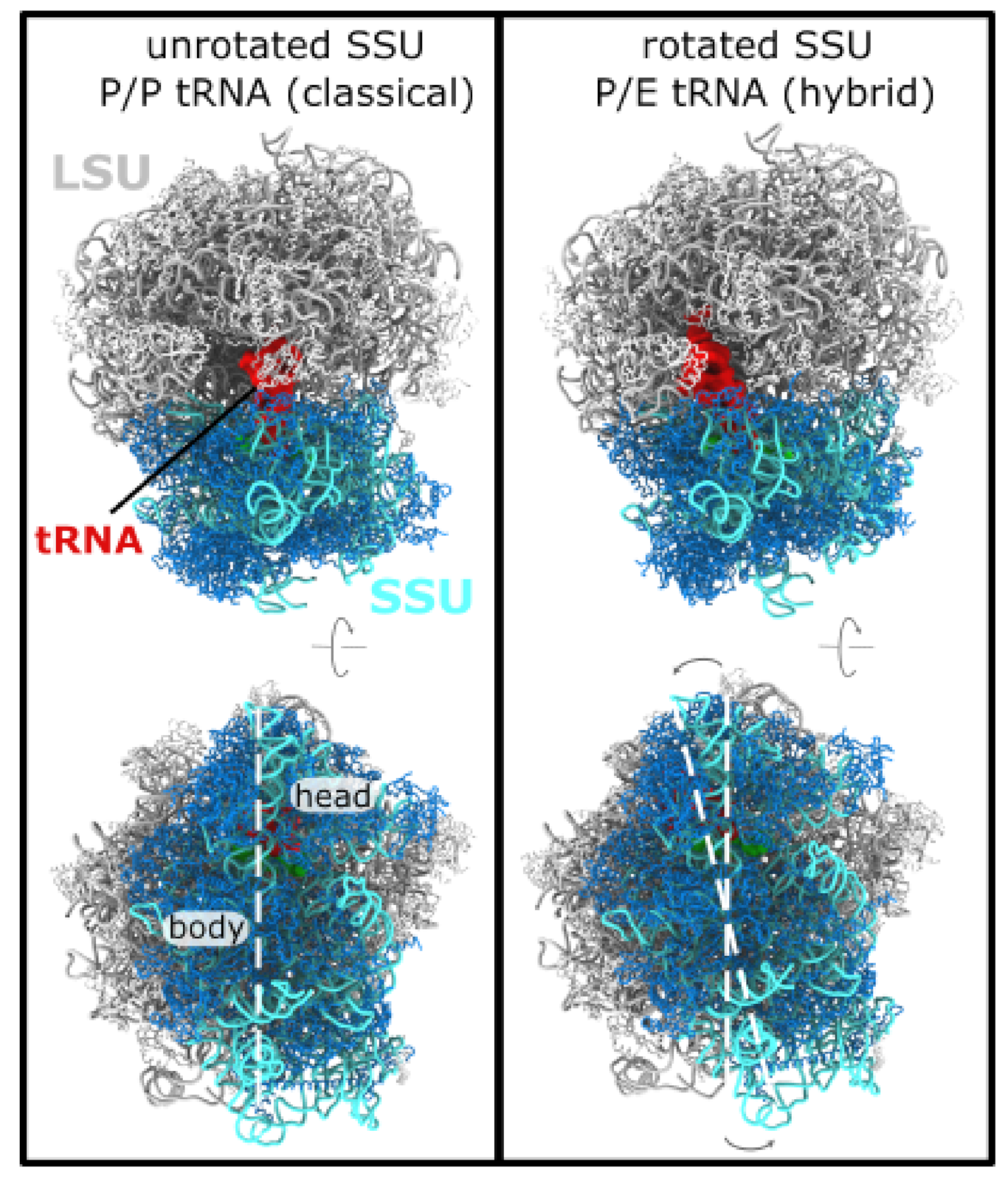
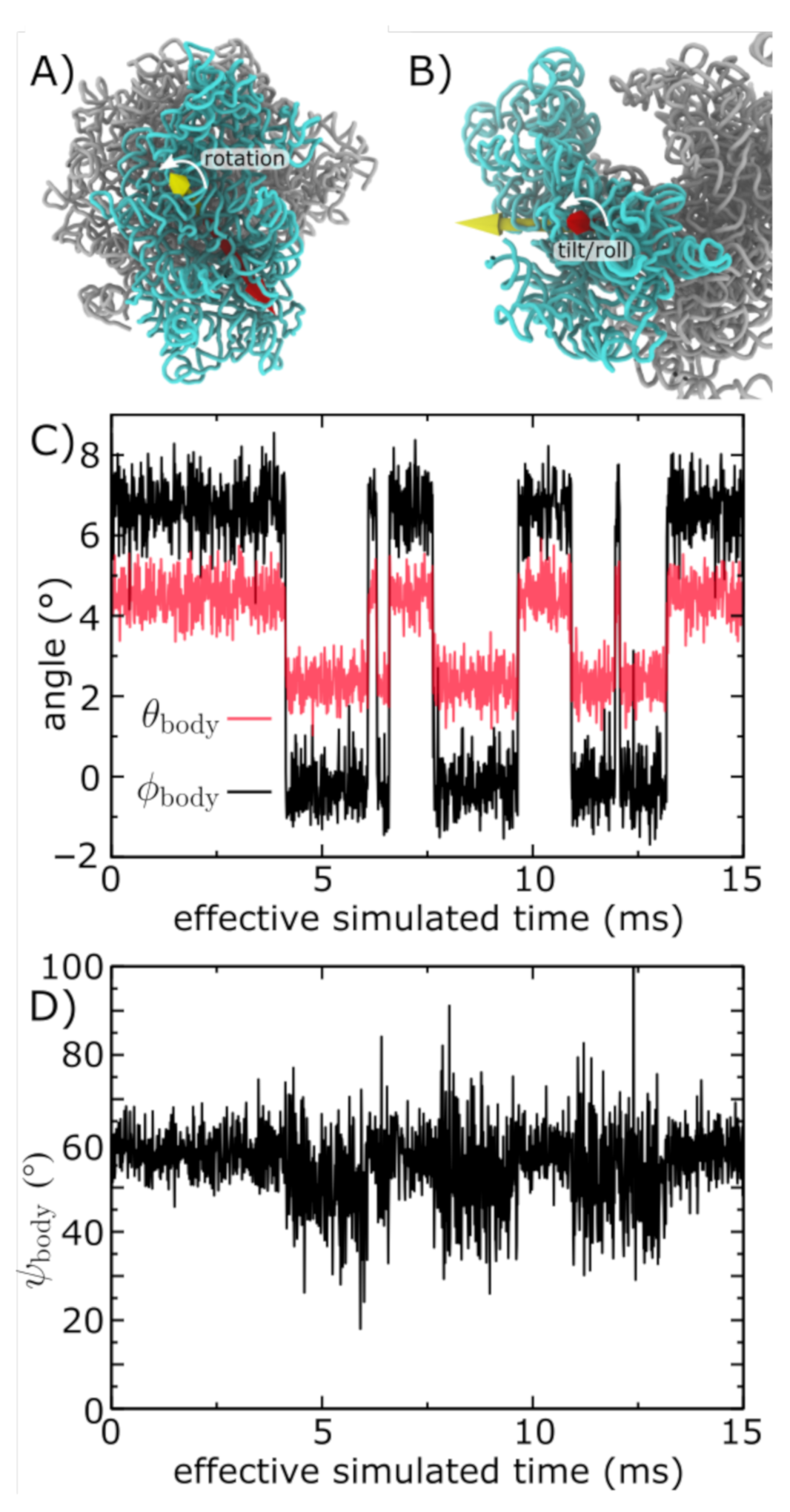
3.1. Simulating Spontaneous Subunit Rotation Events in a Complete Ribosome

To study the dynamics of subunit rotation for a eukaryotic ribosome, we applied molecular dynamics simulations with an all-atom model (206,389 atoms) that employs a simplified energetic representation. Specifically, we used a multi-basin structure-based model, which is inspired by similar models for the study of multi-domain proteins [39]. In this model, all non-hydrogen atoms are represented, and the stabilizing energetic interactions are explicitly defined to stabilize the rotated and unrotated configurations of the ribosome. Here, we define the interactions based on cryo-EM structures of a yeast ribosome in rotated and unrotated conformations (Figure 1). With this representation, we were able to simulate spontaneous (i.e., without targeting techniques) and reversible transitions between rotated and unrotated conformations (See supplementary movie).

When interpreting the physical significance of the simulated dynamics, one should recognize that the modeled interactions are intended to reflect the effective energetics of the system [40,41,42]. That is, structures that have been resolved necessarily represent free-energy minima. In the structure-based model, we directly encode these free-energy minima by defining contacts and dihedrals to stabilize the pre-assigned structures. This general approach has been applied recently to study rotation in a bacterial ribosome with a coarse-grained model [26]. In the current study, we extend to an all-atom representation, such that the presented model may later be used to study the precise influence of sterics during tRNA rearrangements in the ribosome. This consideration is motivated by previous simulations of bacterial ribosomes, which have demonstrated the critical influence of molecular sterics on tRNA dynamics during accommodation [43], A/P hybrid formation [44], P/E hybrid formation [45] and tRNA translocation [32]. In order for our model to have future utility to address these motions in eukaryotic ribosomes, it is necessary to employ atomic resolution. Here, we focus on rotation in the absence of a bound tRNA molecule.

In order to characterize the dynamics of SSU rearrangements, one must define appropriate collective coordinates that distinguish between rotated and unrotated orientations of the small subunit (SSU). To this end, we employed Euler Angle decomposition, as used previously to describe bacterial ribosomes [32,36]. Here, this method was generalized (see Methods for details) for use with non-bacterial ribosomes. Consistent with previous efforts to quantify rotation angles in the ribosome [32], we first defined sets of residues within the LSU and SSU body that undergo minimal intra-domain rearrangements during rotation (called the “core” residues). We then aligned reference structures of the core residues to the LSU and SSU body. The orientations of the aligned structures were then quantified in terms of Euler angles, which may be expressed as a net body rotation angle (${\varphi }_{\mathrm{body}}$), a body tilt angle (${\theta }_{\mathrm{body}}$) and a corresponding tilt direction (${\psi }_{\mathrm{body}}$. See Methods for details). Pure body rotation leads to ${\varphi }_{\mathrm{body}}\ne 0$, while ${\theta }_{\mathrm{body}}=0$, where the rotation axis is parallel to that defined by reference structures of E. coli [6]. Non-zero values of the tilt angle ${\theta }_{\mathrm{body}}$ represent any level of deviation from pure rotation. In relation to other studies of eukaryotic ribosomes, the tilt angle measures the so-called “rolling” rearrangement of the SSU body [19]. For consistency with descriptions of SSU head motion [32], we use the term “tilting” to describe such motions. To complete this description of subunit rotation, the direction of tilting is given by ${\psi }_{\mathrm{body}}$, where ${\psi }_{\mathrm{body}}\sim {50}^{\circ }$ corresponds to the tilting/rolling rearrangement that is apparent in cryo-EM models of the yeast ribosome [18].

Using our structure-based model, we performed several independent simulations, in which a total of 25 rotation and backrotation events were observed (Figure 2C,D). While applying atomic resolution is computationally demanding, the simulated events were obtained without the use of enhanced sampling methods, or artificial targeting forces. Rotation events are apparent in the time traces, where there are sharp changes in ${\varphi }_{\mathrm{body}}$ of approximately $8^{\circ }$ (Figure 2C). There are also abrupt transitions in the tilting angle ${\theta }_{\mathrm{body}}$ that coincide with changes in the rotation angle. Thus, this model suggests that subunit rotation and tilting/rolling are not kinetically-separable processes. While one could expect two-state-like behavior in this type of simplified model, it is common for these models to reveal sterically-induced free-energy minima [46]. That is, while the models define specific conformations as stable, the imperfect complementarity of steric interactions can impede the motion and lead to long-lived intermediates. Despite this possibility, we find that the steric composition of the SSU-LSU interface appears to be sufficiently smooth that rotation and tilting can occur simultaneously and in a two-state manner.

With regards to tilting dynamics, we find there are large variations in the tilt direction ${\psi }_{\mathrm{body}}$ within the unrotated ensemble (Figure 2D). These large variations are expected, since the tilt direction is undefined when the tilt angle is zero. Accordingly, when ${\theta }_{\mathrm{body}}$ is small, structural fluctuations associated with thermal energy can give rise to minimal changes in ${\theta }_{\mathrm{body}}$ and large changes in ${\psi }_{\mathrm{body}}$.

Overall, the presented simulations demonstrate how an all-atom structure-based model can be used to provide a first-order approximation to the dynamics of rotation in a eukaryotic ribosome. Since these simulations describe spontaneous rotation events, the data set provides an opportunity to gain initial insights into the relative timing of inter-subunit contact formation during rotation, as well as the impact of specific interactions on the free-energy landscape.

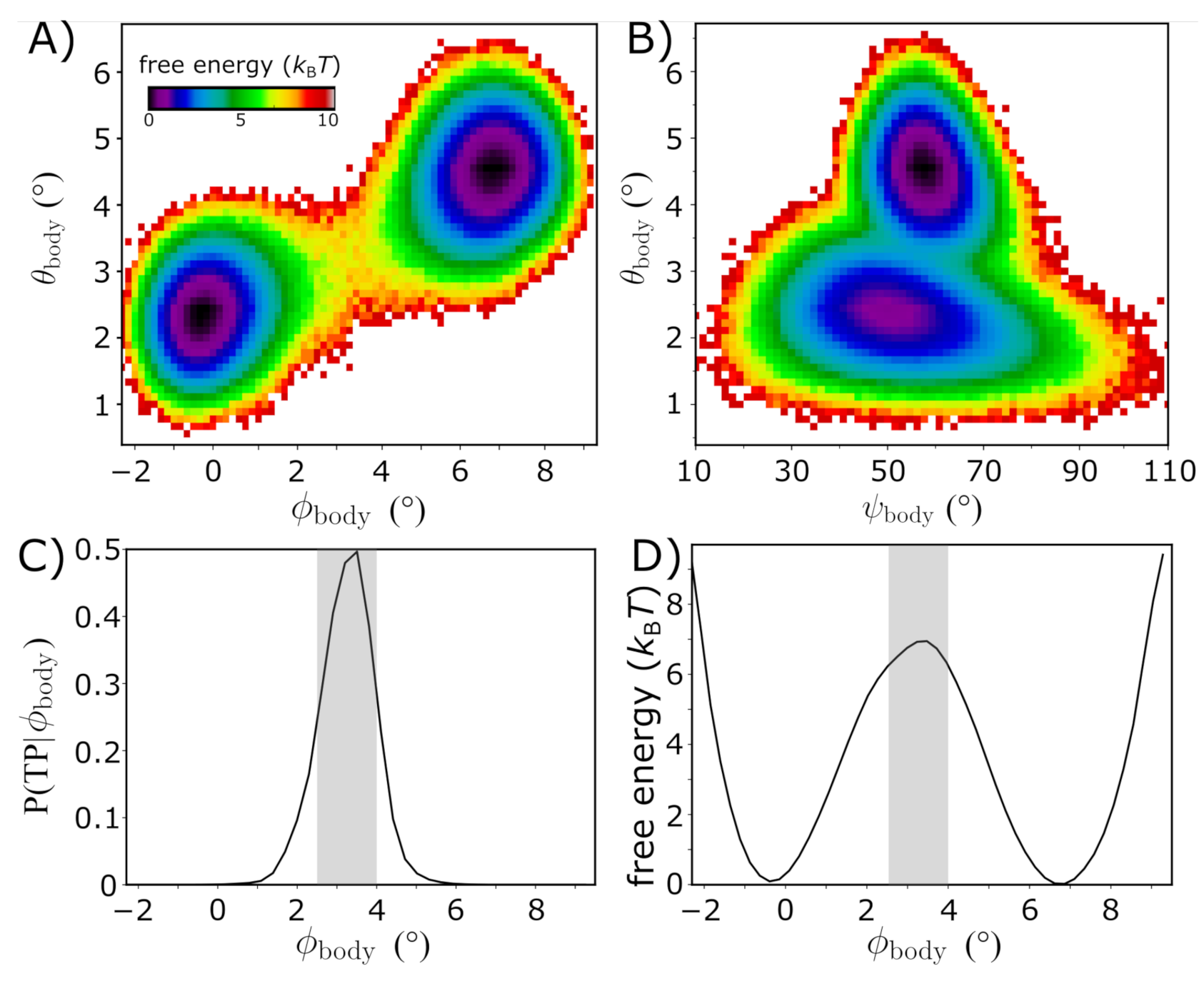
3.2. Quantifying the Energy Landscape of Rotation

A persistent challenge in molecular biophysics is to define low-dimensional measures that can accurately capture the dynamics of complex multi-dimensional processes [47,48,49,50,51]. In addition to posing an intellectual challenge, there is also a practical utility of identifying appropriate coordinates. Specifically, knowledge of appropriate one-dimensional measures can allow one to precisely characterize the relative contributions of individual interactions to biological kinetics. To this end, we explored multiple approaches for describing the simulated SSU rotation events, which together help establish a physical-chemical foundation for the analysis of eukaryotic ribosome dynamics.

Visual inspection of individual simulated time traces suggests there is a strong correlation between SSU rotation and tilting/rolling (Figure 2C). To better understand the relationship between these motions, we calculated the two-dimensional free energy ($-k_{B}Tln\left(P\right)$) as a function of ${\varphi }_{\mathrm{body}}$ and ${\theta }_{\mathrm{body}}$ (Figure 3A). Consistent with the time traces, there are two distinct minima corresponding to the rotated and unrotated ensembles. However, rotation and tilting are not perfectly correlated, and there is a notable degree of variability in ${\theta }_{\mathrm{body}}$ within each ensemble. In the unrotated ensemble (low ${\varphi }_{\mathrm{body}}$ values), the tilt angle samples values that range from 1${}^{\circ }$ to 4${}^{\circ }$. Similarly, in the rotated ensemble (high ${\varphi }_{\mathrm{body}}$ values), ${\theta }_{\mathrm{body}}$ spans a wide range of values (2.5–6.5${}^{\circ }$). Interestingly, as the body tilts/rolls, the direction of tilting also shifts. Specifically, the direction of tilting (${\psi }_{\mathrm{body}}$) within the unrotated ensemble is typically around 50${}^{\circ }$, while the rotated ensemble represents configurations for which the tilt direction is towards higher ${\psi }_{\mathrm{body}}$ values. This illustrates how, upon reaching the rotated/tilted ensemble, the accessible tilting fluctuations change in character. Based on the current simulations, it is not clear whether this change in tilting dynamics has a specific biological role, though it may help coordinate tRNA dynamics during hybrid formation and/or translocation.

Since rotation and tilting are found to be correlated, we asked whether a one-dimensional description can be sufficient to quantify the free-energy barrier and kinetics associated with SSU motion. To determine whether the rotation angle ${\varphi }_{\mathrm{body}}$ provides a kinetically-meaningful approximation to the free-energy barrier, we employed multiple independent analysis strategies. First, we applied transition path analysis [47,52] to assess whether ${\varphi }_{\mathrm{body}}$ can unambiguously identify the transition state ensemble (TSE). That is, we calculated the probability that the system is undergoing a transition (i.e., is on a Transition Path), as a function of ${\varphi }_{\mathrm{body}}$: $P(\mathrm{TP}$|${\varphi }_{\mathrm{body}})$. If ${\varphi }_{\mathrm{body}}$ is able to unambiguously identify the TSE, then $P(\mathrm{TP}$|${\varphi }_{\mathrm{body}})$ will adopt the diffusion-limited value of 0.5. Here, we find that $P(\mathrm{TP}$|${\varphi }_{\mathrm{body}})$ reaches a peak value of $\approx 0.5$, indicating that ${\varphi }_{\mathrm{body}}$ is suitable for describing the underlying barrier. Further, this allows us to identify the position of the TSE: ${\varphi }_{\mathrm{body}}\sim 3.5^{\circ }$. As a point of comparison, in the study of protein folding [47,52], a coordinate $\rho$ is often considered “good” if $P\left(\mathrm{TP}\right|\rho )$ reaches values that are greater than ∼0.4.

In addition to capturing the TSE, we find that ${\varphi }_{\mathrm{body}}$ is able to recapitulate the long-time dynamics of SSU rotation. To illustrate this, we used a Bayesian inference approach [52] to calculate the diffusion coefficient as a function of ${\varphi }_{\mathrm{body}}$. This produced a nearly-uniform value of the diffusion coefficient D that was approximately $0.0025{\left(\mathrm{degrees}\right)}^2/{\tau }_{\mathrm{ru}}$. ${\tau }_{\mathrm{ru}}$ is the reduced time unit. We used this value of D to estimate the mean first passage time via the relation:

$$\langle \tau \rangle ={\int }_{{\rho }_{\mathrm{initial}}}^{{\rho }_{\mathrm{final}}}d\rho {\int }_{{\rho }_{\min }}^{\rho }d{\rho }^{\prime }\frac{exp[(F\left(\rho \right)-F\left({\rho }^{\prime }\right))/k_{B}T]}{D\left(\rho \right)},$$

(2)

where $F\left(\rho \right)$ is the free-energy as a function of $\rho ={\varphi }_{\mathrm{body}}$. ${\rho }_{\mathrm{final}}$ and ${\rho }_{\mathrm{initial}}$ are the values of ${\varphi }_{\mathrm{body}}$ at the free-energy minima, and ${\rho }_{\min }$ is the smallest accessible value of the coordinate. According to this calculation, the inferred timescale is estimated at ${10}^6{\tau }_{\mathrm{ru}}$. This is comparable to the apparent timescale (total simulated time divided by the number of observed transitions) of $\sim 1.8\times {10}^6{\tau }_{\mathrm{ru}}$. Agreement between the apparent and inferred timescales is consistent with $P(\mathrm{TP}$|${\varphi }_{\mathrm{body}})$ reaching the diffusion-limited value of 0.5. Interestingly, even when using intuitively-defined coordinates, there is no guarantee they will be able to accurately recapitulated the long-time kinetics. For example, in a recent study of SSU rotation in bacteria [26], it was shown that a similar coordinate is unable to capture the TSE, and the coordinate dramatically underestimates the height of the free-energy barrier. Fortunately, here, our analysis indicates that ${\varphi }_{\mathrm{body}}$ may be used to probe the factors that govern SSU rotation in a eukaryotic ribosome.

3.3. Simulations Implicate Millisecond-Scale Dynamics of Rotation

Since ${\varphi }_{\mathrm{body}}$ can be used to precisely describe the free-energy barrier for rotation, we next compared the predicted free-energy barrier with biological kinetics. For this, we use the expression:

$$1/\tau =Cexp(-\Delta F/k_{B}T),$$

(3)

where $\Delta F$ is the height of the free-energy barrier and C is the average barrier-crossing attempt frequency. The free-energy barrier along ${\varphi }_{\mathrm{body}}$ is $\Delta F\sim$6–7$k_{B}T$. Here, we used a barrier-crossing attempt frequency for rotation C that was previously estimated from explicit-solvent simulations [23]. This conversion between the free-energy barrier and kinetics implicates a mean first-passage time $\tau$ that is in the millisecond regime (1–5 ms). When interpreting this estimate of the timescale, it is important to recall that C was obtained for a bacterial ribosome, which is ∼70% the mass of the yeast ribosome. In accordance with Stokes-Einstein scaling for rotational diffusion, this would lead one to anticipate that rotational diffusion in yeast will be reduced by a factor of roughly 1.5. Further, since C is linearly proportional to the diffusion coefficient [23], the estimated timescale is likely underestimated by a comparable factor. Accordingly, a timescale of 1–5 ms represents a lower-bound on the mean first-passage time for SSU rotation in our model.

Even though the employed model is not intended to provide a complete description of ribosome energetics, the predicted timescale for SSU rotation is generally compatible with the rate of protein synthesis in the cell. The average rate of protein synthesis in yeast has been estimated to be approximately 10 amino acids per second [53], which imposes an upper limit of 100 ms on the timescale of any individual substep. However, there is no evidence that SSU rotation is rate limiting, which would be consistent with rotation kinetics occuring on substantially shorter timescales. In terms of energetics, this empirically-imposed upper bound on the timescale indicates that the barrier height in vivo is necessarily less than $\approx 10k_{B}T$ (estimated according to ref. [23]). In terms of modeling considerations, a difference in barrier height that is only a few $k_{B}T$ can easily be accounted for by minor changes in the energetic representation. For example, increasing the rotated and unrotated contact strength by ∼2% would increase the barrier by $\sim 4k_{B}T$. To make this estimate, we assume that most contacts will primarily stabilize the endpoint conformations. Since there are approximately 500 unique contacts in each endpoint, each of strength ∼0.2 reduced units (1 reduced energy unit is equal to 2$k_{B}T$), an increase of 2% would stabilize the endpoints by $4k_{B}T$. Since the mechanistic aspects of ribosome dynamics are often robust to parameters changes on this scale [54], we will consider the deviations between the predicted and in vivo upper-bound barrier heights to be minimal. Taken together, the compatibility of the timescales for elongation in vivo and the simulated rotation events suggests the model represents an appropriate first-order approximation to the energetics of subunit rotation.

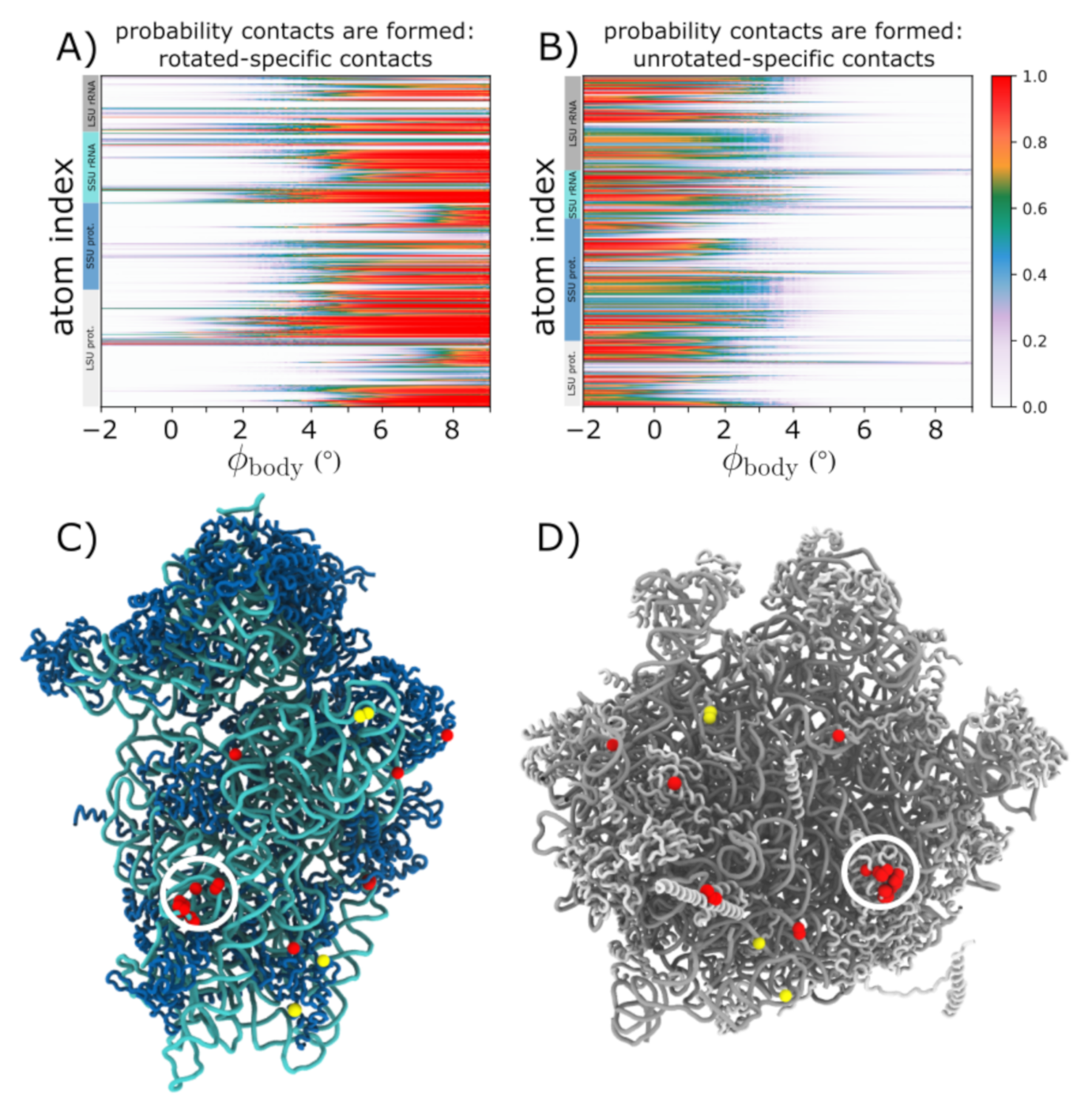
3.4. Asynchronous Dynamics of Subunit Bridge Interactions during Rotation

While applying rigid-body descriptions of biomolecular dynamics is often motivated by analogies with macroscopic systems, the scale of thermal energy in molecular systems gives rise to a fundamentally distinct relationship between structure and dynamics. In the cell, solvent introduces energetic fluctuations that are of the same scale ($k_{B}T$) as the interactions that maintain structural integrity. This leads to heterogeneous and anisotropic structural fluctuations [55] that can manifest in the form of large-scale global motions [22], as well as more localized distortions and partial unfolding [56,57]. In contrast, a corollary of rigid-body arguments would be that all intersubunit bridge interactions should simultaneously interconvert as the SSU rotates. However, such a process would likely be associated with a large free-energy barrier that would lead to prohibitively slow dynamics.

To better understand how molecular flexibility can facilitate collective rotation of the SSU, we used our simulated transitions to explore the ordering of intersubunit bridge rearrangements. Specifically, we calculated the probability that each rotated and unrotated contact is formed: $P_N^{ij}$. N refers to the conformation (rotated, or unrotated) and $ij$ denotes the atom pairs involved in a contact. Here, uniquely rotated/unrotated contacts are defined as atomic interactions that are present (i.e., proximal atoms) in only one of the conformations. Further, to be classified as “unique,” the atom pair must be substantially farther apart (see Methods) in the alternate conformation. We then calculated the average probability of all unique contacts that are defined with a specific atom: $\langle P_N^i\rangle =\frac{{\Sigma }_j{P}_N^{ij}}{N_i}$, where $N_i$ is the number of contacts that are defined with atom i. We then calculated $\langle P_N^i\rangle$ as a function of the rotation angle ${\varphi }_{\mathrm{body}}$, for both the rotated (Figure 4A) and unrotated (Figure 4B) contacts. Since the contact probabilities will be proportional to the stability imparted by a specific interaction, such analysis allows one to categorize interactions in terms of their contributions to the free-energy barrier and biological kinetics.

Contact analysis reveals how specific bridge interactions can have differential effects on the global kinetics of the system. We find that most unrotated contacts break early in the rotation process (Figure 4B), where almost no interactions are still formed by the time the ribosome reaches the TSE (${\varphi }_{\mathrm{body}}\sim 3.5^{\circ }$). This suggests that, while contacts that are unique to the unrotated conformation can contribute to the stability of the classical configuration, these interactions are unlikely to directly affect the free-energy barrier. In contrast, the rotated contacts form over a broad range of ${\varphi }_{\mathrm{body}}$ values, where some contacts are likely to form prior to the system reaching the TSE (Figure 4A).

To visually depict which interface regions are likely to have the strongest influence on the free-energy barrier, we identified all atoms that have at least one contact that is formed ($P_N^{ij}>0.8$) in the TSE. Consistent with Figure 4A,B, there are only four unrotated contacts that are likely to be formed (yellow spheres in Figure 4C,D). This is in sharp contrast with the dynamics of the rotated contacts, for which there are clusters of formed contacts scattered across the subunit interface. In particular, there is a dense cluster of formed contacts that are centered around bridge B8, which is formed between protein L23 and SSU helix 14. This suggests that, during rotation, the flexibility of the bridge allows it to “reach out” and form rotated contacts before the SSU body has fully transitioned to a rotated configuration. This finding suggests new ways in which experiments may modulate rotation kinetics in eukaryotic ribosomes. For example, it may be possible to introduce mutations to protein L23 that will specifically impact the rotated and TS ensembles, while leaving the stability of the classical configuration unperturbed. Together, these calculations provide a physical framework that can guide the development of next-generation experimental techniques that will be able to control biological dynamics.

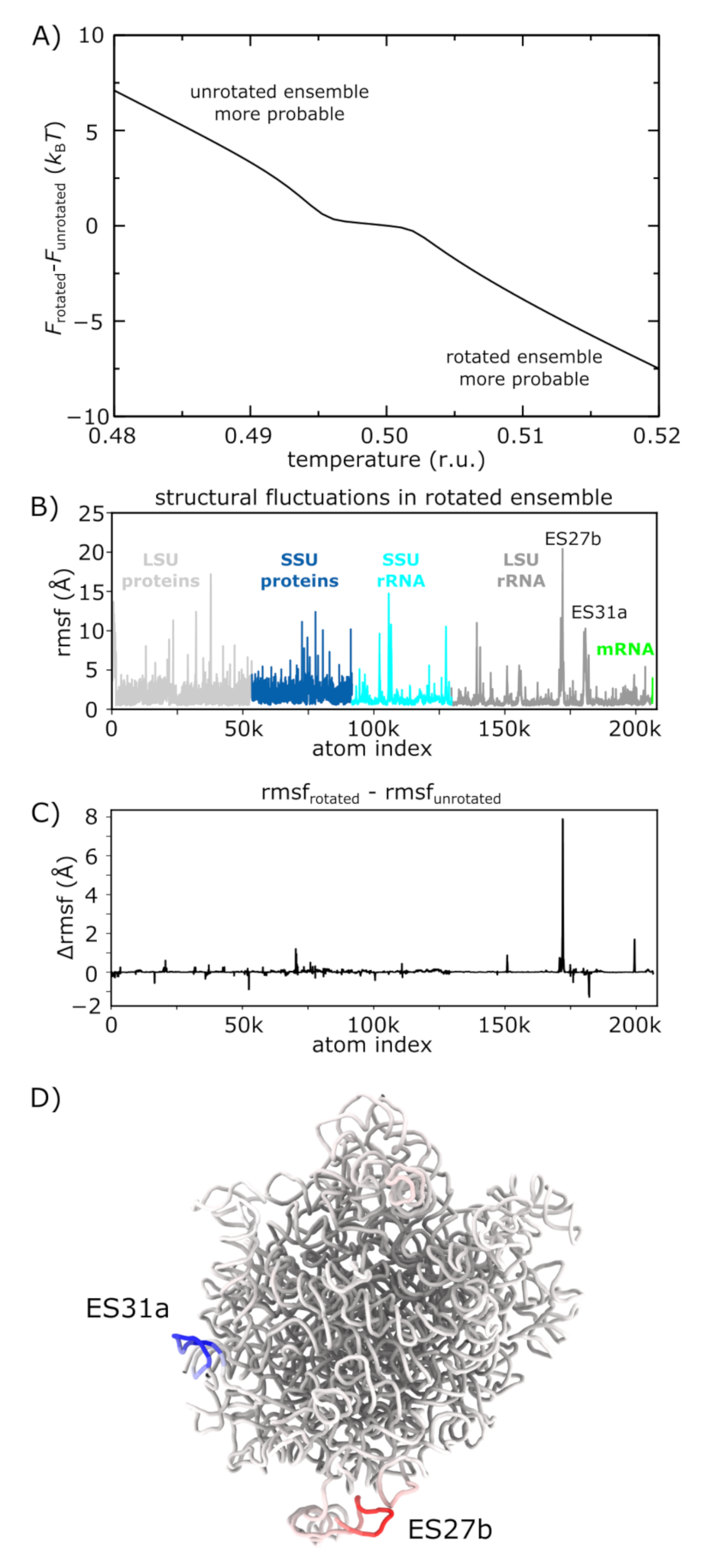
3.5. Molecular Flexibility Leads to Temperature-Induced Population Shift

In addition to characterizing the transition-state ensemble and the dynamics of SSU-LSU bridge interactions, we next asked whether the balance between rotated and unrotated ensembles is likely to be temperature-dependent. To explore this possibility, we calculated the relative free energy of the rotated and unrotated ensembles as a function of temperature. We find that increases in temperature are associated with increased stability of the rotated ensemble (Figure 5A). As described below, one can understand this effect as arising from an increase in mobility of a localized region within the LSU upon rotation.

To probe the temperature dependence of the energy landscape, we pursued a free-energy perturbation (FEP) approach. That is, we calculated the free-energy at arbitrary temperatures according to: $F\left({\varphi }_{\mathrm{body}}\right)=-k_{B}Tln\left(P^{\prime }\left({\varphi }_{\mathrm{body}}\right)\right)$, where $P^{\prime }\left({\varphi }_{\mathrm{body}}\right)$ represents a probability distribution for which each simulated frame was assigned a weight:

$$W=exp\left(-\left(\frac{1}{k_B{T}^{\prime }}-\frac{1}{k_B{T}_0}\right)U\right)$$

(4)

U is the potential energy of the simulated snapshot, $T_0$ is the temperature of the simulation, and $T^{\prime }$ is the temperature of interest. When $T_0=T^{\prime }$ (i.e., original simulated distribution), W reduces to 1. Before discussing the results obtained from the FEP calculations, it is important to explain the rationale and assumptions that are implicit in these types of methods. The first consideration is the scale of the simulations. That is, even though simulations with the simplified model are faster (many orders of magnitude) than simulations with explicit-solvent models, the size (>200,000 atoms) and effective timescale (milliseconds) remains very computationally demanding (∼10${}^{10}$ simulated timesteps, per simulation). Thus, it is not practical to obtain equilibrium sampling for a range of temperatures. The second consideration that supports the use of FEP is that the distributions calculated from different simulations were highly reproducible (not shown). This suggests the acquired sampling provides adequate coverage of the local phase space, which is necessary for FEP techniques to provide reliable estimates. The final consideration is that we are interested in temperature effects over a relatively small temperature range. That is, we consider a temperature range of $\pm 2\%$, where dramatic changes in the accessible conformations of the system are not likely to occur. This is an important point, since one may only extrapolate free energies using perturbation techniques if the perturbed parameter/s (i.e., temperature) would primarily redistribute the probabilities of well-sampled regions of phase space. Since the presented simulations were performed at a temperature for which only modest changes in flexibility are expected with this model [58], it is reasonable to assume that the accessible range of configurations will not be altered dramatically by small changes in temperature.

We find that the rotated ensemble is favored as temperature is increased (Figure 5A). For this, the rotated and unrotated ensembles were defined as $5.4<{\varphi }_{\mathrm{body}}<7.{86}^{\circ }$ and $-1.12<{\varphi }_{\mathrm{body}}<0.{82}^{\circ }$. The difference in free energy of the two ensembles was then defined as $\Delta F=F_{\mathrm{rot}}-F_{\mathrm{unrot}}=k_{B}Tln(P_{\mathrm{unrot}}/P_{\mathrm{rot}})$, where $F_N$ is the free energy of ensemble N. For the reported simulations, the free energy of the two ensembles was comparable (Figure 3D), where the relative stability of the rotated ensemble is found to increase with temperature. This suggests that differences in the configurational entropy of the rotated and unrotated ensembles can lead to a distinct temperature-dependence of the distribution between these states. Since the current model does not provide an explicit treatment of the solvent, this predicted trend is due solely to the contributions of the configurational entropy of the ribosome. Based on available data, it is not known whether solvation entropy will provide a significant contribution to this free-energy difference. If future experiments corroborate the observed trend, then one may infer that configurational entropy considerations are sufficient. If an opposite trend were found in experiments, this would suggest that solvation entropy is the dominant contributor. However, since the majority of the SSU-LSU interface is maintained in the rotated and unrotated states, it is reasonable to expect that solvation entropy changes will be minimal.

To provide a structural interpretation for the origins of the temperature-dependent distributions, we considered the flexibility of the ribosome in each ensemble. To assess the differential flexibility of the ribosome, we calculated the spatial root mean-squared fluctuations (rmsf) of each atom, where the average was calculated separately for the unrotated and rotated (Figure 5C) ensembles. In both cases, the rmsf values are highly heterogeneous, where the stalk regions are most flexible, along with some peripheral protein tails. To identify which regions of the structure contribute to the observed temperature-dependent dynamics, we calculated the difference in rmsf values $\Delta \mathrm{rmsf}={\mathrm{rmsf}}_{\mathrm{rot}}-{\mathrm{rmsf}}_{\mathrm{unrot}}$ for each atom (Figure 5D). This reveals that the differences in flexibility within the ensembles are primarily centered around a small region of H63 (expansion segment 27b, ES27b: red in Figure 5D). Interestingly, there is also one region in the LSU rRNA involved in the formation of a bridge interaction (ES31a in H79; blue in Figure 5D ) that exhibits a decrease in mobility, though the attenuation of mobility is of a smaller scale than the increase in mobility of ES27b.

In summary, as the ribosome rotates, the ES27b region can dissociate from the SSU interface and adopt a wide range of configurations. This increased mobility can then entropically drive the ribosome towards the rotated ensemble. At a qualitative level, this type of behavior of reminiscent of the notion of an entropic spring in polymer physics, where extension-associated reductions in entropy lead to an effective contractile force. There have also been similar examples in the study of protein conformational rearrangements, where increased mobility has been suggested to act as an entropic counterweight [59]. Accordingly, the current study helps elucidate the interplay between flexibility and dynamics in the ribosome, while revealing a common theme with simpler molecular systems.

4. Discussion

While structural methods can reveal atomic details of the ribosome at various stages of function, biological kinetics are controlled by energetics. Due to the complex character of ribosomal motions, directly quantifying the energetics through experiments has proven to be extremely difficult. Thus, there is need for a quantitative physical foundation, with which precise experimental measurements may be devised and executed. In the case of bacterial ribosomes, there are various single-molecule strategies available for studying large-scale conformational changes [12,14,15,60,61,62], where each can provide a unique perspective on the dynamics. To help integrate these complementary insights, molecular simulation represents a powerful approach for reconciling apparent differences within a common framework [26,63] and guiding the development of next-generation experiments. That is, rather than attempting to predict the exact behavior of a complex assembly, simulations may be used to establish broad trends and relationships. In regards to the current study, the presented simulations could be used to identify single-molecule probes that can precisely monitor rotation in eukaryotic ribosomes. These types of analyses can suggest experimental strategies that will be able to isolate the factors that control biological dynamics in the cell.

In the presented study, we illustrate how molecular simulations of a eukaryotic ribosome can provide initial insights into the relationship between molecular flexibility and kinetics. For example, we find that rotation is best described in terms of asynchronous motions that are facilitated by heterogeneous flexibility of the ribosome. As another example, we find that the flexibility of a specific helical region (ES27b) can increase upon rotation. This increase in mobility is accompanied by an increase in configurational entropy that can drive the rotation process. In terms of experiments, these predictions suggest that it may be possible to alter the dynamics of large-scale processes by introducing localized modifications to the ribosome (e.g., mutations, small-molecular binding) that impact flexibility. In future studies, it will be interesting to see the many ways in which flexibility can help orchestrate the dynamics of these assemblies.

With the ever-increasing availability of computational facilities, the ribosome is now becoming a model system for exploring theoretical concepts in biomolecular dynamics. That is, while performing a ribosome simulation used to represent a major technical accomplishment, the field is now entering a stage where the primary challenge is to craft pointed questions and deploy suitable models that can address them. This stage of development is reminiscent of the protein folding field in the late 1990s and early 2000s. At that time, there was an endless stream of proposed theoretical models, where each could be tested by applying simulation techniques. 20 years later, similar approaches are becoming possible for large assemblies, such as the ribosome. Building on current efforts, we anticipate that the continued integration of experiments, theoretical concepts and simulation techniques will allow for the identification of the precise molecular factors that control complex biomolecular assemblies.