Finite Element Analysis (FEA) for the Evaluation of Retention in a Conometric Connection for Implant and Prosthesis
Abstract
:1. Introduction
2. Materials and Methods
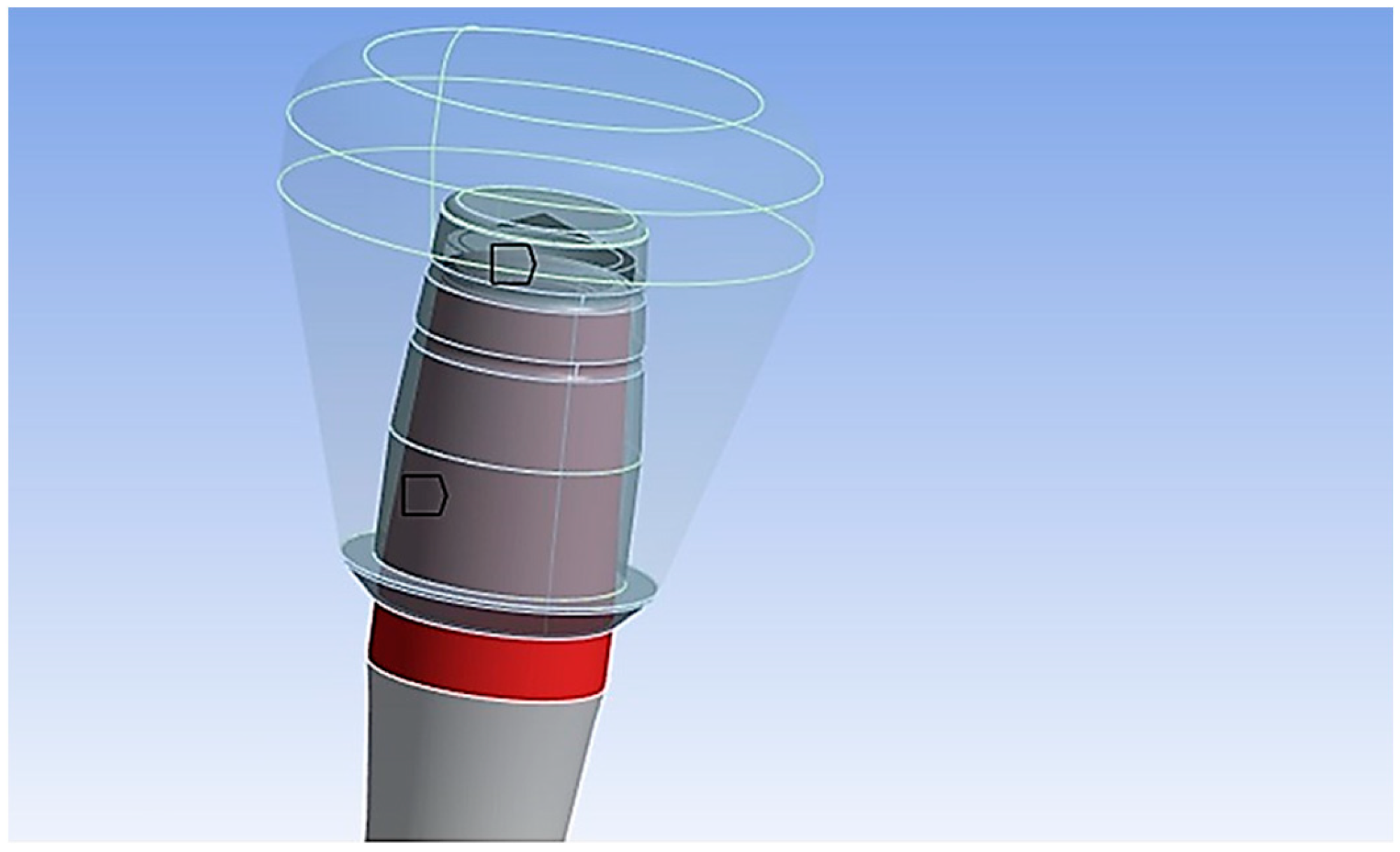
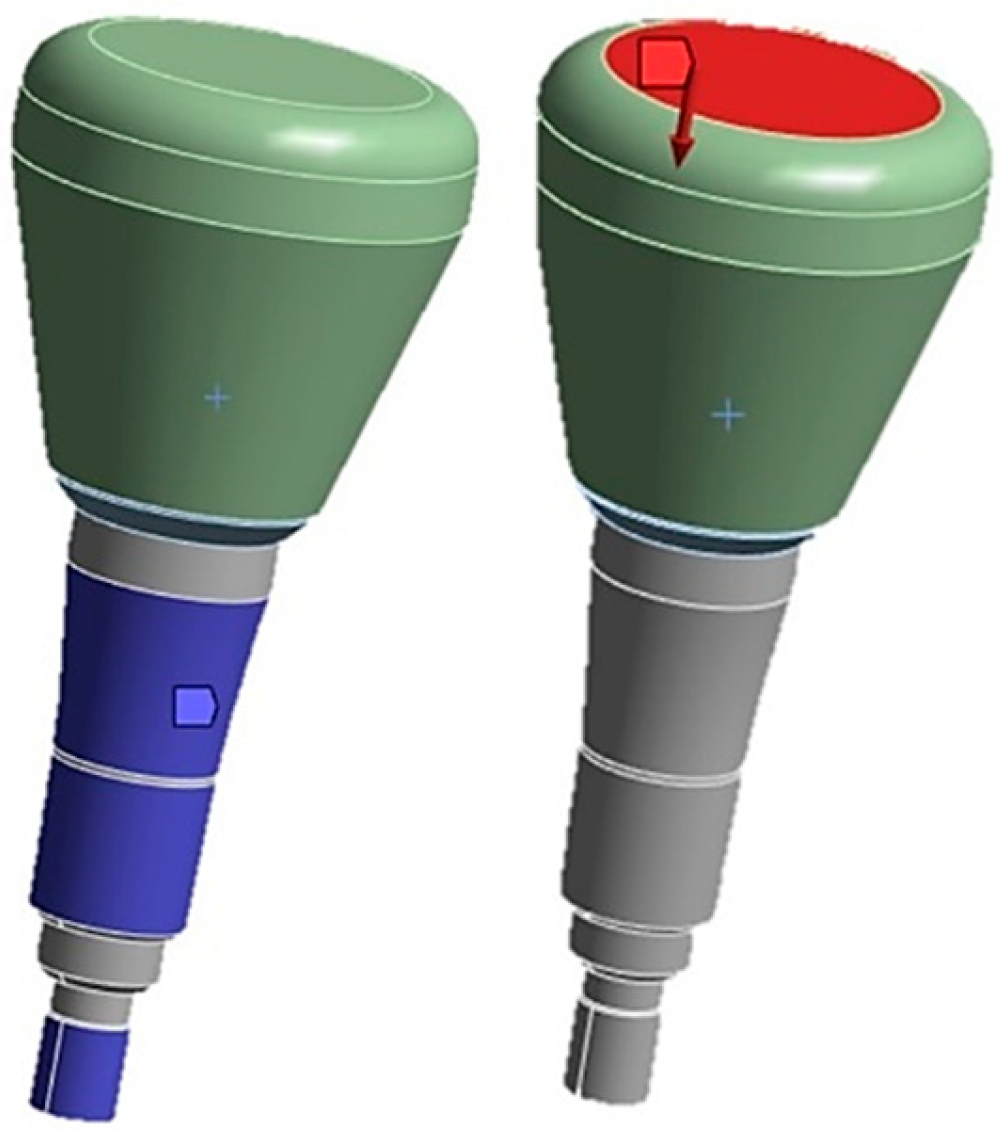
- Model creation: Start by digitally modeling the structure or part to be analyzed. This model is divided into smaller parts known as finite elements. Finite elements are simple geometric shapes, such as triangles or quadrilaterals in two dimensions 2D and tetrahedra or hexahedra in three dimensions 3D.
- Definition of material properties: Each finite element is assigned material properties, including Young’s modulus, Poisson’s coefficient, strength, and other characteristics depending on the material of the part.
- Application of loads: Define loads, such as forces, moments, pressures, constraints, and boundary conditions, to simulate the real environment in which the structure operates.
- Discretization: The model is divided into finite elements, and the nodes of these elements are assigned unknown variables like displacements, stresses, or other relevant quantities. Subsequently, the load/constraint conditions are assigned, and the results are analyzed.
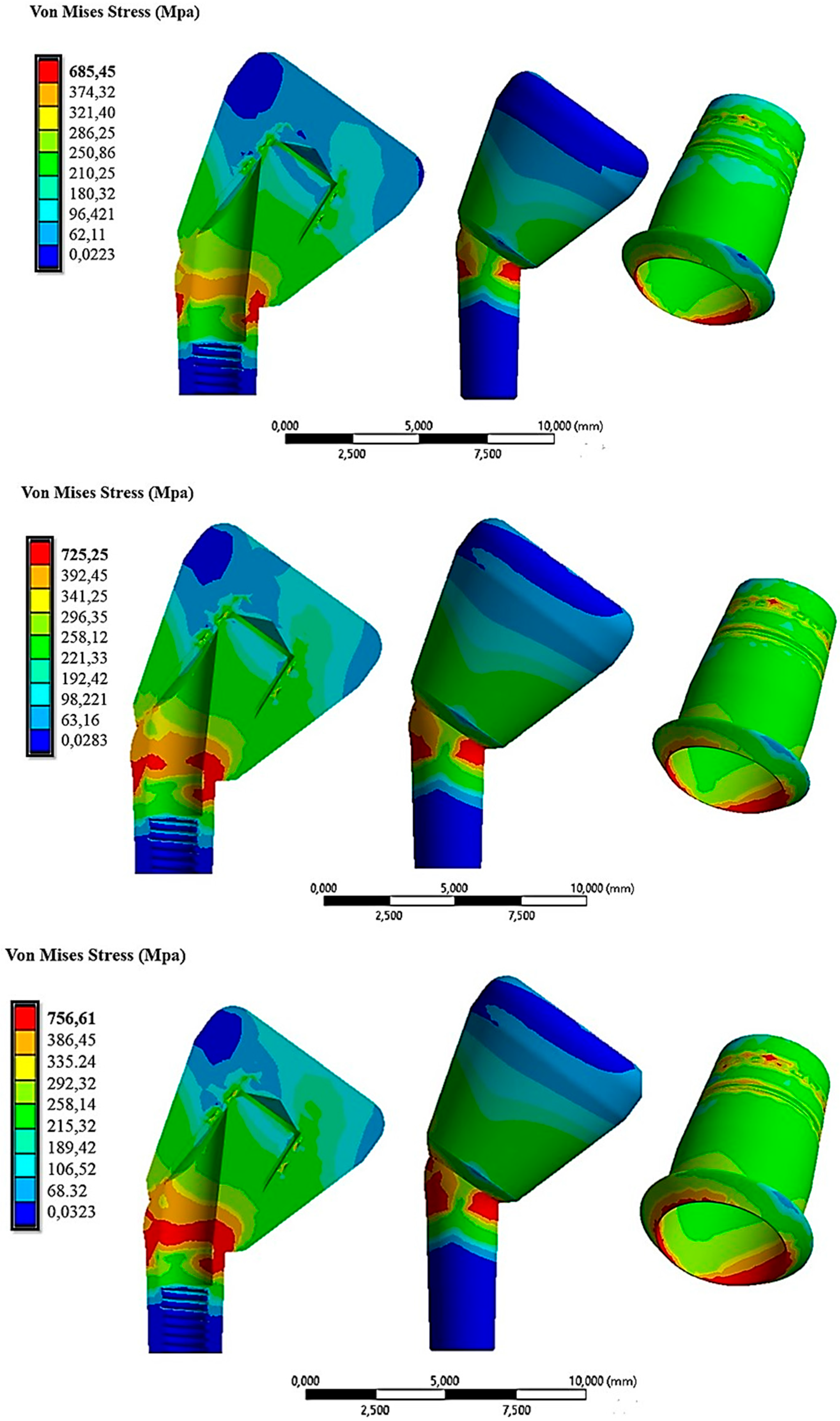
3. Results
4. Discussion
5. Conclusions
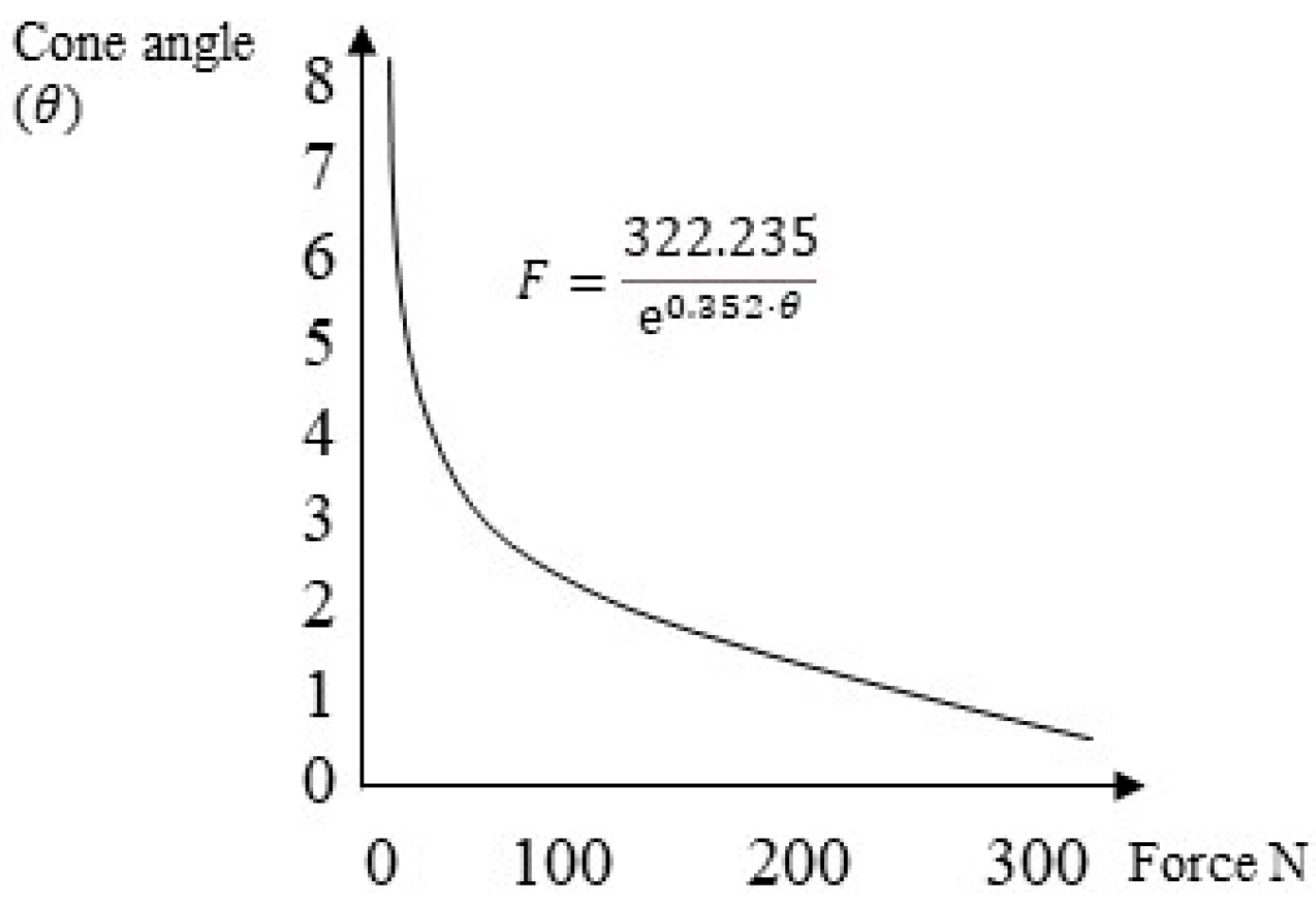
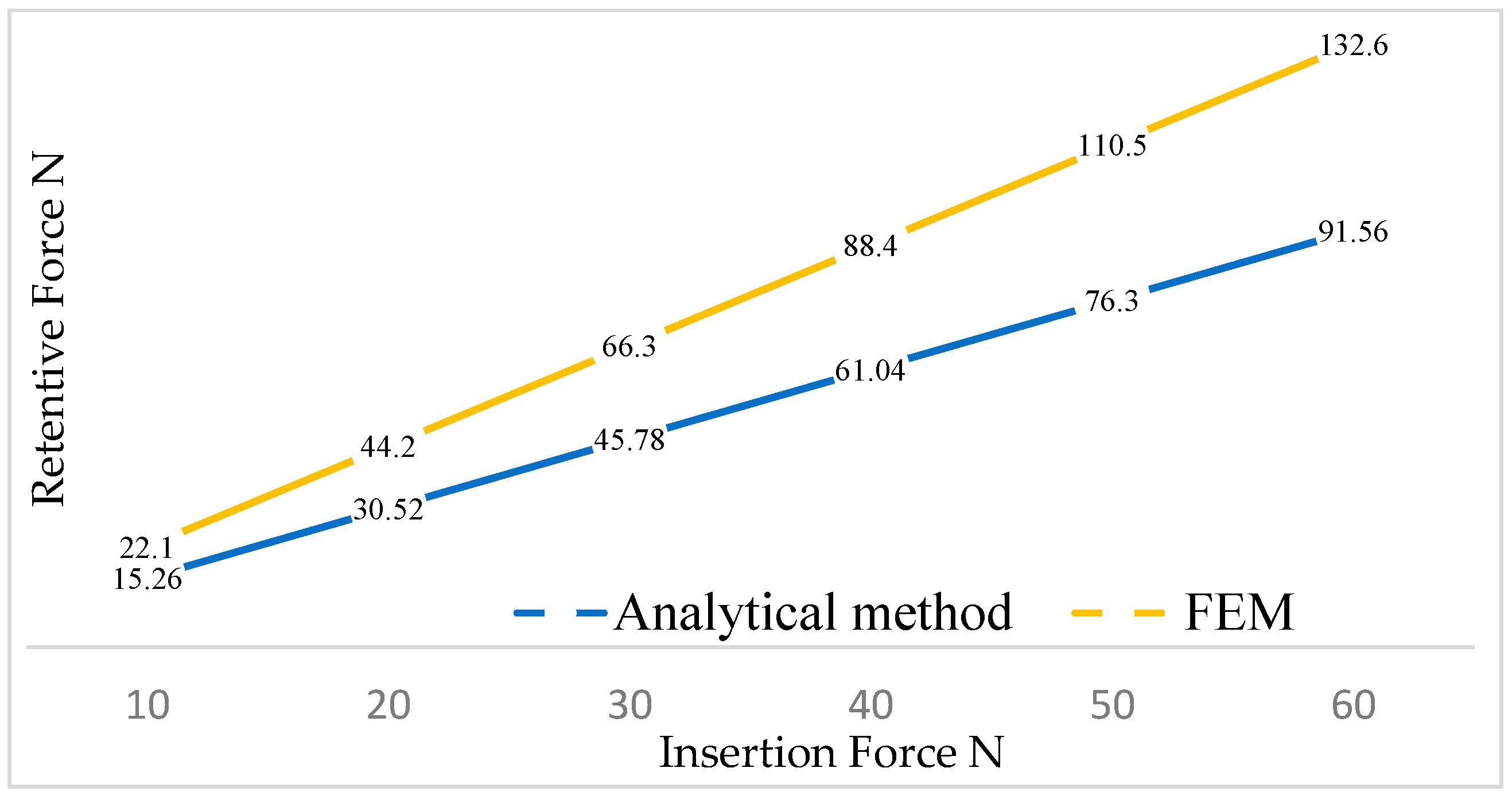
- There existed a linear relationship between the cap activation force and retentive force.
- The analytical method proposed by Bozkaya and Muftu [19] underestimated the system retention.
- The FEA method demonstrated comparable results with in vitro studies. For a connection with a 4° conicity, the retentive force obtained from in vitro studies was 68 N, while with FEA, it was 66 N.
- The force required to activate the connection in the case of a 4° taper was approximately 30 N. Values below 20 N of activation force did not guarantee the required retention.
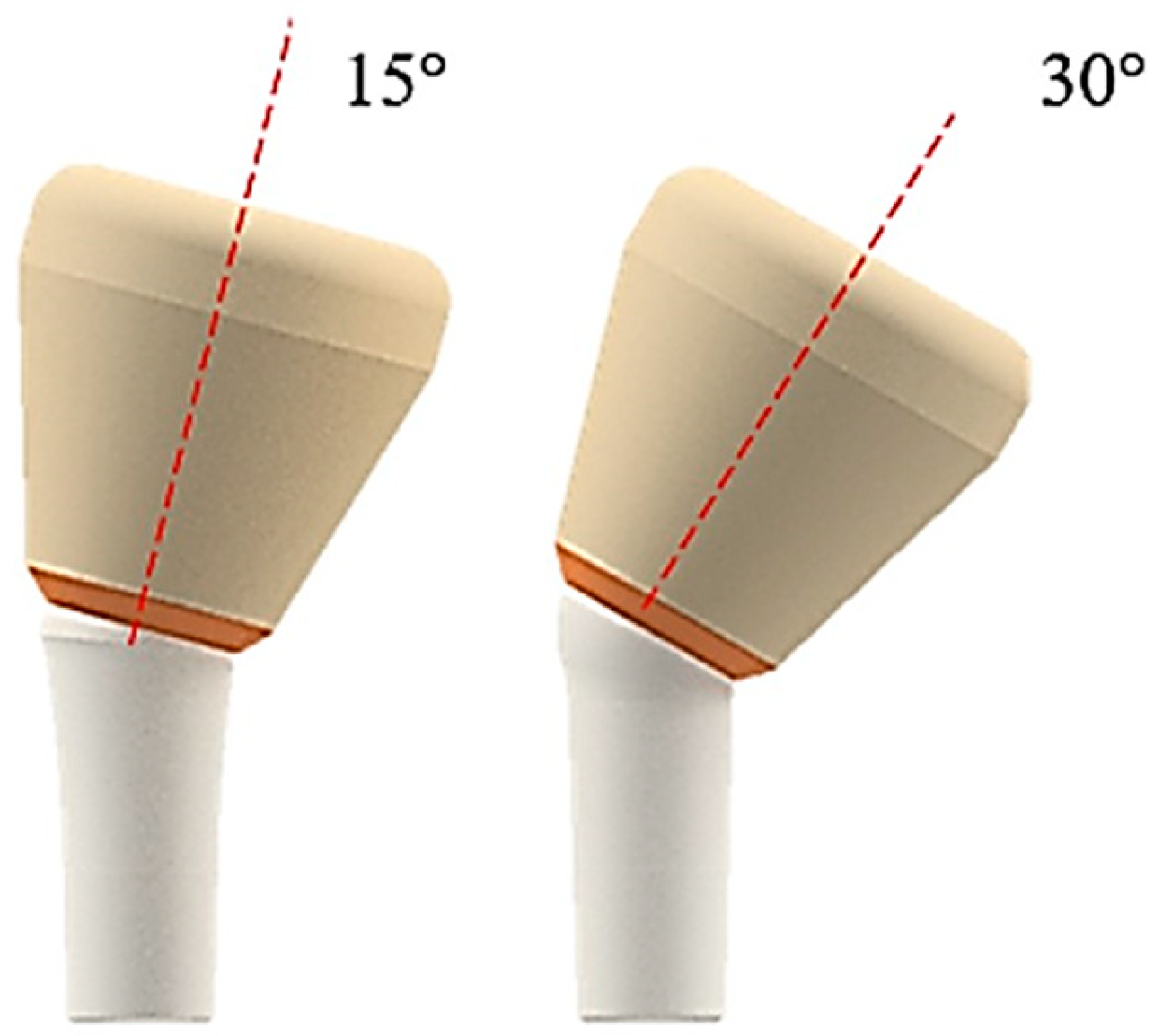
- The inclination of the abutment decreased the retention of the system. To counteract this effect, it was necessary to increase the activation force by 10 N for abutments inclined between 15° and 30°.
- The state of stress acting on the system was greater in the case of inclined abutments.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Heydecke, G.; Thomason, J.M.; Awad, M.A.; Lund, J.P.; Feine, J.S. Do mandibular implant overdentures and conventional complete dentures meet the expectations of edentulous patients? Quintessence Int. 2008, 39, 803–809. [Google Scholar] [PubMed]
- Musacchio, E.; Perissinotto, E.; Binotto, P.; Sartori, L.; Silva-Netto, F.; Zambon, S.; Manzato, E.; Chiara Corti, M.; Baggio, G.; Crepaldi, G. Tooth loss in the elderly and its association with nutritional status, socio-economic and lifestyle factors. Acta Odontol. Scand. 2007, 65, 78–86. [Google Scholar] [CrossRef]
- Yadav, R.; Lee, H.; Lee, J.H.; Singh, R.K.; Lee, H.H. A comprehensive review: Physical, mechanical, and tribological characterization of dental resin composite materials. Tribol. Int. 2023, 179, 108102. [Google Scholar] [CrossRef]
- Yadav, R.; Meena, A.; Patnaik, A. Biomaterials for dental composite applications: A comprehensive review of physical, chemical, mechanical, thermal, tribological, and biological properties. Polym. Adv. Technol. 2022, 33, 1762–1781. [Google Scholar] [CrossRef]
- Michalakis, K.X.; Hirayama, H.; Garefis, P.D. Cement-Retained versus Screw-Retained Implant Restorations: A Critical Review. Int. J. Oral Maxillofac. Implant. 2003, 18, 719–728. [Google Scholar]
- Wilson, T.G., Jr. The Positive Relationship between Excess Cement and Peri-Implant Disease: A Prospective Clinical Endoscopic Study. J. Periodontol. 2009, 80, 1388–1392. [Google Scholar] [CrossRef] [PubMed]
- Salvi, G.E.; Cosgarea, R.; Sculean, A. Prevalence and Mechanisms of Peri-Implant Diseases. J. Dent. Res. 2017, 96, 31–37. [Google Scholar] [CrossRef]
- Bornstein, M.M.; Lussi, A.; Schmid, B.; Belser, U.C.; Buser, D. Early Loading of Nonsubmerged Titanium Implants with a Sandblasted and Acid-Etched (SLA) Surface: 3-Year Results of a Prospective Study in Partially Edentulous Patients. Int. J. Oral Maxillofac. Implant. 2003, 18, 659–666. [Google Scholar]
- Abboud, M.; Koeck, B.; Stark, H.; Wahl, G.; Paillon, R. Immediate Loading of Single-Tooth Implants in the Posterior Region. Int. J. Oral Maxillofac. Implant. 2005, 20, 61–68. [Google Scholar] [CrossRef]
- Tan, B.; Gillam, D.G.; Mordan, N.J.; Galgut, P.N. A Preliminary Investigation into the Ultrastructure of Dental Calculus and Associated Bacteria. J. Clin. Periodontol. 2004, 31, 364–369. [Google Scholar] [CrossRef]
- Schedle, A.; Franz, A.; Rausch-Fan, X.; Andreas, S.; Lucas, T.; Samorapoompichit, P.; Sperr, W.; Boltz-Nitulescu, G. Cytotoxic Effects of Dental Composites, Adhesive Substances, Compomers and Cements. Dent. Mater. 1998, 14, 429–440. [Google Scholar] [CrossRef] [PubMed]
- Agar, J.R.; Cameron, S.M.; Hughbanks, J.C.; Parker, M.H. Cement Removal from Restorations Luted to Titanium Abutments with Simulated Subgingival Margins. J. Prosthet. Dent. 1997, 78, 43–47. [Google Scholar] [CrossRef] [PubMed]
- Gehrke, P.; Hartjen, P.; Smeets, R.; Gosau, M.; Peters, U.; Beikler, T.; Fischer, C.; Stolzer, C.; Geis-Gerstorfer, J.; Weigl, P.; et al. Marginal Adaptation and Microbial Leakage at Conometric Prosthetic Connections for Implant-Supported Single Crowns: An In Vitro Investigation. Int. J. Mol. Sci. 2021, 22, 881. [Google Scholar] [CrossRef] [PubMed]
- Albrektsson, T.; Canullo, L.; Cochran, D.; De Bruyn, H. “Peri-Implantitis”: A Complication of a Foreign Body or a Man-Made “Disease”. Facts and Fiction. Clin. Implant Dent. Relat. Res. 2016, 18, 840–849. [Google Scholar] [CrossRef] [PubMed]
- Goiato, M.C.; Pellizzer, E.P.; da Silva, E.V.F.; da Rocha Bonatto, L.; dos Santos, D.M. Is the internal connection more efficient than external connection in mechanical, biological, and esthetical point of views? A systematic review. Oral Maxillofac. Surg. 2015, 19, 229–242. [Google Scholar] [CrossRef]
- Macedo, J.P.; Pereira, J.; Vahey, B.R.; Henriques, B.; Benfatti, C.A.M.; Magini, R.S.; López-López, J.; Souza, J.C.M. Morse taper dental implants and platform switching: The new paradigm in oral implantology. Eur. J. Dent. 2016, 10, 148–154. [Google Scholar] [CrossRef]
- Vinhas, A.S.; Aroso, C.; Salazar, F.; López-Jarana, P.; Ríos-Santos, J.V.; Herrero-Climent, M. Review of the Mechanical Behavior of Different Implant-Abutment Connections. Int. J. Environ. Res. Public Health 2020, 17, 8685. [Google Scholar] [CrossRef]
- Di Pietro, N.; Ceddia, M.; Romasco, T.; De Bortoli Junior, N.; Mello, B.F.; Tumedei, M.; Specchiulli, A.; Piattelli, A.; Trentadue, B. Finite Element Analysis (FEA) of the Stress and Strain Distribution in Cone-Morse Implant—Abutment Connection Implants Placed Equicrestally and Subcrestally. Appl. Sci. 2023, 13, 8147. [Google Scholar] [CrossRef]
- D’Ercole, S.; Dotta, T.C.; Iezzi, G.; Cipollina, A.; Pedrazzi, V.; Piattelli, A.; Petrini, M. Static Bacterial Leakage in Different Conometric Connections: An In Vitro Study. Appl. Sci. 2023, 13, 2693. [Google Scholar] [CrossRef]
- Albiero, A.M.; Benato, R.; Momic, S.; Degidi, M. Guided-Welded Approach Planning Using a Computer-Aided Designed Prosthetic Shell for Immediately Loaded Complete-Arch Rehabilitations Supported by Conometric Abutments. J. Prosthet. Dent. 2019, 122, 510–515. [Google Scholar] [CrossRef]
- Nardi, D.; Degidi, M.; Sighinolfi, G.; Tebbel, F.; Marchetti, C. Retention Strength of Conical Welding Caps for Fixed Implant Supported Prostheses. Int. J. Prosthodont. 2017, 30, 553–555. [Google Scholar] [CrossRef] [PubMed]
- Bozkaya, D.; Müftü, S. Efficiency considerations for the purely tapered interference fit (TIF) abutments used in dental implants. J. Biomech. Eng. 2004, 126, 393–401. [Google Scholar] [CrossRef] [PubMed]
- Prisco, R.; Troiano, G.; Laino, L.; Zhurakivska, K. Rotational tolerances of a titanium abutment in the as-received condition and after screw tightening in a conical implant connection. J. Adv. Prosthodont. 2021, 13, 343–350. [Google Scholar] [CrossRef] [PubMed]
- Aguirrebeitia, J.; Abasolo, M.; Müftü, S.; Vallejo, J. Influence of design and clinical factors on the removal force ratio in tapered implant-abutment interfaces. J. Prosthet. Dent. 2017, 117, 493–498. [Google Scholar] [CrossRef] [PubMed]
- Tuna, S.H.; Al-Chalabi, Z.S.; Kozak, E. Evaluation of the Effects of Repeated Insertion-Removal Cycles on the Retention of an Indexed Conometric Connection. Int. J. Oral Maxillofac. Implant. 2022, 37, 549–555. [Google Scholar] [CrossRef] [PubMed]
- Antonaya-Martin, J.; Del Rio-Highsmith, J.; Moreno-Hay, I.; Lillo-Rodríguez, J.; Gomez-Polo, M.; Celemin-Viñuela, A. CAD/CAM Conic Crowns for Predictable Retention in Implant-Supported Prostheses. Int. J. Prosthodont. 2016, 29, 230–232. [Google Scholar] [CrossRef] [PubMed]
- Brunski, J.B. In vivo bone response to biomechanical loading at the bone/dental-implant interface. Adv. Dent. Res. 1999, 13, 99–119. [Google Scholar] [CrossRef] [PubMed]
- Lanza, A.; Ruggiero, A.; Sbordone, L. Tribology and dentistry: A commentary. Lubricants 2019, 7, 52. [Google Scholar] [CrossRef]
- Cipollina, A.; Ceddia, M.; Di Pietro, N.; Inchingolo, F.; Tumedei, M.; Romasco, T.; Piattelli, A.; Specchiulli, A.; Trentadue, B. Finite Element Analysis (FEA) of a Premaxillary Device: A New Type of Subperiosteal Implant to Treat Severe Atrophy of the Maxilla. Biomimetics 2023, 8, 336. [Google Scholar] [CrossRef]
- Lee, J.H.; Jang, H.Y.; Lee, S.Y. Finite Element Analysis of Dental Implants with Zirconia Crown Restorations: Conventional Cement-Retained vs. Cementless Screw-Retained. Materials 2021, 14, 2666. [Google Scholar] [CrossRef]
- Kapos, T.; Evans, C. CAD/CAM technology for implant abutments, crowns, and superstructures. Int. J. Oral Maxillofac. Implant. 2014, 29, 117–136. [Google Scholar] [CrossRef] [PubMed]
- Ochiai, K.T.; Ozawa, S.; Caputo, A.A.; Nishimura, R.D. Photoelastic stress analysis of implant-tooth connected prostheses with segmented and nonsegmented abutments. J. Prosthet. Dent. 2003, 89, 495–502. [Google Scholar] [CrossRef] [PubMed]
- Aalaei, S.; Rajabi Naraki, Z.; Nematollahi, F.; Beyabanaki, E.; Shahrokhi Rad, A. Stress distribution pattern of screw-retained restorations with segmented vs. non-segmented abutments: A finite element analysis. J. Dent. Res. Dent. Clin. Dent. Prospect. 2017, 11, 149–155. [Google Scholar] [CrossRef] [PubMed]
- Sato, Y.; Shindoi, N.; Hosokawa, R.; Tsuga, K.; Akagawa, Y. A biomechanical effect of wide implant placement and offset placement of three implants in the posterior partially eden-tulous region. J. Oral Rehabil. 2000, 27, 15–21. [Google Scholar] [CrossRef] [PubMed]
- Gehrke, P.; Fischer, C.; Weinhold, O.; Dhom, G. Das konometrische Konzept für implantatgetragene Einzelkronen: Die definitive Befestigung ohne Zement oder Schrauben. ZWR—Das Dtsch. Zahnärzteblatt 2021, 130, 85–92. [Google Scholar] [CrossRef]
- Canullo, L.; Peñarrocha, M.; Monje, A.; Catena, A.; Wang, H.-L.; Peñarrocha, D. Association Between Clinical and Microbiologic Cluster Profiles and Peri-Implantitis. Int. J. Oral Maxillofac. Implant. 2017, 32, 1054–1064. [Google Scholar] [CrossRef] [PubMed]
- Caricasulo, R.; Malchiodi, L.; Ghensi, P.; Fantozzi, G.; Cucchi, A. The Influence of Implant-Abutment Connection to Peri-Implant Bone Loss: A Systematic Review and Meta-Analysis. Clin. Implant Dent. Relat. Res. 2018, 20, 653–664. [Google Scholar] [CrossRef]
- Gehrke, P.; Burg, S.; Peters, U.; Beikler, T.; Fischer, C.; Rupp, F.; Schweizer, E.; Weigl, P.; Sader, R.; Smeets, R.; et al. Bacterial translocation and microgap formation at a novel conical indexed implant abutment system for single crowns. Clin. Oral Investig. 2022, 26, 1375–1389. [Google Scholar] [CrossRef]
- Gehrke, P.; Bleuel, K.; Fischer, C.; Sader, R. Influence of margin location and luting material on the amount of undetected cement excess on CAD/CAM implant abutments and cement-retained zirconia crowns: An in-vitro study. BMC Oral Health 2019, 19, 111. [Google Scholar] [CrossRef]
- Degidi, M.; Nardi, D.; Sighinolfi, G.; Degidi, D. The conometric concept for the definitive rehabilitation of a single posterior implant by using a conical indexed abutment: A technique. J. Prosthet. Dent. 2020, 123, 576–579. [Google Scholar] [CrossRef]
- Degidi, M.; Nardi, D.; Piattelli, A. The Conometric Concept: Coupling Connection for Immediately Loaded Titanium-Reinforced Provisional Fixed Partial Dentures—A Case Series. Int. J. Periodontics Restor. Dent. 2016, 36, 347–354. [Google Scholar] [CrossRef]
- Degidi, M.; Nardi, D.; Sighinolfi, G.; Degidi, D.; Piattelli, A. The Conometric Concept: A two-year follow-up of fixed partial cerec restorations supported by cone-in-cone abutments. J. Prosthodont. 2019, 28, 780–787. [Google Scholar] [CrossRef] [PubMed]
- Lian, M.; Zhao, K.; Feng, Y.; Yao, Q. Prognosis of Combining Remaining Teeth and Implants in Double-Crown-Retained Removable Dental Prostheses: A Systematic Review and Meta-Analysis. Int. J. Oral Maxillofac. Implant. 2018, 33, 281–297. [Google Scholar] [CrossRef] [PubMed]
- Stefano, M.D.; Ruggiero, A. Real contact area and friction: An overview of different approaches. In Industrial Tribology; CRC Press: Boca Raton, FL, USA, 2022; pp. 1–23. [Google Scholar]
- Beuer, F.; Edelhoff, D.; Gernet, W.; Naumann, M. Parameters Affecting Retentive Force of Electroformed Double-Crown Systems. Clin. Oral Investig. 2010, 14, 129–135. [Google Scholar] [CrossRef] [PubMed]
- Zhang, R.-G.; Hannak, W.B.; Roggensack, M.; Freesmeyer, W.B. Retentive Characteristics of Ankylos SynCone Conical Crown System over Long-Term Use in Vitro. Eur. J. Prosthodont. Restor. Dent. 2008, 16, 61–66. [Google Scholar]
- Mangano, F.; Macchi, A.; Caprioglio, A.; Sammons, R.L.; Piattelli, A.; Mangano, C. Survival and complication rates of fixed restorations supported by locking-taper implants: A prospective study with 1 to 10 years of follow-up. J. Prosthodont. 2014, 23, 434–444. [Google Scholar] [CrossRef]
- Kubo, K.; Koike, T.; Ueda, T.; Sakurai, K. Influence of the mechanical properties of resilient denture liners on the retention of overdenture attachments. J. Prosthet. Dent. 2018, 120, 431–438. [Google Scholar] [CrossRef]
- Türk, P.E.; Geckili, O.; Türk, Y.; Günay, V.; Bilgin, T. In vitro comparison of the retentive properties of ball and locator attachments for implant overdentures. Int. J. Oral Maxillofac. Implant. 2014, 29, 1106–1113. [Google Scholar] [CrossRef]
- Al-Chalabi, Z.S.; Tuna, H.S. The effect of thermomechanical aging on the retention of a conometric system in a chewing simulator. J. Prosthodont. 2023, 1–8. [Google Scholar] [CrossRef]
- Ghodsi, S.; Zeighami, S.; Meisami, A.M. Comparing Retention and Internal Adaptation of Different Implant-Supported, Metal-Free Frameworks. Int. J. Prosthodont. 2018, 31, 475–477. [Google Scholar] [CrossRef]
- Sannino, G.; Barlattani, A. Mechanical evaluation of an implant-abutment self-locking taper connection: Finite element analysis and experimental tests. Int. J. Oral Maxillofac. Implant. 2013, 28, e17–e26. [Google Scholar] [CrossRef] [PubMed]
- Reddy, M.S.; Sundram, R.; Eid Abdemagyd, H.A. Application of Finite Element Model in Implant Dentistry: A Systematic Review. J. Pharm. Bioallied Sci. 2019, 11 (Suppl. 2), S85–S91. [Google Scholar] [CrossRef] [PubMed]
- Baggi, L.; Di Girolamo, M.; Vairo, G.; Sannino, G. Comparative evaluation of osseointegrated dental implants based on platform-switching concept: Influence of diameter, length, thread shape, and in-bone positioning depth on stress-based performance. Comput. Math. Methods Med. 2013, 2013, 250929. [Google Scholar] [CrossRef] [PubMed]
- De Carvalho, N.A.; de Almeida, E.O.; Rocha, E.P.; Freitas, A.C., Jr.; Anchieta, R.B.; Kina, S. Short implant to support maxillary restorations: Bone stress analysis using regular and switching platform. J. Craniofac. Surg. 2012, 23, 678–681. [Google Scholar] [CrossRef] [PubMed]
- Mohanty, A.K.; Varghese, T.; Bhushan, P.; Mahapatro, R.K.; Kashi, A.B.; Kariyatty, P. Influence of Occlusal Stress on Implant Abutment Junction and Implant Bone Interface: A Finite Element Analysis Study. J. Contemp. Dent. Pract. 2022, 23, 1190–1194. [Google Scholar] [PubMed]
- Bressan, E.; Lops, D.; Tomasi, C.; Ricci, S.; Stocchero, M.; Carniel, E.L. Experimental and computational investigation of Morse taper conometric system reliability for the definition of fixed connections between dental implants and prostheses. Proceedings of the Institution of Mechanical Engineers. Part H. J. Eng. Med. 2014, 228, 674–681. [Google Scholar] [CrossRef]
- Bressan, E.; Lops, D. Conometric retention for complete fixed prosthesis supported by four implants: 2-years prospective study. Clin. Oral Implant. Res. 2014, 25, 546–552. [Google Scholar] [CrossRef]
- Schriwer, C.; Skjold, A.; Gjerdet, N.R.; Øilo, M. Monolithic Zirconia Dental Crowns. Internal Fit, Margin Quality, Fracture Mode and Load at Fracture. Dent. Mater. 2017, 33, 1012–1020. [Google Scholar] [CrossRef]
- Howes, D. Angled Implant Design to Accommodate Screw-retained Implant-supported Prostheses. Compend. Contin. Educ. Dent. 2017, 38, 458–463. [Google Scholar]
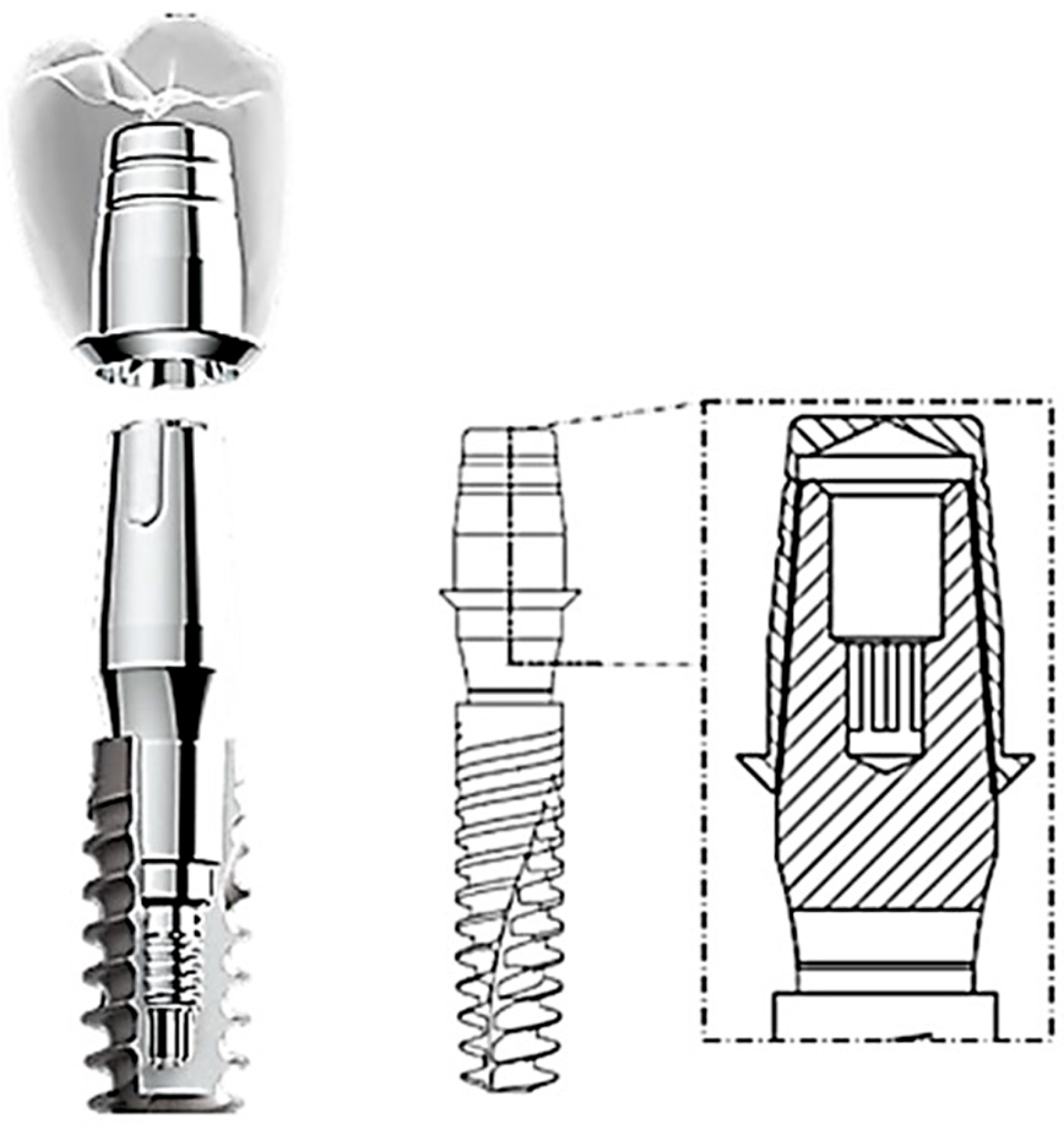

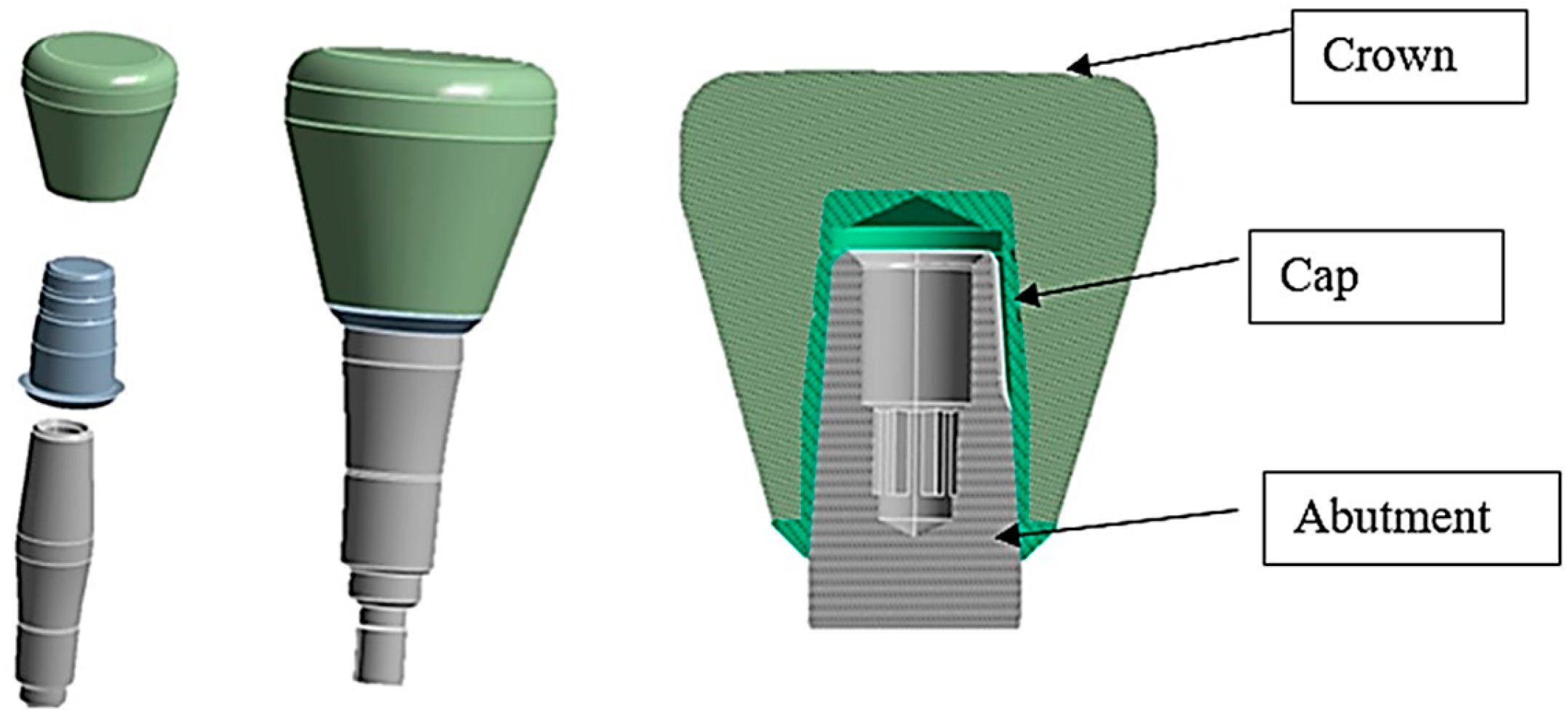
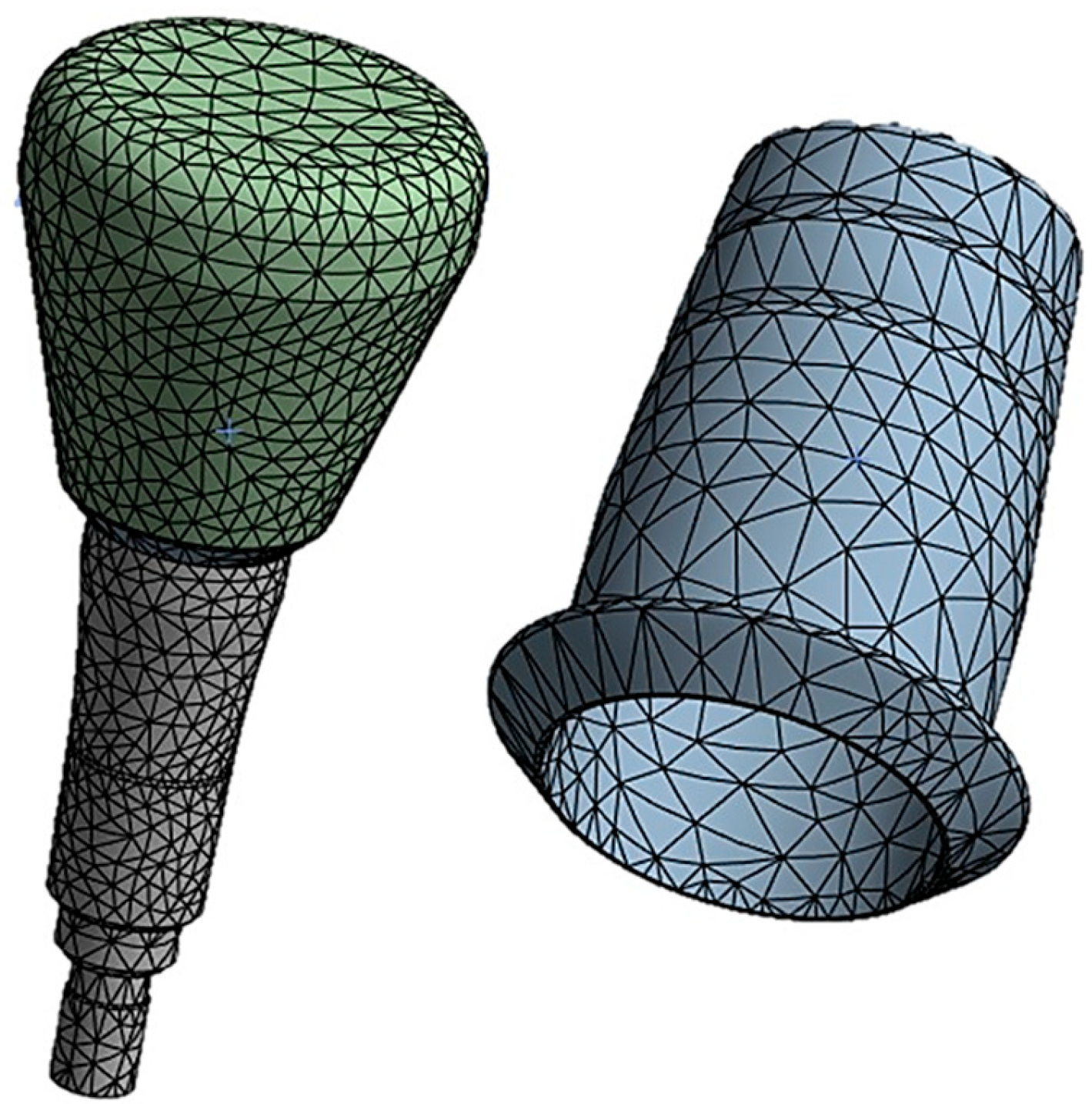
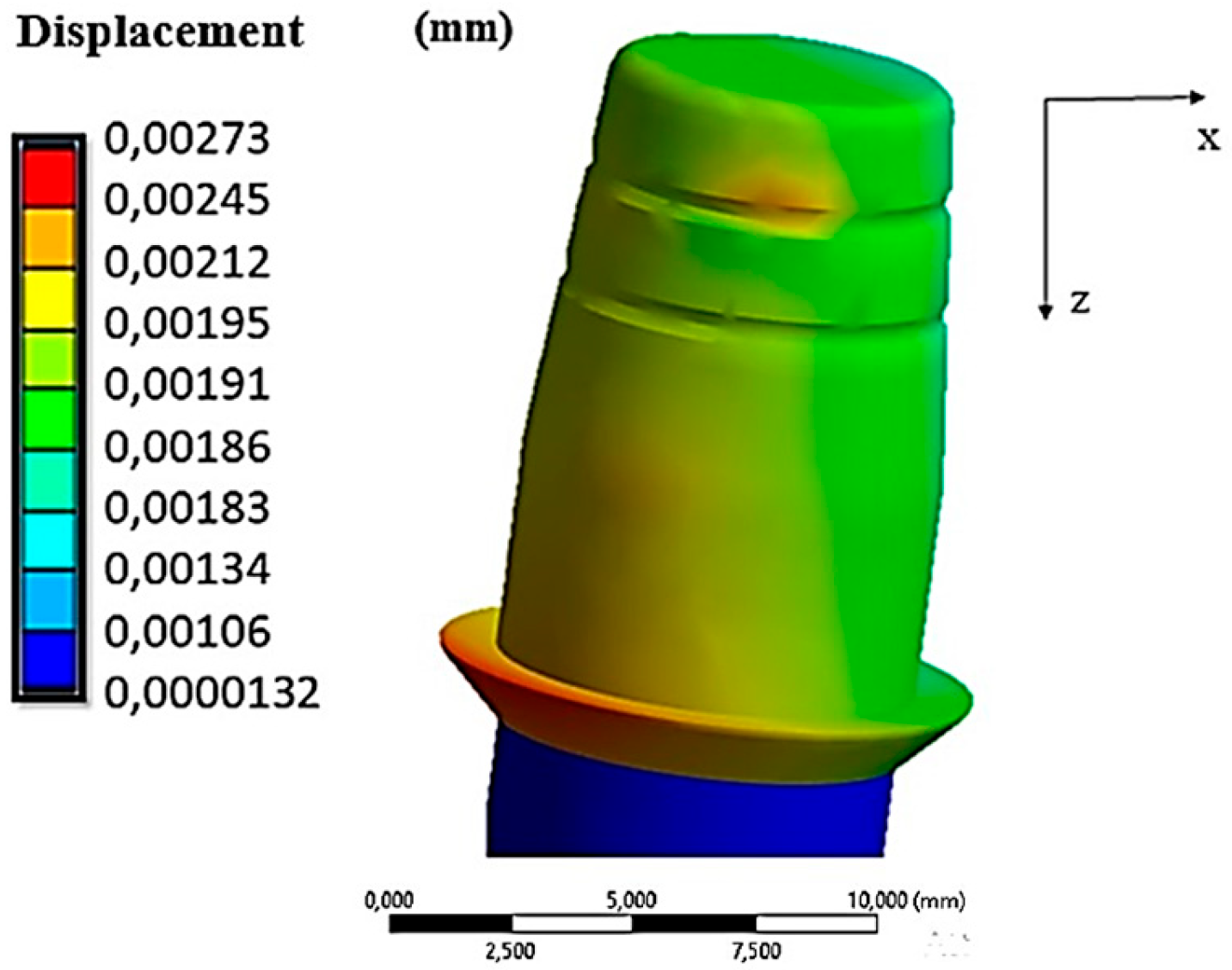
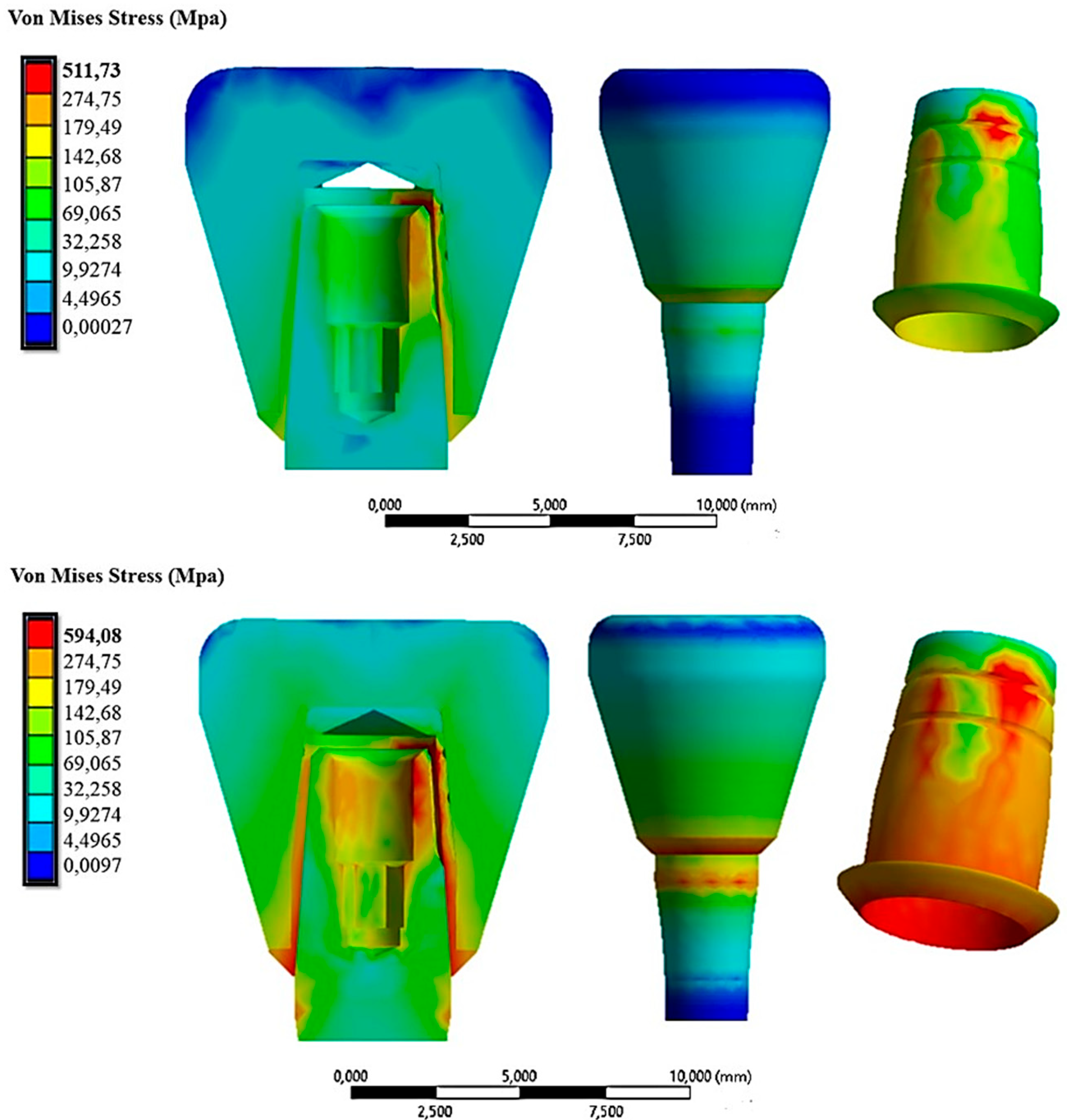

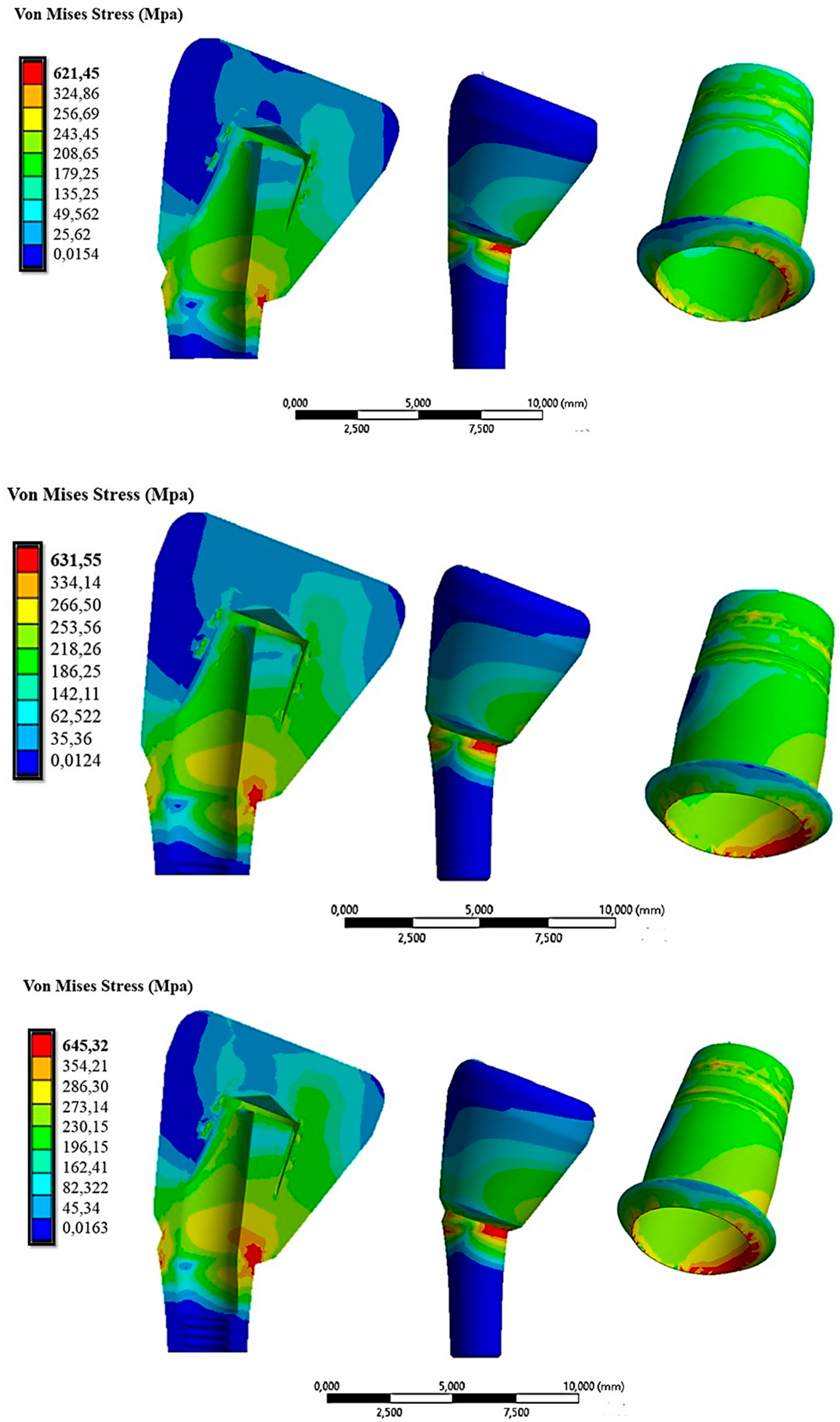

| Young’s Modulus (GPa) | Poisson’s Ratio | Tensile Yield Strength (MPa) | Tensile Ultimate Strength (MPa) | |
|---|---|---|---|---|
| Titanium (Ti6Al4V cap and abutment) | 110 | 0.3 | 830 | 900 |
| Zirconia (crown) | 200 | 0.31 | 330 | 551 |
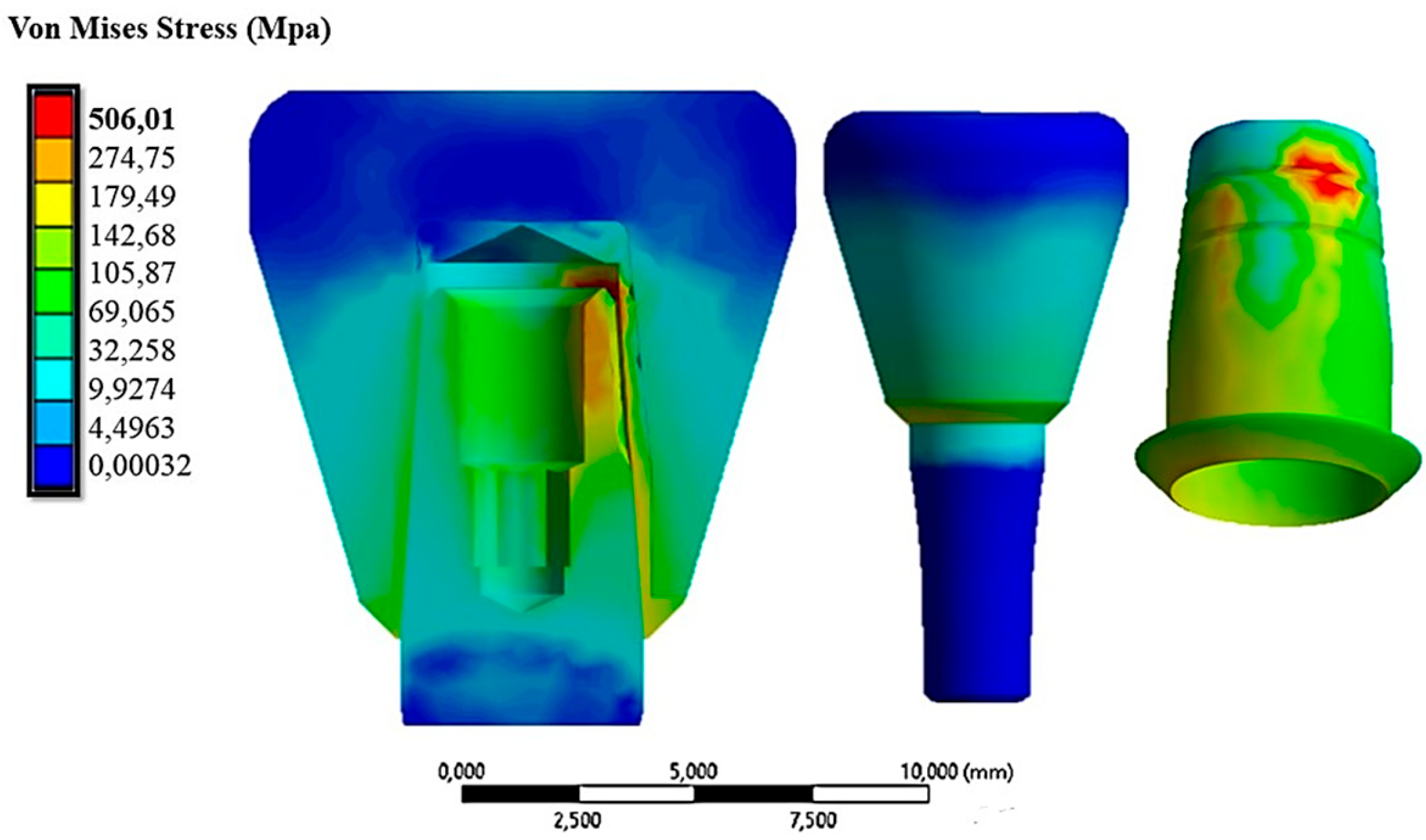
| Insertion Force (N) | Displacement of Coping (mm) | Von Mises Stress (MPa) |
|---|---|---|
| 0 | 0.00273 | 506.01 |
| 20 | 0.00546 | 508.52 |
| 30 | 0.00819 | 511.73 |
| 40 | 0.01092 | 530.56 |
| 50 | 0.01365 | 570.34 |
| 60 | 0.01638 | 594.08 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ceddia, M.; Comuzzi, L.; Di Pietro, N.; Romasco, T.; Specchiulli, A.; Piattelli, A.; Trentadue, B. Finite Element Analysis (FEA) for the Evaluation of Retention in a Conometric Connection for Implant and Prosthesis. Osteology 2023, 3, 140-156. https://doi.org/10.3390/osteology3040015
Ceddia M, Comuzzi L, Di Pietro N, Romasco T, Specchiulli A, Piattelli A, Trentadue B. Finite Element Analysis (FEA) for the Evaluation of Retention in a Conometric Connection for Implant and Prosthesis. Osteology. 2023; 3(4):140-156. https://doi.org/10.3390/osteology3040015
Chicago/Turabian StyleCeddia, Mario, Luca Comuzzi, Natalia Di Pietro, Tea Romasco, Alessandro Specchiulli, Adriano Piattelli, and Bartolomeo Trentadue. 2023. "Finite Element Analysis (FEA) for the Evaluation of Retention in a Conometric Connection for Implant and Prosthesis" Osteology 3, no. 4: 140-156. https://doi.org/10.3390/osteology3040015
APA StyleCeddia, M., Comuzzi, L., Di Pietro, N., Romasco, T., Specchiulli, A., Piattelli, A., & Trentadue, B. (2023). Finite Element Analysis (FEA) for the Evaluation of Retention in a Conometric Connection for Implant and Prosthesis. Osteology, 3(4), 140-156. https://doi.org/10.3390/osteology3040015









