Quiescent Optical Solitons with Quadratic-Cubic and Generalized Quadratic-Cubic Nonlinearities

Abstract

This paper studies the formulation of dark and singular stationary optical solitons that stem from quadratic-cubic and generalized quadratic-cubic forms of nonlinear refractive index coupled with nonlinear chromatic dispersion. The temporal evolution is taken to be of both kinds, namely linear and generalized. The enhanced Kudryashov’s approach enables this retrieval possible.

1. Introduction

The dynamics of soliton propagation through optical fibers and other forms of optical waveguides is a scientifically rich phenomena. Occasionally, it so happens that such an engineering marvel encounters several road blocks. A few of them are soliton radiation and consequently shedding of soliton energy, collision-induced timing jitter, four-wave mixing and many others. Another lesser known and lesser studied detrimental phenomena is the formation of quiescent optical solitons when the chromatic dispersion (CD) is rendered to be nonlinear [1,2,3,4,5,6,7,8,9,10]. This can be triggered from several possible unwanted situations that arise during the course of soliton transmittal across intercontinental distances. These could include rough handling of fibers, twisting and bending of fibers, water pressure from undersea cables, and many others. This would lead to solitons getting stalled during its transmittal. Such a phenomenon is discussed in this paper from a mathematical perspective.

There are two types of nonlinear refractive index structures that are taken into account in this paper. They are quadratic-cubic (QC) and its generalized version that is referred to as generalized QC nonlinearity. The enhanced Kudryashov’s scheme makes the retrieval of the stationary solitons possible. It is dark and singular stationary solitons that are yielded for the model with the two forms of self-phase modulation (SPM) considered in this paper. The temporal evolution is initially considered to be linear and thereafter its generalized version is taken up. The results are derived and exhibited in the rest of the paper. These are penned in detail after a succinct introduction to the model and a quick re-visitation of the integration scheme. To step back in time, the concept of nonlinear CD was introduced by Yan during 2006 that led to the formation of quiescent optical solitons [10]. Thereafter, this concept was further explored for a wide variety of models, which otherwise gave way to mobile solitons, such as the complex Ginzburg–Landau equation, Sasa–Satsuma equation, Lakshmanan–Porsezian–Daniel equation, and many more [11,12,13,14,15]. The current paper revisits the familiar nonlinear Schrödinger’s equation (NLSE) with quadratic-cubic and generalized quadratic-cubic nonlinearities. In this context, for both nonlinear structures, linear temporal evolution as well as generalized temporal evolutions are considered. It must be transparent that the derivation of these quiescent soliton solutions was successful by the aid of direct computer software usage that gave way to implicit forms of quiescent solitons [11,12,13,14,15]. The analytical derivation of such solitons, however, was carried out earlier by the usage of Jacobi’s elliptic function expansion and extended the trial function approach [1,2]. The current paper implements enhanced Kudryashov’s mechanism to recover quiescent solitons to the NLSE.

2. The Enhanced Kudryashov’s Procedure

Consider a governing model

$$G(u,u_x,u_t,u_{xt},u_{xx},\dots )=0,$$

(1)

where $u=u(x,t)$ denotes a wave profile, while t and x depict temporal and spatial variables in sequence.

The relations

$$u(x,t)=U\left(\xi \right),\xi =\mu (x-\upsilon t),$$

(2)

condense (1) to

$$P(U,-\mu \upsilon U^{\prime },\mu U^{\prime },{\mu }^2{U}^{″},...)=0,$$

(3)

where $\mu$ is the inverse wave width, $\xi$ is the wave variable, and $\upsilon$ is the wave velocity.

• Step–1: The reduced model (3) admits the explicit solution

  $$U\left(\xi \right)={\lambda }_0+\sum _{l=1}^N\sum _{i+j=l}{\lambda }_{ij}{Q}^i\left(\xi \right)R^j\left(\xi \right),$$

  (4)

  along with the ancillary equations

  $${R^{\prime }\left(\xi \right)}^2={R\left(\xi \right)}^2(1-\chi {R\left(\xi \right)}^2),$$

  (5)

  and

  $$Q^{\prime }\left(\xi \right)=Q\left(\xi \right)(\eta Q\left(\xi \right)-1),$$

  (6)

  where ${\lambda }_0$, $\chi$, $\eta$, ${\lambda }_{ij}(i,j=0,1,...,N)$ are constants, where N come from the balancing technique in (3).
• Step–2: Equations (5) and (6) also provide the solitons that are of the forms

  $$R\left(\xi \right)=\frac{4c}{4c^2{e}^{\xi }+\chi e^{-\xi }},$$

  (7)

  and

  $$Q\left(\xi \right)=\frac{1}{\eta +b{e}^{\xi }},$$

  (8)

  where c and b are constants.
• Step–3: Substituting (4) along with (5) and (6) into (3) provides us with the much-needed constants in (2)–(6). Lastly, plugging in the obtained parametric restrictions along with (7) or (8) into (4), one arrives straddled solitons which can be reduced to dark or singular solitons.

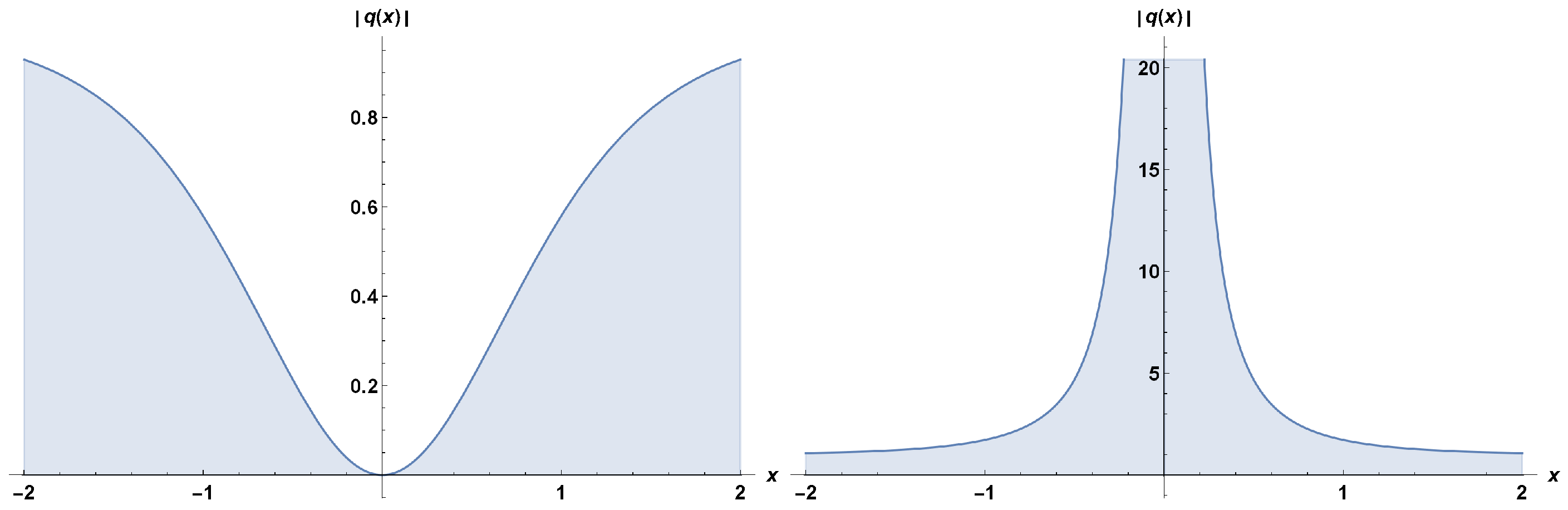
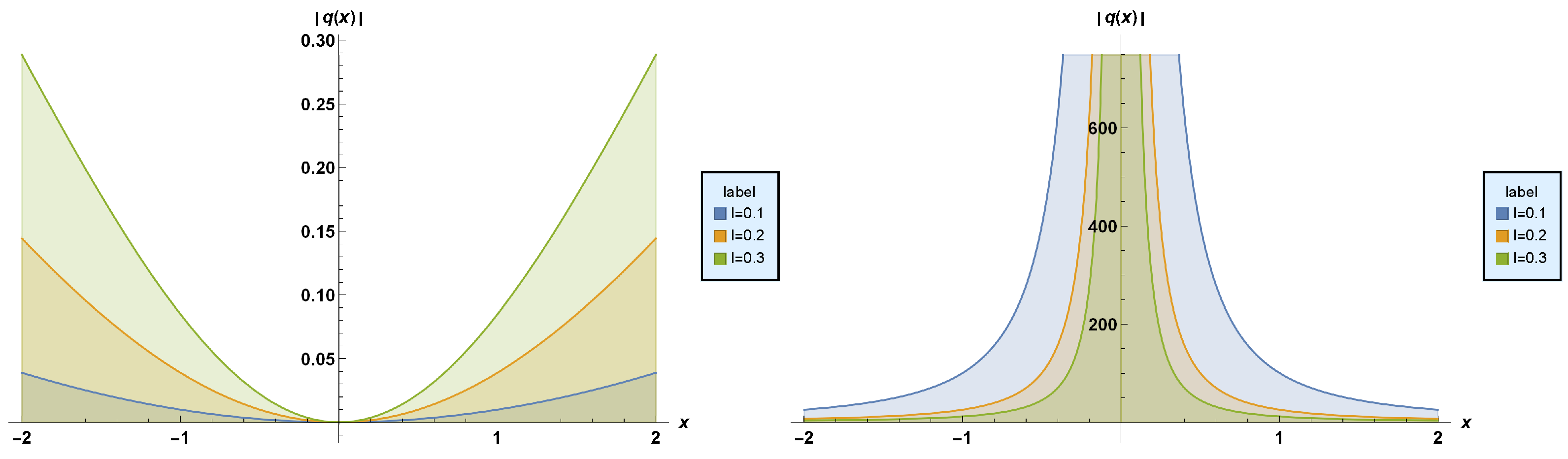
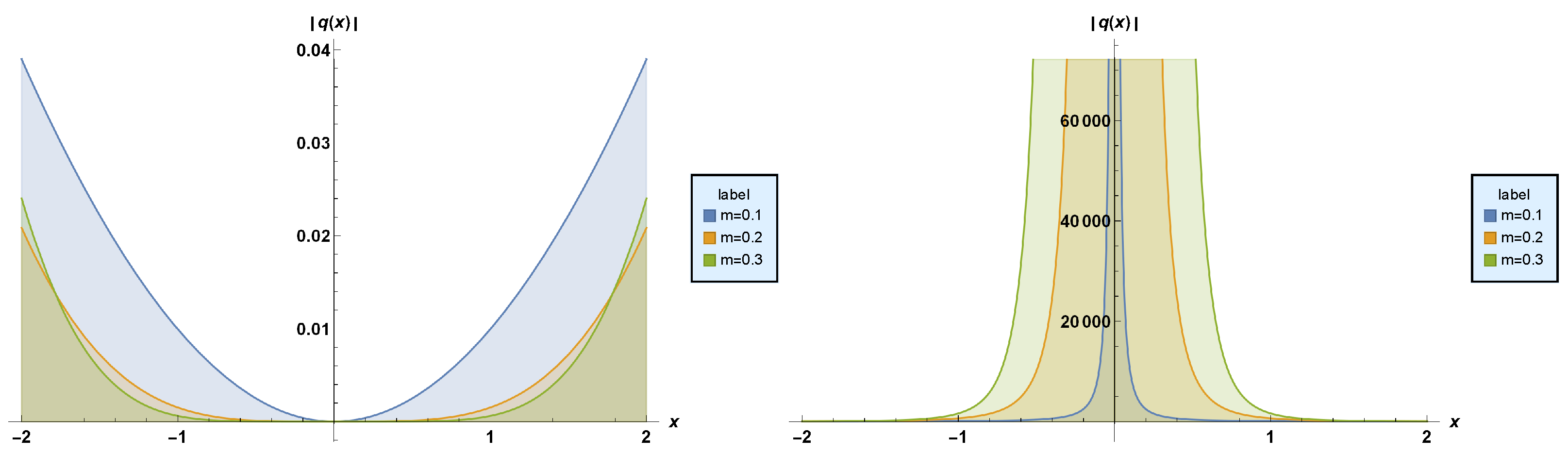
3. Quadratic-Cubic Nonlinearity

The structure of QC nonlinearity was first reported during 1994 [4]. Later, after a long hiatus, this form of SPM was picked up by Fujioka et al. during 2011 [3] and thereafter a deluge of results ensued with such a nonlinearity structure. The current section will derive stationary solitons for QC nonlinearity having nonlinear form of CD.

3.1. Linear Temporal Evolution

In this case, the NLSE shapes up as [3,4]

$$i{q}_t+a{\left({\left|q\right|}^{n}q\right)}_{xx}+\left(b_1\left|q\right|+b_2{\left|q\right|}^2\right)q=0,$$

(9)

where $q=q(x,t)$ purports the wave profile, while x and t stand for the spatial and temporal variables in sequence. The first term signifies the linear temporal evolution, while a comes from the nonlinear CD. Finally, $b_1$ and $b_2$ stem from the SPM, while n arises from the full nonlinearity. It must be noted that, if $n=1$, we recover the linear CD that yields a mobile soliton.

Equation (2) is the general integration algorithm for real-valued partial differential equations (PDEs). However, Equation (9) is a complex-valued PDE and consequently the phase component of the complex variable $q(x,t)$ is split off so that the real part can be further analyzed as explained in (2). Therefore, model (9) permits the wave form

$$q(x,t)=U\left(kx\right)e^{i(\omega t+{\theta }_0)},$$

(10)

where ${\theta }_0$ depicts the phase constant, and $\omega$ denotes the wave number. Inserting (10) into (9) retrieves the governing equation

$$a{k}^2(n+1)U^{n+1}{U}^{″}+a{k}^{2}n(n+1)U^n{U^{\prime }}^2+b_2{U}^4+b_1{U}^3-\omega U^2=0.$$

(11)

Taking $n=1$ simplifies (11) to

$$2a{k}^{2}U{U}^{″}+2a{k}^2{U^{\prime }}^2+b_2{U}^3+b_1{U}^2-\omega U=0.$$

(12)

By the implementation of the balancing approach in (12), one reduces (4) to

$$U\left(\xi \right)={\lambda }_0+{\lambda }_{01}R\left(\xi \right)+{\lambda }_{10}Q\left(\xi \right)+{\lambda }_{11}R\left(\xi \right)Q\left(\xi \right)+{\lambda }_{02}R{\left(\xi \right)}^2+{\lambda }_{20}Q{\left(\xi \right)}^2.$$

(13)

Inserting (13) along with (5) and (6) into (12) provides us with the results:

• Result–1:

  $$\begin{array}{c}{\lambda }_0=-\frac{5b_1}{8b_2},{\lambda }_{01}={\lambda }_{20}={\lambda }_{11}={\lambda }_{10}=0,{\lambda }_{02}=-\chi {\lambda }_0,\hfill \\ k=\pm \frac{1}{4}\sqrt{\frac{b_1}{2a}},\omega =-\frac{15b_1^2}{64b_2}.\hfill \end{array}$$

  (14)

  Putting (14) along with (7) into (13) paves the way for the straddled soliton

  $$q(x,t)=-\frac{5b_1}{8b_2}\left[1-\chi {\left(\frac{4c}{4c^{2}exp\left[\pm \frac{1}{4}\sqrt{\frac{b_1}{2a}}x\right]+\chi exp\left[\mp \frac{1}{4}\sqrt{\frac{b_1}{2a}}x\right]}\right)}^2\right]e^{i\left(-\frac{15b_1^2}{64b_2}t+{\theta }_0\right)}.$$

  (15)

  If $a{b}_1>0$ and $\chi =\pm 4c^2$ in (15), the dark and singular solitons stick out as

  $$q(x,t)=-\frac{5b_1}{8b_2}{tanh}^2\left[\frac{1}{4}\sqrt{\frac{b_1}{2a}}x\right]e^{i\left(-\frac{15b_1^2}{64b_2}t+{\theta }_0\right)},$$

  (16)

  and

  $$q(x,t)=-\frac{5b_1}{8b_2}{coth}^2\left[\frac{1}{4}\sqrt{\frac{b_1}{2a}}x\right]e^{i\left(-\frac{15b_1^2}{64b_2}t+{\theta }_0\right)}.$$

  (17)

  Figure 1 depicts the plots of dark and singular solitons (16) and (17), respectively. The parameter values chosen are: $b_1=1$, $b_2=1$ and $a=1$.
• Result–2:

  $$\begin{array}{c}{\lambda }_0=-\frac{5b_1}{8b_2},{\lambda }_{01}={\lambda }_{11}={\lambda }_{02}=0,{\lambda }_{20}=4{\eta }^2{\lambda }_0,\hfill \\ {\lambda }_{10}=-4\eta {\lambda }_0,k=\pm \frac{1}{2}\sqrt{\frac{b_1}{2a}},\omega =-\frac{15b_1^2}{64b_2}.\hfill \end{array}$$

  (18)

  Putting (18) along with (8) into (13) provides us with the straddled soliton

  $$q(x,t)=-\frac{5b_1}{8b_2}\left\{1-\frac{4b\eta exp\left[\frac{1}{2}\sqrt{\frac{b_1}{2a}}x\right]}{{\left(bexp\left[\frac{1}{2}\sqrt{\frac{b_1}{2a}}x\right]+\eta \right)}^2}\right\}{e}^{i\left(-\frac{15b_1^2}{64b_2}t+{\theta }_0\right)}.$$

  (19)

  When $a{b}_1>0$ and $\eta =\pm b$ in (19), one retrieves the dark and singular solitons

  $$q(x,t)=-\frac{5b_1}{8b_2}{tanh}^2\left[\frac{1}{4}\sqrt{\frac{b_1}{2a}}x\right]e^{i\left(-\frac{15b_1^2}{64b_2}t+{\theta }_0\right)},$$

  (20)

  and

  $$q(x,t)=-\frac{5b_1}{8b_2}{coth}^2\left[\frac{1}{4}\sqrt{\frac{b_1}{2a}}x\right]e^{i\left(-\frac{15b_1^2}{64b_2}t+{\theta }_0\right)}.$$

  (21)

  Figure 2 depicts the plots of dark and singular solitons (20) and (21), respectively. The parameter values chosen are: $b_1=1$, $b_2=1$ and $a=1$.

3.2. Generalized Temporal Evolution

Thus, the model evolves as

$$i{\left(q^l\right)}_t+a{\left({\left|q\right|}^n{q}^l\right)}_{xx}+\left(b_1\left|q\right|+b_2{\left|q\right|}^2\right)q^l=0,$$

(22)

where l stands for the generalized temporal evolution. If $l=1$, Equation (22) collapses to (9) so that one falls back to the case of linear temporal evolution. Plugging (10) into (22) causes the main equation

$$a{k}^2\left(l^2+l(2n-1)+(n-1)n\right)U^n{U^{\prime }}^2+a{k}^2(l+n)U^{n+1}{U}^{″}+b_1{U}^3+b_2{U}^4-l\omega U^2=0.$$

(23)

Setting $n=1$ condenses (23) to

$$a{k}^2(l+1)U{U}^{″}+a{k}^{2}l(l+1){U^{\prime }}^2+b_2{U}^3+b_1{U}^2-l\omega U=0.$$

(24)

By the implementation of the balancing scheme in (24), one collapses (4) to

$$U\left(\xi \right)={\lambda }_0+{\lambda }_{01}R\left(\xi \right)+{\lambda }_{10}Q\left(\xi \right)+{\lambda }_{11}R\left(\xi \right)Q\left(\xi \right)+{\lambda }_{02}R{\left(\xi \right)}^2+{\lambda }_{20}Q{\left(\xi \right)}^2.$$

(25)

Plugging (25) along with (5) and (6) into (24) provides us with the results:

• Result–1:

  $$\begin{array}{c}{\lambda }_0=-\frac{b_1(2l+3)}{4b_2(l+1)},{\lambda }_{01}={\lambda }_{20}={\lambda }_{11}={\lambda }_{10}=0,{\lambda }_{02}=-\chi {\lambda }_0,\hfill \\ k=\pm \frac{1}{2(l+1)}\sqrt{\frac{b_1}{2a}},\omega =-\frac{b_1^2(4l(l+2)+3)}{16b_{2}l{(l+1)}^2}.\hfill \end{array}$$

  (26)

  Inserting (26) along with (7) into (25) reveals the straddled soliton

  $$\begin{array}{c}q(x,t)=-\frac{b_1(2l+3)}{4b_2(l+1)}\left\{1-\chi {\left(\frac{4c}{4c^{2}exp\left[\pm \frac{1}{2(l+1)}\sqrt{\frac{b_1}{2a}}x\right]+\chi exp\left[\mp \frac{1}{2(l+1)}\sqrt{\frac{b_1}{2a}}x\right]}\right)}^2\right\}\hfill \\ \times e^{i\left(-\frac{b_1^2(4l(l+2)+3)}{16b_{2}l{(l+1)}^2}t+{\theta }_0\right)}.\hfill \end{array}$$

  (27)

  Taking $a{b}_1>0$ and $\chi =\pm 4c^2$ in (27) secures the dark and singular solitons

  $$q(x,t)=-\frac{b_1(2l+3)}{4b_2(l+1)}{tanh}^2\left[\frac{1}{2(l+1)}\sqrt{\frac{b_1}{2a}}x\right]e^{i\left(-\frac{b_1^2(4l(l+2)+3)}{16b_{2}l{(l+1)}^2}t+{\theta }_0\right)},$$

  (28)

  and

  $$q(x,t)=-\frac{b_1(2l+3)}{4b_2(l+1)}{coth}^2\left[\frac{1}{2(l+1)}\sqrt{\frac{b_1}{2a}}x\right]e^{i\left(-\frac{b_1^2(4l(l+2)+3)}{16b_{2}l{(l+1)}^2}t+{\theta }_0\right)}.$$

  (29)

  Figure 3 depicts the plots of dark and singular solitons (28) and (29), respectively. The parameter values chosen are: $b_1=1$, $b_2=1$ and $a=1$.
• Result–2:

  $$\begin{array}{c}{\lambda }_0=-\frac{b_1(2l+3)}{4b_2(l+1)},{\lambda }_{01}={\lambda }_{11}={\lambda }_{02}=0,{\lambda }_{20}=4{\eta }^2{\lambda }_0,\hfill \\ {\lambda }_{10}=-4\eta {\lambda }_0,k=\pm \frac{1}{l+1}\sqrt{\frac{b_1}{2a}},\omega =-\frac{b_1^2(2l+1)(2l+3)}{16b_{2}l{(l+1)}^2}.\hfill \end{array}$$

  (30)

  Putting (30) along with (8) into (25) extracts the straddled soliton

  $$q(x,t)=-\frac{b_1(2l+3)}{4b_2(l+1)}\left\{1-\frac{4b\eta exp\left[\frac{1}{l+1}\sqrt{\frac{b_1}{2a}}x\right]}{{\left(bexp\left[\frac{1}{l+1}\sqrt{\frac{b_1}{2a}}x\right]+\eta \right)}^2}\right\}{e}^{i\left(-\frac{b_1^2(2l+1)(2l+3)}{16b_{2}l{(l+1)}^2}t+{\theta }_0\right)}.$$

  (31)

  Setting $a{b}_1>0$ and $\eta =\pm b$ in (31) recovers the dark and singular solitons

  $$q(x,t)=-\frac{b_1(2l+3)}{4b_2(l+1)}{tanh}^2\left[\frac{1}{2(l+1)}\sqrt{\frac{b_1}{2a}}x\right]e^{i\left(-\frac{b_1^2(2l+1)(2l+3)}{16b_{2}l{(l+1)}^2}t+{\theta }_0\right)},$$

  (32)

  and

  $$q(x,t)=-\frac{b_1(2l+3)}{4b_2(l+1)}{coth}^2\left[\frac{1}{2(l+1)}\sqrt{\frac{b_1}{2a}}x\right]e^{i\left(-\frac{b_1^2(2l+1)(2l+3)}{16b_{2}l{(l+1)}^2}t+{\theta }_0\right)}.$$

  (33)

  Figure 4 depicts the plots of dark and singular solitons (32) and (33), respectively. The parameter values chosen are: $b_1=1$, $b_2=1$ and $a=1$.

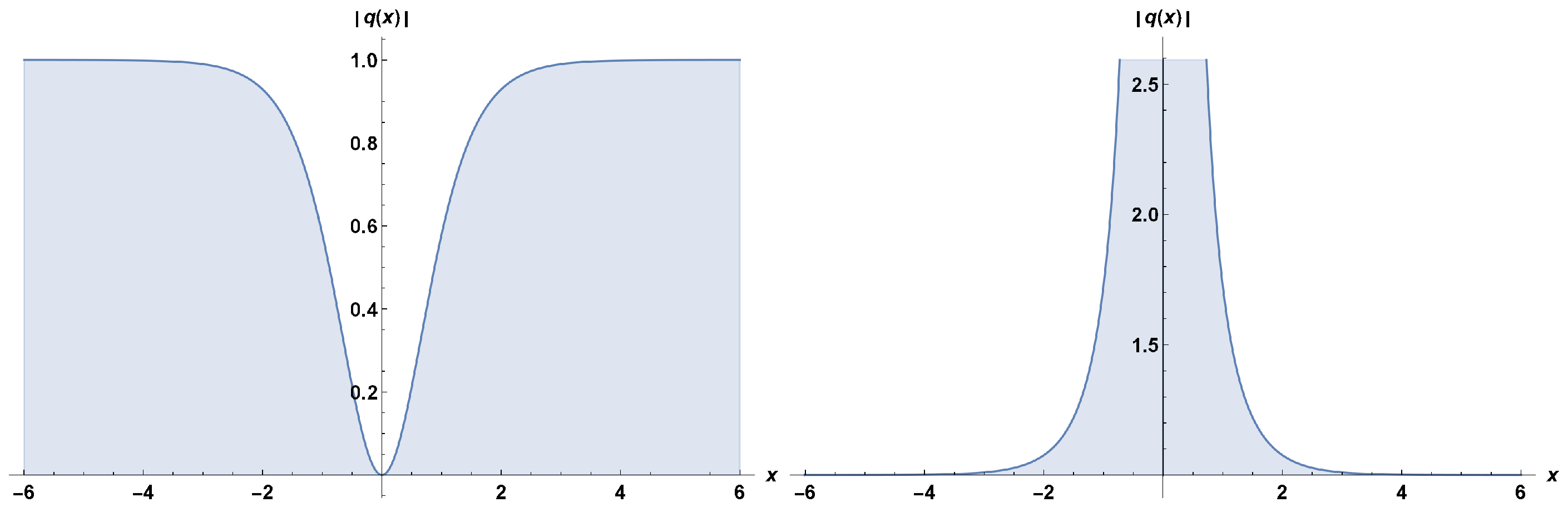
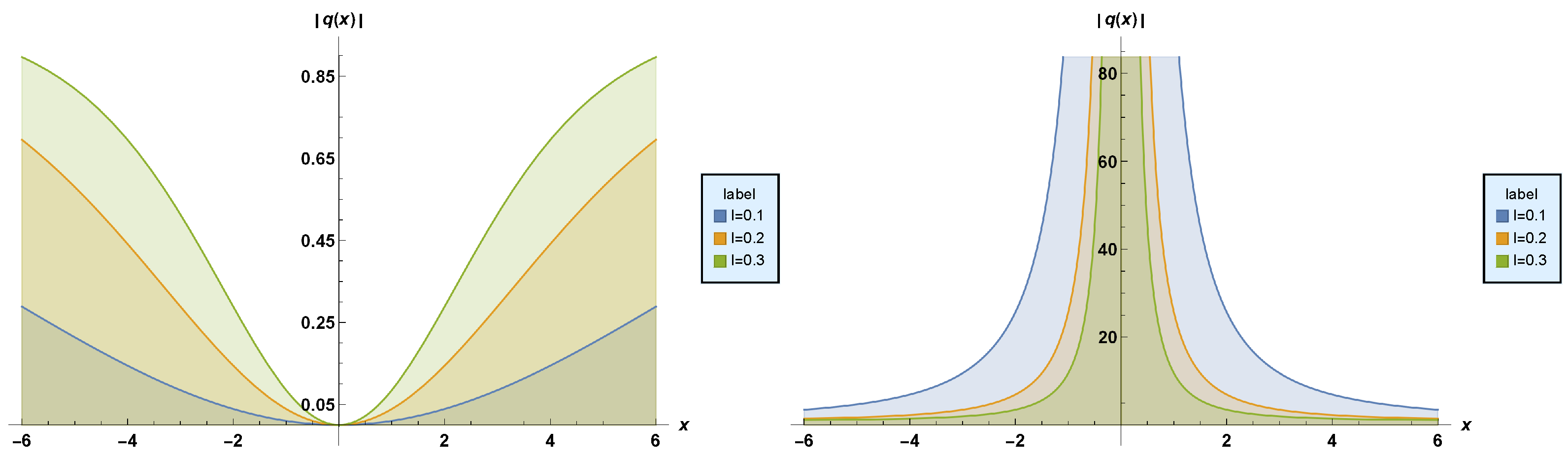
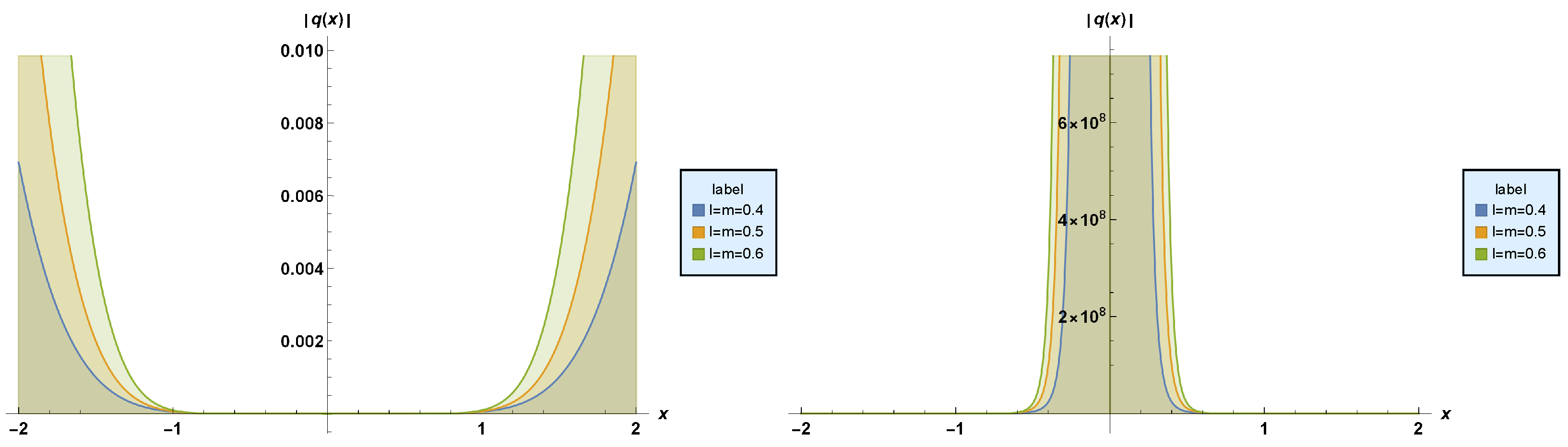
4. Generalized Quadratic-Cubic Nonlinearity

This form of nonlinearity was first studied by Triki et al. during 2019 [8]. It was established that, with linear CD, mobile solitons exist. The current section will discuss the formation of stationary solitons when CD is rendered to be nonlinear with linear as well as generalized temporal evolution effects.

4.1. Linear Temporal Evolution

Therefore, the model stands as [8]

$$i{q}_t+a{\left({\left|q\right|}^{n}q\right)}_{xx}+\left(b_1{\left|q\right|}^m+b_2{\left|q\right|}^{2m}\right)q=0.$$

(34)

Plugging (10) into (34) reveals the strategic equation

$$a{k}^2(n+1)U^{n+1}{U}^{″}+a{k}^{2}n(n+1)U^n{U^{\prime }}^2+b_1{U}^{m+2}+b_2{U}^{2m+2}-U^2\omega =0.$$

(35)

Assume $n=m$. Thus, Equation (35) shapes up as

$$a{k}^2(m+1)U^{m+1}{U}^{″}+a{k}^{2}m(m+1)U^m{U^{\prime }}^2+b_1{U}^{m+2}+b_2{U}^{2m+2}-U^2\omega =0.$$

(36)

The relation

$$U=V^{\frac{1}{m}}$$

(37)

collapses Equation (36) to

$$a{k}^{2}m(m+1)V{V}^{″}+a{k}^2(m+1){V^{\prime }}^2+m^{2}V\left(b_2{V}^2+b_{1}V-\omega \right)=0.$$

(38)

By the implementation of the balancing algorithm in (38), one simplifies (4) to

$$V\left(\xi \right)={\lambda }_0+{\lambda }_{01}R\left(\xi \right)+{\lambda }_{10}Q\left(\xi \right)+{\lambda }_{11}R\left(\xi \right)Q\left(\xi \right)+{\lambda }_{02}R{\left(\xi \right)}^2+{\lambda }_{20}Q{\left(\xi \right)}^2.$$

(39)

Inserting (39) along with (5) and (6) into (38) retrieves the results:

• Result–1:

  $$\begin{array}{c}{\lambda }_0=-\frac{b_1(2+3m)}{4b_2(1+m)},{\lambda }_{01}={\lambda }_{20}={\lambda }_{11}={\lambda }_{10}=0,{\lambda }_{02}=-\chi {\lambda }_0,\hfill \\ k=\pm \frac{m}{2(1+m)}\sqrt{\frac{b_1}{2a}},\omega =-\frac{b_1^2(2+m)(2+3m)}{16b_2{(1+m)}^2}.\hfill \end{array}$$

  (40)

  Putting (40) along with (7) into (39) derives the straddled soliton

  $$\begin{array}{c}q(x,t)={\left[-\frac{b_1(2+3m)}{4b_2(1+m)}\left\{1-\chi {\left(\frac{4c}{4c^{2}exp\left[\pm \frac{m}{2(1+m)}\sqrt{\frac{b_1}{2a}}x\right]+\chi exp\left[\mp \frac{m}{2(1+m)}\sqrt{\frac{b_1}{2a}}x\right]}\right)}^2\right\}\right]}^{\frac{1}{m}}\hfill \\ \times e^{i\left(-\frac{b_1^2(2+m)(2+3m)}{16b_2{(1+m)}^2}t+{\theta }_0\right)}.\hfill \end{array}$$

  (41)

  If $a{b}_1>0$ and $\chi =\pm 4c^2$ in (41), the dark and singular solitons evolve as

  $$q(x,t)={\left\{-\frac{b_1(2+3m)}{4b_2(1+m)}{tanh}^2\left[\frac{m}{2(1+m)}\sqrt{\frac{b_1}{2a}}x\right]\right\}}^{\frac{1}{m}}{e}^{i\left(-\frac{b_1^2(2+m)(2+3m)}{16b_2{(1+m)}^2}t+{\theta }_0\right)},$$

  (42)

  and

  $$q(x,t)={\left\{-\frac{b_1(2+3m)}{4b_2(1+m)}{coth}^2\left[\frac{m}{2(1+m)}\sqrt{\frac{b_1}{2a}}x\right]\right\}}^{\frac{1}{m}}{e}^{i\left(-\frac{b_1^2(2+m)(2+3m)}{16b_2{(1+m)}^2}t+{\theta }_0\right)}.$$

  (43)

  Figure 5 depicts the plots of dark and singular solitons (42) and (43), respectively. The parameter values chosen are: $b_1=1$, $b_2=-1$ and $a=1$.
• Result–2:

  $$\begin{array}{c}{\lambda }_0=-\frac{b_1(2+3m)}{4b_2(1+m)},{\lambda }_{01}={\lambda }_{11}={\lambda }_{02}=0,{\lambda }_{20}=4{\eta }^2{\lambda }_0,\hfill \\ {\lambda }_{10}=-4\eta {\lambda }_0,k=\pm \frac{m}{1+m}\sqrt{\frac{b_1}{2a}},\omega =-\frac{b_1^2(2+m)(2+3m)}{16b_2{(1+m)}^2}.\hfill \end{array}$$

  (44)

  Inserting (44) along with (8) into (39) secures the straddled soliton

  $$q(x,t)={\left\{-\frac{b_1(2+3m)}{4b_2(1+m)}\left(1-\frac{4b\eta exp\left[\frac{m}{1+m}\sqrt{\frac{b_1}{2a}}x\right]}{{\left(bexp\left[\frac{m}{1+m}\sqrt{\frac{b_1}{2a}}x\right]+\eta \right)}^2}\right)\right\}}^{\frac{1}{m}}{e}^{i\left(-\frac{b_1^2(2+m)(2+3m)}{16b_2{(1+m)}^2}t+{\theta }_0\right)}.$$

  (45)

  When $a{b}_1>0$ and $\eta =\pm b$ in (45), one acquires dark and singular solitons

  $$q(x,t)={\left\{-\frac{b_1(2+3m)}{4b_2(1+m)}{tanh}^2\left[\frac{m}{2(1+m)}\sqrt{\frac{b_1}{2a}}x\right]\right\}}^{\frac{1}{m}}{e}^{i\left(-\frac{b_1^2(2+m)(2+3m)}{16b_2{(1+m)}^2}t+{\theta }_0\right)},$$

  (46)

  and

  $$q(x,t)={\left\{-\frac{b_1(2+3m)}{4b_2(1+m)}{coth}^2\left[\frac{m}{2(1+m)}\sqrt{\frac{b_1}{2a}}x\right]\right\}}^{\frac{1}{m}}{e}^{i\left(-\frac{b_1^2(2+m)(2+3m)}{16b_2{(1+m)}^2}t+{\theta }_0\right)}.$$

  (47)

  Figure 6 depicts the plots of dark and singular solitons (46) and (47), respectively. The parameter values chosen are: $b_1=1$, $b_2=-1$ and $a=1$.

4.2. Generalized Temporal Evolution

In this case, Equation (34) reads as

$$i{\left(q^l\right)}_t+a{\left({\left|q\right|}^n{q}^l\right)}_{xx}+\left(b_1{\left|q\right|}^m+b_2{\left|q\right|}^{2m}\right)q^l=0.$$

(48)

Again, (48) reduces to (34) for $l=1$, which represents the case with linear temporal evolution. Plugging (10) into (48) paves the way for the fundamental equation

$$a{k}^2\left(l^2+l(2n-1)+(n-1)n\right)U^n{U^{\prime }}^2+a{k}^2(l+n)U^{n+1}{U}^{″}+b_1{U}^{m+2}+b_2{U}^{2m+2}-l\omega U^2=0.$$

(49)

Assume $n=m$. Thus, Equation (49) changes to

$$a{k}^2\left(l^2+l(2m-1)+(m-1)m\right)U^m{U^{\prime }}^2+a{k}^2(l+m)U^{m+1}{U}^{″}+b_1{U}^{m+2}+b_2{U}^{2m+2}-l\omega U^2=0.$$

(50)

The restriction

$$U=V^{\frac{1}{m}}$$

(51)

collapses Equation (50) to

$$a{k}^{2}m(l+m)V{V}^{″}+a{k}^{2}l(l+m){V^{\prime }}^2+m^{2}V\left(b_2{V}^2+b_{1}V-l\omega \right)=0.$$

(52)

By the implementation of the balancing procedure in (52), one translates (4) to

$$V\left(\xi \right)={\lambda }_0+{\lambda }_{01}R\left(\xi \right)+{\lambda }_{10}Q\left(\xi \right)+{\lambda }_{11}R\left(\xi \right)Q\left(\xi \right)+{\lambda }_{02}R{\left(\xi \right)}^2+{\lambda }_{20}Q{\left(\xi \right)}^2.$$

(53)

Inserting (53) along with (5) and (6) into (52) retrieves the results:

• Result–1:

  $$\begin{array}{c}{\lambda }_0=-\frac{b_1(2l+3m)}{4b_2(l+m)},{\lambda }_{01}={\lambda }_{20}={\lambda }_{11}={\lambda }_{10}=0,{\lambda }_{02}=-\chi {\lambda }_0,\hfill \\ k=\pm \frac{m}{2(l+m)}\sqrt{\frac{b_1}{2a}},\omega =-\frac{b_1^2(2l+m)(2l+3m)}{16b_{2}l{(l+m)}^2}.\hfill \end{array}$$

  (54)

  Putting (54) along with (7) into (53) leaves us with the straddled soliton

  $$\begin{array}{c}q(x,t)={\left[-\frac{b_1(2l+3m)}{4b_2(l+m)}\left\{1-\chi {\left(\frac{4c}{4c^{2}exp\left[\pm \frac{m}{2(l+m)}\sqrt{\frac{b_1}{2a}}x\right]+\chi exp\left[\mp \frac{m}{2(l+m)}\sqrt{\frac{b_1}{2a}}x\right]}\right)}^2\right\}\right]}^{\frac{1}{m}}\hfill \\ \times e^{i\left(-\frac{b_1^2(2l+m)(2l+3m)}{16b_{2}l{(l+m)}^2}t+{\theta }_0\right)}.\hfill \end{array}$$

  (55)

  Taking $a{b}_1>0$ and $\chi =\pm 4c^2$ turns (55) into dark and singular solitons

  $$q(x,t)={\left\{-\frac{b_1(2l+3m)}{4b_2(l+m)}{tanh}^2\left[\frac{m}{2(l+m)}\sqrt{\frac{b_1}{2a}}x\right]\right\}}^{\frac{1}{m}}{e}^{i\left(-\frac{b_1^2(2l+m)(2l+3m)}{16b_{2}l{(l+m)}^2}t+{\theta }_0\right)},$$

  (56)

  and

  $$q(x,t)={\left\{-\frac{b_1(2l+3m)}{4b_2(l+m)}{coth}^2\left[\frac{m}{2(l+m)}\sqrt{\frac{b_1}{2a}}x\right]\right\}}^{\frac{1}{m}}{e}^{i\left(-\frac{b_1^2(2l+m)(2l+3m)}{16b_{2}l{(l+m)}^2}t+{\theta }_0\right)}.$$

  (57)

  Figure 7 depicts the plots of dark and singular solitons (56) and (57), respectively. The parameter values chosen are: $b_1=1$, $b_2=-1$ and $a=1$.
• Result–2:

  $$\begin{array}{c}{\lambda }_0=-\frac{b_1(2l+3m)}{4b_2(l+m)},{\lambda }_{01}={\lambda }_{11}={\lambda }_{02}=0,{\lambda }_{20}=4{\eta }^2{\lambda }_0,\hfill \\ {\lambda }_{10}=-4\eta {\lambda }_0,k=\pm \frac{m}{l+m}\sqrt{\frac{b_1}{2a}},\omega =-\frac{b_1^2(2l+m)(2l+3m)}{16b_{2}l{(l+m)}^2}.\hfill \end{array}$$

  (58)

  Substituting (58) along with (8) into (53) provides us with the straddled soliton

  $$q(x,t)={\left\{-\frac{b_1(2l+3m)}{4b_2(l+m)}\left(1-\frac{4b\eta exp\left[\frac{m}{l+m}\sqrt{\frac{b_1}{2a}}x\right]}{{\left(bexp\left[\frac{m}{l+m}\sqrt{\frac{b_1}{2a}}x\right]+\eta \right)}^2}\right)\right\}}^{\frac{1}{m}}{e}^{i\left(-\frac{b_1^2(2l+m)(2l+3m)}{16b_{2}l{(l+m)}^2}t+{\theta }_0\right)}.$$

  (59)

  Setting $a{b}_1>0$ and $\eta =\pm b$ condenses (59) to dark and singular solitons

  $$q(x,t)={\left\{-\frac{b_1(2l+3m)}{4b_2(l+m)}{tanh}^2\left[\frac{m}{2(l+m)}\sqrt{\frac{b_1}{2a}}x\right]\right\}}^{\frac{1}{m}}{e}^{i\left(-\frac{b_1^2(2l+m)(2l+3m)}{16b_{2}l{(l+m)}^2}t+{\theta }_0\right)},$$

  (60)

  and

  $$q(x,t)={\left\{-\frac{b_1(2l+3m)}{4b_2(l+m)}{coth}^2\left[\frac{m}{2(l+m)}\sqrt{\frac{b_1}{2a}}x\right]\right\}}^{\frac{1}{m}}{e}^{i\left(-\frac{b_1^2(2l+m)(2l+3m)}{16b_{2}l{(l+m)}^2}t+{\theta }_0\right)}.$$

  (61)

  Figure 8 depicts the plots of dark and singular solitons (60) and (61), respectively. The parameter values chosen are: $b_1=1$, $b_2=-1$ and $a=1$.

5. Conclusions

The current paper revealed stationary singular and dark optical solitons for the model that came with QC and generalized AC nonlinearity. The temporal evolutions were both linear and generalized. There are four forms of the governing model that were studied in this work, which were gradual generalizations of the fundamental model. The first form is the NLSE with the QC form of the nonlinear refractive index as given by [9]. This model for the propagation of optical solitons, with linear CD, was first proposed during 1994 and later studied again during 2011 [3,4]. Subsequently, the model is addressed with generalized temporal evolution and nonlinear CD as given by (22) that gave way to quiescent solitons as well. Thereafter, Equations (9) and (22) are formulated with a generalized form of the QC law of the nonlinear refractive index, which are represented by (34) and (48), respectively. It must be noted that (34) with linear CD was first proposed during 2019 [8]. The enhanced Kudrashov’s scheme has made this retrieval a success. However, it is quite noticeable that the approach has its shortcoming. It failed to recover stationary bright solitons for both forms of SPM.

Later, the method will be implemented to retrieve quiescent solitons that come with triple forms of nonlinearly structured SPM such as polynomial law and triple-power law of nonlinearity. These would be taken up with time, and their results and findings would be later revealed and reported. Some of the lesser known nonlinear media would be later considered. One such form is the saturable form of nonlinearity that yields dissipative solitons. The results of the work thus show the consequential catastrophic effect that nonlinear CD would bring in. The mobile solitons would stall during its transmission along transcontinental and transoceanic distances. Therefore, it is imperative to make sure that such an unwanted feature of nonlinear CD in an optical fiber, or any other forms of waveguides such as optical couplers or PCF or optical metamaterials, must be avoided at all costs. Telecommunication engineers must therefore exercise extreme caution while laying such fiber optic cables underground or under sea. This is therefore a vital component and a vital feature of soliton transmission along intercontinental distances.