Wideband Mixed Signal Separation Based on Photonic Signal Processing
Abstract
:1. Introduction
2. Interference Management
2.1. Related Work
2.1.1. Photonic Circuit
2.1.2. Digital System
2.2. Challenges
2.3. Future Perspectives
2.3.1. Ultra-Fast Sampling with Pico-Second Laser Pulse
2.3.2. Separation of Mixed Signal with Progressive Cancellation
- ADC resolution: ADC resolution determines the accuracy of by introducing digitization error. In a completely digital system, the cancellation ratio depends on the ADC resolution in the first stage. In the ill-condition cases, the SOI amplitudes are comparable to the ADC resolution, and the signal information is lost in the first at ADC and cannot be recovered. For example, in an 8-bit ADC system, the digitization error is of the signal peak amplitude. In the first ill-condition case, the received signal peak amplitude is defined by the interference. If the signal is not pre-separated in an analog way, the digitization error is comparable to or larger than the amplitude of the SOI, which causes unsuccessful separation. The analog system is different from the digital system in a way that the analog system maintains all the signal information, and the cancellation ratio in a multi-stage system can be progressively improved.
- Weight tunability: Weight tunability determines the accuracy of The de-mixing matrix is implemented by adding weights to the received signal. By applying multiple stages with coarse and fine adjustment of the weights, the mixed signals can be progressively separated at each stage with the cancellation ratio from each of the stages multiplied.
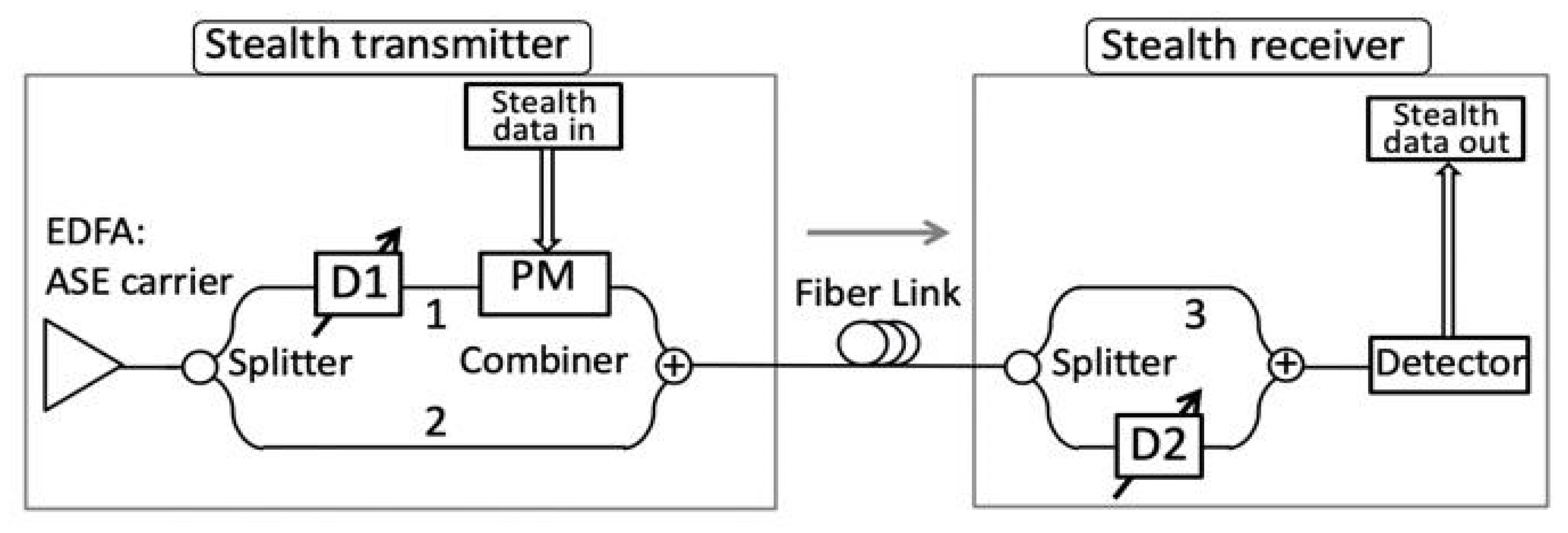
3. Stealth Communication
3.1. Related Work
3.2. Challenges and Threats to the Existing System
Methods to Address the Threats
- Gaussianity and kurtosis of the signal: Most BSS methods, including the one discussed in Section 2, cannot separate the mixed signals when all the original signals are Gaussian signals. This is because the last step (ICA) is to rotate the mixed signal based on the change of kurtosis at different independent component directions. If both the stealth signal and noise have Gaussian distribution, or Gaussian-like signals (Equations (8) and (9)), the kurtosis is equal to 3 at all the directions, and the mixed signals cannot be rotated to separate the mixed signal. Therefore, the system is immune to BSS attacks when both the stealth signal and noise are Gaussian signals.
- Bandwidth of the signal: The requirement of a Gaussian signal is a strict restriction to the stealth signal since most digital signals are not Gaussian signals. Another means of defending against the BSS attack is to expend the bandwidth of the noise signal. The sampling time must be short enough, so the sampled signal is not a time average of the mixed signal. By using the mode-locked laser to improve the sampling time, mixed signals with a bandwidth of up to 50 GHz can be properly sampled. Bandwidth of 50 GHz is an ultra-wideband for interference management, while for stealth communication with noise applied intentionally, bandwidth beyond 50 GHz can be deployed. Experimental results have demonstrated using noise bandwidth of 150 GHz–5000 GHz to hide signals.
- Linear and nonlinear operation: For interference management, the signals are mixed with linear functions. To hide signals in noise, both linear and nonlinear operations can be applied to mix the signals and noise. Experimental results show that with nonlinear operations, the SOI cannot be identified by the statistical properties of the mixed signals, which means the stealth system with a nonlinear mixing function can effectively defend the BSS attack.
3.3. Future Perspectives
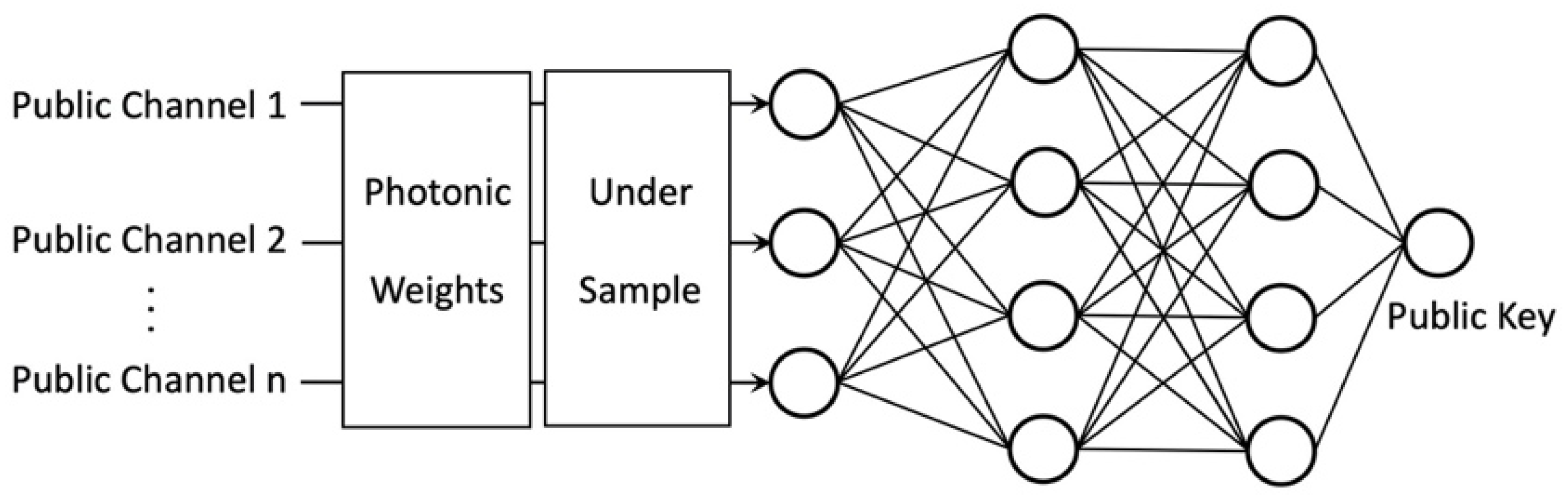
3.3.1. Wireless Stealth Communication and Hybrid with Interference Management
3.3.2. Coexistence of Stealth Channel and Public Channel
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
Appendix A
| Symbol | Definition |
|---|---|
| SOI | Signal of interest |
| BSS | Blind source separation |
| RF | Radio frequency |
| CDMA | Code-division multiple access |
| OFDM | Orthogonal frequency-division multiplexing |
| PCA | Principal component analysis |
| ICA | Independent component analysis |
| ASE | Amplified spontaneous emission |
| Received mixed signal | |
| Mixing matrix | |
| Source signal | |
| Signal received by Receiver 1, 2 | |
| Channel coefficient | |
| Added weights for PCA to mixed signal | |
| for PCA | |
| for PCA | |
| Rectangular diagonal matrix for PCA | |
| for ICA | |
| Added weights for ICA to mixed signal | |
| 4th order moments (kurtosis) for ICA |
References
- Hossain, E.; Rasti, M.; Tabassum, H.; Abdelnasser, A. Evolution toward 5G multi-tier cellular wireless networks: An interference management perspective. IEEE Wirel. Commun. 2014, 21, 3. [Google Scholar] [CrossRef] [Green Version]
- Bloch, M.R. Covert Communication over Noisy Channels: A Resolvability Perspective. IEEE Trans. Inf. Theory. 2016, 62, 5. [Google Scholar] [CrossRef] [Green Version]
- Wu, B.; Wang, Z.; Tian, Y.; Fok, M.; Shastri, B.; Kanoff, D.; Prucnal, P.R. Optical steganography based on amplified spontaneous emission noise. Opt. Express 2013, 21, 2065–2071. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Wu, B.; Shastri, B.; Mittal, P.; Tait, A.N.; Prucnal, P.R. Optical Signal Processing and Stealth Transmission for Privacy. IEEE J. Sel. Top. Signal Process. 2015, 9, 1185–1194. [Google Scholar]
- Akhgar, B.; Arabnia, H.R. Secure Communication in Fiber-Optic Networks; Elsevier Inc.: Amsterdam, The Netherlands, 2013. [Google Scholar]
- Capmany, J.; Mora, J.; Gasulla, I.; Sancho, J.; Lloret, J.; Sales, S. Microwave photonic signal processing. J. Light. Technol. 2013, 4, 571–586. [Google Scholar] [CrossRef]
- Wu, B.; Chang, M.P.; Shastri, B.J.; Tait, A.N.; Prucnal, P.R. Optical steganography based on amplified spontaneous. OSA 2013, 21, 250–251. [Google Scholar]
- Giuliano, R.; Mazzenga, F. On the coexistence of power-controlled ultrawide-band systems with UMTS, GPS, DCS1800, and fixed wireless systems. IEEE Trans. Veh. Technol. 2015, 54, 1. [Google Scholar] [CrossRef]
- Guidotti, A.; Guiducci, D.; Barbiroli, M.; Carciofi, C.; Grazioso, P.; Riva, G. Coexistence and Mutual Interference between Mobile and Broadcasting Systems. In Proceedings of the IEEE Transactions on Vehicular Technology, Budapest, Hungary, 15–18 May 2011; pp. 1–15. [Google Scholar]
- Bash, B.A.; Goeckel, D.; Towsley, D.; Guha, S. Hiding information in noise: Fundamental limits of covert wireless communication. IEEE Commun. 2015, 53, 12. [Google Scholar] [CrossRef] [Green Version]
- Kohno, R.; Meidan, R.; Milstein, L.B. Spread Spectrum Access Methods for Wireless Communications. IEEE Commun. Mag. 1995, 33, 1. [Google Scholar] [CrossRef] [Green Version]
- Zhou, M.; van der Veen, A.J. Blind separation of partially overlapping data packets. Digit. Signal Process. A Rev. J. 2017, 68, 154–166. [Google Scholar] [CrossRef]
- Hajisami, A.; Pompili, D. Cloud-BSS: Joint intra- and inter-Cluster interference cancellation in uplink 5G cellular networks. Comput. Netw. 2018, 147, 180–190. [Google Scholar] [CrossRef]
- Yu, J.; Li, J.; Sun, B.; Chen, J.; Li, C. Multiclass radio frequency interference detection and suppression for SAR based on the single shot multibox detector. Sensors 2018, 18, 4034. [Google Scholar] [CrossRef] [Green Version]
- Luo, Z.; Li, C.; Zhu, L. Full-Duplex Cognitive Radio Using Guided Independent Component Analysis and Cumulant Criterion. IEEE Access 2019, 7, 27065–27074. [Google Scholar] [CrossRef]
- Boya, C.; Rojas-Moreno, M.V.; Ruiz-Llata, M.; Robles, G. Location of partial discharges sources by means of blind source separation of UHF signals. IEEE Trans. Dielectr. Electr. Insul. 2015, 22, 4. [Google Scholar] [CrossRef]
- Fabrizio, G.; Farina, A. Blind source separation with the generalised estimation of multipath signals algorithm. IET Radar Sonar Navig. 2014, 8, 1255–1266. [Google Scholar] [CrossRef]
- Sun, S.; Rappaport, T.S.; Heath, R.W.; Nix, A.; Rangan, S. MIMO for millimeter-wave wireless communications: Beamforming, spatial multiplexing, or both? IEEE Commun. Mag. 2014, 12, 110–121. [Google Scholar] [CrossRef]
- Jensen, M.A.; Wallace, J.W. A review of antennas and propagation for MIMO wireless communications. IEEE Trans. Antennas Propag. 2004, 52, 2810–2824. [Google Scholar] [CrossRef] [Green Version]
- Vook, F.W.; Ghosh, A.; Thomas, T.A. MIMO and Beamforming Solutions for 5G Technology. In Proceedings of the IEEE MTT-S International Microwave Symposium Digest, Tampa, FL, USA, 1–6 June 2014; pp. 1–4. [Google Scholar]
- Dean, T.R.; Wootters, M.; Goldsmith, A.J. Blind Joint MIMO Channel Estimation and Decoding. IEEE Trans. Inf. Theory 2019, 65, 4. [Google Scholar] [CrossRef]
- Tait, A.N.; Ma, P.; Ferreira de Lima, T.; Blow, E.; Chang, M.; Nahmias, M.; Shastri, B.; Prucnal, P. Demonstration of Multivariate Photonics: Blind Dimensionality Reduction With Integrated Photonics. J. Lightwave Technol. 2019, 37, 5996–6006. [Google Scholar] [CrossRef]
- Björnson, E.; Sanguinetti, L.; Wymeersch, H.; Hoydis, J.; Marzetta, T.L. Massive MIMO is a reality—What is next? Five promising research directions for antenna arrays. Digit. Signal Process. Rev. J. 2019, 94, 3–20. [Google Scholar] [CrossRef]
- Liu, B.; Dai, W.; Peng, W.; Meng, X. Spatiotemporal analysis of GPS time series in vertical direction using independent component analysis. Earth Planets Space 2015, 67, 1. [Google Scholar] [CrossRef] [Green Version]
- Gualandi, A.; Serpelloni, E.; Belardinelli, M.E. Blind source separation problem in GPS time series. J. Geod. 2016, 90, 4. [Google Scholar] [CrossRef]
- Kharbech, S.; Dayoub, I.; Simon, E.; Zwingelstein-Colin, M. Blind digital modulation detector for MIMO systems over high-speed railway channels. Lect. Notes Comput. Sci. 2013, 7865, 232–241. [Google Scholar]
- Lü, X.; Zhang, H.; Liu, Z.; Sun, Z.; Liu, P. Research on Co-channel Base Station Interference Suppression Method of Passive Radar Based on LTE Signal. J. Electron. Inf. Technol. 2019, 41, 9. [Google Scholar]
- Delfosse, N.; Loubaton, P. Adaptive blind separation of independent sources: A deflation approach. Signal Process. 1995, 45, 1. [Google Scholar] [CrossRef]
- Bach, F.R.; Jordan, M.I. Kernel independent component analysis. J. Mach. Learn. Res. 2003, 3, 1. [Google Scholar]
- Comon, P.; Jutten, C. Handbook of Blind Source Separation; Academic Press: Cambirgde, MA, USA, 2010. [Google Scholar]
- Cardoso, J.F. Blind signal separation: Statistical principles. Proc. IEEE 1998, 86, 10. [Google Scholar] [CrossRef] [Green Version]
- Agiwal, M.; Roy, A.; Saxena, N. Next generation 5G wireless networks: A comprehensive survey. IEEE Commun. Surv. Tutor. 2016, 18, 3. [Google Scholar] [CrossRef]
- Amjad, M.; Musavian, L.; Rehmani, M.H. Effective Capacity in Wireless Networks: A Comprehensive Survey. IEEE Commun. Surv. Tutor. 2019, 21, 4. [Google Scholar] [CrossRef] [Green Version]
- Tajvidy, A. Channel capacity model in urban areas at 20–70 GHz for 5G systems. Electromagnetics 2020, 40, 1. [Google Scholar] [CrossRef]
- Ban, Y.L.; Li, C.; Sim, C.Y.D.; Wu, G.; Wong, K.L. 4G/5G Multiple Antennas for Future Multi-Mode Smartphone Applications. IEEE Access 2016, 4, 2981–2988. [Google Scholar] [CrossRef]
- Hong, S.; Brand, J.; Choi, J., II; Jain, M.; Mehlman, J.; Katti, S.; Levis, P. Applications of self-interference cancellation in 5G and beyond. IEEE Commun. Mag. 2014, 52, 2. [Google Scholar]
- Balakrishnan, J.; Batra, A.; Dabak, A. A Multi-Band OFDM System for UWB Communication. In Proceedings of the IEEE Conference on Ultra Wideband Systems and Technologies, Reston, VA, USA, 16–19 November 2003; pp. 354–358. [Google Scholar]
- Wang, F.; Yang, A.H.; Kimball, D.F.; Larson, L.E.; Asbeck, P.M. Design of wide-bandwidth envelope-tracking power amplifiers for OFDM applications. IEEE Trans. Microw. Theory Tech. 2005, 53, 4. [Google Scholar]
- Ma, L.; Jiang, W.; Xiang, H. Parameter estimation based on factor graph in wide-band OFDM systems. IET Commun. 2019, 13, 12. [Google Scholar] [CrossRef]
- Bourgeois, P.; Imaike, T.; Goavec-Merou, G.; Rubiola, E. Noise in High-Speed Digital-to-Analog Converters. In Proceedings of the IEEE International Frequency Control Symposium and European Frequency and Time Forum, Denver, CO, USA, 12–16 April 2015; pp. 672–675. [Google Scholar]
- Walden, R.H. Analog-to-digital converter survey and analysis. IEEE J. Sel. Areas Commun. 1999, 17, 539–550. [Google Scholar] [CrossRef] [Green Version]
- Larsson, E.G.; Edfors, O.; Tufvesson, F.; Marzetta, T.L. Massive MIMO for next generation wireless systems. IEEE Commun. Mag. 2014, 52, 2. [Google Scholar] [CrossRef] [Green Version]
- Wu, B.; Chang, M.P.; Shastri, B.J.; Wang, Z.; Prucnal, P.R. Analog noise protected optical encryption with two-dimensional key space. Opt. Express 2014, 22, 14568. [Google Scholar] [CrossRef]
- Yang, Q.; Wu, B. Photonic Interference Cancellation with Free-Space Optical Link for Cellular Networks. In Frontiers in Optics; Optical Society of America: Washington, DC, USA, 2020; p. FTh5E.2. [Google Scholar]
- Yang, Q.; Wu, B. Radio Frequency Spectrum Control Based on Wideband Jamming and Photonic Jamming Cancellation. In Laser Science; Optical Society of America: Washington, DC, USA, 2019; p. JW3A.65. [Google Scholar]
- Wu, B.; Chang, M.P.; Wang, Z.; Shastri, B.J.; Prucnal, P.R. Optical Encryption based on Cancellation of Analog Noise. In Proceedings of the CLEO—Laser Science to Photonic Applications, San Jose, CA, USA, 8–13 June 2014; p. AW3P. [Google Scholar]
- Chang, M.P.; Wang, N.; Wu, B.; Prucnal, P.R. A Simultaneous Variable Optical Weight and Delay in a Semiconductor Optical Amplifier for Microwave Photonics. J. Light. Technol. 2015, 33, 2120–2126. [Google Scholar] [CrossRef]
- Chang, M.P.; Lee, C.L.; Wu, B.; Prucnal, P.R. Adaptive optical self-interference cancellation using a semiconductor optical amplifier. IEEE Photonics Technol. Lett. 2015, 27, 1018–1021. [Google Scholar] [CrossRef]
- Tait, A.N.; Nahmias, M.A.; Shastri, B.J.; Chang, M.P.; Wu, A.X.; Zhou, E.; Blow, E.C.; Ferreira de Lima, T.; Wu, B.; Prucnal, P.R. Balanced WDM weight banks for analog optical processing and networking in silicon. IEEE Summer Top. Meet. Ser. 2015, 3, 21–22. [Google Scholar]
- Shi, T.; Veneziano, N.; Qi, Y.; Chen, H.; Wu, B. Photonic Weight Banks based on Multimode Fiber for Neuromorphic Networks. In Frontiers in Optics; Optical Society of America: Washington, DC, USA, 2020; p. FM7D.2. [Google Scholar]
- Hyvärinen, A.; Oja, E. Independent component analysis: Algorithms and applications. Neural Netw. 2000, 13, 411–430. [Google Scholar] [CrossRef] [Green Version]
- Tait, A.N.; de Lima, T.F.; Ma, P.Y.; Chang, M.P.; Nahmias, M.A.; Shastri, B.J.; Mittal, P.; Prucnal, P.R. Blind Source Separation in the Physical Layer. In Proceedings of the 52nd Annual Conference on Information Sciences and Systems (CISS), Princeton, NJ, USA, 21–23 March 2018; pp. 1–6. [Google Scholar]
- Shi, T.; Qi, Y.; Zhang, W.; Prucnal, P.; Li, J.; Wu, B. Wideband photonic blind source separation with optical pulse sampling. Opt. Express 2021, 29, 23. [Google Scholar]
- Belsley, D.A.; Kuh, E.; Welsch, R.E. Chapter the Condition Number. In Regression Diagnostics: Identifying Influential Data and Sources of Collinearity; John Wiley & Sons: Hoboken, NJ, USA, 2004. [Google Scholar]
- Liu, H.; Cheung, Y.M. A New Blind Separation Approach to Ill-Condition Mixed Sources. In Proceedings of the IEEE International Workshop on VLSI Design and Video Technology, Suzhou, China, 28–30 May 2005; pp. 133–136. [Google Scholar]
- Lin, C.H.; Bioucas Dias, J.M. New Theory for Unmixing ILL-Conditioned Hyperspectral Mixtures. In Proceedings of the IEEE Sensor Array and Multichannel Signal Processing Workshop, Sheffield, UK, 8–11 July 2018; pp. 430–434. [Google Scholar]
- Perotin, L.; Serizel, R.; Vincent, E.; Guérin, A. CRNN-based multiple DoA estimation using Ambisonics acoustic intensity features. IEEE Int. Conf. Acoust. Speech Signal Process. Proc. 2018, 12, 4. [Google Scholar] [CrossRef] [Green Version]
- Liu, H.L. Blind separation of ill-condition mixed sources based on generalized eigenvalue. Tien Tzu Hsueh Pao/Acta Electron. Sin. 2006, 34, 11. [Google Scholar]
- Zhou, G.; Xie, S.; Yang, Z.; Zhang, J. Nonorthogonal approximate joint diagonalization with well-conditioned diagonalizers. IEEE Trans. Neural Netw. 2009, 20, 11. [Google Scholar]
- Lee, I.; Jang, G.J. Independent vector analysis based on overlapped cliques of variable width for frequency-domain blind signal separation. EURASIP J. Adv. Signal Process. 2012, 1, 113. [Google Scholar] [CrossRef] [Green Version]
- Yang, S.; Li, J.; He, B. A Novel Interference Suppression Method in Spread Spectrum Communication based on Blind Source Separation. In Proceedings of the IEEE International Conference on Cognitive Computing, San Francisco, CA, USA, 2–7 July 2018. [Google Scholar]
- Kang, C.Y. Directional interference supression based on blind source separation with beamforming. Zidonghua Xuebao/Acta Autom. Sin. 2014, 40, 5. [Google Scholar]
- Li, C.; Zhu, L.; Xie, A.; Luo, Z. A Novel Blind Source Separation Algorithm and Performance Analysis of Weak Signal against Strong Interference in Passive Radar Systems. Int. J. Antennas Propag. 2016, 2016, 6203972. [Google Scholar] [CrossRef] [Green Version]
- Mitra, U.; Poor, H.V. Neural Network Techniques for Adaptive Multiuser Demodulation. IEEE J. Sel. Areas Commun. 1994, 12, 9. [Google Scholar] [CrossRef] [Green Version]
- Kang, C.; Zhang, X.; Fan, W.; Li, J. Suppressing directional interference combining frequency domain blind source separation with beamforming. Acta Acust. 2014, 39, 5. [Google Scholar]
- Baan, W.A.; Fridman, P.A.; Millenaar, R.P. Radio Frequency Interference Mitigation at the Westerbork Synthesis Radio Telescope: Algorithms, Test Observations, and System Implementation. Astron. J. 2004, 128, 2. [Google Scholar] [CrossRef] [Green Version]
- Akeret, J.; Chang, C.; Lucchi, A.; Refregier, A. Radio frequency interference mitigation using deep convolutional neural networks. Astron. Comput. 2017, 18, 35–39. [Google Scholar] [CrossRef] [Green Version]
- Leshem, A.; van der Veen, A.; Boonstra, A. Multichannel Interference Mitigation Techniques in Radio Astronomy. Astrophys. J. Suppl. Ser. 2000, 131, 1. [Google Scholar] [CrossRef]
- Umar, R.; Abidin, Z.Z.; Ibrahim, Z.A.; Hassan, M.S.R.; Rosli, Z.; Hamidi, Z.S. Population Density Effect on Radio Frequencies Interference (RFI) in Radio Astronomy. In AIP Conference Proceedings; American Institute of Physics: College Park, MA, USA, 2011; Volume 1454, p. 1. [Google Scholar]
- Umar, R.; Abidin, Z.Z.; Ibrahim, Z.A.; Rosli, Z.; Noorazlan, N. Selection of radio astronomical observation sites and its dependence on human generated RFI. Res. Astron. Astrophys. 2014, 14, 2. [Google Scholar] [CrossRef] [Green Version]
- Kish, L.B. Stealth communication: Zero-power classical communication, zero-quantum quantum communication and environmental-noise communication. Appl. Phys. Lett. 2005, 87, 23. [Google Scholar] [CrossRef] [Green Version]
- Sheikholeslami, A.; Ghaderi, M.; Towsley, D.; Bash, B.A.; Guha, S.; Goeckel, D. Multi-Hop Routing in Covert Wireless Networks. IEEE Trans. Wirel. Commun. 2018, 17, 3656–3669. [Google Scholar] [CrossRef]
- Soltani, R.; Goeckel, D.; Towsley, D.; Houmansadr, A. Fundamental Limits of Covert Packet Insertion. IEEE Trans. Commun. 2020, 68, 3401–3414. [Google Scholar] [CrossRef] [Green Version]
- Bloch, M.R.; Guha, S. Optimal covert communications using pulse-position modulation. In Proceedings of the IEEE International Symposium on Information Theory, Aachen, Germany, 25–30 June 2017; pp. 2825–2829. [Google Scholar]
- Wu, B.; Chang, M.P.; Shastri, B.J.; Ma, P.Y.; Prucnal, P.R. Dispersion Deployment and Compensation for Optical Steganography Based on Noise. IEEE Photonics Technol. Lett. 2016, 28, 421–424. [Google Scholar] [CrossRef]
- Wu, B.; Shastri, B.J.; Prucnal, P.R. System performance measurement and analysis of optical steganography based on noise. IEEE Photonics Technol. Lett. 2014, 26, 1920–1923. [Google Scholar] [CrossRef]
- Wu, B.; Wang, Z.; Shastri, B.J.; Chang, M.P.; Frost, N.A.; Prucnal, P.R. Temporal phase mask encrypted optical steganography carried by amplified spontaneous emission noise. Opt. Express 2014, 22, 954. [Google Scholar] [CrossRef]
- Wu, B.; Tait, A.N.; Chang, M.P.; Prucnal, P.R. WDM optical steganography based on amplified spontaneous emission noise. Opt. Lett. 2014, 39, 5925–5928. [Google Scholar] [CrossRef]
- Wu, B.; Huang, Y.K.; Zhang, S.; Shastri, B.J.; Prucnal, P.R. Long range secure key distribution over multiple amplified fiber spans based on environmental instabilities. CLEO 2016, 20, 4–5. [Google Scholar]
- Qi, Y.; Wu, B. Free-Space Optical Stealth Communication based on Wideband Noise. In Frontiers in Optics; Optical Society of America: Washington, DC, USA, 2018; p. FW5B.5. [Google Scholar]
- Wu, B.; Chang, M.P.; Shastri, B.J.; Tait, A.N.; Prucnal, P.R. Compact Optical Steganography based on Amplified Spontaneous Emission Noise. In Proceedings of the IEEE Photonics Conference, Reston, VA, USA, 4–8 October 2015; p. MG1.3. [Google Scholar]
- Wu, B.; Chang, M.P.; Caldwell, N.R.; Caldwell, M.E.; Prucnal, P.R. Amplifier Noise Based Optical Steganography with Coherent Detection. Coherent Phenom. 2015, 2, 13–18. [Google Scholar] [CrossRef]
- Wu, B.; Wang, Z.; Shastri, B.J.; Tian, Y.; Prucnal, P.R. Two Dimensional Encrypted Optical Steganography Based on Amplified Spontaneous Emission Noise. In CLEO Applications and Technology; Optical Society of America: Washington, DC, USA, 2013; Volume 21, p. AF1H.5. [Google Scholar]
- Wu, B.; Wang, Z.; Shastri, B.J.; Tian, Y.; Prucnal, P.R. Phase-Mask Covered Optical Steganography based on Amplified Spontaneous Emission Noise. In Proceedings of the IEEE Photonics Conference, Bellevue, WA, USA, 8–12 September 2013; pp. 137–138. [Google Scholar]




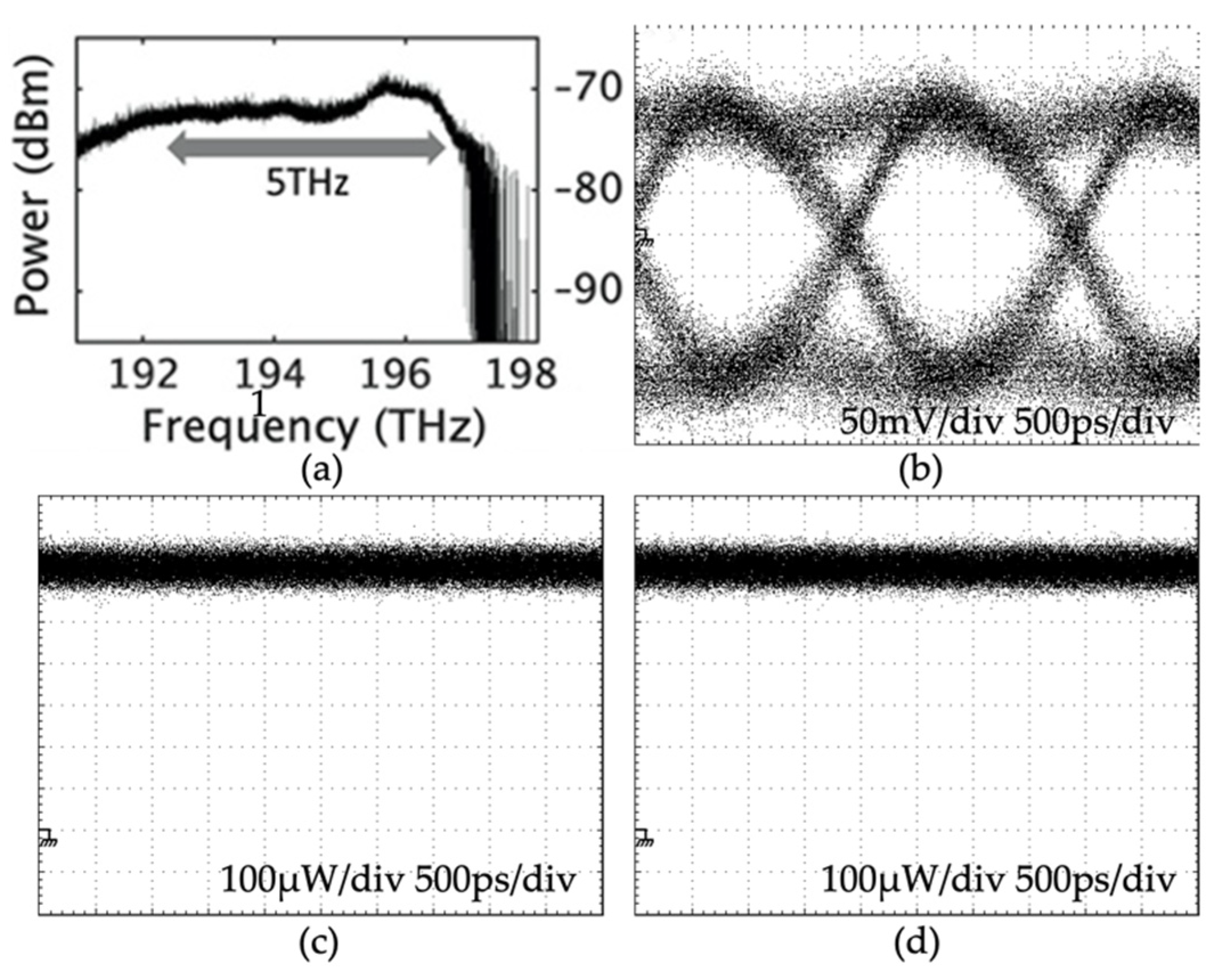

| Interference Bandwidth | Cancellation Ratio |
|---|---|
| 200 MHz | 60 dB |
| 1 GHz | 36 dB |
| 3 GHz | 30 dB |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Qi, Y.; Shi, T.; Wu, B. Wideband Mixed Signal Separation Based on Photonic Signal Processing. Telecom 2021, 2, 413-429. https://doi.org/10.3390/telecom2040024
Qi Y, Shi T, Wu B. Wideband Mixed Signal Separation Based on Photonic Signal Processing. Telecom. 2021; 2(4):413-429. https://doi.org/10.3390/telecom2040024
Chicago/Turabian StyleQi, Yang, Taichu Shi, and Ben Wu. 2021. "Wideband Mixed Signal Separation Based on Photonic Signal Processing" Telecom 2, no. 4: 413-429. https://doi.org/10.3390/telecom2040024
APA StyleQi, Y., Shi, T., & Wu, B. (2021). Wideband Mixed Signal Separation Based on Photonic Signal Processing. Telecom, 2(4), 413-429. https://doi.org/10.3390/telecom2040024






