Modeling Transmission Dynamics of Tuberculosis–HIV Co-Infection in South Africa
Abstract
:1. Introduction
2. Materials and Methods
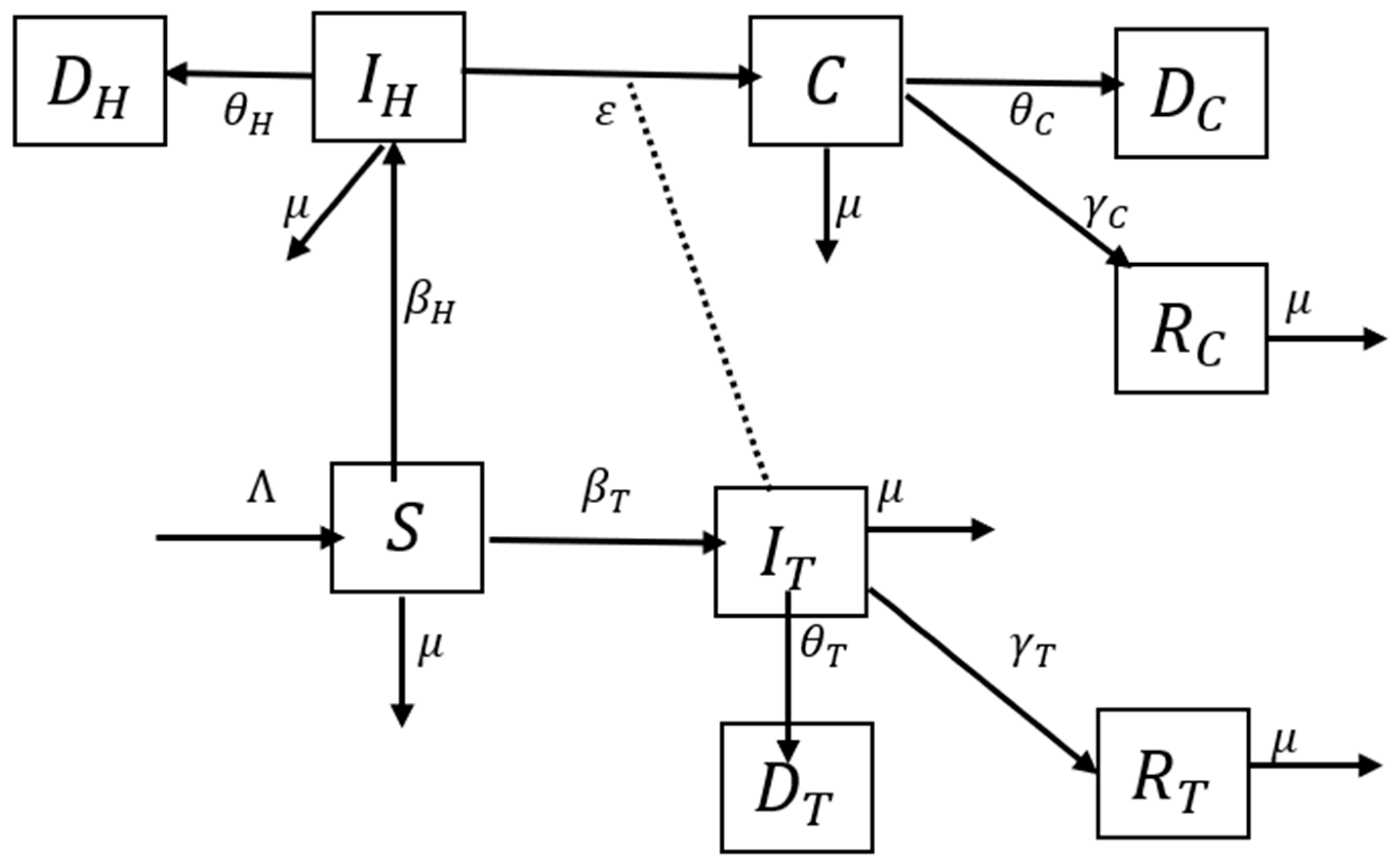
2.1. Model Specification
2.2. Parameter Estimation
2.3. Disease-Free Equilibrium
2.4. Basic Reproduction Number
3. Results
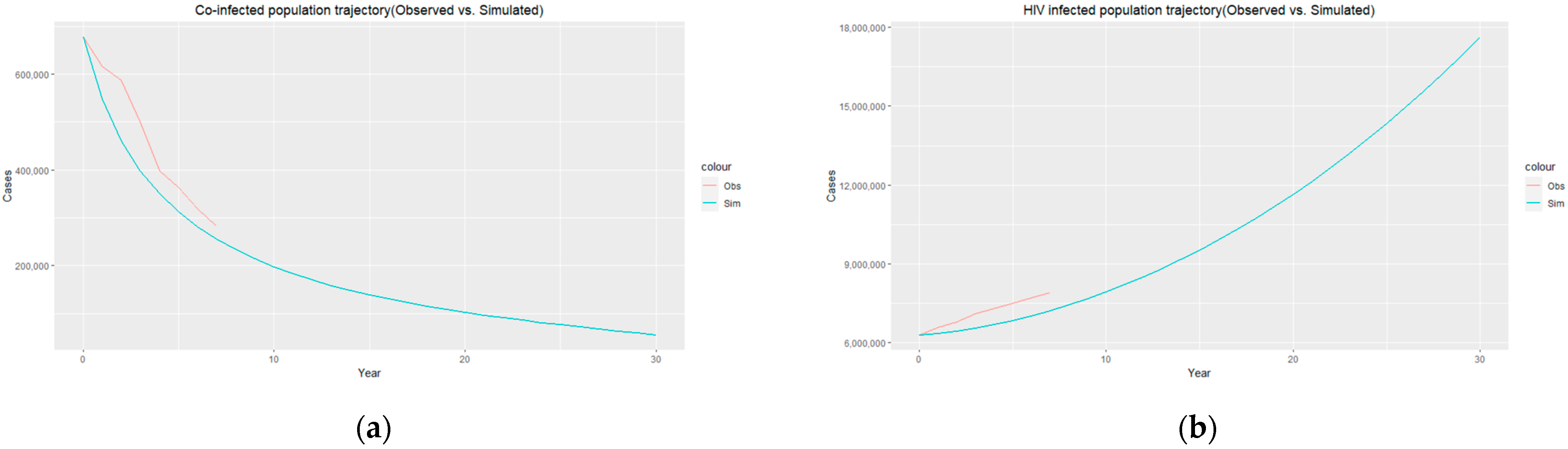
3.1. Parameter Estimates
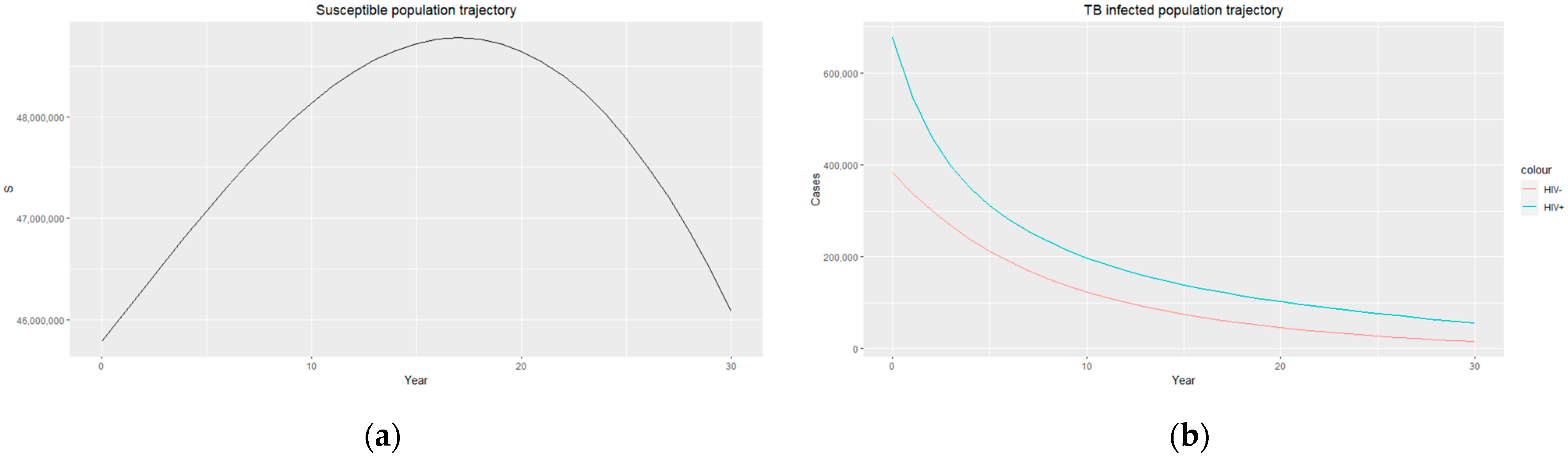
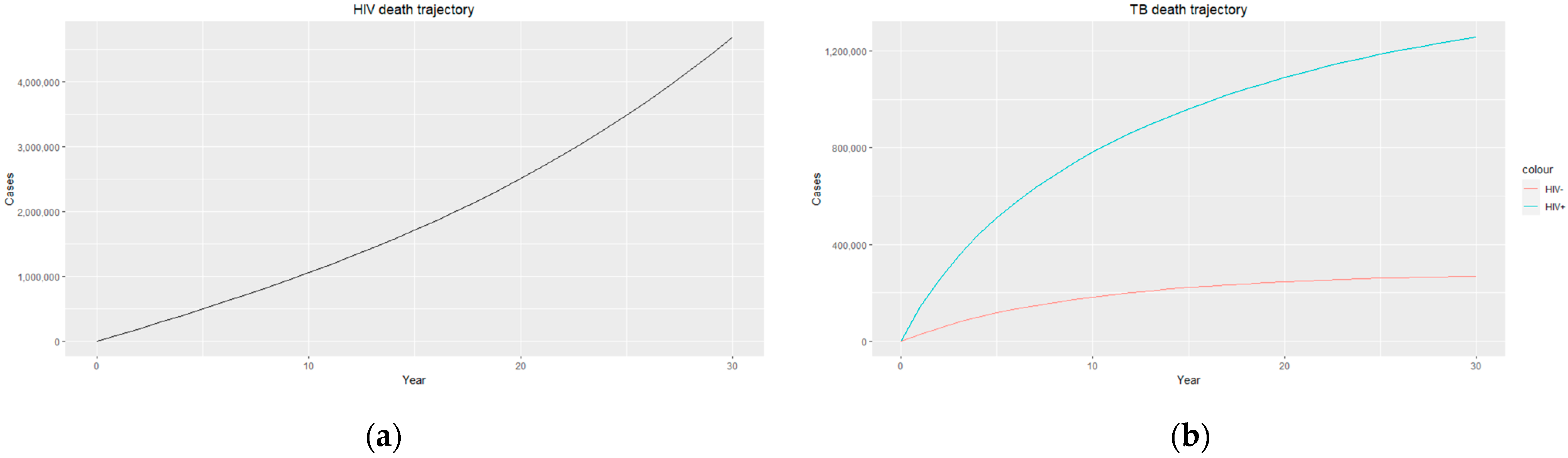
3.2. Numerical Simulation
4. Discussion
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- World Health Organization. Global Tuberculosis Control: Epidemiology, Planning, Financing: WHO Report; World Health Organization: Geneva, Switzerland, 2009; Available online: https://apps.who.int/iris/handle/10665/44035 (accessed on 10 April 2023).
- Vassal, A. South Africa Perspective: Tuberculosis. Copenhagen Consensus Center 2015. Available online: https://www.copenhagenconsensus.com/publication/south-africa-perspective-tuberculosis (accessed on 10 April 2023).
- Otiende, V.; Achia, T.; Mwambi, H. Bayesian modeling of spatiotemporal patterns of TB-HIV co-infection risk in Kenya. BMC Infect. Dis. 2019, 19, 902. [Google Scholar] [CrossRef] [PubMed]
- Mekonen, K.G.; Balcha, S.F.; Obsu, L.L.; Hassen, A. Mathematical Modeling and Analysis of TB and COVID-19 Coinfection. J. Appl. Math. 2022, 2022, 2449710. [Google Scholar] [CrossRef]
- Mukandavire, Z.; Gumel, A.B.; Garira, W.; Tchuenche, J.M. Mathematical analysis of a model for HIV-malaria co-infection. Math. Biosci. Eng. 2009, 6, 333–362. [Google Scholar] [CrossRef] [PubMed]
- Brauer, F. Mathematical epidemiology is not an oxymoron. BMC Public Health 2009, 9, S1–S2. [Google Scholar] [CrossRef] [PubMed]
- Aparicio, J.P.; Capurro, A.F.; Castillo-Chavez, C. Long-term dynamics and re-emergence of tuberculosis. In Mathematical Approaches for Emerging and Reemerging Infectious Diseases: An Introduction; Springer: Berlin/Heidelberg, Germany, 2002; pp. 351–360. [Google Scholar]
- Aparicio, J.P.; Castillo-Chávez, C. Mathematical modelling of tuberculosis epidemics. Math. Biosci. Eng. 2009, 6, 209–237. [Google Scholar] [CrossRef] [PubMed]
- Kermack, W.O.; McKendrick, A.G. Contributions to the mathematical theory of epidemics—I. Bull. Math. Biol. 1991, 53, 33–55. [Google Scholar] [CrossRef] [PubMed]
- Awoke, T.D.; Kassa, S.M. Optimal Control Strategy for TB-HIV/AIDS Co-Infection Model in the Presence of Behaviour Modification. Processes 2018, 6, 48. [Google Scholar] [CrossRef]
- Azeez, A.; Ndege, J.; Mutambayi, R.; Qin, Y. A Mathematical Model for TB/HIV Coinfection Treatment and Transmission Mechanism. Asian J. Math. Comput. Res. 2017, 22, 180–192. [Google Scholar]
- Ali, S.; Raina, A.A.; Iqbal, J.; Mathur, R.; López-Bonilla, J.L. Mathematical Modeling and Stability Analysis of HIV/AIDS-TB Co-infection. Palest. J. Math. 2009, 8, 380–391. [Google Scholar]
- Joyce, K.; Manyonge, N.A. Mathematical Modelling of Tuberculosis as an Opportunistic Respiratory Co-Infection in HIV/AIDS in the Presence of Protection. Appl. Math. Sci. 2009, 9, 5215–5233. [Google Scholar]
- Agusto, F.; Adekunle, A. Optimal control of a two-strain tuberculosis-HIV/AIDS co-infection model. Biosystems 2014, 119, 20–44. [Google Scholar] [CrossRef]
- Kaur, N.; Ghosh, M.; Bhatia, D. HIV-TB co-infection: A simple mathematical model. J. Adv. Res. Dyn. Control. Syst. 2015, 7, 66–81. [Google Scholar]
- Zhang, L.; Rahman, M.; Arfan, M.; Ali, A. Investigation of mathematical model of transmission co-infection TB in HIV com-munity with a non-singular kernel. Results Phys. 2021, 28, 104559. [Google Scholar] [CrossRef]
- Inayaturohmat, F.; Anggriani, N.; Supriatna, A.K. A mathematical model of tuberculosis and COVID-19 coinfection with the effect of isolation and treatment. Front. Appl. Math. Stat. 2022, 8, 95808. [Google Scholar] [CrossRef]
- Ganatra, S.R.; Bucşan, A.N.; Alvarez, X.; Kumar, S.; Chatterjee, A.; Quezada, M.; Fish, A.I.; Singh, D.K.; Singh, B.; Sharan, R.; et al. Antiretroviral therapy does not reduce tuberculosis reactivation in a tuberculosis-HIV coinfection model. J. Clin. Investig. 2020, 130, 5171–5179. [Google Scholar] [CrossRef] [PubMed]
- Letang, E.; Ellis, J.; Naidoo, K.; Casas, E.C.; Sánchez, P.; Hassan-Moosa, R.; García-Basteiro, A.L. Tuberculosis-HIV co-infection: Progress and challenges after two decades of global antiretroviral treatment roll-out. Arch. Bronconeumol. 2020, 56, 446–454. [Google Scholar] [CrossRef] [PubMed]
- Bruchfeld, J.; Correia-Neves, M.; Källenius, G. Tuberculosis and HIV Coinfection; Cold Spring Harbor Laboratory Press: New York, NY, USA, 2015; p. a017871. [Google Scholar]
- van der Werf, M.J.; Ködmön, C.; Zucs, P.; Hollo, V.; Amato-Gauci, A.J.; Pharris, A. Tuberculosis and HIV coinfection in Europe: Looking at one reality from two angles. AIDS 2016, 30, 2845. [Google Scholar] [CrossRef] [PubMed]
- van den Driessche, P.; Watmough, J. Reproduction numbers and sub-threshold endemic equilibria for compartmental models of disease transmission. Math. Biosci. 2002, 180, 29–48. [Google Scholar] [CrossRef]
- Blubaugh, D.J.; Garira, W.; Mukandavire, Z. Modeling HIV/AIDS and Tuberculosis Coinfection. Bull. Math. Biol. 2009, 71, 1745–1780. [Google Scholar] [CrossRef]
- Silva, C.J.; Torres, D.F.M. A TB-HIV/AIDS coinfection model and optimal control treatment. Discret. Contin. Dyn. Syst. A 2015, 35, 4639–4663. [Google Scholar] [CrossRef]


| Year | TB | TB–HIV Co-Infection | HIV |
|---|---|---|---|
| 2012 | 383,000 | 677,000 | 6,300,000 |
| 2013 | 383,000 | 615,000 | 6,600,000 |
| 2014 | 377,000 | 588,000 | 6,800,000 |
| 2015 | 382,000 | 500,000 | 7,100,000 |
| 2016 | 273,000 | 398,000 | 7,300,000 |
| 2017 | 237,000 | 363,000 | 7,500,000 |
| 2018 | 223,000 | 319,000 | 7,700,000 |
| 2019 | 203,000 | 282,000 | 7,900,000 |
| 2020 | 132,000 | 313,000 | 8,000,000 |
| Year | TB Death | TB–HIV Co-Infection Death | HIV Death |
|---|---|---|---|
| 2012 | 23,000 | 131,000 | 160,000 |
| 2013 | 22,000 | 117,000 | 130,000 |
| 2014 | 21,000 | 111,000 | 120,000 |
| 2015 | 21,000 | 100,000 | 110,000 |
| 2016 | 22,000 | 90,000 | 100,000 |
| 2017 | 22,000 | 78,000 | 94,000 |
| 2018 | 22,000 | 72,000 | 81,000 |
| 2019 | 23,000 | 67,000 | 72,000 |
| 2020 | 23,000 | 66,000 | 67,000 |
| Year | TB Recovery | TB–HIV Recovery |
|---|---|---|
| 2012 | 105,000 | 148,000 |
| 2013 | 107,000 | 145,000 |
| 2014 | 108,000 | 140,000 |
| 2015 | 104,000 | 133,000 |
| 2016 | 87,000 | 107,000 |
| 2017 | 84,000 | 101,000 |
| 2018 | 99,000 | 63,000 |
| 2019 | 94,000 | 77,000 |
| 2020 | 87,000 | 90,000 |
| Parameter | Estimated Value |
|---|---|
| Recruitment rate (λ) | |
| ) | |
| ) | |
| TB–HIV co-infection rate (ε) | |
| ) | |
| ) | |
| ) | |
| ) | |
| ) |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Adeyemo, S.; Sangotola, A.; Korosteleva, O. Modeling Transmission Dynamics of Tuberculosis–HIV Co-Infection in South Africa. Epidemiologia 2023, 4, 408-419. https://doi.org/10.3390/epidemiologia4040036
Adeyemo S, Sangotola A, Korosteleva O. Modeling Transmission Dynamics of Tuberculosis–HIV Co-Infection in South Africa. Epidemiologia. 2023; 4(4):408-419. https://doi.org/10.3390/epidemiologia4040036
Chicago/Turabian StyleAdeyemo, Simeon, Adekunle Sangotola, and Olga Korosteleva. 2023. "Modeling Transmission Dynamics of Tuberculosis–HIV Co-Infection in South Africa" Epidemiologia 4, no. 4: 408-419. https://doi.org/10.3390/epidemiologia4040036
APA StyleAdeyemo, S., Sangotola, A., & Korosteleva, O. (2023). Modeling Transmission Dynamics of Tuberculosis–HIV Co-Infection in South Africa. Epidemiologia, 4(4), 408-419. https://doi.org/10.3390/epidemiologia4040036






