Numerical Study on Free Convection in an Inclined Wavy Porous Cavity with Localized Heating
Abstract
1. Introduction
2. Mathematical Modeling and Formulation
3. Method of Solution
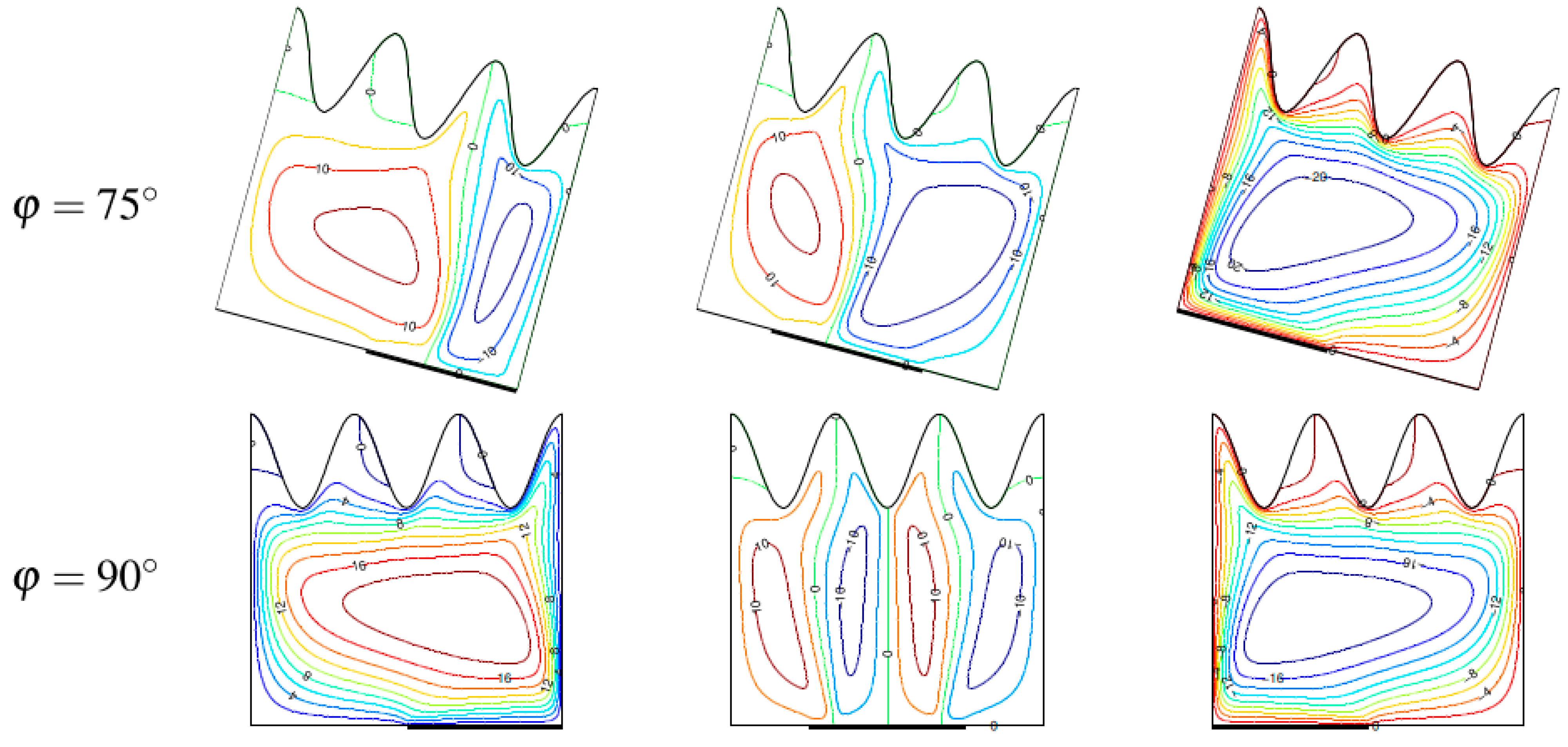
4. Results and Discussion
4.1. Thermal Distribution
4.2. Flow Field
4.3. Heat Transfer Rate
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Selimefendigil, F.; Oztop, H.F. Effects of corrugation on convective heat transfer and power generation in a wavy walled triangular cavity equipped with inclined elastic fin and piezo-energy harvester assembly. Case Stud. Therm. Eng. 2024, 62, 05126. [Google Scholar] [CrossRef]
- Roy, N.C.; Monira, S. Natural convection of a reacting hybrid nanofluid in an open porous cavity bounded by vertical wavy walls. Int. J. Numer. Methods Heat Fluid Flow 2023, 33, 3202–3227. [Google Scholar] [CrossRef]
- Pandit, S.; Mondal, M.K.; Sanyal, D.; Manna, N.K.; Biswas, N.; Mandal, D.K. Multi-segmental heating of facing vertical walls in porous systems filled with hybrid nanofluid in a constant-strength magnetic environment. Int. J. Numer. Methods Heat Fluid Flow 2024, 34, 1658–1698. [Google Scholar] [CrossRef]
- Biswas, N.; Mandal, D.K.; Manna, N.K.; Gorla, R.S.R.; Chamkha, A.J. Magneto hydrodynamic thermal characteristics of water-based hybrid nanofluid-filled non-Darcian porous wavy enclosure: Effect of undulation. Int. J. Numer. Methods Heat Fluid Flow 2022, 32, 1742–1777. [Google Scholar] [CrossRef]
- Alhashash, A.; Saleh, H. Unsteady free convection in a composite enclosure having flexible wall. Adv. Mech. Eng. 2023, 15, 16878132231167947. [Google Scholar] [CrossRef]
- Cheng, H.-S.; Liu, D.; Hu, J.-T.; Zhao, F.-Y.; Wang, H.-Q. Double-diffusive mixed convection in the slot ventilated enclosure with different arrangements of supplying air flow ports. Adv. Mech. Eng. 2015, 7, 1687814015573771. [Google Scholar] [CrossRef]
- Shehata, A.I.; Dawood, M.M.K.; Amer, M.; William, M.A. Enhancement of mixed convection in a lid driven enclosure based on magnetic field presence with nanofluid. Adv. Mech. Eng. 2023, 15, 1–13. [Google Scholar] [CrossRef]
- Murthy, P.V.S.N.; Rathish Kumar, B.V.; Singh, P. Natural convection heat transfer from a horizontal wavy surface in a porous enclosure. Numer. Heat Transf. A 1997, 31, 207–221. [Google Scholar] [CrossRef]
- Rathish Kumar, B.V. A study of free convection induced by a vertical wavy surface with heat flux in a porous enclosure. Numer. Heat Transf. A 2000, 37, 493–510. [Google Scholar] [CrossRef]
- Rathish Kumar, B.V.; Shalini. Free convection in a non-Darcian wavy porous enclosure. Int. J. Eng. Sci. 2003, 41, 1827–1848. [Google Scholar] [CrossRef]
- Rathish Kumar, B.V.; Shalini. Natural convection in a thermally stratified wavy vertical porous enclosure. Numer. Heat Transf. A 2003, 43, 753–776. [Google Scholar] [CrossRef]
- Rathish Kumar, B.V.; Shalini. Double diffusive natural convection in a doubly stratified wavy porous enclosure. Appl. Math Comput. 2005, 171, 180–202. [Google Scholar]
- Khanafer, K.; Al-Azmi, B.; Marafie, A.; Pop, I. Non-Darcian effects on natural convection heat transfer in a wavy porous enclosure. Int. J. Heat Mass Transf. 2009, 52, 1887–1896. [Google Scholar] [CrossRef]
- Bhardwaj, S.; Dalal, A. Effect of undulations on the natural convection heat transfer and entropy generation inside a porous right-angled triangular enclosure. Numer. Heat Transf. A 2015, 67, 972–991. [Google Scholar] [CrossRef]
- Bhardwaj, S.; Dalal, A.; Pati, S. Influence of wavy wall and non-uniform heating on natural convection heat transfer and entropy generation inside porous complex enclosure. Energy 2015, 79, 467–481. [Google Scholar] [CrossRef]
- Salah, T.; Mansour, M.A.; Rashad, A.M.; HossamA, N.; Jakeer, S. Analyzing geometric parameters in an inclined wavy-porous cavity filled with magnetic hybrid nanofluid containing a square solid block. Prog. Nucl. Energy 2024, 171, 105159. [Google Scholar]
- Sayyou, H.; Belabid, J.; Öztop, H.F.; Allali, K. Effects of vibration on natural convection in a square inclined porous enclosure filled with Cu-water nanofluid. Int. J. Numer. Methods Heat Fluid Flow 2024, 34, 2399–2428. [Google Scholar] [CrossRef]
- Sivaraj, P.; Chinnasamy, S. Magneto-thermal convection and entropy production of hybrid nanofluid in an inclined chamber having a solid block. Int. J. Numer. Methods Heat Fluid Flow 2024, 34, 773–808. [Google Scholar] [CrossRef]
- Adjlout, L.; Imine, O.; Azzi, A.; Belkadi, M. Laminar natural convection in an inclined cavity with a wavy wall. Int. J. Heat Mass Transf. 2002, 45, 2141–2152. [Google Scholar] [CrossRef]
- Sabeur-Bendehina, A.; Imine, O.; Adjlout, L. Laminar free convection in undulated cavity with non-uniform boundary conditions. Comptes Rendus Mec. 2011, 339, 42–57. [Google Scholar] [CrossRef]
- Varol, Y.; Oztop, H.F. Buoyancy induced heat transfer and fluid flow inside a tilted wavy solar collector. Build Environ. 2007, 42, 2062–2071. [Google Scholar] [CrossRef]
- Varol, Y.; Oztop, H.F. A comparative numerical study on natural convection in inclined wavy and flat-plate solar collectors. Build Environ. 2008, 43, 1535–1544. [Google Scholar] [CrossRef]
- Mekroussi, S.; Nehari, D.; Bouzit, M.; Chemloul, N.-E.S. Analysis of mixed convection in an inclined lid-driven cavity with a wavy wall. J. Mech. Sci. Technol. 2013, 27, 2191. [Google Scholar] [CrossRef]
- Bourich, M.; Hasnaoui, M.; Amahmid, A. Double-diffusive natural convection in a porous enclosure partially heated from below and differentially salted. Int. J. Heat Fluid Flow 2004, 25, 1034–1046. [Google Scholar] [CrossRef]
- Zhao, F.-Y.; Liu, D.; Tang, G.-F. Natural convection in a porous enclosure with partial heating and salting element. Int. J. Therm. Sci. 2008, 47, 569–583. [Google Scholar] [CrossRef]
- Varol, Y.; Oztop, H.F.; Avci, E. Estimation of thermal and flow fields due to natural convection using support vector machines (SVM) in a porous cavity with discrete heat sources. Int. Common Heat Mass Transf. 2008, 35, 928–936. [Google Scholar] [CrossRef]
- Bhuvaneswari, M.; Sivasankaran, S.; Kim, Y.J. Effect of aspect ratio on natural convection in a porous enclosure with partially active thermal walls. Comput. Math Appl. 2011, 62, 3844–3856. [Google Scholar] [CrossRef]
- Sivasankaran, S.; Do, Y.; Sankar, M. Effect of discrete heating on natural convection in a rectangular porous enclosure. Transp. Porous Media 2011, 86, 261–281. [Google Scholar] [CrossRef]
- Nguyen, M.T.; Aly, A.M.; Lee, S.-W. Unsteady natural convection heat transfer in a nanofluid-filled square cavity with various heat source conditions. Adv. Mech. Eng. 2016, 8, 1687814016646547. [Google Scholar] [CrossRef]
- Benyoucef, D.; Noui, S.; Djaraoui, A. Numerical study of natural convection nanofluids flow in tilted cavities with a partially thermally heat source. Adv. Mech. Eng. 2021, 13, 16878140211060682. [Google Scholar] [CrossRef]
- Sun, Q.; Pop, I. Free convection in a titled triangle porous cavity filled with Cu-water nanofluid with flush mounted heater on the wall. Int. J. Numer. Methods Heat Fluid Flow 2014, 24, 2–20. [Google Scholar] [CrossRef]
- Ahmed, S.E.; Oztop, H.F.; Al-Salem, K. Natural convection coupled with radiation heat transfer in an inclined porous cavity with corner heater. Comput. Fluids 2014, 102, 74–84. [Google Scholar] [CrossRef]
- Cho, C.-C. Heat transfer and entropy generation of natural convection in nanofluid-filled square cavity with partially-heated wavy surface. Int. J. Heat Mass Transf. 2014, 77, 818–827. [Google Scholar] [CrossRef]
- Hansda, S. Effect of thermal radiation and inclined magnetic field on thermosolutal mixed convection in a partially active wavy trapezoidal enclosure filled with hybrid nanofluid. Int. J. Thermofluids 2024, 24, 100888. [Google Scholar] [CrossRef]
- Alloui, Z.; Dufau, L.; Beji, H.; Vasseur, P. Multiple steady states in a porous enclosure partially heated and fully salted from below. Int. J. Therm. Sci. 2009, 48, 521–534. [Google Scholar] [CrossRef]





| Grid Size | ||
|---|---|---|
| A = 0, λ = 0 | A = 0.25, λ = 5 | |
| 60 × 60 | 16.9241 | 18.9059 |
| 100 × 100 | 18.7268 | 20.3882 |
| 140 × 140 | 19.5645 | 21.1107 |
| 150 × 140 | 19.6678 | 21.1786 |
| 150 × 160 | 19.7478 | 21.2945 |
| 150 × 200 | 19.8642 | 21.4658 |
| 160 × 160 | 19.8394 | 21.3554 |
| 200 × 200 | 20.2404 | 21.7189 |
| 300 × 300 | 20.8197 | 22.2612 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sivanandam, S.; Cheong, H.T.; Thangaraj, A. Numerical Study on Free Convection in an Inclined Wavy Porous Cavity with Localized Heating. Modelling 2025, 6, 30. https://doi.org/10.3390/modelling6020030
Sivanandam S, Cheong HT, Thangaraj A. Numerical Study on Free Convection in an Inclined Wavy Porous Cavity with Localized Heating. Modelling. 2025; 6(2):30. https://doi.org/10.3390/modelling6020030
Chicago/Turabian StyleSivanandam, Sivasankaran, Huey Tyng Cheong, and Aasaithambi Thangaraj. 2025. "Numerical Study on Free Convection in an Inclined Wavy Porous Cavity with Localized Heating" Modelling 6, no. 2: 30. https://doi.org/10.3390/modelling6020030
APA StyleSivanandam, S., Cheong, H. T., & Thangaraj, A. (2025). Numerical Study on Free Convection in an Inclined Wavy Porous Cavity with Localized Heating. Modelling, 6(2), 30. https://doi.org/10.3390/modelling6020030







