Global Buckling Simulation and Design of a Novel Concrete-Filled Corrugated Steel Tubular Column
Abstract
1. Introduction
2. Establishment and Validation of FE Models
2.1. FE Model Establishment
2.2. FE Model Validation
2.2.1. Sensitivity Analysis
2.2.2. Comparison Between FE Models and Tests
3. Numerical Results
4. Parametric Analysis
4.1. Effect of Widths of Columns and Steel Bars
4.2. Effect of Corrugation Dimensions
4.3. Effect of Material Strengths
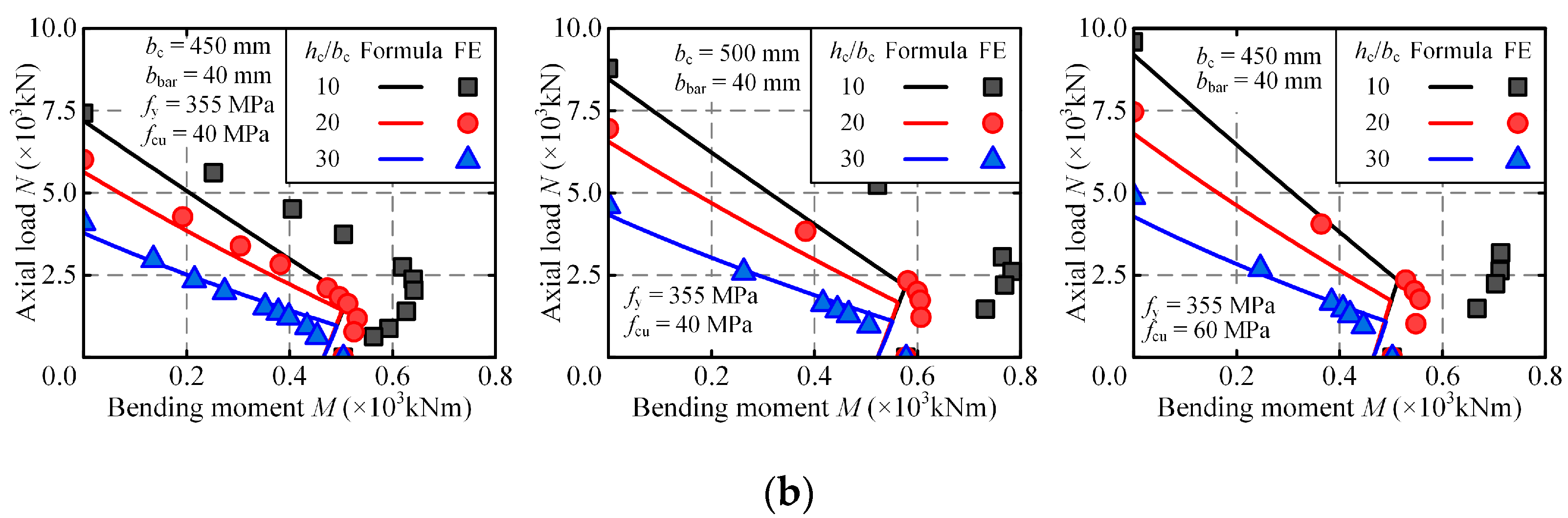
5. Design Curves of CFCST Columns
6. Conclusions
- The finite element (FE) model was built and validated through existing test results, demonstrating that the FE model could be used in analyzing the stability performance of the CFCST column.
- The N–M interaction curve was convex at small height-to-width ratios, which was close to a straight line as the ratio increased. Numerical analysis indicated that the contribution of concrete to the load-bearing capacity of the CFCST column decreased with the increasing eccentricity ratio.
- Parametric analysis indicated that increasing the column width and the concrete strength was more economical for enhancing the stability resistance of the column with small eccentricity ratios, while increasing the steel strength and the steel bar width was recommended for large eccentricity ratios. Additionally, the effects of corrugation amplitude and corrugated steel plate thickness on stability resistance were relatively small.
- Based on the FE results, a design formula to predict the stability resistance of the CFCST column under eccentric compression was proposed with acceptable accuracy and safety, which could be used for practical engineering applications.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Yu, C.Q.; Tong, J.Z.; Zhou, S.M.; Zhang, J.M.; Shen, J.J.; Zhang, L.; Tong, G.S.; Li, Q.H.; Xu, S.L. State-of-the-art review on steel-concrete composite walls. Sustain. Struct. 2024, 4, 000035. [Google Scholar] [CrossRef]
- Chen, Y.L.; Tong, J.Z.; Li, Q.H.; Xu, S.L.; Shen, L.M. Application of high-performance cementitious composites in steel–concrete composite bridge deck systems: A review. J. Intell. Constr. 2024, 2, 9180012. [Google Scholar] [CrossRef]
- Varma, A.H.; Malushte, S.R.; Sener, K.C.; Lai, Z.C. Steel-plate composite (SC) walls for safety related nuclear facilities: Design for in-plane forces and out-of-plane moments. Nucl. Eng. Des. 2014, 269, 240–249. [Google Scholar] [CrossRef]
- Li, P.P.; Jiang, J.F.; Li, Q.; Ren, Z.G. Axial compression performance and optimum design of round-cornered square CFST with high-strength materials. J. Build. Eng. 2023, 68, 106145. [Google Scholar] [CrossRef]
- Yang, S.L.; Zhang, L.; Zhang, J.W.; Fu, B.; Tong, G.S.; Jing, T. Seismic behavior of concrete-filled wide rectangular steel tubular (CFWRST) stub columns. J. Constr. Steel Res. 2022, 196, 107402. [Google Scholar] [CrossRef]
- Yang, Y.L.; Wang, G.J.; Yang, W.Q.; Wei, X.; Chen, Y.F. Experimental research on fire behavior of L-shaped CFST columns under axial compression. J. Constr. Steel Res. 2022, 198, 107505. [Google Scholar] [CrossRef]
- Wang, E.; Ding, F.X.; Wang, L.P.; Chen, Y.B.; Sheng, S.J.; Shen, J.T.; Lin, Q.T. Analytical study on the composite behavior of rectangular CFST columns under combined compression and torsion. Case Stud. Constr. Mater. 2022, 16, e01074. [Google Scholar] [CrossRef]
- Furlong, R.W. Strength of steel-encased concrete beam columns. J. Struct. Div. 1967, 93, 113–124. [Google Scholar] [CrossRef]
- Han, L.H.; Li, W.; Bjorhovde, R. Developments and advanced applications of concrete-filled steel tubular (CFST) structures: Members. J. Constr. Steel Res. 2014, 100, 211–228. [Google Scholar] [CrossRef]
- Li, W.J.; Li, W. Performance of spirally reinforced high-strength circular CFST column under eccentric compression. J. Constr. Steel Res. 2024, 216, 108576. [Google Scholar] [CrossRef]
- Medall, D.; Ibáñez, C.; Espinós, A.; Romero, M.L. Experimental and numerical study of high-strength materials on slender steel-reinforced CFST columns in fire. Thin Walled Struct. 2025, 209, 112904. [Google Scholar] [CrossRef]
- Zhang, W.H.; Wang, R.; Zhao, H.; Lam, D.; Chen, P. Axial-load response of CFST stub columns with external stainless steel and recycled aggregate concrete: Testing, mechanism analysis and design. Eng. Struct. 2022, 256, 113968. [Google Scholar] [CrossRef]
- Chen, M.H.; Yan, J.B. Compression behaviours of CFSTs using stainless-steel tubes at low temperatures. J. Constr. Steel Res. 2023, 211, 108120. [Google Scholar] [CrossRef]
- Zhi, D.; Xia, P.; Wang, S.Q.; Gong, F.Y.; Cao, W.L.; Wang, D.M.; Ueda, T. RBSM-based mesoscale study of mechanical properties and frost damage behaviors for recycled fine aggregate concrete. Constr. Build. Mater. 2024, 416, 135136. [Google Scholar] [CrossRef]
- Wang, S.Q.; Xia, P.; Gong, F.Y.; Zeng, Q.; Chen, K.Y.; Zhao, Y.X. Multi objective optimization of recycled aggregate concrete based on explainable machine learning. J. Clean. Prod. 2024, 445, 141045. [Google Scholar] [CrossRef]
- Yang, Z.M.; Chen, J.; Wang, J.; Zeng, C.Q. Axial compressive behavior of FRP-confined square CFST columns reinforced with internal latticed steel angles for marine structures. Eng. Struct. 2024, 319, 118847. [Google Scholar] [CrossRef]
- Zeng, J.J.; Zheng, Y.W.; Liu, F.; Guo, Y.C.; Hou, C. Behavior of FRP Ring-Confined CFST columns under axial compression. Compos. Struct. 2021, 257, 113166. [Google Scholar] [CrossRef]
- Uy, B. Local and postlocal buckling of fabricated steel and composite cross sections. J. Struct. Eng. 2001, 127, 666–677. [Google Scholar] [CrossRef]
- Liang, Q.Q.; Uy, B.; Richard Liew, J.Y. Nonlinear analysis of concrete-filled thin-walled steel box columns with local buckling effects. J. Constr. Steel Res. 2006, 62, 581–591. [Google Scholar] [CrossRef]
- Liang, Q.Q.; Uy, B.; Richard Liew, J.Y. Local buckling of steel plates in concrete-filled thin-walled steel tubular beam–columns. J. Constr. Steel Res. 2007, 63, 396–405. [Google Scholar] [CrossRef]
- Long, Y.L.; Zeng, L.; Gardner, L.; Wadee, M.A. A new model for calculating the elastic local buckling stress of steel plates in square CFST columns. Thin Walled Struct. 2022, 171, 108756. [Google Scholar] [CrossRef]
- Li, J.W.; Jia, C.; Gao, S.; Guo, L.H. Experimental and numerical study on axial compression behavior of slender CFST columns with localized pitting corrosion damage. Constr. Build. Mater. 2024, 414, 134858. [Google Scholar] [CrossRef]
- Zhang, L.; Mao, C.X.; Li, X.G.; Tong, G.S.; Jing, T.; Wang, Y.C. Experimental study on CFNRST members under combined compression and bending. J. Constr. Steel Res. 2020, 167, 105950. [Google Scholar] [CrossRef]
- Yan, Y.H.; Liang, H.J.; Lu, Y.Y.; Zhao, X.B. Slender CFST columns strengthened with textile-reinforced engineered cementitious composites under axial compression. Eng. Struct. 2021, 241, 112483. [Google Scholar] [CrossRef]
- Tong, J.Z.; Wu, R.M.; Wang, L.Q. Experimental and numerical investigations on seismic behavior of stiffened corrugated steel plate shear walls. Earthq. Eng. Struct. Dyn. 2023, 52, 3551–3574. [Google Scholar] [CrossRef]
- Xie, C.; Dou, C.; Ru, Y. Cyclic test and lateral resistant design of corrugated plate shear walls. Structures 2023, 49, 748–764. [Google Scholar] [CrossRef]
- Jiang, Z.Q.; Niu, Z.Y.; Zhang, A.L.; Liu, X.C. Design method of axial compression stability for cross-section corrugated plate steel special-shaped column. Thin Walled Struct. 2024, 194, 111243. [Google Scholar] [CrossRef]
- Tong, J.Z.; Zhang, J.M.; Yu, C.Q.; Tong, G.S.; Li, Q.H.; Xu, S.L. Seismic experiments and shear resistance prediction of multi-celled corrugated-plate CFST walls. Earthq. Eng. Struct. Dyn. 2024, 53, 1681–1704. [Google Scholar] [CrossRef]
- Fang, Y.; Yang, H.; Lu, B.; Wang, Y.Y.; Dong, J.C. Experimental investigation on shear-bending performances of concrete-filled thin-walled corrugated steel tubes. Eng. Struct. 2023, 274, 115147. [Google Scholar] [CrossRef]
- Fang, Y.; Wang, Y.Y.; Elchalakani, M.; Yang, H. Experimental investigation on concrete-filled corrugated steel tubular column under constant axial load and cyclic load. Eng. Struct. 2021, 248, 113245. [Google Scholar] [CrossRef]
- Yang, L.G.; Wang, Y.Y.; Liew, J.Y.R. Compression-bending strength model for corrugated steel tube confined reinforced concrete section. J. Struct. Eng. 2021, 147, 04021187. [Google Scholar] [CrossRef]
- Wang, Y.Y.; Yang, L.G.; Yang, H.; Liu, C.Y. Behaviour of concrete-filled corrugated steel tubes under axial compression. Eng. Struct. 2019, 183, 475–495. [Google Scholar] [CrossRef]
- Zou, Y.; Wang, L.; Sun, Z.X.; Pan, J.; Chen, M.; Wu, Y.C. Experimental and numerical studies of concrete-filled corrugated steel tubular column under axial compression. Eng. Struct. 2023, 276, 114813. [Google Scholar] [CrossRef]
- Sun, Z.X.; Zou, Y.; Wang, C.Q.; Pan, J.; Wang, L.; Chen, M. Study on confinement mechanism of core concrete in steel tubular-corrugated steel plate confined concrete columns. J. Build. Eng. 2022, 52, 104497. [Google Scholar] [CrossRef]
- Tong, J.Z.; Zhang, J.M.; Yu, C.Q.; Wu, R.M.; Tong, G.S.; Chen, M.; Gao, W. Experimental study on axial compressive behavior of concrete-filled corrugated steel tubular columns. Eng. Struct. 2024, 313, 118267. [Google Scholar] [CrossRef]
- Liao, X.; Li, X.; Zhao, S.D.; Yang, C.; Yin, D.D. Behavior of eccentrically loaded rectangular CFST components for shear walls using double horizontally-corrugated steel plates. Eng. Struct. 2024, 303, 117470. [Google Scholar] [CrossRef]
- Li, Y.D.; Yu, C.Q.; Zhu, H.C.; Tong, J.Z.; Tong, G.S.; Xiao, Z.B. Global stability design of concrete-filled corrugated steel tubular columns. Structures 2024, 62, 106149. [Google Scholar] [CrossRef]
- Yu, C.Q.; Tong, G.S.; Duan, S.J.; Chen, M.; Tong, J.Z. Combined axial and flexural behavior of concrete-filled corrugated steel tubular columns. J. Constr. Steel Res. 2024, 221, 108912. [Google Scholar] [CrossRef]
- Abaqus. Abaqus User’s Manual, Version 6.14; Dassault Systemes Simulia Corp.: Paris, France, 2014. [Google Scholar]
- Han, L.H.; Yao, G.H.; Tao, Z. Performance of concrete-filled thin-walled steel tubes under pure torsion. Thin Walled Struct. 2007, 45, 24–36. [Google Scholar] [CrossRef]
- Huang, Y.; Li, W.J.; Lu, Y.Y.; Liang, H.J.; Yan, Y.H. Behaviour of CFST slender columns strengthened with steel tube and sandwiched concrete jackets under axial loading. J. Build. Eng. 2022, 45, 103613. [Google Scholar] [CrossRef]
- Tao, Z.; Wang, Z.B.; Yu, Q. Finite element modelling of concrete-filled steel stub columns under axial compression. J. Constr. Steel Res. 2013, 89, 121–131. [Google Scholar] [CrossRef]
- ACI 318-19; Building Code Requirements for Structural Concrete (ACI 318-19) and Commentary (ACI 318R-19). American Concrete Institute: Farmington Hills, MI, USA, 2019.
- GB50010-2010; Code for Design of Concrete Structures. China Architecture & Building Press: Beijing, China, 2015. (In Chinese)
- Shen, J.; Wang, C.; Jiang, J. Finite Element Method of Reinforced Concrete and Limit Analysis of Plates and Shells; Tsinghua University Press: Beijing, China, 1993. [Google Scholar]
- Kang, J.X.; Zou, Y.; Wang, C.Q.; Wu, Y.C.; Chen, M. Study on behavior of corrugated plate-square concrete filled steel tubular columns under axial compression. J. Build. Struct. 2020, 41, 146–153. (In Chinese) [Google Scholar]
- Dou, C.; Xie, C.; Wang, Y.; Yang, N. Cyclic loading test and lateral resistant behavior of flat-corrugated steel plate shear walls. J. Build. Eng. 2023, 66, 105831. [Google Scholar] [CrossRef]
- Dou, C.; Ru, Y.; Jiang, Z.Q.; Wang, Y. Lateral resistant behavior of grid-reinforced steel corrugated shear walls. J. Struct. Eng. 2024, 150, 04024047. [Google Scholar] [CrossRef]
- AISC 360-16; Specification for Structural Steel Buildings. American Institute of Steel Construction: Chicago, IL, USA, 2016.
- GB 50936; Technical Code for Concrete Filled Steel Tubular Structures. China Architecture & Building Press: Beijing, China, 2014. (In Chinese)
- Tong, G.S. Design Method of Steel Structure and Steel-Concrete Composite Structure; China Architecture & Building Press: Beijing, China, 2022. [Google Scholar]
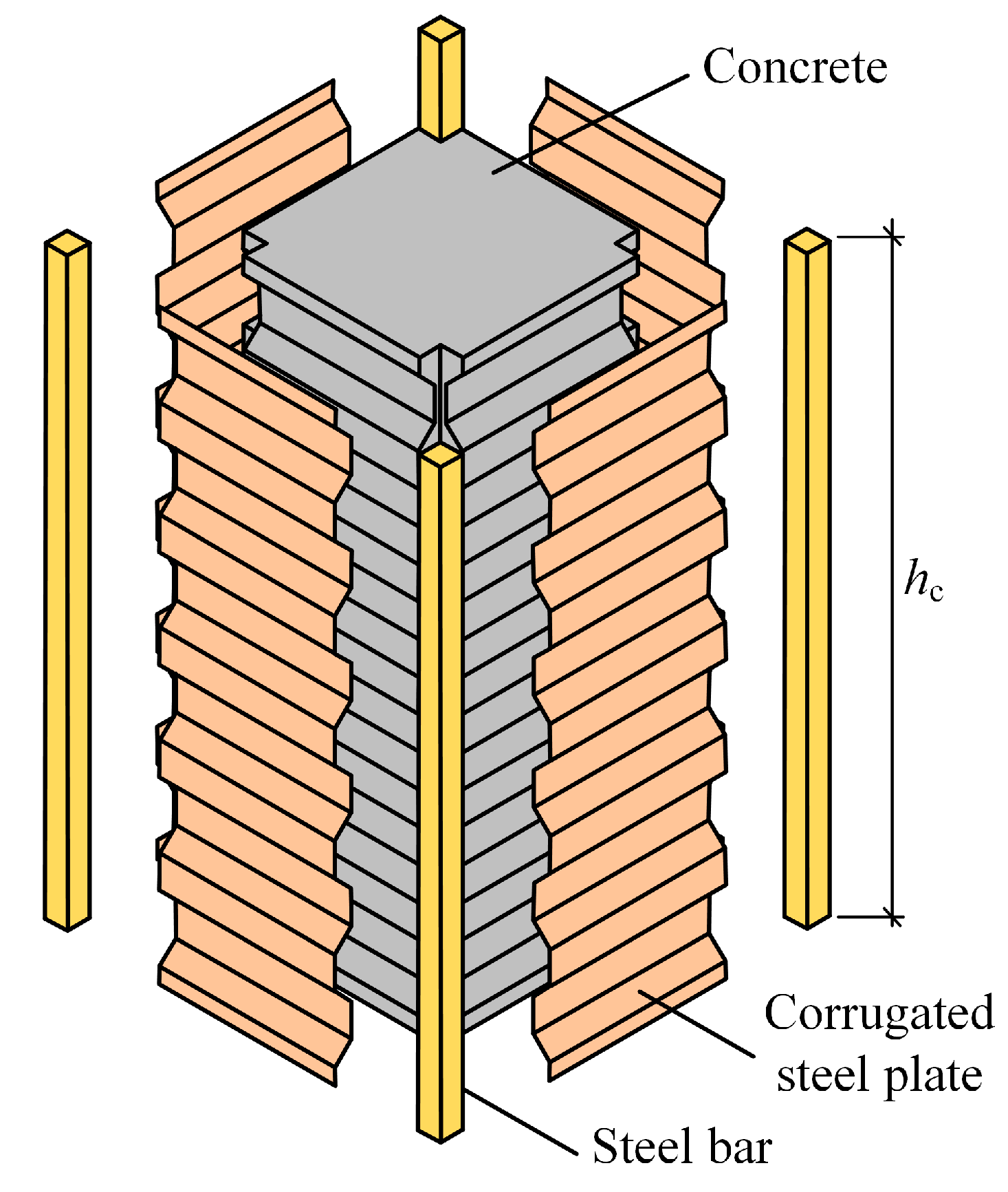



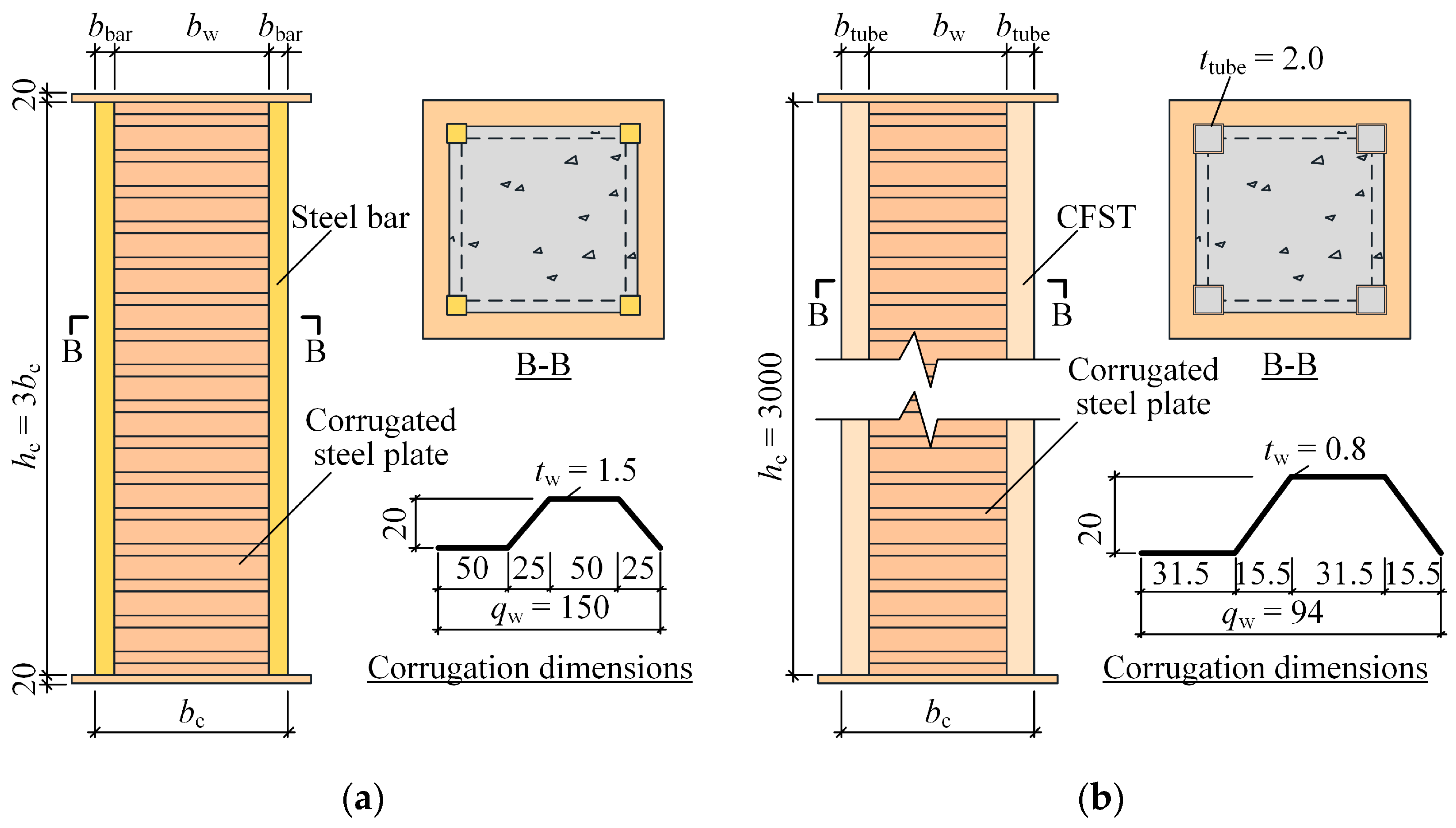

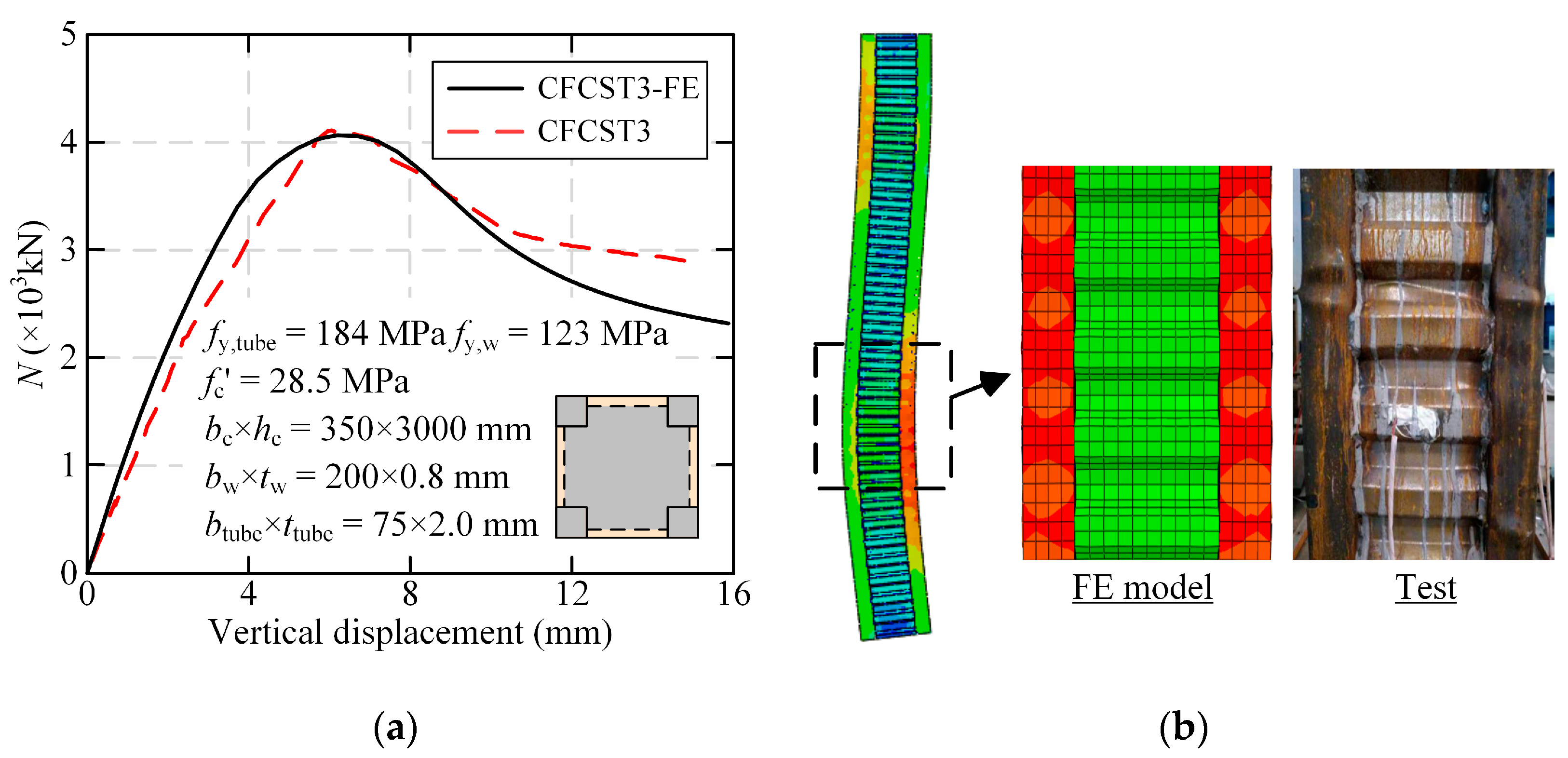

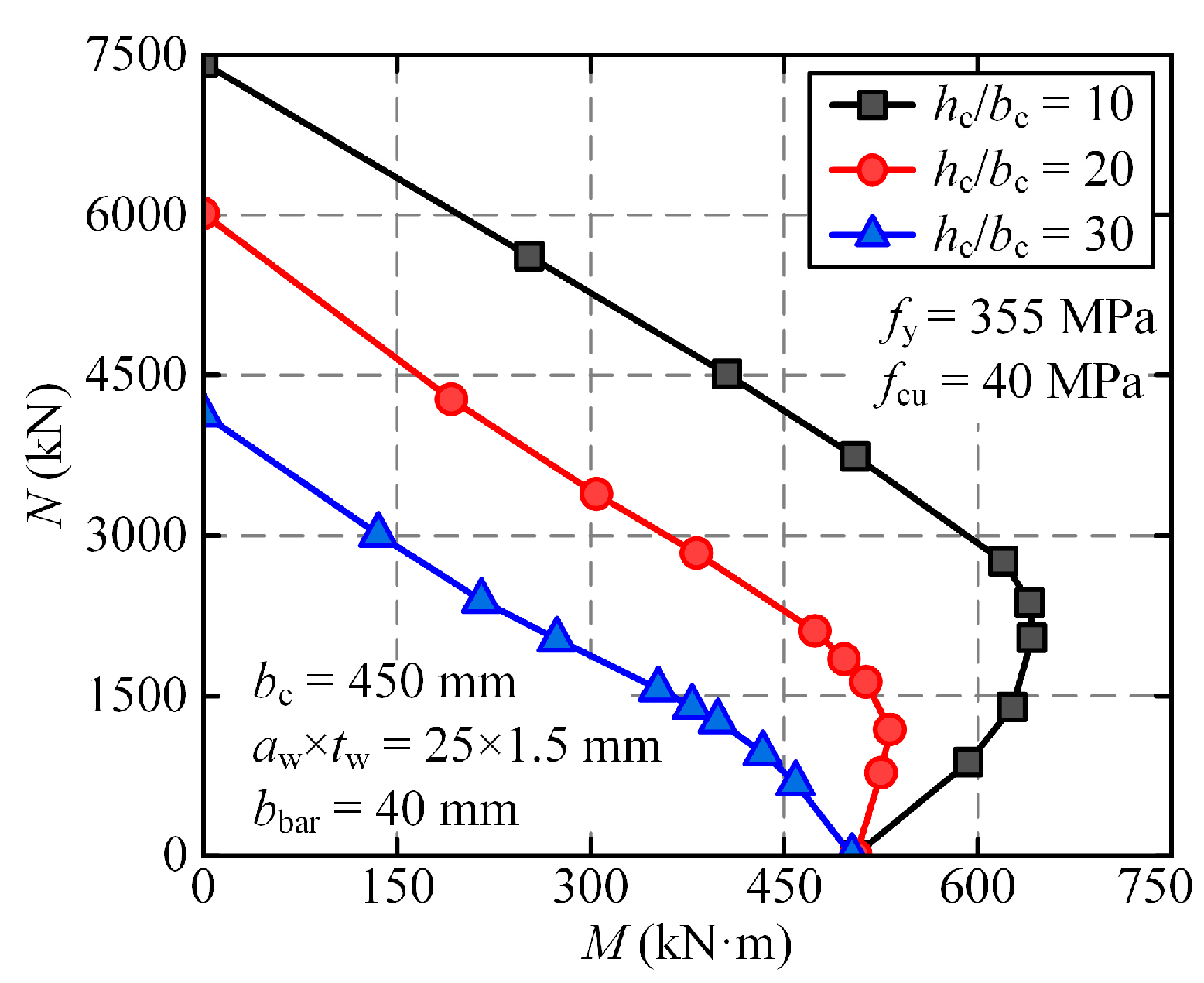











| Group | Cross-Sectional Dimensions (mm) | Height-to-Width Ratio | Corrugation Dimensions (mm) | Material Strength (MPa) | ||||
|---|---|---|---|---|---|---|---|---|
| bc | bbar | hc/bc | aw | tw | fy,w | fy,bar | fcu | |
| Group 1 | 400–500 | 40 | 10, 20, 30 | 25 | 1.5 | 355 | 355 | 40 |
| Group 2 | 450 | 40–50 | 10, 20, 30 | 25 | 1.5 | 355 | 355 | 40 |
| Group 3 | 450 | 50 | 10, 20, 30 | 15–35 | 1.5 | 355 | 355 | 40 |
| Group 4 | 450 | 40 | 10, 20, 30 | 25 | 1.5–2.5 | 355 | 355 | 40 |
| Group 5 | 450 | 40 | 10, 20, 30 | 25 | 1.5 | 355 | 235–460 | 40 |
| Group 6 | 450 | 40 | 10, 20, 30 | 25 | 1.5 | 355 | 355 | 40–60 |
| Material Strength | Eccentricity Ratio | |||||
|---|---|---|---|---|---|---|
| 0 | 0.2 | 0.4 | 0.6 | 0.8 | 1.0 | |
| fy,bar = 355 Mpa, fcu = 40 MPa | 6010 | 3384 | 2431 | 1839 | 1432 | 1152 |
| fy,bar = 420 Mpa, fcu = 40 MPa | 6642 | 3760 | 2689 | 2068 | 1685 | 1410 |
| fy,bar = 355 Mpa, fcu = 60 MPa | 7462 | 4053 | 2777 | 2020 | 1540 | 1222 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yu, C.-Q.; Duan, S.-J.; Tong, J.-Z. Global Buckling Simulation and Design of a Novel Concrete-Filled Corrugated Steel Tubular Column. Modelling 2025, 6, 22. https://doi.org/10.3390/modelling6010022
Yu C-Q, Duan S-J, Tong J-Z. Global Buckling Simulation and Design of a Novel Concrete-Filled Corrugated Steel Tubular Column. Modelling. 2025; 6(1):22. https://doi.org/10.3390/modelling6010022
Chicago/Turabian StyleYu, Chao-Qun, Sheng-Jie Duan, and Jing-Zhong Tong. 2025. "Global Buckling Simulation and Design of a Novel Concrete-Filled Corrugated Steel Tubular Column" Modelling 6, no. 1: 22. https://doi.org/10.3390/modelling6010022
APA StyleYu, C.-Q., Duan, S.-J., & Tong, J.-Z. (2025). Global Buckling Simulation and Design of a Novel Concrete-Filled Corrugated Steel Tubular Column. Modelling, 6(1), 22. https://doi.org/10.3390/modelling6010022







