Fatigue Reliability Modelling and Assessment of Carbon Fiber Reinforced Polymer/Epoxy Resin Bonded Structure Incorporating Multiple Environmental Stresses and Size Effects
Abstract
1. Introduction
- (1)
- The degradation of adhesive performance is analyzed using the cyclic cohesive zone theory, which assesses cumulative fatigue damage in adhesive bonding. Additionally, a model depicting the degradation process is constructed.
- (2)
- The impact of various environmental stresses and size effects on the fatigue performance of the carbon fiber and epoxy adhesive bonding structures is investigated using a combination of experimental and simulation tests conducted on adhesive joints.
- (3)
- A novel approach is developed to model the fatigue reliability of the carbon fiber and epoxy adhesive bonding structures, taking into consideration the combined impacts of multiple environmental stresses and size effects.
2. A Novel Adhesive Bonding Fatigue Reliability Modelling and Assessment Approach
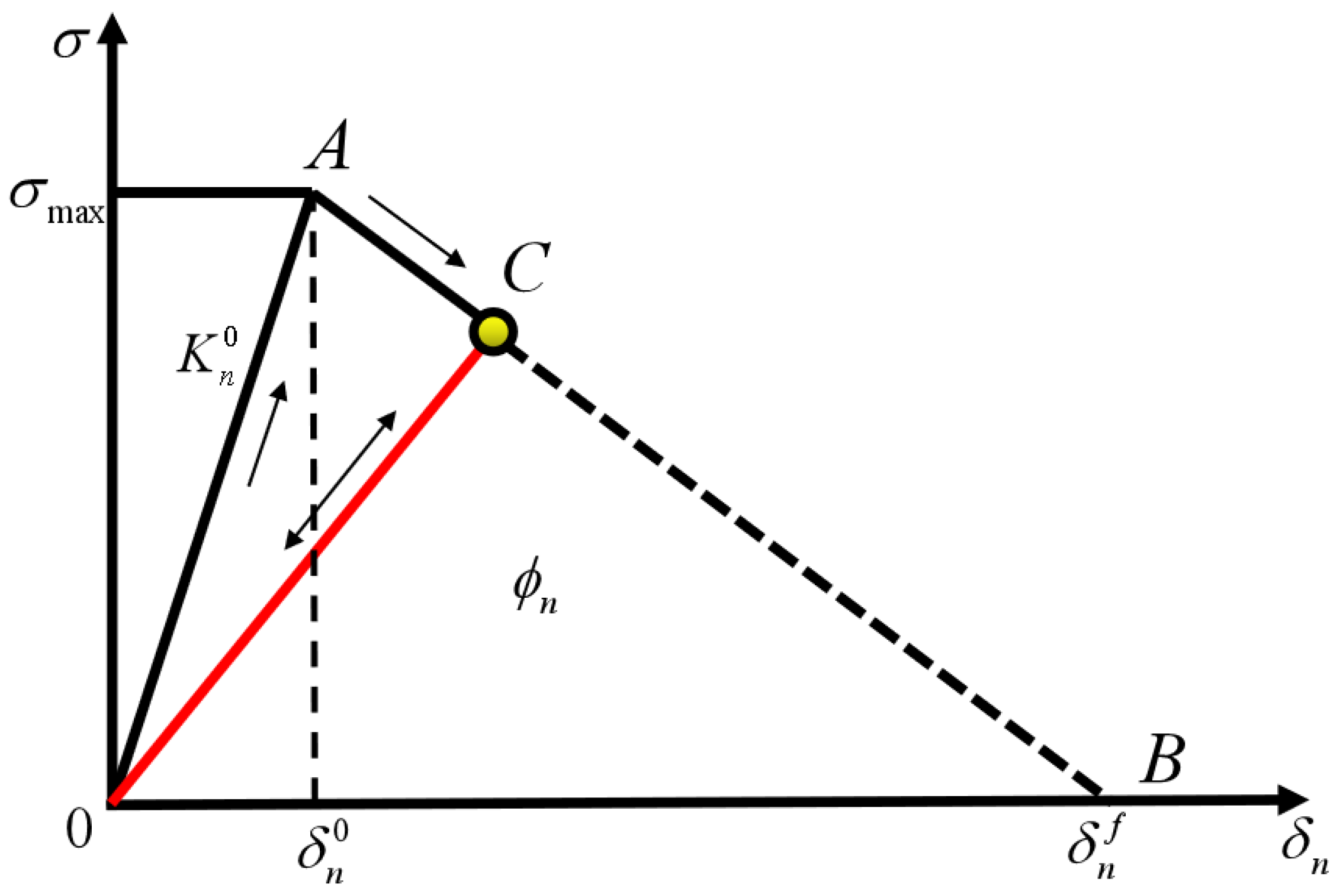
2.1. Cyclic Cohesive Zone Model (CCZM)
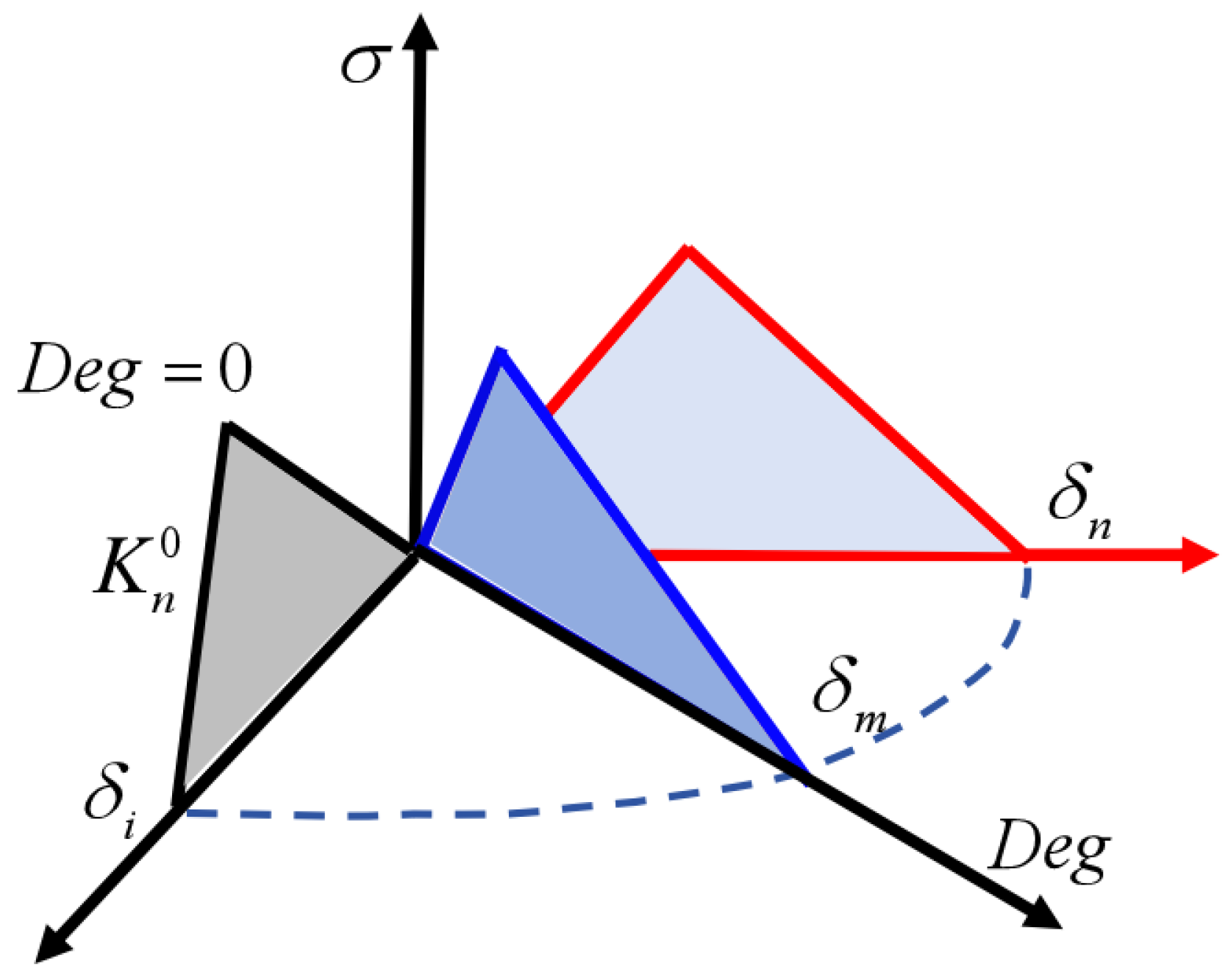
2.2. A Modified CCZM Considering the Effects of Environmental Stresses
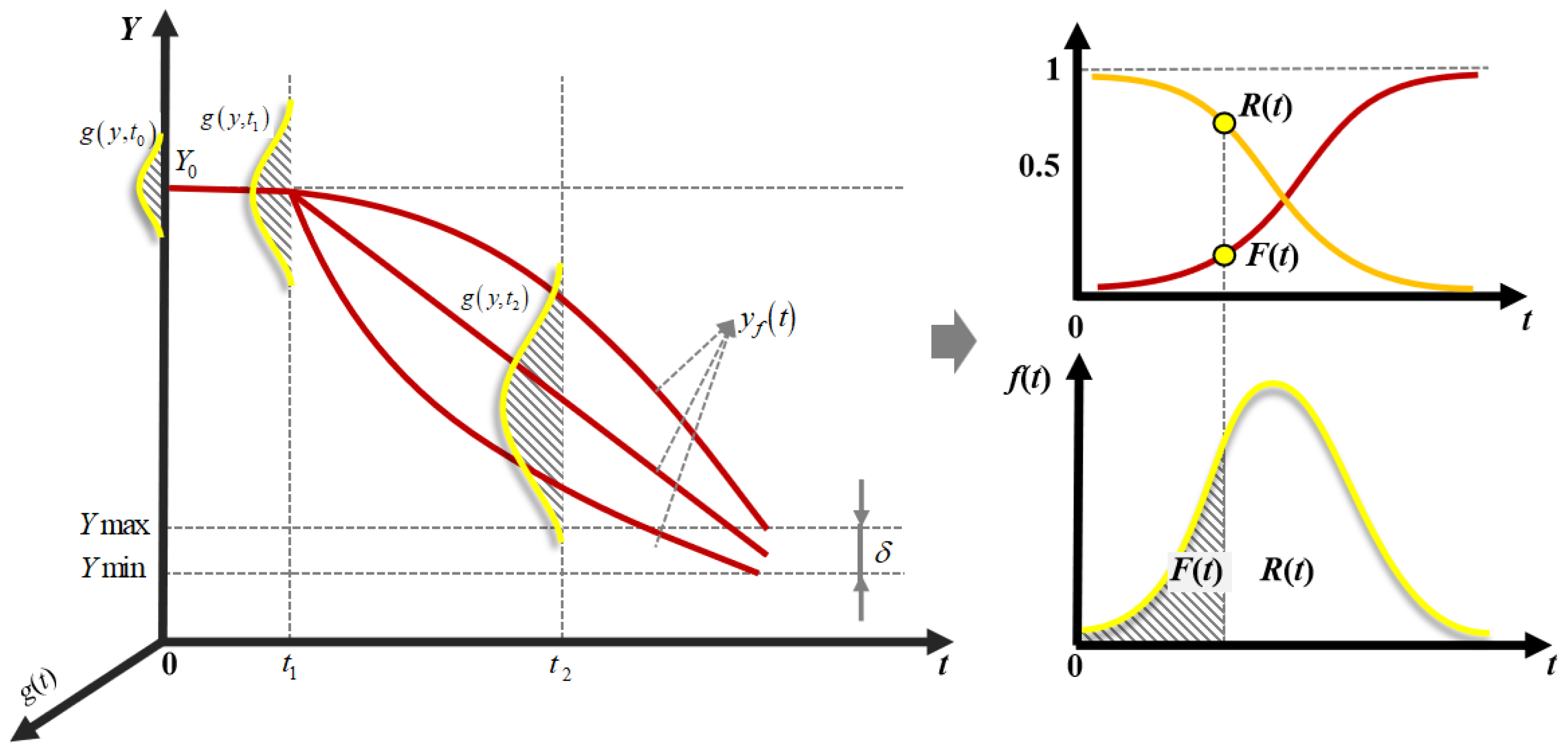
2.3. Performance Degradation Analysis-Based Adhesive Fatigue Reliability Modelling and Assessment
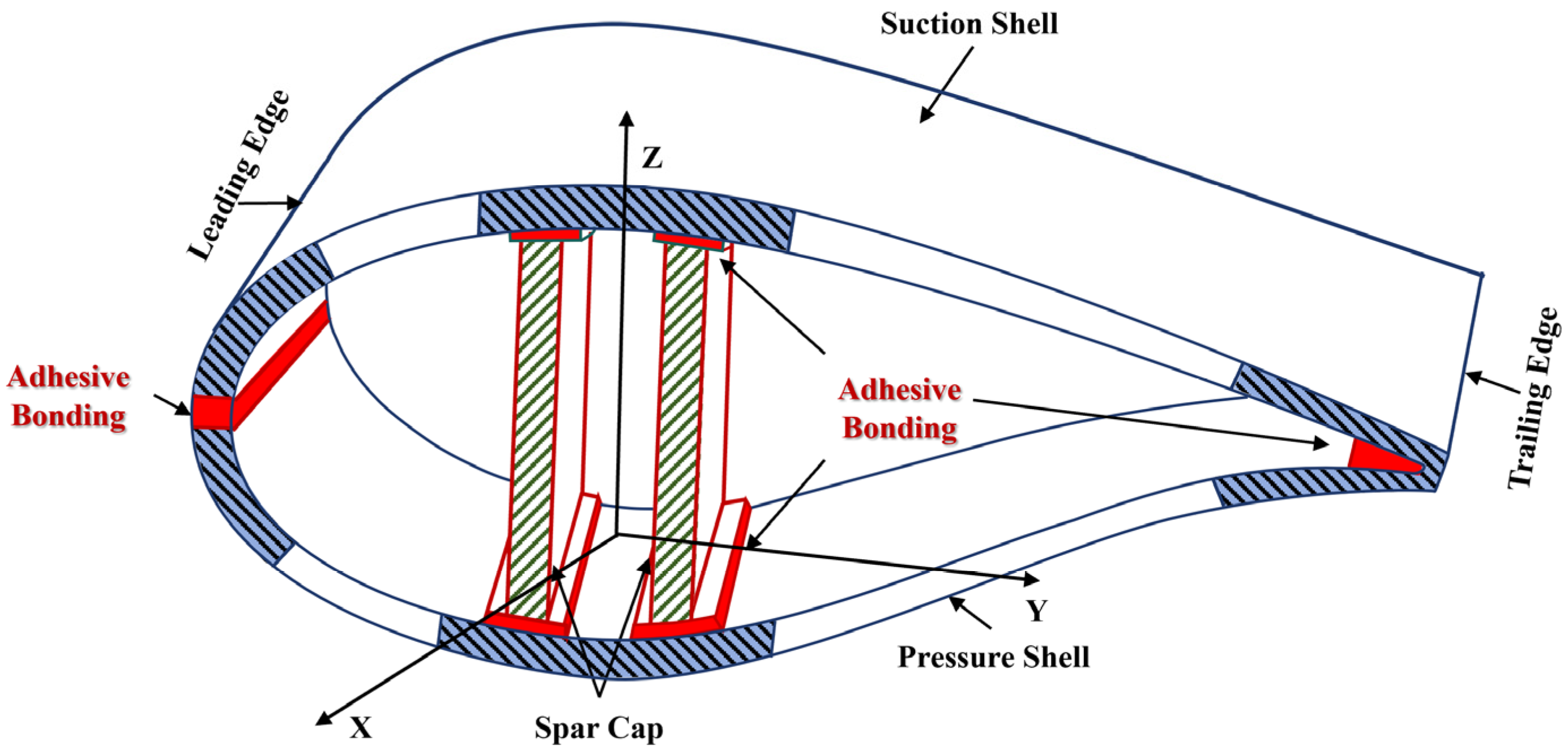
3. Experimental–Numerical Simulation Analysis of Carbon Fiber/Epoxy Resin Bonded Structure Incorporating Multienvironmental Stresses and Size Effects
3.1. Environmental Aging Tests and Fatigue Tests
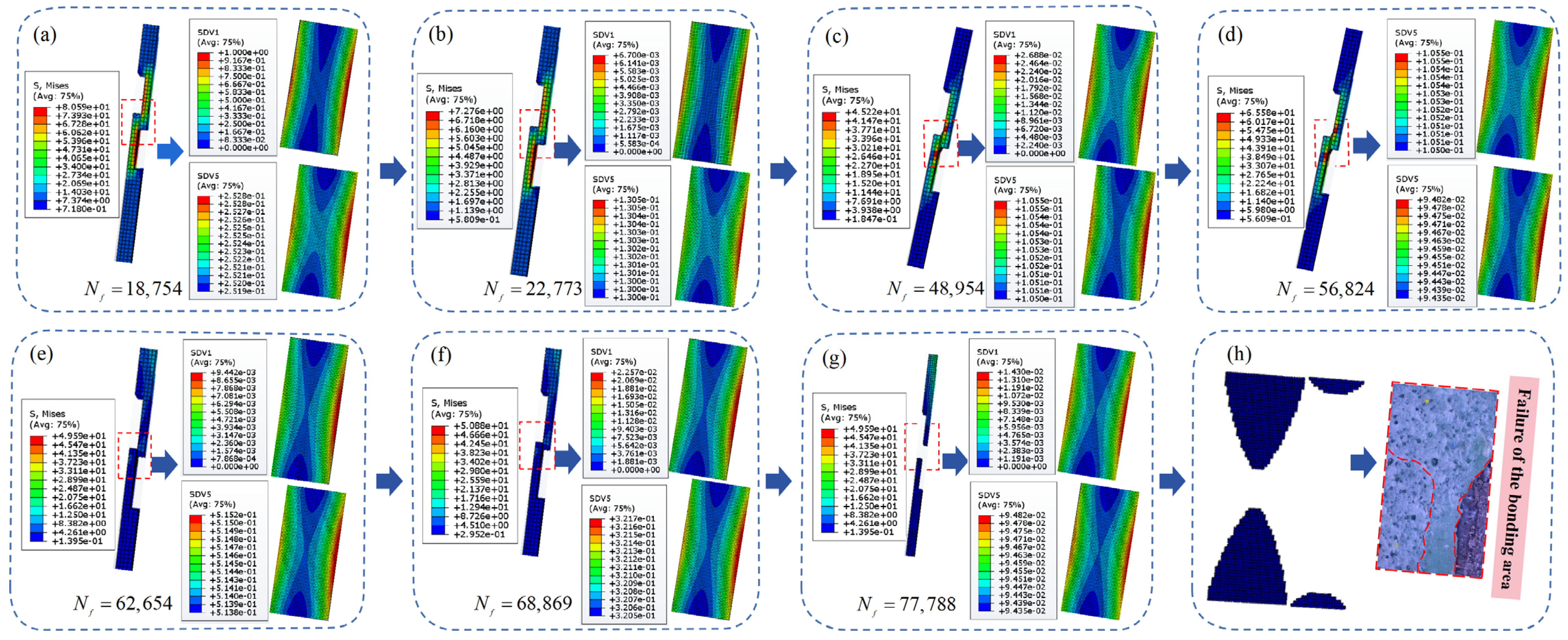
3.2. Fatigue Numerical Simulation Analysis
4. Fatigue Reliability Modelling and Assessment of Adhesive Bonding Incorporating Multiple Environmental Stresses and Size Effects
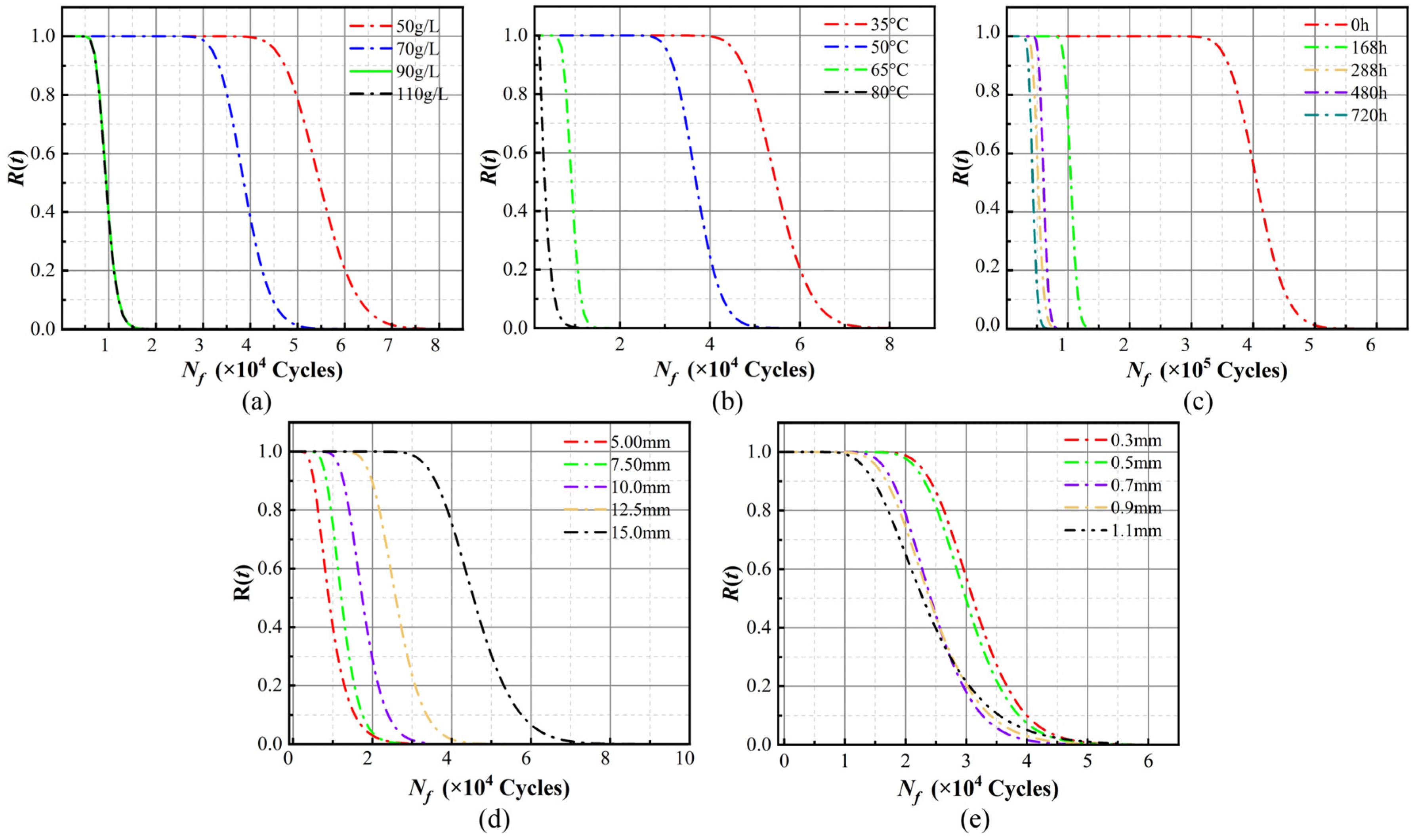
4.1. Fatigue Performance Degradation Analysis
4.2. Fatigue Reliability Modelling and Assessment Based on Fatigue Performance Degradation Analysis
5. Conclusions
- (1)
- Multienvironmental aging tests exposed pronounced size effects in the adhesive structures of various dimensions when subjected to multienvironmental stress. The damage accumulation in specimens escalated by 75% as a result of these stresses. Moreover, with the compounding influence of size effects, this figure escalated further to 85%, precipitating a shift in the failure mode for CFRP/epoxy adhesive joints from mixed to interfacial.
- (2)
- This paper introduces environmental degradation factors and size factors into the CCZM, taking into account the coupled effects of multienvironmental stresses and size effects on carbon fiber/epoxy adhesive structures. The proposed numerical simulation method can effectively simulate the fatigue life of blade adhesive structures, with a simulation error within 10%.
- (3)
- To address the characteristics of adhesive fatigue joint performance degradation, this paper employed a nonlinear Wiener process to describe the degradation process. Consequently, a fatigue reliability model for bonding joints was established, considering the impact of multiple environmental stresses and size effects. The reliability of bonding joints was assessed accordingly.
- (4)
- This paper introduces environmental degradation coefficients and size effect factors into the CCZM, effectively assessing the fatigue damage in wind turbine blade adhesive samples due to multienvironmental stresses and size effects. Although the assumptions of the proposed model have certain limitations, it still provides a theoretical method for the reliability research of adhesive structures in wind turbine blades.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Li, H.; Soares, C.G. Assessment of failure rates and reliability of floating offshore wind turbines. Reliab. Eng. Syst. Saf. 2022, 228, 108777. [Google Scholar] [CrossRef]
- Yan, J.; Tong, L.; Xiang, P. Free vibration analysis of single-walled boron nitride nanotubes based on a computational mechanics framework. Superlattices Microstruct. 2017, 112, 230–248. [Google Scholar] [CrossRef]
- Liao, D.; Zhu, S.-P.; Correia, J.A.; De Jesus, A.M.; Veljkovic, M.; Berto, F. Fatigue reliability of wind turbines: Historical perspectives, recent developments and future prospects. Renew. Energy 2022, 200, 724–742. [Google Scholar] [CrossRef]
- Li, Y.; Liu, Z.; He, Z.; Tu, L.; Huang, H.-Z. Fatigue reliability analysis and assessment of offshore wind turbine blade adhesive bonding under the coupling effects of multiple environmental stresses. Reliab. Eng. Syst. Saf. 2023, 238, 109426. [Google Scholar] [CrossRef]
- Xu, K.; Liu, F.; Liu, D. A multifield loads evaluation method for offshore wind turbines considering multivariate coherence effect. Ocean Eng. 2023, 280, 114586. [Google Scholar] [CrossRef]
- Shafiee, M.; Finkelstein, M.; Bérenguer, C. An opportunistic condition-based maintenance policy for offshore wind turbine blades subjected to degradation and environmental shocks. Reliab. Eng. Syst. Saf. 2015, 142, 463–471. [Google Scholar] [CrossRef]
- Wang, B.; Wang, L.; Liang, X.; Sheng, F.; Zhang, J.; Hong, Y.; Wang, L. 3D multiscale dynamic analysis of offshore wind turbine blade under fully coupled loads. Renew. Energy 2024, 223, 119985. [Google Scholar] [CrossRef]
- Rezaee, M.; Aly, A.M. Vibration Control in Wind Turbines to Achieve Desired System-Level Performance under Single and Multiple Hazard Loadings. Struct. Control Health Monit. 2018, 25, e2261. [Google Scholar] [CrossRef]
- Horn, J.-T.; Leira, B.J. Fatigue reliability assessment of offshore wind turbines with stochastic availability. Reliab. Eng. Syst. Saf. 2019, 191, 106550. [Google Scholar] [CrossRef]
- Na, J.; Mu, W.; Qin, G.; Tan, W.; Pu, L. Effect of temperature on the mechanical properties of adhesively bonded basalt FRP-aluminum alloy joints in the automotive industry. Int. J. Adhes. Adhes. 2018, 85, 138–148. [Google Scholar] [CrossRef]
- Khoshmanesh, S.; Watson, S.; Zarouchas, D. The effect of the fatigue damage accumulation process on the damping and stiffness properties of adhesively bonded composite structures. Compos. Struct. 2022, 287, 115328. [Google Scholar] [CrossRef]
- Jørgensen, J.B.; Sørensen, B.F.; Kildegaard, C. Tunneling cracks in full scale wind turbine blade joints. Eng. Fract. Mech. 2018, 189, 361–376. [Google Scholar] [CrossRef]
- Zheng, T.; Zhao, C.; He, J. Research on fatigue performance of offshore wind turbine blade with basalt fiber bionic plate. Structures 2023, 47, 466–481. [Google Scholar] [CrossRef]
- Verma, A.S.; Vedvik, N.P.; Gao, Z.; Castro, S.G.P.; Teuwen, J.J.E. Bondline Thickness Effects on Damage Tolerance of Adhesive Joints Subjected to Localized Impact Damages: Application to Leading Edge of Wind Turbine Blades. Materials 2021, 14, 7526. [Google Scholar] [CrossRef]
- Raman, V.; Drissi-Habti, M.; Guillaumat, L.; Khadhour, A. Numerical simulation analysis as a tool to identify areas of weakness in a turbine wind-blade and solutions for their reinforcement. Compos. Part B Eng. 2016, 103, 23–39. [Google Scholar] [CrossRef]
- Li, H.; Zhang, X.; Zhou, B.; Wang, L.; Zhu, C. Study on the repair parameters for trailing-edge bonding failure of wind turbine blade in service. Energy Sci. Eng. 2022, 11, 143–163. [Google Scholar] [CrossRef]
- Yang, Y.; Zhao, J.; Zhang, S.; Yang, Z.; Biscaia, H. Influence of salt fog and ambient condition exposure on CFRP-to-steel bonded joints. Compos. Struct. 2022, 280, 114874. [Google Scholar] [CrossRef]
- Lin, C. Study on the interlaminar fracture toughness of stitched composites type I under salt spray environment. Master’s Thesis, Tiangong University, Tianjin, China, 2021. [Google Scholar] [CrossRef]
- Mu, W.-L.; Xu, Q.-H.; Na, J.-X.; Wang, H.; Tan, W.; Li, D.-F. Influence of temperature and humidity on the fatigue behaviour of adhesively bonded CFRP/aluminium alloy joints. J. Adhes. 2022, 98, 1358–1376. [Google Scholar] [CrossRef]
- Na, J.X.; Wang, G.B.; Zhuang, W.M.; Mu, W.L.; Xi, Q.H. A review on structural strength and environmental durability of composite bonded structures. J. Transp. Eng. 2021, 21, 78–93. [Google Scholar] [CrossRef]
- Rafiee, R.; Hashemi-Taheri, M.R. Failure analysis of a composite wind turbine blade at the adhesive joint of the trailing edge. Eng. Fail. Anal. 2021, 121, 105148. [Google Scholar] [CrossRef]
- El Hage, J.; Challita, G.; Capelle, J.; Gilgert, J.; Alhussein, A. Influence of short-time thermal ageing on the behaviour of double lap composite adhesively bonded joints. SN Appl. Sci. 2020, 2, 1734. [Google Scholar] [CrossRef]
- Taskin, N.U.; Sahin, A. Effect of aging time at high temperature on the shear strength of adhesively bonded aluminum composite foam joints. J. Adhes. 2019, 95, 308–324. [Google Scholar] [CrossRef]
- Makkonen, M. Notch size effects in the fatigue limit of steel. Int. J. Fatigue 2003, 25, 17–26. [Google Scholar] [CrossRef]
- Sun, L.; Tie, Y.; Hou, Y. Prediction of failure behavior of adhesively bonded CFRP scarf joints using a cohesive zone model. Eng Fract Mech 2020, 228, 1068977. [Google Scholar] [CrossRef]
- Zou, T.C.; Fu, J.; Li, L.H.; Liu, Z.H.; Zhu, H. Effect of lap length on the tensile properties and damage characteristics of CFRP single-lap glued joints. Mater. Eng. 2021, 49, 158–165. [Google Scholar] [CrossRef]
- Zhao, L.Y.; Liu, Y.; Xu, Y.M.; Jiang, Y. A brief discussion on the application of PFMEA in the bonding process of offshore wind turbine blades. FRP/Composites 2019, 303, 58–61. [Google Scholar] [CrossRef]
- Tanulia, V.; Wang, J.; Pearce, G.M.; Baker, A.; Chang, P.; Prusty, B.G. Experimental and computational assessment of disbond growth and fatigue life of bonded joints and patch repairs for primary airframe structures. Int. J. Fatigue 2022, 159, 106776. [Google Scholar] [CrossRef]
- Rajad, O.; Mounir, H.; El Marjani, A.; Fertahi, S.E.-D. Nonlinear modeling analysis of the coupled mechanical strength and stiffness enhancement of composite materials of a Horizontal Axis Wind Turbine Blade (HAWTB). Int. J. Interact. Des. Manuf. 2022, 16, 469–492. [Google Scholar] [CrossRef]
- Siegmund, T. A numerical study of transient fatigue crack growth by use of an irreversible cohesive zone model. Int. J. Fatigue 2004, 26, 929–939. [Google Scholar] [CrossRef]
- Beber, V.; Schneider, B.; Brede, M. Efficient critical distance approach to predict the fatigue lifetime of structural adhesive joints. Eng. Fract. Mech. 2019, 214, 365–377. [Google Scholar] [CrossRef]
- Roth, S.; Kuna, M. Prediction of size-dependent fatigue failure modes by means of a cyclic cohesive zone model. Int. J. Fatigue 2017, 100, 58–67. [Google Scholar] [CrossRef]
- Yan, T.; Lei, Y.; Li, N.; Wang, B.; Wang, W. Degradation modeling and remaining useful life prediction for dependent competing failure processes. Reliab. Eng. Syst. Saf. 2021, 212, 107638. [Google Scholar] [CrossRef]
- Yan, T.; Wang, D.; Hou, B.; Peng, Z. Generic framework for integration of first prediction time detection with machine degradation modelling from frequency domain. IEEE Trans. Reliab. 2022, 71, 1464–1476. [Google Scholar] [CrossRef]
- Yang, J.; Meng, D.B.; Zhang, X.L. Reliability Based Design Optimization of Aero-Engine Spindle Ball Bearings. J. Donghua Univ. 2014, 31, 853–855. [Google Scholar]
- Wang, X.; Wang, B.X.; Jiang, P.H.; Hong, Y. Accurate reliability inference based on Wiener process with random effects for degradation data. Reliab. Eng. Syst. Saf. 2020, 193, 106631. [Google Scholar] [CrossRef]
- Zhao, J.; Zhou, Y.; Zhu, Q.; Song, Y.; Liu, Y.; Luo, H. A remaining useful life prediction method of aluminum electrolytic capacitor based on wiener process and similarity measurement. Microelectron. Reliab. 2023, 142, 114928. [Google Scholar] [CrossRef]
- GB/T 2573-2008; Test Method for Aging Performance of Glass Fiber Reinforced Plastics. National Standards of People’s Republic of China: Beijing, China, 2008.
- GB/T 16779-2008; Test Method for Tensile-Tensile Fatigue Properties of Fiber-Reinforced Plastic Laminates. National Standards of People’s Republic of China: Beijing, China, 2008.
- Zhu, S.-P.; Liu, Q.; Lei, Q.; Wang, Q. Probabilistic fatigue life prediction and reliability assessment of a high pressure turbine disc considering load variations. Int. J. Damage Mech. 2018, 27, 1569–1588. [Google Scholar] [CrossRef]
- Li, Y.-F.; Liu, Y.; Huang, T.; Huang, H.-Z.; Mi, J. Reliability assessment for systems suffering common cause failure based on Bayesian networks and proportional hazards model. Qual. Reliab. Eng. Int. 2020, 36, 2509–2520. [Google Scholar] [CrossRef]
- Mi, J.; Lu, N.; Li, Y.-F.; Huang, H.-Z.; Bai, L. An evidential network-based hierarchical method for system reliability analysis with common cause failures and mixed uncertainties. Reliab. Eng. Syst. Saf. 2022, 220, 108295. [Google Scholar] [CrossRef]
- Mi, J.; Li, Y.-F.; Peng, W.; Huang, H.-Z. Reliability analysis of complex multi-state system with common cause failure based on evidential networks. Reliab. Eng. Syst. Saf. 2018, 174, 71–81. [Google Scholar] [CrossRef]
- Zhang, X.; Huang, H.; Zhang, X.; Zhang, K. A multiaxial probabilistic fatigue life prediction method for nickel-based single crystal turbine blade considering mean stress correction. Qual. Reliab. Eng. Int. 2023, 39, 1735–1755. [Google Scholar] [CrossRef]
- Zhang, X.; Zhang, K.; Yang, X.; Fazeres-Ferradosa, T.; Zhu, S.-P. Transfer learning and direct probability integral method based reliability analysis for offshore wind turbine blades under multi-physics coupling. Renew. Energy 2023, 206, 552–565. [Google Scholar] [CrossRef]
- Malhotra, P.; Hyers, R.; Manwell, J.; McGowan, J. A review and design study of blade testing systems for utility-scale wind turbines. Renew. Sustain. Energy Rev. 2012, 16, 284–292. [Google Scholar] [CrossRef]



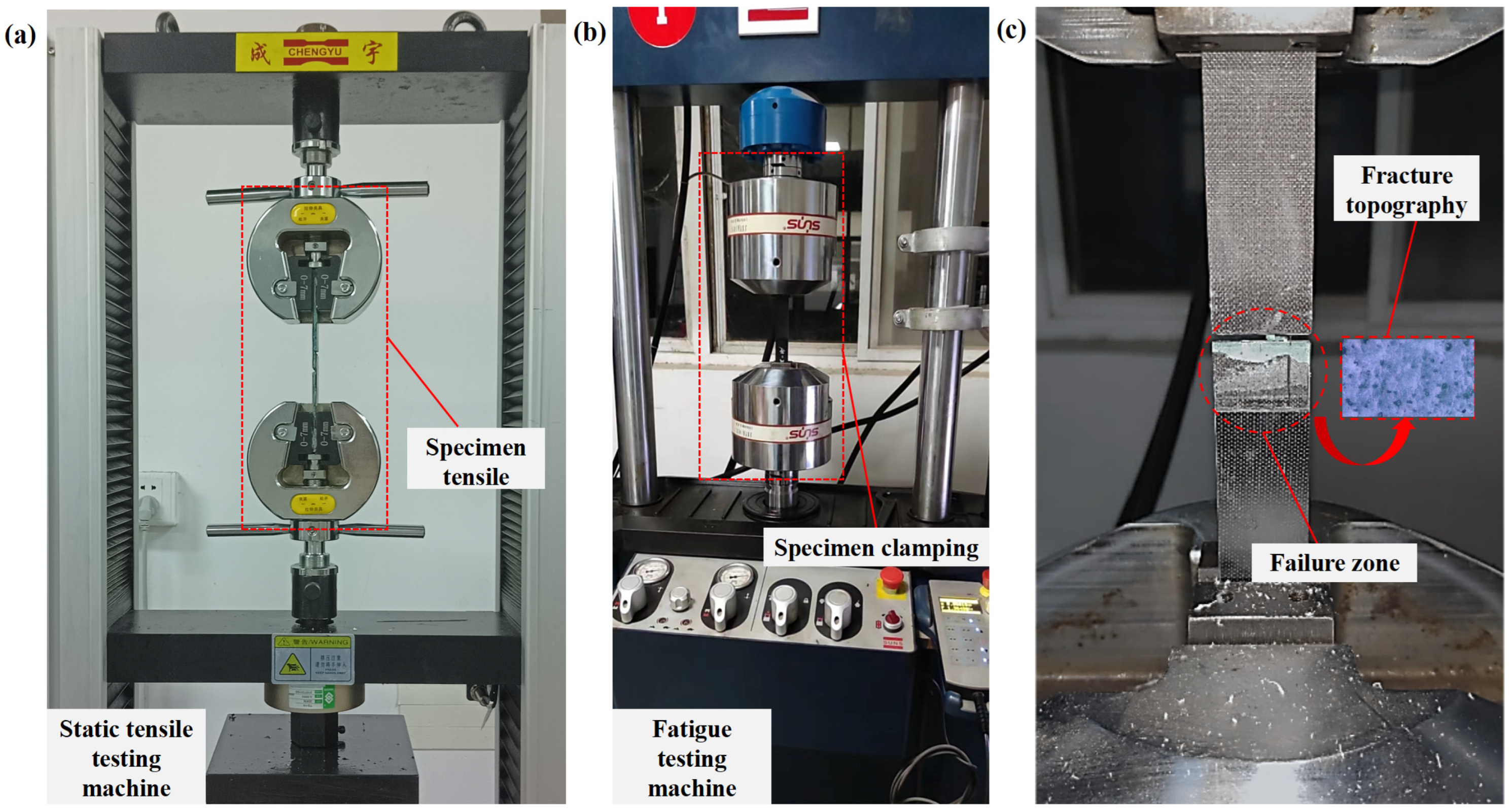
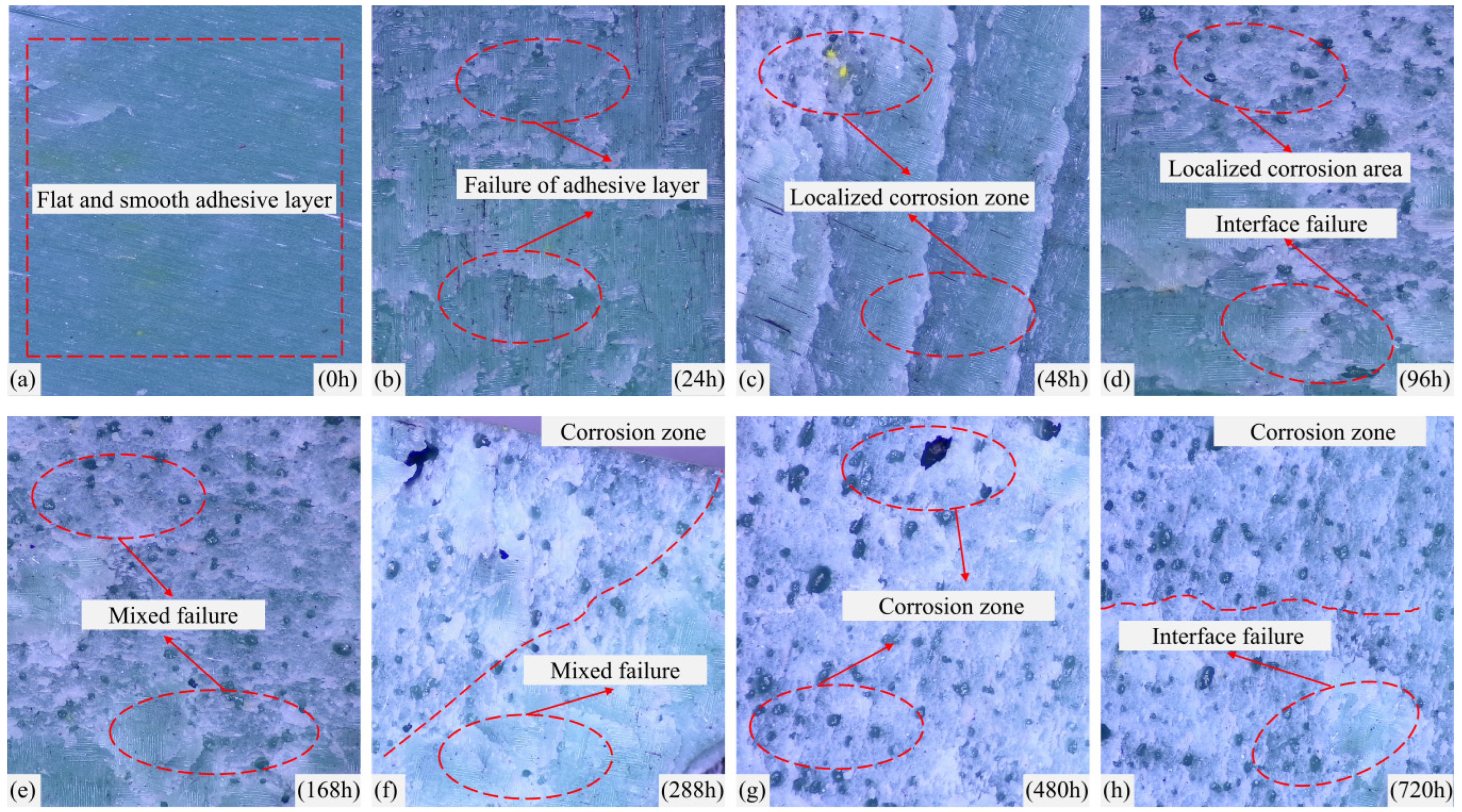
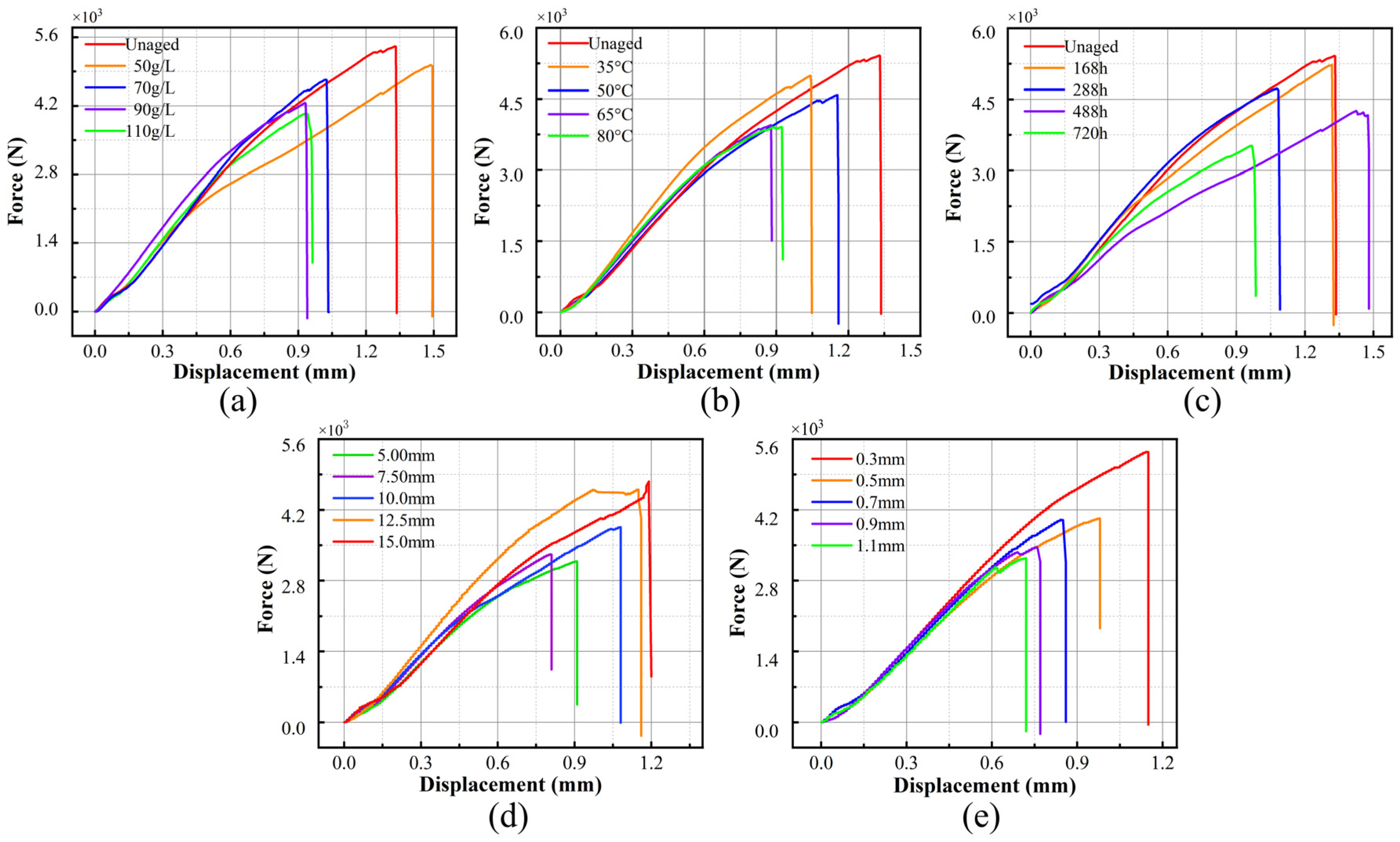
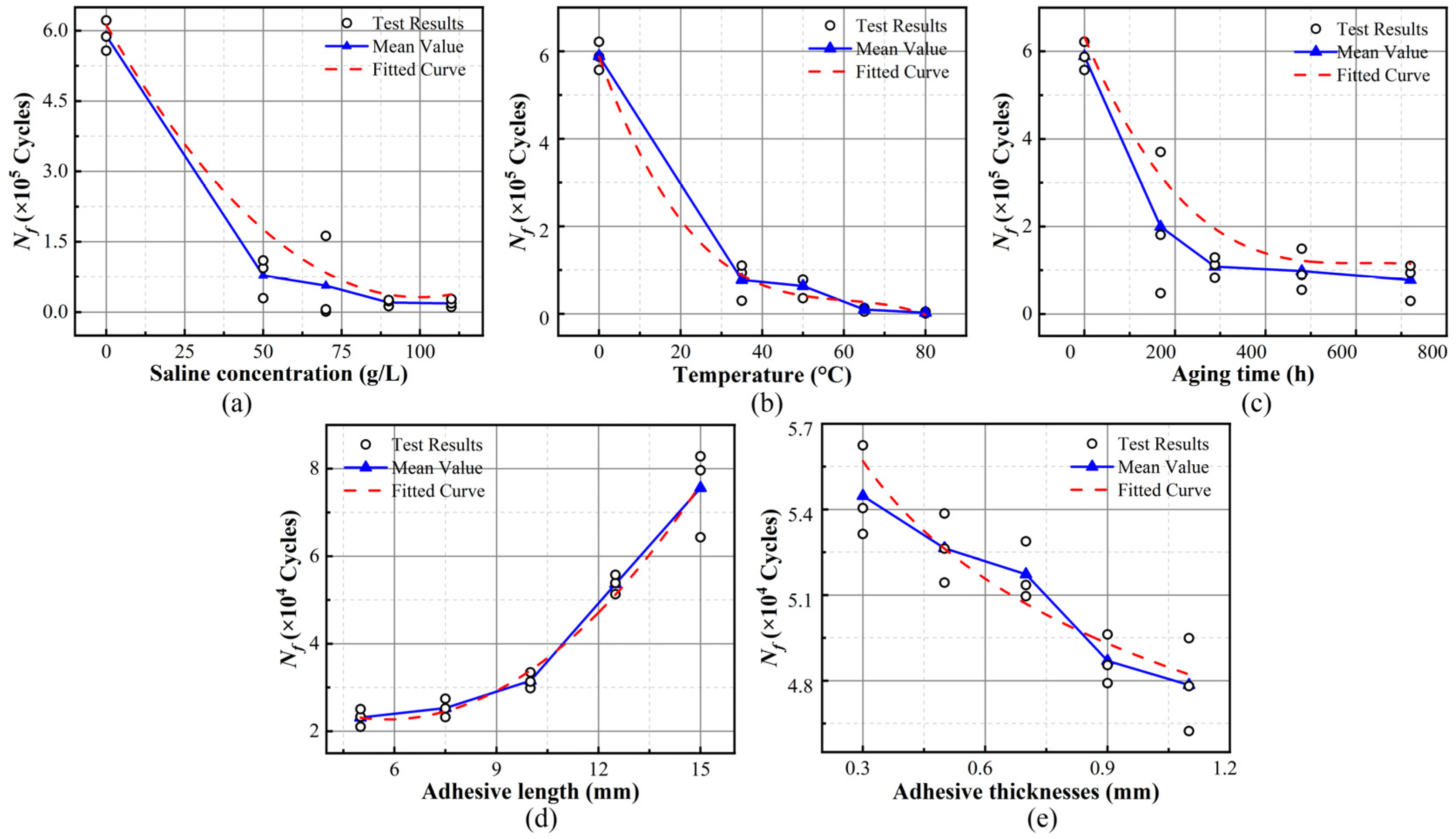



| Environmental Test Gradients | Size Gradients | ||||
|---|---|---|---|---|---|
| Saline Concentration (g/L) | Aging Time (h) | Temperature (°C) | Bonding Length (mm) | Bonding Width (mm) | Bonding Thickness (mm) |
| 50 | 168 | 35 | 5 | 25 | 0.3 |
| 70 | 288 | 50 | 7.5 | 25 | 0.5 |
| 90 | 480 | 65 | 10 | 25 | 0.7 |
| 110 | 720 | 80 | 12.5 | 25 | 0.9 |
| / | / | / | 15 | 25 | 1.1 |
| Number | Environmental Factors | Fatigue Life (Cycles) | SIF | |||
|---|---|---|---|---|---|---|
| C (g/L) | T (°C) | |||||
| 1 | / | / | / | 588,754 | 0 | 0.54 |
| 2 | 50 | 35 | 168 | 434,940 | 0.9261 | 0.54 |
| 3 | 90 | 65 | 288 | 40,127 | 0.9318 | 0.54 |
| … | … | … | … | … | … | … |
| 12 | 90 | 80 | 480 | 2265 | 0.9962 | 0.54 |
| 13 | 50 | 35 | 720 | 77,788 | 0.8679 | 0.54 |
| Number | Size Factors | Fatigue Life (Cycles) | ||||
|---|---|---|---|---|---|---|
| (mm) | (mm) | (mm) | ||||
| 1 | 5 | 25 | 0.5 | 23,302 | 0.3655 | 0.267 |
| 2 | 7.5 | 25 | 0.5 | 25,224 | 0.4246 | 0.367 |
| … | … | … | … | … | … | … |
| 5 | 15 | 25 | 0.5 | 82,802 | 0.7325 | 0.667 |
| 6 | 12.5 | 25 | 0.3 | 54,044 | 0.2446 | 0.524 |
| 7 | 12.5 | 25 | 0.5 | 53,856 | 0.2896 | 0.54 |
| … | … | … | … | … | … | … |
| 10 | 12.5 | 25 | 1.1 | 47,812 | 0.7344 | 0.588 |
| Number | Model Parameters | |
|---|---|---|
| 1 | 7.125536 × 10−7 | 2.075582 × 10−9 |
| 2 | 9.589038 × 10−7 | 2.792616 × 10−9 |
| 3 | 2.377422 × 10−6 | 7.276249 × 10−9 |
| … | … | … |
| 12 | 7.718361 × 10−6 | 3.040484 × 10−8 |
| 13 | 5.527738 × 10−6 | 1.864005 × 10−8 |
| Number | Model Parameters | |
|---|---|---|
| 1 | 2.035398 × 10−5 | 7.950124 × 10−7 |
| 2 | 3.166352 × 10−5 | 1.058724 × 10−6 |
| … | … | … |
| 5 | 6.487835 × 10−6 | 6.738933 × 10−8 |
| 6 | 6.314948 × 10−6 | 4.980836 × 10−8 |
| 7 | 6.554756 × 10−6 | 5.366307 × 10−8 |
| … | … | … |
| 10 | 8.255034 × 10−6 | 2.029376 × 10−7 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Shao, Z.; Liu, Z.; Liang, J.; Liu, H.; Zhang, Y. Fatigue Reliability Modelling and Assessment of Carbon Fiber Reinforced Polymer/Epoxy Resin Bonded Structure Incorporating Multiple Environmental Stresses and Size Effects. Modelling 2024, 5, 1116-1134. https://doi.org/10.3390/modelling5030058
Shao Z, Liu Z, Liang J, Liu H, Zhang Y. Fatigue Reliability Modelling and Assessment of Carbon Fiber Reinforced Polymer/Epoxy Resin Bonded Structure Incorporating Multiple Environmental Stresses and Size Effects. Modelling. 2024; 5(3):1116-1134. https://doi.org/10.3390/modelling5030058
Chicago/Turabian StyleShao, Zhenjiang, Zheng Liu, Jinlong Liang, Haodong Liu, and Yuhao Zhang. 2024. "Fatigue Reliability Modelling and Assessment of Carbon Fiber Reinforced Polymer/Epoxy Resin Bonded Structure Incorporating Multiple Environmental Stresses and Size Effects" Modelling 5, no. 3: 1116-1134. https://doi.org/10.3390/modelling5030058
APA StyleShao, Z., Liu, Z., Liang, J., Liu, H., & Zhang, Y. (2024). Fatigue Reliability Modelling and Assessment of Carbon Fiber Reinforced Polymer/Epoxy Resin Bonded Structure Incorporating Multiple Environmental Stresses and Size Effects. Modelling, 5(3), 1116-1134. https://doi.org/10.3390/modelling5030058






