Hybrid Approach for Multiscale and Multimodal Time-Resolved Diagnosis of Ultrafast Processes in Materials via Tailored Synchronization of Laser and X-ray Sources at MHz Repetition Rates
Abstract
1. Introduction
2. Materials and Methods
2.1. Samples
2.2. Optical Pump–Probe Diagnostics
2.3. Optical Pump–X-ray Probe Diagnostics
2.3.1. X-ray Diffraction Module
2.3.2. Laser–Matter Interaction Setup
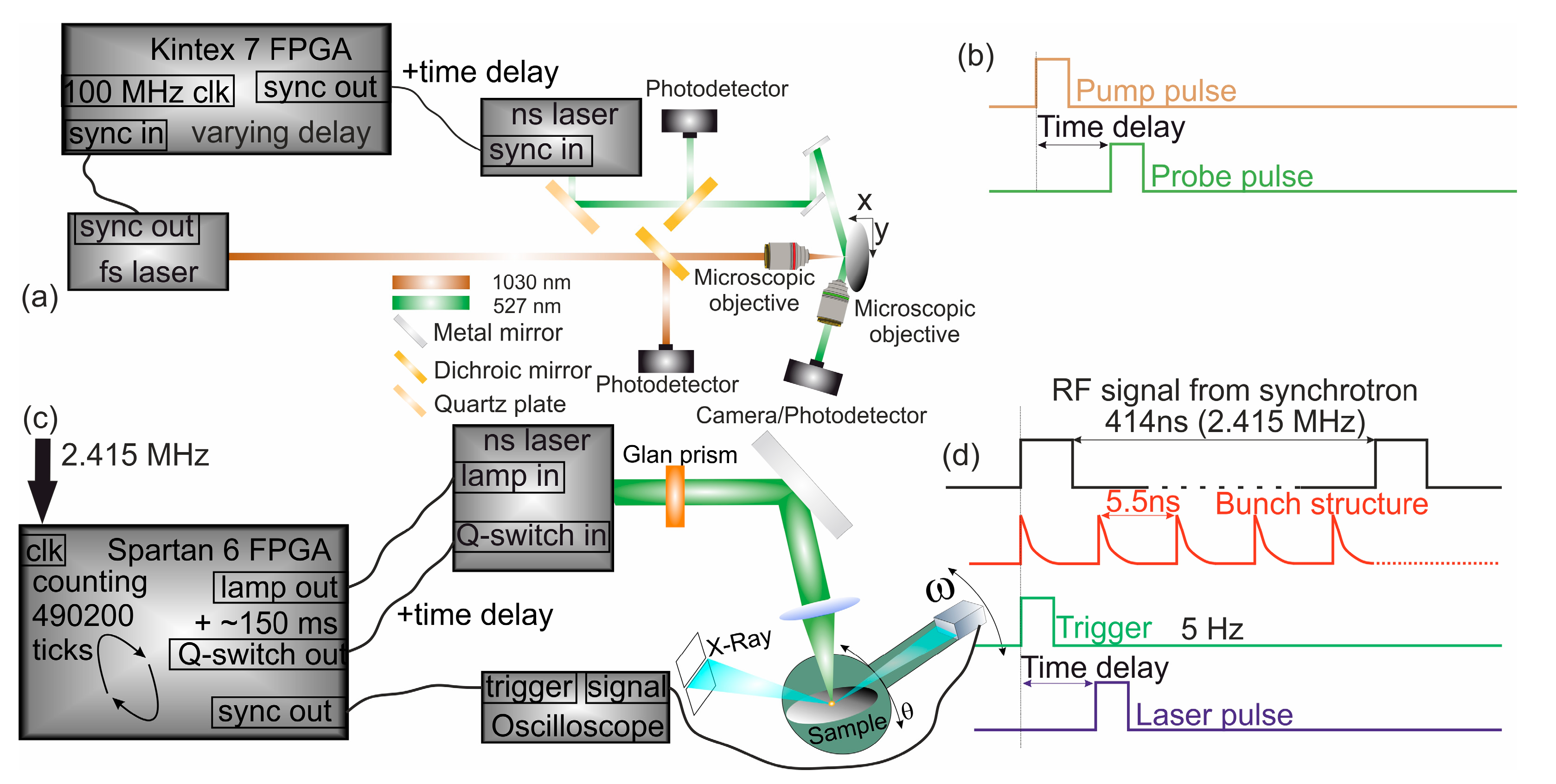
2.3.3. Synchronization System
2.3.4. Comparison with Other Synchronization Systems
3. Results and Discussion
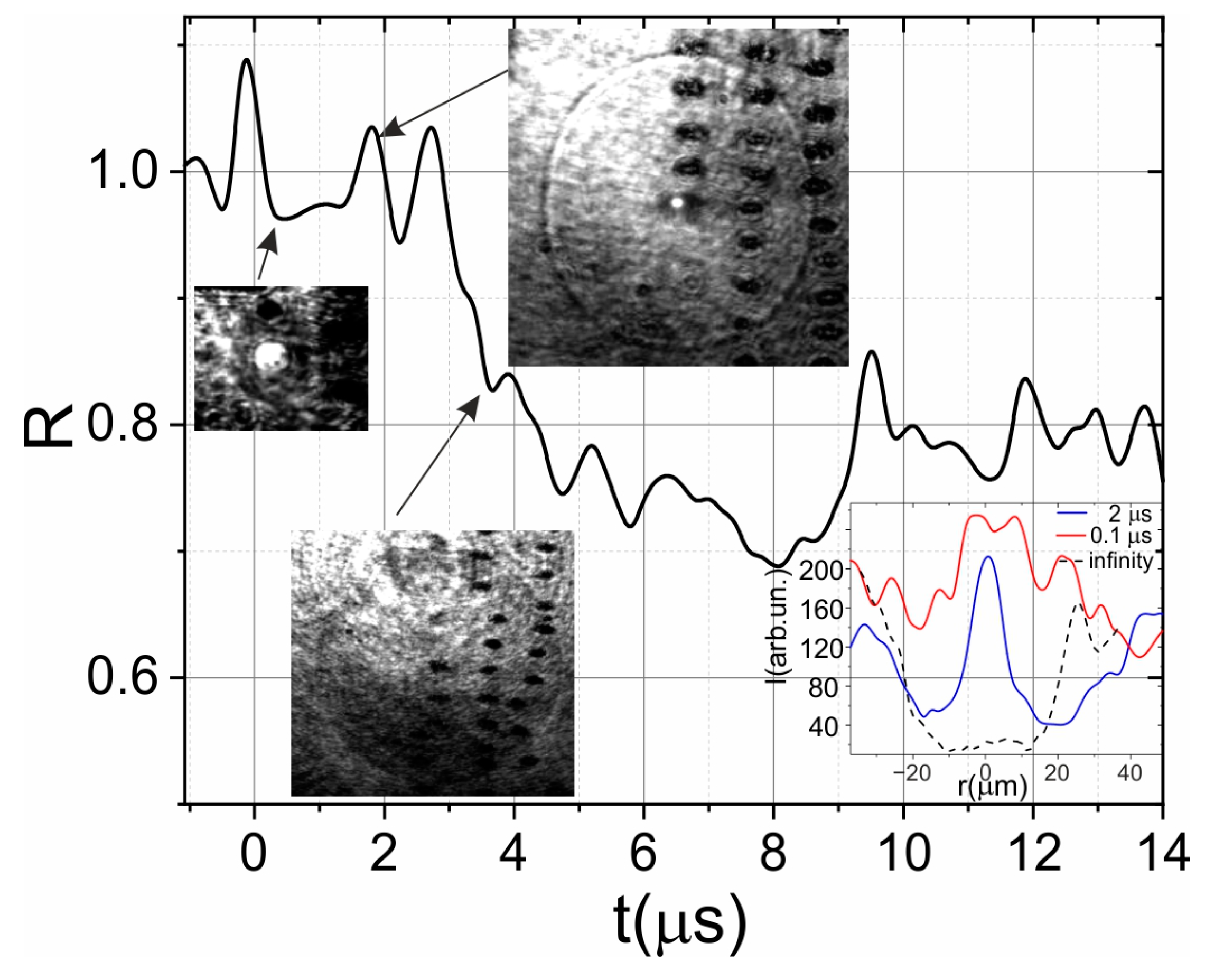
3.1. Optical Diagnostics of Shock Wave Generation and Micromodification Formation
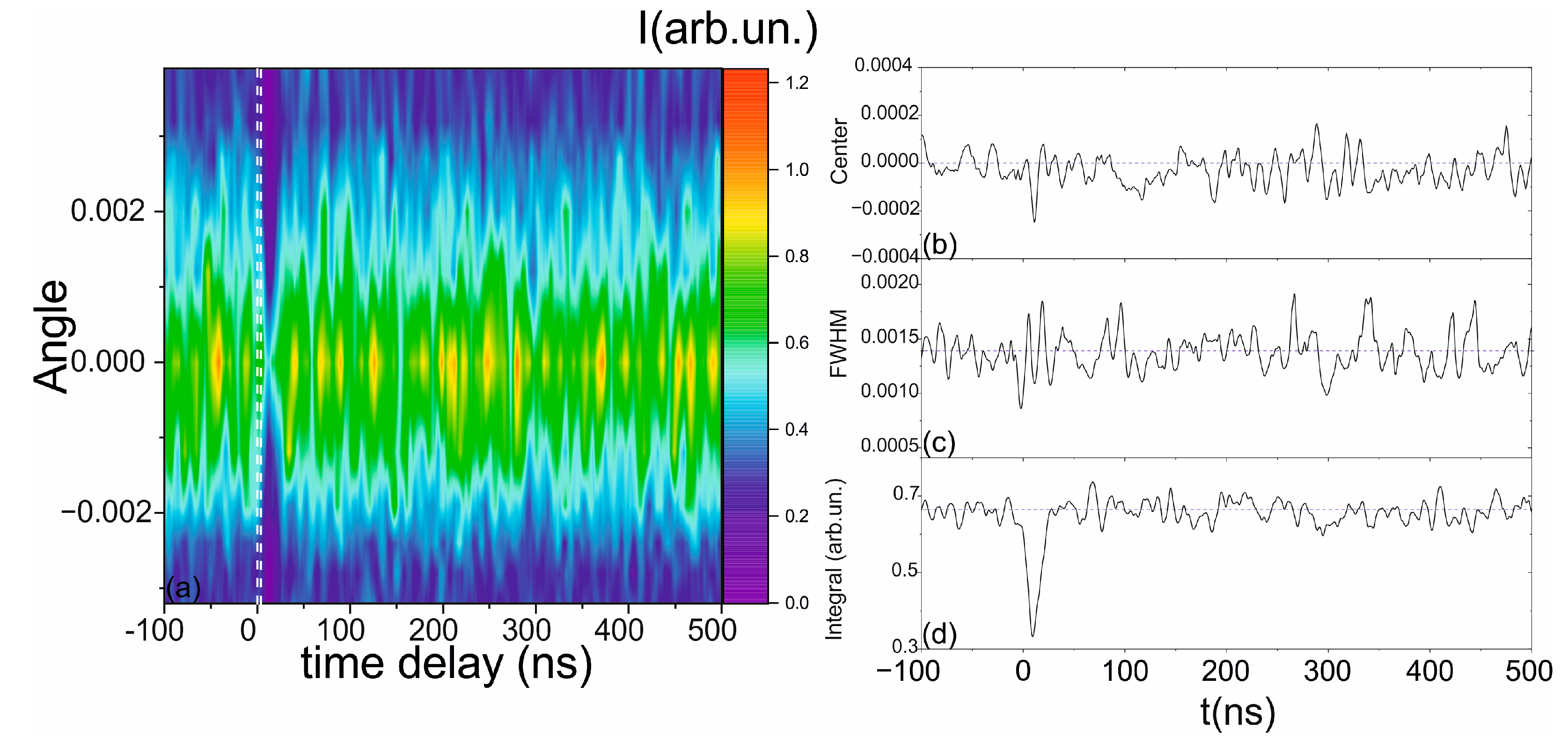
3.2. X-ray Time-Resolved Diagnostics of the Piezo-Effect in LiNbO3
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Fetisov, G.V. X-ray Diffraction Methods for Structural Diagnostics of Materials: Progress and Achievements. Uspekhi Fiz. Nauk 2020, 190, 2–36. [Google Scholar] [CrossRef]
- Sokolowski-Tinten, K.; Von Der Linde, D. Ultrafast phase transitions and lattice dynamics probed using laser-produced X-ray pulses. J. Phys. Condens. Matter 2004, 16, R1517. [Google Scholar] [CrossRef]
- Mareev, E.; Minaev, N.; Epifanov, E.; Tsymbalov, I.; Sviridov, A.; Gordienko, V. Time-Resolved Optical Probing of the Non-Equilibrium Supercritical State in Molecular Media under Ns Laser-Plasma Impact. Opt. Express 2021, 29, 33592. [Google Scholar] [CrossRef] [PubMed]
- Li, R.; Ashour, O.A.; Chen, J.; Elsayed-Ali, H.E.; Rentzepis, P.M. Femtosecond Laser Induced Structural Dynamics and Melting of Cu (111) Single Crystal. An Ultrafast Time-Resolved X-ray Diffraction Study. J. Appl. Phys. 2017, 121, 055102. [Google Scholar] [CrossRef]
- Pushkin, A.; Migal, E.; Suleimanova, D.; Mareev, E.; Potemkin, F. High-Power Solid-State Near- and Mid-IR Ultrafast Laser Sources for Strong-Field Science. Photonics 2022, 9, 90. [Google Scholar] [CrossRef]
- Guo, B.; Sun, J.; Lu, Y.F.; Jiang, L. Ultrafast Dynamics Observation during Femtosecond Laser-Material Interaction. Int. J. Extrem. Manuf. 2019, 1, 032004. [Google Scholar] [CrossRef]
- Noack, J.; Vogel, A. Single-Shot Spatially Resolved Characterization of Laser-Induced Shock Waves in Water. Appl. Opt. 1998, 37, 4092–4099. [Google Scholar] [CrossRef]
- Lindenberg, A.M.; Larsson, J.; Sokolowski-Tinten, K.; Gaffney, K.J.; Blome, C.; Synnergren, O.; Sheppard, J.; Caleman, C.; MacPhee, A.G.; Weinstein, D.; et al. Atomic-Scale Visualization of Inertial Dynamics. Science 2005, 308, 392–395. [Google Scholar] [CrossRef]
- Reiter, F.; Graf, U.; Serebryannikov, E.E.; Schweinberger, W.; Fiess, M.; Schultze, M.; Azzeer, A.M.; Kienberger, R.; Krausz, F.; Zheltikov, A.M.; et al. Route to Attosecond Nonlinear Spectroscopy. Phys. Rev. Lett. 2010, 105, 243902. [Google Scholar] [CrossRef] [PubMed]
- Mcbride, E.E.; Krygier, A.; Ehnes, A.; Galtier, E.; Harmand, M.; Konôpková, Z. Phase Transition Lowering in Dynamically-Compressed Silicon. Nat. Phys. 2019, 15, 89–94. [Google Scholar] [CrossRef]
- Brown, S.B.; Gleason, A.E.; Galtier, E.; Higginbotham, A.; Arnold, B.; Fry, A.; Granados, E.; Hashim, A.; Schroer, C.G.; Schropp, A.; et al. Direct Imaging of Ultrafast Lattice Dynamics. Sci. Adv. 2019, 5, eaau8044. [Google Scholar] [CrossRef]
- Trigo, M.; Dean, M.P.M.; Reis, D.A. Ultrafast X-ray Probes of Dynamics in Solids. arXiv 2021, arXiv:2108.05456. [Google Scholar]
- Siders, C.W.; Cavalleri, A.; Sokolowski-Tinten, K.; Tóth, C.; Guo, T.; Kammler, M.; Horn Von Hoegen, M.; Wilson, K.R.; Von Der Linde, D.; Barty, C.P.J. Detection of Nonthermal Melting by Ultrafast X-ray Diffraction. Science 1999, 286, 1340–1342. [Google Scholar] [CrossRef] [PubMed]
- Potemkin, F.V.; Mareev, E.I.; Garmatina, A.A.; Nazarov, M.M.; Fomin, E.A.; Stirin, A.I.; Korchuganov, V.N.; Kvardakov, V.V.; Gordienko, V.M.; Panchenko, V.Y.; et al. Hybrid X-Ray Laser-Plasma/Laser-Synchrotron Facility for Pump-Probe Studies of the Extreme State of Matter at NRC “Kurchatov Institute”. Rev. Sci. Instrum. 2021, 92, 053101. [Google Scholar] [CrossRef] [PubMed]
- Schropp, A.; Hoppe, R.; Meier, V.; Patommel, J.; Seiboth, F.; Ping, Y.; Hicks, D.G.; Beckwith, M.A.; Collins, G.W.; Higginbotham, A.; et al. Imaging Shock Waves in Diamond with Both High Temporal and Spatial Resolution at an XFEL. Sci. Rep. 2015, 5, 11089. [Google Scholar] [CrossRef]
- Inoue, I.; Inubushi, Y.; Sato, T.; Tono, K.; Katayama, T.; Kameshima, T.; Ogawa, K.; Togashi, T.; Owada, S.; Amemiya, Y.; et al. Observation of Femtosecond X-ray Interactions with Matter Using an X-ray-X-ray Pump-Probe Scheme. Proc. Natl. Acad. Sci. USA 2016, 113, 1492–1497. [Google Scholar] [CrossRef]
- Holtz, M.; Hauf, C.; Hernández Salvador, A.A.; Costard, R.; Woerner, M.; Elsaesser, T. Shift-Current-Induced Strain Waves in LiNbO3 Mapped by Femtosecond X-Rray Diffraction. Phys. Rev. B 2016, 94, 104302. [Google Scholar] [CrossRef]
- Weisshaupt, J.; Juvé, V.; Holtz, M.; Ku, S.; Woerner, M.; Elsaesser, T.; Baltuška, A. High-brightness table-top hard X-ray source driven by sub-100-femtosecond mid-infrared pulses. Nat. Photonics 2014, 8, 927–930. [Google Scholar] [CrossRef]
- Martín, L.; Benlliure, J.; Cortina-Gil, D.; Haruna, A.; Ruiz, C. Validation of a Laser Driven Plasma X-ray Microfocus Source for High Resolution Radiography Imaging. Phys. Medica 2021, 82, 163–170. [Google Scholar] [CrossRef]
- Banchi, L.; Rossi, F.; Ferianis, M.; Bogoni, A.; Potì, L.; Ghelfi, P. Synchronization of 3GHZ Repetition Rate Harmonically Mode-Locked Fiber Laser for Optical Timing Applications. In Proceedings of the 8th European Workshop Beam Diagnostics Instrumentation Particle Accelerator DIPAC 2007, Venezia, Italy, 20–23 May 2007; pp. 358–360. [Google Scholar]
- Gerson, R.; Kirchhoff, J.F.; Halliburton, L.E.; Bryan, D.A. Photoconductivity Parameters in Lithium Niobate. J. Appl. Phys. 1986, 60, 3553–3557. [Google Scholar] [CrossRef]
- Gerson, R.; Bryan, A.; Douglas, M.; Louis, S. Bulk Photovoltaic Effect in Commercial Lithium Niobate Crystals. SPIE 1983, 380, 261–265. [Google Scholar]
- Rapp, L.; Haberl, B.; Pickard, C.J.; Bradby, J.E.; Gamaly, E.G.; Williams, J.S.; Rode, A.V. Experimental Evidence of New Tetragonal Polymorphs of Silicon Formed through Ultrafast Laser-Induced Confined Microexplosion. Nat. Commun. 2015, 6, 7555. [Google Scholar] [CrossRef] [PubMed]
- Liu, Y.; Ding, Y.; Xie, J.; Xu, L.; Wha Jeong, I.; Yang, L. One-Step Femtosecond Laser Irradiation of Single-Crystal Silicon: Evolution of Micro-Nano Structures and Damage Investigation. Mater. Des. 2023, 225, 111443. [Google Scholar] [CrossRef]
- Kovalchuk, M.V.; Borisov, M.M.; Garmatina, A.A.; Gordienko, V.M.; Zheltikov, A.M.; Kvardakov, V.V.; Korchuganov, V.N.; Likhachev, I.A.; Mareev, E.I.; Mitrofanov, A.V.; et al. Laser-Synchrotron Facility of the National Research Centre “Kurchatov Institute”. Crystallogr. Rep. 2022, 67, 717–728. [Google Scholar] [CrossRef]
- Norman, G.E.; Starikov, S.V.; Stegailov, V.V.; Saitov, I.M.; Zhilyaev, P.A. Atomistic Modeling of Warm Dense Matter in the Two-Temperature State. Contrib. Plasma Phys. 2013, 53, 129–139. [Google Scholar] [CrossRef]
| Method | Cost | Scalability | Ease of Implementation | Time Step | Maximal Time Delay | Jitter |
|---|---|---|---|---|---|---|
| PLL | High | High | Hard | <1 ps | Variable | ~10–100 fs |
| FPGA based | Low | High | Easy | ~100 ps | Not limited | ~100 ps |
| Analog | Average | Extremely low | Average | ~100 ps | Variable | ~100 ps |
| All optical | Variable | Low | Variable | ~10 fs | ~10 ns | ~1 fs |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Marchenkov, N.; Mareev, E.; Kulikov, A.; Pilyak, F.; Ibragimov, E.; Pisarevskii, Y.; Potemkin, F. Hybrid Approach for Multiscale and Multimodal Time-Resolved Diagnosis of Ultrafast Processes in Materials via Tailored Synchronization of Laser and X-ray Sources at MHz Repetition Rates. Optics 2024, 5, 1-10. https://doi.org/10.3390/opt5010001
Marchenkov N, Mareev E, Kulikov A, Pilyak F, Ibragimov E, Pisarevskii Y, Potemkin F. Hybrid Approach for Multiscale and Multimodal Time-Resolved Diagnosis of Ultrafast Processes in Materials via Tailored Synchronization of Laser and X-ray Sources at MHz Repetition Rates. Optics. 2024; 5(1):1-10. https://doi.org/10.3390/opt5010001
Chicago/Turabian StyleMarchenkov, Nikita, Evgenii Mareev, Anton Kulikov, Fedor Pilyak, Eduard Ibragimov, Yuri Pisarevskii, and Fedor Potemkin. 2024. "Hybrid Approach for Multiscale and Multimodal Time-Resolved Diagnosis of Ultrafast Processes in Materials via Tailored Synchronization of Laser and X-ray Sources at MHz Repetition Rates" Optics 5, no. 1: 1-10. https://doi.org/10.3390/opt5010001
APA StyleMarchenkov, N., Mareev, E., Kulikov, A., Pilyak, F., Ibragimov, E., Pisarevskii, Y., & Potemkin, F. (2024). Hybrid Approach for Multiscale and Multimodal Time-Resolved Diagnosis of Ultrafast Processes in Materials via Tailored Synchronization of Laser and X-ray Sources at MHz Repetition Rates. Optics, 5(1), 1-10. https://doi.org/10.3390/opt5010001







