Active Optics—Progress in Modeling of Tulip-like Variable Curvature Mirrors
Abstract
:1. Introduction
2. Theory—Thin Circular Plate VCMs
2.1. Preliminarily Definition of the Curvature Mode
2.2. Analytic Theory—VCM with Constant Thickness Distribution (CTD)
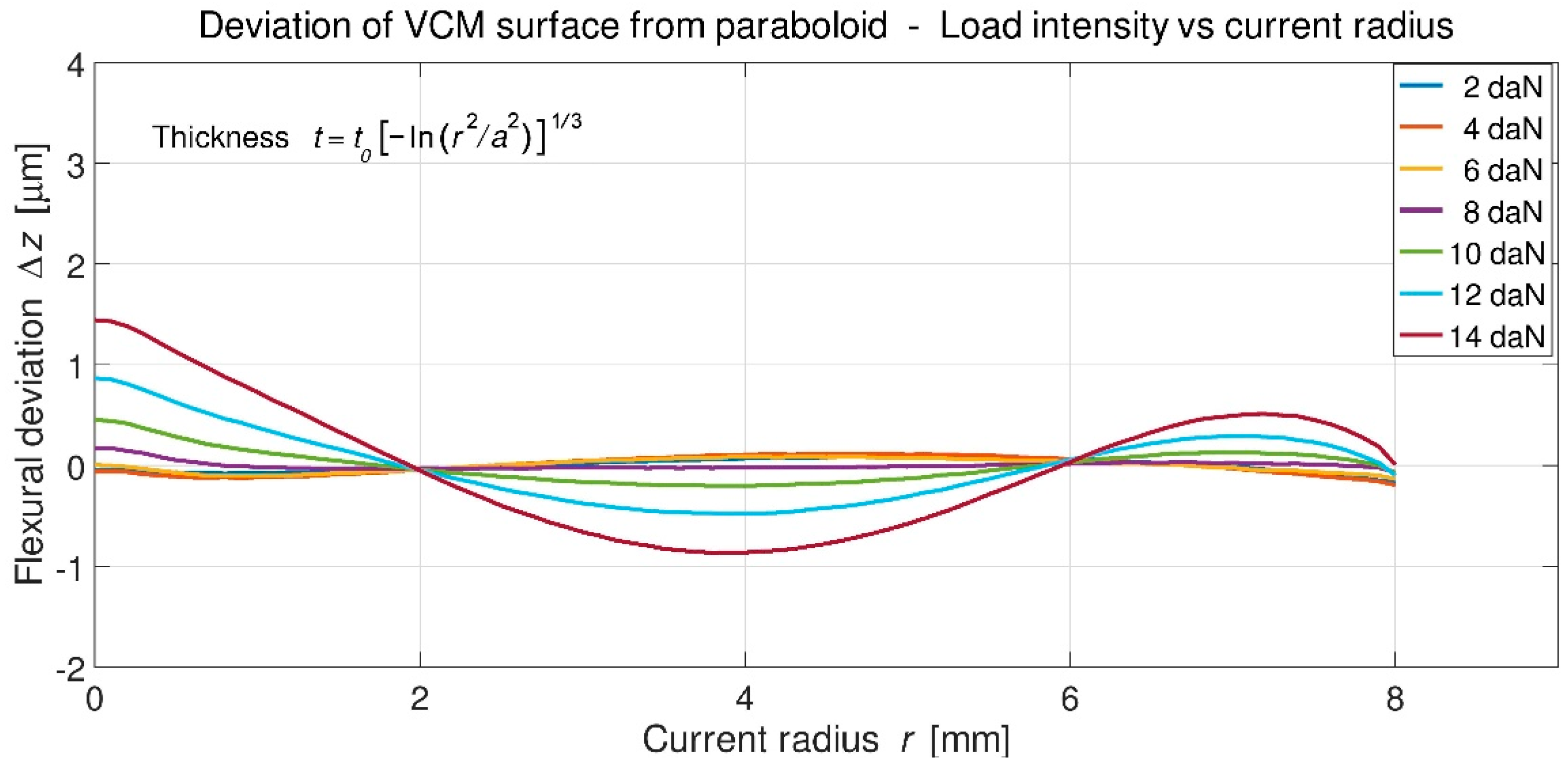
2.3. Analytic Theory—VCMs with Variable Thickness Distribution (VTD)
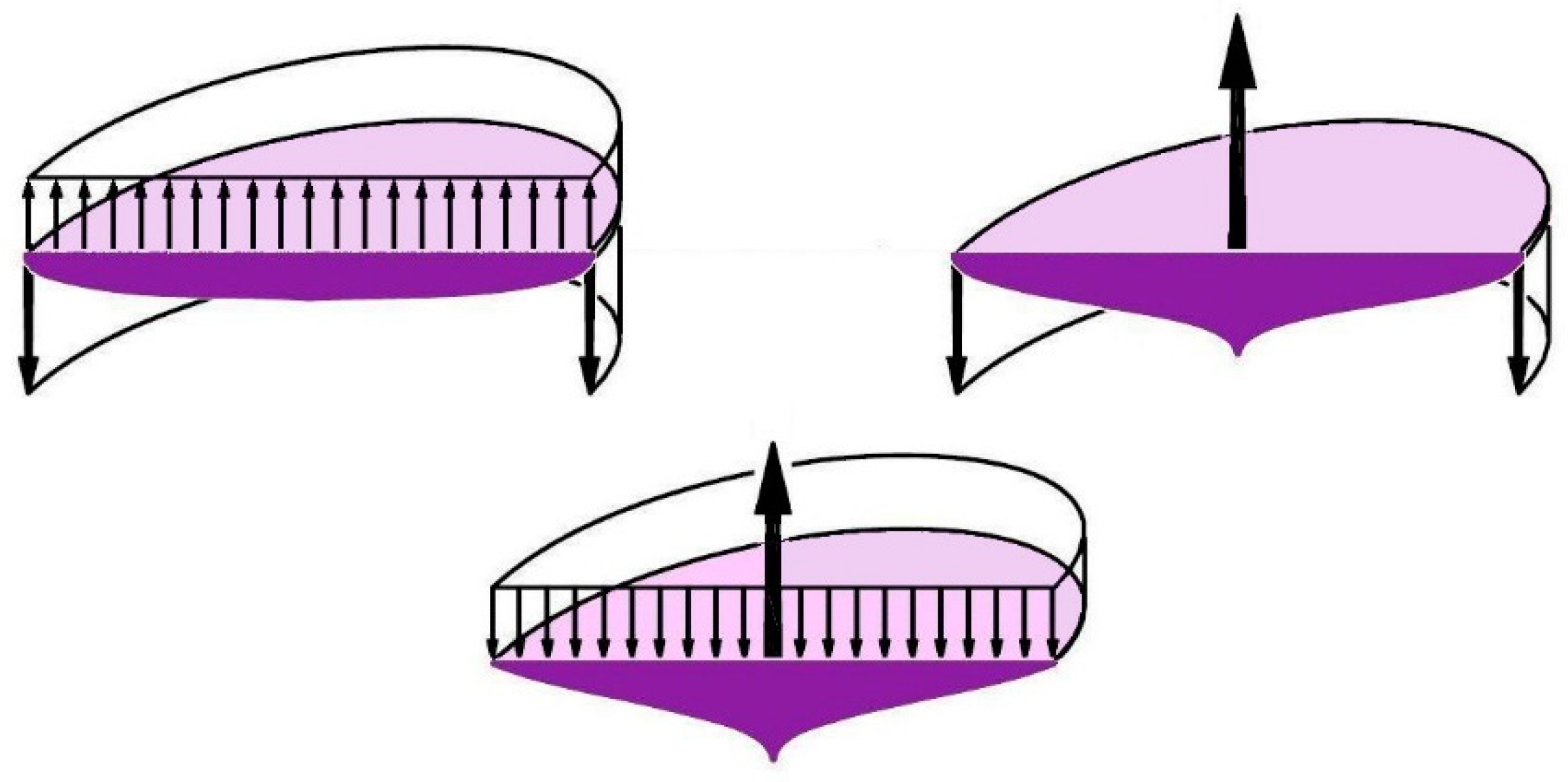
2.4. Tulip-like VCM with a Central Force and Edge Reaction
3. Optical Focal Ratio, Buckling Instability, VCM Zoom Range and Metal Choice
3.1. Optical f-Ratio
3.2. Buckling Instability
3.3. VCM Zoom Range
3.4. Metal Choice
4. Simulation Methodology—FEA of a Tulip-like VCM Bent by a Central Force
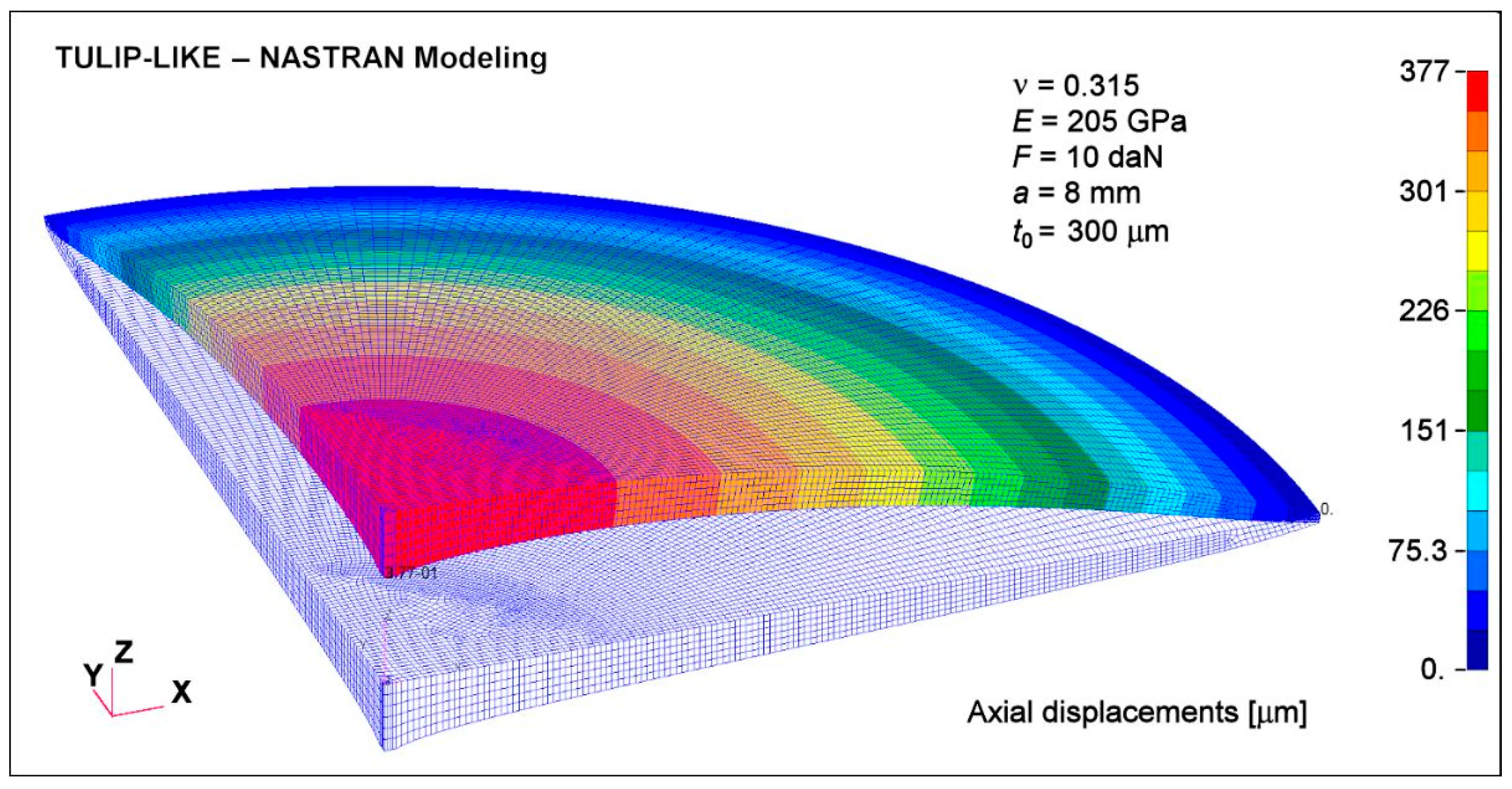
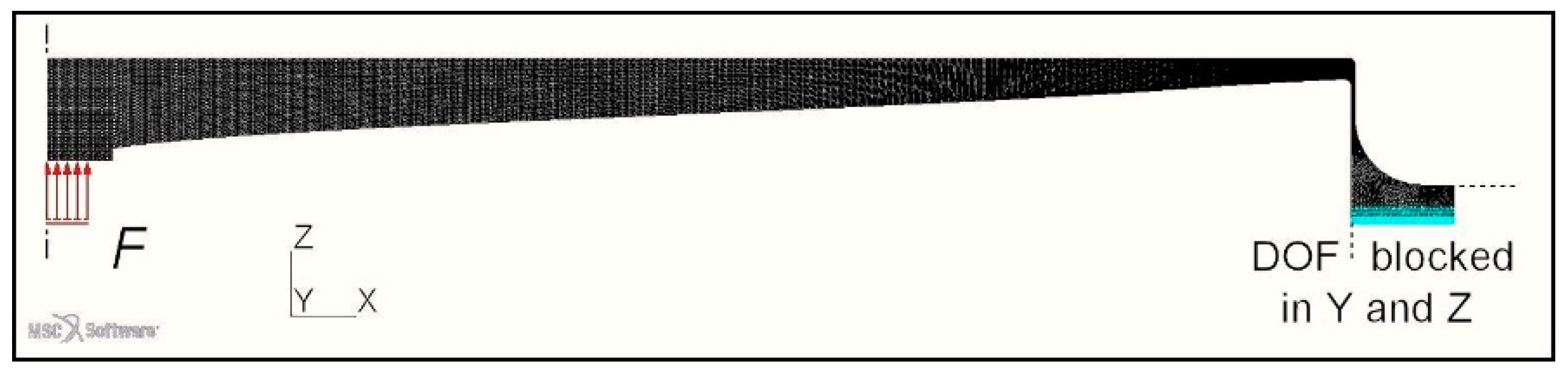
4.1. Modeling of VCM for the Plate Alone
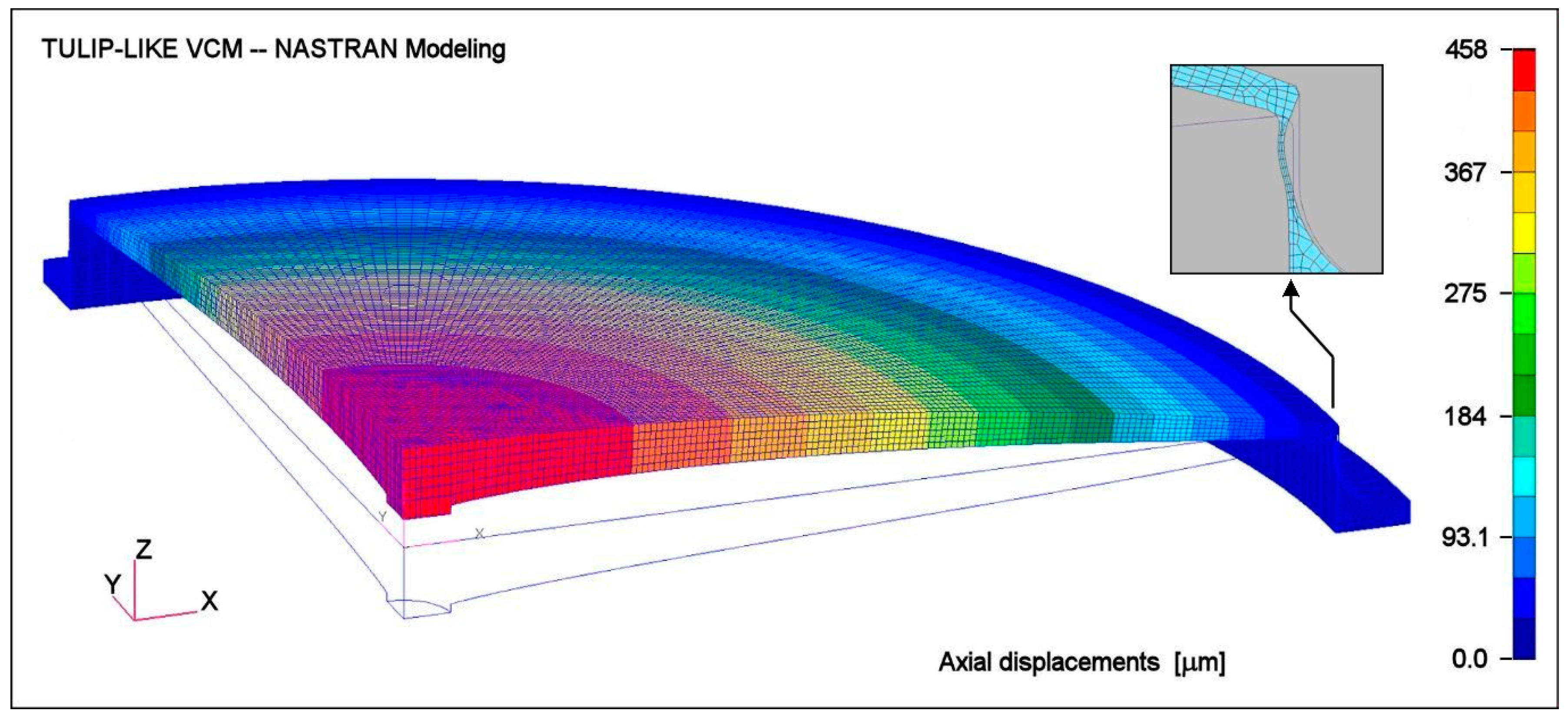
4.2. Modeling of VCM with an Outer Cylinder Collarette and Rigid Ring
5. Simulation Results
5.1. Final Data of the Tulip-like VCM Modeling
5.2. Maximum Stresses, Pre-Stressing and Creep Deformation
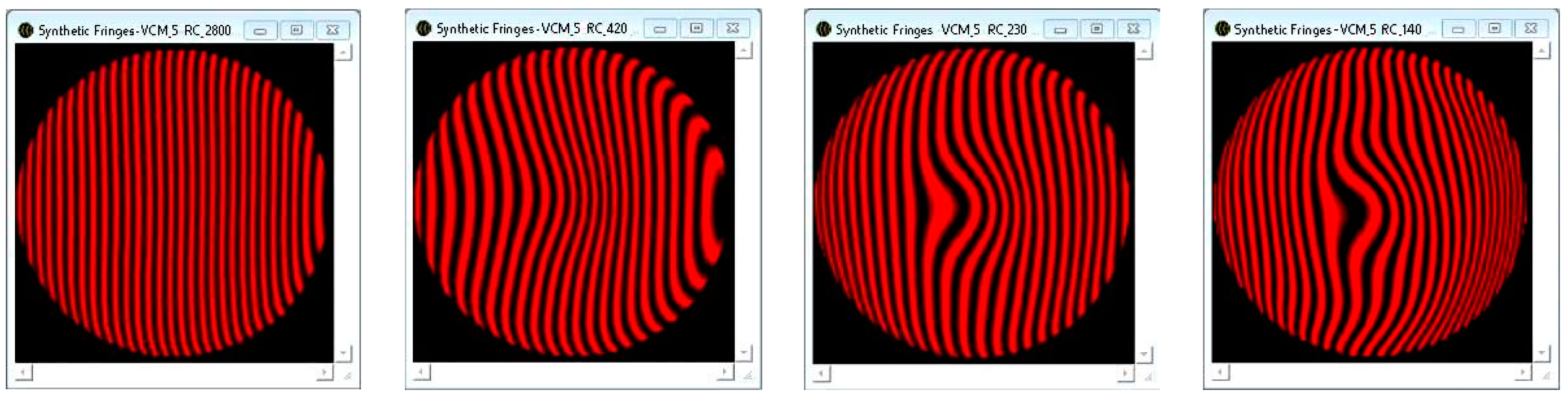
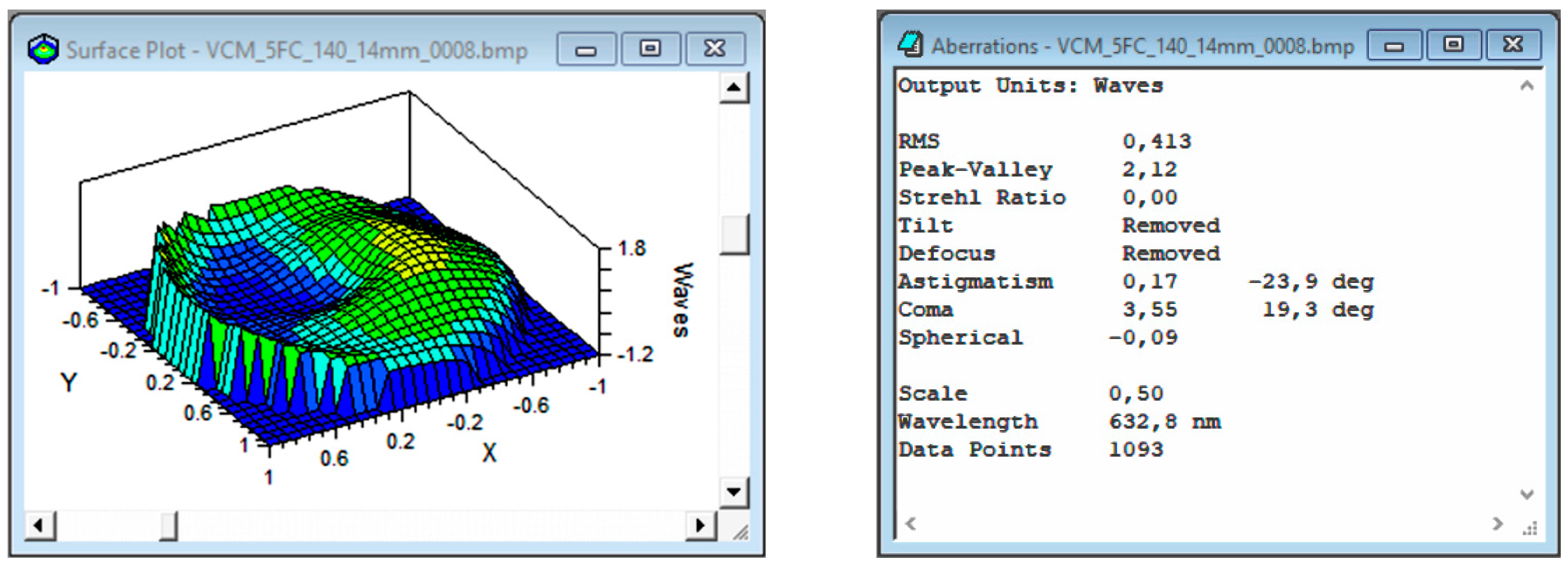
6. Experimental Results
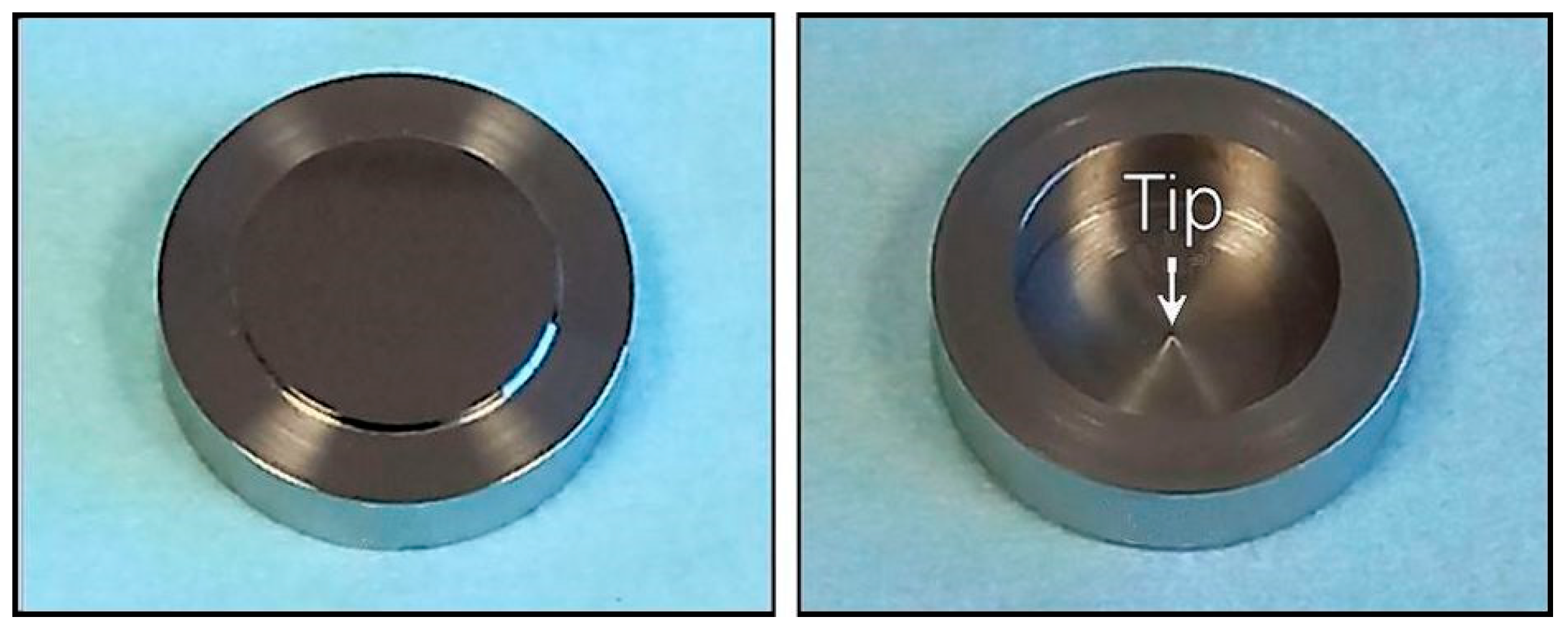
6.1. Mechanical Assembly of a Prototype and Actuator
6.2. Results from Realization and Optical Testing
7. Conclusions
8. Patents
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Lemaitre, G.R. Elasticité et miroirs à focale variable. Comptes Rendus Académie Des. Sci. 1976, 282B, 87–89. Available online: https://gallica.bnf.fr/ark:/12148/bpt6k62352030/f101.item (accessed on 1 June 2022).
- Lemaitre, G.R. Elasticity Theory and Astronomical Optics—Active Optics Methods; Springer-Verlag Edt.: Heidelberg, Germany, 2009; ISBN 978-3-540-68905-8. [Google Scholar]
- Ferrari, M.; Lemaitre, G.R. Analysis of large deflection zoom mirrors for the ESO VLTI. Astron. Astrophys. 1993, 274, 12–18. Available online: https://adsabs.harvard.edu/full/1993A%26A...274...12F (accessed on 1 June 2022).
- Ferrari, M. Development of variable curvature mirrors for the delay lines of the VLTI. Astron. Astrophys. Suppl. Ser. 1998, 128, 221–227. [Google Scholar] [CrossRef] [Green Version]
- Belomestnov, P.I.; Vyazovich, E.I.; Soloukhin, R.I.; Yakobi, Y.A. Tunable resonator with a mirror of variable curvature. Sov. J. Quantum Electron. 1974, 3, 347–348. [Google Scholar] [CrossRef]
- Ziegler, E.; Hignette, O.; Morawe, C.; Tucoulou., R. Miroir simple ou multi-couches à courbure variable pour microfocalisation de rayonnement synchrotron X. J. Phys. IV France 2001, 11, 7–21. [Google Scholar] [CrossRef]
- Kamachi, N.; Endo, K.; Ohashiound, H.; Ishikawaound, T. Characteristics of mechanically—Bent—Shaped mirror: Experimental study on stability using LTP II. In AIP Conference Proceedings; American Institute of Physics: New York, NY, USA, 2004; Volume 705, p. 788. [Google Scholar] [CrossRef]
- Xie, X.; Hugot, E.; Li, C.; Xu, L.; Lemared, S.; Liu, M.; Fan, X.; Zhao, H. Elastic bending of variable curvature mirrors: Validation of a simplified analytical method. Appl. Opt. 2019, 58, 7121–7126. [Google Scholar] [CrossRef] [PubMed]
- Lemaitre, G.R.; Lanzoni, P. Active optics—Freeform segment mirror replications from a deformable matrix. Photonics 2022, 9, 206. [Google Scholar] [CrossRef]
- Timoshenko, S.P.; Woinowsky-Krieger, S. Theory of Plates and Shells; McGraw-Hill Edt.: NewYork, NY, USA, 1959; ISBN 0-07-085820-9. [Google Scholar]
- Ugitech Corp. UGIMA 4028W. 1990. Available online: https://eservices.ugitech.com/CatalogDocuments/BarProducts.pdf (accessed on 1 June 2022).
- MSC Software Paris. Parc Technopolis—Immeuble Gamma, 3 Avenue du Canada, 91978 Courtaboeuf—Les Ulis, France. Available online: https://www.mscsoftware.com/fr/terms-and-conditions-use-and-disclaimer (accessed on 1 June 2022).
- Aubert & Duval Corp. Strength of Quenched Chromium Stainless Steel. 2000. Available online: https://www.aubertduval.com/wp-media/uploads/sites/2/pdf/fr_X13.pdf (accessed on 1 June 2022).
- Yadav, H.K.; Yadav, H.K.; Ballal, A.R.; Thawre, M.M.; Vijayanand, V.D. Creep studies of Cold Worked Austenitic Stainless Steel. In Procedia Structural Integrity; Elsevier Edt.: Amsterdam, The Netherlands, 2019; Volume 14, pp. 605–611. [Google Scholar] [CrossRef]
- Micro-Controle-Spectra Physics Corp. Now Associated with Newport Corp., Is Based at ZI, 7 Rue Des Plantes, 45340 Beaune-La-Rolande, France. Available online: https://pdf.directindustry.fr/pdf/micro-controle-spectra-physics-7436.html (accessed on 1 June 2022).
- Lemaitre, G.R.; Mazzanti, S.; Ferrari, M. Tulip form variable curvature mirrors: Interferometry and field compensation. In Proceedings of the SPIE Conference on Astronomical Interferometry, Kona, HI, USA, 24 July 1998; Volume 3350, pp. 373–380. [Google Scholar] [CrossRef]
- Lemaitre, G.R.; Vola, P.; Lanzoni, P.; Mazzanti, M.; Dérie, F.J.; Gonté, F.Y. Active optics—Progress and modeling of cycloid-like variable curvature mirrors for the VLTI Array. Photonics 2022, 9, 66. [Google Scholar] [CrossRef]




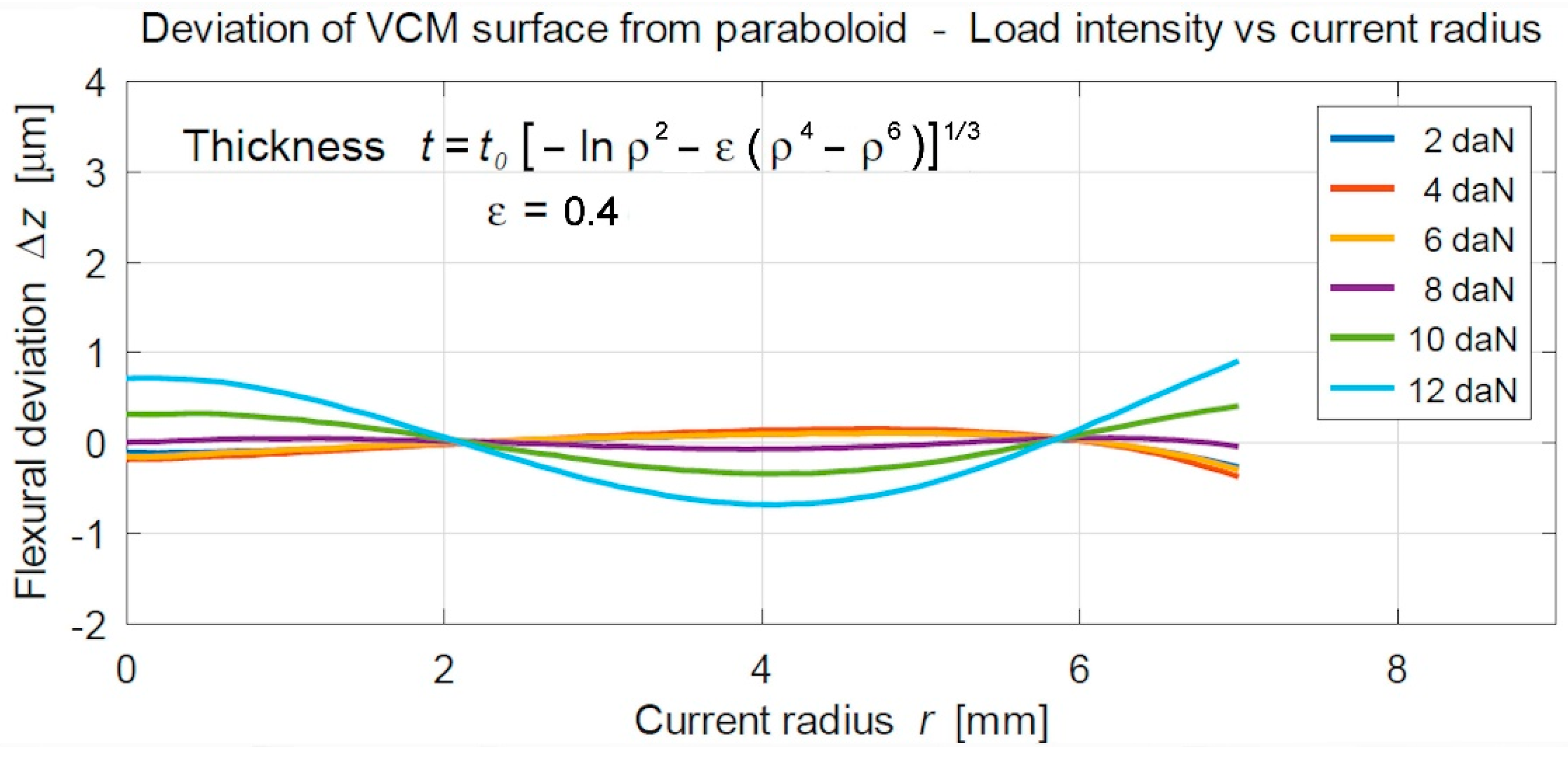
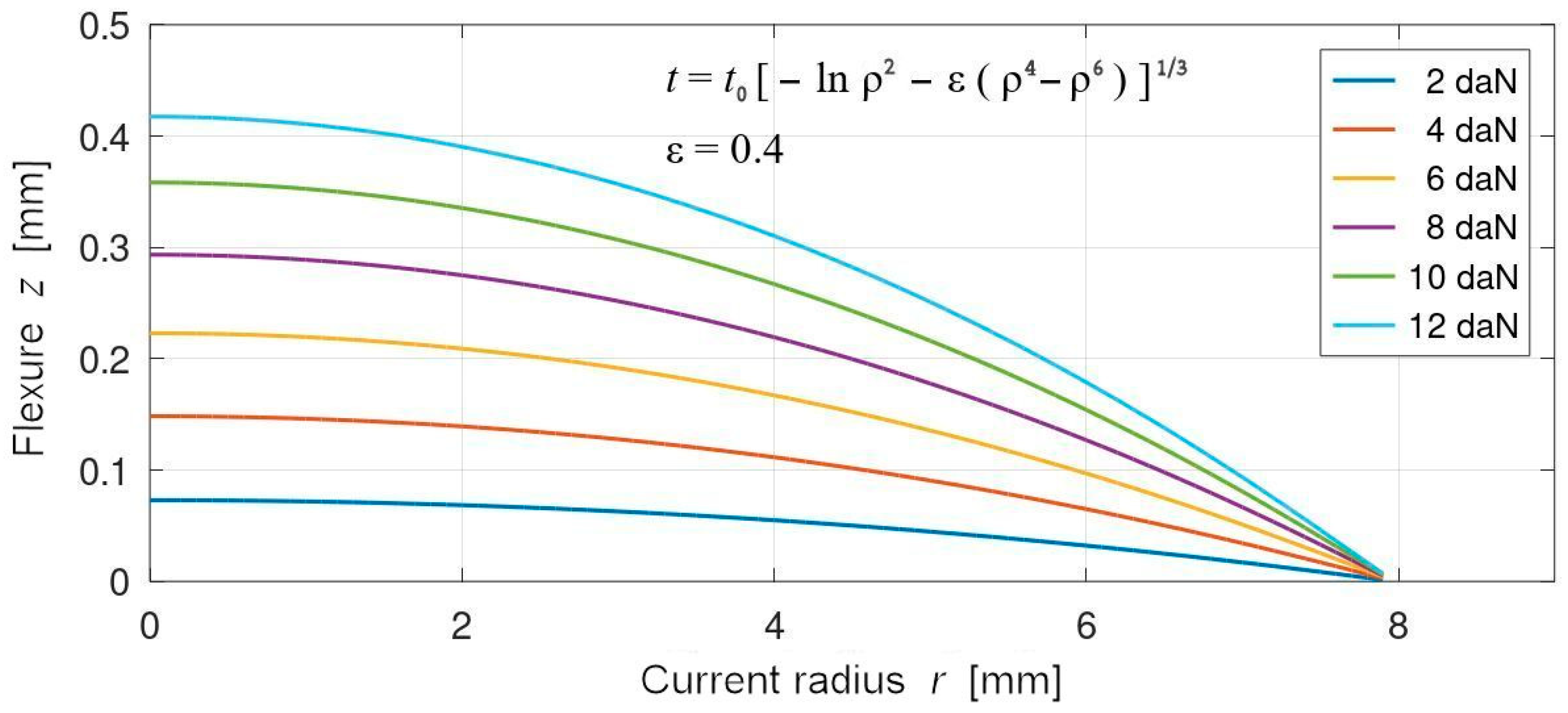




| t/t0 = [−ln ρ2 − ε (ρ4 – ρ6)]1/3 for 0.4 < r = ρ, a < 7.2 mm. Conical 7.2 < r < 8, ε = 0.4 | ||||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| Units: r [mm] t, t* [μm] | ||||||||||
| R | 0 | 0.4− | 0.4+ | 4 | 6 | 7.2 | 8− | 8 | 8+ | 12 |
| t | 618 | 618 | 545 | 333 | 241 | 163 | 120 | 6750 | 6000 | 6000 |
| t* | 628 | 628 | 555 | 343 | 251 | 173 | 130 | 6750 | 6000 | 6000 |
| Poisson ratio | ν = 0.315 | Young’s modulus | E = 205 GPa |
| Mean thickness | t0 = 300 µm | Nastran optimal profile | 0.4 < r < 7.2 mm |
| Central cut thickness | tc = 618 µm | Collarette radial thickness | Δr = 20 µm |
| Force range | F ≤ 12 daN | Collarette stress max | σmax = 920 MPa |
| Flexural sag F = 10 daN | z0 = 355 µm | Radius of curvature | R = 90.1 mm |
| Outer diameter | 2a = 16 mm | Zoom f-ratio | f/∞–f/2.82 |
| Clear aperture dia. | dOpt = 13 mm | Zoom f-ratio | f/∞–f/3.47 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lemaitre, G.R.; Vola, P.; Lanzoni, P. Active Optics—Progress in Modeling of Tulip-like Variable Curvature Mirrors. Optics 2023, 4, 39-54. https://doi.org/10.3390/opt4010004
Lemaitre GR, Vola P, Lanzoni P. Active Optics—Progress in Modeling of Tulip-like Variable Curvature Mirrors. Optics. 2023; 4(1):39-54. https://doi.org/10.3390/opt4010004
Chicago/Turabian StyleLemaitre, Gerard R., Pascal Vola, and Patrick Lanzoni. 2023. "Active Optics—Progress in Modeling of Tulip-like Variable Curvature Mirrors" Optics 4, no. 1: 39-54. https://doi.org/10.3390/opt4010004
APA StyleLemaitre, G. R., Vola, P., & Lanzoni, P. (2023). Active Optics—Progress in Modeling of Tulip-like Variable Curvature Mirrors. Optics, 4(1), 39-54. https://doi.org/10.3390/opt4010004







