New Evidence for a Nonclassical Behavior of Laser Multimode Light
Abstract
:1. Introduction
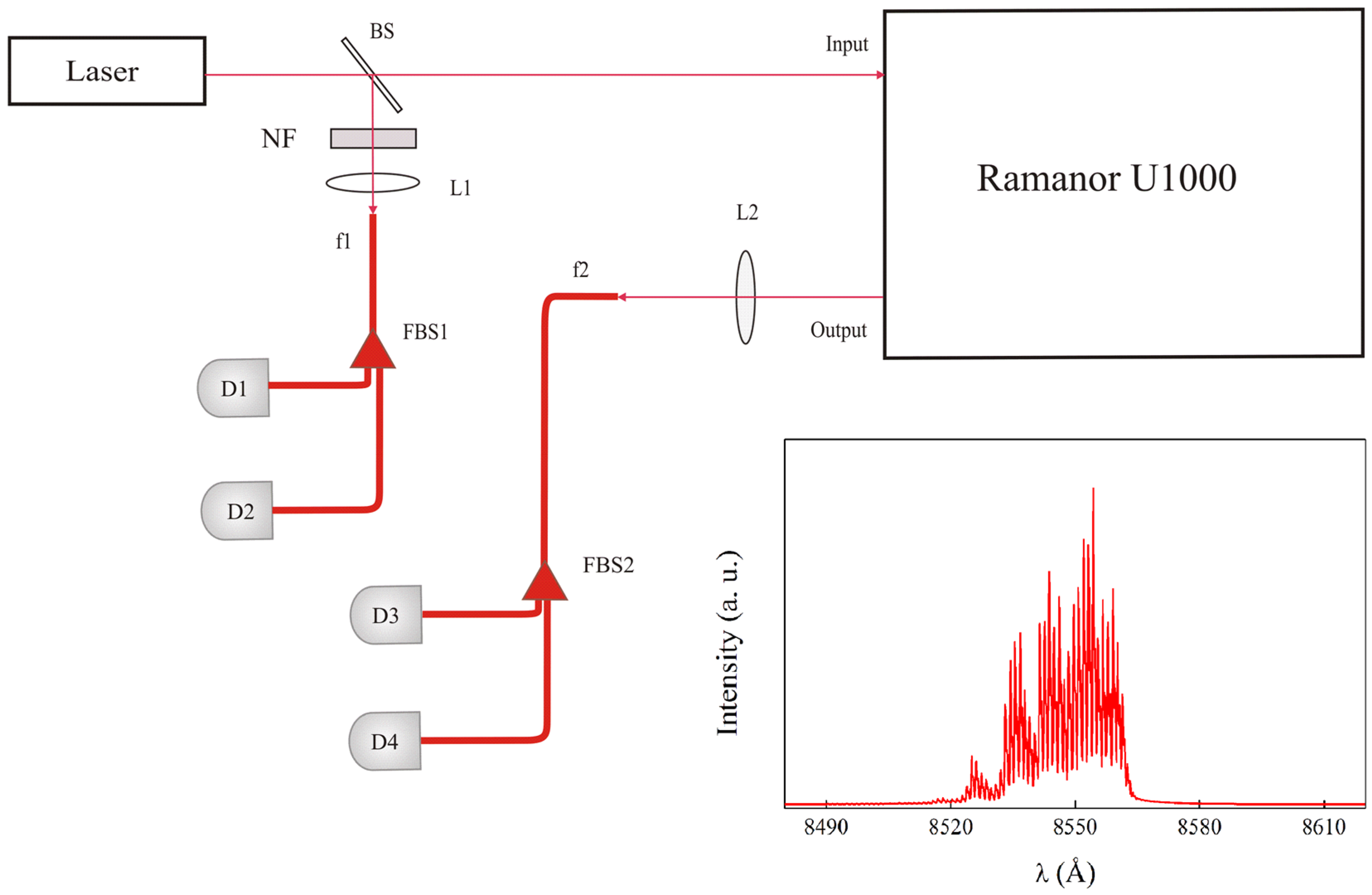
2. Experiments and Methods
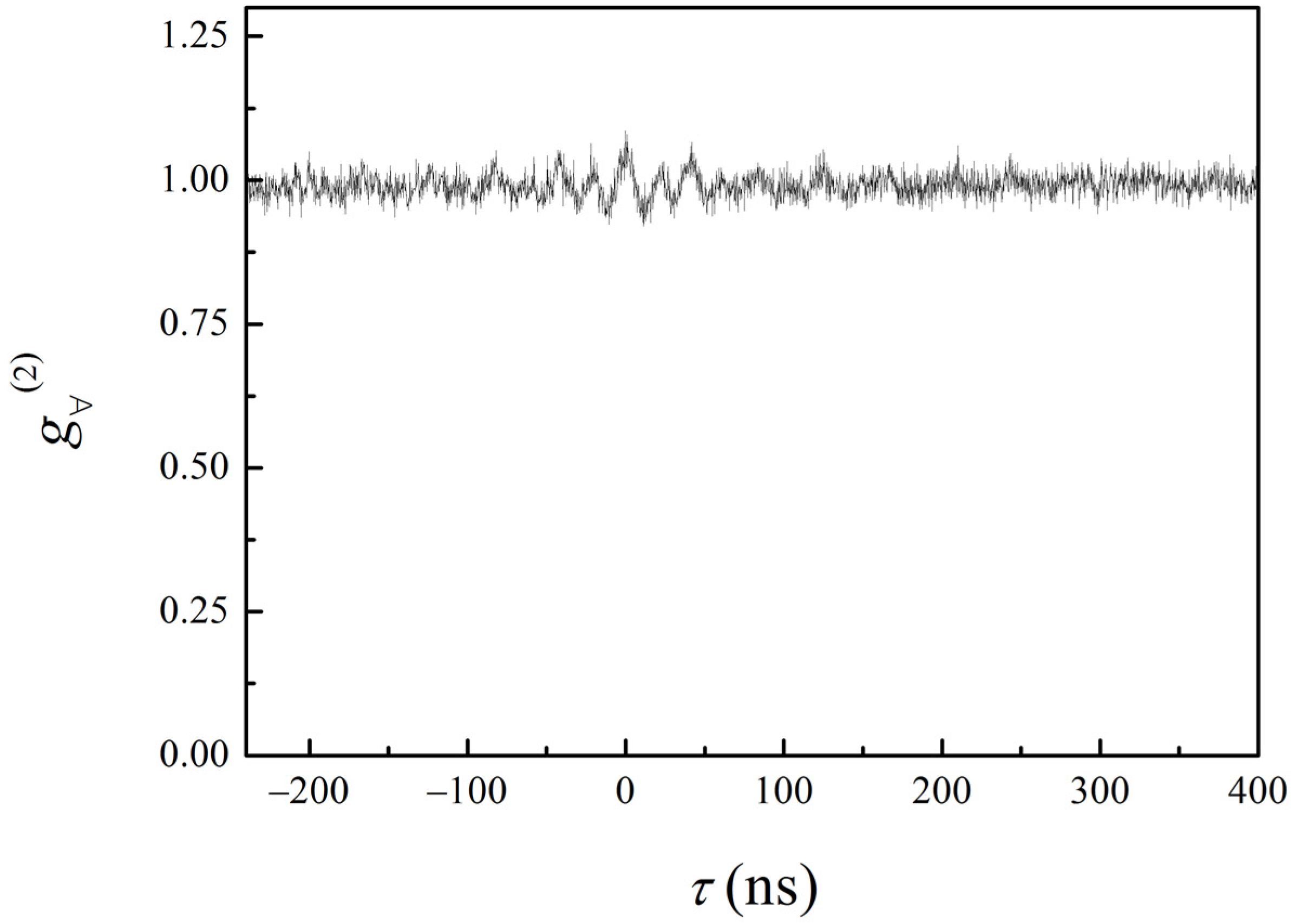
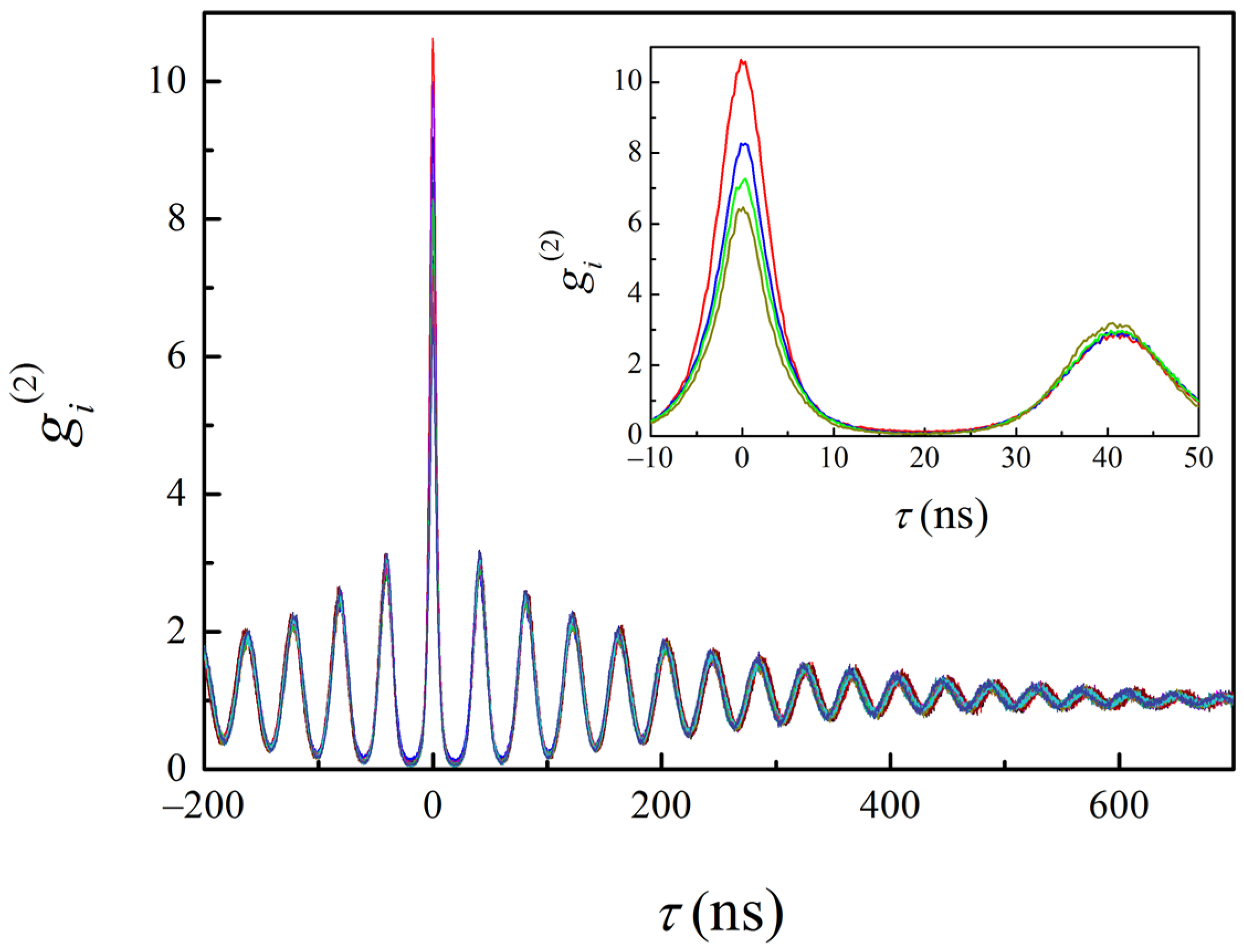
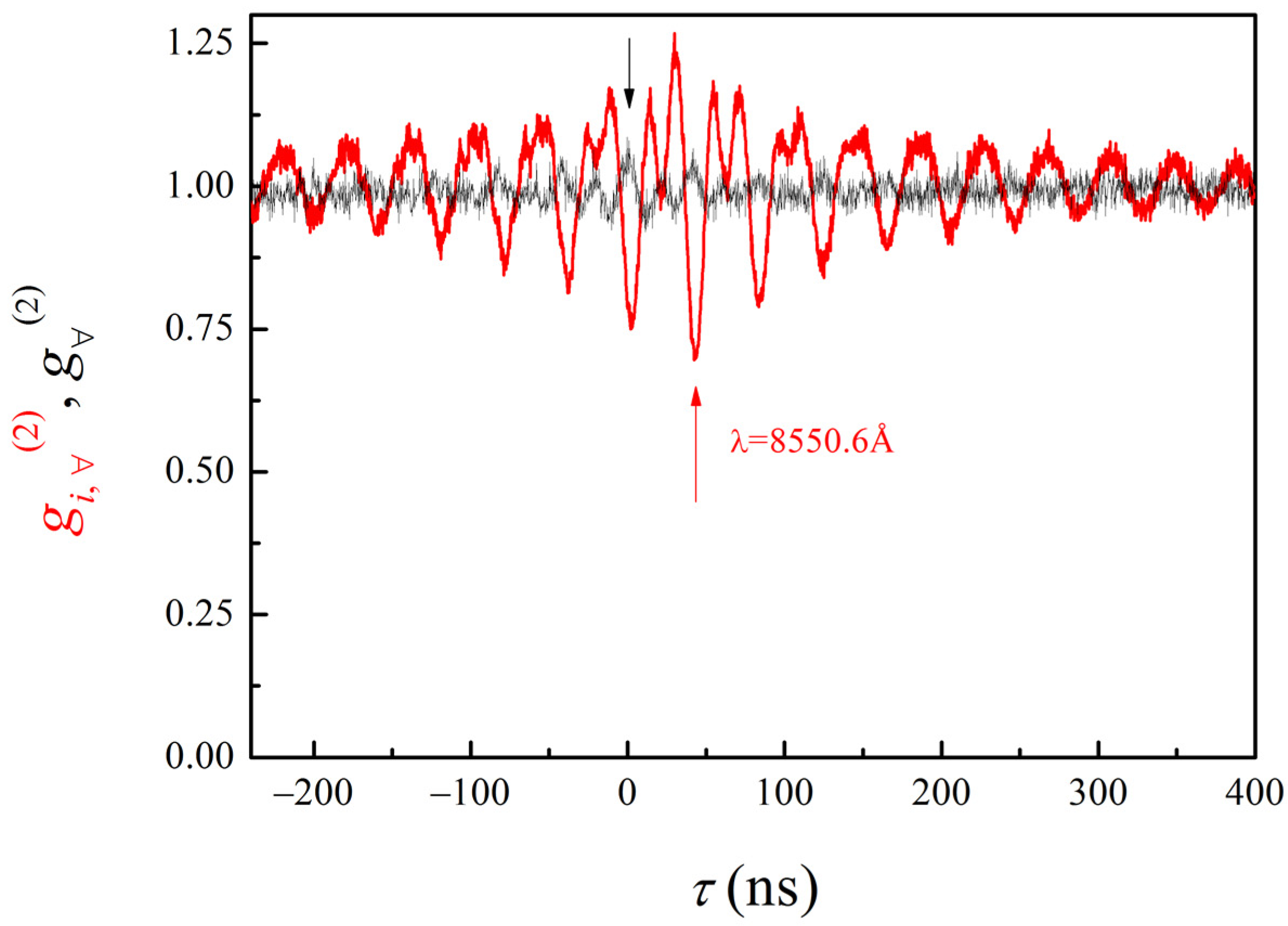
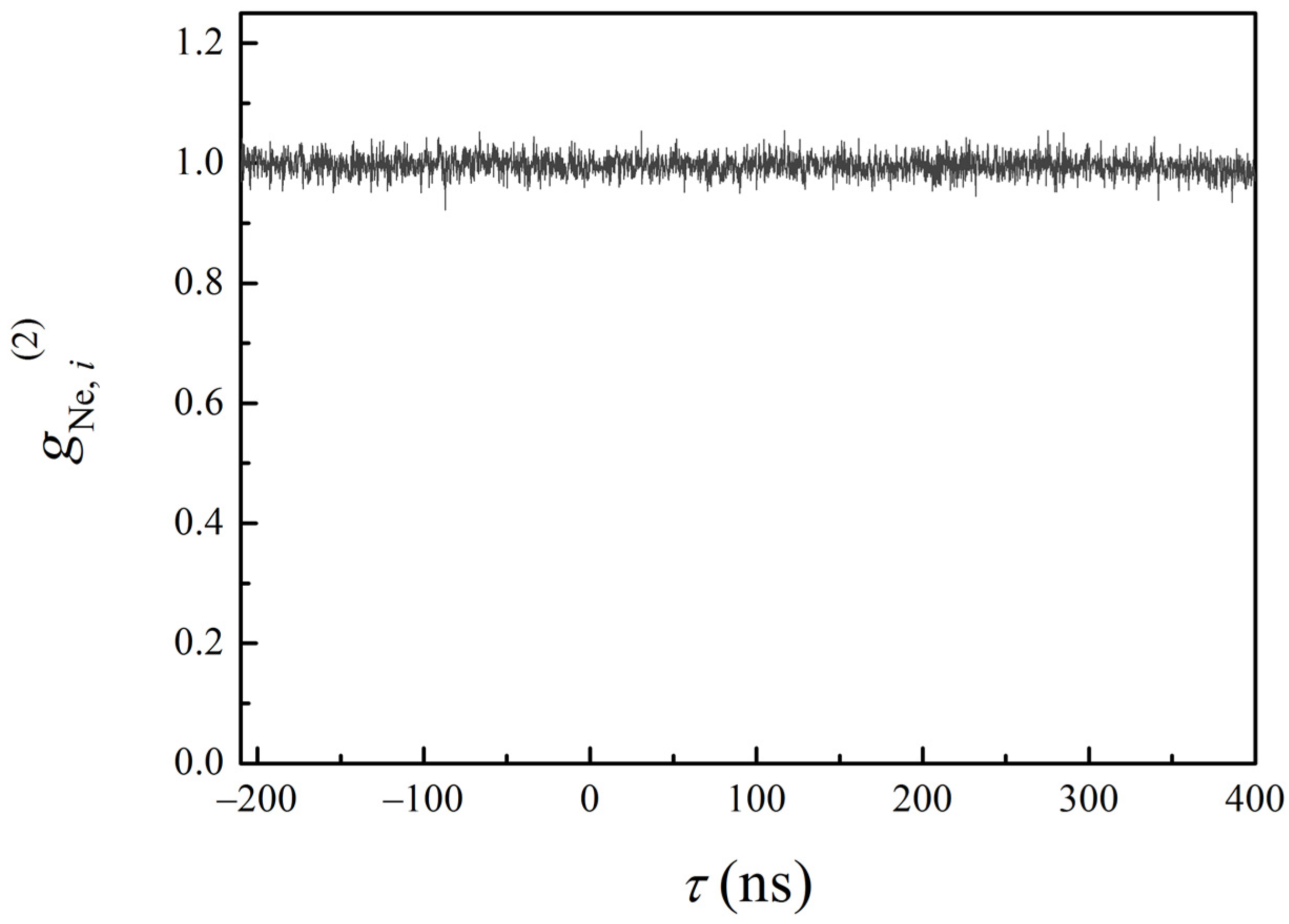
3. Results
4. Discussion
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Klyshko, D.N. The nonclassical light. Phys. Uspekhi. 1996, 39, 573–596. [Google Scholar] [CrossRef]
- Pirandola, S.; Andersen, U.L.; Banchi, L.; Berta, M.; Bunandar, D.; Colbeck, R.; Englund, D.; Gehring, T.; Lupo, C.; Ottaviani, C.; et al. Advances in quantum cryptography. Adv. Opt. Photonics 2020, 12, 1012–1236. [Google Scholar] [CrossRef] [Green Version]
- Ladd, T.D.; Jelezko, F.; Laflamme, R.; Nakamura, Y.; Monroe, C.; O’Brien, J.L. Quantum computers. Nature 2010, 464, 45–53. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Pirandola, S.; Eisert, J.; Weedbrook, C.; Furusawa, A.; Braunstein, S.L. Advances in Quantum Teleportation. Nat. Photonics 2015, 9, 641–652. [Google Scholar] [CrossRef]
- Zwinkels, J.C.; Ikonen, E.; Fox, N.P.; Ulm, G.; Rastello, M.L. Photometry, radiometry and ‘the candela’: Evolution in the classical and quantum world. Metrologia 2010, 47, R15–R32. [Google Scholar] [CrossRef] [Green Version]
- Kwiat, P.; Mattle, K.; Weinfurter, H.; Zeilinger, A.; Sergienko, A.V.; Shih, Y. New High-Intensity Source of Polarization-Entangled Photon Pairs. Phys. Rev. Lett. 1995, 75, 4337–4341. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Bock, M.; Lenhard, A.; Chunnilall, C.; Becher, C. Highly efficient heralded single-photon source for telecom wavelengths based on a PPLN waveguide. Opt. Express 2016, 24, 23992–24001. [Google Scholar] [CrossRef] [PubMed]
- Knill, E. Quantum computing with realistically noisy devices. Nature 2005, 434, 39–44. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Michler, P.; Kiraz, A.; Becher, C.; Schoenfeld, W.V.; Petroff, P.M.; Hu, E.; Zhang, L.; Imamoglu, A. A quantum dot single-photon turnstile device. Science 2000, 290, 2282–2285. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Andersen, U.L.; Gehring, T.; Marquardt, C.M.; Leuchs, G. 30 years of squeezed light generation. Phys. Scr. 2016, 91, 053001. [Google Scholar] [CrossRef]
- Lebedev, M.V.; Parakhonskii, A.L.; Demenev, A.A. Generation of nonclassical light upon resonant excitation of a semiconductor microcavity. JETP Lett. 2015, 102, 571–576. [Google Scholar] [CrossRef]
- Eleuch, H. Photon statistics of light in semiconductor microcavities. J. Phys. B At. Mol. Opt. Phys. 2008, 41, 055502. [Google Scholar] [CrossRef]
- Demenev, A.A.; Domaretskii, D.R.; Parakhonskii, A.L.; Lebedev, M.V. On the origin of nonclassical light generation upon resonant excitation of a GaAs semiconductor microcavity. JETP Lett. 2017, 106, 549–554. [Google Scholar] [CrossRef]
- Yacomotti, A.M.; Furfaro, L.; Hachair, X.; Pedaci, F.; Giudici, M.; Tredicce, J.; Javaloyes, J.; Balle, S.; Viktorov, E.A.; Mandel, P. Dynamics of multimode semiconductor lasers. Phys. Rev. A 2004, 69, 053816. [Google Scholar] [CrossRef] [Green Version]
- Lebedev, M.V.; Demenev, A.A.; Parakhonsky, A.L.; Misochko, O.V. Multimode semiconductor laser: Quantum versus classical behavior. J. Russ. Laser Res. 2019, 40, 64–70. [Google Scholar] [CrossRef] [Green Version]
- Lebedev, M.; Demenev, A.; Parakhonsky, A.; Misochko, O. A triple correlator of radiation intensities of a multimode semiconductor laser. Optics 2020, 1, 32–39. [Google Scholar] [CrossRef] [Green Version]
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lebedev, M.; Demenev, A.; Parakhonsky, A.; Misochko, O. New Evidence for a Nonclassical Behavior of Laser Multimode Light. Optics 2022, 3, 46-52. https://doi.org/10.3390/opt3010006
Lebedev M, Demenev A, Parakhonsky A, Misochko O. New Evidence for a Nonclassical Behavior of Laser Multimode Light. Optics. 2022; 3(1):46-52. https://doi.org/10.3390/opt3010006
Chicago/Turabian StyleLebedev, Michael, Andrey Demenev, Andrey Parakhonsky, and Oleg Misochko. 2022. "New Evidence for a Nonclassical Behavior of Laser Multimode Light" Optics 3, no. 1: 46-52. https://doi.org/10.3390/opt3010006
APA StyleLebedev, M., Demenev, A., Parakhonsky, A., & Misochko, O. (2022). New Evidence for a Nonclassical Behavior of Laser Multimode Light. Optics, 3(1), 46-52. https://doi.org/10.3390/opt3010006





