Broadband Variable Transmission Sphere for Fizeau Interferometry
Abstract
1. Introduction
1.1. Transmission Spheres in Fizeau Interferometry
1.2. Alvarez Lenses
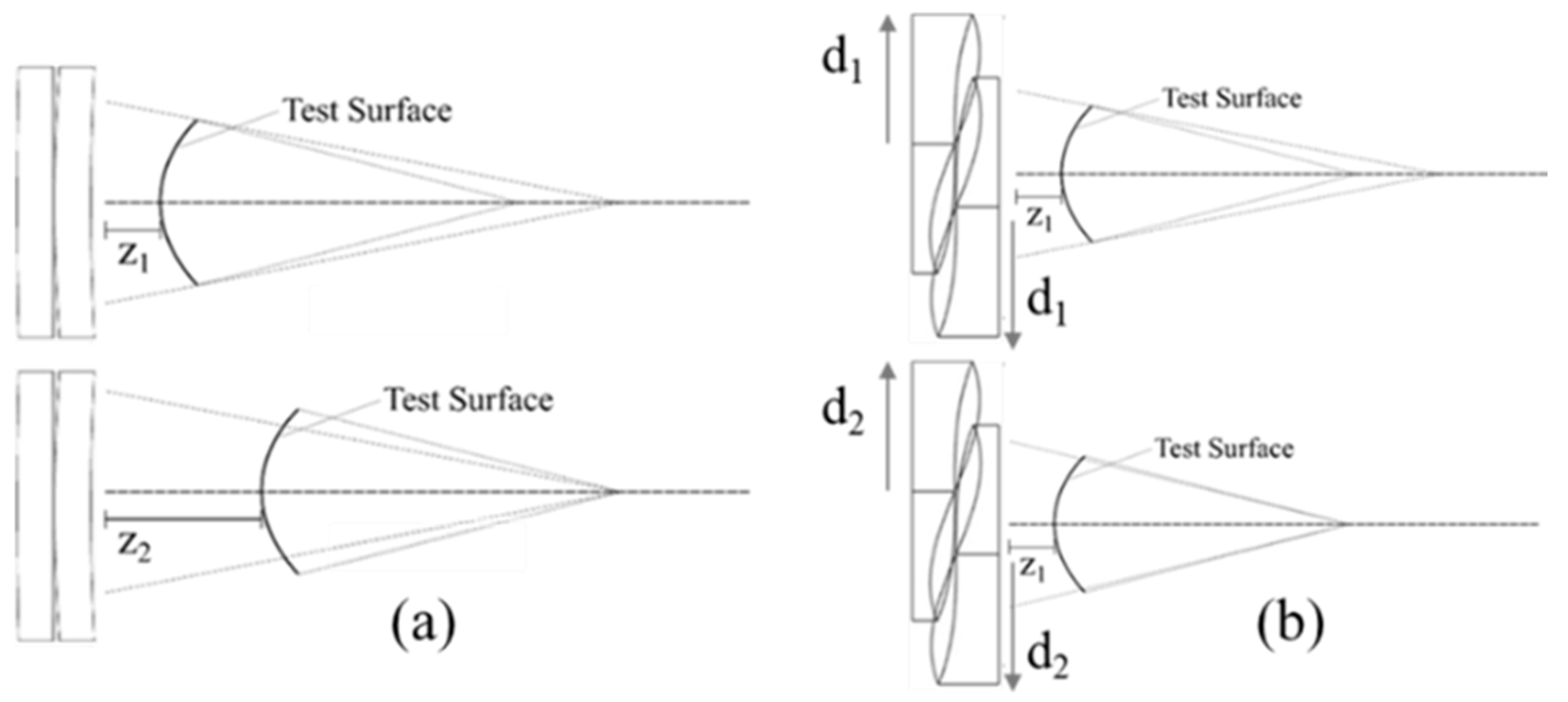
2. Design of BVTS Systems
2.1. System Parameters
2.2. BVTS Example 1
2.3. BVTS Example 2
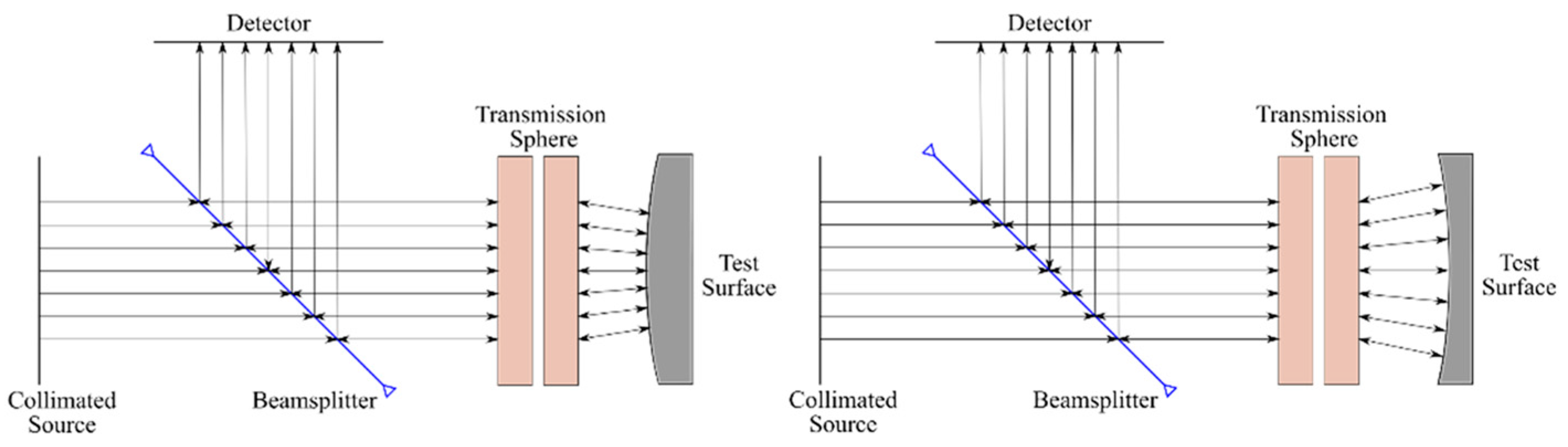
3. Fizeau Interferometer Simulation
4. Results and Discussion
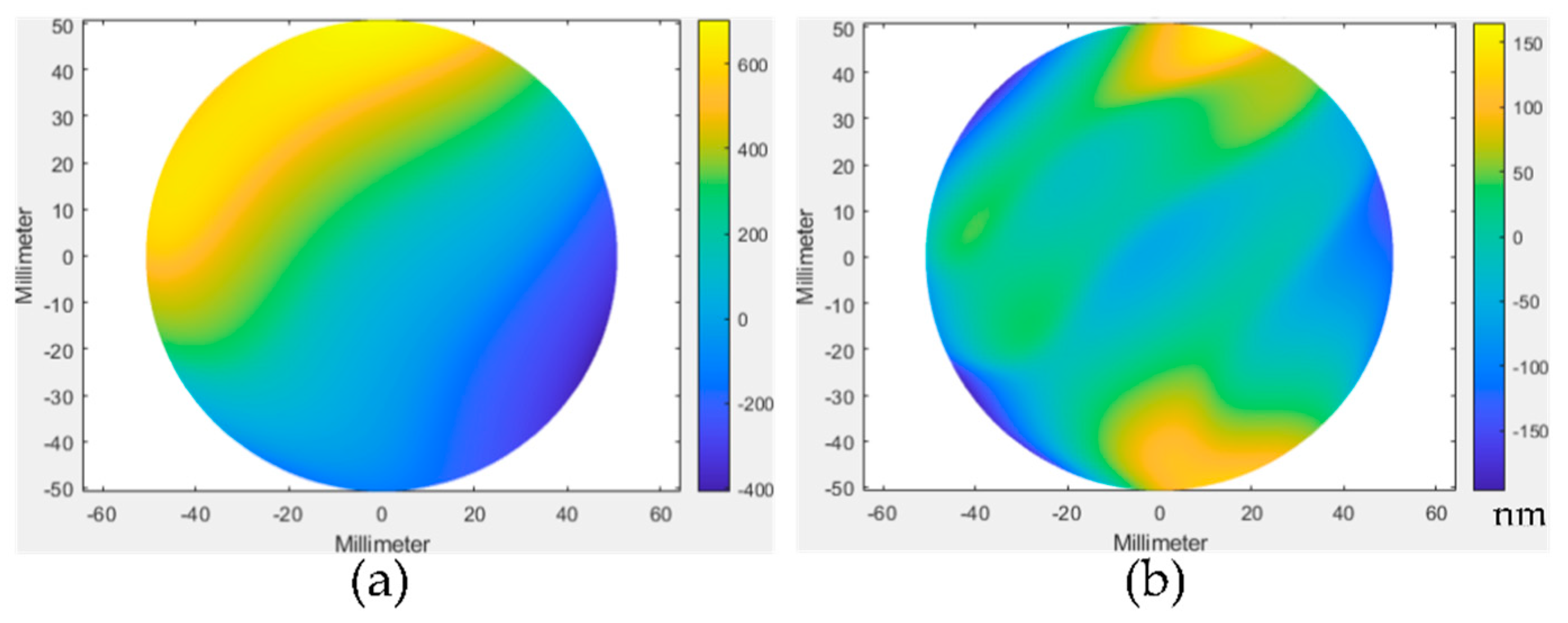
4.1. Surface Measurements of Ideal Spherical Surfaces Using BVTS Systems
4.2. Higher-Order Surface Aberrations
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- ZYGO. ZYGO Transmission Sphere Selection Guide; ZYGO: Middlefield, CT, USA, 2014. [Google Scholar]
- Yang, P.; Xu, J.; Zhu, J.; Hippler, S. Transmission sphere calibration and its current limits. In Proceedings of the SPIE 8082, Optical Measurement Systems for Industrial Inspection VII, Munich, Germany, 23–26 May 2011; p. 80822L. [Google Scholar] [CrossRef]
- Gao, Z.; Chen, L.; Zhou, S.; Zhu, R. Computer-aided alignment for a reference transmission sphere of an interferometer. Opt. Eng. 2004, 43, 69–74. [Google Scholar] [CrossRef]
- Peng, W.-J.; Ho, C.-F.; Lin, W.-L.; Yu, Z.-R.; Huang, C.-Y.; Hsu, W.-Y. Design and tolerance analysis of a transmission sphere by interferometer model. In Proceedings of the SPIE 9582, Optical System Alignment, Tolerancing, and Verification IX, San Diego, CA, USA, 9 August 2015; p. 958208. [Google Scholar] [CrossRef]
- Peng, W.-J.; Ho, C.-F.; Lin, W.-L.; Yu, Z.-R.; Huang, C.-Y.; Kuo, C.-H.; Hsu, W.-Y. Design, tolerance analysis, fabrication, and testing of a 6-in. dual-wavelength transmission sphere for a Fizeau interferometer. Opt. Eng. 2017, 56, 035105. [Google Scholar] [CrossRef]
- Peng, W.-J.; Ho, C.-F.; Yu, Z.-R.; Huang, C.-Y.; Kuo, C.-H.; Hsu, W.-Y. Mounting of reference surface for a transmission sphere. In Proceedings of the SPIE 9684, 8th International Symposium on Advanced Optical Manufacturing and Testing Technologies: Optical Test, Measurement Technology, and Equipment, Suzhou, China, 27 September 2016; p. 96840G. [Google Scholar] [CrossRef]
- Pinto, J. Transmission spheres more than just a reference surface! In Proceedings of the SPIE 10314, Optifab 2003: Technical Digest, Rochester, NY, USA, 19–22 May 2003; p. 1031415. [Google Scholar] [CrossRef]
- Rolland, J.P.; Davies, M.A.; Suleski, T.J.; Evans, C.; Bauer, A.; Lambropoulos, J.C.; Falaggis, K. Freeform optics for imaging. Optica 2021, 8, 161–176. [Google Scholar] [CrossRef]
- Dumas, P. Enabling Higher Precision Freeform Surfaces though Developments in Magnetorheological Finishing (MRF) and Subaperture Stitching Interferometry (SSI). In Proceedings of the Renewable Energy and the Environment, Tucson, AZ, USA, 3 November 2013; p. FT3B.1. [Google Scholar]
- Murphy, P.; Supranowitz, C. Freeform testability considerations for subaperture stitching interferometry. In Proceedings of the SPIE 11175, Optifab, Rochester, NY, USA, 15 November 2019; p. 111750Z. [Google Scholar] [CrossRef]
- Supranowitz, C.; Lormeau, J.-P.; Maloney, C.; Murphy, P.; Dumas, P. Freeform metrology using subaperture stitching interferometry. In Proceedings of the SPIE 10151 Optics and Measurement International Conference, Liberec, Czech Republic, 11 November 2016; p. 101510D. [Google Scholar] [CrossRef]
- Wang, Y.; Zhang, Q.; Chen, Q.; Xu, S.; Han, S. Design of multi-wavelength achromatic transmission sphere based on zoom principle. In Proceedings of the SPIE 11552, Optical Metrology and Inspection for Industrial Applications VII, Online, 10 October 2020; p. 1155217. [Google Scholar] [CrossRef]
- Bielke, A.; Baer, G.; Pruss, C.; Osten, W. Model-based calibration of an interferometric setup with a diffractive zoom-lens. In Proceedings of the SPIE 9618, 2015 International Conference on Optical Instruments and Technology: Optical Systems and Modern Optoelectronic Instruments, Beijing, China, 5 August 2015; p. 961807. [Google Scholar] [CrossRef]
- Ohsaki, Y.; Suzuki, A.; Saitoh, K. Interferometer and Interferance Measurement Method. U.S. Patent 6,842,255, 11 January 2005. [Google Scholar]
- Suzuki, A.; Sekine, Y. Interferometer and Interferance Measurement Method. U.S. Patent 7,106,455, 12 September 2006. [Google Scholar]
- Bielke, A.; Pruss, C.; Osten, W. Design of a variable diffractive zoom lens for interferometric purposes. Opt. Eng. 2017, 56, 14104. [Google Scholar] [CrossRef]
- Bielke, A.; Pruss, C.; Osten, W. Experimental demonstration of a diffractive zoom-lens for an interferometric setup. In Proceedings of the Optical Fabrication and Testing Classical Optics, Kohala Coast, HI, USA, 22–26 June 2014; p. OTu4A. 3. [Google Scholar]
- Reichelt, S.; Zappe, H. Design of spherically corrected, achromatic variable-focus liquid lenses. Opt. Express 2007, 15, 14146–14154. [Google Scholar] [CrossRef] [PubMed]
- Alvarez, L.W. Two-Element Variable-Power Spherical Lens. U.S. Patent 3,305,294, 21 February 1967. [Google Scholar]
- Babington, J. Alvarez lens systems: Theory and applications. In Proceedings of the SPIE 9626, Optical Systems Design 2015: Optical Design and Engineering VI, Jena, Germany, 23 September 2015; p. 962615. [Google Scholar] [CrossRef]
- Barbero, S. The Alvarez and Lohmann refractive lenses revisited. Opt. Express 2009, 17, 9376–9390. [Google Scholar] [CrossRef] [PubMed]
- Barbero, S.; Rubinstein, J. Adjustable-focus lenses based on the Alvarez principle. J. Opt. 2011, 13, 125705. [Google Scholar] [CrossRef][Green Version]
- Grewe, A.; Hillenbrand, M.; Sinzinger, S. Aberration analysis of optimized Alvarez–Lohmann lenses. Appl. Opt. 2014, 53, 7498–7506. [Google Scholar] [CrossRef] [PubMed]
- Roth, E.; Scheibe, H.; Koehler, T.; Schindler, C. Building Challenging Optical Systems with Alvarez Lenses. In Proceedings of the Optical Design and Fabrication 2019 (Freeform, OFT), Washington, DC, USA, 10–12 June 2019; p. FW4B.6. [Google Scholar]
- Smilie, P.J.; Suleski, T.J.; Dutterer, B.; Lineberger, J.L.; Davies, M.A. Design and characterization of an infrared Alvarez lens. Opt. Eng. 2012, 51, 13006. [Google Scholar] [CrossRef]
- Lohmann, A.W. A new class of varifocal lenses. Appl. Opt. 1970, 9, 1669–1671. [Google Scholar] [CrossRef]
- Burge, J.H. Fizeau interferometry for large convex surfaces. In Proceedings of the SPIE 2536, Optical Manufacturing and Testing, San Diego, CA, USA, 8 September 1995; pp. 127–138. [Google Scholar] [CrossRef]
- Fizeau Interferometer for Optical Testing. Available online: https://www.lighttrans.com/use-cases/application-use-cases/fizeau-interferometer-for-optical-testing.html (accessed on 24 July 2021).
- Herráez, M.A.; Burton, D.R.; Lalor, M.J.; Gdeisat, M.A. Fast two-dimensional phase-unwrapping algorithm based on sorting by reliability following a noncontinuous path. Appl. Opt. 2002, 41, 7437–7444. [Google Scholar] [CrossRef]
- Zhao, Z.; Zhang, H.; Xiao, Z.; Du, H.; Zhuang, Y.; Fan, C.; Zhao, H. Robust 2D phase unwrapping algorithm based on the transport of intensity equation. Meas. Sci. Technol. 2018, 30, 015201. [Google Scholar] [CrossRef]
- Gray, R. ZernikeCalc, MATLAB Central File Exchange; MathWorks: Natick, MA, USA, 2021. [Google Scholar]
- Lakshminarayanan, V.; Fleck, A. Zernike polynomials: A guide. J. Mod. Opt. 2011, 58, 1678. [Google Scholar] [CrossRef]



| z (mm) | d (mm) | ||
|---|---|---|---|
| f-Number | TS | BVTS | BVTS 1st Element |
| 80 | 127.9 | 25.0 | 7.504 |
| 45 | 71.9 | 25.2 | 13.283 |
| 32 | 55.9 | 25.1 | 17.035 |
| 25 | 39.9 | 25.0 | 23.745 |
| 15 | 23.9 | 25.0 | 39.181 |
| A mm−2 | B mm−2 | C mm−1 | D mm−1 | E |
|---|---|---|---|---|
| 3.06901 × 10−6 | −5.56451 × 10−13 | −7.67717 × 10−8 | 5.19685 × 10−5 | −0.02 |
| Source Wavelength (μm) | Source Wavelength (μm) | ||||||||
|---|---|---|---|---|---|---|---|---|---|
| 0.6328 | 1.55 | 3.39 | 10.6 | 0.6328 | 1.55 | 3.39 | 10.6 | ||
| f-Number | PV (Waves) | f-Number | RMS (Waves) | ||||||
| f/15 | 0.504 | 0.192 | 0.087 | 0.027 | f/15 | 0.085 | 0.033 | 0.015 | 0.006 |
| f/25 | 0.340 | 0.129 | 0.058 | 0.017 | f/25 | 0.051 | 0.019 | 0.009 | 0.003 |
| f/35 | 0.273 | 0.102 | 0.046 | 0.014 | f/35 | 0.041 | 0.015 | 0.007 | 0.002 |
| f/45 | 0.244 | 0.088 | 0.040 | 0.012 | f/45 | 0.040 | 0.014 | 0.006 | 0.002 |
| f/80 | 0.350 | 0.123 | 0.054 | 0.015 | f/80 | 0.048 | 0.017 | 0.007 | 0.002 |
| A mm−2 | B mm−2 | C mm−1 | D mm−1 | E | F mm−3 | G mm−3 | H mm−3 | I mm−1 |
|---|---|---|---|---|---|---|---|---|
| 3.12039 × 10−6 | −6.01610 × 10−18 | 3.74566 × 10−12 | −5.99074 × 10−6 | −9.3728 × 10−3 | 5.8794 × 10−12 | 3.52603 × 10−11 | 1.36198 × 10−9 | −2.29134 × 10−7 |
| Source Wavelength (μm) | Source Wavelength (μm) | ||||||||
|---|---|---|---|---|---|---|---|---|---|
| 0.6328 | 1.55 | 3.39 | 10.6 | 0.6328 | 1.55 | 3.39 | 10.6 | ||
| f-Number | PV (Waves) | f-Number | RMS (Waves) | ||||||
| f/15 | 0.447 | 0.172 | 0.078 | 0.024 | f/15 | 0.109 | 0.043 | 0.019 | 0.006 |
| f/25 | 0.270 | 0.101 | 0.045 | 0.014 | f/25 | 0.058 | 0.022 | 0.010 | 0.003 |
| f/35 | 0.237 | 0.087 | 0.039 | 0.011 | f/35 | 0.051 | 0.019 | 0.008 | 0.002 |
| f/45 | 0.227 | 0.083 | 0.037 | 0.011 | f/45 | 0.049 | 0.018 | 0.008 | 0.002 |
| f/80 | 0.222 | 0.080 | 0.036 | 0.010 | f/80 | 0.049 | 0.018 | 0.008 | 0.002 |
| BVTS Example 1 | BVTS Example 2 | ||||||||
|---|---|---|---|---|---|---|---|---|---|
| Source Wavelength (μm) | Source Wavelength (μm) | ||||||||
| 0.6328 | 1.55 | 3.39 | 10.6 | 0.6328 | 1.55 | 3.39 | 10.6 | ||
| r-Number | PV (Waves) | r-Number | PV (Waves) | ||||||
| 80 | 0.695 | 0.517 | 0.137 | 0.027 | 80 | 0.831 | 0.512 | 0.060 | 0.008 |
| 45 | 0.713 | 0.515 | 0.060 | 0.042 | 45 | 0.776 | 0.579 | 0.279 | 0.032 |
| 35 | 0.681 | 0.513 | 0.067 | 0.030 | 35 | 0.735 | 0.599 | 0.221 | 0.041 |
| 25 | 0.739 | 0.569 | 0.139 | 0.021 | 25 | 0.958 | 0.560 | 0.189 | 0.033 |
| 15 | 0.572 | 0.439 | 0.256 | 0.026 | 15 | 1.174 | 0.417 | 0.214 | 0.044 |
| −81.5 | 1.005 | 0.720 | 0.073 | 0.025 | −81.5 | 0.567 | 0.509 | 0.077 | 0.022 |
| −46 | 1.194 | 0.570 | 0.329 | 0.035 | −46 | 0.738 | 0.516 | 0.206 | 0.047 |
| −36 | 1.270 | 0.590 | 0.158 | 0.054 | −36 | 1.012 | 0.542 | 0.160 | 0.074 |
| −26 | 1.649 | 0.897 | 0.380 | 0.068 | −26 | 0.887 | 0.616 | 0.157 | 0.019 |
| −16 | 2.089 | 1.075 | 0.469 | 0.223 | −16 | 1.232 | 0.665 | 0.271 | 0.071 |
| Source Wavelength (μm) | r/# | Z5 | Z6 | Z7 | Z8 | Z9 |
|---|---|---|---|---|---|---|
| 0.6328 | 80 | 1.64 | 44.69 | 35.63 | 12.47 | 37.48 |
| 45 | 56.55 | 59.16 | 44.44 | 38.47 | 37.02 | |
| 35 | 31.75 | 31.31 | 92.48 | 50.94 | 14.70 | |
| 25 | 81.56 | 3.64 | 71.79 | 16.11 | 27.83 | |
| 15 | 131.84 | 8.24 | 38.15 | 7.90 | 57.62 | |
| 1.55 | 80 | 0.60 | 17.08 | 7.30 | 9.38 | 15.00 |
| 45 | 14.81 | 29.26 | 7.75 | 12.82 | 14.23 | |
| 35 | 30.20 | 43.46 | 12.93 | 15.31 | 12.82 | |
| 25 | 13.29 | 47.70 | 52.46 | 19.51 | 31.78 | |
| 15 | 55.96 | 62.56 | 16.20 | 37.55 | 12.53 | |
| 3.39 | 80 | 1.66 | 9.83 | 2.58 | 0.47 | 2.09 |
| 45 | 2.57 | 1.08 | 4.12 | 0.16 | 4.48 | |
| 35 | 6.11 | 2.49 | 4.32 | 0.09 | 2.66 | |
| 25 | 32.75 | 42.36 | 5.77 | 2.84 | 11.75 | |
| 15 | 32.04 | 17.02 | 1.42 | 14.88 | 5.61 | |
| 10.6 | 80 | 0.06 | 0.05 | 0.43 | 0.07 | 0.52 |
| 45 | 0.90 | 5.46 | 5.73 | 1.22 | 13.95 | |
| 35 | 1.55 | 0.18 | 1.61 | 0.08 | 1.56 | |
| 25 | 2.51 | 0.16 | 1.48 | 0.08 | 0.67 | |
| 15 | 4.26 | 0.44 | 1.01 | 0.01 | 1.97 |
| Source Wavelength (μm) | r/# | Z5 | Z6 | Z7 | Z8 | Z9 |
|---|---|---|---|---|---|---|
| 0.6328 | 80 | 38.03 | 0.01 | 49.38 | 0.00 | 17.19 |
| 45 | 7.38 | 0.01 | 39.75 | 0.01 | 71.64 | |
| 35 | 1.39 | 0.01 | 49.38 | 0.00 | 60.96 | |
| 25 | 149.14 | 0.01 | 34.29 | 0.00 | 68.05 | |
| 15 | 179.65 | 0.02 | 41.32 | 0.00 | 112.80 | |
| 1.55 | 80 | 59.97 | 0.01 | 4.56 | 0.00 | 38.23 |
| 45 | 27.87 | 0.00 | 8.43 | 0.00 | 15.11 | |
| 35 | 22.48 | 0.00 | 19.67 | 0.00 | 56.48 | |
| 25 | 2.96 | 0.01 | 23.93 | 0.00 | 11.80 | |
| 15 | 6.12 | 0.01 | 47.38 | 0.00 | 41.79 | |
| 3.39 | 80 | 0.19 | 0.00 | 1.71 | 0.00 | 5.43 |
| 45 | 66.11 | 0.00 | 19.02 | 0.00 | 2.46 | |
| 35 | 63.25 | 0.00 | 38.66 | 0.00 | 8.31 | |
| 25 | 64.70 | 0.00 | 10.18 | 0.00 | 38.85 | |
| 15 | 26.70 | 0.00 | 7.52 | 0.00 | 0.77 | |
| 10.6 | 80 | 0.09 | 0.00 | 0.44 | 0.00 | 1.57 |
| 45 | 0.41 | 0.00 | 0.56 | 0.00 | 1.29 | |
| 35 | 1.16 | 0.00 | 0.93 | 0.00 | 1.36 | |
| 25 | 0.83 | 0.00 | 1.60 | 0.00 | 1.91 | |
| 15 | 4.95 | 0.00 | 12.54 | 0.00 | 4.65 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Koerber, J.; Boreman, G.D.; Suleski, T.J. Broadband Variable Transmission Sphere for Fizeau Interferometry. Optics 2022, 3, 88-98. https://doi.org/10.3390/opt3010011
Koerber J, Boreman GD, Suleski TJ. Broadband Variable Transmission Sphere for Fizeau Interferometry. Optics. 2022; 3(1):88-98. https://doi.org/10.3390/opt3010011
Chicago/Turabian StyleKoerber, Jonathan, Glenn D. Boreman, and Thomas J. Suleski. 2022. "Broadband Variable Transmission Sphere for Fizeau Interferometry" Optics 3, no. 1: 88-98. https://doi.org/10.3390/opt3010011
APA StyleKoerber, J., Boreman, G. D., & Suleski, T. J. (2022). Broadband Variable Transmission Sphere for Fizeau Interferometry. Optics, 3(1), 88-98. https://doi.org/10.3390/opt3010011





