Analysis of the Aeroelastic Dynamics of Lightweight Flexible Variations of the SNL-NRT Turbine
Abstract
1. Introduction
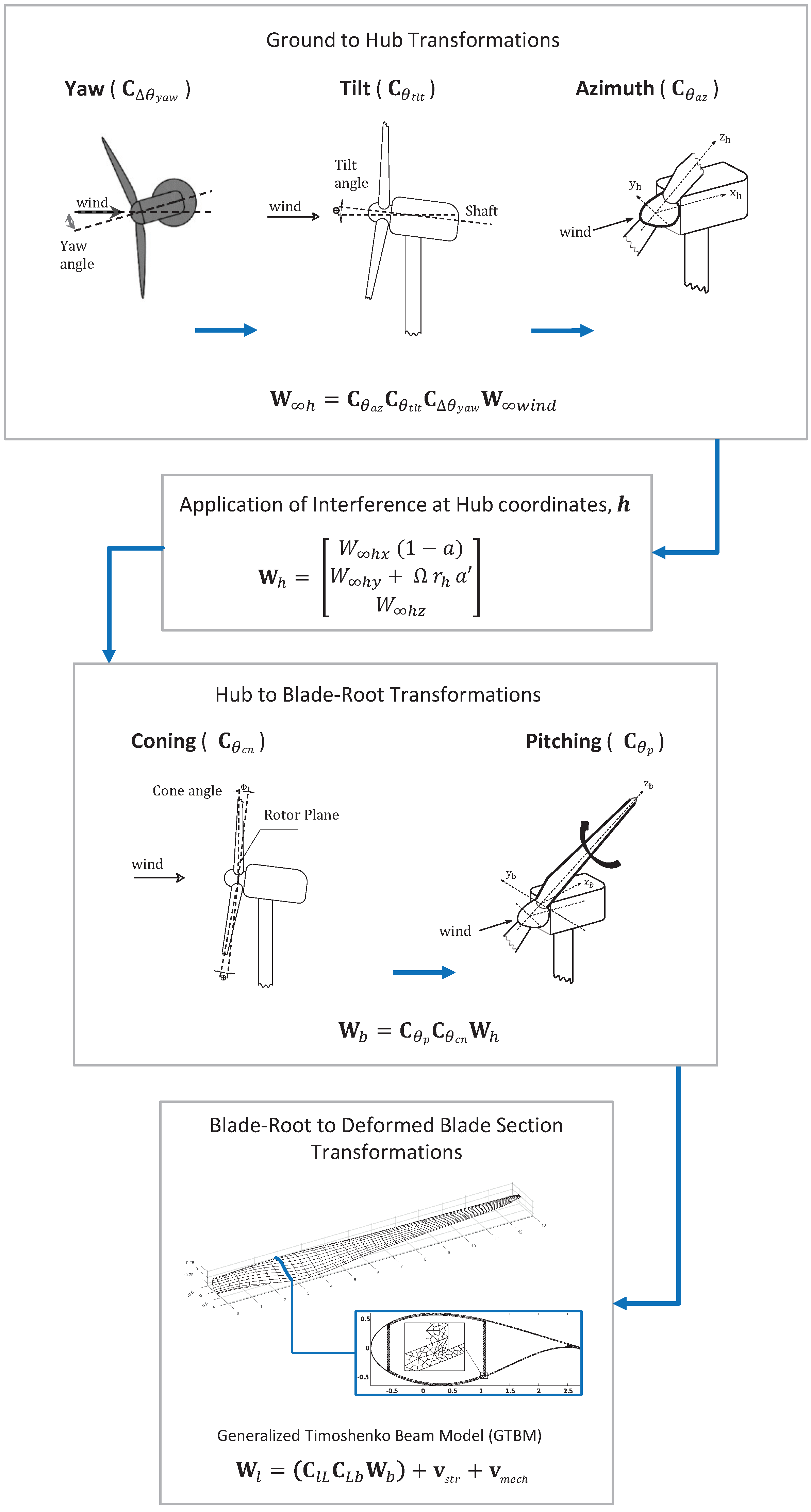
2. The Common ODE Framework
2.1. Aeroelastic Modules of CODEF
2.2. The Gaussian-Core Vortex Lattice Model
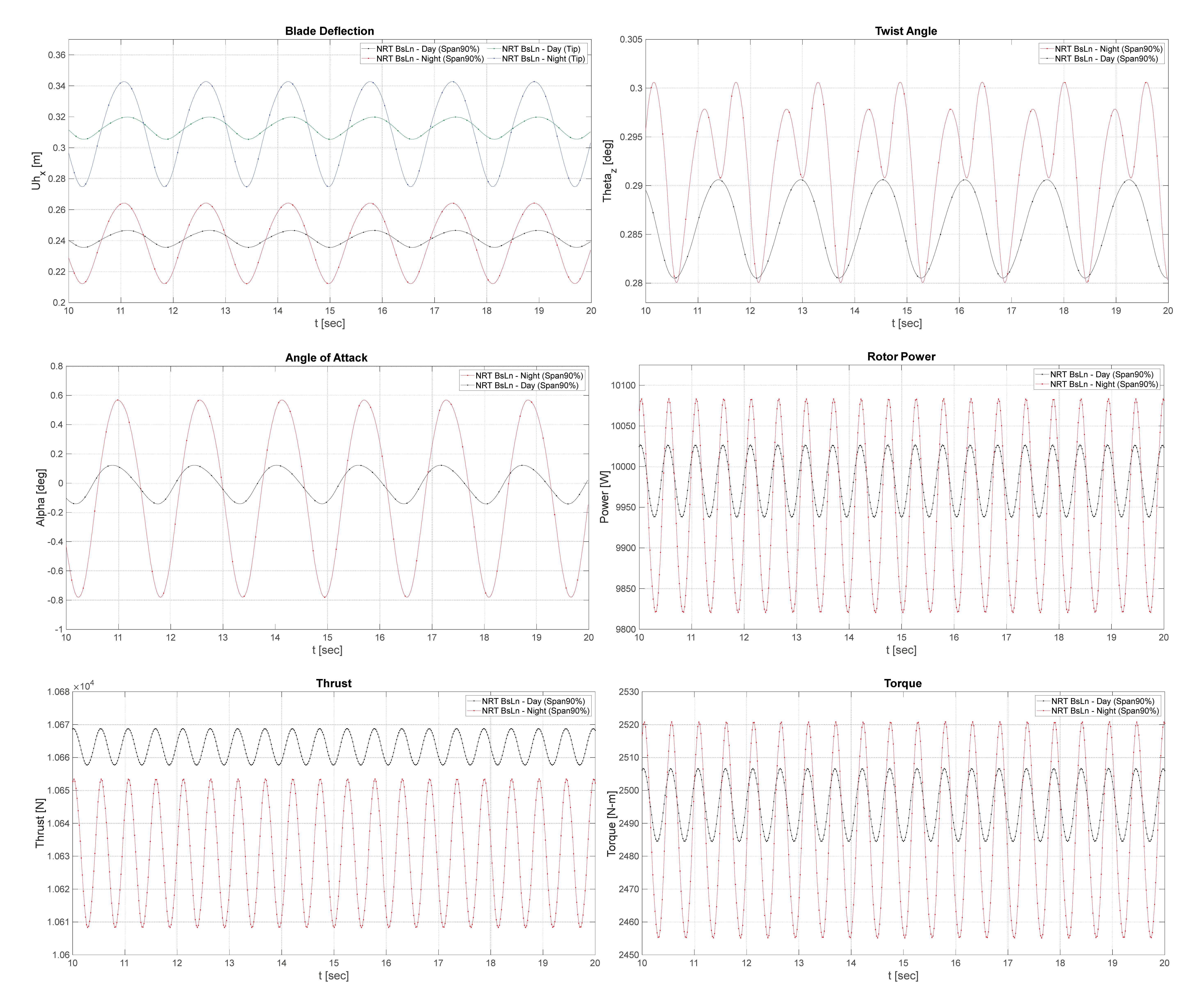
3. Numerical Experimentation and Analysis of Results
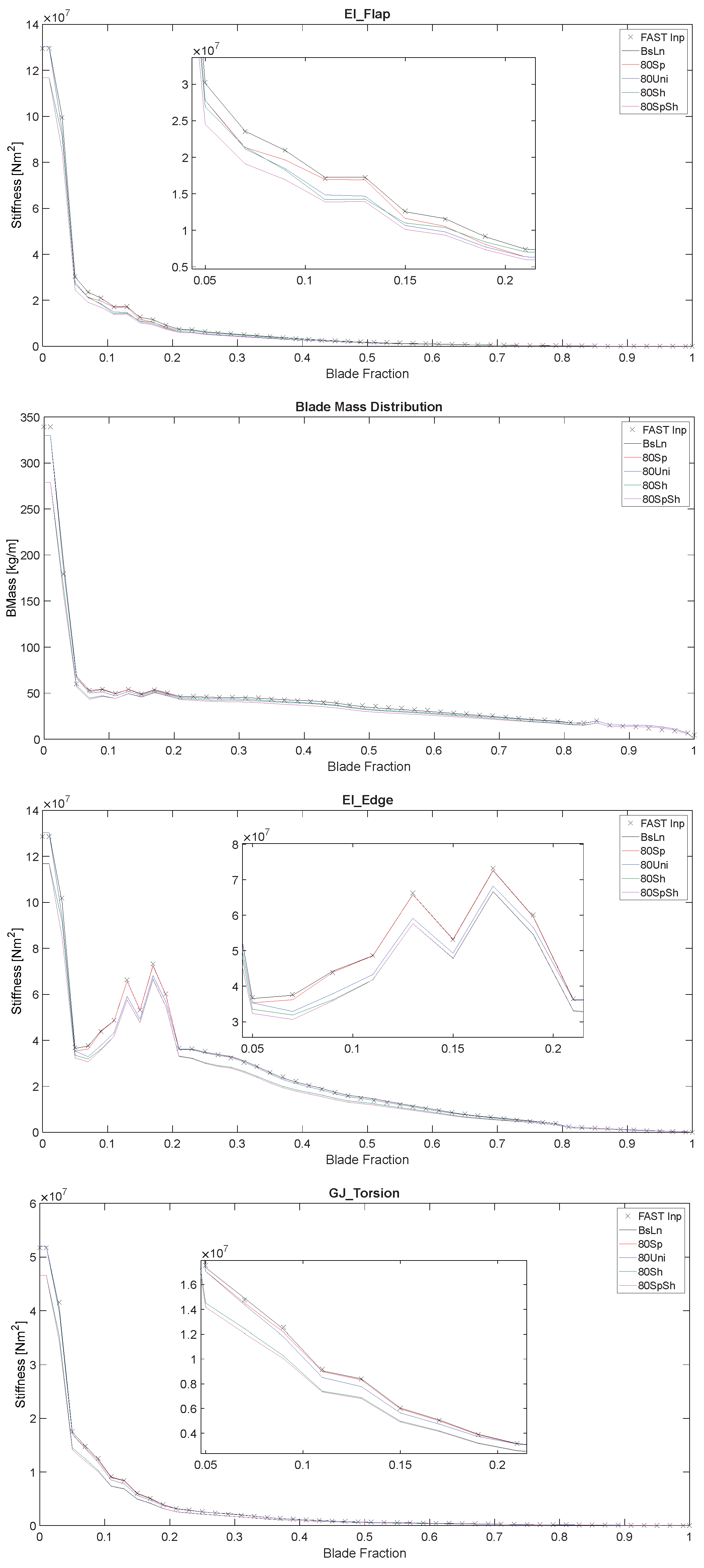
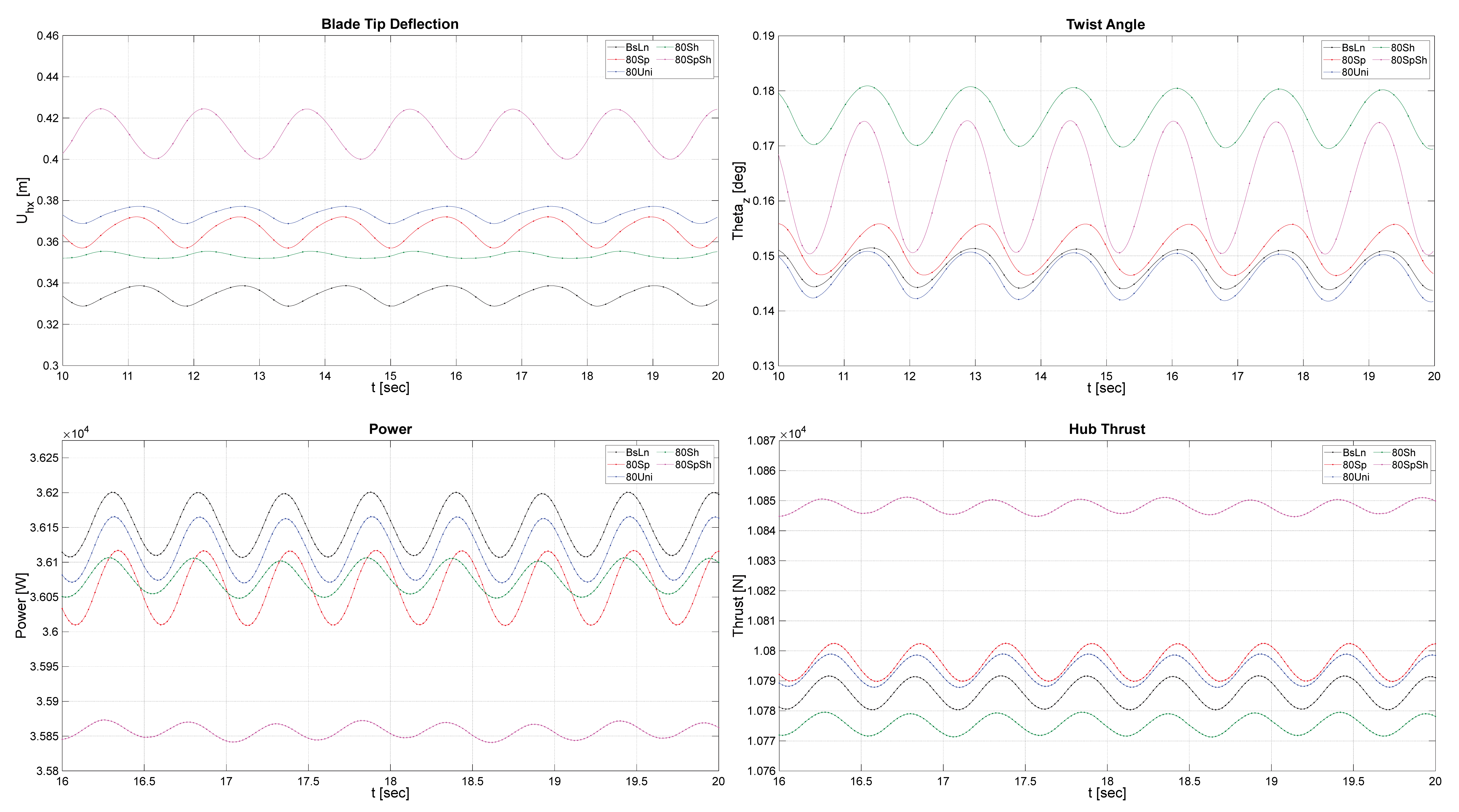
3.1. Aeroelastic Simulations of the NRT Blade Flexible Variations with Refined Geometry and Internal Structure
- (A)
- “BsLn” NRT baseline blade;
- (B)
- “80Sp” 80% adjustment of UNI material in spar cap;
- (C)
- “80Uni” 80% adjustment of all UNI material (spar cap and shell);
- (D)
- “80Sh” 80% adjustment of all shell material;
- (E)
- “80SpSh” 80% adjustment of all material (spar cap and shell).
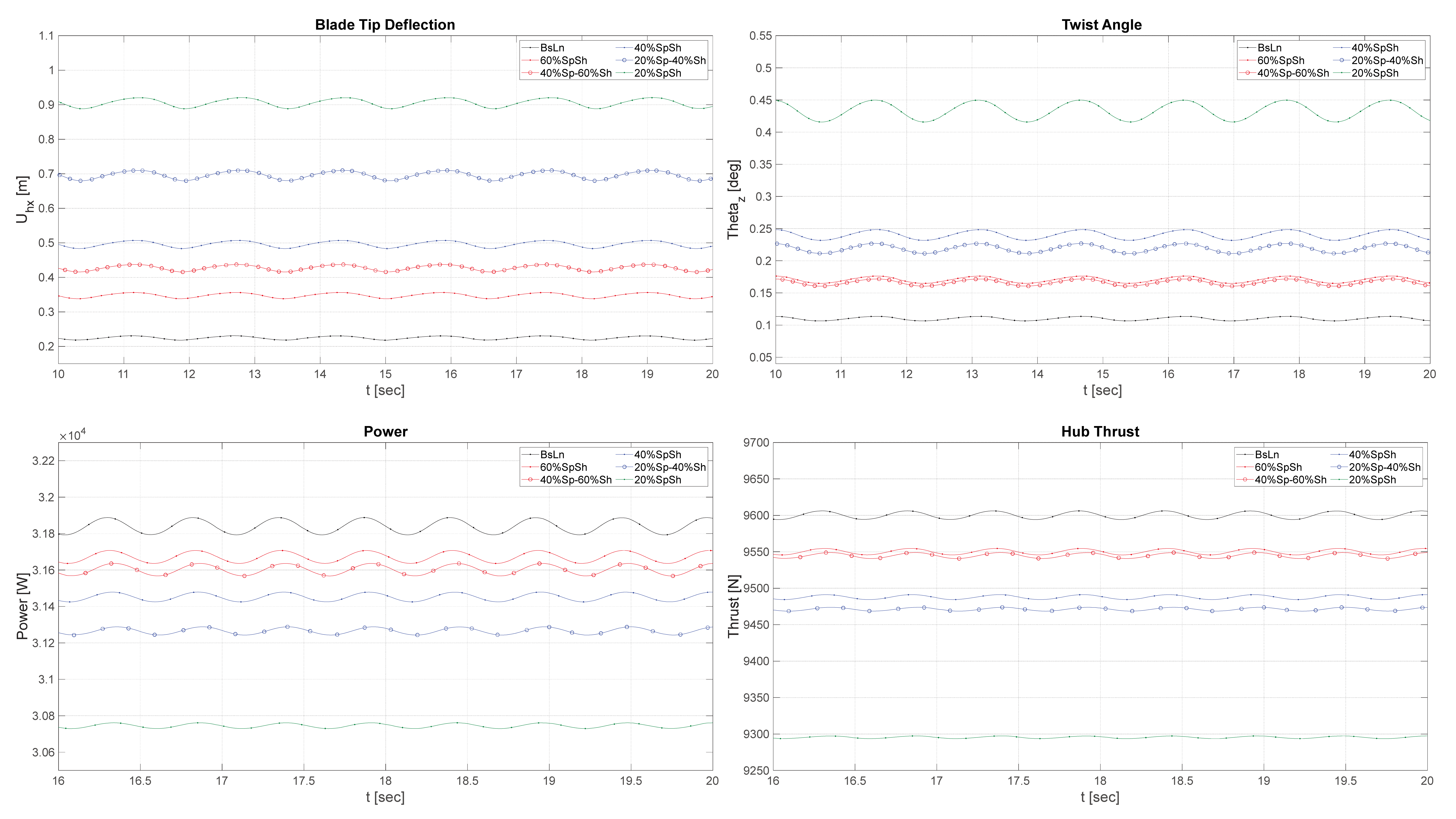
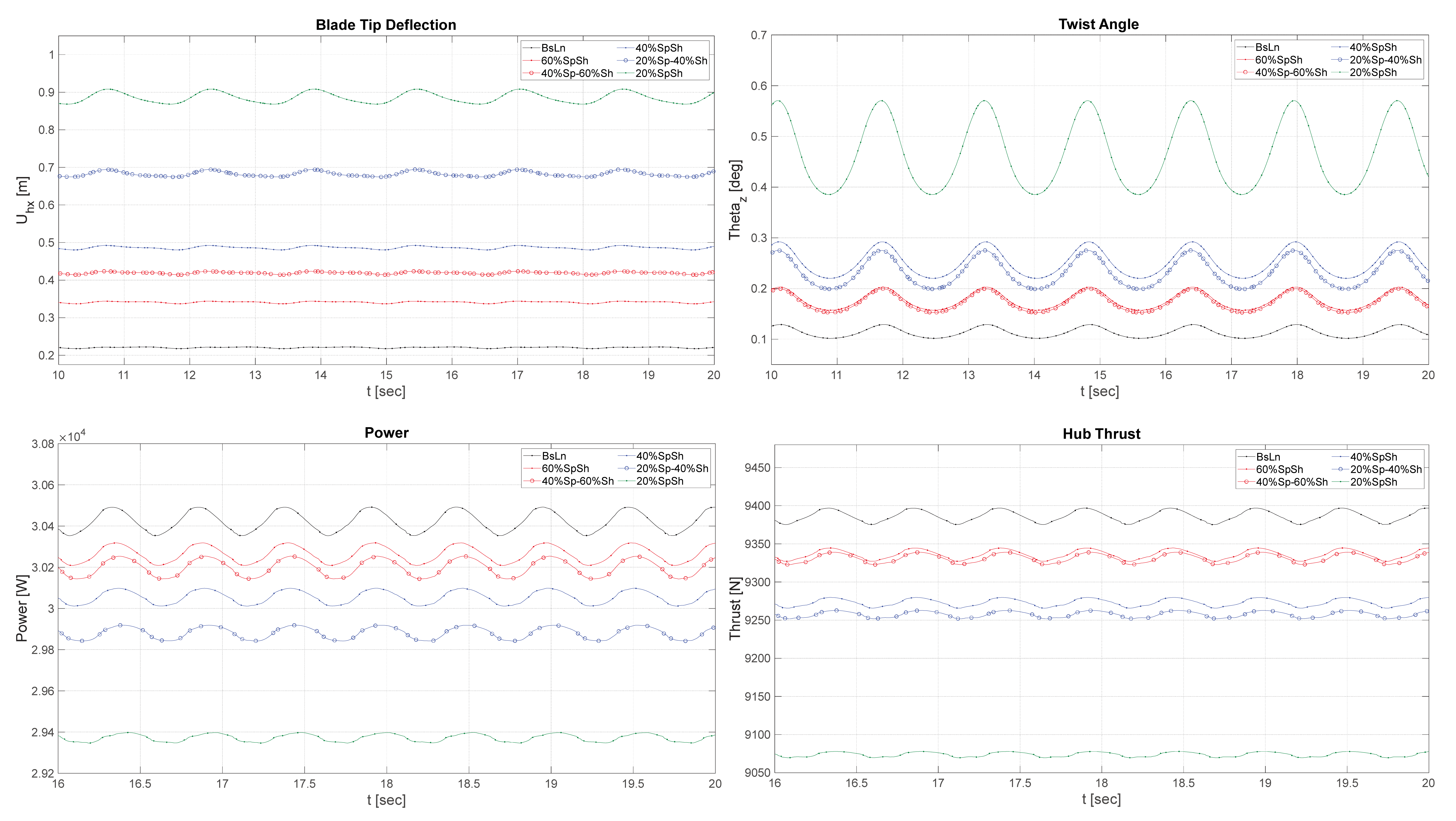
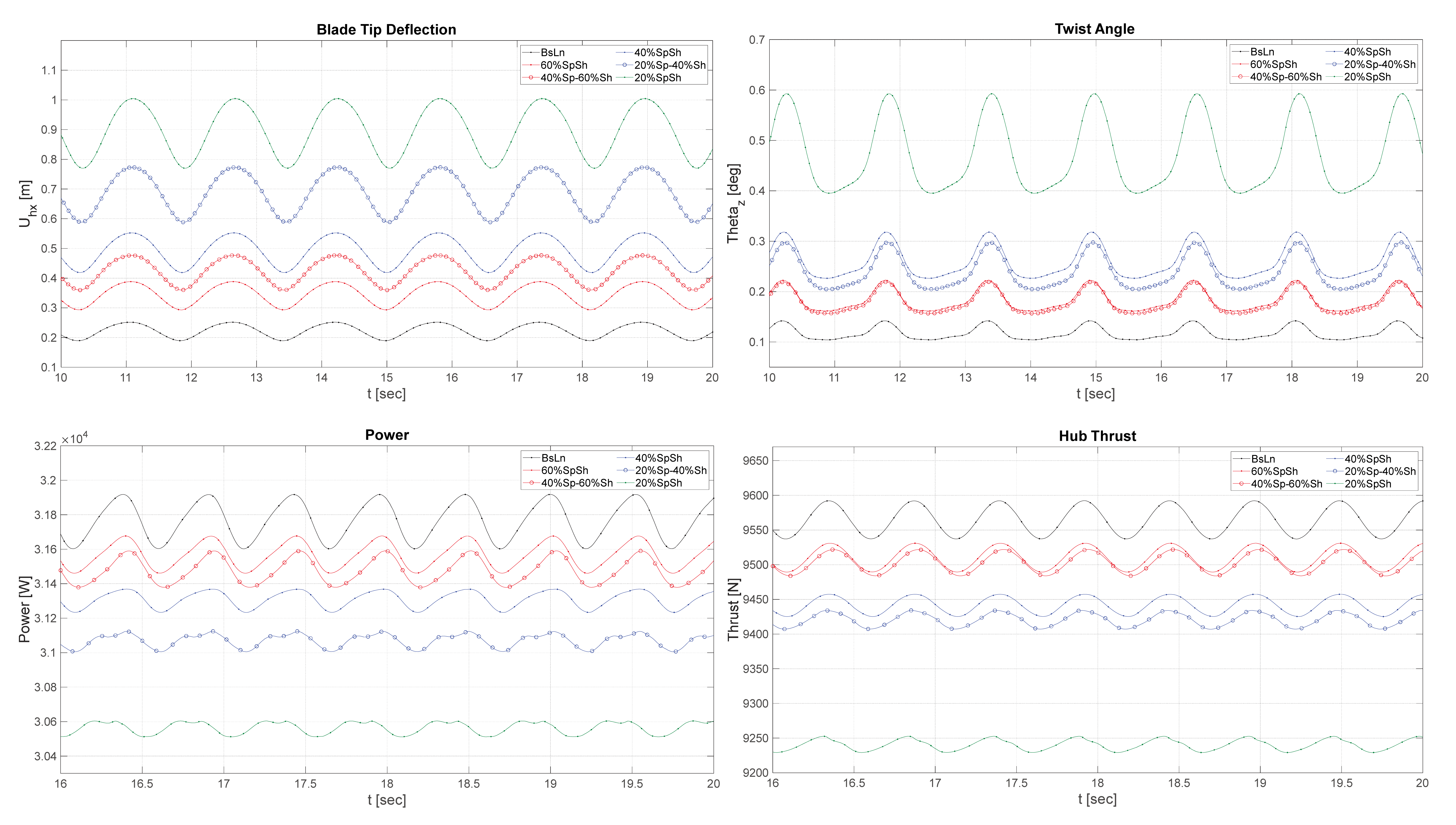
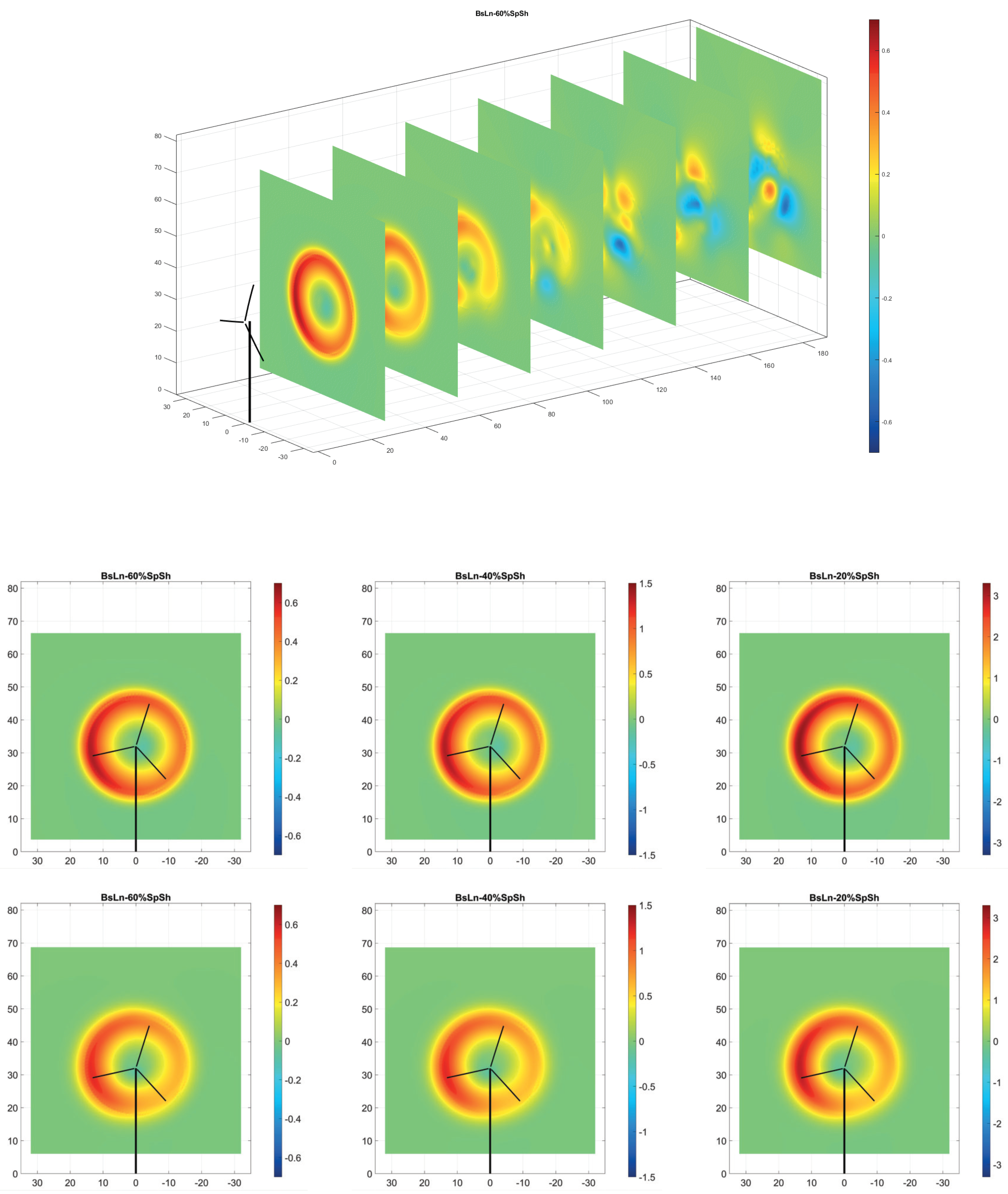
3.2. Aeroelastic Simulations of Additional Flexible Variations of the NRT Blade
- (A)
- “BsLn” NRT baseline blade;
- (B)
- “60%SpSh” 60% shell and spar cap materials;
- (C)
- “40%Sp–60%Sh” 40% spar cap and 60% shell materials;
- (D)
- “40%SpSh” 40% shell and spar cap materials;
- (E)
- “20%Sp–40%Sh” 20% spar cap and 40% shell materials;
- (F)
- “20%SpSh” 20% shell and spar cap materials.
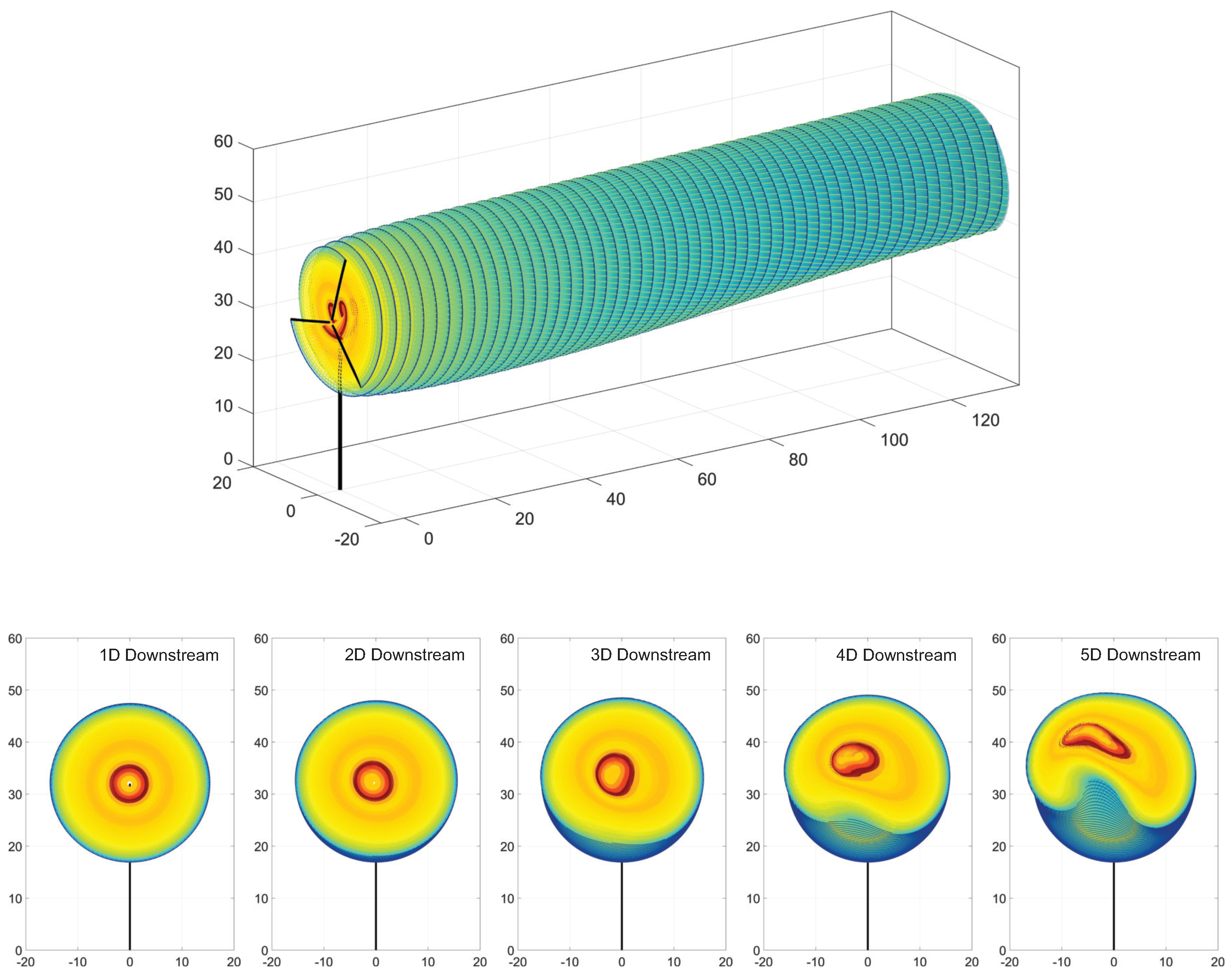
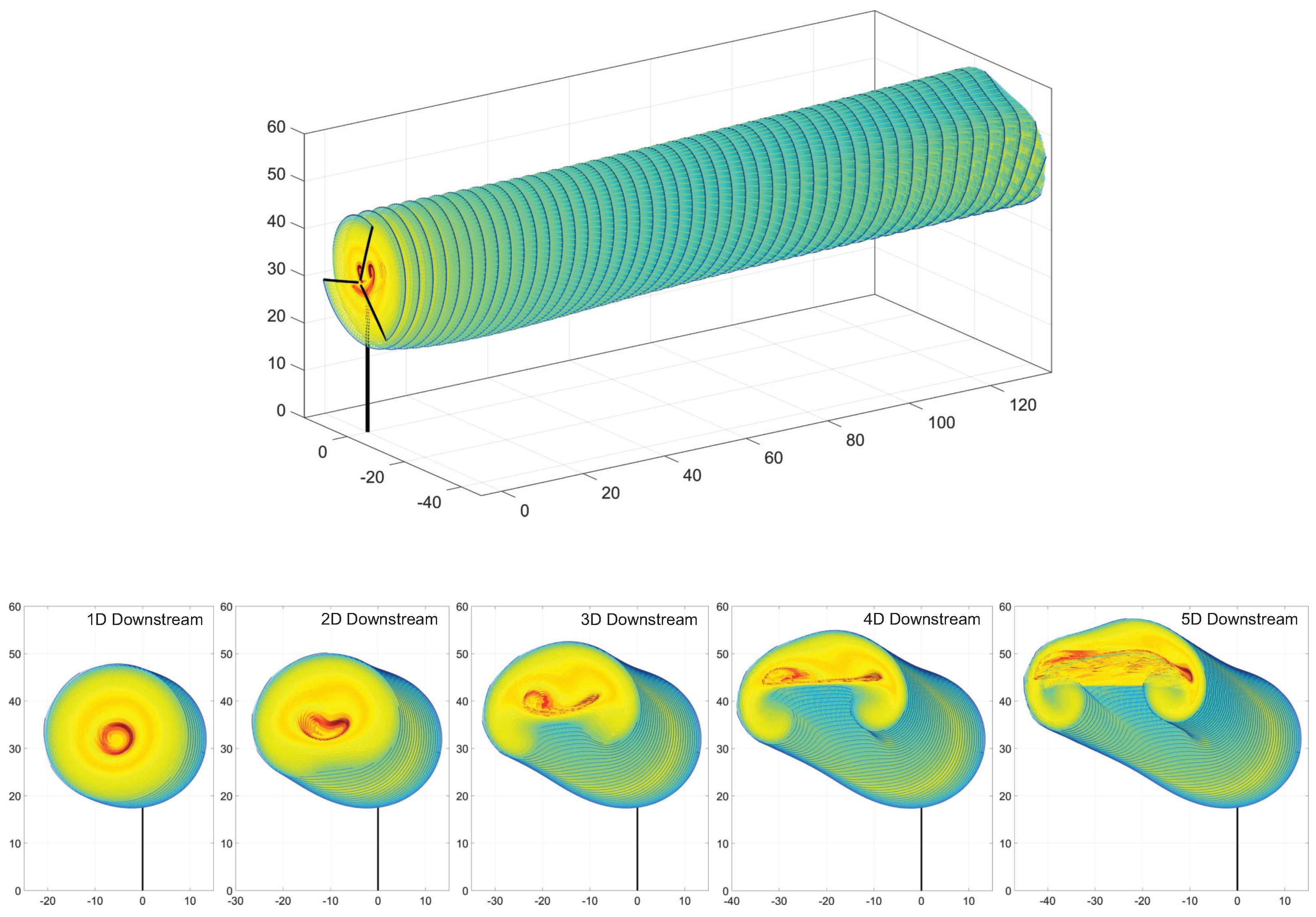
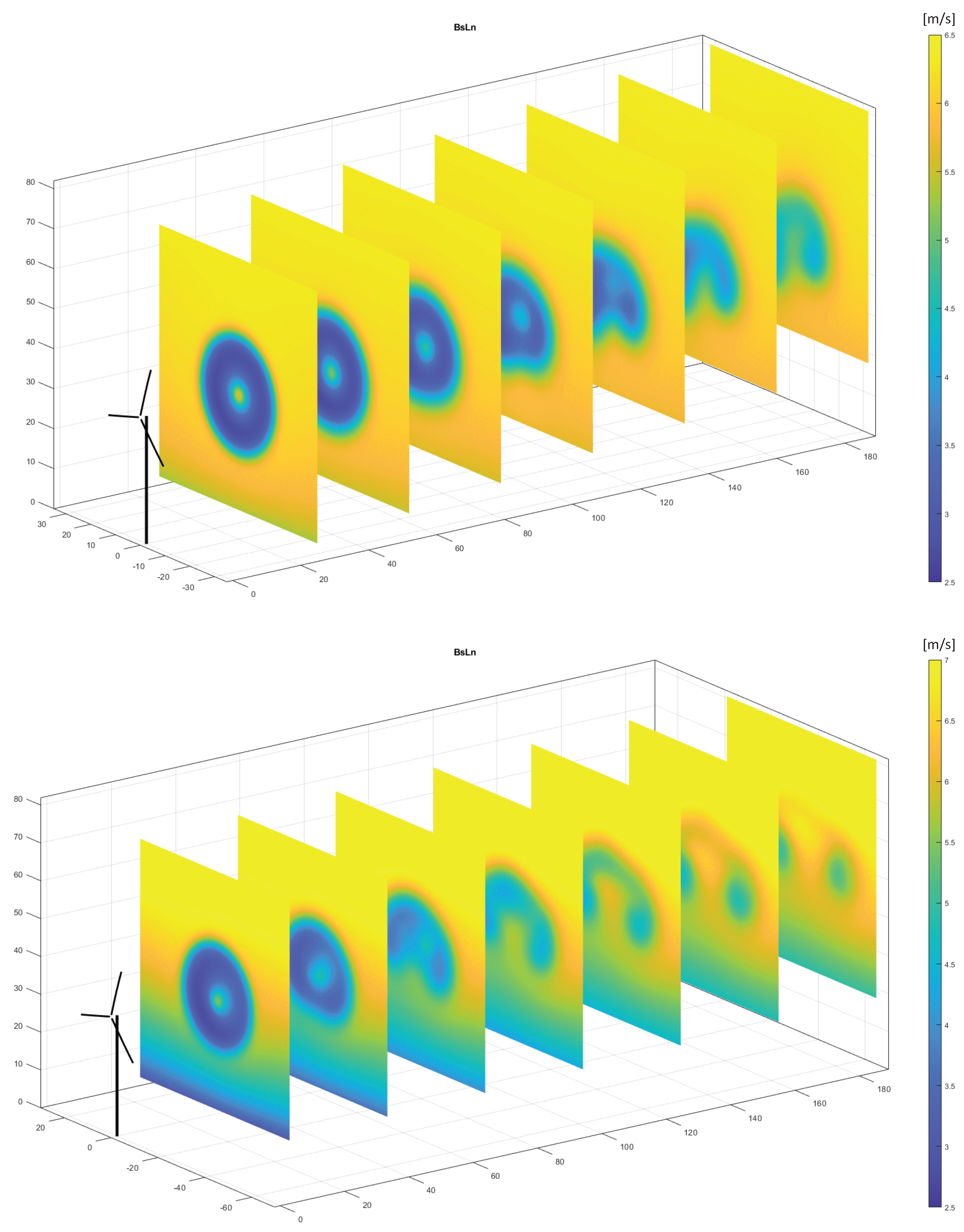
3.3. Wake Structure and Velocity Patterns Simulated for Flexible Variations of the NRT Blade
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- IRENA. Global Energy Transformation: A Roadmap to 2050; Technical Report; International Renewable Energy Agency: Abu Dhabi, United Arab Emirates, 2019. [Google Scholar]
- Dykes, K.L.; Veers, P.S.; Lantz, E.J.; Holttinen, H.; Carlson, O.; Tuohy, A.; Sempreviva, A.M.; Clifton, A.; Rodrigo, J.S.; Berry, D.S.; et al. IEA Wind TCP: Results of IEA Wind TCP Workshop on a Grand Vision for Wind Energy Technology; Technical Report NREL/TP-5000-72437; National Renewable Energy Laboratory: Golden, CO, USA, 2019.
- TPI Composites Inc. Parametric Study for Large Wind Turbine Blades; Report SAND2002-2519; Sandia National Laboratories: Albuquerque, NM, USA, 2002.
- Griffin, D.A. Blade System Design Studies Volume I: Composite Technologies for Large Wind Turbine Blades; Report SAND2002-1879; Sandia National Laboratories: Albuquerque, NM, USA, 2002.
- Veers, P.; Dykes, K.; Basu, S.; Bianchini, A.; Clifton, A.; Green, P.; Holttinen, H.; Kitzing, L.; Kosovic, B.; Lundquist, J.K.; et al. Grand Challenges: Wind energy research needs for a global energy transition. Wind. Energy Sci. 2022, 7, 2491–2496. [Google Scholar] [CrossRef]
- Stiesdal, H. Rotor loadings on the Bonus 450 kW turbine. J. Wind Eng. Ind. Aerodyn. 1992, 39, 303–315. [Google Scholar] [CrossRef]
- Kong, C.; Bang, J.; Sugiyama, Y. Structural investigation of composite wind turbine blade considering various load cases and fatigue life. Energy 2005, 30, 2101–2114. [Google Scholar] [CrossRef]
- Loth, E.; Fingersh, L.; Griffith, D.; Kaminski, M.; Qin, C. Gravo-aeroelastically scaling for extreme-scale wind turbines. In Proceedings of the 35th AIAA Applied Aerodynamics Conference, Denver, CO, USA, 5–9 June 2017. [Google Scholar]
- Otero, A.D.; Ponta, F.L.; Lago, L.I. Structural Analysis of Complex Wind Turbine Blades: Flexo-Torsional Vibrational Modes. In Advances in Wind Power; Carriveau, R., Ed.; InTech: London, UK, 2012; pp. 123–149. [Google Scholar]
- Tabor, A. Testing on the Ground Before You Fly: Wind Tunnels at NASA Ames. 2020. Available online: https://www.nasa.gov/centers-and-facilities/ames/testing-on-the-ground-before-you-fly-wind-tunnels-at-nasa-ames/ (accessed on 10 January 2024).
- Gebraad, P.M.; Teeuwisse, F.W.; Van Wingerden, J.; Fleming, P.A.; Ruben, S.D.; Marden, J.R.; Pao, L.Y. Wind plant power optimization through yaw control using a parametric model for wake effects—A CFD simulation study. Wind Energy 2016, 19, 95–114. [Google Scholar] [CrossRef]
- Van Bussel, G.J. The Aerodynamics of Horizontal Axis Wind Turbine Rotors Explored with Asymptotic Expansion Methods. Ph.D. Thesis, Delft University of Technology, Delft, The Netherlands, 1995. [Google Scholar]
- Hansen, M.; Sorensen, J.; Michelsen, J.; Sorensen, N.; Hansen, M.; Sorensen, J.; Michelsen, J.; Sorensen, N. A global Navier-Stokes rotor prediction model. In Proceedings of the 35th Aerospace Sciences Meeting and Exhibit, Reno, NV, USA, 6–9 January 1997; p. 970. [Google Scholar]
- Maronga, B.; Gryschka, M.; Heinze, R.; Hoffmann, F.; Kanani-Sühring, F.; Keck, M.; Ketelsen, K.; Letzel, M.O.; Sühring, M.; Raasch, S. The Parallelized Large-Eddy Simulation Model (PALM) version 4.0 for atmospheric and oceanic flows: Model formulation, recent developments, and future perspectives. Geosci. Model Dev. 2015, 8, 2515–2551. [Google Scholar] [CrossRef]
- Churchfield, M.; Lee, S.; Moriarty, P.; Martinez, L.; Leonardi, S.; Vijayakumar, G.; Brasseur, J. A large-eddy simulation of wind-plant aerodynamics. In Proceedings of the 50th AIAA Aerospace Sciences Meeting including the New Horizons Forum and Aerospace Exposition, Nashville, TN, USA, 9–12 January 2012; p. 537. [Google Scholar]
- Domino, S. Sierra Low Mach Module: Nalu Theory Manual 1.0; Sandia National Laboratories: Albuquerque, NM, USA, 2015.
- Ekaterinaris, J.A. Numerical simulation of incompressible two-blade rotor flowfields. J. Propuls. Power 1998, 14, 367–374. [Google Scholar] [CrossRef]
- Duque, E.; Van Dam, C.; Hughes, S. Navier-Stokes simulations of the NREL combined experiment phase II rotor. In Proceedings of the 37th Aerospace Sciences Meeting and Exhibit, Reno, NV, USA, 11–14 January 1999; p. 37. [Google Scholar]
- Sorensen, N. Aerodynamic predictions for the unsteady aerodynamics experiment phase-II rotor at the National Renewable Energy Laboratory. In Proceedings of the 2000 ASME Wind Energy Symposium, Reno, NV, USA, 10–13 January 2000; p. 37. [Google Scholar]
- Sprague, M.A.; Geers, T.L. Legendre spectral finite elements for structural dynamics analysis. Commun. Numer. Methods Eng. 2007, 24, 1953–1965. [Google Scholar] [CrossRef]
- Doubrawa, P.; Quon, E.W.; Martinez-Tossas, L.A.; Shaler, K.; Debnath, M.; Hamilton, N.; Herges, T.G.; Maniaci, D.; Kelley, C.L.; Hsieh, A.S.; et al. Multimodel validation of single wakes in neutral and stratified atmospheric conditions. Wind Energy 2020, 23, 2027–2055. [Google Scholar] [CrossRef]
- Lignarolo, L.E.; Mehta, D.; Stevens, R.J.; Yilmaz, A.E.; van Kuik, G.; Andersen, S.J.; Meneveau, C.; Ferreira, C.J.; Ragni, D.; Meyers, J.; et al. Validation of four LES and a vortex model against stereo-PIV measurements in the near wake of an actuator disc and a wind turbine. Renew. Energy 2016, 94, 510–523. [Google Scholar] [CrossRef]
- Burton, T.; Sharpe, D.; Jenkins, N.; Bossanyi, E. Wind Energy Handbook; Wiley: Chichester, UK, 2001. [Google Scholar]
- Manwell, J.F.; McGowan, J.G.; Rogers, A.L. Wind Energy Explained: Theory, Design and Application; Wiley: Chichester, UK, 2009. [Google Scholar]
- Ponta, F.L.; Otero, A.D.; Lago, L.I.; Rajan, A. Effects of rotor deformation in wind-turbine performance: The Dynamic Rotor Deformation Blade Element Momentum model (DRD–BEM). Renew. Energy 2016, 92, 157–170. [Google Scholar] [CrossRef]
- Kelley, C.L. Aerodynamic Design of the National Rotor Testbed; Technical Report SAND2015-8989; Sandia National Laboratory: Albuquerque, NM, USA, 2015.
- Kelley, C.L.; Ennis, B.L. SWiFT Site Atmospheric Characterization; Technical Report SAND2016-0216; Sandia National Laboratory: Albuquerque, NM, USA, 2016.
- Berg, J.; Bryant, J.; LeBlanc, B.; Maniaci, D.C.; Naughton, B.; Paquette, J.A.; Resor, B.R.; White, J.; Kroeker, D. Scaled wind farm technology facility overview. In Proceedings of the 32nd ASME Wind Energy Symposium, National Harbor, MD, USA, 13–17 January 2014; p. 1088. [Google Scholar]
- Barone, M.F.; White, J. DOE/SNL-TTU Scaled Wind Farm Technology Facility; Technical Report SAND2011-6522; Sandia National Laboratory: Albuquerque, NM, USA, 2011.
- Jonkman, J.; Butterfield, S.; Musial, W.; Scott, G. Definition of a 5-MW Reference Wind Turbine for Offshore System Development; Technical Report NREL/TP-500-38060; National Renewable Energy Laboratory: Golden, CO, USA, 2009.
- Xudong, W.; Shen, W.Z.; Zhu, W.J.; Sorensen, J.; Jin, C. Shape optimization of wind turbine blades. Wind Energy 2009, 12, 781–803. [Google Scholar] [CrossRef]
- Menon, M.; Ponta, F. Dynamic Aeroelastic Behavior of Wind Turbine Rotors in Rapid Pitch-Control Actions. Renew. Energy 2017, 107, 327–339. [Google Scholar] [CrossRef]
- Menon, M.; Ponta, F. Aeroelastic Response of Wind Turbine Rotors under Rapid Actuation of Flap-Based Flow Control Devices. Fluids 2022, 7, 129. [Google Scholar] [CrossRef]
- Otero, A.D.; Ponta, F.L. On the sources of cyclic loads in horizontal-axis wind turbines: The role of blade-section misalignment. Renew. Energy 2018, 117, 275–286. [Google Scholar] [CrossRef]
- Jalal, S.; Ponta, F.; Baruah, A.; Rajan, A. Dynamic Aeroelastic Response of Stall-Controlled Wind Turbine Rotors in Turbulent Wind Conditions. Appl. Sci. 2021, 11, 6886. [Google Scholar] [CrossRef]
- Jalal, S.; Ponta, F.; Baruah, A. Aeroelastic Response of Variable-Speed Stall-Controlled Wind Turbine Rotors. In Proceedings of the ASME 2019 13th International Conference on Energy Sustainability, Bellevue, WA, USA, 14–17 July 2019; American Society of Mechanical Engineers: New York, NY, USA, 2019; p. ES2019-3803. [Google Scholar]
- Lago, L.I.; Ponta, F.L.; Otero, A.D. Analysis of alternative adaptive geometrical configurations for the NREL-5 MW wind turbine blade. Renew. Energy 2013, 59, 13–22. [Google Scholar] [CrossRef]
- Rajan, A.; Ponta, F.L. A Novel Correlation Model for Horizontal Axis Wind Turbines Operating at High-Interference Flow Regimes. Energies 2019, 12, 1148. [Google Scholar] [CrossRef]
- Hodges, D.H. Nonlinear Composite Beam Theory; AIAA: Reston, VA, USA, 2006. [Google Scholar]
- Yu, W.; Hodges, D.H.; Volovoi, V.; Cesnik, C.E.S. On Timoshenko-like modeling of initially curved and twisted composite beams. Int. J. Sol. Struct. 2002, 39, 5101–5121. [Google Scholar] [CrossRef]
- Otero, A.D.; Ponta, F.L. Structural Analysis of Wind-Turbine Blades by a Generalized Timoshenko Beam Model. J. Sol. Energy Eng. 2010, 132, 011015. [Google Scholar] [CrossRef]
- Baruah, A.; Ponta, F. Analysis of Wind Turbine Wake Dynamics by a Gaussian-Core Vortex Lattice Technique. Dynamics 2024, 4, 97–118. [Google Scholar] [CrossRef]
- Herges, T.; Maniaci, D.C.; Naughton, B.T.; Mikkelsen, T.; Sjöholm, M. High resolution wind turbine wake measurements with a scanning lidar. J. Phys. Conf. Ser. 2017, 854, 012021. [Google Scholar] [CrossRef]
- Batchelor, G.K. An Introduction to Fluid Dynamics; Cambridge University Press: Cambridge, UK, 2000. [Google Scholar]
- Ponta, F.L.; Jacovkis, P.M. A vortex model for Darrieus turbine using finite element techniques. Renew. Energy 2001, 24, 1–18. [Google Scholar] [CrossRef]
- Strickland, J.H.; Webster, B.T.; Nguyen, T. A Vortex Model of the Darrieus Turbine: An Analytical and Experimental Study. J. Fluids Eng. 1979, 101, 500–505. [Google Scholar] [CrossRef]
- Ponta, F.L. Vortex decay in the Kármán eddy street. Phys. Fluids 2010, 22, 093601. [Google Scholar] [CrossRef]
- Trieling, R.R.; van Wesenbeeck, J.M.A.; van Heijst, G.J.F. Dipolar vortices in a strain flow. Phys. Fluids 1998, 10, 144–159. [Google Scholar] [CrossRef]
- Flór, J.B.; van Heijst, G.J.F. An experimental study of dipolar structures in a stratified fluid. J. Fluid Mech. 1994, 279, 101–133. [Google Scholar] [CrossRef]
- Lamb, H. Hydrodynamics, 6th ed.; Cambridge University Press: Cambridge, UK, 1932. [Google Scholar]
- Hooker, S.G. On the action of viscosity in increasing the spacing ration of a vortex street. Proc. R. Soc. 1936, A154, 67–89. [Google Scholar]
- Karamcheti, K. Principles of Ideal-Fluid Aerodynamics; Wiley: New York, NY, USA, 1966. [Google Scholar]
- Cottet, G.H.; Koumoutsakos, P.D. Vortex Methods: Theory and Practice; Cambridge University Press: London, UK, 2000. [Google Scholar]
- Kelley, C.; Naughton, B. Surface Meteorological Station-SWiFT Southwest-METa1-Reviewed Data. 2021. Available online: https://www.osti.gov/biblio/1349888 (accessed on 20 December 2023).
- Trudnowski, D.; LeMieux, D. Independent pitch control using rotor position feedback for wind-shear and gravity fatigue reduction in a wind turbine. In Proceedings of the 2002 American Control Conference (IEEE Cat. No. CH37301), Anchorage, AK, USA, 8–10 May 2002; Volume 6, pp. 4335–4340. [Google Scholar]
- EWEA. Upwind: Design Limits and Solutions for Very Large Wind Turbines; Sixth Framework Programme; European Wind Energy Association: Brussels, Belgium, 2011. [Google Scholar]
- Hohenemser, K.H.; Swift, A.; Peters, D. Yawing of Wind Turbines with Blade Cyclic Pitch Variation; Technical Report; Solar Energy Research Institute: Golden, CO, USA; Washington University Technology Associates, Inc.: St. Louis, MO, USA, 1981. [Google Scholar]
- Jonkman, J.M.; Buhl, M.L., Jr. FAST User’s Guide; Technical Report NREL/EL-500-38230; National Renewable Energy Laboratory (NREL): Golden, CO, USA, 2005.
- Zong, H.; Porté-Agel, F. Experimental investigation and analytical modelling of active yaw control for wind farm power optimization. Renew. Energy 2021, 170, 1228–1244. [Google Scholar] [CrossRef]
- Porté-Agel, F.; Bastankhah, M.; Shamsoddin, S. Wind-Turbine and Wind-Farm Flows: A Review. Bound.-Layer Meteorol. 2020, 174, 1–59. [Google Scholar] [CrossRef]
- Abkar, M.; Sørensen, J.N.; Porté-Agel, F. An Analytical Model for the Effect of Vertical Wind Veer on Wind Turbine Wakes. Energies 2018, 11, 1838. [Google Scholar] [CrossRef]
- Su, K.; Bliss, D. A numerical study of tilt-based wake steering using a hybrid free-wake method. Wind Energy 2020, 23, 258–273. [Google Scholar] [CrossRef]



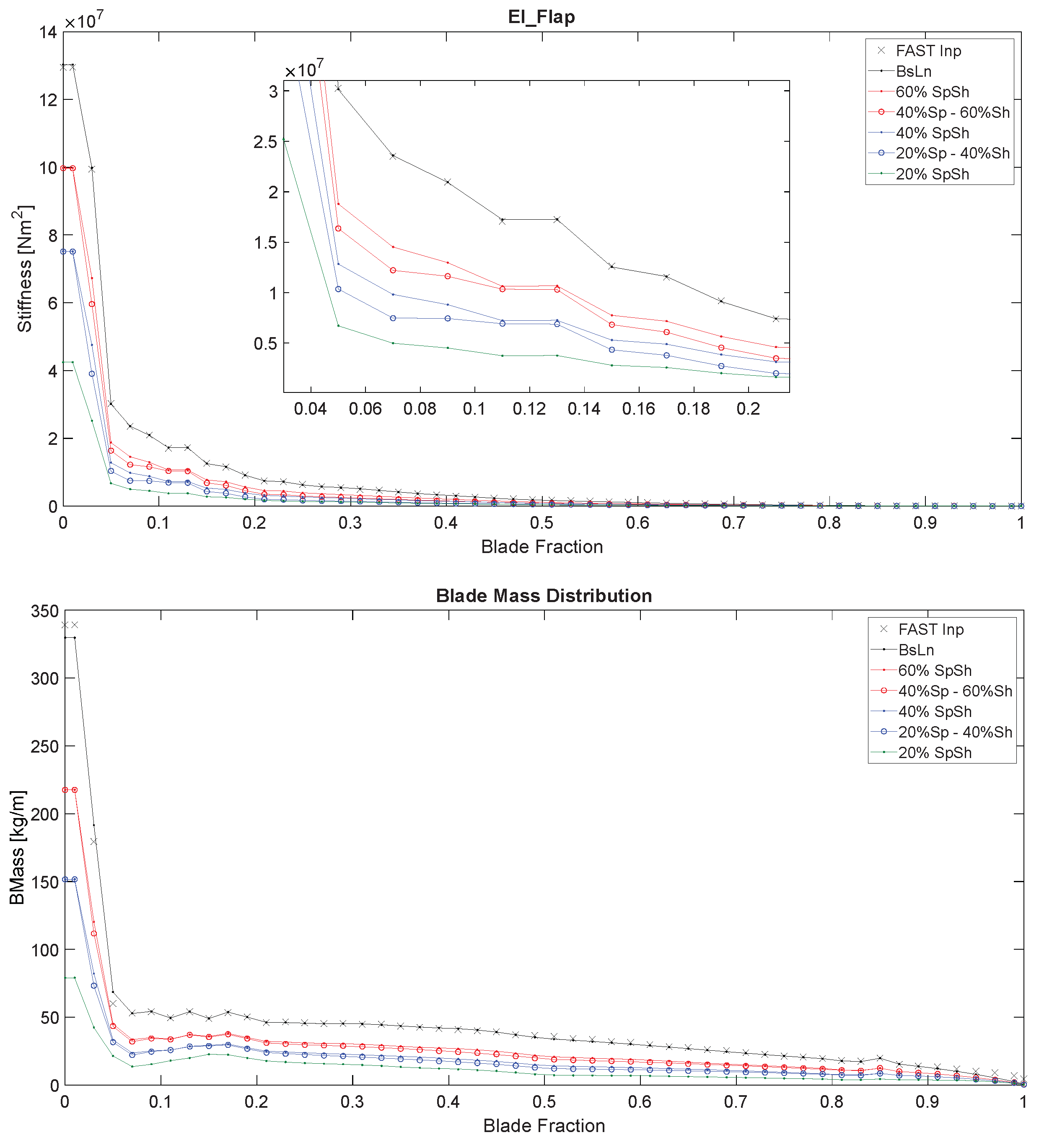


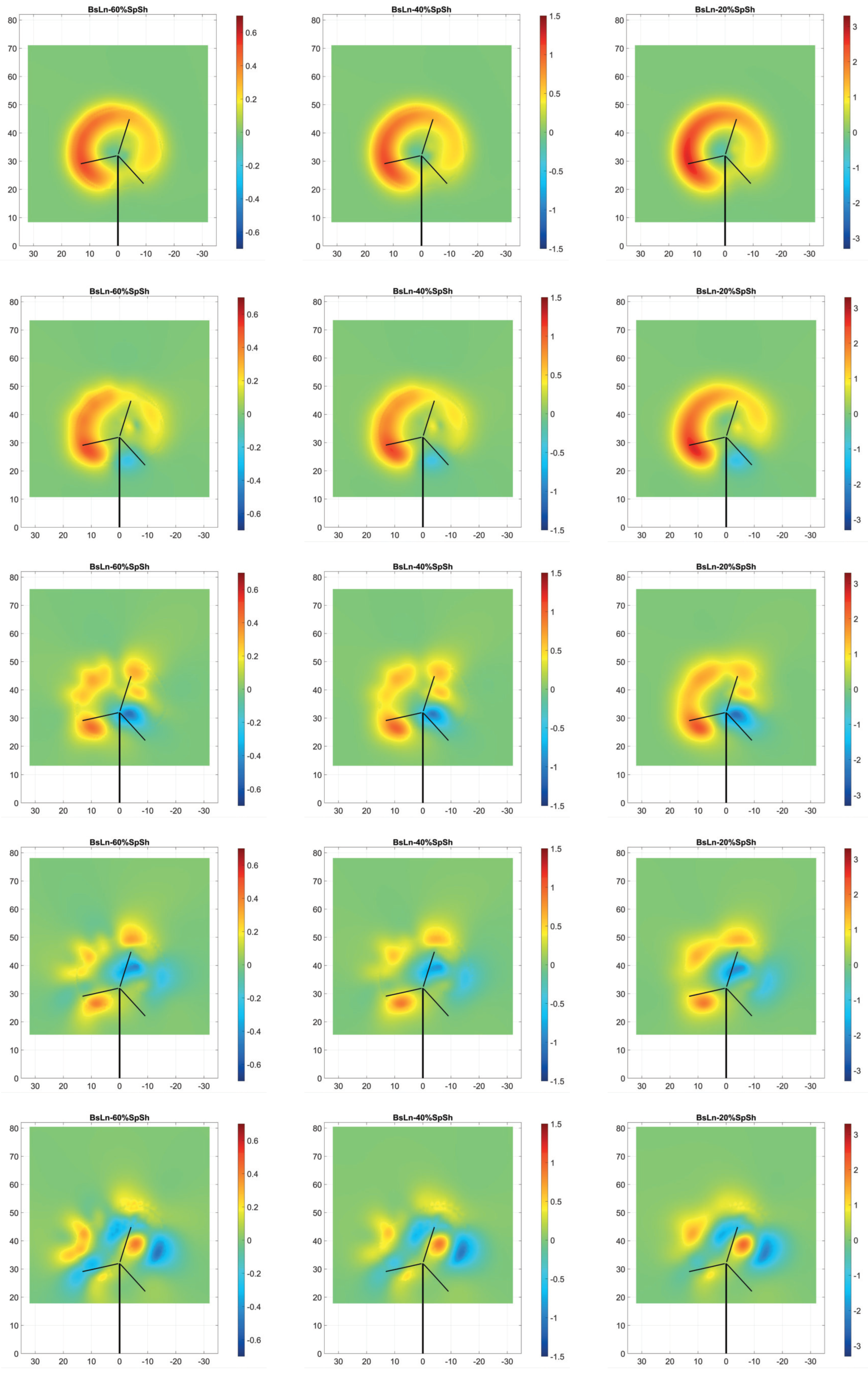
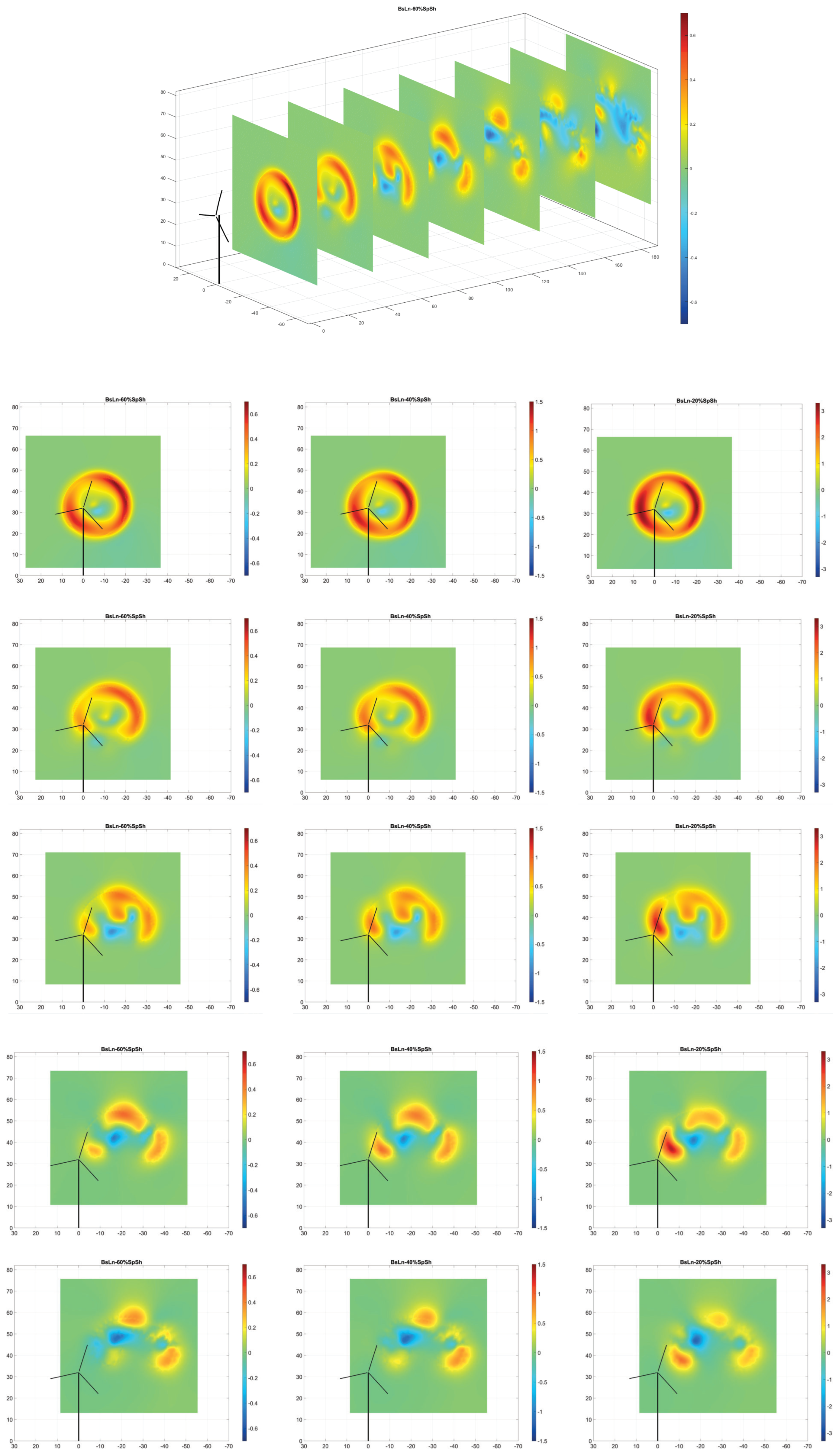

| Blade Variant | Mass [Kg] | % BsLn Mass |
|---|---|---|
| BsLn | 551.157 | 100.000 |
| 60%SpSh | 360.594 | 65.425 |
| 40%Sp–60%Sh | 344.863 | 62.571 |
| 40%SpSh | 260.445 | 47.254 |
| 20%Sp–40%Sh | 244.601 | 44.380 |
| 20%SpSh | 156.286 | 28.356 |
| Blade | Thrust | Thrust | Torque | Torque | BTD | BTD |
|---|---|---|---|---|---|---|
| Variation | Mean [N] | StD [N] | Mean [Nm] | StD [Nm] | Mean [m] | StD [m] |
| BsLn | 9600.17 | 4.18 | 7960.01 | 8.35 | 0.2249 | 0.0042 |
| 60%SpSh | 9550.13 | 3.11 | 7917.92 | 6.21 | 0.3480 | 0.0062 |
| 40%Sp–60%Sh | 9544.84 | 2.89 | 7900.56 | 5.99 | 0.4276 | 0.0074 |
| 40%SpSh | 9487.81 | 2.34 | 7862.99 | 4.69 | 0.4963 | 0.0081 |
| 20%Sp–40%Sh | 9471.22 | 1.83 | 7816.39 | 3.95 | 0.6964 | 0.0105 |
| 20%SpSh | 9295.47 | 1.32 | 7686.43 | 2.77 | 0.9060 | 0.0112 |
| Blade | Thrust | Thrust | Torque | Torque | BTD | BTD |
|---|---|---|---|---|---|---|
| Variation | Mean [N] | StD [N] | Mean [Nm] | StD [Nm] | Mean [m] | StD [m] |
| BsLn | 9334.01 | 23.29 | 7603.79 | 31.74 | 0.2186 | 0.0188 |
| 60%SpSh | 9279.05 | 17.92 | 7557.24 | 21.86 | 0.3380 | 0.0292 |
| 40%Sp–60%Sh | 9272.04 | 17.31 | 7535.57 | 23.14 | 0.4152 | 0.0362 |
| 40%SpSh | 9209.71 | 13.61 | 7495.70 | 14.82 | 0.4813 | 0.0417 |
| 20%Sp–40%Sh | 9188.77 | 12.21 | 7436.90 | 15.03 | 0.6748 | 0.0581 |
| 20%SpSh | 8998.35 | 8.48 | 7307.43 | 15.34 | 0.8750 | 0.0782 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Farrell, A.; Ponta, F.; Baruah, A. Analysis of the Aeroelastic Dynamics of Lightweight Flexible Variations of the SNL-NRT Turbine. Appl. Mech. 2024, 5, 280-304. https://doi.org/10.3390/applmech5020017
Farrell A, Ponta F, Baruah A. Analysis of the Aeroelastic Dynamics of Lightweight Flexible Variations of the SNL-NRT Turbine. Applied Mechanics. 2024; 5(2):280-304. https://doi.org/10.3390/applmech5020017
Chicago/Turabian StyleFarrell, Alayna, Fernando Ponta, and Apurva Baruah. 2024. "Analysis of the Aeroelastic Dynamics of Lightweight Flexible Variations of the SNL-NRT Turbine" Applied Mechanics 5, no. 2: 280-304. https://doi.org/10.3390/applmech5020017
APA StyleFarrell, A., Ponta, F., & Baruah, A. (2024). Analysis of the Aeroelastic Dynamics of Lightweight Flexible Variations of the SNL-NRT Turbine. Applied Mechanics, 5(2), 280-304. https://doi.org/10.3390/applmech5020017





