Analysis and Research on the Comprehensive Performance of Vehicle Magnetorheological Regenerative Suspension
Abstract
1. Introduction
2. 1/4 MR Regenerative Suspension System
2.1. MR Semi-Active Vehicle Suspension
2.2. Design of Regenerative TPMLM
3. Establishment of a Joint Simulation Platform
4. Comprehensive Performance Analysis of Regenerative Suspension
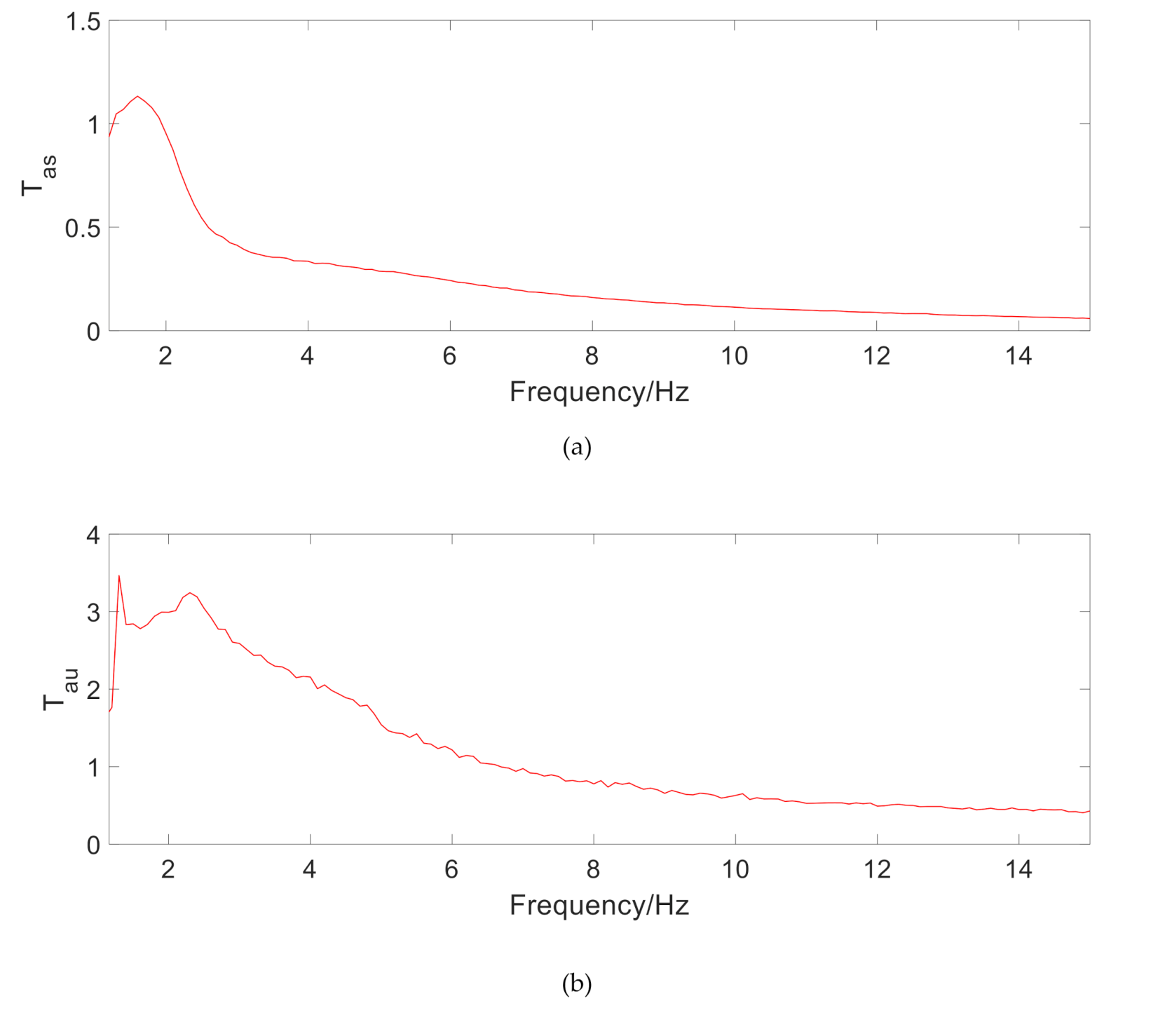
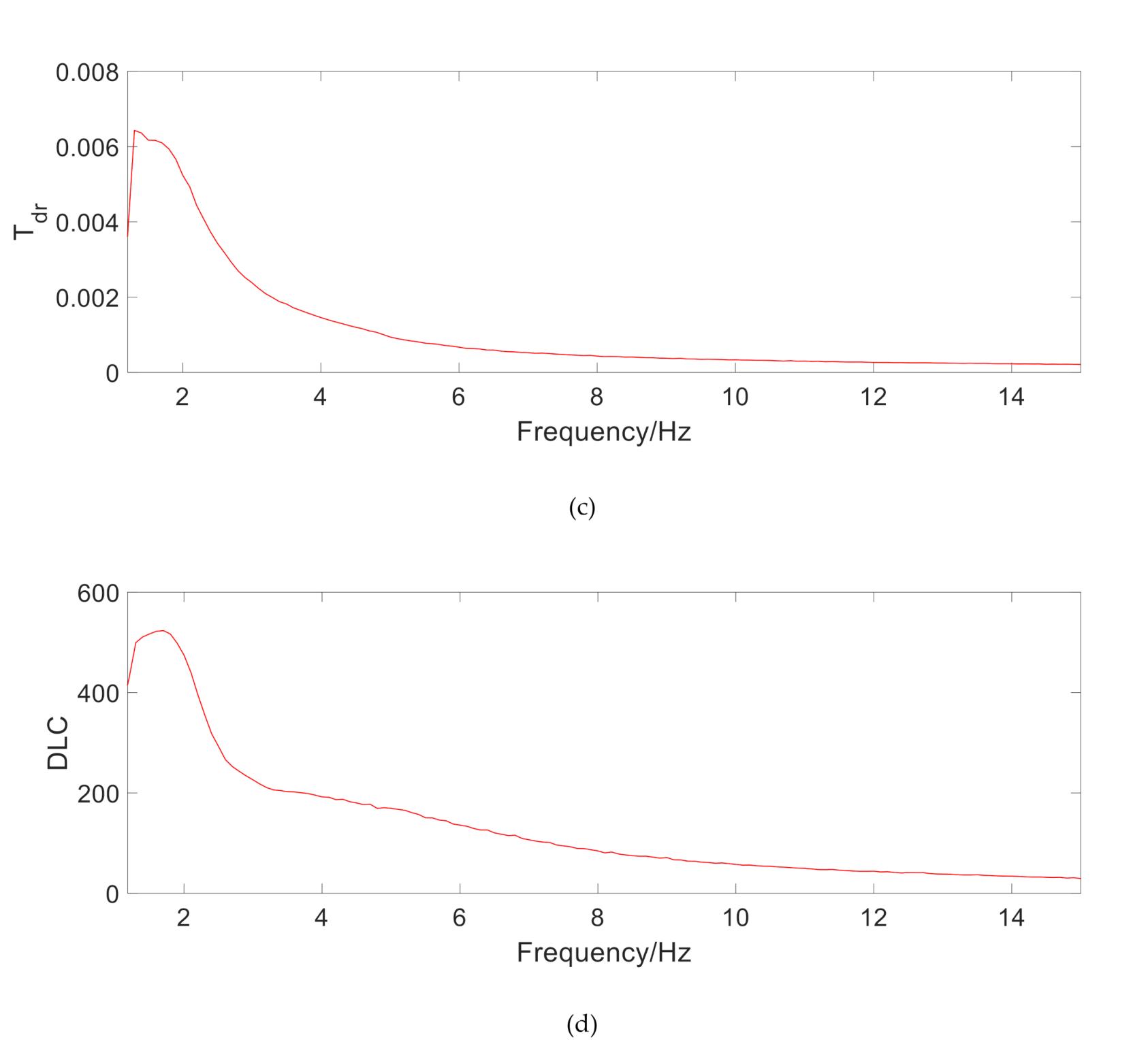
4.1. Suspension Performance
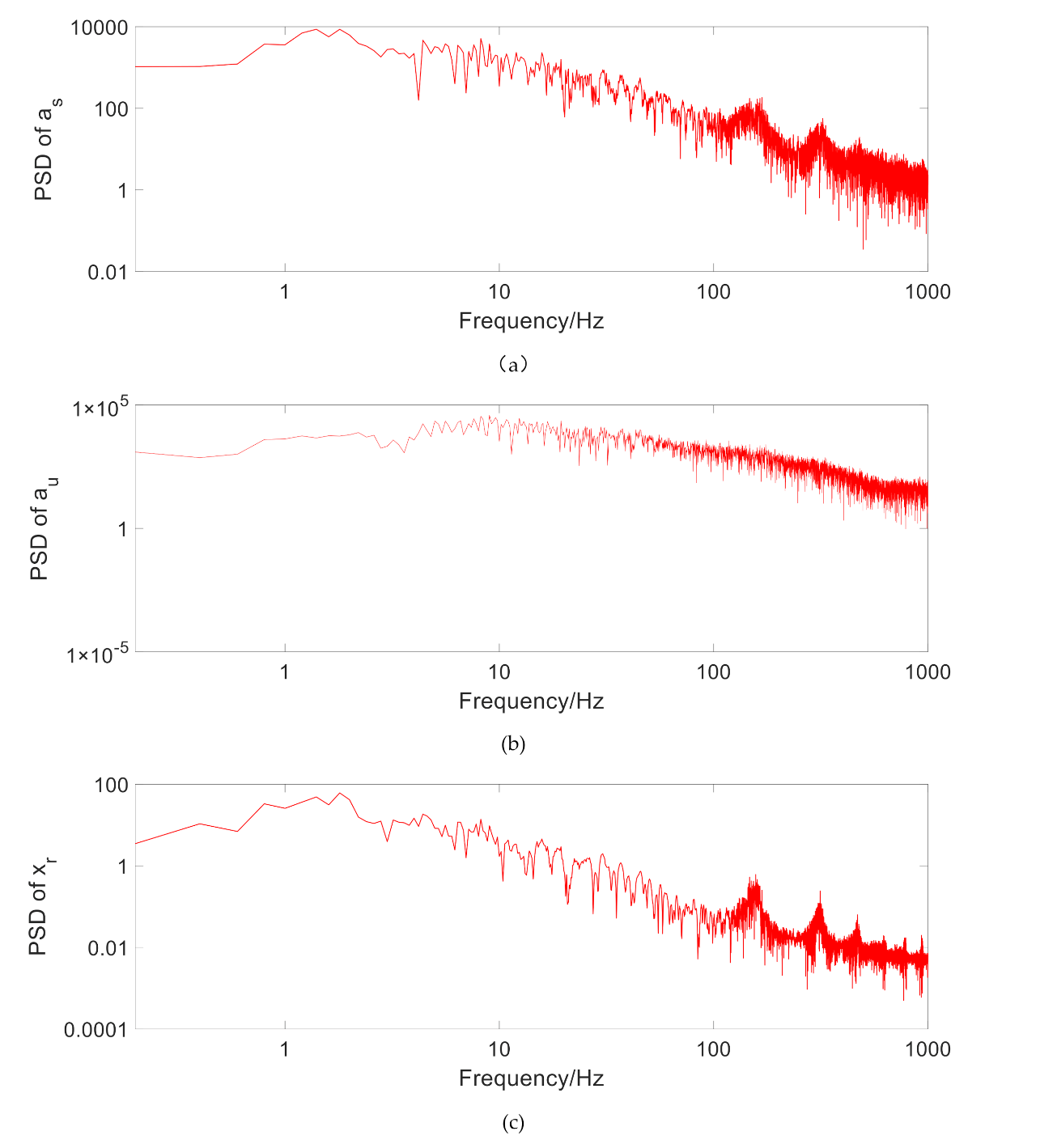
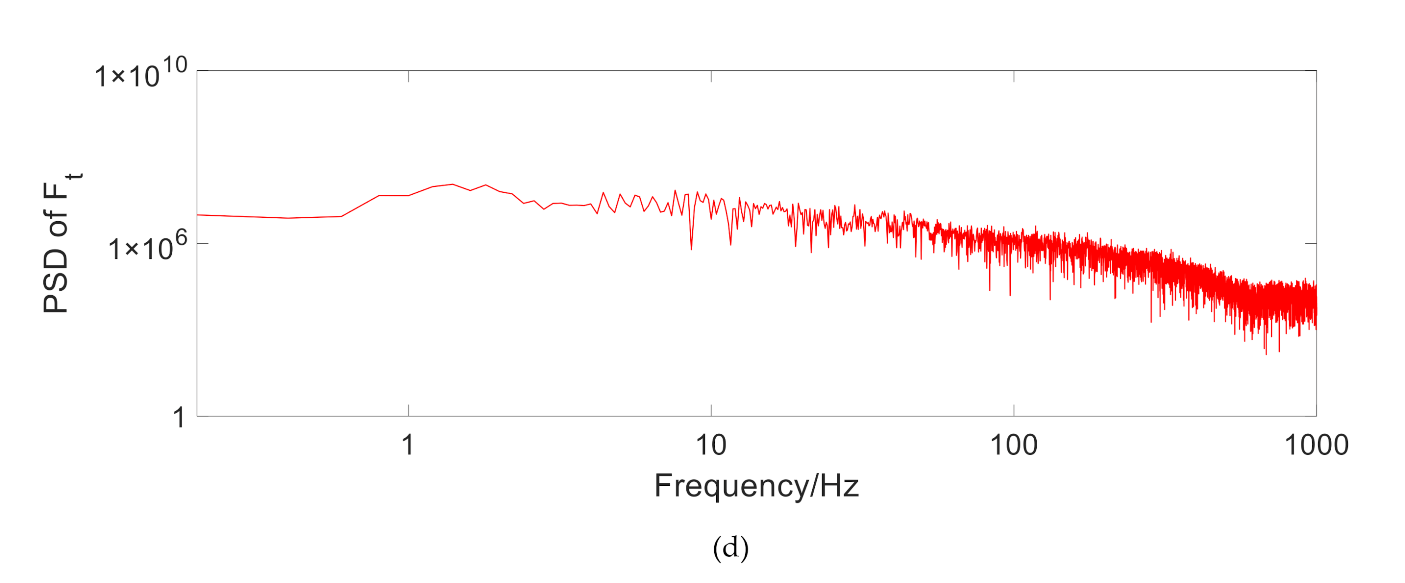
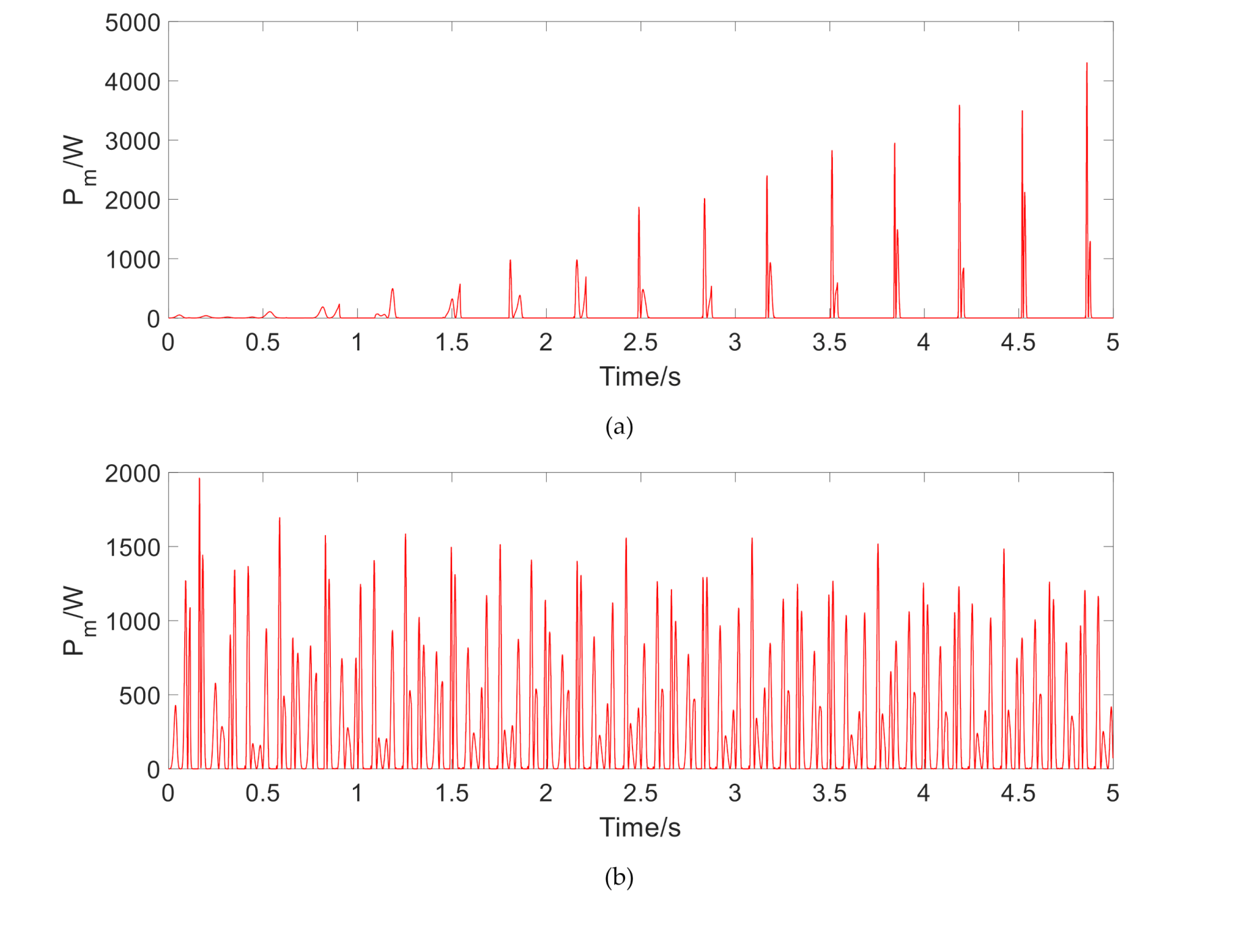
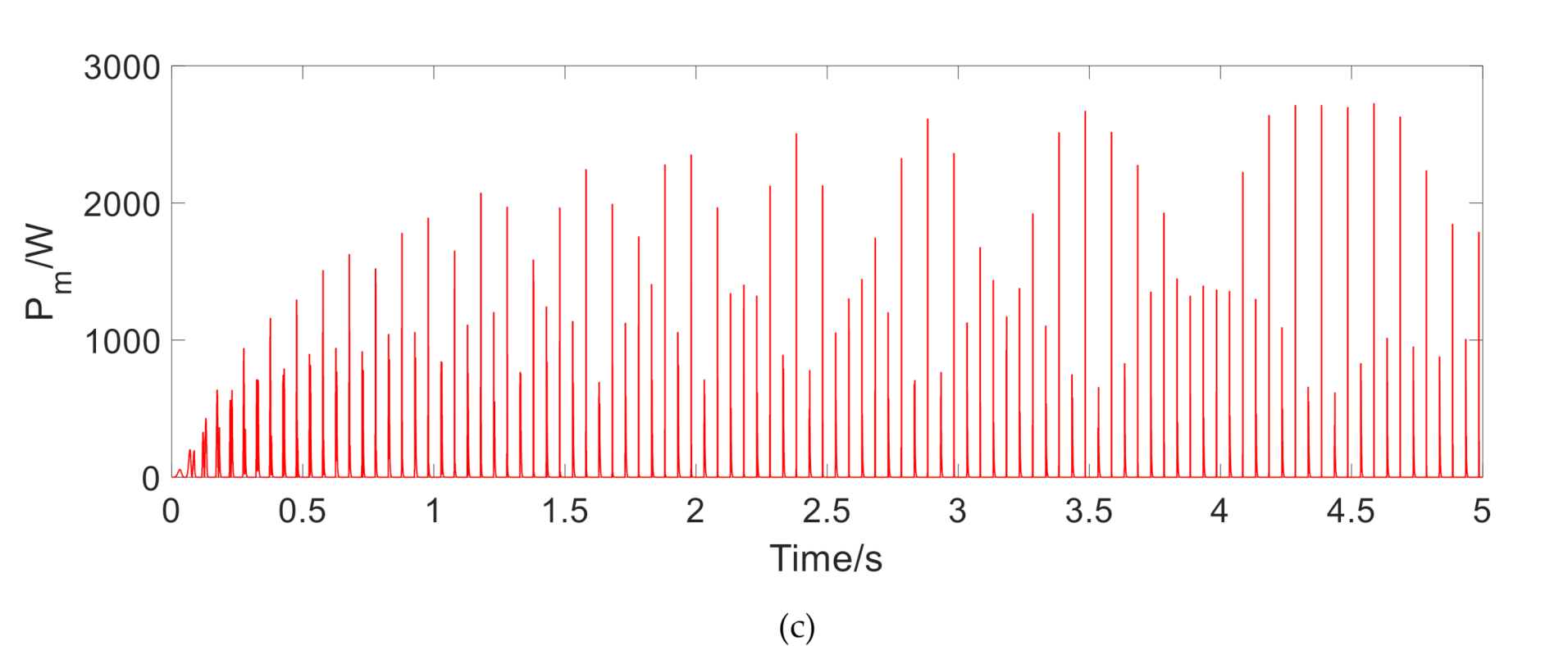
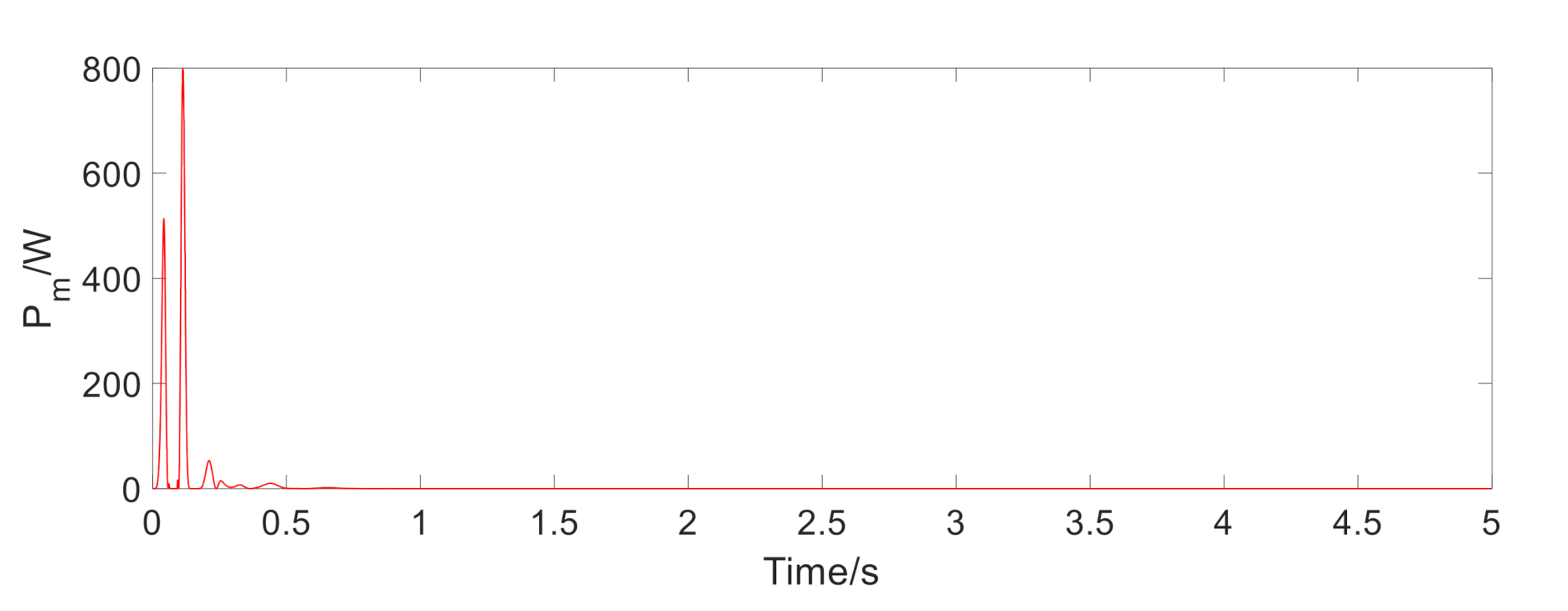
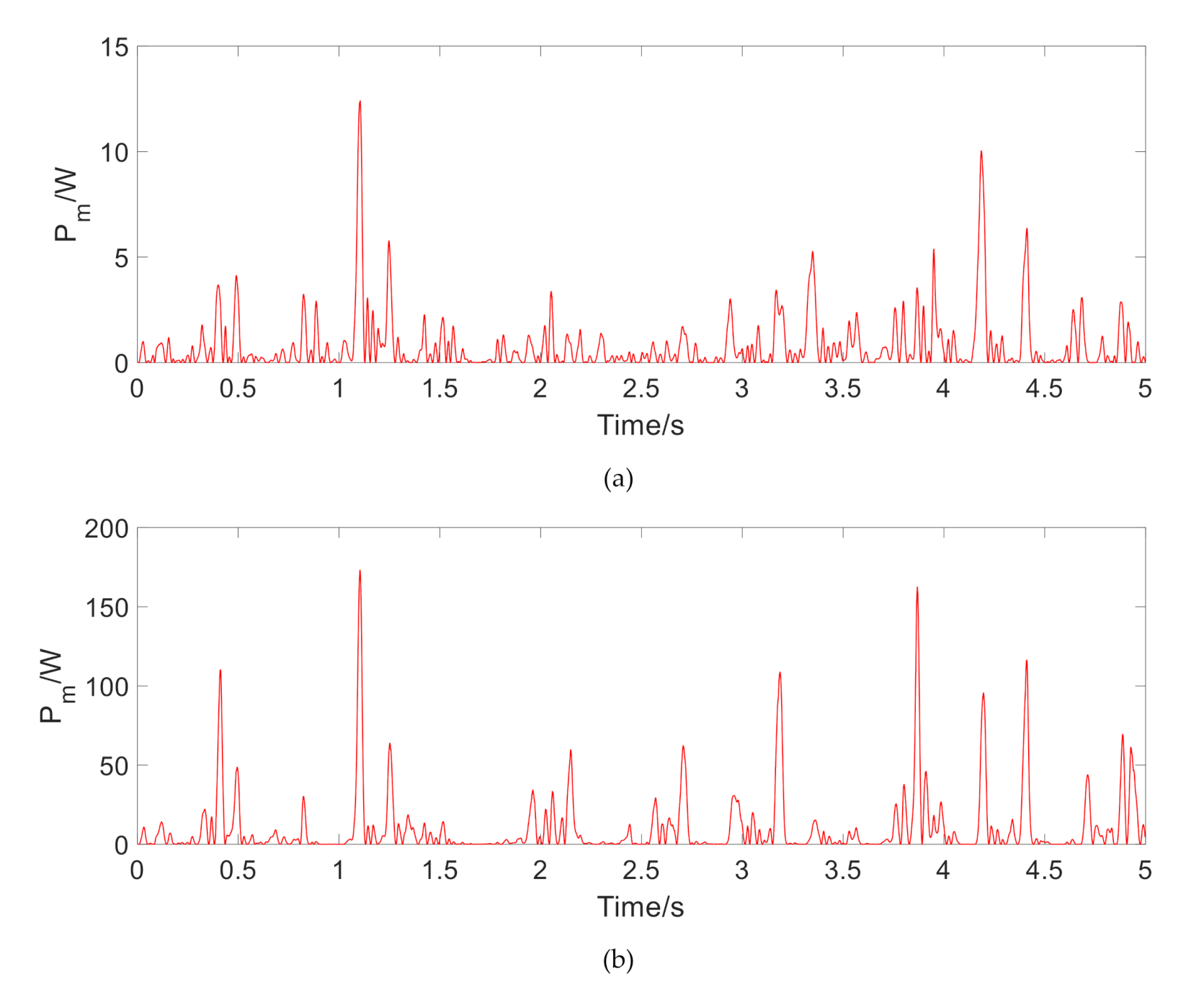
4.2. Regenerative Characteristics
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Where the Energy Goes: Gasoline Vehicles. Available online: https://www.fueleconomy.gov/FEG/atv.shtml (accessed on 6 May 2019).
- Karnopp, D. Power requirements for traversing uneven roadways. Veh. Syst. Dyn. 1978, 7, 135–152. [Google Scholar] [CrossRef]
- Browne, A.L.; Hamburg, J.A. On-road measurement of the energy dissipated in automotive shock absorbers. In Symposium on Simulation and Control of Ground Vehicles and Transportation Systems; American Society of Mechanical Engineers: New York, NY, USA, 1986; Volume 80, pp. 1–10. [Google Scholar]
- Huang, K.; Zhang, Y.C.; Yu, F. Coordinated optimization of comprehensive performance of electric active energy feedback suspension. J. Shanghai Jiaotong Uni. 2009, 43, 226–230. [Google Scholar]
- Yu, F.; Zhang, Y.C. Active suspension technology of energy feedback vehicle. Trans. Chin. Soc. Agric. Mach. 2010, 41, 1–6. [Google Scholar]
- Dong, X.M.; Peng, S.J.; Yu, J.Q. Study on characteristics of self powered vehicle magnetorheological damper. J. Mech. Eng. 2016, 52, 83–91. [Google Scholar] [CrossRef]
- Kou, F.R.; Liang, J.; Wei, D.D. Research on a parallel semi-active suspension actuator. China Mech. Eng. 2017, 28, 2318–2324. [Google Scholar]
- Zhu, Z.Y.; Zheng, L.; Wang, K. Energy feedback characteristics and experimental study of self powered rheological damper. In Proceedings of the 2014 Annual Meeting of China Society of Automotive Engineering, Shanghai, China, 22 October 2014; pp. 1210–1216. [Google Scholar]
- Kou, F.R.; Chen, L.; Zhang, W. Study on characteristics of self powered magnetorheological semi-active suspension. Hydraul. Pneum. 2016, 11, 10–14. [Google Scholar]
- Lv, X.Y.; Ji, Y.J.; Zhao, H.Y.; Zhang, J.; Zhang, G.; Zhang, L. Research Review of a Vehicle Energy-Regenerative Suspension System. Energies 2020, 13, 441. [Google Scholar] [CrossRef]
- Zhang, H.L.; Wang, E.R.; Min, F.H.; Subash, R.; Su, C. Skyhook-based Semi-active Control of Full-vehicle Suspension with Magneto-rheological Dampers. Chin. J. Mech. Eng. 2013, 26, 498–505. [Google Scholar] [CrossRef]
- Zhu, Z.Q.; Howe, D. Halbach Permanent Magnet Machines and Applications: A Review. IEE Proc. Electr. Power Appl. 2001, 148, 299–308. [Google Scholar] [CrossRef]
- Lu, D.S.; Zhang, H.L.; Liu, J.; Wang, E. Structural Parameter Optimization of a Tubular Permanent-Magnet Linear Machine for Regenerative Suspension. Shock Vib. 2019, 2019, 11. [Google Scholar] [CrossRef]
- Young, K.D.; Utkin, V.I.; Ozguner, U. A control engineer’s guide to sliding mode control. IEEE Trans. Control Syst. Technol. 1999, 7, 328–342. [Google Scholar] [CrossRef]

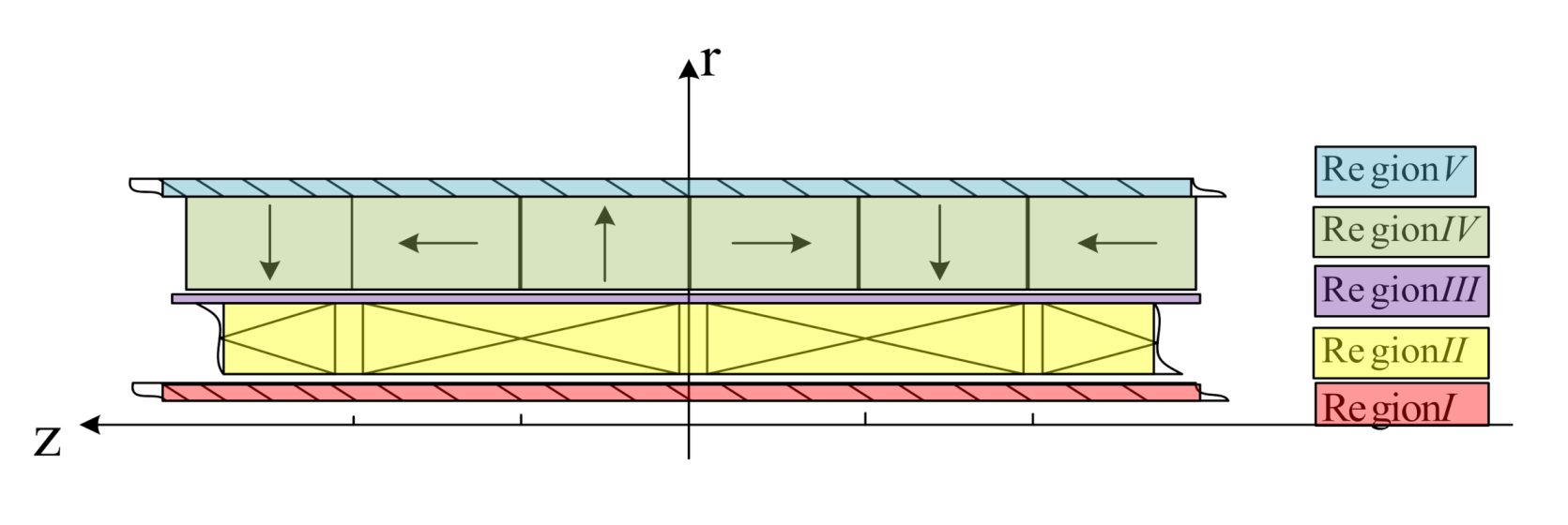



| Structural Parameters | Value | Unit | |
|---|---|---|---|
| Cross-sectional radius | 4 | mm | |
| RI | 8 | ||
| RII | 16 | ||
| RIII | 17 | ||
| RIV | 36 | ||
| RV | 40 | ||
| Relative permeability | μrI | 400 | H/m |
| μrII | 1 | ||
| μrIII | 1 | ||
| μrIV | 1.02 | ||
| μrV | 400 | ||
| Permanent magnet polar distance | 33 | mm | |
| Permanent magnet remanence | 1.23 | T | |
| Winding slots number | 6 | ||
| Winding turns number | 100 | ||
| Winding full slot rate | 0.9 | ||
| Winding direct current resistance | 0.42 | ||
| The conductivity of the winding wire | 17.5 | nS/m | |
| Winding single slot cross-sectional area | 264 | ||
| Regenerative suspension parameters | 562.5 | Kg | |
| 90 | Kg | ||
| 57,000 | N/m | ||
| 2500 | N/ | ||
| 285,000 | N/m | ||
| 100 | N/ | ||
| Types of Road Excitation | ||||
|---|---|---|---|---|
| Sinusoidal | Frequency (Hz) | |||
| 1.5 | 142.2825 | 497.2003 | ||
| 6 | 308.8829 | 493.1127 | ||
| 15 | 389.2749 | 771.0291 | ||
| Smooth pulse | 5.8977 | 52.2608 | ||
| Random road | Road class | |||
| A | 0.7540 | 1.4778 | ||
| B | 9.7138 | 22.2265 | ||
| C | 18.6516 | 46.0925 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Huang, J.; Wang, E.; Zhang, H. Analysis and Research on the Comprehensive Performance of Vehicle Magnetorheological Regenerative Suspension. Vehicles 2020, 2, 576-588. https://doi.org/10.3390/vehicles2040033
Huang J, Wang E, Zhang H. Analysis and Research on the Comprehensive Performance of Vehicle Magnetorheological Regenerative Suspension. Vehicles. 2020; 2(4):576-588. https://doi.org/10.3390/vehicles2040033
Chicago/Turabian StyleHuang, Jinhui, Enrong Wang, and Hailong Zhang. 2020. "Analysis and Research on the Comprehensive Performance of Vehicle Magnetorheological Regenerative Suspension" Vehicles 2, no. 4: 576-588. https://doi.org/10.3390/vehicles2040033
APA StyleHuang, J., Wang, E., & Zhang, H. (2020). Analysis and Research on the Comprehensive Performance of Vehicle Magnetorheological Regenerative Suspension. Vehicles, 2(4), 576-588. https://doi.org/10.3390/vehicles2040033




