Thermal Decomposition of Compounds Derived from 2H-Dihydropyran: A Computational Study
Abstract
1. Introduction
2. Methods
3. Results and Discussion
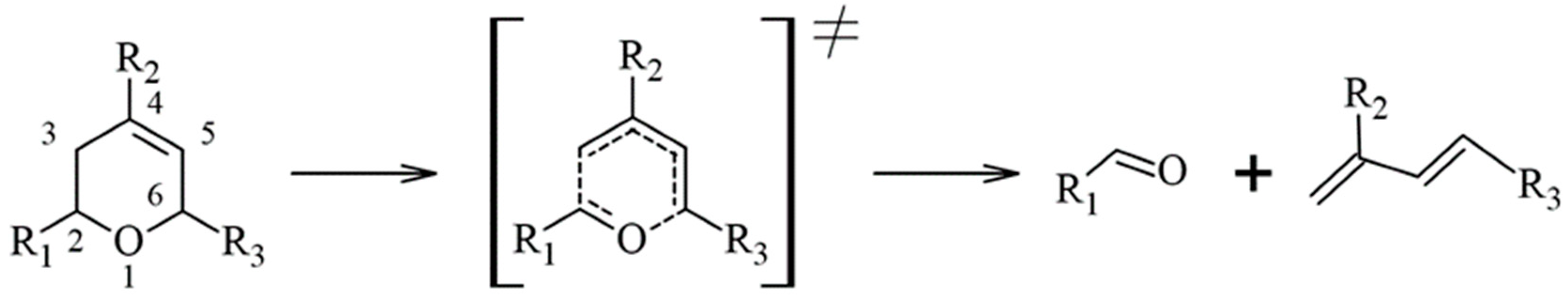
3.1. Reaction Mechanism
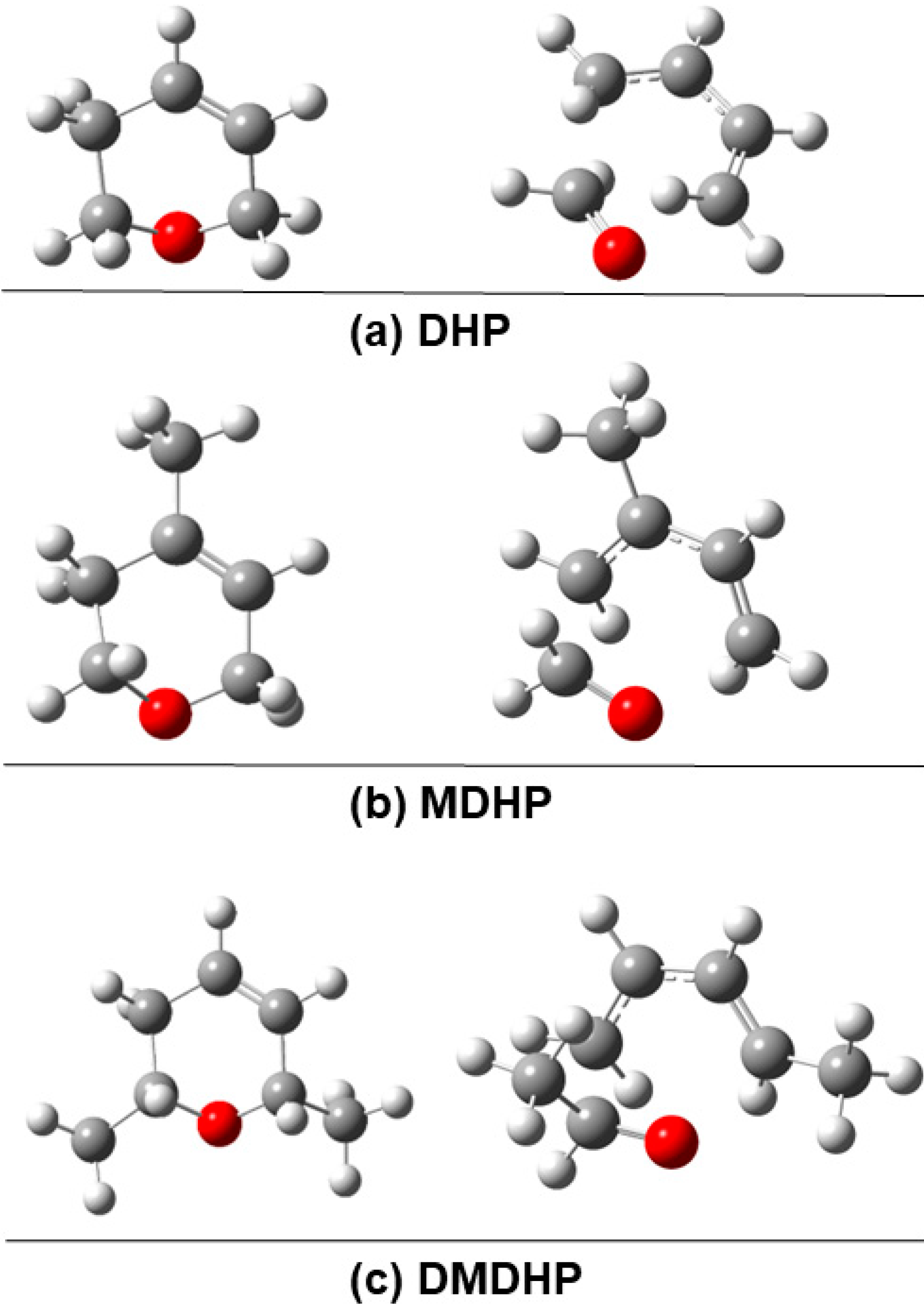
3.2. Structure Optimization
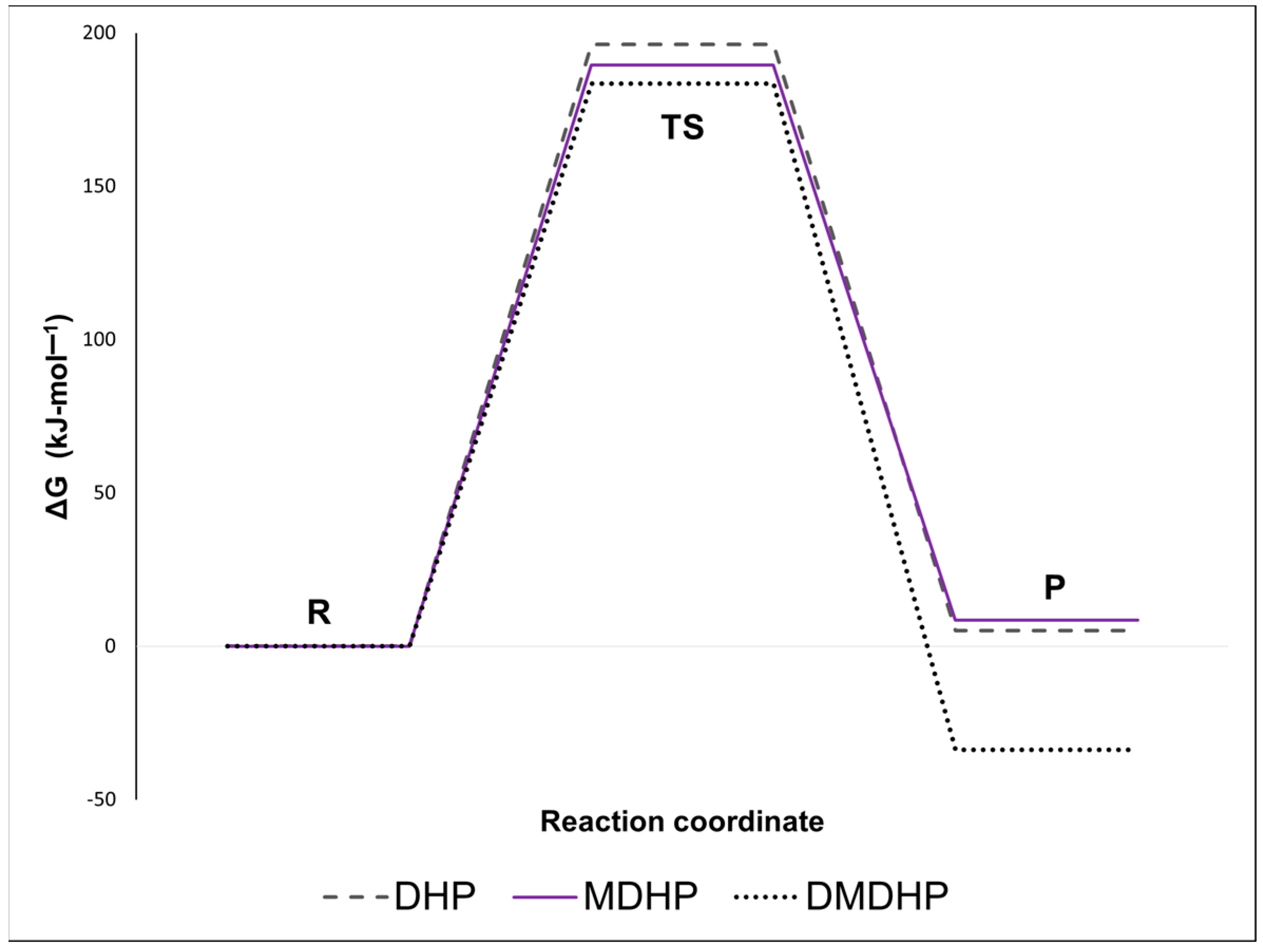
3.3. Reaction Kinetics
3.4. Population Analysis (NBO)
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Mcnaught, A.D.; Smith, P.A.S.; García Alcolea, E. Nomenclatura de Compuestos Heterociclos; Ediciones de la Universidad de Murcia (Editum): Murcia, Spain, 1992; pp. 91–122. [Google Scholar]
- Abrishami, F.; Teimuri-Mofrad, R.; Bayat, Y.; Shahrisa, A. Synthesis of some aldoxime derivatives of 4H-pyran-4-ones. Molecules 2002, 7, 154–196. [Google Scholar] [CrossRef]
- Eskandari, K.; Rafieian-Kopaei, M. Synthesis of 5,6-dihydro-2H-pyran-2-ones (microreview). Chem. Heterocycl. Compd. 2016, 52, 158–160. [Google Scholar] [CrossRef]
- Kate, P.; Pandit, V.; Jawale, V.; Bachute, M.L. Proline catalyzed one-pot three-component synthesis and evaluation for biological activities of tetrahydrobenzo[b]pyran: Evaluation by green chemistry metrics. J. Chem. Sci. 2022, 134, 4. [Google Scholar] [CrossRef]
- Borah, B.; Dwivedi, K.D.; Chowhan, L.R. Review on synthesis and medicinal application of dihydropyrano [3,2-b]pyrans and spiro-pyrano[3,2-b]pyrans by employing the reactivity of 5-hydroxy-2-(hydroxymethyl)-4hpyran-4-one. Polycycl. Aromat. Compd. 2022, 42, 5893–5937. [Google Scholar] [CrossRef]
- Alam, S.; Sarkar, Z.; Islam, A. Synthesis and studies of antibacterial activity of pongaglabol. J. Chem. Sci. 2004, 116, 29–32. [Google Scholar] [CrossRef]
- Aytemir, M.D.; Erol, D.D.; Hider, R.C.; Özalp, M. Synthesis and evaluation of antimicrobial activity of new 3-hydroxy-6-methyl-4-oxo-4H-pyran-2-carboxamide derivatives. Turkish J. Chem. 2003, 27, 757–764. [Google Scholar]
- Mahdavi, S.M.; Habibi, A.; Dolati, H.; Mohammad, S.; Sardari, S.; Azerang, P. Synthesis and antimicrobial evaluation of 4H-pyrans and schiff bases fused 4H-pyran derivatives as inhibitors of mycobacterium bovis (BCG). Iran. J. Pharm. Res. 2018, 17, 1229–1239. [Google Scholar]
- Randhawa, P.; Farasati, N.A.; Huang, Y. BK virus replication in vitro: Limited effect of drugs interfering with viral uptake and intracellular transport. Antimicrob. Agents Chemother. 2007, 51, 4492–4494. [Google Scholar] [CrossRef]
- Akhlaghi, Z.; Naimi-Jamal, M.R.; Panahi, L.; Dekamin, M.G.; Far, B.F. Solvent-free mechanochemical multicomponent preparation of 4H-pyrans catalyzed by Cu2(NH2-BDC)2(DABCO) metal-organic framework. Heliyon 2023, 9, e13522. [Google Scholar] [CrossRef]
- Aytemir, M.D.; Çalış, Ü. Synthesis of some novel Mannich bases derived from allomaltol and evaluation of their anticonvulsant activities. Hacettepe Univ. J. Fac. Pharm. 2007, 27, 1–10. [Google Scholar]
- Kaplan, J.; Verheijen, J.C.; Brooijmans, N.; Toral-Barza, L.; Hollander, I.; Yu, K.; Zask, A. Discovery of 3,6-dihydro-2H-pyran as a morpholine replacement in 6-aryl-1H-pyrazolo[3,4-d]pyrimidines and 2-arylthieno[3,2-d]pyrimidines: ATP-competitive inhibitors of the mammalian target of rapamycin (mTOR). Bioorg. Med. Chem. Lett. 2010, 20, 640–643. [Google Scholar] [CrossRef] [PubMed]
- Bonsignorel, L.; Loyl, G.; Seccil, D.; Calignanoz, A. Synthesis and pharmacological activity of 2-oxo-(2H) 1-benzopyran-3-carboxamide derivatives. Eur. J. Med. Chem. 1993, 28, 517–520. [Google Scholar] [CrossRef]
- Uenishi, J.; Ohmi, M. Total synthesis of (-)-laulimalide: Pd-catalyzed stereospecific ring construction of the substituted 3,6-dihydro[2H]pyran units. Angew. Chem. Int. Ed. 2005, 44, 2756–2760. [Google Scholar] [CrossRef] [PubMed]
- Maulide, N. Six-membered rings with one heteroatom, and their fused carbocyclic derivatives. In Comprehensive Heterocyclic Chemistry IV, 1st ed.; Black, D., Cossy, J., Stevens, C., Eds.; Elsevier: Amsterdam, The Netherlands, 2021; Volume 7, pp. 491–511. [Google Scholar]
- Mousavi, M.R.; Maghsoodlou, M.T.; Noori, F.; Hazeri, N. A facile and efficient synthesis of tetrahydrobenzo[b]pyrans using sucrose as green, inexpensive, natural and biodegradable catalyst. Org. Chem. Res. 2015, 1, 66–71. [Google Scholar]
- Baker, C.D.L.; Fawcett, J.; Insley, C.D.; Messenger, D.S.; Newland, C.L.; Overend, H.L.; Patel, A.B.; Shah, M.; Vara, B.; Virdee, D.; et al. Triol protection with 6-benzoyl-3,4-dihydro-(2H)-pyran. Chem. Commun. 2005, 1883–1885. [Google Scholar] [CrossRef]
- Joule, J.A.; Mills, K. Heterocyclic Chemistry, 5th ed.; John Wiley & Sons, Ltd.: Chichester, UK, 2010; pp. 1–689. [Google Scholar]
- Babinszki, B.; Jakab, E.; Terjék, V.; Sebestyén, Z.; Várhegyi, G.; May, Z.; Mahakhant, A.; Attanatho, L.; Suemanotham, A.; Thanmongkhon, Y.; et al. Thermal decomposition of biomass wastes derived from palm oil production. J. Anal. Appl. Pyrolysis 2021, 155, 105069. [Google Scholar] [CrossRef]
- Chithiravel, R.; Rajaguru, K.; Muthusubramanian, S.; Bhuvanesh, N. Characterization of the major isomers of substituted tetrahydro-2H-pyrans from 1,3,5-triarylpentane-1,5-diols by p-toluenesulfonic acid treatment. J. Mol. Struct. 2018, 1156, 684–689. [Google Scholar] [CrossRef]
- Cano, R.; Ramón, D.J.; Yus, M. Unmodified nano-powder magnetite or iron(III) oxide catalyze the easy and fast synthesis of 4-substituted-4H-pyrans. Synlett 2011, 14, 2017–2020. [Google Scholar] [CrossRef]
- Uenishi, J.; Ohmi, M.; Ueda, A. PdII-catalyzed stereospecific formation of tetrahydro- and 3,6-dihydro [2H] pyran rings: 1,3-chirality transfer by intramolecular oxypalladation reaction. Tetrahedron Asymmetry 2005, 16, 1299–1303. [Google Scholar] [CrossRef]
- Maddila, S.; Kerru, N.; Jonnalagadda, S.B. Recent progress in the multicomponent synthesis of pyran derivatives by sustainable catalysts under green conditions. Molecules 2022, 27, 6347. [Google Scholar] [CrossRef]
- Ahmad, I.; Jasim, S.A.; Yasin, G.; Al-Qargholi, B.; Hammid, A.T. Synthesis and characterization of new 1,4-dihydropyran derivatives by novel Ta-MOF nanostructures as reusable nanocatalyst with antimicrobial activity. Front. Chem. 2022, 10, 967111. [Google Scholar] [CrossRef] [PubMed]
- Wellington, A. Gas-phase Pyrolysis of 3,4-dihydro-2H-pyran. J Chem. Soc. A 1969, 2584–2587. [Google Scholar] [CrossRef]
- Caton, C.S. The thermal decomposition of 6-methyl-3,4-dihydro-2H-pyran. J. Am. Chem. Soc. 1969, 91, 7569–7572. [Google Scholar] [CrossRef]
- Frey, H.M.; Hopkins, R.G.; Isaac, N.S. The thermal unimolecular decomposition of 2-methoxy-3,4-dihydro-2H-pyran. J. Chem. Soc. Perkin Trans.2 1972, 73, 2082–2084. [Google Scholar] [CrossRef]
- Collins, J.F.; Frey, H.M.; Isaacs, N.S. The thermal decomposition of cis- and trans-2-methoxy-4-methyl-3,4-di hydro-2H-pyran. J. Chem. Soc. Perkin Trans. 2 1975, 2, 1–3. [Google Scholar] [CrossRef]
- Bailey, I.M.; Frey, H.M. Thermal unimolecular decomposition of 2-ethoxy-3,4-dihydro-2H-pyran. J. Chem. Soc. Perkin Trans. 2 1977, 870–872. [Google Scholar] [CrossRef]
- Frey, H.M.; Lodge, S.P. Thermal decomposition of 3,6-dihydro-2H-pyran. J. Chem. Soc. Perkin Trans. 2 1979, 1463–1464. [Google Scholar] [CrossRef]
- Frey, H.M.; Pottinger, R.; Carless, H.A.J.; Lingley, D.J. The thermal decomposition of cis-2,6-dimethyl-3,6-dihydro-2H-pyran. J. Chem. Soc. Perkin Trans. 2 1979, 1460–1462. [Google Scholar] [CrossRef]
- Frey, H.M.; Watts, H.P. The thermal decomposition of 4-methyl-3,6-dihydro-2H-pyran. Int. J. Chem. Kinet. 1981, 13, 729–733. [Google Scholar] [CrossRef]
- Taylor, R. The mechanism of thermal eliminations. Part 25. Arrhenius data for pyrolysis of isochroman-3-one, benzyl methyl ether, 2-hydroxyethylbenzene, phenyl acetate, and 3,4-dihydro-2H-pyran. J. Chem. Soc. Perkin Trans. 1988, 2, 183–189. [Google Scholar] [CrossRef]
- Saito, K.; Adachi, K.; Watanabe, M.; Shimofuji, K.; Imamura, A. The thermal unimolecular decomposition of 3,4-dihydro-2H-pyran behind reflected shock waves. Chem. Phys. Lett. 1990, 165, 383–385. [Google Scholar] [CrossRef]
- Alvarez-Aular, A.; Cartaya, L.; Maldonado, A.; Coll, D.S.; Chuchani, G. The kinetics and mechanism of the homogeneous, unimolecular gas-phase elimination of 2-(4-substituted-phenoxy)tetrahydro-2H-pyranes. J. Phys. Org. Chem. 2017, 31, e3778. [Google Scholar] [CrossRef]
- Adamo, C.; Barone, V. Toward reliable density functional methods without adjustable parameters: The PBE0 model. J. Chem. Phys. 1999, 110, 6158–6170. [Google Scholar] [CrossRef]
- Ditchfield, R.; Hehre, W.; Pople, J. Self-Consistent Molecular-Orbital Methods. IX. An Extended Gaussian-Type Basis for Molecular-Orbital Studies of Organic Molecules. J. Chem. Phys. 1971, 54, 724–728. [Google Scholar]
- Frisch, M.J.; Trucks, G.W.; Schlegel, H.B.; Scuseria, G.E.; Robb, M.A.; Cheeseman, J.R.; Scalmani, G.; Barone, V.; Mennucci, B.; Petersson, G.A.; et al. Gaussian 09, Revision B.01; Gaussian, Inc.: Wallingford, CT, USA, 2010. [Google Scholar]
- Fukui, K. A formulation of the reaction coordinate. J. Phys. Chem. 1970, 74, 4161–4163. [Google Scholar] [CrossRef]
- Merrick, J.P.; Moran, D.; Radom, L. An evaluation of harmonic vibrational frequency scale factors. J. Phys. Chem. A 2007, 111, 11683–11700. [Google Scholar] [CrossRef]
- McQuarrie, D.A.; Simon, J.D. Molecular Thermodynamics; University Science Books: Sausalito, CA, USA, 1999. [Google Scholar]
- Glasstone, K.J.; Laidler, K.J.; Eyring, H. The Theory of Rate Processes, 1st ed.; McGraw-Hill: New York, NY, USA, 1941. [Google Scholar]
- Benson, S.W. The Foundations of Chemical Kinetics; McGraw-Hill: New York, NY, USA, 1969. [Google Scholar]
- Reed, A.E.; Weinhold, F. Natural bond orbital analysis of near-Hartree–Fock water dimer. J. Chem. Phys. 1983, 78, 4066–4073. [Google Scholar] [CrossRef]
- Reed, A.E.; Curtiss, L.A.; Weinhold, F. Intermolecular interactions from a natural bond orbital, donor-acceptor viewpoint. Chem. Rev. 1988, 88, 899–926. [Google Scholar] [CrossRef]
- Glendening, E.D.; Reed, A.E.; Carpenter, J.E.; Weinhold, F. NBO, version 3.1; University of Wisconsin: Madison, WI, USA, 1988. [Google Scholar]
- Wiberg, K.B. Application of the pople-santry-segal CNDO method to the cyclopropylcarbinyl and cyclobutyl cation and to bicyclobutane. Tetrahedron 1968, 24, 1083–1096. [Google Scholar] [CrossRef]
- Moyano, A.; Pericas, M.; Valentí, E. A Theoretical study on the mechanism of the thermal and the acid-catalyzed decarboxylation of 2-oxetanones (β-Lactones). J. Org. Chem. 1989, 54, 573–582. [Google Scholar] [CrossRef]

| Compound | Temperature Range (°C) | Ea (kJ·mol−1) |
|---|---|---|
| 3,4-dihydro-2H-pyran | 316–389 | 219.4 |
| 3,4-dihydro-2H-pyran | 343–393 | 209.0 |
| 3,4-dihydro-2H-pyran | 990–1245 | 215.9 |
| 6-methyl-3,4-dihydro-2H-pyran | 330–370 | 214.2 |
| 2-methoxy-3,4-dihydro-2H-Pyran | 296–353 | 203.1 |
| 2-methoxy-4-methyl-3,4-dihydro-2H-pyran | 287–345 | 201.5 (trans) 196.0 (cis) |
| 2-ethoxy-3,4-dihydro-2H-pyran | 288–355 | 202.1 |
| 3,6-dihydro-2H-pyran (DHP) | 329–374 | 208.1 |
| cis-2,6-dimethyl-3,6-dihydro-2H-pyran (DMDHP) | 300–351 | 196.3 |
| 4-methyl-3,6-dihydro-2H-pyran (MDHP) | 311–361 | 209.5 |
| 2-methyl-3,4-dihydro-2H-pyran | 364–393 | 191.5 |
| Ea (kJ·mol−1) | ||||||
|---|---|---|---|---|---|---|
| Computational Method | Experimental | |||||
| Reaction | B3LYP | M062X | PBE0 | ωB97XD | MP2 | |
| DHP | 185.4 (10.9%) | 221.2 (6.3%) | 214.3 (2.9%) | 217.5 (4.6%) | 199.6 (4.0%)) | 208 |
| MDHP | 186.1 (11.4%) | 221.7 (5.6%) | 215.0 (2.4%) | 217.1 (3.4%) | 200.3 (4.6%) | 210 |
| DMDHP | 175.3 (10.6%) | 210.5 (7.4%) | 202.1 (3.1%) | 206.6 (5.4%) | 193.3 (1.4%) | 196 |
| Compound | ΔG≠ (kJ·mol−1) | ΔH≠ (kJ·mol−1) | ΔS≠ (J-mol−1·K−1) | Ea (kJ·mol−1) | Log A (s−1) |
|---|---|---|---|---|---|
| DHP | 196 | 208 | 20 | 214 | 14.59 |
| (208) | (14.31) | ||||
| MDHP | 190 | 209 | 33 | 215 | 15.24 |
| (210) | (14.62) | ||||
| DMDHP | 183 | 196 | 21 | 202 | 14.66 |
| (196) | (19.91) |
| Reaction | DHP | MDHP | DMDHP |
|---|---|---|---|
| qRC2 | −0.036 | −0.034 | 0.121 |
| qTSC2 | 0.103 | 0.103 | 0.260 |
| qRC4 | −0.196 | −0.017 | −0.186 |
| qTSC4 | −0.132 | 0.050 | −0.126 |
| O1-C2 | C2-C3 | C3-C4 | C4-C5 | C5-C6 | C6-O1 | |
|---|---|---|---|---|---|---|
| βiR | 0.918 | 1.013 | 1.034 | 1.910 | 1.034 | 0.930 |
| 0.921 | 1.012 | 1.015 | 1.846 | 1.033 | 0.929 | |
| 0.894 | 0.998 | 1.034 | 1.917 | 1.012 | 0.906 | |
| βiTS | 1.449 | 0.483 | 1.433 | 1.363 | 1.574 | 0.266 |
| 1.448 | 0.496 | 1.394 | 1.309 | 1.605 | 0.242 | |
| 1.399 | 0.490 | 1.409 | 1.393 | 1.493 | 0.266 | |
| βiP | 1.944 | 0 | 1.890 | 1.128 | 1.890 | 0 |
| 1.944 | 0 | 1.831 | 1.097 | 1.893 | 0 | |
| 1.879 | 0 | 1.885 | 1.131 | 1.827 | 0 | |
| %EV | 51.7 | 52.3 | 46.7 | 69.9 | 63.1 | 71.4 |
| 51.5 | 51.0 | 46.4 | 71.7 | 66.5 | 74.0 | |
| 51.3 | 50.9 | 44.1 | 66.6 | 59.1 | 70.6 | |
| δβav | 0.59 | Sy | 0.93 | |||
| 0.60 | 0.92 | |||||
| 0.57 | 0.94 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ruiz, P.; Bucheli, S.; Fernández, P.; Quijano, S.; Quijano, J.; Gaviria, J. Thermal Decomposition of Compounds Derived from 2H-Dihydropyran: A Computational Study. Chemistry 2024, 6, 1385-1395. https://doi.org/10.3390/chemistry6060082
Ruiz P, Bucheli S, Fernández P, Quijano S, Quijano J, Gaviria J. Thermal Decomposition of Compounds Derived from 2H-Dihydropyran: A Computational Study. Chemistry. 2024; 6(6):1385-1395. https://doi.org/10.3390/chemistry6060082
Chicago/Turabian StyleRuiz, Pablo, Sara Bucheli, Paula Fernández, Silvia Quijano, Jairo Quijano, and Jair Gaviria. 2024. "Thermal Decomposition of Compounds Derived from 2H-Dihydropyran: A Computational Study" Chemistry 6, no. 6: 1385-1395. https://doi.org/10.3390/chemistry6060082
APA StyleRuiz, P., Bucheli, S., Fernández, P., Quijano, S., Quijano, J., & Gaviria, J. (2024). Thermal Decomposition of Compounds Derived from 2H-Dihydropyran: A Computational Study. Chemistry, 6(6), 1385-1395. https://doi.org/10.3390/chemistry6060082








