Potential Fault Displacement Hazard Assessment Using Stochastic Source Models: A Retrospective Evaluation for the 1999 Hector Mine Earthquake
Abstract
:1. Introduction
- (i)
- The surface displacement equation is dependent on a faulting mechanism (i.e., normal, strike-slip, and reverse), and is based on different assumptions and approaches;
- (ii)
- There is large scatter of fault displacement data around the empirical equation;
- (iii)
- The prediction equation does not distinguish three translational components of the fault displacement (e.g., E-W, N-S, and U-D); and
- (iv)
- The method is mainly applicable to a single site, and no correlation of prediction errors of the fault displacement prediction equation has been characterized for applying it to multiple sites.
2. Methodology
2.1. Formulation
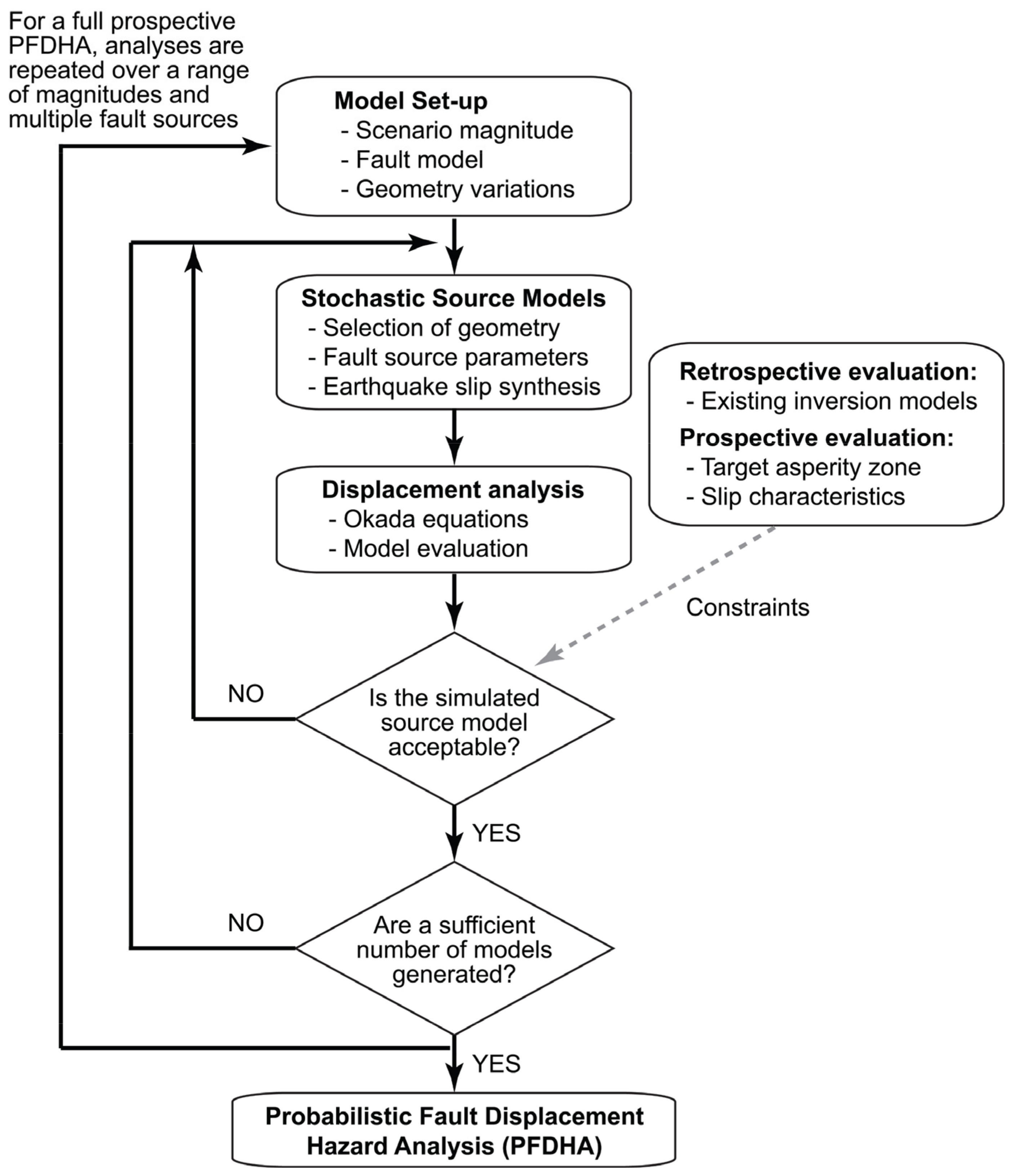
2.2. Stochastic Source Modelling and Fault Displacement Modelling
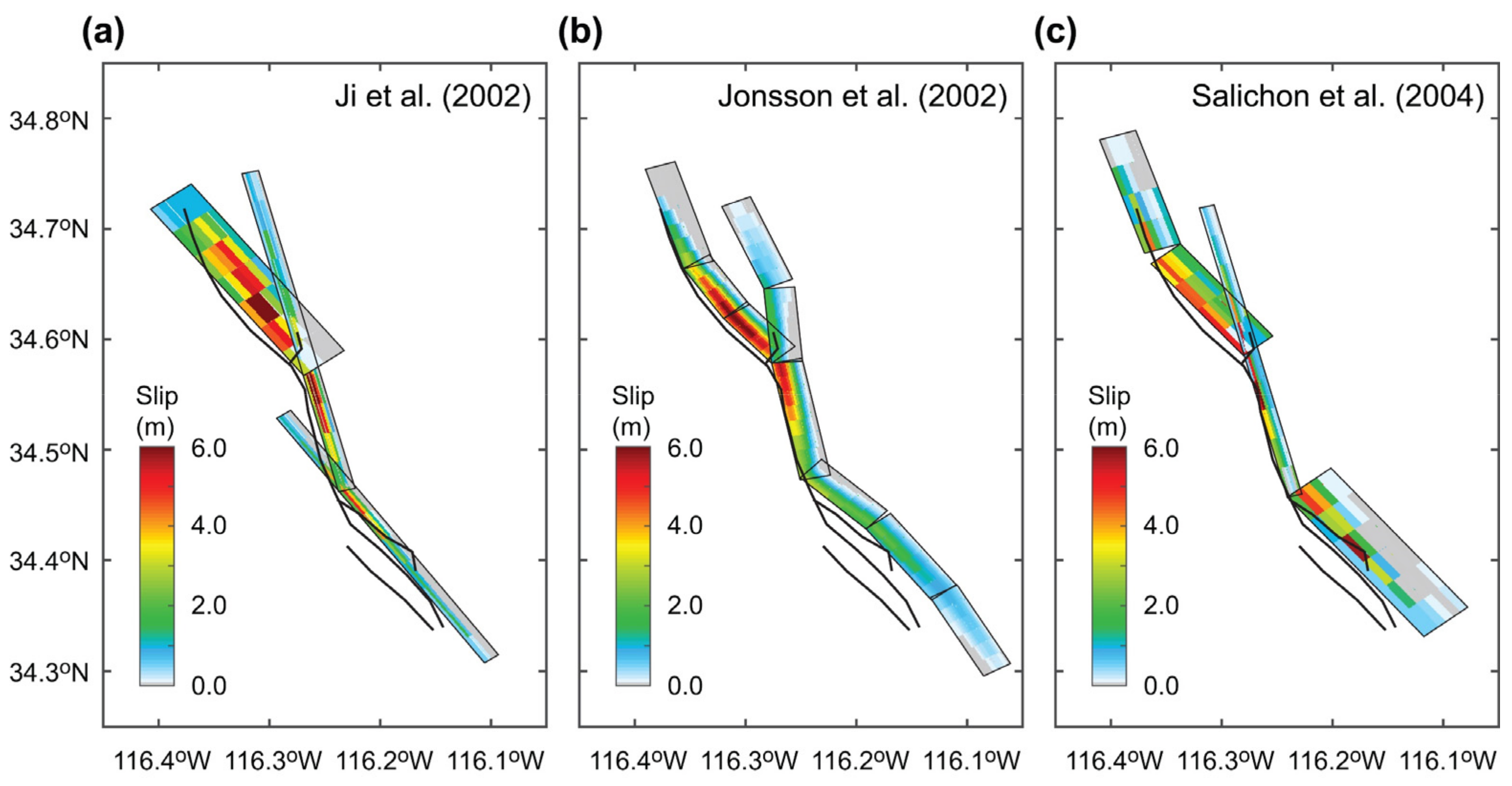
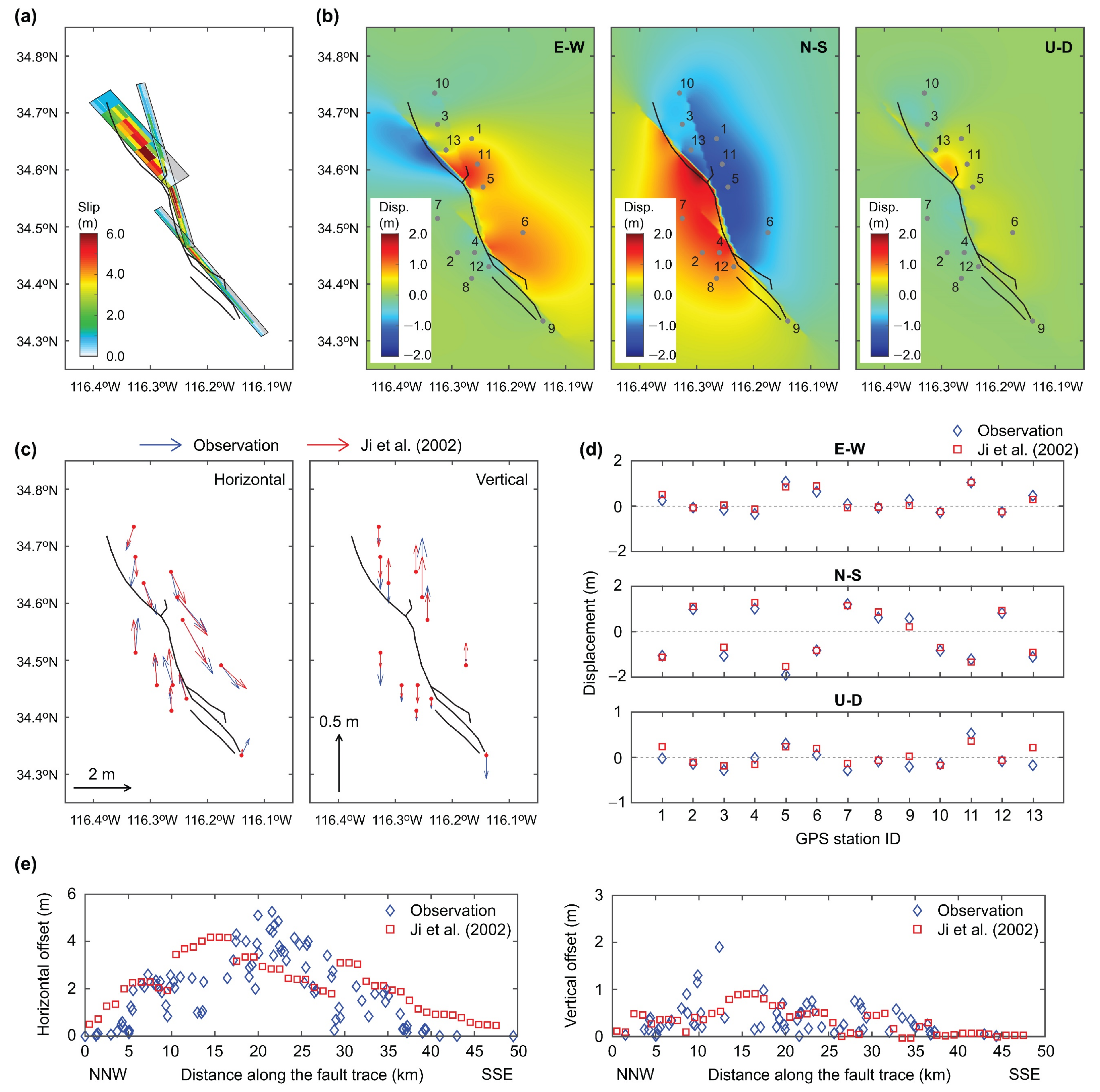
3. 1999 Hector Mine Earthquake
4. Retrospective Fault Displacement Hazard Analysis of the 1999 Hector Mine Earthquake
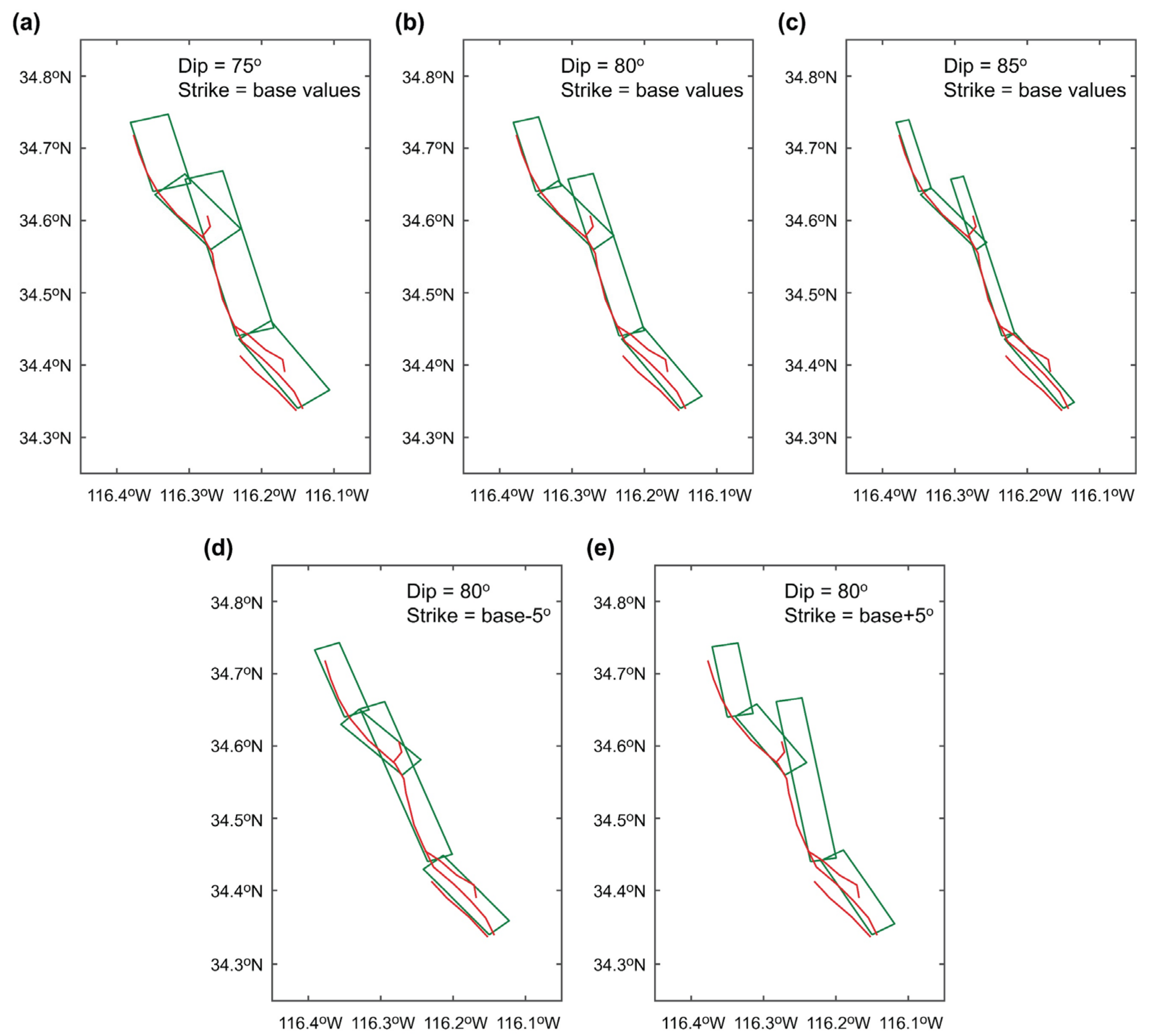
4.1. Model Set-Up
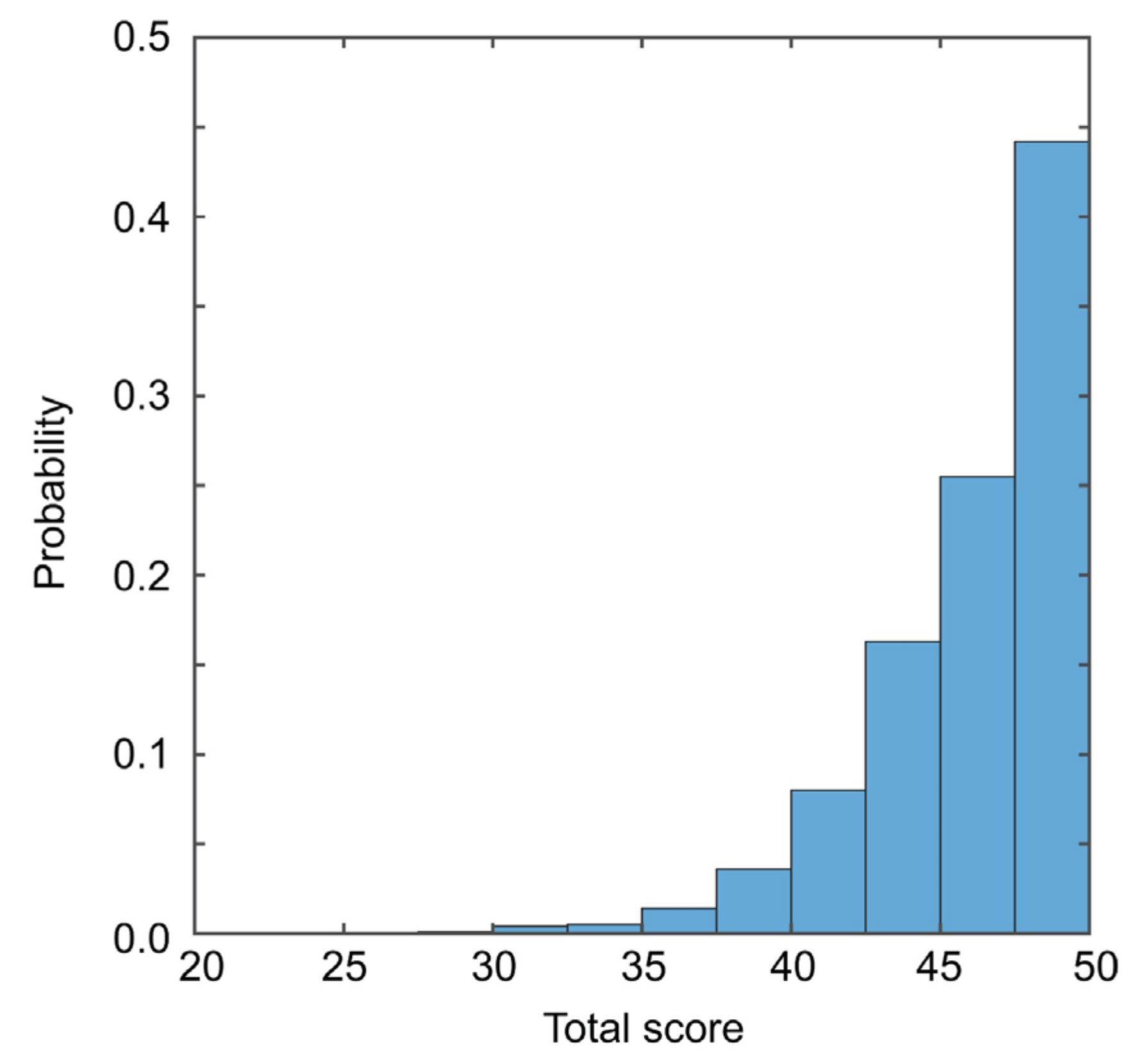
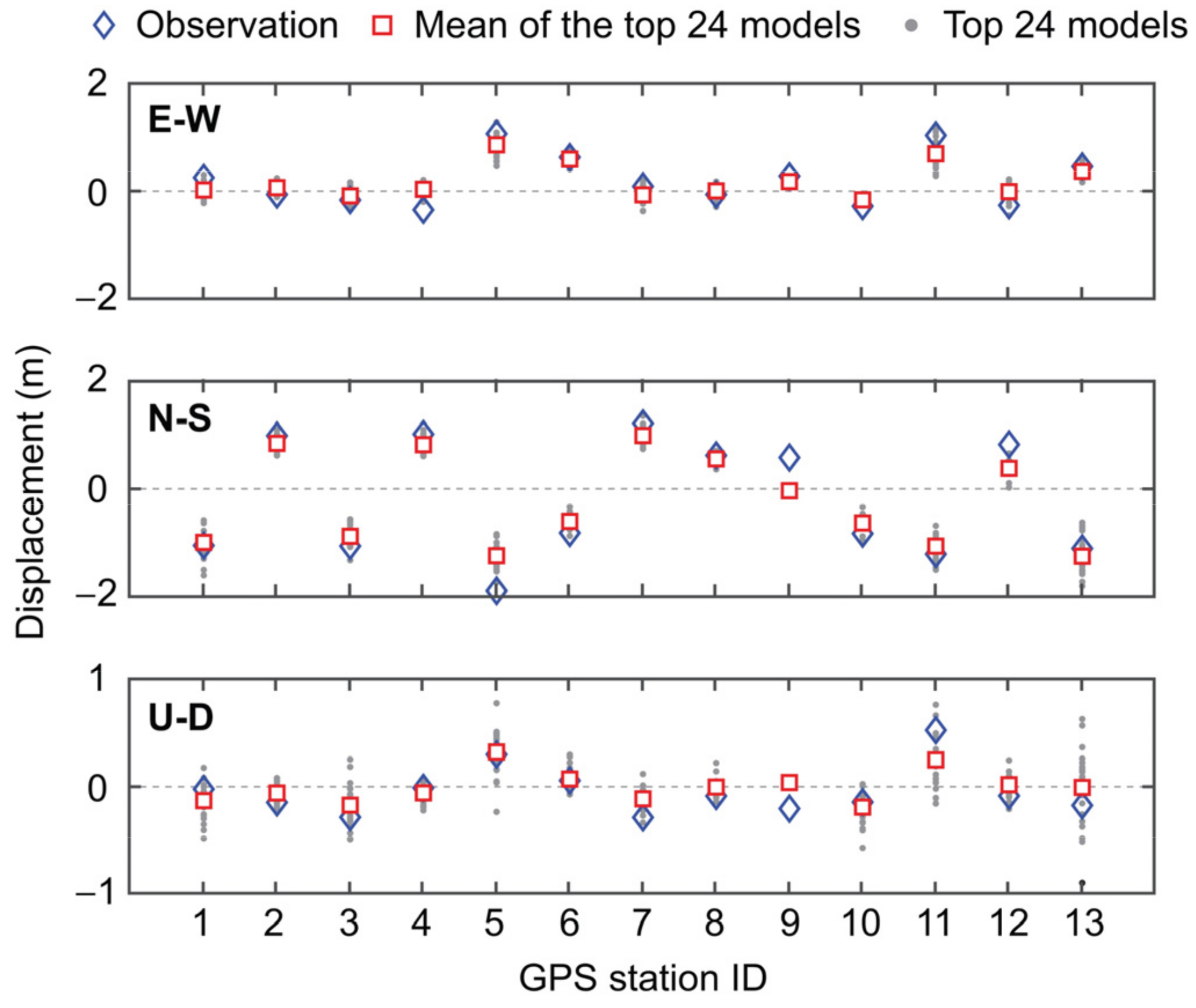
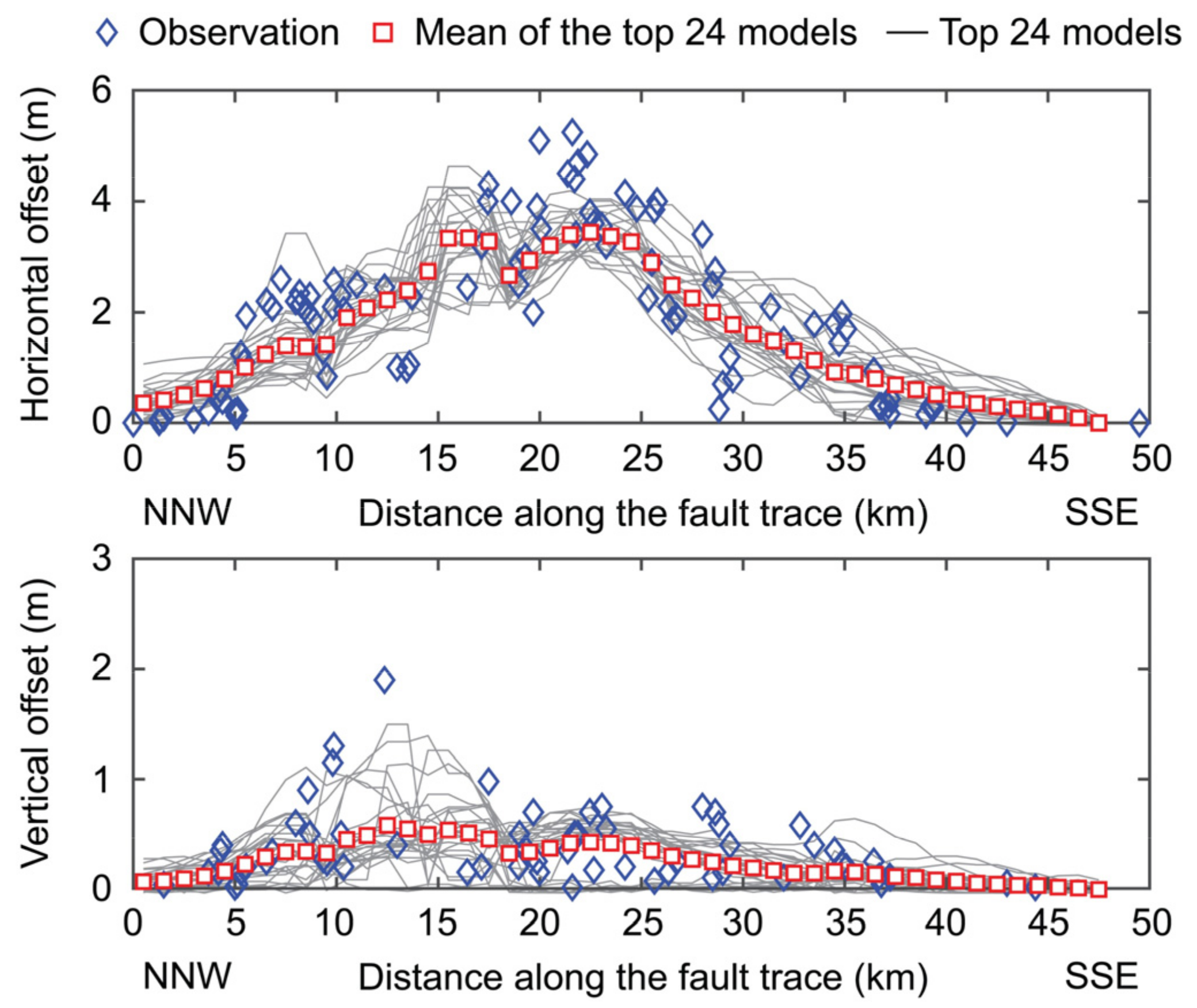
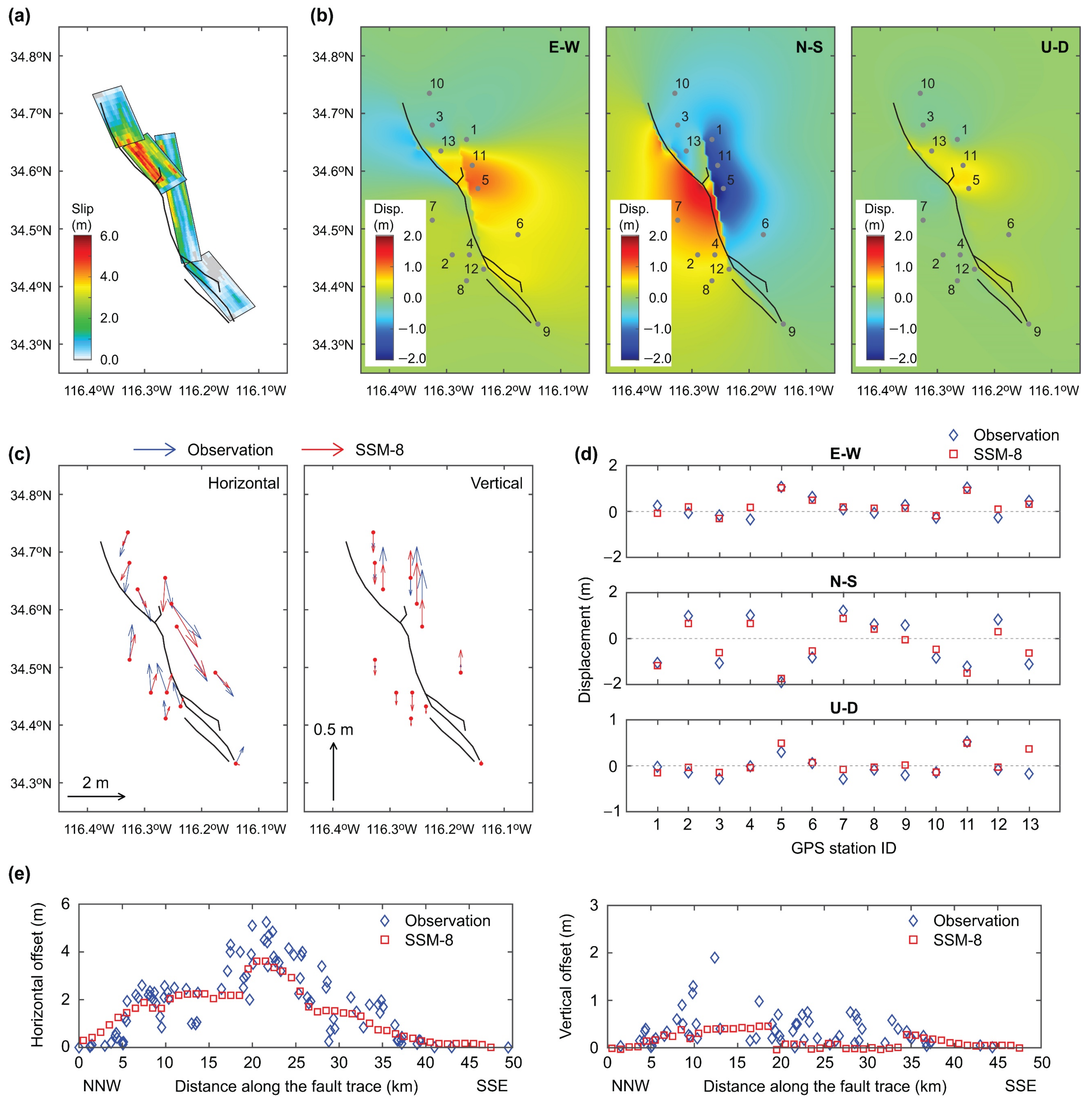
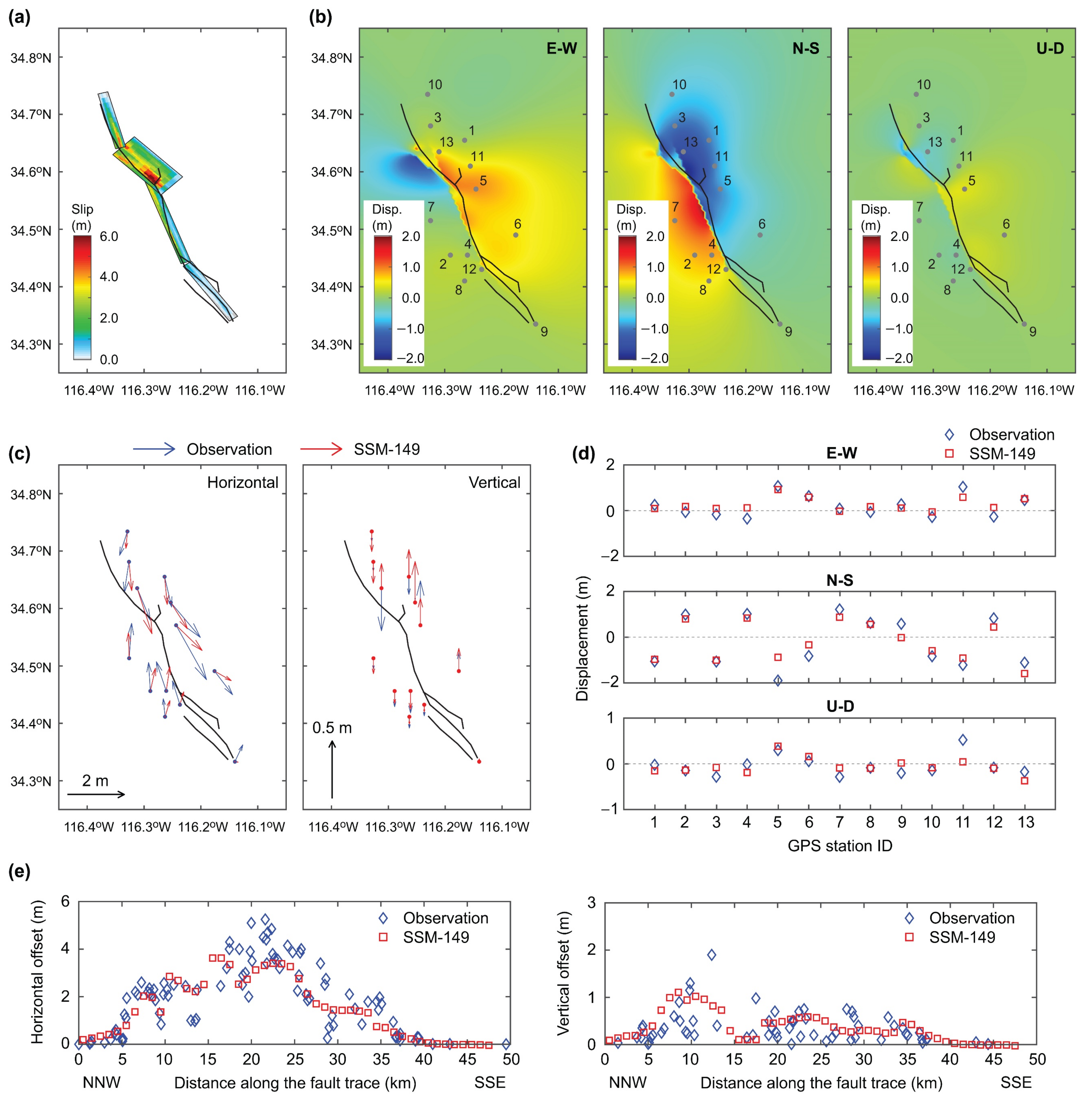
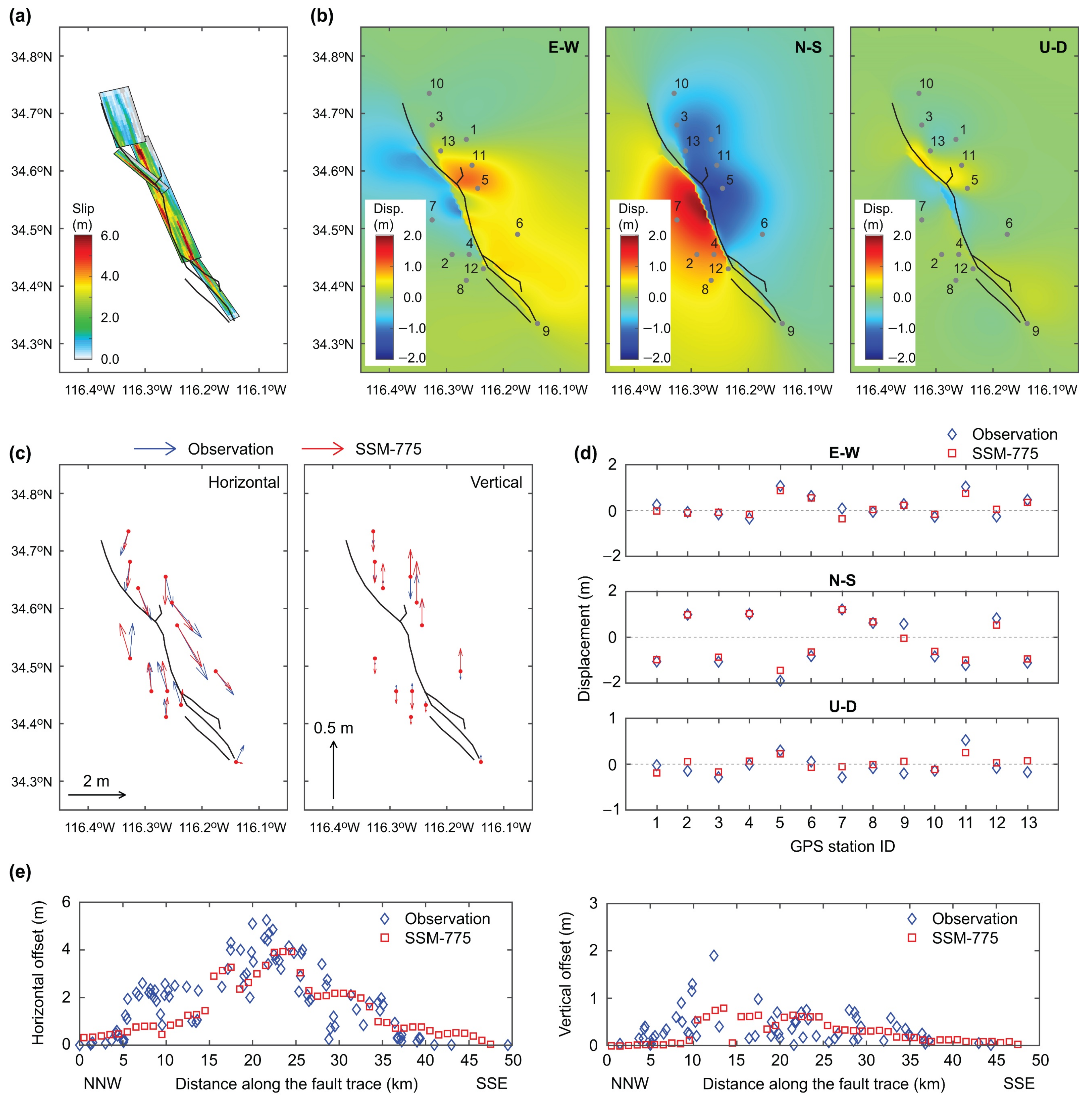
4.2. Results
4.3. Comparison with the Current Probabilistic Fault Displacement Hazard Analysis
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Conflicts of Interest
References
- Oettle, N.K.; Bray, J.D. Geotechnical Mitigation Strategies for Earthquake Surface Fault Rupture. J. Geotech. Geoenvironmental Eng. 2013, 139, 1864–1874. [Google Scholar] [CrossRef] [Green Version]
- Cheng, Y.; Akkar, S. Probabilistic permanent fault displacement hazard via Monte Carlo simulation and its consideration for the probabilistic risk assessment of buried continuous steel pipelines. Earthq. Eng. Struct. Dyn. 2016, 46, 605–620. [Google Scholar] [CrossRef]
- Katona, T.; Tóth, L.; Győri, E. Fault Displacement Hazard Analysis Based on Probabilistic Seismic Hazard Analysis for Specific Nuclear Sites. Appl. Sci. 2021, 11, 7162. [Google Scholar] [CrossRef]
- Valentini, A.; Fukushima, Y.; Contri, P.; Ono, M.; Sakai, T.; Thompson, S.C.; Viallet, E.; Annaka, T.; Chen, R.; Moss, R.E.S.; et al. Probabilistic Fault Displacement Hazard Assessment (PFDHA) for Nuclear Installations According to IAEA Safety Standards. Bull. Seism. Soc. Am. 2021, 111, 2661–2672. [Google Scholar] [CrossRef]
- Youngs, R.R.; Arabasz, W.J.; Anderson, R.E.; Ramelli, A.R.; Ake, J.P.; Slemmons, D.B.; McCalpin, J.; Doser, D.I.; Fridrich, C.J.; Swan, F.H.; et al. A Methodology for Probabilistic Fault Displacement Hazard Analysis (PFDHA). Earthq. Spectra 2003, 19, 191–219. [Google Scholar] [CrossRef]
- Petersen, M.D.; Dawson, T.E.; Chen, R.; Cao, T.; Wills, C.; Schwartz, D.P.; Frankel, A.D. Fault Displacement Hazard for Strike-Slip Faults. Bull. Seism. Soc. Am. 2011, 101, 805–825. [Google Scholar] [CrossRef] [Green Version]
- Moss, R.E.S.; Ross, Z.E. Probabilistic Fault Displacement Hazard Analysis for Reverse Faults. Bull. Seism. Soc. Am. 2011, 101, 1542–1553. [Google Scholar] [CrossRef]
- Takao, M.; Tsuchiyama, J.; Annaka, T.; Kurita, T. Application of Probabilistic Fault Displacement Hazard Analysis in Japan. J. Jpn. Assoc. Earthq. Eng. 2013, 13, 17–36. [Google Scholar] [CrossRef]
- Nurminen, F.; Boncio, P.; Visini, F.; Pace, B.; Valentini, A.; Baize, S.; Scotti, O. Probability of Occurrence and Displacement Regression of Distributed Surface Rupturing for Reverse Earthquakes. Front. Earth Sci. 2020, 8, 3. [Google Scholar] [CrossRef]
- Wells, D.L.; Kulkarni, V.S. Probabilistic and deterministic fault displacement hazard analysis—Sensitivity analyses and recom-mended practices for developing design fault displacements. In Proceedings of the 10th National Conference in Earthquake Engineering, Anchorage, AK, USA, 21–25 July 2014. [Google Scholar]
- Wesnousky, S.G. Displacement and Geometrical Characteristics of Earthquake Surface Ruptures: Issues and Implications for Seismic-Hazard Analysis and the Process of Earthquake Rupture. Bull. Seism. Soc. Am. 2008, 98, 1609–1632. [Google Scholar] [CrossRef]
- Baize, S.; Nurminen, F.; Sarmiento, A.; Dawson, T.; Takao, M.; Scotti, O.; Azuma, T.; Boncio, P.; Champenois, J.; Cinti, F.R.; et al. A Worldwide and Unified Database of Surface Ruptures (SURE) for Fault Displacement Hazard Analyses. Seism. Res. Lett. 2019, 91, 499–520. [Google Scholar] [CrossRef] [Green Version]
- Sarmiento, A.; Madugo, D.; Bozorgnia, Y.; Shen, A.; Mazzoni, S.; Lavrentiadis, G.; Dawson, T.; Madugo, C.; Kottke, A.; Thompson, S.; et al. Fault Displacement Hazard Initiative Database; Report GIRS-2021-08; University of California: Los Angeles, CA, USA, 2021. [Google Scholar] [CrossRef]
- Goda, K. Probabilistic characterization of seismic ground deformation due to tectonic fault movements. Soil Dyn. Earthq. Eng. 2017, 100, 316–329. [Google Scholar] [CrossRef] [Green Version]
- Goda, K.; Yasuda, T.; Mori, N.; Maruyama, T. New Scaling Relationships of Earthquake Source Parameters for Stochastic Tsunami Simulation. Coast. Eng. J. 2016, 58, 1650010–1650011. [Google Scholar] [CrossRef] [Green Version]
- Mai, P.M.; Beroza, G.C. A spatial random field model to characterize complexity in earthquake slip. J. Geophys. Res. Space Phys. 2002, 107, ESE 10-11. [Google Scholar] [CrossRef]
- Goda, K.; Mai, P.M.; Yasuda, T.; Mori, N. Sensitivity of tsunami wave profiles and inundation simulations to earthquake slip and fault geometry for the 2011 Tohoku earthquake. Earth, Planets Space 2014, 66, 105. [Google Scholar] [CrossRef] [Green Version]
- Okada, Y. Surface deformation due to shear and tensile faults in a half-space. Bull. Seism. Soc. Am. 1985, 75, 1135–1154. [Google Scholar] [CrossRef]
- Field, E.H.; Arrowsmith, R.J.; Biasi, G.P.; Bird, P.; Dawson, T.E.; Felzer, K.R.; Jackson, D.D.; Johnson, K.M.; Jordan, T.H.; Madden, C.; et al. Uniform California Earthquake Rupture Forecast, Version 3 (UCERF3)—The Time-Independent Model. Bull. Seism. Soc. Am. 2014, 104, 1122–1180. [Google Scholar] [CrossRef]
- Scientists of the U.S. Geological Survey, Southern California Earthquake Center, and California Division of Mines and Geology y (USGS, SCEC, and CDMG) Preliminary report on the 10/16/1999 M 7.1 Hector Mine, California earthquake. Seismol. Res. Lett. 2000, 71, 11–23. [Google Scholar]
- Agnew, D.C. Coseismic Displacements from the Hector Mine, California, Earthquake: Results from Survey-Mode Global Positioning System Measurements. Bull. Seism. Soc. Am. 2002, 92, 1355–1364. [Google Scholar] [CrossRef]
- Treiman, J.A. Primary Surface Rupture Associated with the Mw 7.1 16 October 1999 Hector Mine Earthquake, San Bernardino County, California. Bull. Seism. Soc. Am. 2002, 92, 1171–1191. [Google Scholar] [CrossRef]
- Ji, C. Source Description of the 1999 Hector Mine, California, Earthquake, Part II: Complexity of Slip History. Bull. Seism. Soc. Am. 2002, 92, 1208–1226. [Google Scholar] [CrossRef]
- Jonsson, S. Fault Slip Distribution of the 1999 Mw 7.1 Hector Mine, California, Earthquake, Estimated from Satellite Radar and GPS Measurements. Bull. Seism. Soc. Am. 2002, 92, 1377–1389. [Google Scholar] [CrossRef]
- Salichon, J. Slip History of the 16 October 1999 Mw 7.1 Hector Mine Earthquake (California) from the Inversion of InSAR, GPS, and Teleseismic Data. Bull. Seism. Soc. Am. 2004, 94, 2015–2027. [Google Scholar] [CrossRef]
- Mai, P.M.; Thingbaijam, K.K.S. SRCMOD: An Online Database of Finite-Fault Rupture Models. Seism. Res. Lett. 2014, 85, 1348–1357. [Google Scholar] [CrossRef]


| Earthquake Source Parameter | Equation |
|---|---|
| Length L (km) | |
| Width W (km) | |
| Mean slip Da (m) | |
| Maximum slip Dm (m) | |
| Box-Cox parameter λBC | Normal variable with mean equal to 0.312 and standard deviation equal to 0.278 |
| Along-strike correlation length CLL (km) | |
| Along-dip correlation length CLW (km) | |
| Hurst number H | Random variable that takes a value of 0.99 with probability of 0.43 or a value sampled from the normal distribution with mean equal to 0.714 and standard deviation equal to 0.172 with probability of 0.57 |
| 1 |
| Segment | Upper Left Corner [Latitude, Longitude, Top Depth] | [Length, Width] | [Strike, Dip, Rake] |
|---|---|---|---|
| Segment 1 | [34.340°, −116.150°, 0.0 km] | [13 km, 19 km] | [325°, 80°, 175°] |
| Segment 2 | [34.440°, −116.235°, 0.0 km] | [25 km, 19 km] | [345°, 80°, 175°] |
| Segment 3 | [34.560°, −116.270°, 0.0 km] | [11 km, 19 km] | [320°, 80°, 175°] |
| Segment 4 | [34.640°, −116.350°, 0.0 km] | [11 km, 19 km] | [345°, 80°, 175°] |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Goda, K. Potential Fault Displacement Hazard Assessment Using Stochastic Source Models: A Retrospective Evaluation for the 1999 Hector Mine Earthquake. GeoHazards 2021, 2, 398-414. https://doi.org/10.3390/geohazards2040022
Goda K. Potential Fault Displacement Hazard Assessment Using Stochastic Source Models: A Retrospective Evaluation for the 1999 Hector Mine Earthquake. GeoHazards. 2021; 2(4):398-414. https://doi.org/10.3390/geohazards2040022
Chicago/Turabian StyleGoda, Katsuichiro. 2021. "Potential Fault Displacement Hazard Assessment Using Stochastic Source Models: A Retrospective Evaluation for the 1999 Hector Mine Earthquake" GeoHazards 2, no. 4: 398-414. https://doi.org/10.3390/geohazards2040022
APA StyleGoda, K. (2021). Potential Fault Displacement Hazard Assessment Using Stochastic Source Models: A Retrospective Evaluation for the 1999 Hector Mine Earthquake. GeoHazards, 2(4), 398-414. https://doi.org/10.3390/geohazards2040022






