Nuclear Laboratory Setup for Measuring the Soil Water Content in Engineering Physics Teaching Laboratories

Abstract

Soil water content (θ) is a crucial soil parameter that is determined in many studies involving engineering, geology, and soil and environmental sciences. For instance, evaluating the soil strength, groundwater recharge, hydraulic conductivity, and soil aeration status depends on θ. The measurement of θ is fundamental for monitoring and controlling several soil processes. The gamma-ray attenuation (GRA) technique is a fast and non-destructive way of evaluating θ in soils with very contrasting compositions. Although, GRA is rarely explored in lab physics classes. The proposal of an experiment using a teaching GRA apparatus for measuring θ is presented. The experimental setup consisted of a ¹³⁷Cs radioactive source, a Geiger-Müller detector, and a radiation counter. Soil samples with four distinct granulometric compositions were analyzed. Strong linear correlations were found between the transmitted gamma-ray photon intensity and θ (correlation coefficients varying from −0.95 to −0.98). The soil porosity, measured by the conventional and GRA methods, presented differences that varied from c. 7.8% to c. 18.2%. In addition, strong linear relationships (correlation coefficients from 0.90 to 0.98) were observed between the GRA and the traditional (gravimetric) method of θ measurement. It was verified that the teaching GRA apparatus is useful for measuring θ. In addition, the apparatus allows the introduction of some important aspects related to the study of modern physics for undergraduate students of many fields of knowledge.

1. Introduction

Water is an essential element for life. For plants, it is stored and retained by capillarity in the pores of the soil skeleton [1]. The soil water content (θ), which can be expressed in terms of mass or volume percentages, is a measure that often is related to the maintenance and establishment of irrigated crops. Rational water usage in agriculture is an issue of great relevance since it consumes 70% of all the freshwater used worldwide [2]. Unfortunately, half of this consumed water is wasted due to inappropriate usage in different agricultural practices developed all around the world. In addition, to be absorbed, the water present in the soil must be available to the plant [3].

Soil is considered saturated when its entire pore space is filled with water [4]. In opposition, the soil is dry when all its pore space is filled with air. Between these two extremes, the soil is said to be moist. According to the existing literature, there are different methodologies for determining θ [1]. The standard (traditional) method is based on the determination of the mass or volume of water present in the sample at the time it is analyzed [5]. Other methods for measuring θ are based on the interaction of photons or particles with the sample. Among them, there are methods based on the moderation of fast neutrons or the attenuation of gamma radiation [6]. Soil water content analysis can be accomplished by investigating laboratory samples or under field conditions, depending on the study carried out.

In undergraduate courses in applied physics to engineering, the concept of soil water content is fundamental for determining the physical-hydric behavior of the soil [7,8]. Additionally, θ determination is fundamental for the evaluation of the soil water retention curve and hydraulic conductivity [9,10,11]. Equipment based on the interaction of radiation with the matter for measuring θ is rarely available to engineering undergraduate students. In general, these types of equipment are found in institutions that develop research related to applied nuclear techniques and are restricted to graduate research activities [12,13,14,15,16].

This study presents the use of a simplified gamma-ray attenuation (GRA) system, developed for teaching purposes, as an alternative to the traditional GRA systems usually employed in soil properties analyses [6,10,12,13,14,15,16]. The simplified system consists of a Geiger-Müller detector, low-activity radioactive sources, and a photon counting system. To the best of our knowledge, there are no articles exploring GRA systems for θ measurements aimed at undergraduate engineering students. Thus, our study proposes a procedure for monitoring θ in soil samples under different moisture conditions using a simplified GRA system. Moreover, the suggested approach presents a detailed description of the theoretical aspects of the measurements, which can guide professors in conducting teaching activities involving this knowledge.

The results obtained and presented here indicate that the proposed approach is quite adequate in determining the θ of soil samples of contrasting textures (granulometries). However, this study is not intended to replace the existing traditional GRA systems. Our approach just consists of a good alternative for teaching activities, in which the concepts of soil water content, soil pore space, radiation interaction with matter, and radiation production and detection can be satisfactorily explored.

2. Basic Theory

Beer-Lambert’s law is used for describing the gamma-ray attenuation by any material, including soil samples. Soil is a three-phase system composed of solids (minerals and organic materials), solutes (water), and gases (air) (Figure 1a). For a collimated beam of gamma-rays interacting with the soil, Beer-Lambert’s law is written as follows [13]:

$$\mathrm{I}={\mathrm{I}}_0 {\mathrm{e}}^{-({\mathsf{\mu }}_{\mathrm{p}}{\mathsf{\rho }}_{\mathrm{p}}{\mathrm{x}}_{\mathrm{p}}+{\mathsf{\mu }}_{\mathrm{w}}{\mathsf{\rho }}_{\mathrm{w}}{\mathrm{x}}_{\mathrm{w}}+{\mathsf{\mu }}_{\mathrm{a}}{\mathsf{\rho }}_{\mathrm{a}}{\mathrm{x}}_{\mathrm{a}}),}$$

(1)

where I₀ (cps: counts per second) is the intensity of the incident beam, I (cps) is the beam intensity transmitted through the sample (Figure 1b), μ (cm² g⁻¹) is the mass attenuation coefficient, ρ (g cm⁻³) is the sample density, and x (cm) is the sample thickness. The subscripts p, w, and a refer to particles, water, and air, respectively.

Frequently, the interaction of the gamma-rays with the air is usually ignored as the air density and its mass attenuation coefficient are much smaller than the density and mass attenuation coefficient of the solids and water. Thus, Equation (1) can be simplified to:

$$\mathrm{I}={\mathrm{I}}_0 {\mathrm{e}}^{-({\mathsf{\mu }}_{\mathrm{p}}{\mathsf{\rho }}_{\mathrm{p}}{\mathrm{x}}_{\mathrm{p}}+{\mathsf{\mu }}_{\mathrm{w}}{\mathsf{\rho }}_{\mathrm{w}}{\mathrm{x}}_{\mathrm{w}})}.$$

(2)

As it is known, the soil water content can be measured based on its gravimetric (G: g g⁻¹) or volumetric (θ: cm³ cm⁻³) content [5]. The gravimetric water content represents the water mass (m_w: g) per unit of dry soil mass (m_s: g) (Equation (3)), while the volumetric water content is given by the water volume (V_w: cm³) per unit of soil volume (V_s: cm³) (Equation (4)), usually expressed as a percentage [4]:

$$\mathrm{G}=\frac{{\mathrm{m}}_{\mathrm{w}}}{{\mathrm{m}}_{\mathrm{s}}},$$

(3)

$$\mathsf{\theta }=\frac{{\mathrm{V}}_{\mathrm{w}}}{{\mathrm{V}}_{\mathrm{s}}}=\frac{{\mathsf{\rho }}_{\mathrm{s}}}{{\mathsf{\rho }}_{\mathrm{w}}}\mathrm{G},$$

(4)

where ρ_s (g cm⁻³) is the soil bulk density.

Based on the concepts of soil bulk and particle densities [1], Equation (2) becomes:

$$\mathrm{I}={\mathrm{I}}_0 {\mathrm{e}}^{-\mathrm{x}({\mathsf{\mu }}_{\mathrm{p}}{\mathsf{\rho }}_{\mathrm{s}}+{\mathsf{\mu }}_{\mathrm{w}}\mathsf{\theta }{\mathsf{\rho }}_{\mathrm{w}})}.$$

(5)

Equation (5) is utilized for measuring, for example, the bulk density when the soil is dry (θ = 0 cm³ cm⁻³) (Figure 1a—left):

$${\mathsf{\rho }}_{\mathrm{s}}=\frac{1}{\mathrm{x}{\mathsf{\mu }}_{\mathrm{p}}}\mathrm{l}\mathrm{n}\left(\frac{{\mathrm{I}}_0}{\mathrm{I}}\right).$$

(6)

If the soil is moist (Figure 1a—center and right), Equation (5) can also be employed for monitoring the sample water content:

$$\mathsf{\theta }=\frac{1}{\mathrm{x}{\mathsf{\mu }}_{\mathrm{w}}{\mathsf{\rho }}_{\mathrm{w}}}\left[\mathrm{l}\mathrm{n}\left(\frac{{\mathrm{I}}_0}{\mathrm{I}}\right)-\mathrm{x}{\mathsf{\mu }}_{\mathrm{p}}{\mathsf{\rho }}_{\mathrm{s}}\right].$$

(7)

3. Materials and Methods

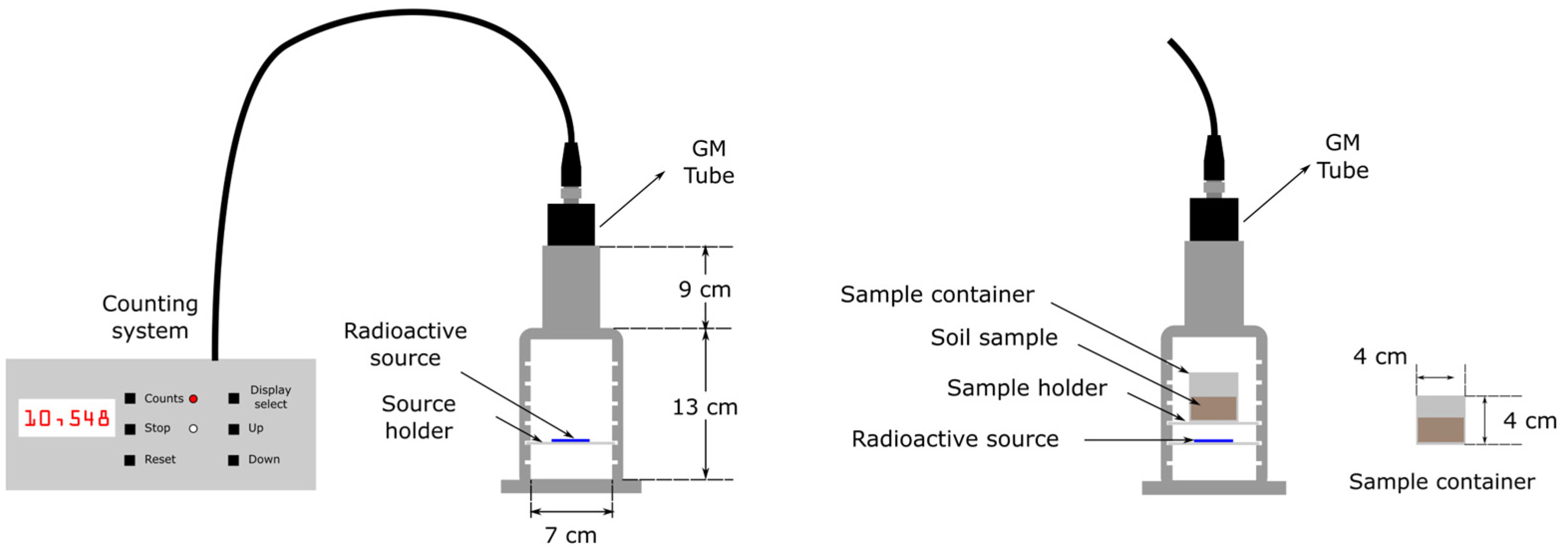
A PASCO kit [17] was used to determine the water content of the investigated soil samples (Figure 2). The setup consists of a ¹³⁷Cs radioactive source (reference number SN-7972A), a Geiger-Müller (GM) detector (reference number SN-7970-A), a radiation counter (high voltage source (0 to 1200 V) + timer + counter—reference number SN-7907), a soil sample, and source holders (sample + GM + radioactive source) (see Supplementary Figure S1). The Caesium-137 source of the kit is a sealed plastic pellet (2.5 cm diameter), whose activity is c. 3.4 μCi. This radioactive source emits gamma-ray photons with an energy of c. 0.662 MeV. Its half-life is around 30.2 years. According to the manufacturer’s specifications, the radioactive source has a stated uncertainty of ±15%. It is worth mentioning that these radioactive sources are USNRC License Exempt (US) according to the kit manufacturer. In the proposed experiment, three ¹³⁷Cs sources were pilled (set one above the other) to increase the flux of photons transmitted through the samples. Thus, the total activity of the source assembly was c. 10.2 μCi [18].

The GM detector used in the experiment has a 35-mm diameter window made of mica (2 mg cm⁻²) and is designed to enable good counting efficiency for low-activity radioactive sources. The dead time of the tube is around 200 μs. The operating voltage selected to supply the GM was 920 V. A previous experiment was performed to determine the counting plateau of the GM detector, which varied from 760 to 980 V. For the experiment, the radioactive sources and soil samples were placed inside the holder, and since it has slots spaced 1.0 cm apart, the teaching instructor can vary the source and sample positions quickly and easily (Figure 2 and see Supplementary Figure S1).

Due to radiological protection concerns, the classroom instructor is allowed to handle the radioactive sources. However, it is an extra concern, as in this kind of system, the radioactive sources have very low activity. Even to increase this protection, lead plates with a thickness of c. 3 mm can be placed around the radioactive source during the measurements. In these experimental activities, the undergraduate students, placed at a safe distance from the experimental setup (radioactive source + GM detector + sample), only operate the electronics responsible for counting the incident and transmitted gamma-ray photons reaching the GM detector. Additionally, the radioactive source is sealed and has a small window that permits the upward emission of photons perpendicularly to the students’ position.

The counts were registered in a radiation counter system (PASCO—Spectech ST-360 Counter) composed of a timer, preset counter, digital ratemeter, computer interface, and battery power for field applications. The interval of time selected for each measurement was 10³ s (c. 17 min) (Silt Loam and Heavy Clay soils) and 5 × 10² s (c. 8 min) (Sandy Clay Loam and Clay soils). For these time intervals, the obtained uncertainty in the measurements (total counts recorded) was around 1% or less. This time interval was also selected to permit the experimental measurements in two to three classes of 50 min each.

Soil samples with four contrasting particle size fractions, identified as Silt Loam—SiLo, Heavy Clay—HeCa, Sandy Clay Loam—SaCLo, and Clay—Cla, were selected for the experimental measurements (Figure 3). Approximately 100 g of each soil was sieved at 2 mm (10 Mesh). This procedure was performed to produce as much as possible homogeneous soil samples. Before sieving, the soil samples were oven-dried (air-forced circulation) for 24 h at 105 °C.

The soil samples were then placed in cylindrical plastic containers with diameters of 3.25 cm and volumes of c. 35 cm³ (see Supplementary Table S1). Each soil was carefully placed inside the containers for the experiment. To standardize the samples, the sample containers were filled until the sample thickness (soil depth) crossed by the gamma-ray photons from the radioactive source was approximately 2.65 to 2.70 cm (see Supplementary Table S1). This thickness was equivalent to a soil volume of approximately 25 cm³ inside each container. A pestle with the same container’s internal diameter was employed for leveling the soil inside its interior (see Supplementary Figure S1). After filling the container, the soil bulk density of the samples was determined by the gravimetric method (

Table 1. Soil bulk density (ρ_s), soil particle density (ρ_p), and volume of pores (V_por) of the contrasting soils studied.

Soil/Properties	ρs (g cm−3)	ρp (g cm−3)	Vpor (cm3 cm−3)
HeCa	1.08	2.63	14.77
Cla	1.20	2.16	11.13
SaCLo	1.10	2.22	12.62
SiLo	1.03	2.25	13.50

HeCa: Heavy Clay; Cla: Clay; SaCLo: Sandy Clay Loam; SiLo: Silt Loam. The soil bulk density was determined using Equation (8), and the volume of pores was determined using Equation (10).

) [1]:

$${\mathsf{\rho }}_{\mathrm{s}}=\frac{{\mathrm{m}}_{\mathrm{s}}}{{\mathrm{V}}_{\mathrm{s}}}.$$

(8)

The porosity (φ: cm³ cm⁻³) and volume of pores (V_por: cm³) of the soil samples were calculated using Equations (9) and (10), respectively. Afterwards, the samples were moistened by selecting five different θ (see Supplementary Table S2). Five different volumes of water were selected (V_por divided by five) to be added, little by little, to the samples. The last volume of water added saturated the sample (Table 1) [5].

$$\mathsf{\phi }=1-\frac{{\mathsf{\rho }}_{\mathrm{s}}}{{\mathsf{\rho }}_{\mathrm{p}}},$$

(9)

$${\mathrm{V}}_{\mathrm{p}\mathrm{o}\mathrm{r}}={\mathrm{V}}_{\mathrm{s}}\mathsf{\phi },$$

(10)

where the particle density (ρ_p: g cm⁻³) of the contrasting soils was determined by the gas pycnometer method (see Supplementary Table S1) [19]. In the absence of this measure, in general, the soil particle density is assumed as 2.65 g cm⁻³.

The soil sample (soil + container) under different moisture conditions was placed right above the radioactive source (sealed plastic pellet) to perform the experiments. The volume of water to be added to the soil was controlled using a pipette. The mass measurements were carried out with a precision balance (Gehara AG200, 10⁻⁴ g accuracy). The distance between the radioactive source and the detector was c. 6.5 cm. To prevent the detection of beta particles (energy of c. 0.512 MeV) from the ¹³⁷Cs radioactive source, a 0.050 mm thick aluminum plate (supplied by PASCO) was placed between the sample top and the detector window.

Before calculating the soil water content, we measured the photon intensity transmitted through the dry soil samples (I) and then the photons transmitted through the moisture soil samples (I_θ) (see Supplementary Table S3). Using Equation (11) the volumetric water content of the sample could be determined as follows:

$$\mathsf{\theta }=\frac{1}{\mathrm{x}{\mathsf{\mu }}_{\mathrm{w}}{\mathsf{\rho }}_{\mathrm{w}}}\mathrm{l}\mathrm{n}\left(\frac{\mathrm{I}}{{\mathrm{I}}_{\mathsf{\theta }}}\right).$$

(11)

The water density value utilized in the calculations was 0.998 g cm⁻³. According to the literature, the water mass attenuation coefficient for photons from ¹³⁷Cs is c. 0.0767 cm² g⁻¹ [20].

Figure 4 shows a flow chart containing the basic steps followed for the experimental θ measurements.

4. Results and Discussion

The graphs of the number of photons transmitted (I) as a function of the θ of the samples showed a linear behavior (R ranging from −0.95 to −0.98) for all the soil types studied (Figure 5). The higher count values observed for HeCa and SiLo were due to the longer counting time adopted for these particular soil samples.

The differences in the photon counts observed for the dry soil samples (θ = 0 cm³ cm⁻³) are associated with the differences in their compositions. For example, the soil’s chemical composition (mainly due to oxide content) and mineralogy will influence the soil properties, such as the particle density, which are directly related to the radiation attenuation (Equation (2)). Another parameter also affected by the soil’s chemical composition is the attenuation coefficient [21,22,23]. For low-energy photons (<100 keV), the attenuation coefficient is influenced mainly by the photoelectric effect, whose cross-section is proportional to Z⁴⁻⁵ and inversely proportional to the photon energy (E⁻³ when <500 keV) [24,25]. For the intermediate energy region c. 100 keV to c. 10 MeV), the incoherent scattering influences the photon interaction and, consequently, the attenuation coefficient, whose cross-section has a Z dependence [26,27]. For high-energy photons (E > 10 MeV), pair production is the most important process in the attenuation of the radiation associated with the Z² dependence of its cross-section. In our study, incoherent scattering was the main process influencing the photon (¹³⁷Cs) interaction and the attenuation coefficient. For example, Camargo et al. [26], working with tropical/subtropical soils with contrasting major oxide (SiO₂, Al₂O₃, Fe₂O₃, TiO₂) compositions, demonstrated that for the photon energy of ¹³⁷Cs, the total attenuation coefficient is completely influenced by the incoherent scattering (>99%). Furthermore, these authors mainly associated the dominance of each one of the partial effects (photoelectric effect, incoherent scattering, and pair production) with the Z dependence on the partial cross-sections, as discussed earlier.

The equations obtained when fitting the experimental data (Figure 5) make it possible to predict θ (interpolation) only by knowing the photons transmitted by the sample. However, two precautions for this interpolation are necessary: the sample water volume should not exceed the maximum volume necessary for the saturation of the sample, and for each soil type, a proper calibration equation must be determined. From the θ values obtained at saturation, we calculated the total porosity of the analyzed soils by the simplified GRA method using Equation (11) (

Table 2. Total porosity (φ) obtained by the traditional (TRA) and simplified gamma-ray attenuation (GRA) methods.

Soil/Methods	TRA	GRA
	φ (cm3 cm−3)
HeCa	0.591	0.515
Cla	0.445	0.377
SaCLo	0.505	0.413
SiLo	0.540	0.582

HeCa: Heavy Clay; Cla: Clay; SaCLo: Sandy Clay Loam; SiLo: Silt Loam. The traditional soil total porosity was determined through Equation (9), while the porosity based on the radiation attenuation was determined using Equation (11).

). We found total porosity deviations from c. 7.8% (SiLo) to c. 18.2% (SaCLo) when compared to the traditional method (Equation (9)).

The characteristics of each soil and the presence of entrapped air bubbles during the saturation process could affect the obtained results of the total porosity. Thus, assuming θ at saturation as the total porosity should be used cautiously. Another reason for the observed discrepancies is that the radioactive source’s experimental apparatus is not collimated and there is not a single-channel analyzer to select the photopeak region related to the Caesium-emitted photons. Therefore, both scattered and transmitted photons are detected by the GM detector [27,28]. This fact causes drawbacks in using Equation (11), which should have a correction parameter (buildup factor), which was not covered in our study [29,30] to maintain the analysis as simple as possible.

When we analyze the results of the total porosity (Table 2—traditional method) concerning the particles of the soils (Figure 3), we observe that, in general, higher total porosities are observed in soils with a larger clay content (HeCa). On the other hand, the predominance of sand particles (SaCLo) causes the total porosity to decrease. Soils with a higher sand content tend to be denser and, consequently, less porous (low porosities), in opposition to clayey soils [1,4]. Thus, our results seem to be consistent with what is expected. Finally, the silt content exerts an intermediate (between clay and sand) influence on this particular soil property.

The comparison of the θ values obtained by the traditional and simplified GRA methods shows that, except for HeCa (R = 0.90), there was an adequate agreement between the results (Figure 6). The best results were observed for Clay and Silt Loam soils, where the determinations were closer to the 1:1 line. Some reasons can explain the observed differences between the methods. One of them is the simplicity of the electronic system (i.e., no channel analyzer) used to detect the gamma-rays by the nuclear method, i.e., the PASCO kit is designed primarily for teaching purposes, which makes it simpler and more limited compared to those employed in applied nuclear physics research [18]. For example, scattered photons can be detected by the simplified GRA system; this is something that is minimized in systems in which the photon energy to be analyzed can be chosen. Furthermore, the detection and counting of scattered photons limit the use of Equation (11), as mentioned before. Finally, the type of detector utilized (GM) is based on the gas ionization mechanism, which has a lower efficiency in detecting gamma-ray photons of higher energies when compared, for instance, to solid scintillation detectors that are usually employed in research systems [31].

We have to emphasize that although numerous other techniques are used in θ monitoring, the technique based on gamma-ray attenuation is well established and employed to this day [12,32,33,34,35,36]. This nuclear method enables fast (for high-activity radioactive sources) and accurate measurements of θ [20]. Furthermore, this versatile method allows measurements under field and laboratory conditions [32,33]. Our study proposed applying the simplified nuclear system in laboratory measurements of θ, utilizing disturbed soil samples with small volumes as a didactic activity. These samples were idealized considering the limitations of the Intermediate Nuclear Laboratory System [17], such as the low activity of the radioactive source (radiological protection issue for teaching activities), the type and size of the detector used, and the dimensions of the sample holder. Nonetheless, our study’s core idea was to show that even the simplified GRA system gives satisfactory results and might be employed to explore concepts related to the technique itself (physical principles) and the principles of operation of the electronics and gamma-ray detector.

5. Conclusions

The present study showed the feasibility of a simplified gamma-ray attenuation system for measuring θ. In addition, determining θ at saturation by the Beer-Lambert law allowed us to evaluate the samples’ total porosity. The nuclear method proposed for θ monitoring had a different configuration and was very limited compared with the traditional methods based on radiation attenuation. As pointed out, we employed a non-collimated system, low-activity radioactive sources, a gas-filled (GM) tube detector, and basic electronics in the measurements. Thus, the differences between the conventional method of θ evaluation and the GRA method might be mainly explained by the limitations of the simplified nuclear system. However, despite these limitations, the described nuclear method permitted us to determine θ with relative accuracy and the total porosity of soil samples with contrasting textural fractions. The differences in the soil composition (grain sizes and chemical composition) explain the necessity for specific calibrations for estimating θ based on the nuclear methodology. Nonetheless, the calibration process can be considered as an initial didactic activity to show variations in the photon counts as a function of the changes in the soil composition. We also mentioned that the counting time needs to be improved to increase the method sensitivity intrinsically related to the GRA method. As future improvements, we suggest using radioactive sources emitting less energetic gamma-ray photons (e.g., ¹³³Ba or ²⁴¹Am) and collimating the photon beam before it reaches the GM tube. Overall, our findings demonstrated that the simplified nuclear system and described methodology might be an exciting tool for teaching physical concepts associated with gamma-ray attenuation, nuclear electronics, radiation detection, and the investigation of some selected soil properties to undergraduate students.