From Spherical Harmonics to Gaussian Beampatterns
Abstract
1. Introduction
2. Theory
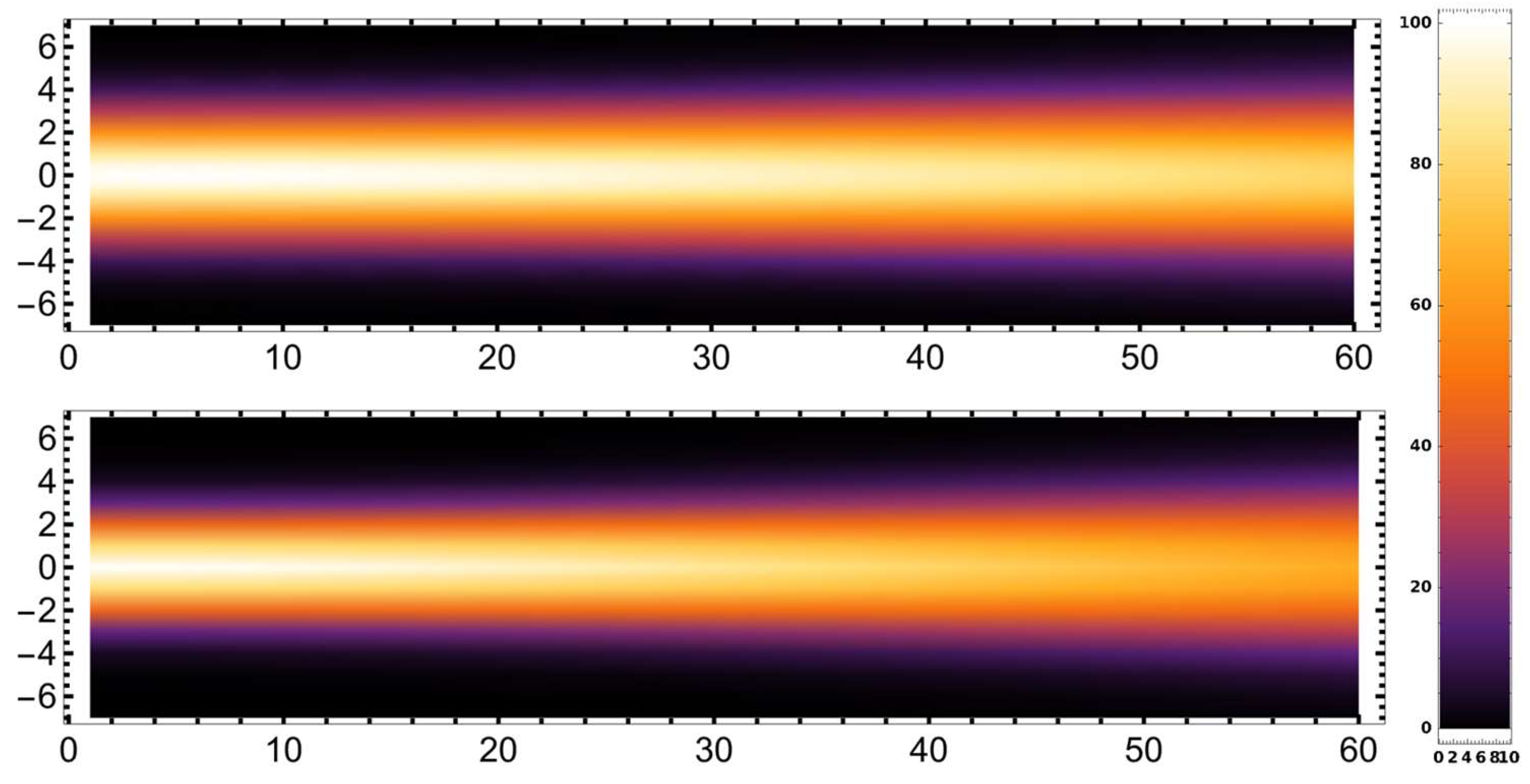
3. Results
4. Discussion and Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Goldsmith, P.F. Gaussian beam propagation. In Quasioptical Systems: Gaussian Beam Quasioptical Propogation and Applications; IEEE: New York, NY, USA, 1998; pp. 9–38. [Google Scholar]
- Bracewell, R.N. The Fourier Transform and Its Applications, 1st ed.; McGraw-Hill: New York, NY, USA, 1965. [Google Scholar]
- Papoulis, A. The Fourier Integral and Its Applications; McGraw-Hill: New York, NY, USA, 1987. [Google Scholar]
- Du, G.; Breazeale, M.A. The ultrasonic field of a gaussian transducer. J. Acoust. Soc. Am. 1985, 78, 2083–2086. [Google Scholar] [CrossRef]
- Huang, D.; Breazeale, M.A. An ultrasonic Gaussian transducer and its diffraction field: Theory and practice. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 2006, 53, 1018–1027. [Google Scholar] [CrossRef] [PubMed]
- Moore, N.J.; Alonso, M.A. Closed form formula for Mie scattering of nonparaxial analogues of Gaussian beams. Opt. Express 2008, 16, 5926–5933. [Google Scholar] [CrossRef] [PubMed]
- Moore, N.J.; Alonso, M.A. Mie scattering of highly focused, scalar fields: An analytic approach. J. Opt. Soc. Am. A Opt. Image Sci. Vis. 2016, 33, 1236–1243. [Google Scholar] [CrossRef] [PubMed]
- Gutiérrez-Cuevas, R.; Moore, N.J.; Alonso, M.A. Lorenz-Mie scattering of focused light via complex focus fields: An analytic treatment. Phys. Rev. A 2018, 97, 053848. [Google Scholar] [CrossRef]
- Alonso, M.A.; Moore, N.J. Basis expansions for monochromatic field propagation in free space. In Mathematical Optics: Classical, Quantum, and Computational Methods; Lakshminarayanan, V., Calvo, M.L., Alieva., T., Eds.; CRC Press: Boca Raton, Fl, USA, 2013; pp. 98–141. [Google Scholar]
- Kravtsov, Y.A. Complex rays and complex caustics. Radiophys. Quantum El 1967, 10, 719–730. [Google Scholar] [CrossRef]
- Deschamps, G.A. Gaussian beam as a bundle of complex rays. Electron. Lett. 1971, 7, 684–685. [Google Scholar] [CrossRef]
- Felsen, L.B. Evanescent waves. J. Opt. Soc. Am. 1976, 66, 751–760. [Google Scholar] [CrossRef]
- Cullen, A.L.; Yu, P.K. Complex source-point theory of the electromagnetic open resonator. P. Roy. Soc. Lond. A Mat. 1979, 366, 155–171. [Google Scholar] [CrossRef]
- Lindell, I.V. Exact-image method for gaussian-beam problems involving a planar interface. J. Opt. Soc. Am. A 1987, 4, 2185–2190. [Google Scholar] [CrossRef]
- Parker, K.J.; Alonso, M.A. The spherical harmonic family of beampatterns. Acoustics 2022, 4, 958–966. [Google Scholar] [CrossRef]
- Abramowitz, M.; Stegun, I.A. Handbook of Mathematical Functions with Formulas, Graphs, and Mathematical Tables; U.S. Govt. Print. Off.: Washington, DC, USA, 1964. [Google Scholar]
- Kaplan, W. Fourier-Bessel series. In Advanced Calculus, 4th ed.; Addison-Wesley: Reading, MA, USA, 1992; pp. 512–518. [Google Scholar]
- Stevenson, G. Expansions of the Neumann type in terms of products of Bessel functions. Am. J. Math. 1928, 50, 569–590. [Google Scholar] [CrossRef]
- Watson, G.N. Chapter 16. In A Treatise on the Theory of Bessel Functions, 2nd ed.; Cambridge University Press: London, UK, 1944. [Google Scholar]
- Erdélyi, A.; Magnus, W.; Oberhettinger, F.; Tricomi, F.G. Higher Transcendental Functions/Vol. 1; McGraw-Hill: New York, NY, USA; London, UK, 1953. [Google Scholar]
- Williams, E.G. Fourier Acoustics: Sound Radiation and Nearfield Acoustical Holography; Academic Press: San Diego, CA, USA, 1999. [Google Scholar]
- Parker, K.J.; Alonso, M.A. The Concentrated Toroidal Wave. arXiv 2024, arXiv:2410.11700. [Google Scholar]


Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Parker, K.J.; Alonso, M.A. From Spherical Harmonics to Gaussian Beampatterns. Acoustics 2025, 7, 14. https://doi.org/10.3390/acoustics7010014
Parker KJ, Alonso MA. From Spherical Harmonics to Gaussian Beampatterns. Acoustics. 2025; 7(1):14. https://doi.org/10.3390/acoustics7010014
Chicago/Turabian StyleParker, Kevin J., and Miguel A. Alonso. 2025. "From Spherical Harmonics to Gaussian Beampatterns" Acoustics 7, no. 1: 14. https://doi.org/10.3390/acoustics7010014
APA StyleParker, K. J., & Alonso, M. A. (2025). From Spherical Harmonics to Gaussian Beampatterns. Acoustics, 7(1), 14. https://doi.org/10.3390/acoustics7010014






