Chalcogen Atom-Doped Graphene and Its Performance in N2 Activation
Abstract
:1. Introduction
2. Computational Methods
3. Results
3.1. Validation of Simulation Parameters
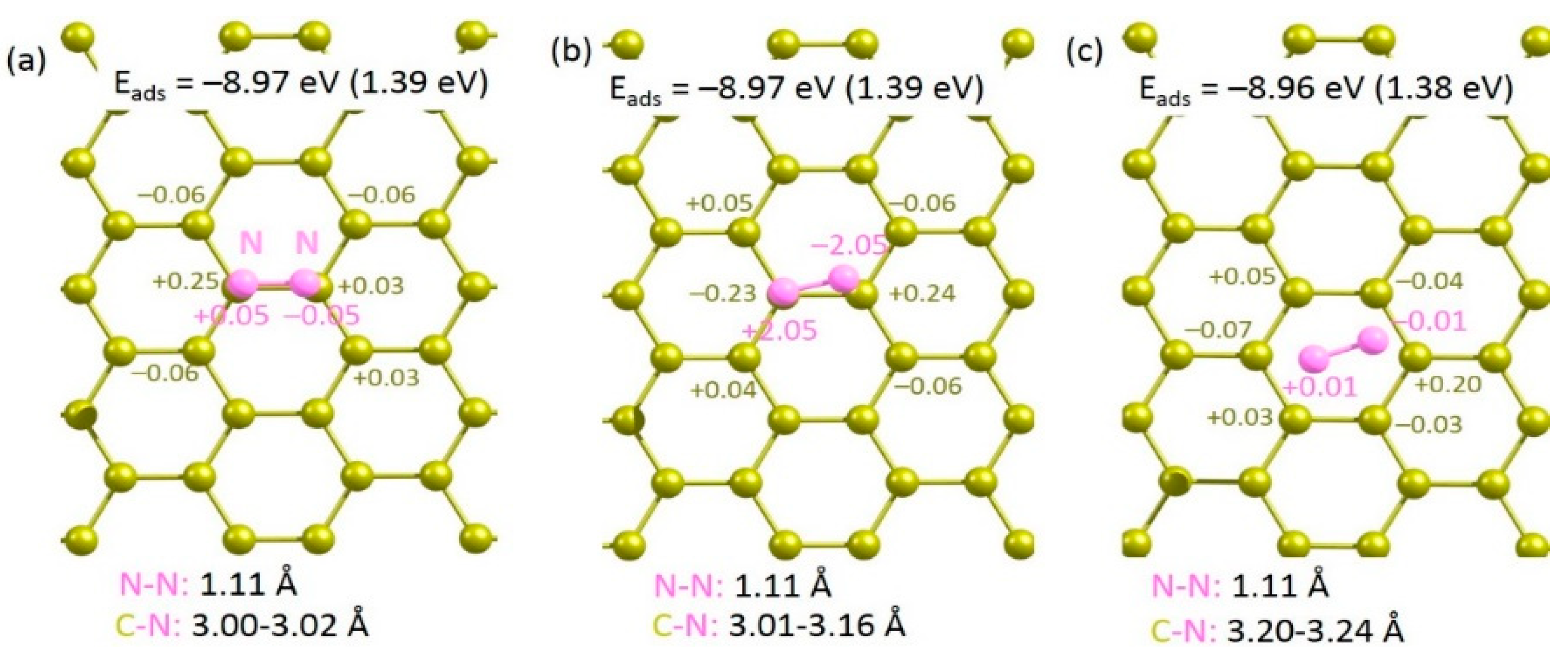
3.2. Adsorption of N2 on the Graphene
3.3. Adsorption of N2 on the Graphene Adsorbed with O, S, Se and Te
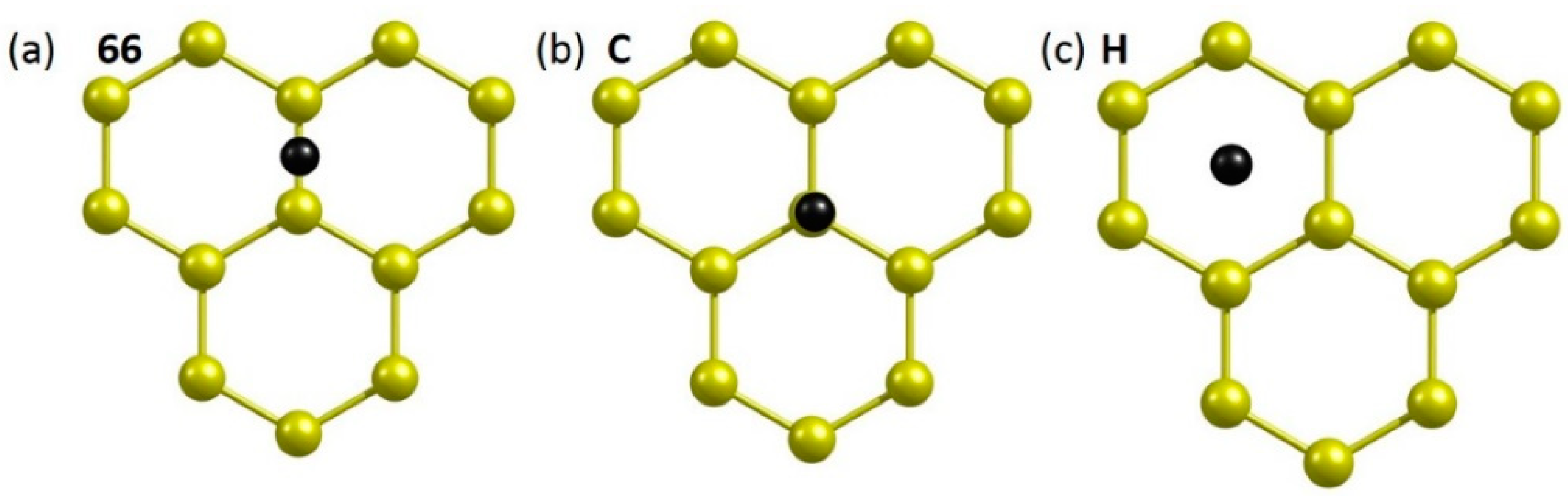
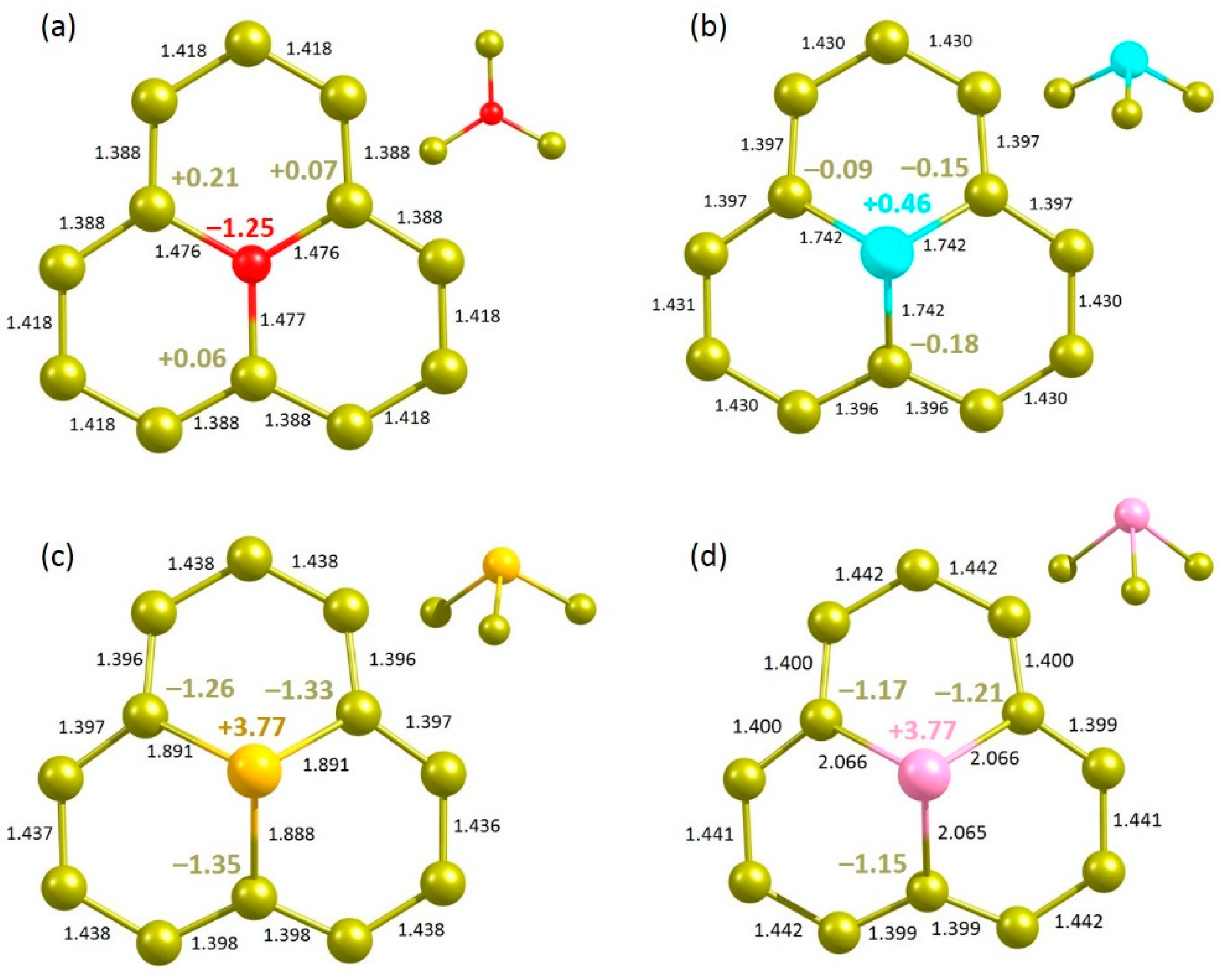
3.4. Doping of O, S, Se and Te
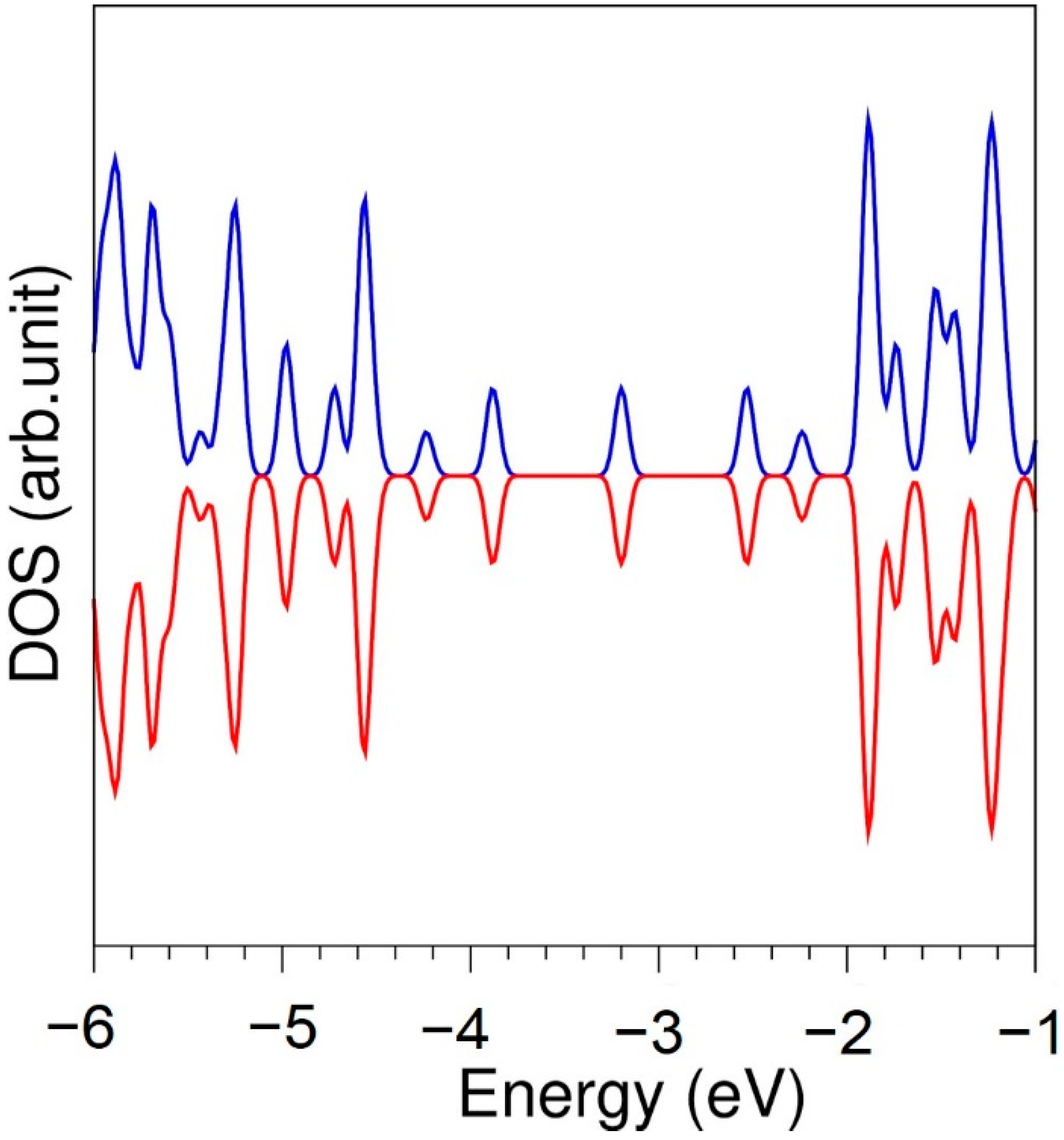
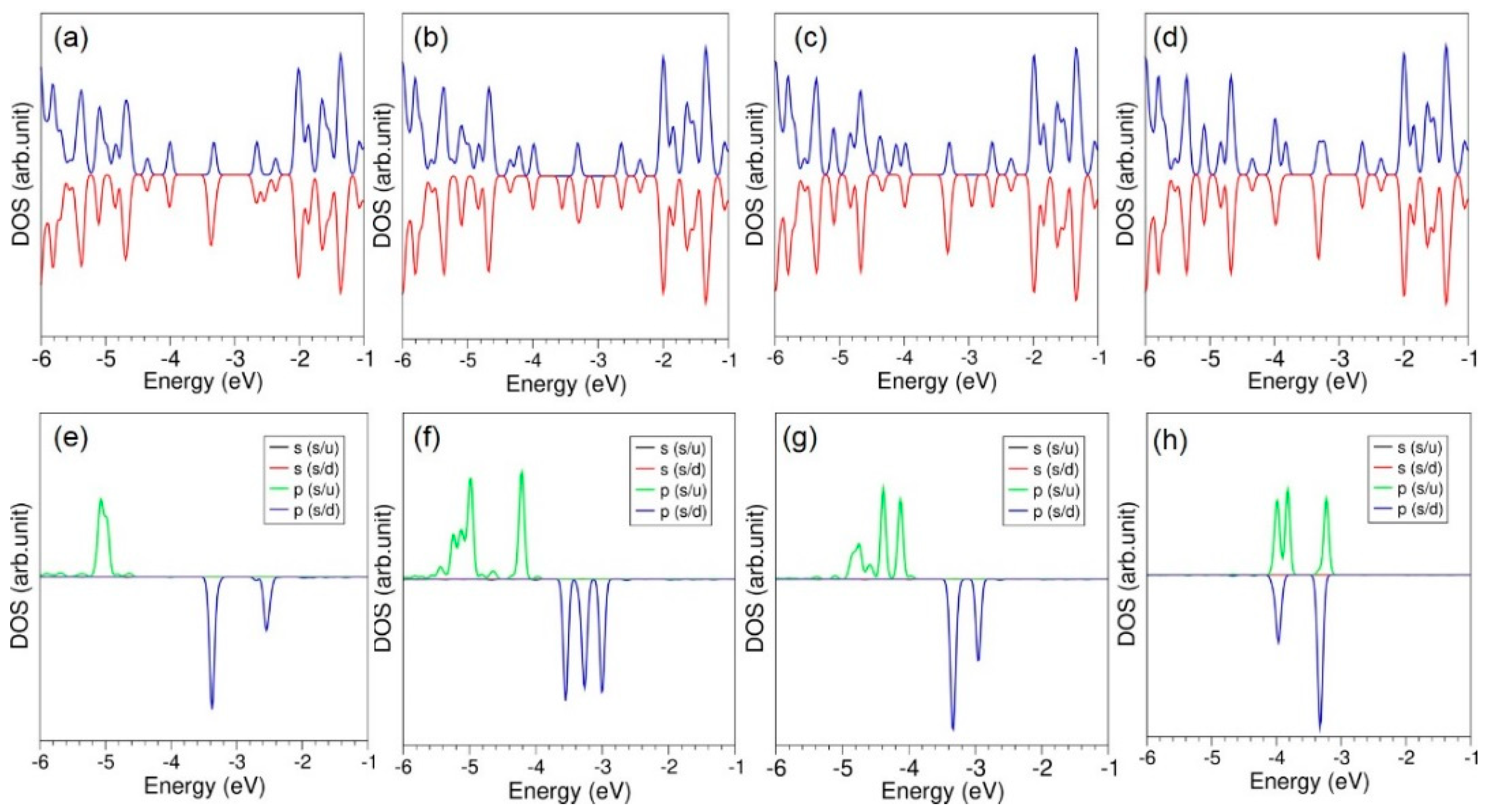
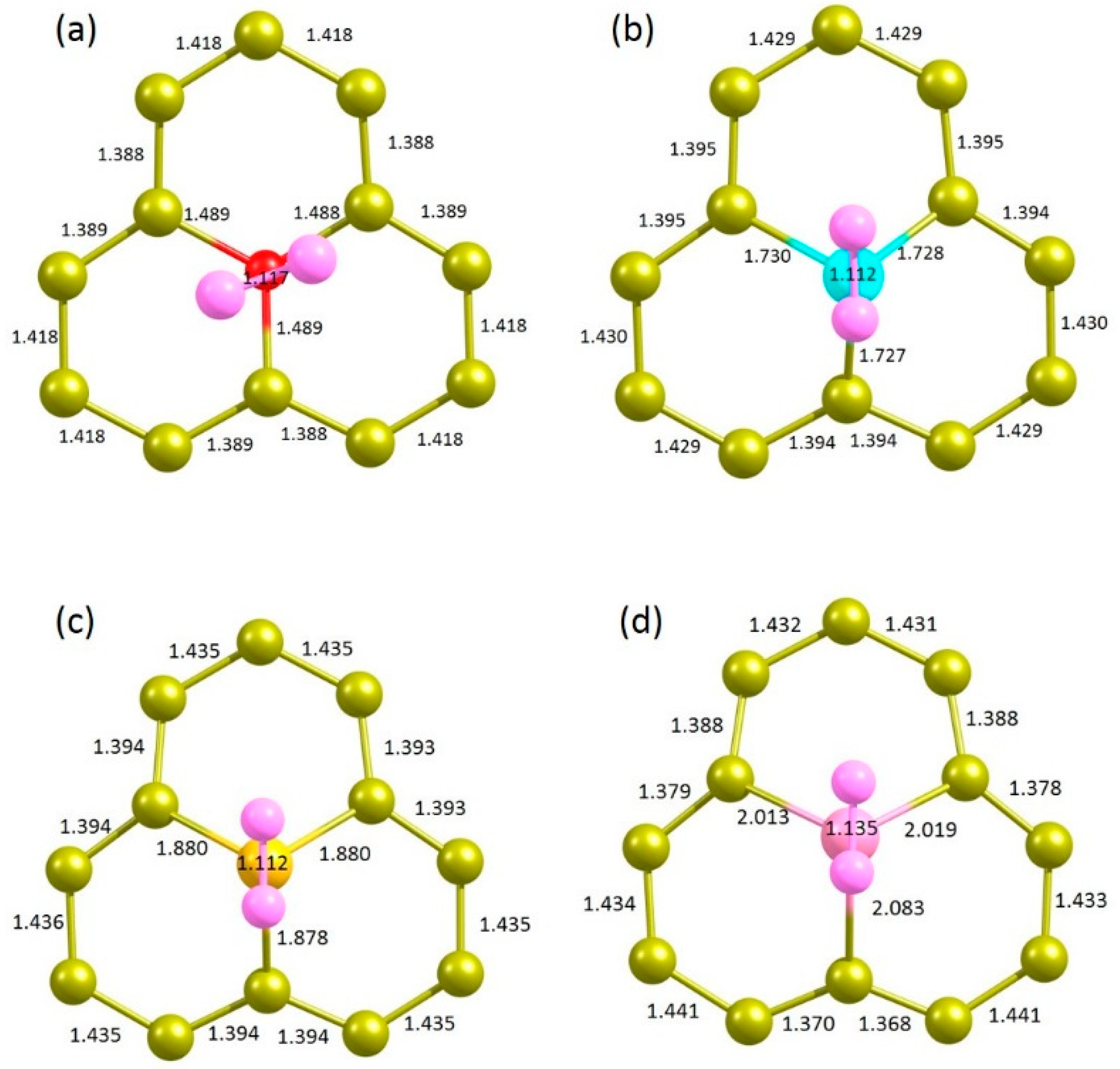
3.5. N2 Adsorption onto the Surface of Doped Graphene
4. Conclusions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Katsnelson, M.I. Graphene: Carbon in two dimensions. Mater. Today 2007, 10, 20–27. [Google Scholar] [CrossRef]
- Allen, M.J.; Tung, V.C.; Kaner, R.B. Honeycomb Carbon: A Review of Graphene. Chem. Rev. 2010, 110, 132–145. [Google Scholar] [CrossRef]
- Wu, Y.; Wang, S.; Komvopoulos, K. A review of graphene synthesis by indirect and direct deposition methods. J. Mater. Res. 2020, 35, 76–89. [Google Scholar] [CrossRef]
- Tiwari, S.K.; Sahoo, S.; Wang, N.; Huczko, A. Graphene research and their outputs: Status and prospect. J. Sci. Adv. Mater. Devices 2020, 5, 10–29. [Google Scholar] [CrossRef]
- Papageorgiou, D.G.; Kinloch, I.A.; Young, R.J. Mechanical properties of graphene and graphene-based nanocomposites. Prog. Mater. Sci. 2017, 90, 75–127. [Google Scholar] [CrossRef]
- Sang, M.; Shin, J.; Kim, K.; Yu, K.J. Electronic and Thermal Properties of Graphene and Recent Advances in Graphene Based Electronics Applications. Nanomaterials 2019, 9, 374. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Kim, Y.-J.; Kim, Y.; Novoselov, K.; Hong, B.H. Engineering electrical properties of graphene: Chemical approaches. 2D Mater. 2015, 2, 042001. [Google Scholar] [CrossRef]
- Lee, J.H.; Park, S.J.; Choi, J.W. Electrical Property of Graphene and Its Application to Electrochemical Biosensing. Nanomaterials 2019, 9, 297. [Google Scholar] [CrossRef] [Green Version]
- Olabi, A.G.; Abdelkareem, M.A.; Wilberforce, T.; Sayed, E.T. Application of graphene in energy storage device—A review. Renew. Sustain. Energy Rev. 2021, 135, 110026. [Google Scholar] [CrossRef]
- Syama, S.; Mohanan, P.V. Comprehensive Application of Graphene: Emphasis on Biomedical Concerns. Nano-Micro Lett. 2019, 11, 6. [Google Scholar] [CrossRef] [Green Version]
- Madurani, K.A.; Suprapto, S.; Machrita, N.I.; Bahar, S.L.; Illiya, W.; Kurniawan, F. Progress in Graphene Synthesis and its Application: History, Challenge and the Future Outlook for Research and Industry. ECS J. Solid State Sci. Technol. 2020, 9, 093013. [Google Scholar] [CrossRef]
- Dhinakaran, V.; Lavanya, M.; Vigneswari, K.; Ravichandran, M.; Vijayakumar, M.D. Review on exploration of graphene in diverse applications and its future horizon. Mater. Today Proc. 2020, 27, 824–828. [Google Scholar] [CrossRef]
- Zheng, W.; Zhao, X.; Fu, W. Review of Vertical Graphene and its Applications. ACS Appl. Mater. Interfaces 2021, 13, 9561–9579. [Google Scholar] [CrossRef]
- Chae, S.; Panomsuwan, G.; Bratescu, M.A.; Teshima, K.; Saito, N. p-Type Doping of Graphene with Cationic Nitrogen. ACS Appl. Nano Mater. 2019, 2, 1350–1355. [Google Scholar] [CrossRef]
- Liu, H.; Liu, Y.; Zhu, D. Chemical doping of graphene. J. Mater. Chem. 2011, 21, 3335–3345. [Google Scholar] [CrossRef]
- Meng, X.; Tongay, S.; Kang, J.; Chen, Z.; Wu, F.; Li, S.-S.; Xia, J.B.; Li, J.; Wu, J. Stable p- and n-type doping of few-layer graphene/graphite. Carbon 2013, 57, 507–514. [Google Scholar] [CrossRef]
- Du, J.; Duan, J.; Yang, X.; Duan, Y.; Zhou, Q.; Tang, Q. p-Type Charge Transfer Doping of Graphene Oxide with (NiCo)1−yFeyOx for Air-Stable, All-Inorganic CsPbIBr2 Perovskite Solar Cells. Angew. Chem. Int. Ed. 2021, 60, 10608–10613. [Google Scholar] [CrossRef] [PubMed]
- Feng, L.; Qin, Z.; Huang, Y.; Peng, K.; Wang, F.; Yan, Y.; Chen, Y. Boron-, sulfur-, and phosphorus-doped graphene for environmental applications. Sci. Total Environ. 2020, 698, 134239. [Google Scholar] [CrossRef]
- Han, J.; Zhang, L.L.; Lee, S.; Oh, J.; Lee, K.S.; Potts, J.R.; Ji, J.; Zhao, X.; Ruoff, R.S.; Park, S. Generation of B-Doped Graphene Nanoplatelets Using a Solution Process and Their Supercapacitor Applications. ACS Nano 2013, 7, 19–26. [Google Scholar] [CrossRef] [PubMed]
- Sahoo, M.; Sreena, K.P.; Vinayan, B.P.; Ramaprabhu, S. Green synthesis of boron doped graphene and its application as high performance anode material in Li ion battery. Mater. Res. Bull. 2015, 61, 383–390. [Google Scholar] [CrossRef]
- Wang, H.; Zhou, Y.; Wu, D.; Liao, L.; Zhao, S.; Peng, H.; Liu, Z. Synthesis of Boron-Doped Graphene Monolayers Using the Sole Solid Feedstock by Chemical Vapor Deposition. Small 2013, 9, 1316–1320. [Google Scholar] [CrossRef] [PubMed]
- Fang, L.; Cao, Z. CO2 activation at atomically dispersed Si sites of N-doped graphenes: Insight into distinct electron mechanisms from first-principles calculations. AIP Adv. 2021, 11, 115302. [Google Scholar] [CrossRef]
- Kuganathan, N.; Anurakavan, S.; Abiman, P.; Iyngaran, P.; Gkanas, E.I.; Chroneos, A. Adsorption of lead on the surfaces of pristine and B, Si and N-doped graphene. Phys. B Condens. Matter 2021, 600, 412639. [Google Scholar] [CrossRef]
- Chen, Y.; Liu, Y.J.; Wang, H.X.; Zhao, J.X.; Cai, Q.H.; Wang, X.Z.; Ding, Y.H. Silicon-Doped Graphene: An Effective and Metal-Free Catalyst for NO Reduction to N2O? ACS Appl. Mater. Interfaces 2013, 5, 5994–6000. [Google Scholar] [CrossRef]
- Su, H.; Hu, Y.H. Recent advances in graphene-based materials for fuel cell applications. Energy Sci. Engin. 2021, 9, 958–983. [Google Scholar] [CrossRef]
- Yoo, E.; Nakamura, J.; Zhou, H. N-Doped graphene nanosheets for Li–air fuel cells under acidic conditions. Energy Environ. Sci. 2012, 5, 6928–6932. [Google Scholar] [CrossRef]
- Elessawy, N.A.; El Nady, J.; Wazeer, W.; Kashyout, A.B. Development of High-Performance Supercapacitor based on a Novel Controllable Green Synthesis for 3D Nitrogen Doped Graphene. Sci. Rep. 2019, 9, 1129. [Google Scholar] [CrossRef] [Green Version]
- Yang, Z.; Yao, Z.; Li, G.; Fang, G.; Nie, H.; Liu, Z.; Zhou, X.; Chen, X.; Huang, S. Sulfur-Doped Graphene as an Efficient Metal-free Cathode Catalyst for Oxygen Reduction. ACS Nano 2012, 6, 205–211. [Google Scholar] [CrossRef]
- Shen, B.; Chen, J.; Yan, X.; Xue, Q. Synthesis of fluorine-doped multi-layered graphene sheets by arc-discharge. RSC Adv. 2012, 2, 6761–6764. [Google Scholar] [CrossRef]
- Yao, Z.; Nie, H.; Yang, Z.; Zhou, X.; Liu, Z.; Huang, S. Catalyst-free synthesis of iodine-doped graphenevia a facile thermal annealing process and its use for electrocatalytic oxygen reduction in an alkaline medium. Chem. Commun. 2012, 48, 1027–1029. [Google Scholar] [CrossRef]
- Li, R.; Wei, Z.; Gou, X.; Xu, W. Phosphorus-doped graphene nanosheets as efficient metal-free oxygen reduction electrocatalysts. RSC Adv. 2013, 3, 9978–9984. [Google Scholar] [CrossRef]
- Albero, J.; Vidal, A.; Migani, A.; Concepción, P.; Blancafort, L.; García, H. Phosphorus-Doped Graphene as a Metal-Free Material for Thermochemical Water Reforming at Unusually Mild Conditions. ACS Sustain. Chem. Eng. 2019, 7, 838–846. [Google Scholar] [CrossRef]
- Liu, A.; Guan, W.; Wu, K.; Ren, X.; Gao, L.; Ma, T. Density functional theory study of nitrogen-doped graphene as a high-performance electrocatalyst for CO2RR. Appl. Surf. Sci. 2021, 540, 148319. [Google Scholar] [CrossRef]
- Tyagi, J.; Sharma, L.; Kakkar, R. Graphene and doped graphene: A comparative DFT study. Adv. Mater. Lett. 2019, 10, 484–490. [Google Scholar] [CrossRef]
- Fang, Z.; Wang, Q.; Li, Y.; Li, Y.; Huang, S.; Lin, W.; Chen, W.; Zhang, Y. Theoretical insights into the thermal reduction of N2 to NH3 over a single metal atom incorporated nitrogen-doped graphene. J. Chem. Phys. 2021, 154, 054703. [Google Scholar] [CrossRef]
- Askari Ardehjani, N.; Farmanzadeh, D. DFT investigation of metal doped graphene capacity for adsorbing of ozone, nitrogen dioxide and sulfur dioxide molecules. Adsorption 2019, 25, 661–667. [Google Scholar] [CrossRef]
- Kresse, G.; Furthmüller, J. Efficient iterative schemes for ab initio total-energy calculations using a plane-wave basis set. Phys. Rev. B 1996, 54, 11169–11186. [Google Scholar] [CrossRef]
- Blöchl, P.E. Projector augmented-wave method. Phys. Rev. B 1994, 50, 17953–17979. [Google Scholar] [CrossRef] [Green Version]
- Perdew, J.P.; Burke, K.; Ernzerhof, M. Generalized Gradient Approximation Made Simple. Phys. Rev. Lett. 1996, 77, 3865–3868. [Google Scholar] [CrossRef] [Green Version]
- Press, W.H.; Teukolsky, S.A.; Vetterling, W.T.; Flannery, B.P. Numerical Recipes in C: The Art of Scientific Computing, 2nd ed.; Cambridge University Press: New York, NY, USA, 1992. [Google Scholar]
- Monkhorst, H.J.; Pack, J.D. Special points for Brillouin-zone integrations. Phys. Rev. B 1976, 13, 5188–5192. [Google Scholar] [CrossRef]
- Grimme, S.; Antony, J.; Ehrlich, S.; Krieg, H. A consistent and accurate ab initio parametrization of density functional dispersion correction (DFT-D) for the 94 elements H-Pu. J. Chem. Phys. 2010, 132, 154104. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Bader, R.F.W. The zero-flux surface and the topological and quantum definitions of an atom in a molecule. Theor. Chem. Acc. 2001, 105, 276–283. [Google Scholar] [CrossRef]
- Yamanaka, T.; Morimoto, S. Isotope effect on anharmonic thermal atomic vibration and [kappa] refinement of 12C and 3C diamond. Acta Crystallogr. Sect. B 1996, 52, 232–238. [Google Scholar] [CrossRef]
- Bosak, A.; Krisch, M.; Mohr, M.; Maultzsch, J.; Thomsen, C. Elasticity of single-crystalline graphite: Inelastic x-ray scattering study. Phys. Rev. B 2007, 75, 153408. [Google Scholar] [CrossRef] [Green Version]
- Abrahams, S. The crystal and molecular structure of orthorhombic sulfur. Acta Crystallogr. 1955, 8, 661–671. [Google Scholar] [CrossRef] [Green Version]
- Keller, R.; Holzapfel, W.B.; Schulz, H. Effect of pressure on the atom positions in Se and Te. Phys. Rev. B 1977, 16, 4404–4412. [Google Scholar] [CrossRef]
- Adenis, C.; Langer, V.; Lindqvist, O. Reinvestigation of the structure of tellurium. Acta Crystallogr. Sect. C 1989, 45, 941–942. [Google Scholar] [CrossRef]
- Singh, S.; Faraz, M.; Khare, N. Recent Advances in Semiconductor–Graphene and Semiconductor–Ferroelectric/Ferromagnetic Nanoheterostructures for Efficient Hydrogen Generation and Environmental Remediation. ACS Omega 2020, 5, 11874–11882. [Google Scholar] [CrossRef]
- Lide, D.R. CRC Handbook of Chemistry and Physics; ACS Publications: Florida, CA, USA, 2008; pp. 12–114. [Google Scholar]



| Diamond [44] | |||
|---|---|---|---|
| Expt | Calc | |∆| (%) | |
| a = b = c (Å) | 3.567 | 3.573 | 0.31 |
| α = β = γ (°) | 90.0 | 90.0 | 0.00 |
| Graphite [P63mc] [45] | |||
| a = b (Å) | 2.468 | 2.469 | 0.04 |
| c (Å) | 8.685 | 8.686 | 0.01 |
| α = β (°) | 90.0 | 90.0 | 0.00 |
| γ (°) | 120.0 | 120.0 | 0.00 |
| Bulk S8 [F d d d] [46] | |||
| a (Å) | 10.437 | 10.624 | 1.79 |
| b (Å) | 12.845 | 13.068 | 1.74 |
| c (Å) | 24.369 | 24.799 | 1.76 |
| α = β = γ (°) | 90.0 | 90.0 | 0.00 |
| Bulk Se [P 31 2 1] [47] | |||
| a = b (Å) | 4.052 | 4.122 | 1.73 |
| c (Å) | 5.038 | 5.172 | 2.67 |
| α = β (°) | 90.0 | 90.0 | 0.00 |
| γ (°) | 120.0 | 120.0 | 0.00 |
| Bulk Te [P 31 2 1] [48] | |||
| a = b (Å) | 4.456 | 4.413 | 0.96 |
| c (Å) | 5.921 | 5.942 | 0.35 |
| α = β (°) | 90.0 | 90.0 | 0.00 |
| γ (°) | 120.0 | 120.0 | 0.00 |
| Atoms | Relative Energy (eV) | ||
|---|---|---|---|
| 66 | C | H | |
| O | 0.00 | +0.01 | +0.02 |
| S | 0.00 | +0.03 | +0.05 |
| Se | 0.00 | +0.01 | +0.02 |
| Te | 0.00 | +0.01 | +0.02 |
| Atom (X) | Electronegativity of X [50] | Adsorption Energy (eV) | Bader Charge on X (|e|) | C-X (Å) |
|---|---|---|---|---|
| O | 3.44 | −0.84 | −0.34 | 2.84 |
| S | 2.58 | −0.55 | −0.29 | 3.14 |
| Se | 2.55 | −0.42 | −0.26 | 3.25 |
| Te | 2.10 | −0.35 | −0.19 | 3.49 |
| Configuration | Adsorption Energy (eV) | Bader Charge (e) | C-X (Å) | N-N (Å) | |
|---|---|---|---|---|---|
| X (X=O, S, Se and Te) | N2 | ||||
| O | +0.65 | −0.31 | +0.04, +0.13 | 2.76 | 1.195 |
| S | +0.44 | +0.14 | −0.04, −0.14 | 2.87 | 1.182 |
| Se | +1.26 | −0.04 | −0.01, −0.02 | 2.17 | 1.115 |
| Te | +1.68 | +0.08 | +0.18, −0.26 | 2.42 | 1.119 |
| X in X@Graphene (X = O, S, Se and Te) | Electronegativity of X [50] | Substitution Energy (eV) | C-X (Å) | Bader Charge on X (e) |
|---|---|---|---|---|
| O | 3.44 | 7.43 | 1.46 | −1.25 |
| S | 2.58 | 8.33 | 1.74 | +0.46 |
| Se | 2.55 | 9.77 | 1.89 | +3.77 |
| Te | 2.10 | 11.06 | 2.06 | +3.77 |
| Configuration | Adsorption Energy (eV) | Bader Charge (e) | N-X (Å) | N-N (Å) | |
|---|---|---|---|---|---|
| X (X = O, S, Se and Te) | N2 | ||||
| O | −0.07 | +0.18, +0.08, +0.08 | −2.04, +2.04 | 3.03 | 1.12 |
| S | +0.07 | −0.10, −0.17, −0.27 | −0.10, +0.10 | 3.16 | 1.11 |
| Se | +0.27 | −0.37, −0.23, −0.28 | −0.01, +0.01 | 3.38 | 1.11 |
| Te | +5.65 | −0.37, −0.23, −0.40 | −0.16, −0.31 | 3.47 | 1.14 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kuganathan, N. Chalcogen Atom-Doped Graphene and Its Performance in N2 Activation. Surfaces 2022, 5, 228-237. https://doi.org/10.3390/surfaces5020016
Kuganathan N. Chalcogen Atom-Doped Graphene and Its Performance in N2 Activation. Surfaces. 2022; 5(2):228-237. https://doi.org/10.3390/surfaces5020016
Chicago/Turabian StyleKuganathan, Navaratnarajah. 2022. "Chalcogen Atom-Doped Graphene and Its Performance in N2 Activation" Surfaces 5, no. 2: 228-237. https://doi.org/10.3390/surfaces5020016
APA StyleKuganathan, N. (2022). Chalcogen Atom-Doped Graphene and Its Performance in N2 Activation. Surfaces, 5(2), 228-237. https://doi.org/10.3390/surfaces5020016






