Design of a Pre-Bunched THz Free Electron Laser
Abstract
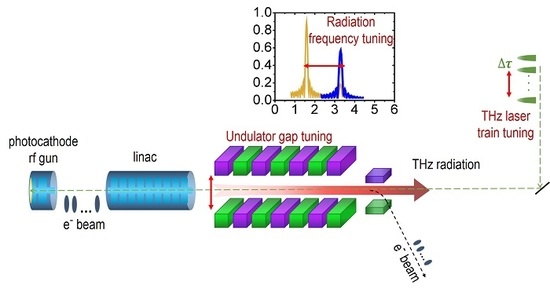
:1. Introduction
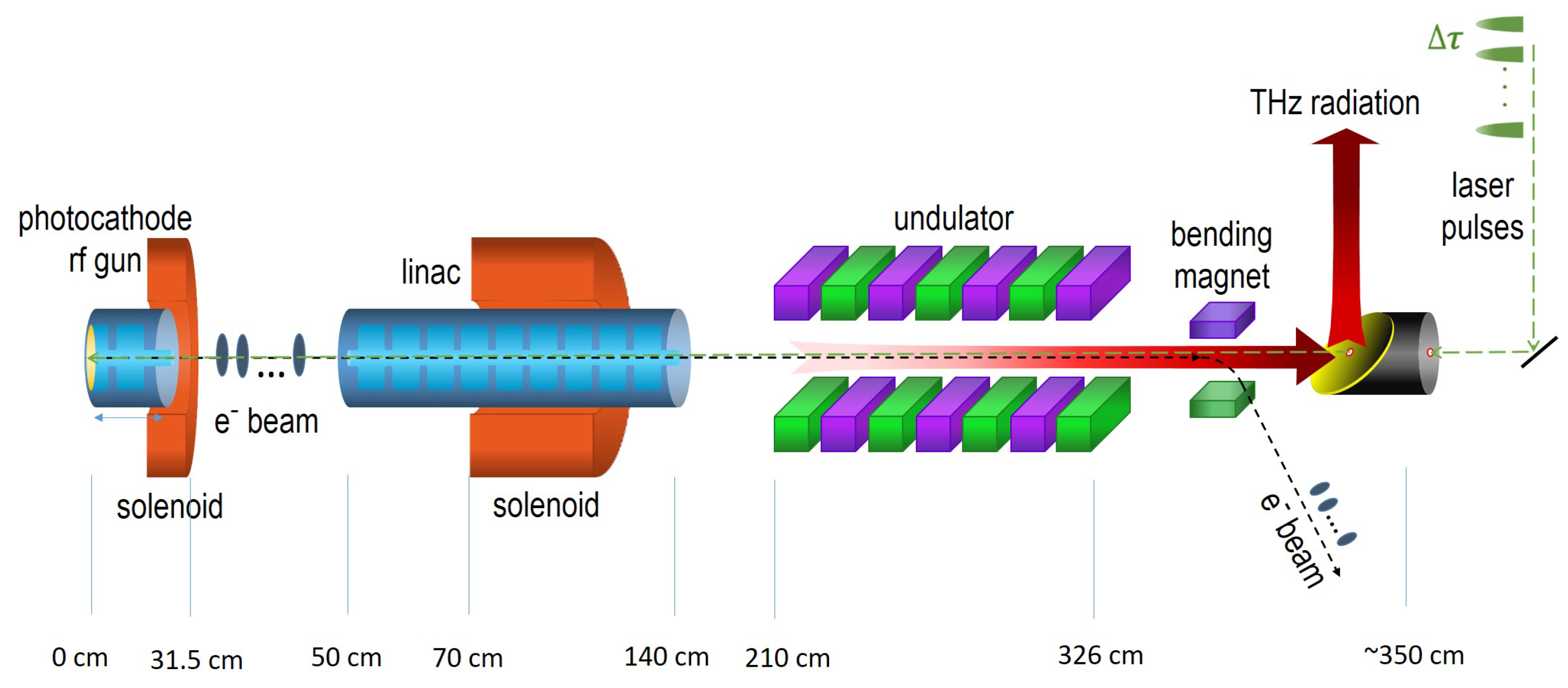
2. Experimental Apparatus
2.1. Photocathode rf Gun
2.2. Laser System
2.3. Linear Accelerator
2.4. Undulator
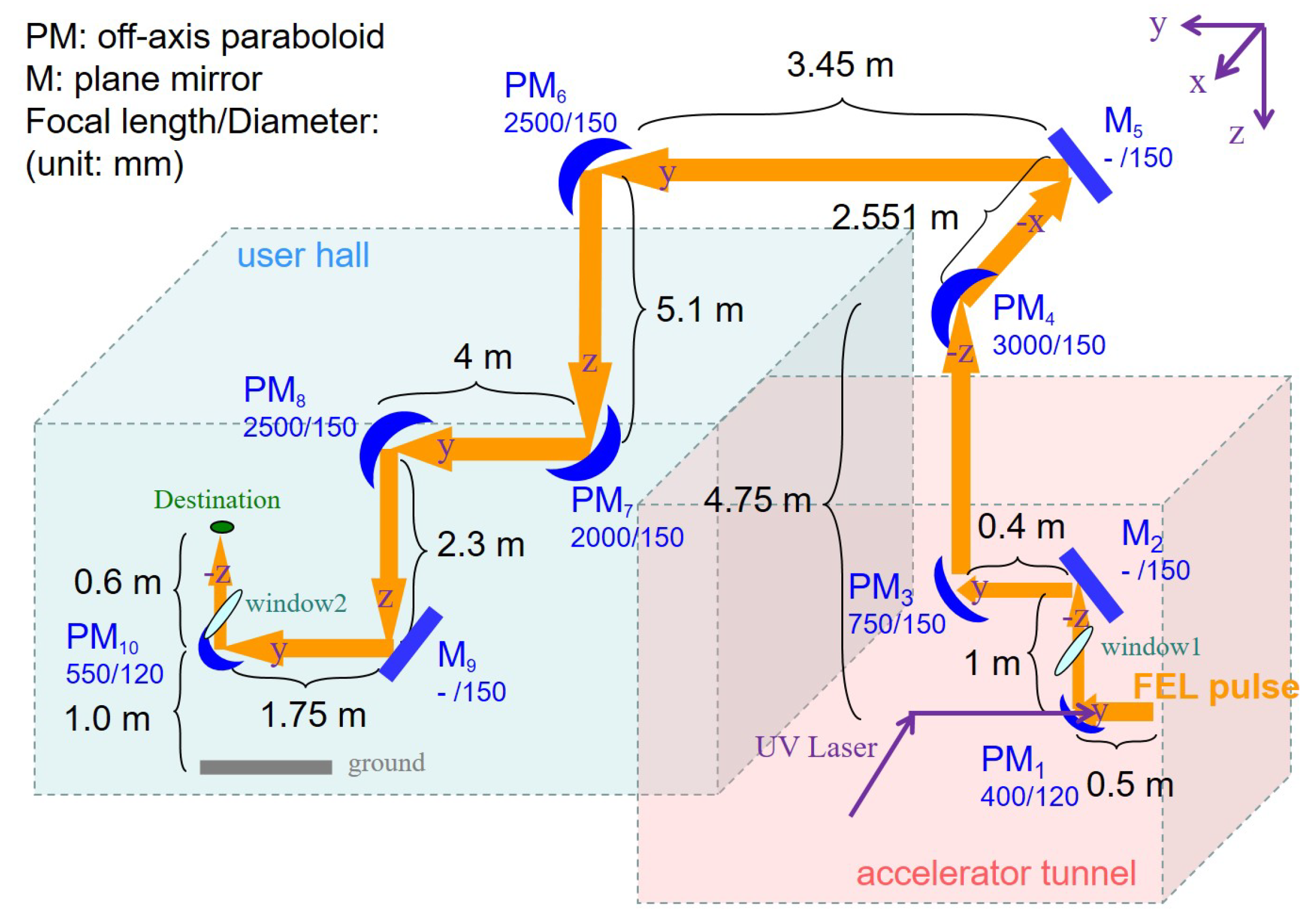
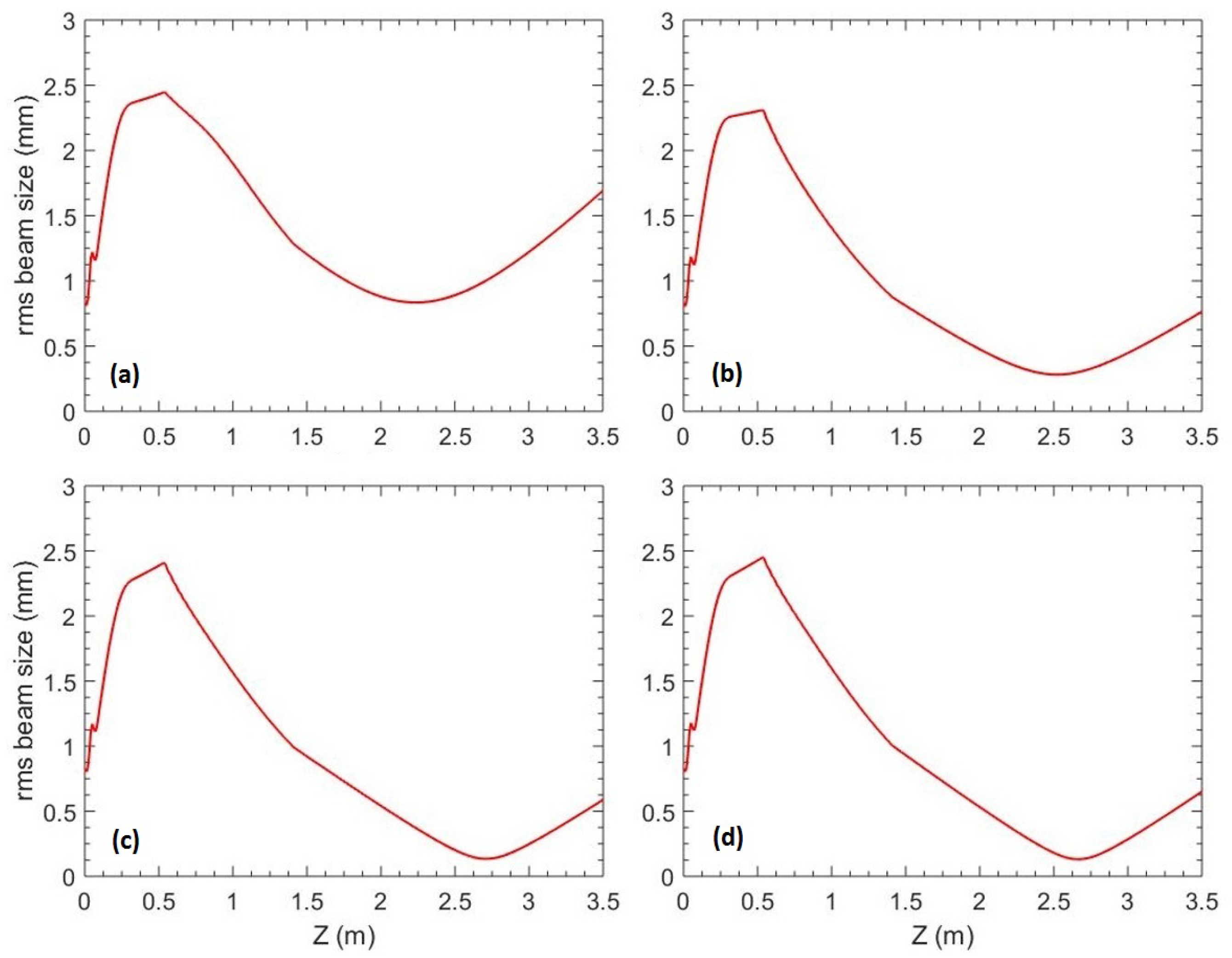
2.5. Transport Optics
3. Simulation Results
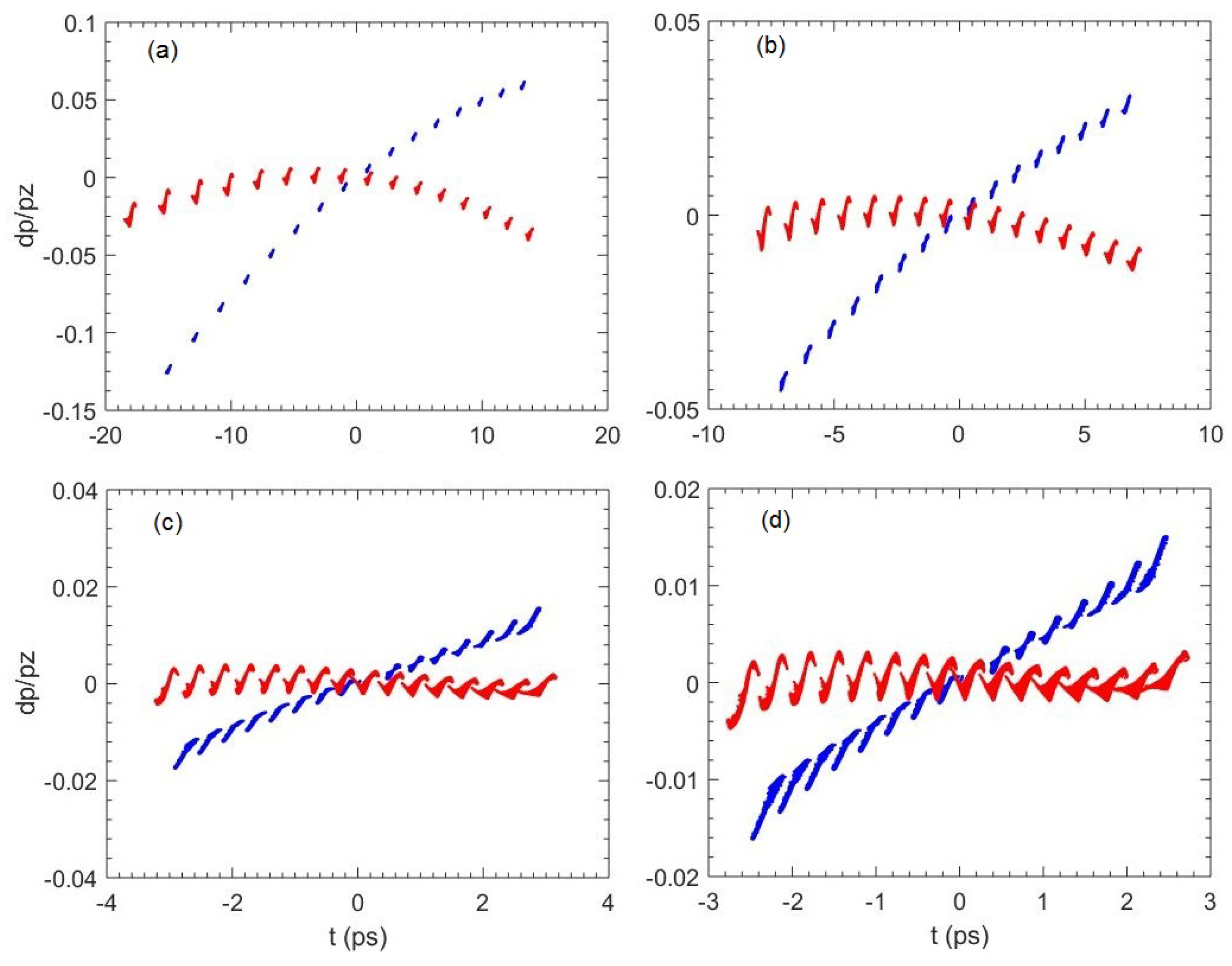
3.1. Electron Beam
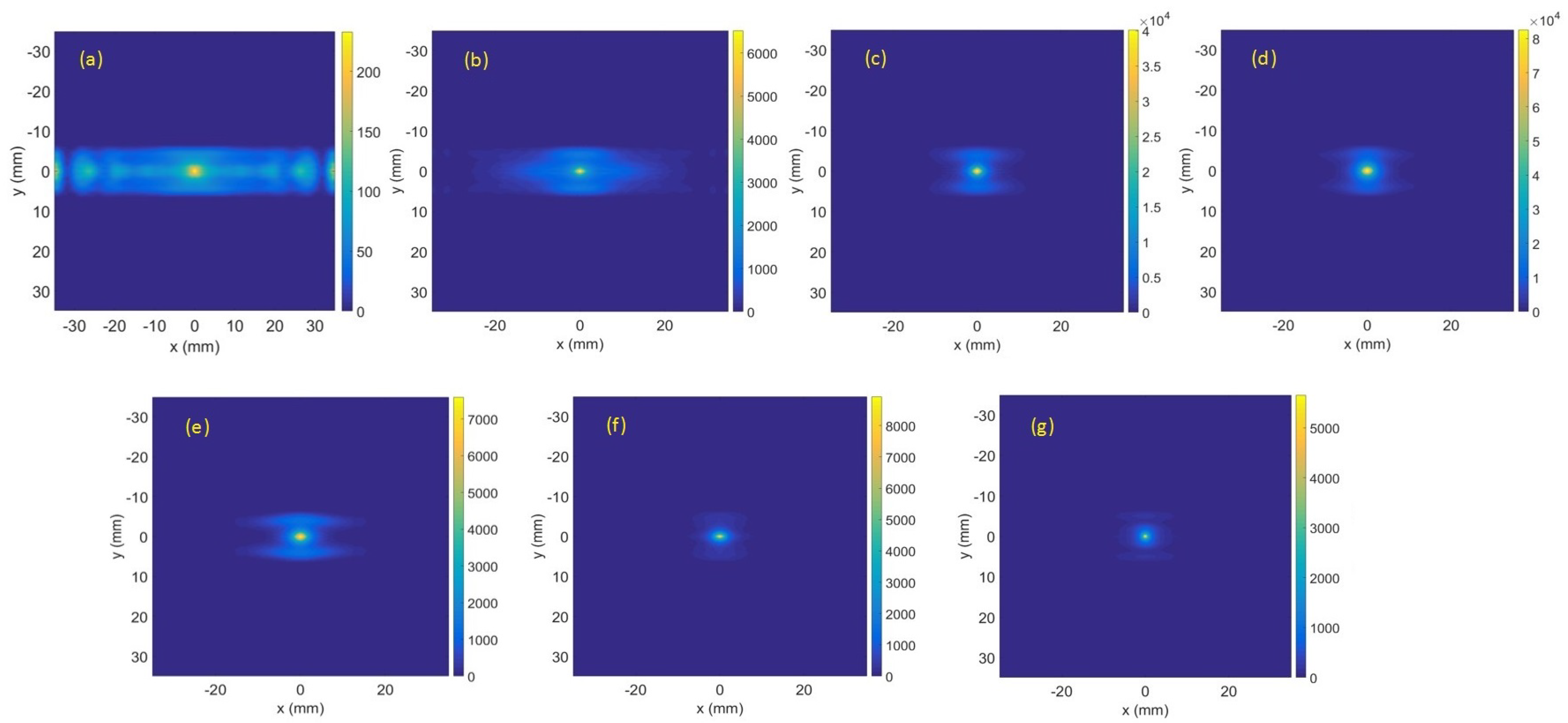
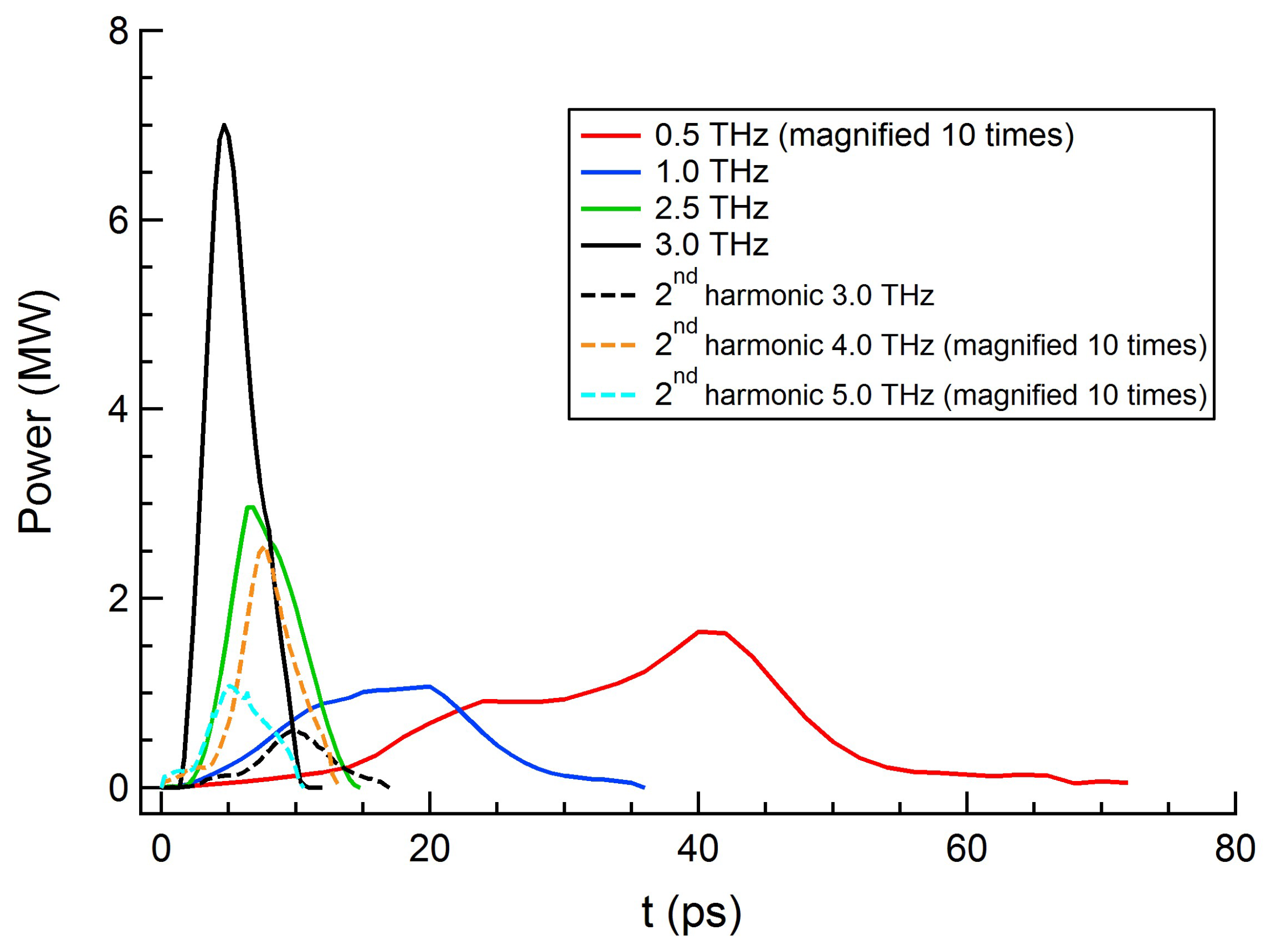
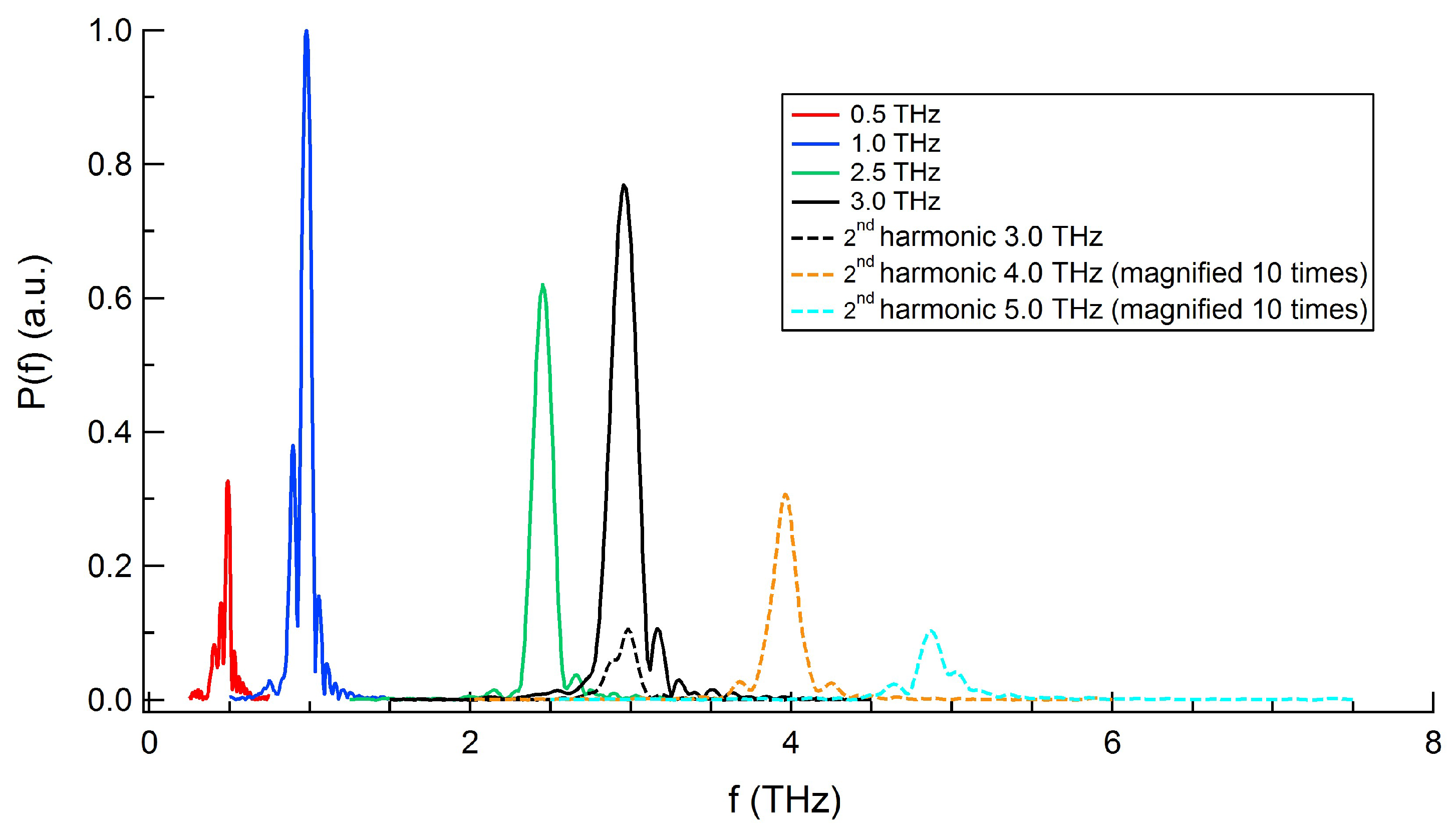
3.2. Coherent Radiation
3.3. THz Transmission
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
Abbreviations
| THz | terahertz |
| FEL | free electron laser |
| MW | megawatt |
| fs | femtosecond |
| linac | linear accelerator |
| UV | ultraviolet |
| PM | paraboloid |
| UHV | ultrahigh vacuum |
| ps | picosecond |
References
- Zhang, X.C.; Shkurinov, A.; Zhang, Y. Extreme terahertz science. Nat. Photonics 2017, 11, 16–18. [Google Scholar] [CrossRef]
- Pawar, A.Y.; Sonawane, D.D.; Erande, K.B.; Derle, D.V. Terahertz technology and its applications. Drug Invent. Today 2013, 5, 157–163. [Google Scholar] [CrossRef]
- Abina, A.; Puc, U.; Jeglič, A.; Zidanšek, A. Applications of terahertz spectroscopy in the field of construction and building materials. Appl. Spectrosc. Rev. 2015, 50, 279–303. [Google Scholar] [CrossRef]
- Gu, J.; Singh, R.; Tian, Z.; Cao, W.; Xing, Q.; He, M.; Zhang, J.W.; Han, J.; Chen, H.T.; Zhang, W. Terahertz superconductor metamaterial. Appl. Phys. Lett. 2010, 97, 071102. [Google Scholar] [CrossRef]
- Smye, S.W.; Chamberlain, J.M.; Fitzgerald, A.J.; Berry, E. The interaction between terahertz radiation and biological tissue. Phys. Med. Biol. 2001, 46, R101–R112. [Google Scholar] [CrossRef] [PubMed]
- Hoffmann, M.C.; Fülöp, J.A. Intense ultrashort terahertz pulses: Generation and applications. J. Phys. D Appl. Phys. 2011, 44, 083001. [Google Scholar] [CrossRef]
- Xiang, D.; Stupakov, G. Enhanced tunable narrow-band THz emission from laser-modulated electron beams. Phys. Rev. Spec. Top. Accel. Beams 2009, 12, 080701. [Google Scholar] [CrossRef]
- Dunning, M.; Hast, C.; Hemsing, E.; Jobe, K.; McCormick, D.; Nelson, J.; Raubenheimer, T.O.; Soong, K.; Szalata, Z.; Walz, D.; et al. Generating periodic terahertz structures in a relativistic electron beam through frequency down-conversion of optical lasers. Phys. Rev. Lett. 2012, 109, 074801. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Gover, A. Superradiant and stimulated-superradiant emission in prebunched electron-beam radiators. I. Formulation. Phys. Rev. Spec. Top. Accel. Beams 2005, 8, 030701. [Google Scholar] [CrossRef]
- Shen, Y.; Yang, X.; Carr, G.L.; Hidaka, Y.; Murphy, J.B.; Wang, X. Tunable few-cycle and multicycle coherent terahertz radiation from relativistic electrons. Phys. Rev. Lett. 2011, 107, 204801. [Google Scholar] [CrossRef] [PubMed]
- Li, H.; Lu, Y.; He, Z.; Jia, Q.; Wang, L. Generation of Intense Narrow-Band Tunable Terahertz Radiation from Highly Bunched Electron Pulse Train. J. Infrared Millim. Terahertz Waves 2016, 37, 649–657. [Google Scholar] [CrossRef]
- Zhou, F.; Sheppard, J.C.; Vecchione, T.; Jongewaard, E.; Brachmann, A.; Corbett, J.; Gilevich, S.; Weathersby, S. Establishing reliable good initial quantum efficiency and in-situ laser cleaning for the copper cathodes in the RF gun. Nucl. Instrum. Methods Phys. Res. Sect. A 2015, 783, 51–57. [Google Scholar] [CrossRef] [Green Version]
- Zhou, F.; Brachmann, A.; Emma, P.; Gilevich, S.; Huang, Z. Impact of the spatial laser distribution on photocathode gun operation. Phys. Rev. Spec. Top. Accel. Beams. 2012, 15, 090701. [Google Scholar] [CrossRef]
- He, Z.; Xu, Y.; Li, W.; Jia, Q. Generation of quasiequally spaced ultrashort microbunches in a photocathode rf gun. Nucl. Instrum. Methods Phys. Res. Sect. A 2015, 775, 77–83. [Google Scholar] [CrossRef]
- Floettmann, K. ASTRA User Manual. Available online: http://www.desy.de/~mpyflo/ (accessed on 30 October 2018 ).
- Huang, Y.C. Desktop megawatt superradiant free-electron laser at terahertz frequencies. Appl. Phys. Lett. 2010, 96, 231503. [Google Scholar] [CrossRef]
- Reiche, S. GENESIS 1.3: A fully 3D time-dependent FEL simulation code. Nucl. Instrum. Methods Phys. Res. Sect. A 1999, 429, 243–248. [Google Scholar] [CrossRef]
- Li, W.; Li, H.; He, Z.; Jia, Q.; Wang, L.; Lu, Y. Numerical Method for Free Electron Laser using an Overmoded Rectangular Waveguide. arXiv, 2018; arXiv:1806.00162. [Google Scholar]
- Casalbuoni, S.; Schmidt, B.; Schmüser, P.; Arsov, V.; Wesch, S. Ultrabroadband terahertz source and beamline based on coherent transition radiation. Phys. Rev. Spec. Top. Accel. Beams 2009, 12, 030705. [Google Scholar] [CrossRef]


| Parameter | Value |
|---|---|
| electron energy | 11 to 18 MeV |
| microbunches | 16 |
| total charge | 240 pC |
| fundamental frequency | 0.5 to 3.0 THz |
| bunching factor | ≥0.4 |
| Radiation Frequency (THz) | Radiation Characteristics at Undulator Exit | ||||
|---|---|---|---|---|---|
| Peak Power (MW) | Pulse Energy (J) | Pulse Width (ps) | Radiation Bandwidth (%) | ||
| 0.5 | Fundamental wave | 0.165 | 4.01 | 72 | 19.8 |
| 1.0 | 1.07 | 18.03 | 36 | 8.5 | |
| 2.5 | 2.97 | 18.18 | 14.4 | 4.6 | |
| 3.0 | 7.00 | 31.01 | 12 | 5.2 | |
| 3.0 | 2nd harmonic wave | 0.61 | 3.83 | 17.3 | 6.8 |
| 4.0 | 0.256 | 1.21 | 13 | 6.2 | |
| 5.0 | 0.11 | 0.54 | 10.4 | 9.4 | |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Huang, R.; Li, W.; Zhao, Z.; Li, H.; Wang, J.; Ma, T.; Huang, Q.; He, Z.; Jia, Q.; Wang, L.; et al. Design of a Pre-Bunched THz Free Electron Laser. Particles 2018, 1, 267-278. https://doi.org/10.3390/particles1010021
Huang R, Li W, Zhao Z, Li H, Wang J, Ma T, Huang Q, He Z, Jia Q, Wang L, et al. Design of a Pre-Bunched THz Free Electron Laser. Particles. 2018; 1(1):267-278. https://doi.org/10.3390/particles1010021
Chicago/Turabian StyleHuang, Ruixuan, Weiwei Li, Zhouyu Zhao, Heting Li, Jigang Wang, Tian Ma, Qiuping Huang, Zhigang He, Qika Jia, Lin Wang, and et al. 2018. "Design of a Pre-Bunched THz Free Electron Laser" Particles 1, no. 1: 267-278. https://doi.org/10.3390/particles1010021
APA StyleHuang, R., Li, W., Zhao, Z., Li, H., Wang, J., Ma, T., Huang, Q., He, Z., Jia, Q., Wang, L., & Lu, Y. (2018). Design of a Pre-Bunched THz Free Electron Laser. Particles, 1(1), 267-278. https://doi.org/10.3390/particles1010021