Evaluating the Ceiling Gas Temperature in a Branched Tunnel Fire with a Sloped Mainline Region under Natural Ventilation
Abstract
1. Introduction
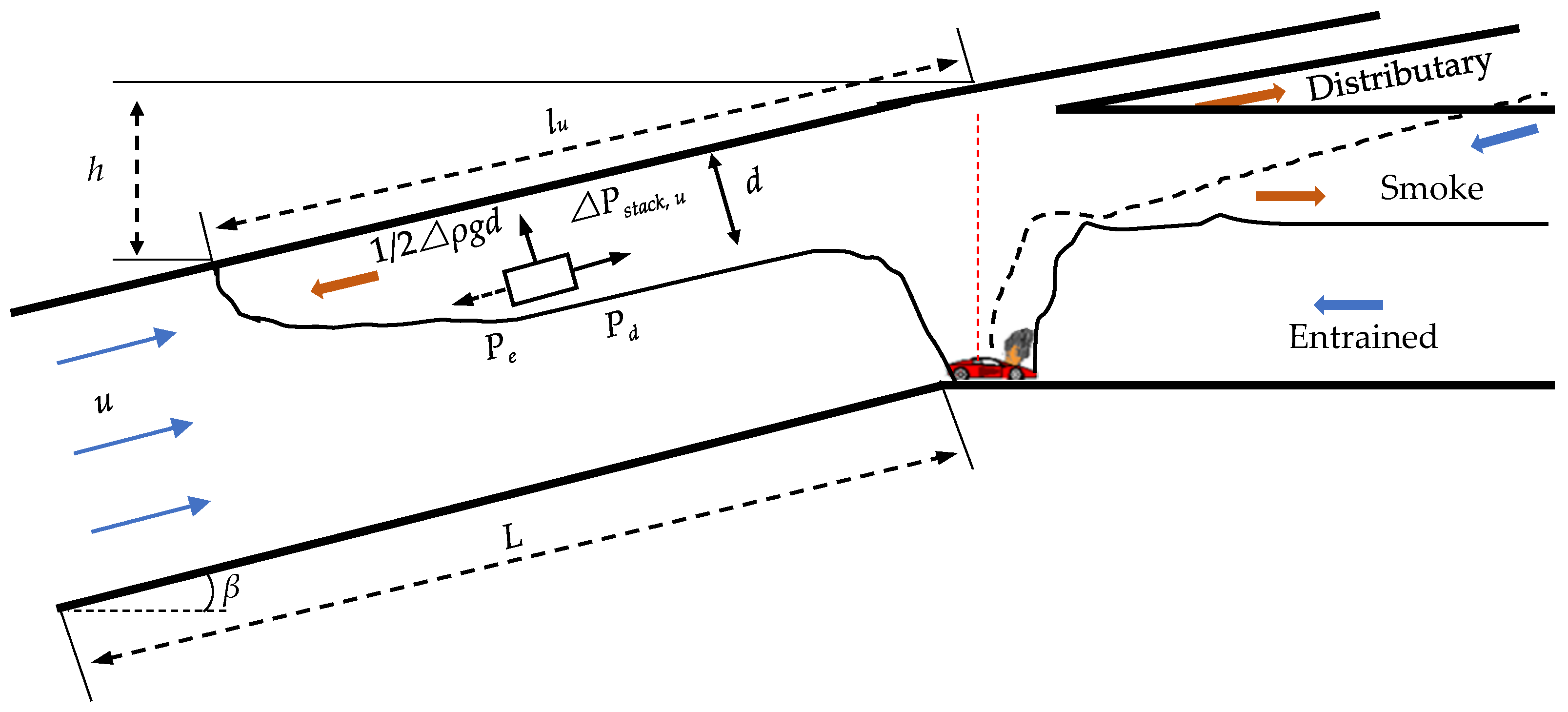
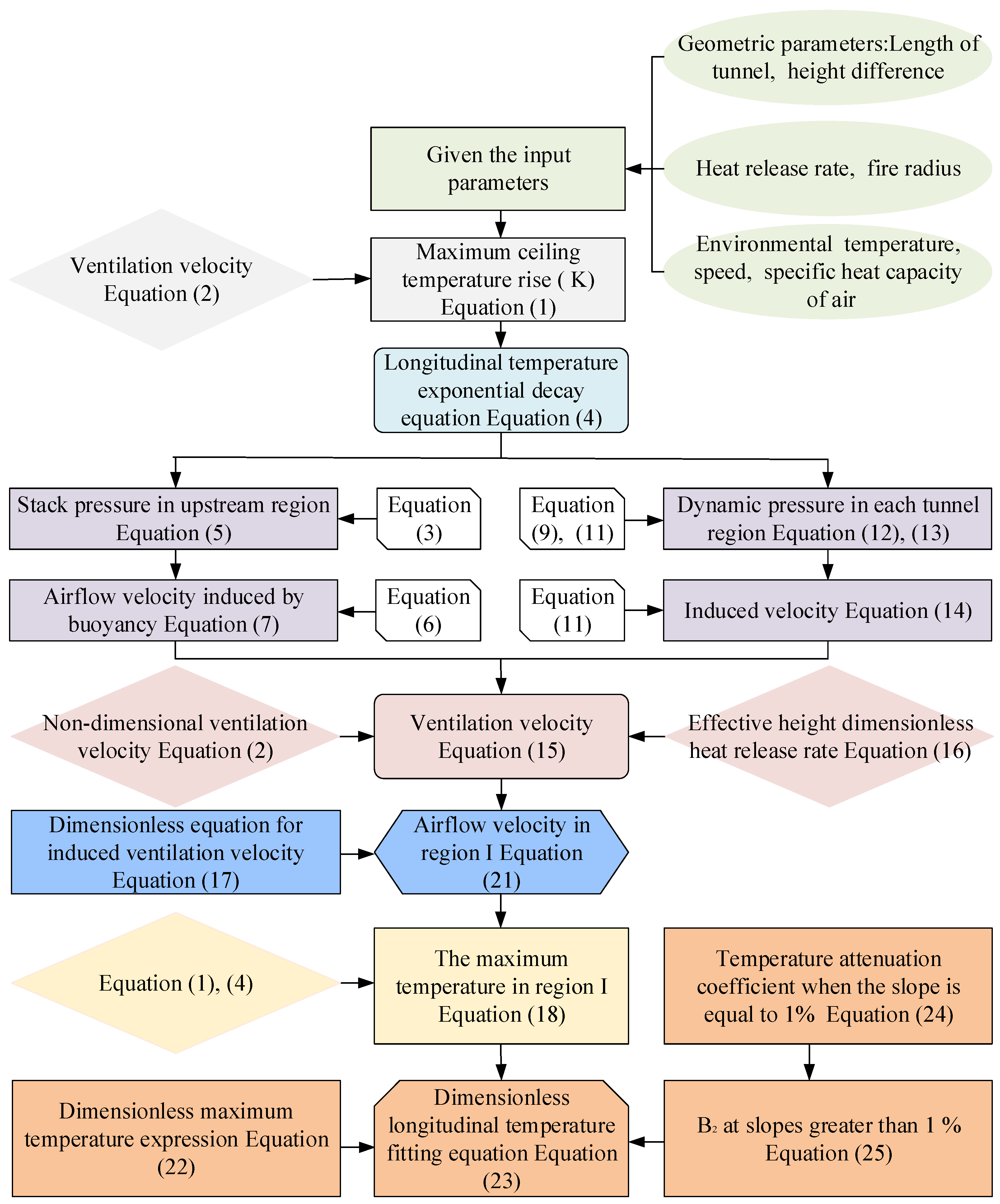
2. Theoretical Analysis
3. Numerical Method
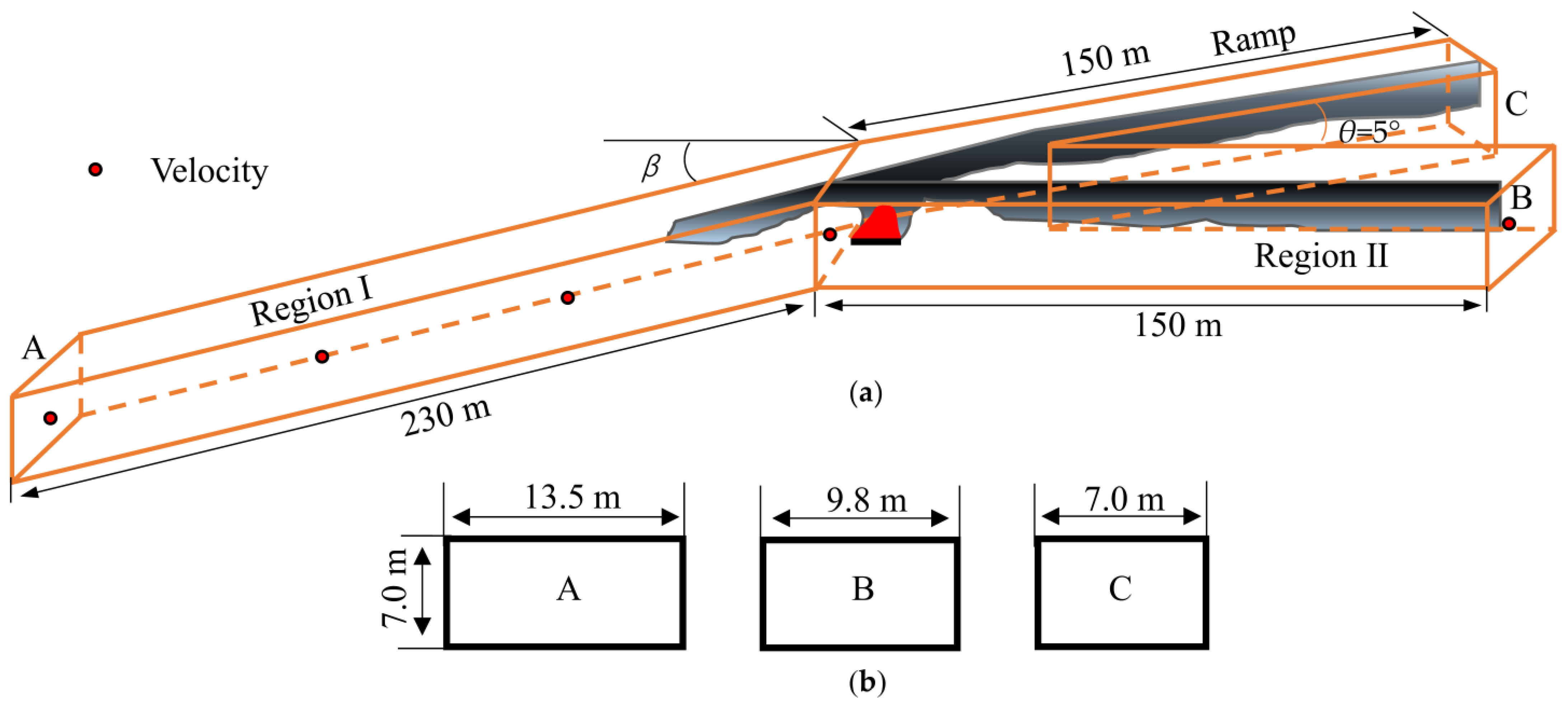
3.1. Physical Model Set-Up
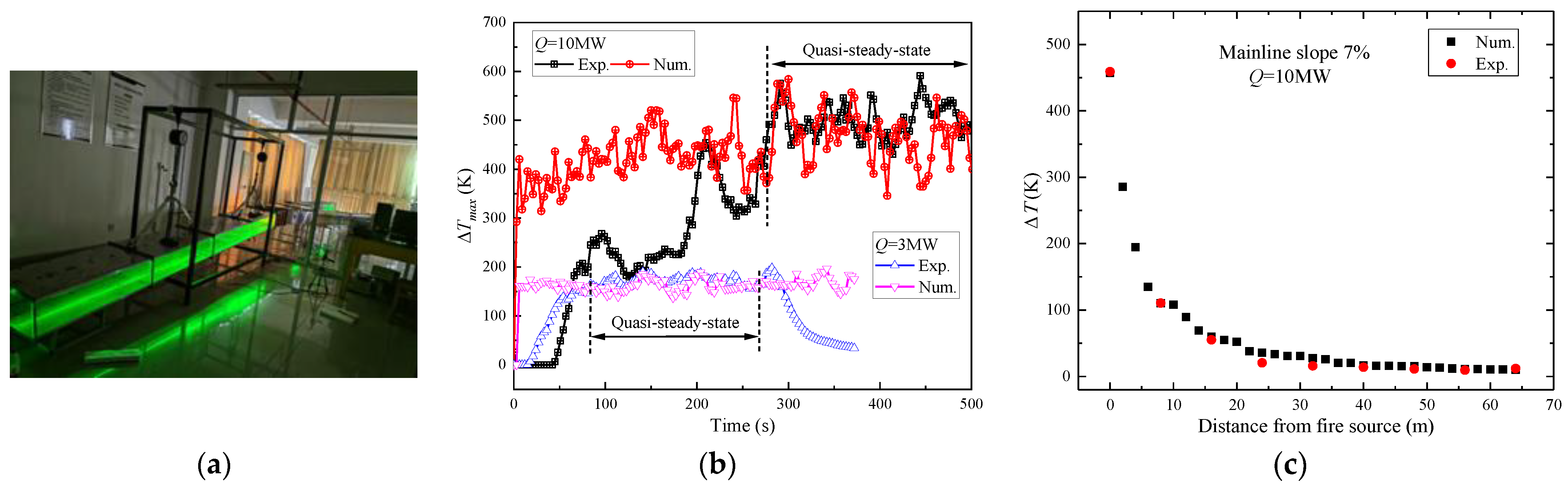
3.2. Grid Sensitivity Analysis
4. Results and Discussion
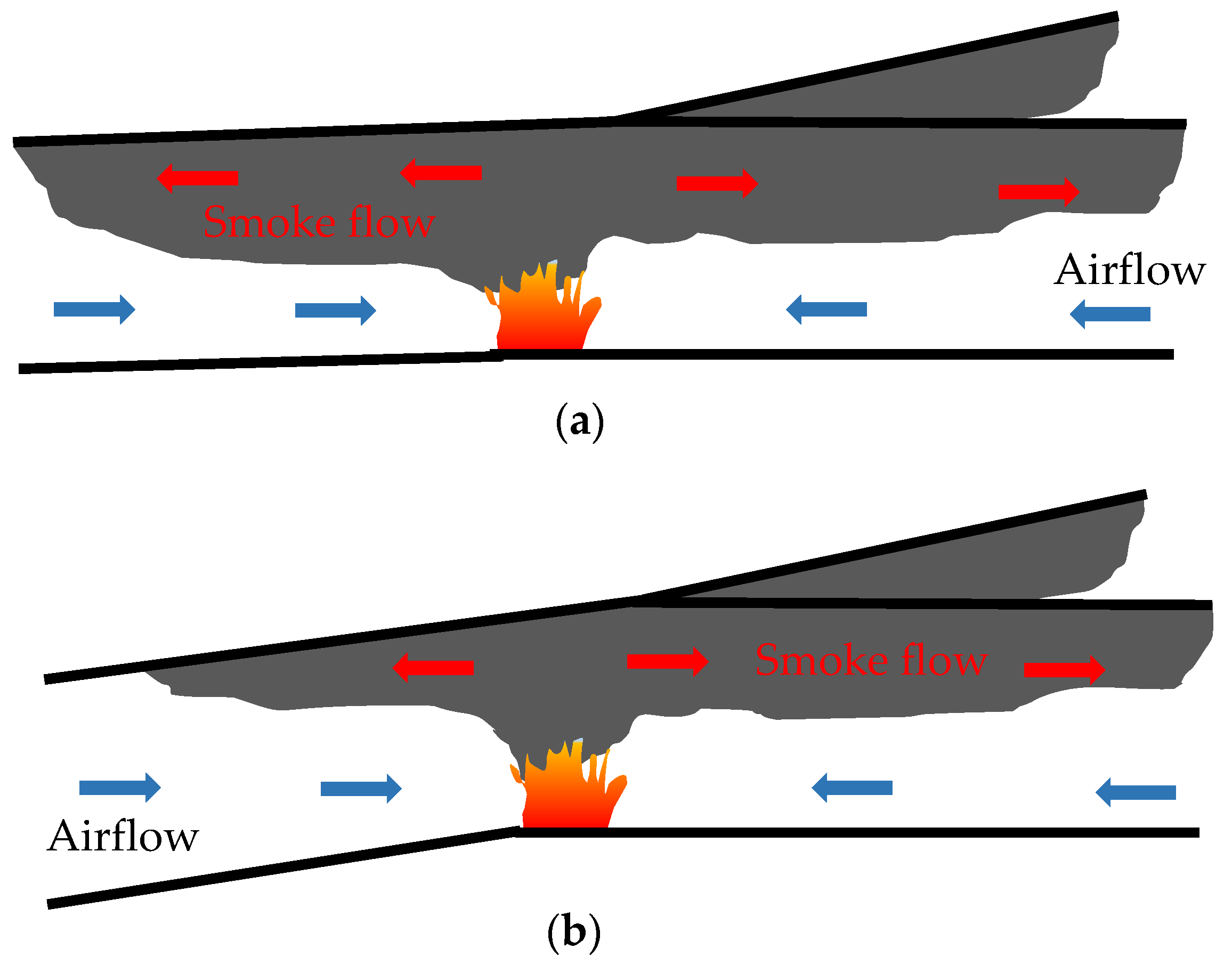
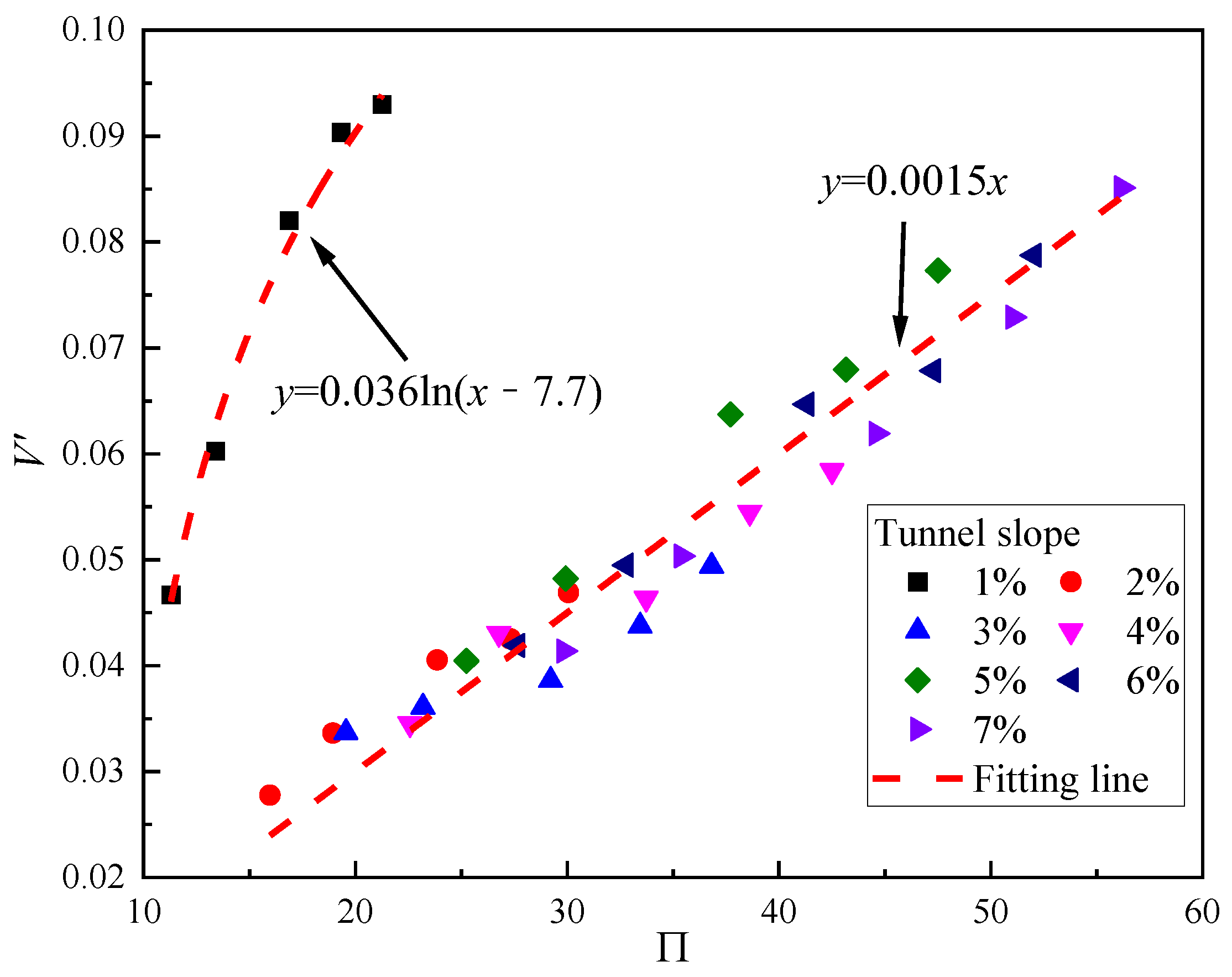
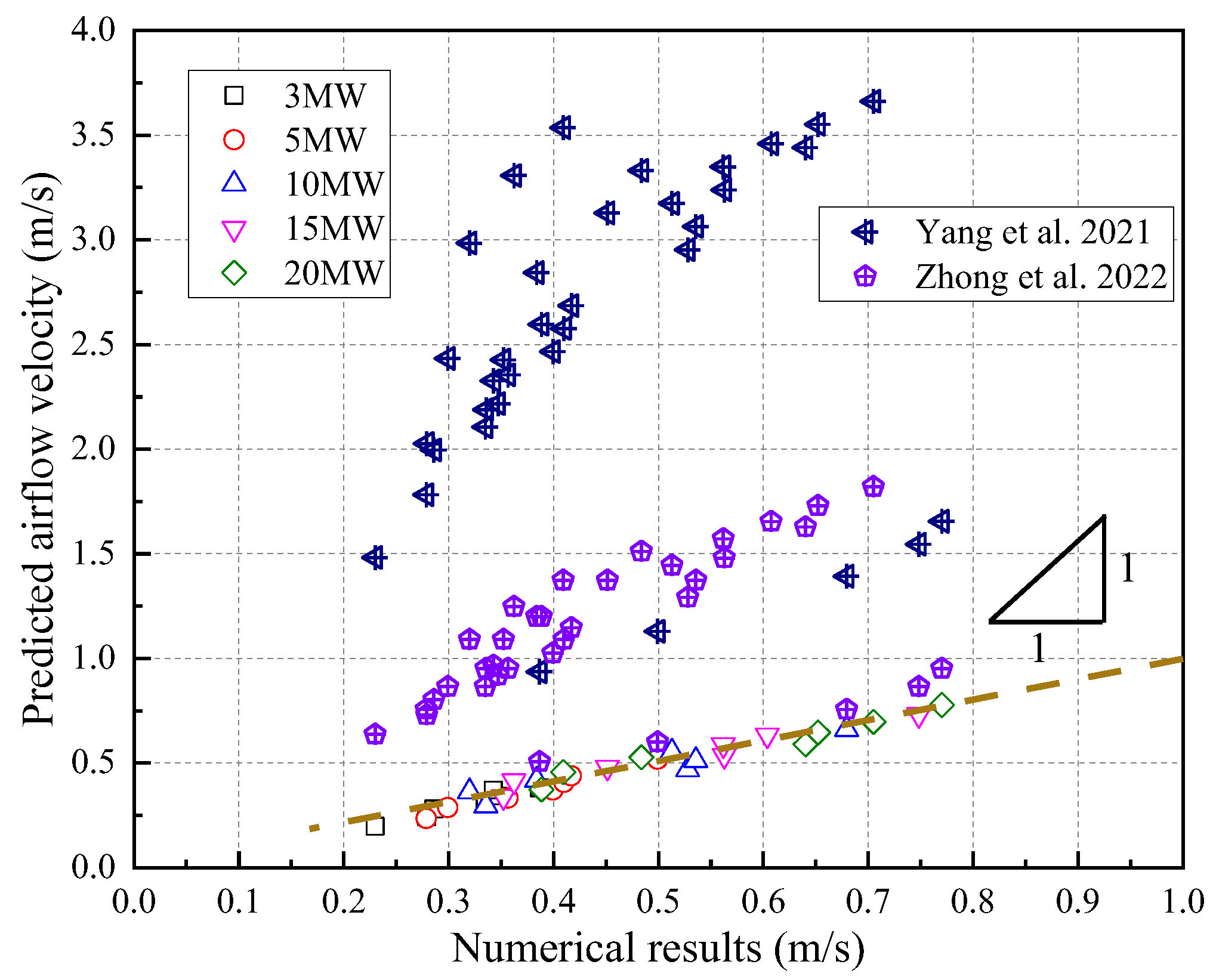
4.1. Induced Velocity in Sloped Mainline
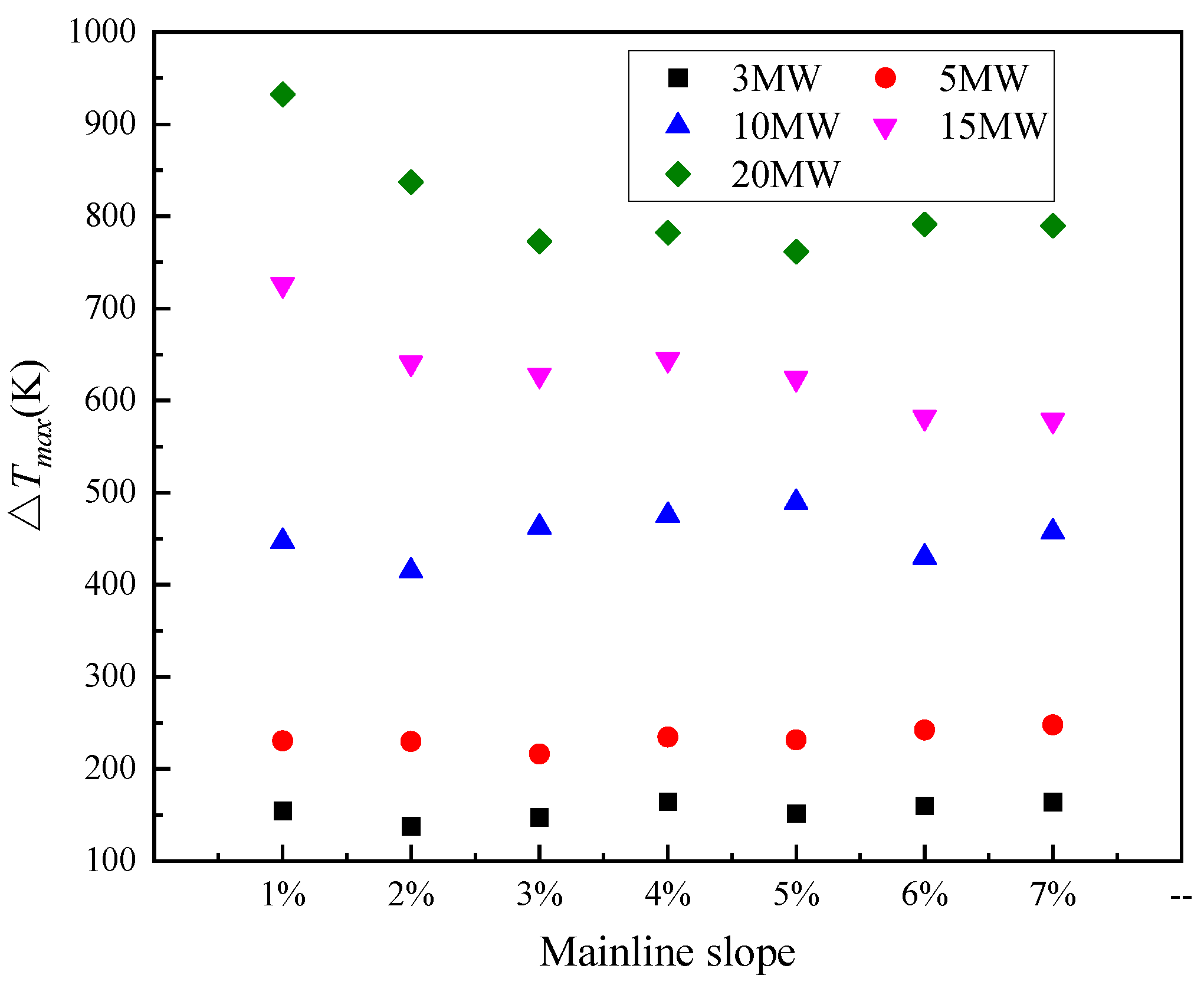
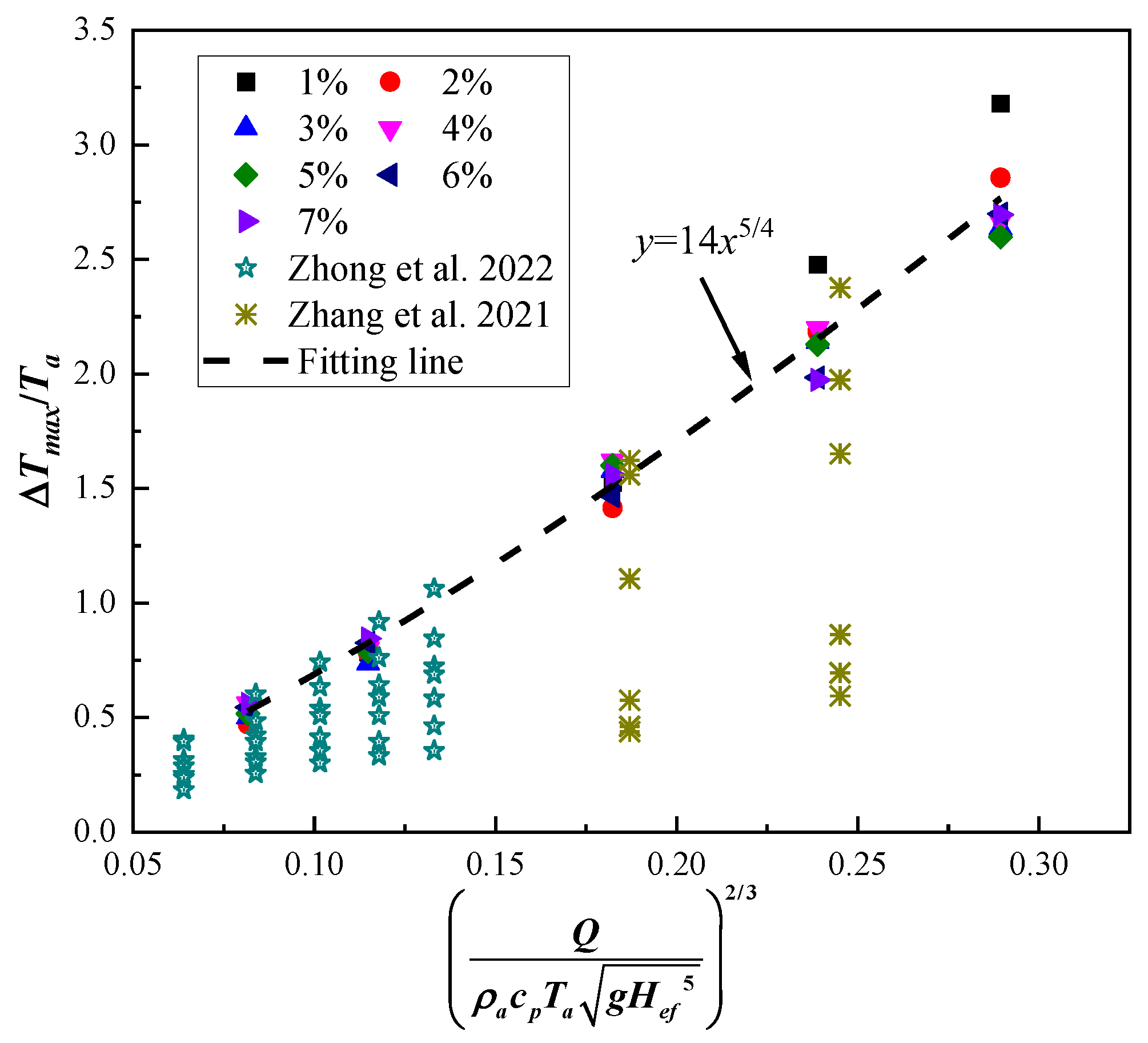
4.2. Maximum Temperature in Branched Tunnel
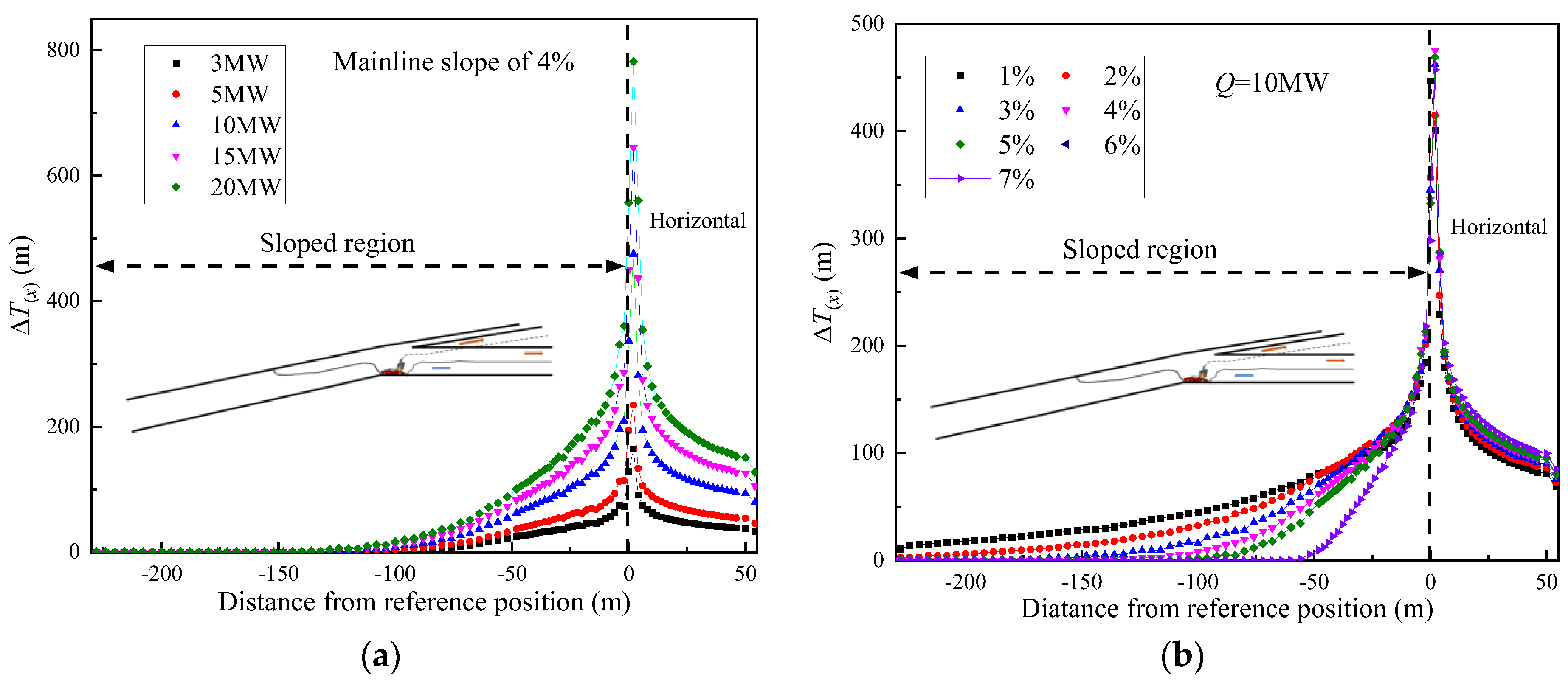
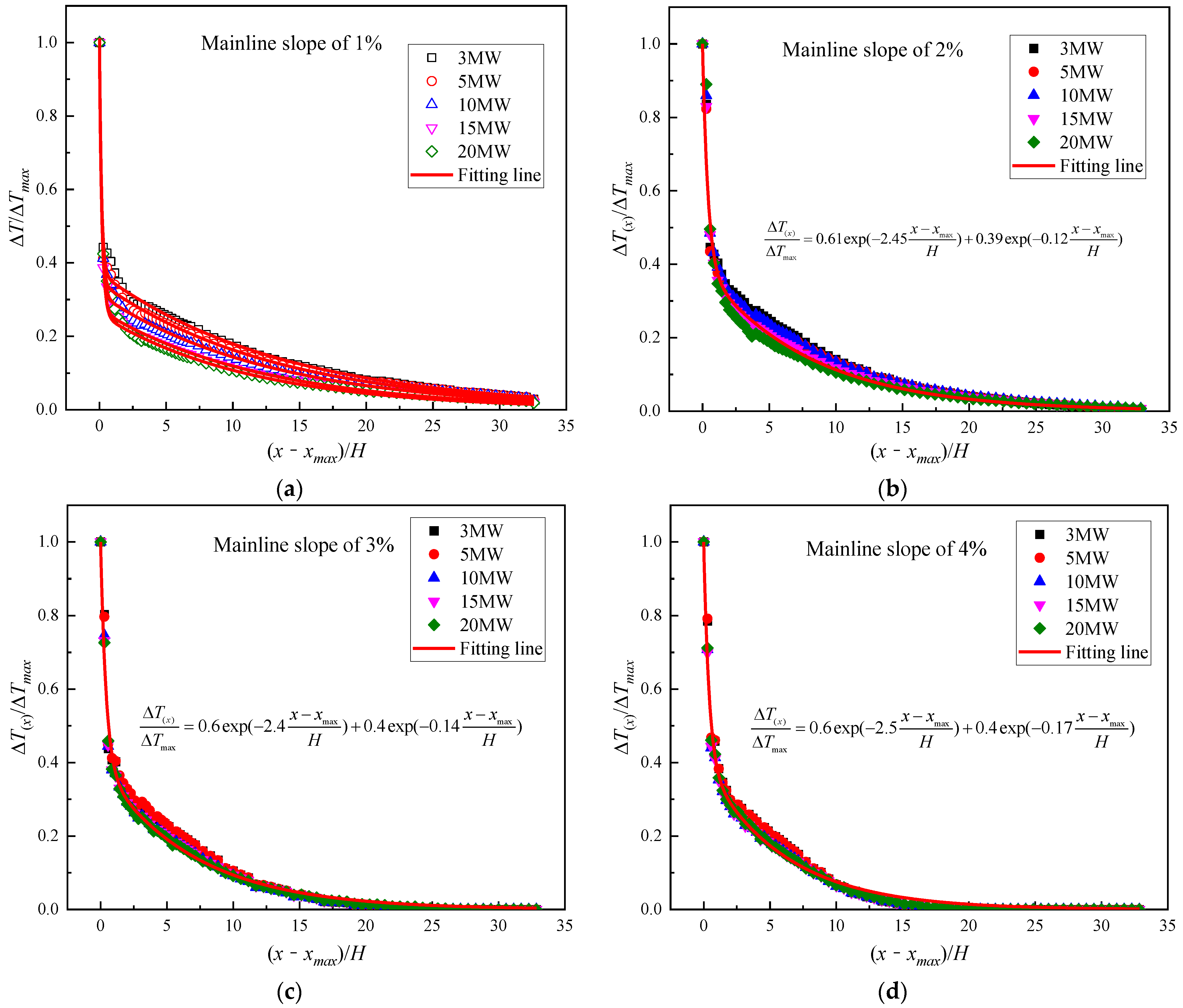
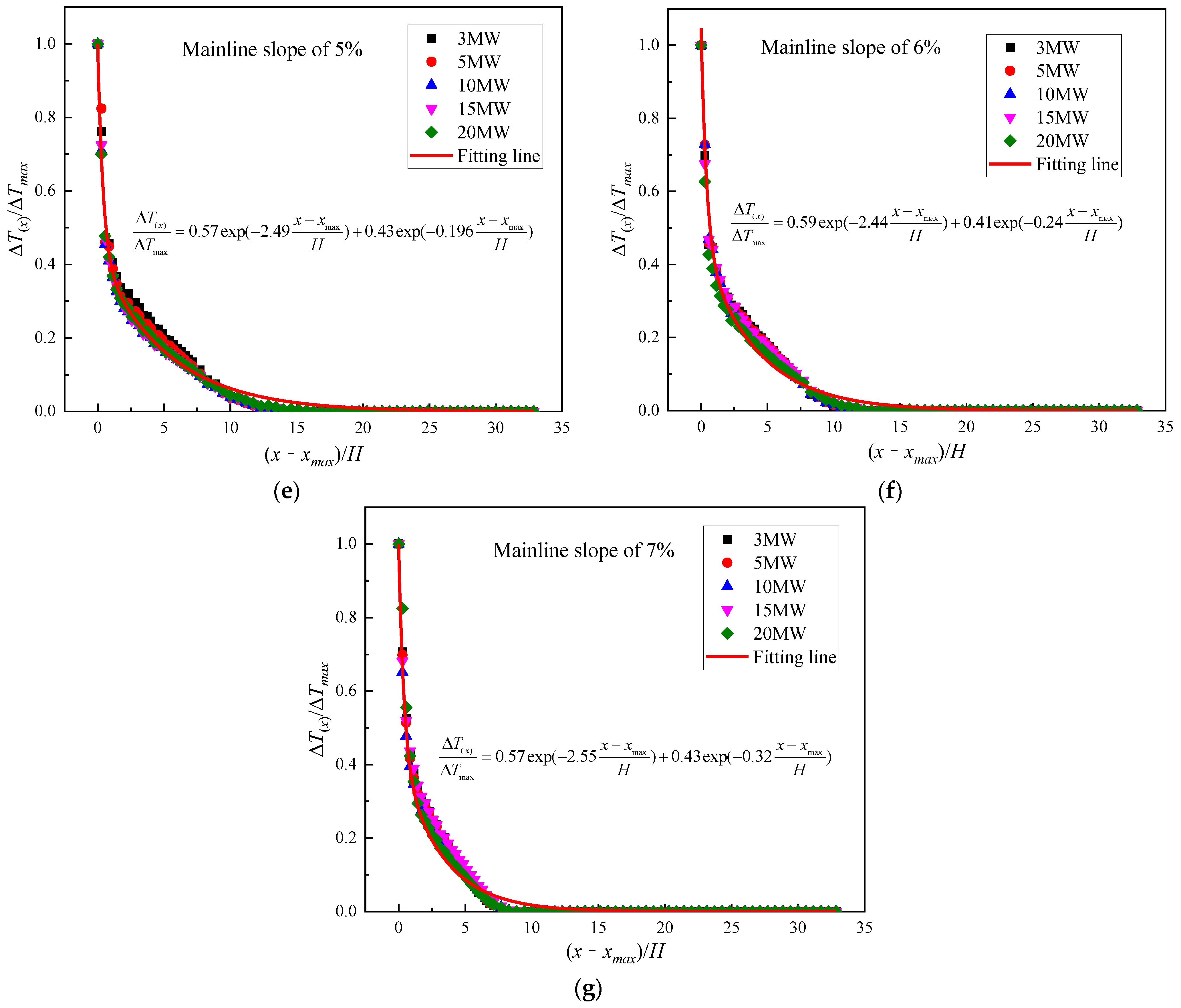
4.3. Temperature Longitudinal Decay in Sloped Mainline
5. Conclusions
- (1)
- The unidirectional airflow velocity in the inclined mainline region is induced during a slope larger than 1% due to the stack effect. The induced velocity increased with the upstream mainline slope that prevents the smoke reverse flow. A dimensionless expression is proposed to correlate the induced airflow velocity that shows a good linearly increasing for the mainline slope larger than 1%.
- (2)
- The effect of the mainline slope on the maximum temperature beneath the tunnel ceiling is limited, especially for relatively small fire power. The dimensionless maximum temperature can be well collapsed using Qef*2/3 but is independent on a mainline slope. The growth rate of maximum temperature is divided into two parts by a slope of 1%. A two-piecewise formula is developed for the maximum temperature beneath the ceiling in the branched tunnel with an inclined upstream mainline.
- (3)
- The mainline slope before shunting significantly affects the temperature longitudinal decay in the inclined mainline region, which can be well correlated using the sum of two exponential functions. The attenuation coefficients relate to heat release rate under a 1% slope, but it is independent of fire power during a slope larger than 1%. The empirical model is proposed to predict the longitudinal ceiling temperature in the sloped mainline region. This study contributes to the understanding of smoke temperature profiles in naturally branched tunnels with negative mainlines and guides extraction design.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Abbreviations
| A1, A2 | constant coefficient | ΔT(x) | ceiling excess temperature (K) |
| B1, B2 | coefficients varied with different branched tunnel region | ΔTmax | maximum ceiling temperature rise (K) |
| cp | specific heat of air (kJ/kg·K) | ui | smoke movement velocity in tunnel (m/s) |
| CT | coefficient account for bifurcation structure | U’ | non-dimensional ventilation velocity |
| d | thickness of smoke layer (m) | ve | induced velocity (m/s) |
| D* | characteristic fire diameter | vbuo | airflow velocity induced by buoyancy (m/s) |
| Ds | hydraulic diameter of smoke layer (m) | v | ventilation velocity (m/s) |
| f | full size parameter | V’ | dimensionless induced velocity |
| g | gravitational acceleration (m/s2) | w* | characteristic plume velocity (m/s) |
| h | height difference between inclined mainline two ends (m) | x | axis distance (m) |
| Hef | effective tunnel height (m) | xmax | position of maximum temperature (m) |
| H | tunnel height (m) | ||
| kr | coefficient induced by branched structure | Greek symbols | |
| Ku | temperature exponential decay coefficient | △ | difference |
| lu | smoke back-layering length at upstream (m) | θ | bifurcation angle (°) |
| li | length of relative tunnel region (m) | ρa | ambient density (kg/m3) |
| L | length between inclined mainline two ends (m) | ρsmoke | density of smoke layer (kg/m2) |
| Pa, Pb, Pc | kinetic energy in mainline before shunting, mainline after shunting, and ramp (Pa) | Δρ | density difference (kg/m3) |
| Pi | dynamic pressure of smoke movement in difference tunnel region (Pa) | β | tunnel slope angle in degree |
| ΔPe | pressure difference induced by asymmetric entrainment (Pa) | λ | friction coefficient |
| ΔPbuo | pressure difference induced by buoyancy (Pa) | ||
| ΔPstack | stack pressure difference (Pa) | Subscripts and Superscripts | |
| ΔPstack, u | pressure difference induced by stack effect between smoke stagnation and joint node (Pa) | a | ambient |
| Q | heat release rate (kW) | buo | buoyancy |
| Qef | heat release rate for effective tunnel height | e | entrainment |
| Q* | dimensionless heat release rate | ef | effective |
| Qef* | dimensionless heat release rate based on the effective tunnel height | i | relative tunnel region |
| r | radius of fire source (m) | s | smoke |
| Ta | ambient temperature (K) | stack, u | stack effect at upstream |
| ΔT | longitudinal temperature rise (K) | u | upstream |
References
- Deng, Y.W.; Chen, C.; Li, Q.; Hu, Q.Q.; Yuan, H.T.; Li, J.M.; Li, Y. Measurements of real-world vehicle CO and NOx fleet average emissions in urban tunnels of two cities in China. Atmos. Environ. 2015, 122, 417–426. [Google Scholar] [CrossRef]
- Meng, N.; Shu, Y.M.; Zhang, S.H. Study on smoke temperature induced by two fires in a naturally ventilated tunnel. Tunn. Undergr. Space Technol. 2023, 131, 104774. [Google Scholar] [CrossRef]
- Wan, H.X.; Jiang, Y.J.; Jiang, J.P. A survey of fire accidents during the process of highway tunnel operation in China from 2010 to 2021: Characteristics and countermeasures. Tunn. Undergr. Space Technol. 2023, 139, 105237. [Google Scholar] [CrossRef]
- Caliendo, C.; Genovese, G.; Russo, I. A 3D CFD modeling for assessing the effects of both longitudinal slope and traffic volume on user safety within a naturally ventilated road tunnel in the event of a fire accident. IATSS Res. 2022, 46, 547–558. [Google Scholar] [CrossRef]
- Yang, D.; Liu, Y.L.; Zhao, C.M.; Mao, S.H. Multiple steady states of fire smoke transport in a multi-branch tunnel: Theoretical and numerical studies. Tunn. Undergr. Space Technol. 2017, 61, 189–197. [Google Scholar] [CrossRef]
- Li, Q.; Chen, C.; Deng, Y.W.; Li, J.M.; Xie, G.Y.; Li, Y.; Hu, Q.Q. Influence of traffic force on pollutant dispersion of CO, NO and particle matter (PM2.5) measured in an urban tunnel in Changsha, China. Tunn. Undergr. Space Technol. 2015, 49, 400–407. [Google Scholar] [CrossRef]
- Du, T.; Yang, D.; Peng, S.N.; Xiao, Y.M. A method for design of smoke control of urban traffic link tunnel (UTLT) using longitudinal ventilation. Tunn. Undergr. Space Technol. 2015, 48, 35–42. [Google Scholar] [CrossRef]
- Li, Q.; Chen, C.; Yuan, H.T.; Wang, L.Y.; Xu, S.H.; Li, Y.R. Prediction of pollutant concentration and ventilation control in urban bifurcate tunnel, China. Tunn. Undergr. Space Technol. 2018, 82, 406–415. [Google Scholar] [CrossRef]
- Liu, C.; Zhong, M.H.; Tian, X.L.; Zhang, P.H.; Xiao, Y.; Mei, Q. Experimental and numerical study on fire-induced smoke temperature in connected area of metro tunnel under natural ventilation. Int. J. Therm. Sci. 2019, 138, 84–97. [Google Scholar] [CrossRef]
- Jiang, L.; Creyssels, M.; Hunt, G.R.; Salizzoni, P. Control of light gas releases in ventilated tunnels. J. Fluid Mech. 2019, 872, 515–531. [Google Scholar] [CrossRef]
- Alpert, R.L. Calculation of response time of ceiling-mounted fire detectors. Fire Technol. 1972, 8, 181–195. [Google Scholar] [CrossRef]
- Alpert, R.L. Turbulent ceiling-jet induced by large-scale fires. Combust. Sci. Technol. 1975, 11, 197–213. [Google Scholar] [CrossRef]
- Kurioka, H.; Oka, Y.; Satoh, H.; Sugawa, O. Fire properties in near field of square fire source with longitudinal ventilation in tunnels. Fire Saf. J. 2003, 38, 319–340. [Google Scholar] [CrossRef]
- Hu, L.H.; Huo, R.; Peng, W.; Chow, W.K.; Yang, R.X. On the maximum smoke temperature under the ceiling in tunnel fires. Tunn. Undergr. Space Technol. 2006, 21, 650–655. [Google Scholar] [CrossRef]
- Li, Y.Z.; Lei, B.; Ingason, H. The maximum temperature of buoyancy-driven smoke flow beneath the ceiling in tunnel fires. Fire Saf. J. 2011, 46, 204–210. [Google Scholar] [CrossRef]
- Salizzoni, P.; Peruzzi, C.; Marro, M.; Cingi, P.; Angeli, D.; Kubwimana, T.; Mos, A. Measurements and scaling of buoyancy-induced flows in ventilated tunnels. Flow 2023, 3, E15. [Google Scholar] [CrossRef]
- Tang, F.; Mei, F.Z.; Wang, Q.; He, Z.; Fan, C.G.; Tao, C.F. Maximum temperature beneath the ceiling in tunnel fires with combination of ceiling mechanical smoke extraction and longitudinal ventilation. Tunn. Undergr. Space Technol. 2017, 68, 231–237. [Google Scholar] [CrossRef]
- Zhou, T.N.; He, Y.P.; Lin, X.; Wang, X.H.; Wang, J. Influence of constraint effect of sidewall on maximum smoke temperature distribution under a tunnel ceiling. Appl. Therm. Eng. 2017, 112, 932–941. [Google Scholar] [CrossRef]
- Zhou, T.N.; Li, H.H.; Chen, Q.P.; Wei, R.C.; Wang, J. Understanding sidewall constraint involving ventilation effects on temperature distribution of fire-induced thermal flow under a tunnel ceiling. Int. J. Therm. Sci. 2018, 129, 290–300. [Google Scholar] [CrossRef]
- Hu, L.H.; Huo, R.; Li, Y.Z.; Wang, H.B.; Chow, W.K. Full-scale burning tests on studying smoke temperature and velocity along a corridor. Tunn. Undergr. Space Technol. 2005, 20, 223–229. [Google Scholar] [CrossRef]
- Tang, F.; Cao, Z.L.; Chen, Q.; Meng, N.; Wang, Q.; Fan, C.G. Effect of blockage-heat source distance on maximum temperature of buoyancy-induced smoke flow beneath ceiling in a longitudinal ventilated tunnel. Int. J. Heat Mass Transf. 2017, 109, 683–688. [Google Scholar] [CrossRef]
- Chen, C.K.; Zhu, C.X.; Liu, X.Y.; Yu, N.H. Experimental investigation on the effect of asymmetrical sealing on tunnel fire behavior. Int. J. Heat Mass Transf. 2016, 92, 55–65. [Google Scholar] [CrossRef]
- Yao, Y.Z.; He, K.; Peng, M.; Shi, L.; Cheng, X.D.; Zhang, H.P. Maximum gas temperature rise beneath the ceiling in a portals-sealed tunnel fire. Tunn. Undergr. Space Technol. 2018, 80, 10–15. [Google Scholar] [CrossRef]
- Huang, Y.B.; Li, Y.F.; Dong, B.Y.; Li, J.M.; Liang, Q. Numerical investigation on the maximum ceiling temperature and longitudinal decay in a sealing tunnel fire. Tunn. Undergr. Space Technol. 2018, 72, 120–130. [Google Scholar] [CrossRef]
- Shi, J.K.; Zuo, C.; Xiong, Y.; Zhou, M.; Lin, P. Experimental study of different sealing ratios on the self-extinction of tunnel fires. Tunn. Undergr. Space Technol. 2021, 112, 103894. [Google Scholar] [CrossRef]
- Tang, F.; Cao, Z.L.; Palacios, A.; Wang, Q. A study on the maximum temperature of ceiling jet induced by rectangular-source fires in a tunnel using ceiling smoke extraction. Int. J. Therm. Sci. 2018, 127, 329–334. [Google Scholar] [CrossRef]
- Takeuchi, S.; Aoki, T.; Tanaka, F.; Moinuddin, K.A.M. Modeling for predicting the temperature distribution of smoke during a fire in an underground road tunnel with vertical shafts. Fire Saf. J. 2017, 91, 312–319. [Google Scholar] [CrossRef]
- Cong, H.Y.; Bi, M.S.; Bi, Y.B.; Jiang, H.P.; Li, Y.C.; Gao, W. Experimental and theoretical studies on the smoke temperature distribution along the tunnel ceiling with natural ventilation by the board-coupled shafts. Int. J. Therm. Sci. 2021, 159, 106639. [Google Scholar] [CrossRef]
- Oka, Y.; Imazeki, O. Temperature distribution within a ceiling jet propagating in an inclined flat-ceilinged tunnel with natural ventilation. Fire Saf. J. 2015, 71, 20–33. [Google Scholar] [CrossRef]
- Huo, Y.; Gao, Y.; Chow, W.K. A study on ceiling jet characteristics in an inclined tunnel. Tunn. Undergr. Space Technol. 2015, 50, 32–46. [Google Scholar] [CrossRef]
- Zhang, X.L.; Lin, Y.J.; Shi, C.L.; Zhang, J.P. Numerical simulation on the maximum temperature and smoke back-layering length in a tilted tunnel under natural ventilation. Tunn. Undergr. Space Technol. 2021, 107, 103661. [Google Scholar] [CrossRef]
- Hu, L.H.; Chen, L.F.; Wu, L.; Li, Y.F.; Zhang, J.Y.; Meng, N. An experimental investigation and correlation on buoyant gas temperature below ceiling in a slopping tunnel fire. Appl. Therm. Eng. 2013, 51, 246–254. [Google Scholar] [CrossRef]
- Ji, J.; Wang, Z.Y.; Ding, L.; Yu, L.X.; Gao, Z.H.; Wan, H.X. Effects of ambient pressure on smoke movement and temperature distribution in inclined tunnel fires. Int. J. Therm. Sci. 2019, 145, 106006. [Google Scholar] [CrossRef]
- Han, J.Q.; Liu, F.; Wang, F.; Weng, M.C.; Wang, J. Study on the smoke movement and downstream temperature distribution in a sloping tunnel with one closed portal. Int. J. Therm. Sci. 2020, 149, 106165. [Google Scholar] [CrossRef]
- Chen, C.K.; Nie, Y.L.; Zhang, Y.L.; Lei, P.; Fan, C.G.; Wang, Z.Y. Experimental investigation on the influence of ramp slope on fire behaviors in a bifurcated tunnel. Tunn. Undergr. Sp. Technol. 2020, 104, 103522. [Google Scholar] [CrossRef]
- Huang, Y.B.; Li, Y.F.; Li, J.M.; Li, J.X.; Wu, K.; Zhu, K.; Li, H.H. Experimental investigation on maximum gas temperature beneath the ceiling in a branched tunnel fire. Int. J. Therm. Sci. 2019, 145, 105997. [Google Scholar] [CrossRef]
- Huang, Y.B.; Li, Y.F.; Li, J.X.; Wu, K.; Li, H.H.; Zhu, K. Experimental study on the temperature longitudinal distribution induced by a branched tunnel fire. Int. J. Therm. Sci. 2021, 170, 107175. [Google Scholar] [CrossRef]
- Lei, P.; Chen, C.K.; Zhang, Y.L.; Xu, T.; Sun, H.K. Experimental study on temperature profile in a branched tunnel fire under natural ventilation considering different fire locations. Int. J. Therm. Sci. 2021, 159, 106631. [Google Scholar] [CrossRef]
- Lei, P.; Chen, C.K.; Zhao, D.Y.; Zhang, Y.L.; Xu, T.; Jiao, W.B. Study on heat allocation and temperature profile in a T-shaped branched tunnel fire with different branch slopes under natural ventilation. Tunn. Undergr. Space Technol. 2022, 126, 104508. [Google Scholar] [CrossRef]
- Liu, C.; Zhong, M.H.; Shi, C.L.; Zhang, P.H.; Tian, X.L. Temperature profile of fire-induced smoke in node area of a full-scale mine shaft tunnel under natural ventilation. Appl. Therm. Eng. 2017, 110, 382–389. [Google Scholar] [CrossRef]
- Li, Z.S.; Gao, Y.J.; Li, X.S.; Mao, P.F.; Zhang, Y.C.; Jin, K.Y.; Li, T.; Chen, L.F. Effects of transverse fire locations on flame length and temperature distribution in a bifurcated tunnel fire. Tunn. Undergr. Space Technol. 2021, 112, 103893. [Google Scholar] [CrossRef]
- Liang, K.; Hao, X.F.; An, W.G.; Tang, Y.H.; Cong, Y.Z. Study on cable fire spread and smoke temperature distribution in T-shaped utility tunnel. Case Stud. Therm. Eng. 2019, 14, 100433. [Google Scholar] [CrossRef]
- Cheng, H.H.; Liu, C.; Chen, J.F.; Wu, L.; Zhao, Y.T.; Zhong, M.H. Full-scale experimental study on fire under natural ventilation in the T-shaped and curved tunnel groups. Tunn. Undergr. Space Technol. 2022, 123, 104442. [Google Scholar] [CrossRef]
- Huang, Y.B.; Liu, X.; Dong, B.Y.; Zhong, H.; Wang, B.; Dong, Q.W. Effect of inclined mainline on smoke back-layering length in a naturally branched tunnel fire. Tunn. Undergr. Space Technol. 2023, 134, 104985. [Google Scholar] [CrossRef]
- Gao, Z.H.; Liu, Z.X.; Ji, J.; Fan, C.G.; Li, L.J.; Sun, J.H. Experimental study of tunnel sidewall effect on flame characteristics and air entrainment factor of methanol pool fires. Appl. Therm. Eng. 2016, 102, 1314–1319. [Google Scholar] [CrossRef]
- Huang, Y.B.; Li, Y.F.; Li, J.X.; Wu, K.; Li, H.H.; Zhu, K.; Li, J.M. Experimental investigation of the thermal back-layering length in a branched tunnel fire under longitudinal ventilation. Int. J. Therm. Sci. 2022, 173, 107415. [Google Scholar] [CrossRef]
- Blanchard, E.; Boulet, P.; Desanghère, S.; Carlotti, P. Energy balance in a tunnel fire: Midscale tests and CFD simulations. ISAT 2011, 14, 349–362. [Google Scholar]
- Yao, Y.Z.; Li, Y.Z.; Ingason, H.; Cheng, X.D. Numerical study on overall smoke control using naturally ventilated shafts during fires in a road tunnel. Int. J. Therm. Sci. 2019, 140, 491–504. [Google Scholar] [CrossRef]
- Deng, T.; Norris, S.; Sharma, R.N. Numerical study of the flame geometry of pool fires in longitudinally ventilated tunnels. Tunn. Undergr. Space Technol. 2023, 132, 104882. [Google Scholar] [CrossRef]
- Wang, Z.Y.; Ding, L.; Wan, H.X.; Ji, J.; Gao, Z.H.; Yu, L.X. Numerical investigation on the effect of tunnel width and slope on ceiling gas temperature in inclined tunnels. Int. J. Therm. Sci. 2020, 152, 106272. [Google Scholar] [CrossRef]
- Gao, Z.H.; Li, L.J.; Sun, C.P.; Zhong, W.; Yan, C.B. Effect of longitudinal slope on the smoke propagation and ceiling temperature characterization in sloping tunnel fires under natural ventilation. Tunn. Undergr. Space Technol. 2022, 123, 104396. [Google Scholar] [CrossRef]
- Yang, Y.X.; Long, Z.; Cheng, H.H.; Chen, J.F.; Liu, C.; Zhong, M.H. Experimental and numerical study of smoke temperature distribution characteristics in a sloped tunnel. Sustain. Cities Soc. 2021, 73, 103091. [Google Scholar] [CrossRef]
- Zhou, Y.; Yang, Y.; Mao, Z.L.; Bu, R.W.; Gong, J.H.; Wang, Y.X.; Yi, L. Analytical and numerical study on natural ventilation performance in single- and gable-slope city tunnels. Sustain. Cities Soc. 2019, 45, 258–270. [Google Scholar] [CrossRef]
- Zhong, W.; Liu, L.; Han, N.; Gao, Z.H. Investigation on the maximum ceiling temperature of the weak plume impingement flow in tunnel fires under longitudinal ventilation. Tunn. Undergr. Sp. Technol. 2022, 123, 104396. [Google Scholar] [CrossRef]

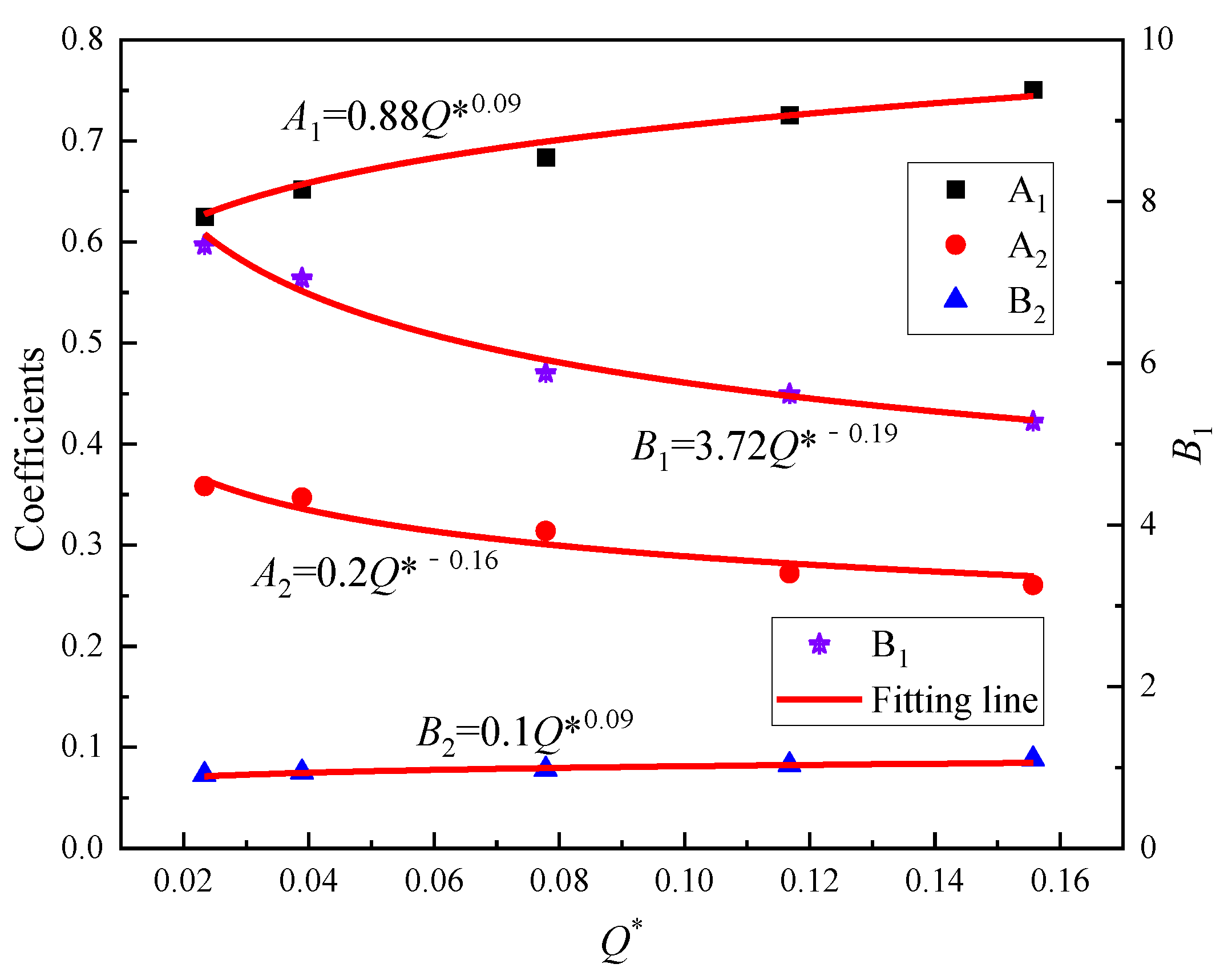
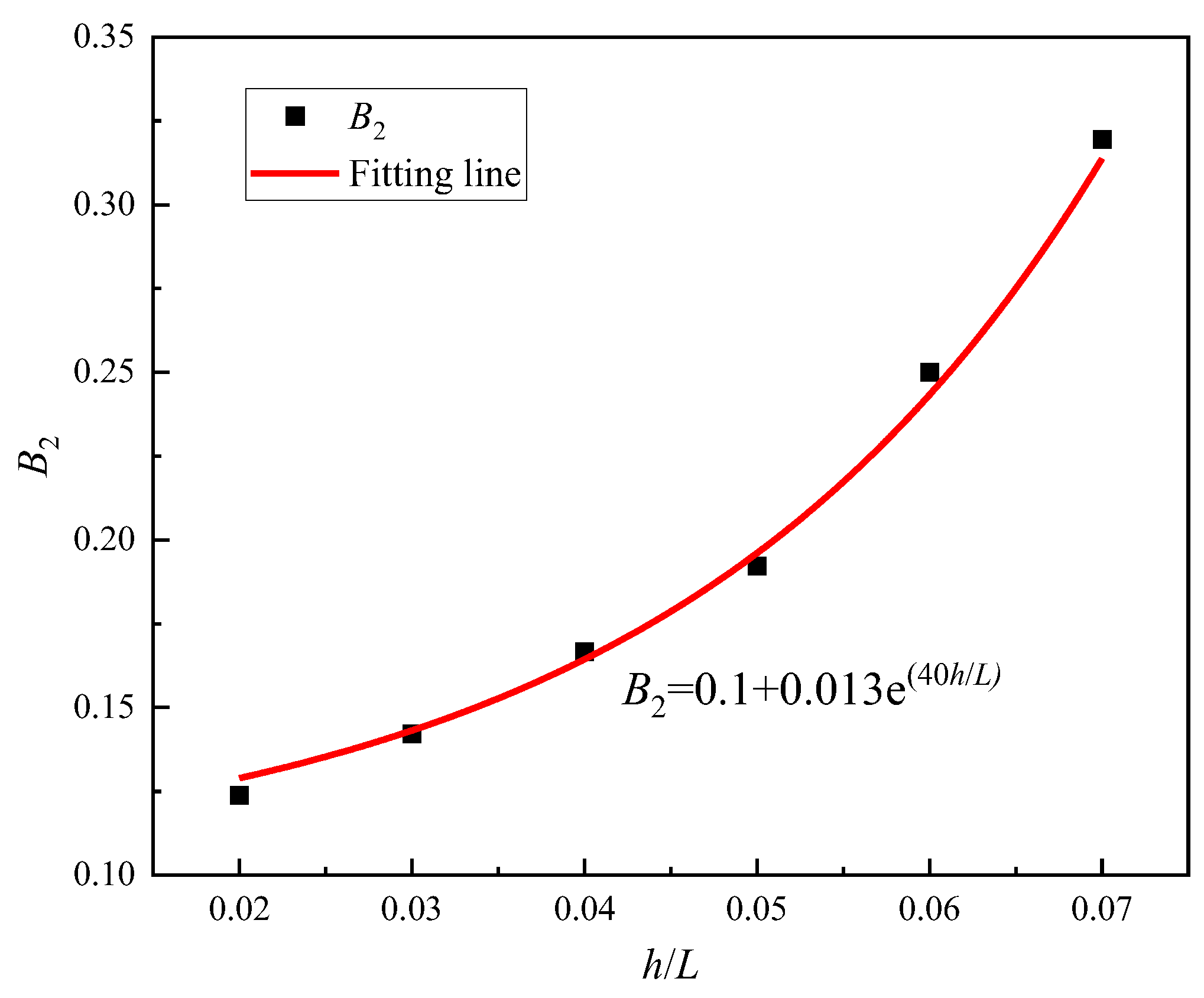
| No. | Heat Release Rate (MW) | Slope (%) |
|---|---|---|
| 1–7 | 3 | 1, 2, 3, 4, 5, 6, 7 |
| 8–14 | 5 | |
| 15–21 | 10 | |
| 22–28 | 15 | |
| 29–35 | 20 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lu, N.; Yao, X.; Yang, J.; Huang, Y. Evaluating the Ceiling Gas Temperature in a Branched Tunnel Fire with a Sloped Mainline Region under Natural Ventilation. Fire 2024, 7, 152. https://doi.org/10.3390/fire7050152
Lu N, Yao X, Yang J, Huang Y. Evaluating the Ceiling Gas Temperature in a Branched Tunnel Fire with a Sloped Mainline Region under Natural Ventilation. Fire. 2024; 7(5):152. https://doi.org/10.3390/fire7050152
Chicago/Turabian StyleLu, Ning, Xiaolin Yao, Jinming Yang, and Youbo Huang. 2024. "Evaluating the Ceiling Gas Temperature in a Branched Tunnel Fire with a Sloped Mainline Region under Natural Ventilation" Fire 7, no. 5: 152. https://doi.org/10.3390/fire7050152
APA StyleLu, N., Yao, X., Yang, J., & Huang, Y. (2024). Evaluating the Ceiling Gas Temperature in a Branched Tunnel Fire with a Sloped Mainline Region under Natural Ventilation. Fire, 7(5), 152. https://doi.org/10.3390/fire7050152





