Reliability Analysis of a Building Real Fire Simulation Training System
Abstract
:1. Introduction
2. Analysis of Basic Reliability Theory
2.1. Classification of Reliability Systems
2.2. System Reliability Indicators
2.2.1. Reliability [29,30]
2.2.2. Failure Rate [30]
2.2.3. Average Lifetime
2.3. Reliability of the Irreparable System
3. Structural Analysis and Reliability Model Establishment of the Building Real Fire Simulation Training System
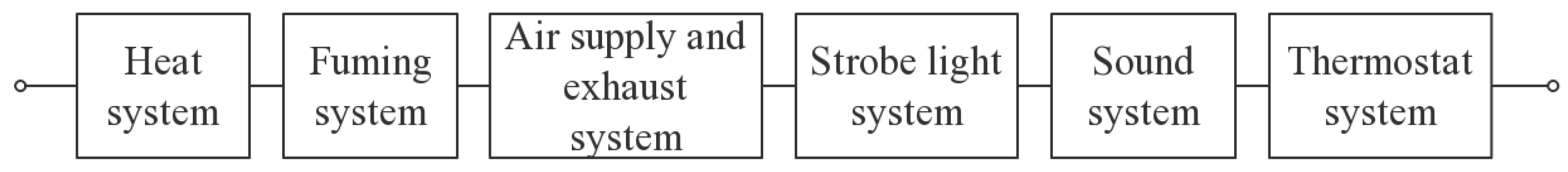
3.1. Structural Analysis of the Fume–Heat Training Room
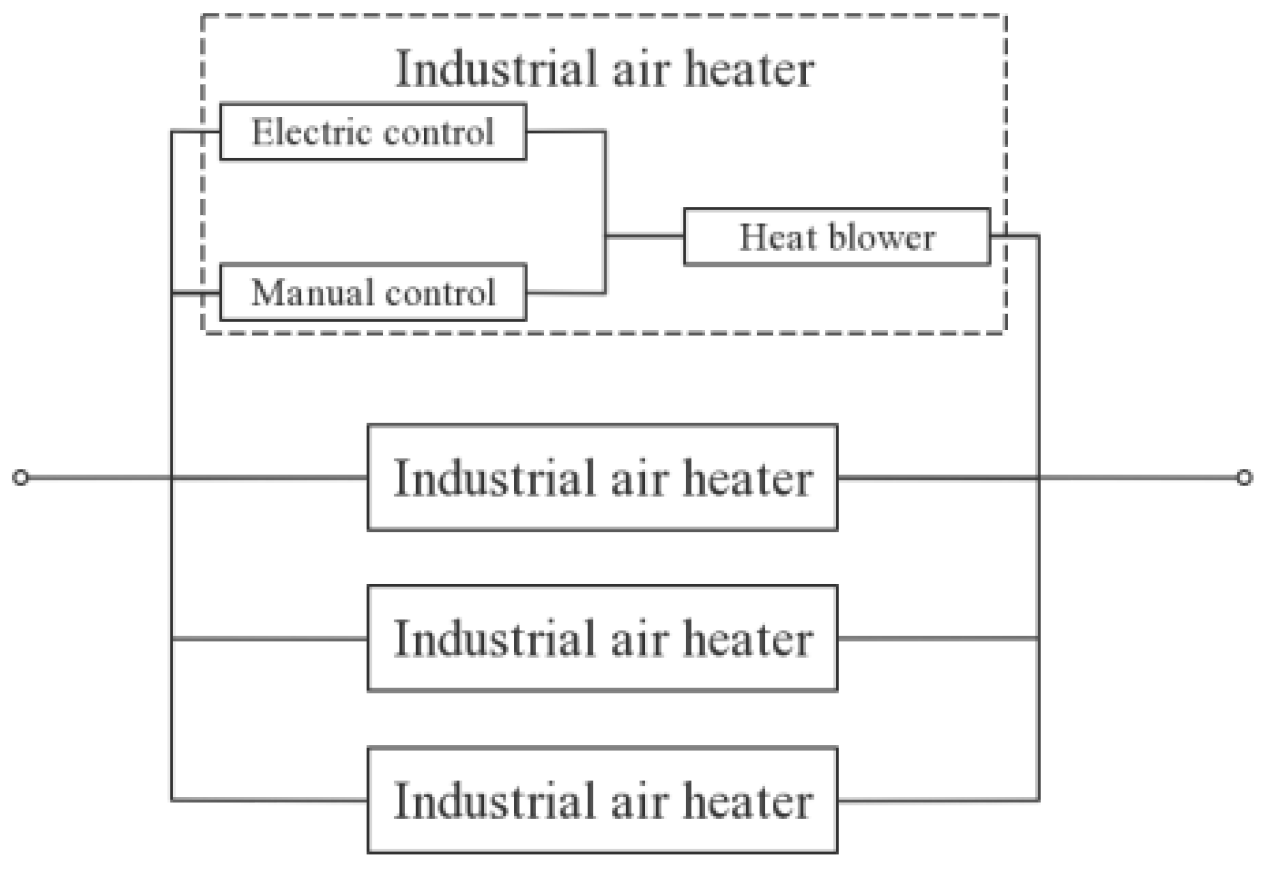
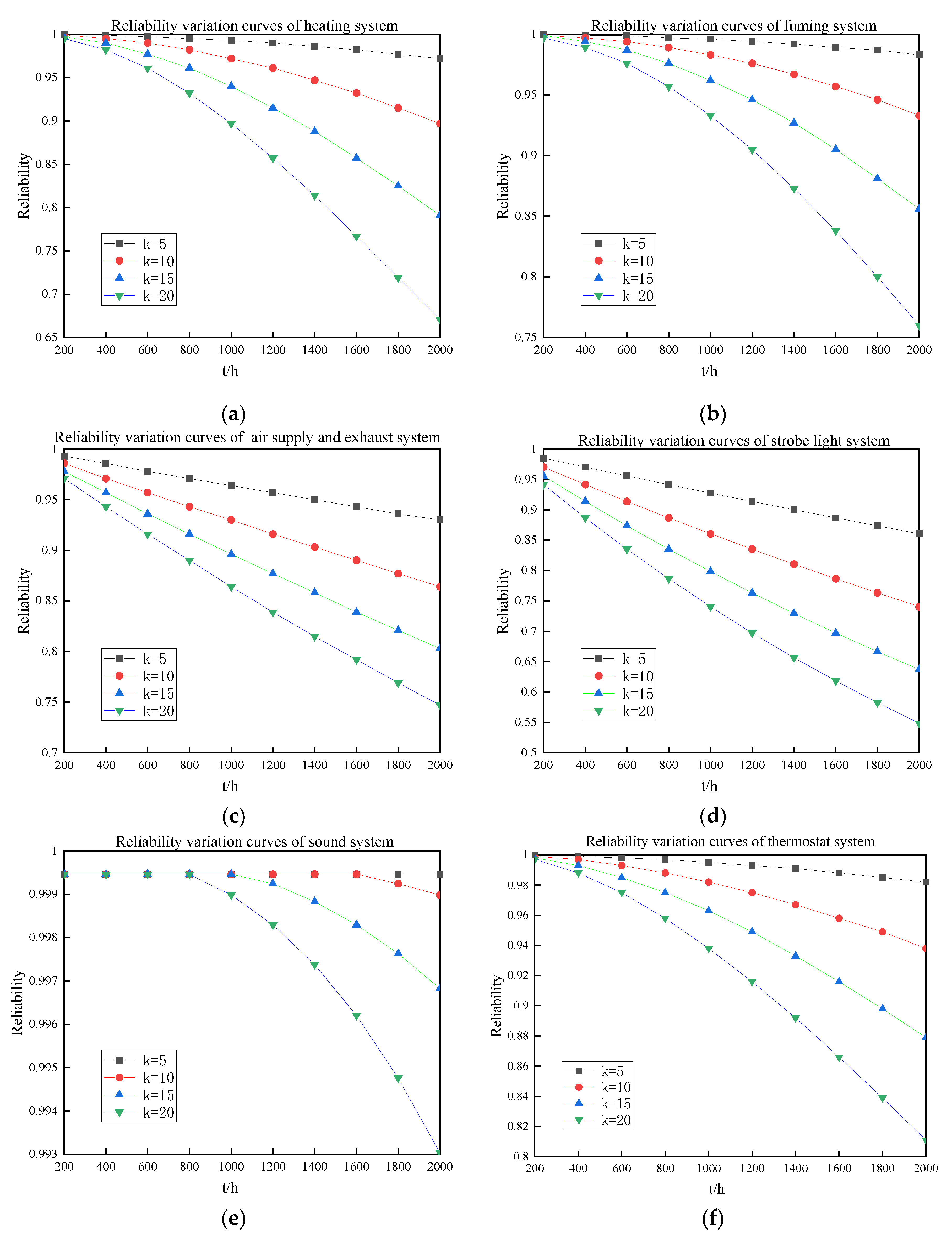
3.1.1. Heating System
3.1.2. Fuming System
3.1.3. Air Supply and Exhaust System
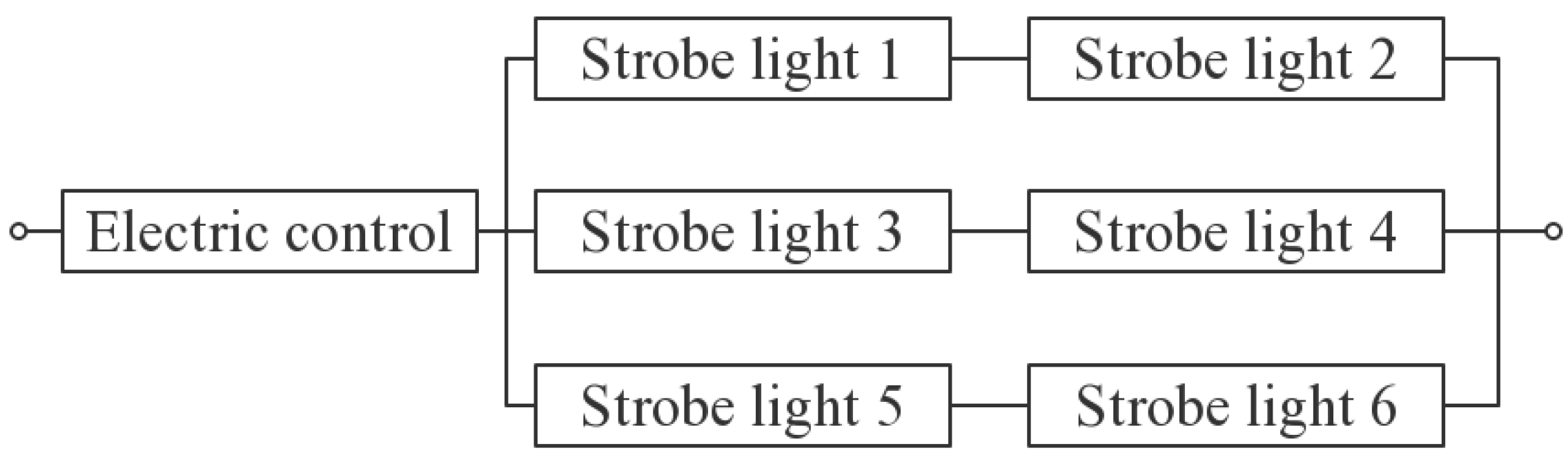
3.1.4. Strobe Light System
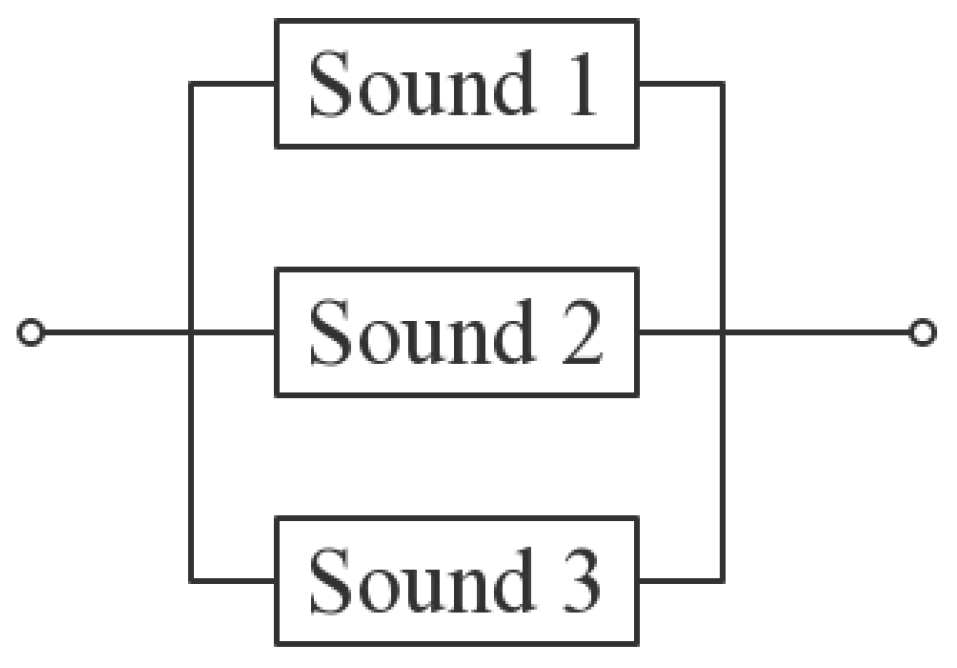
3.1.5. Sound System
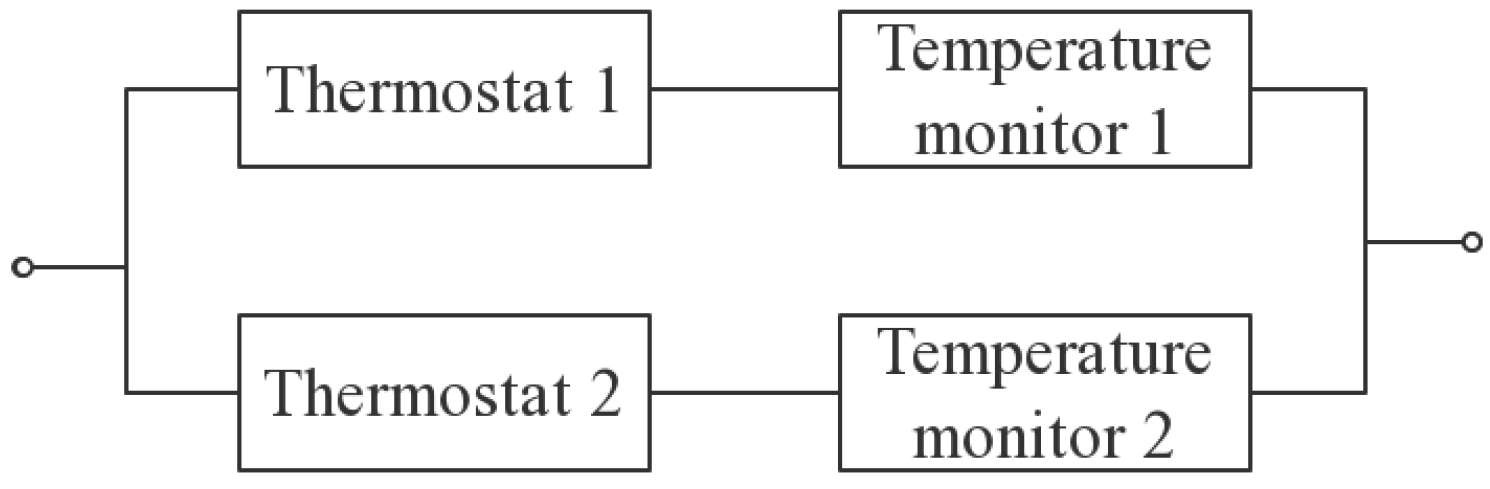
3.1.6. Thermostat System
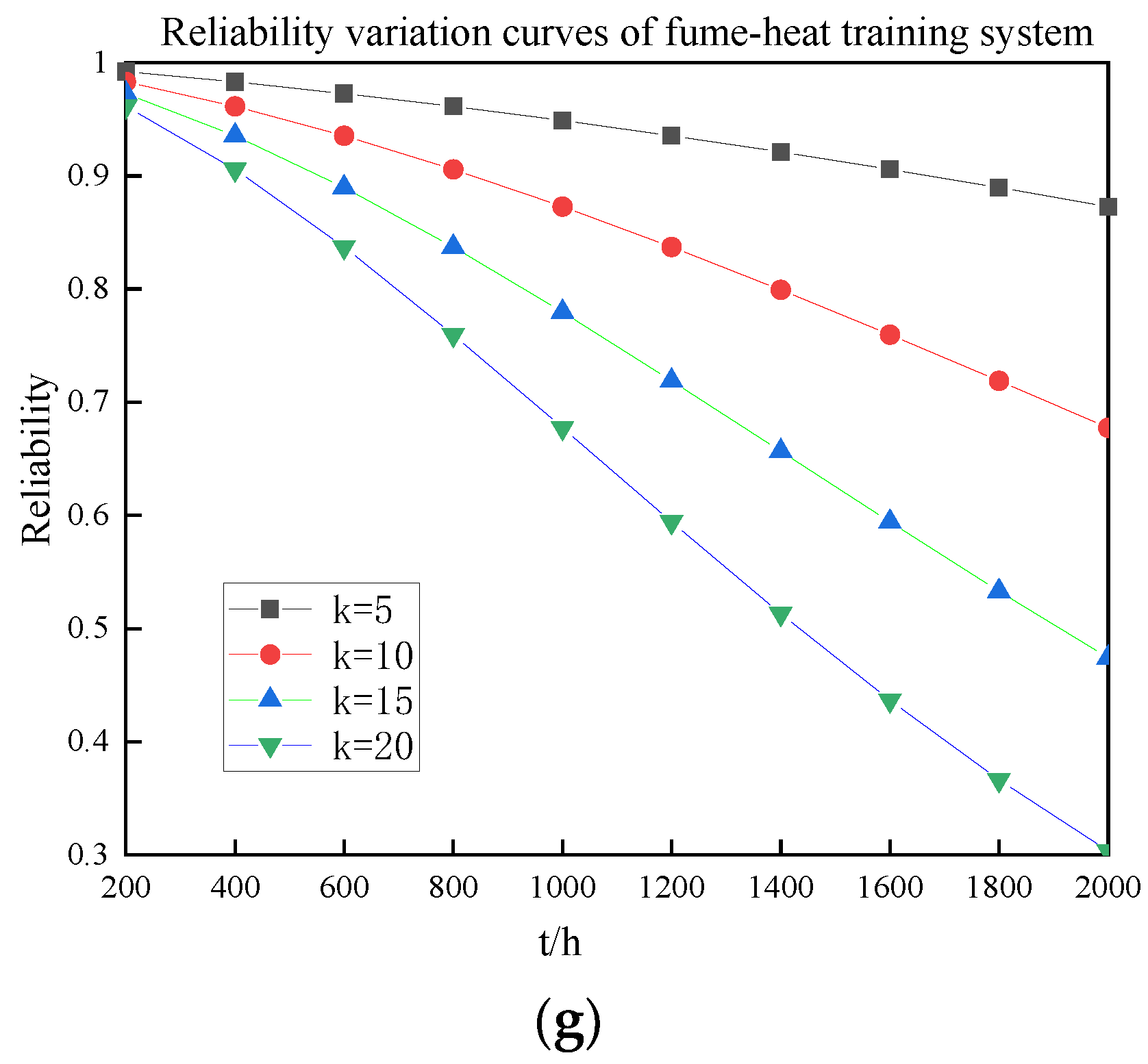
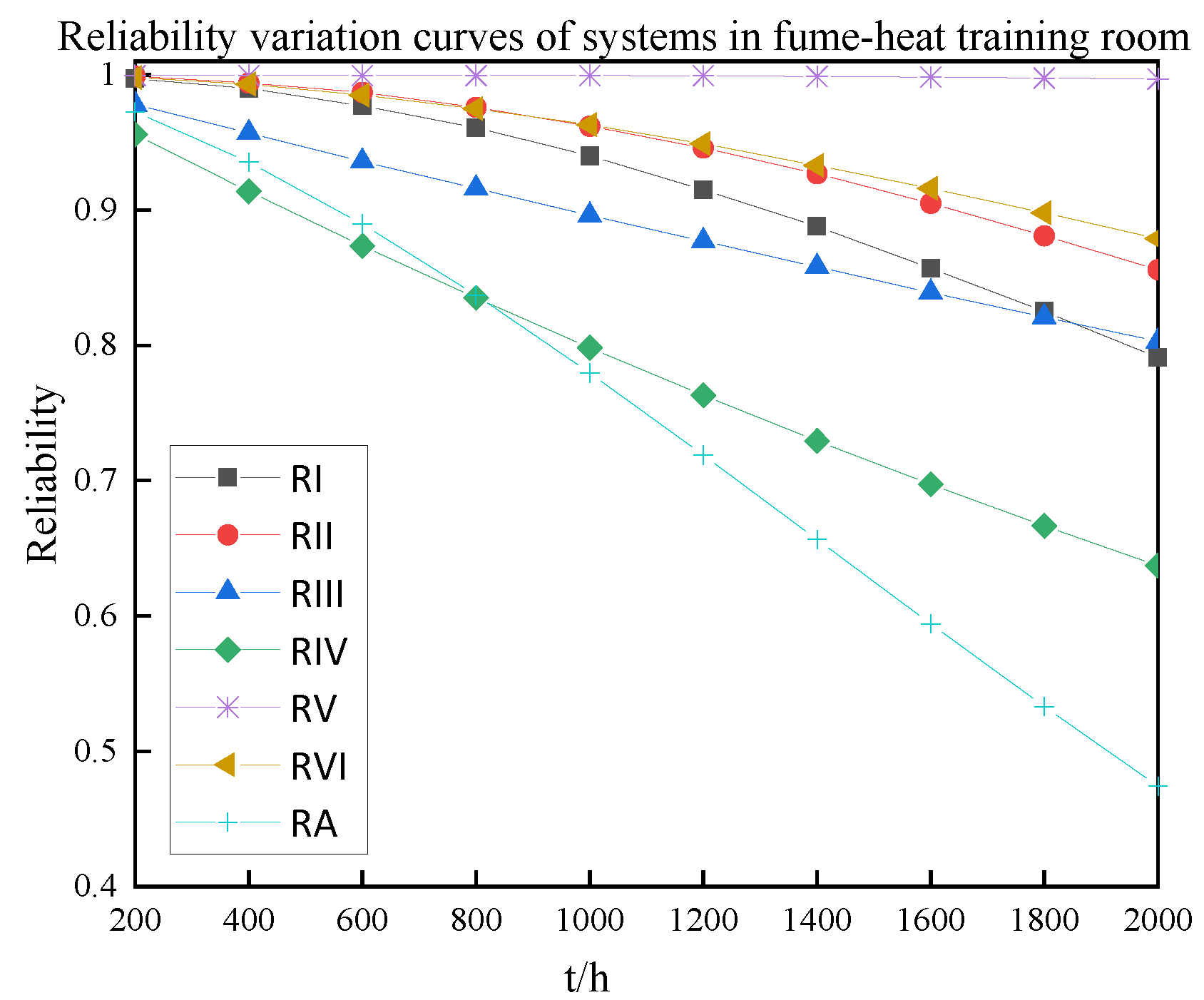
3.1.7. Reliability Model Analysis of the Fume–Heat Training Room
3.2. Structural Analysis of the Combustion Training Room
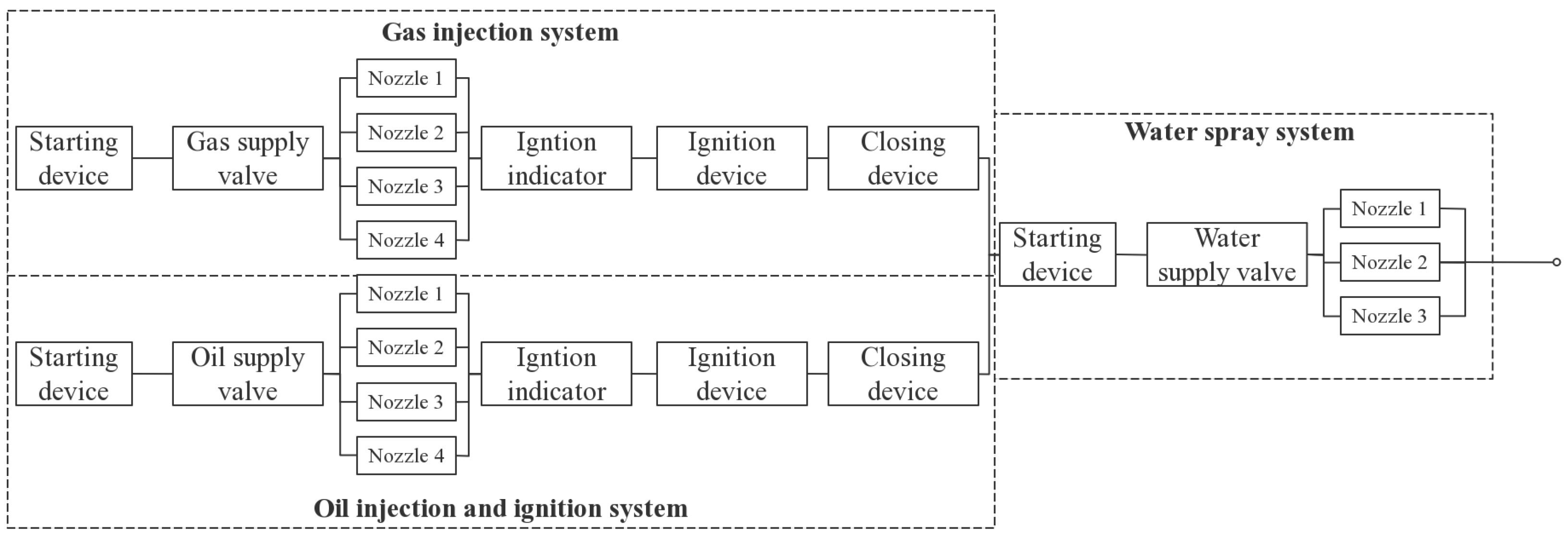
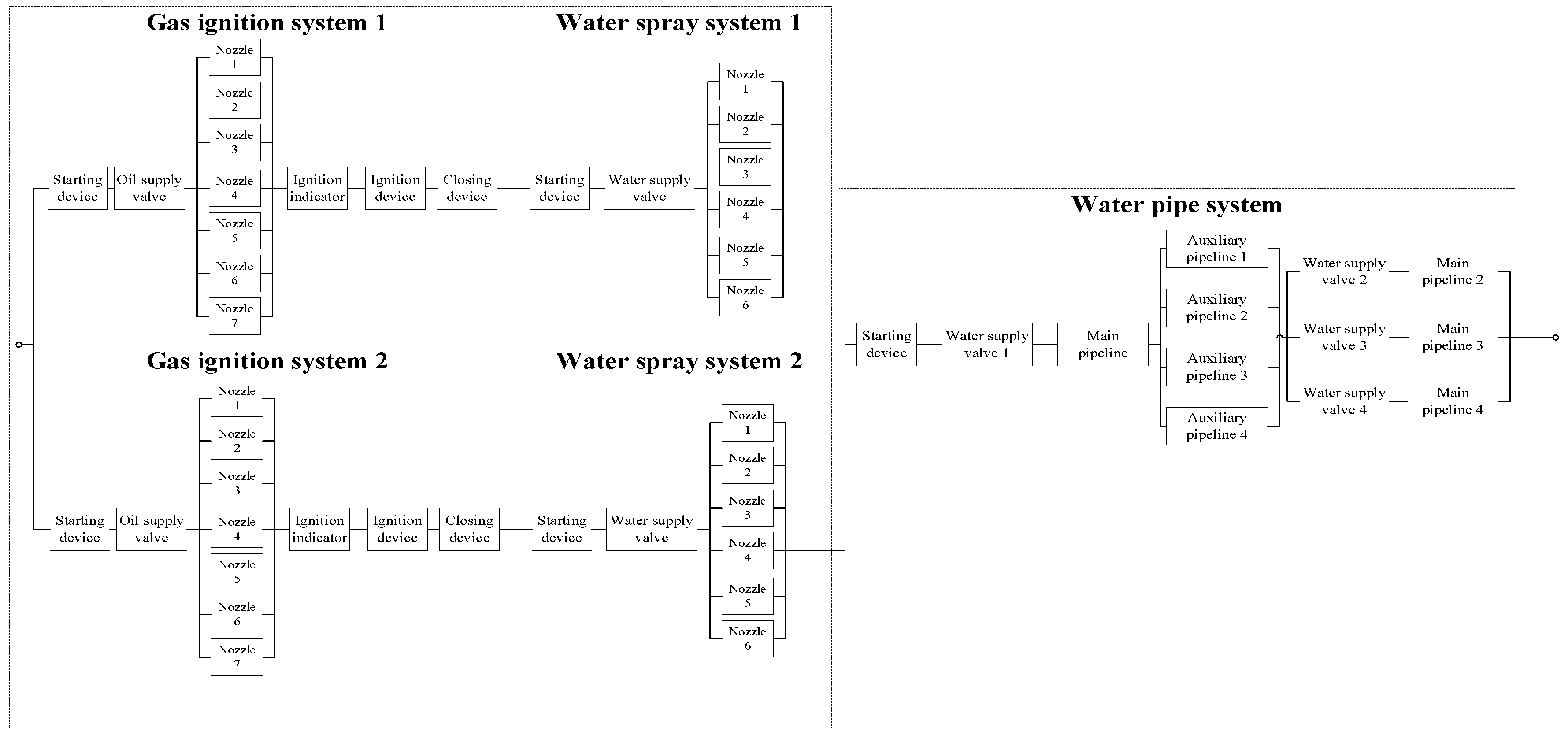
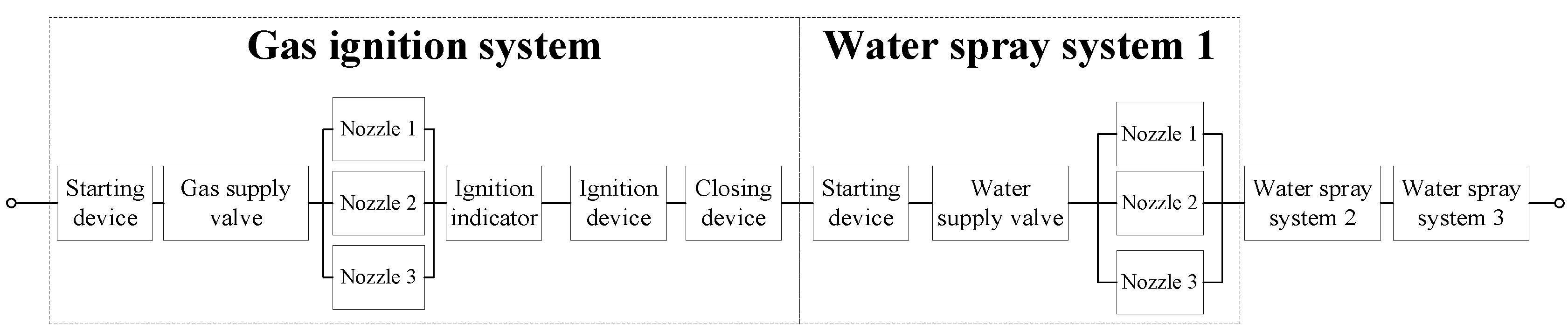
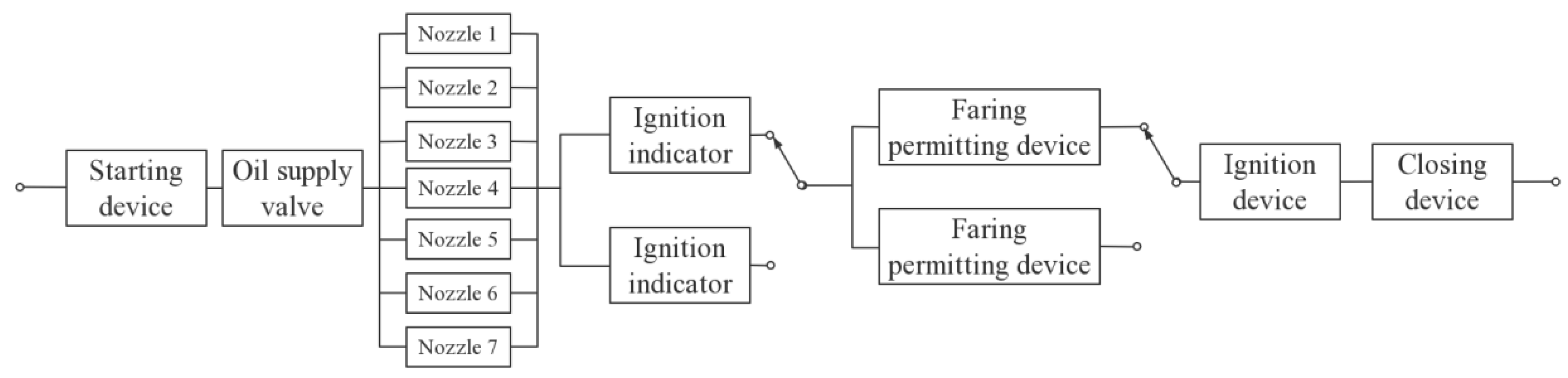
3.2.1. Ground Flow Fire System
3.2.2. Hearth Fire System
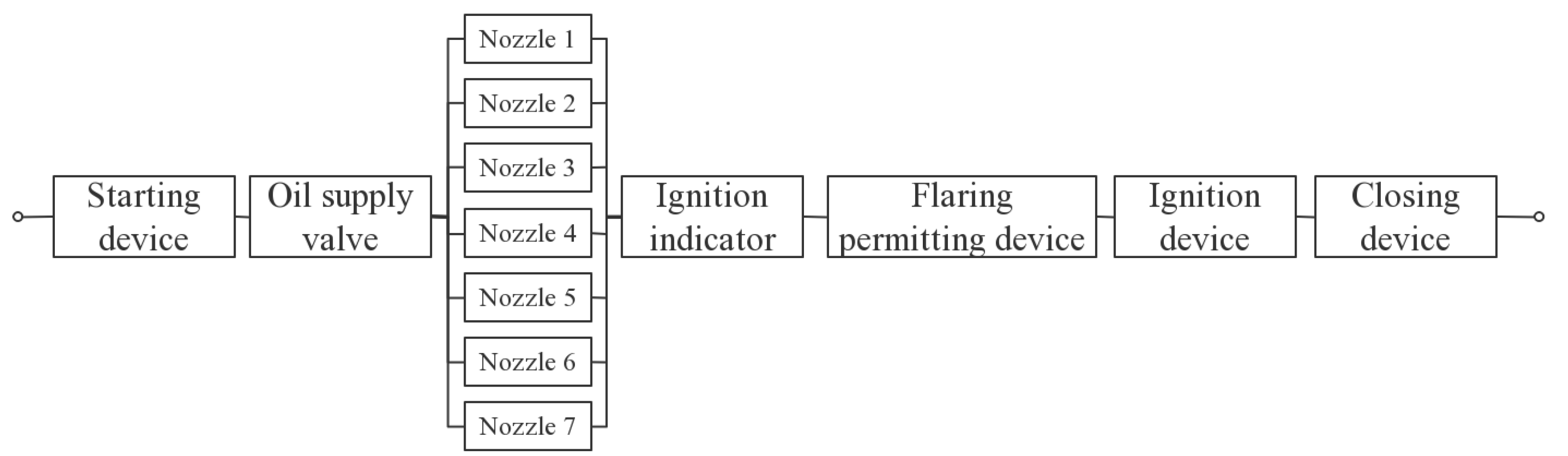
3.2.3. Flaring System
3.2.4. Combustion Bed System
3.2.5. Stair Fire and TV Fire System
3.2.6. Reliability Model Analysis of the Combustion Training Room
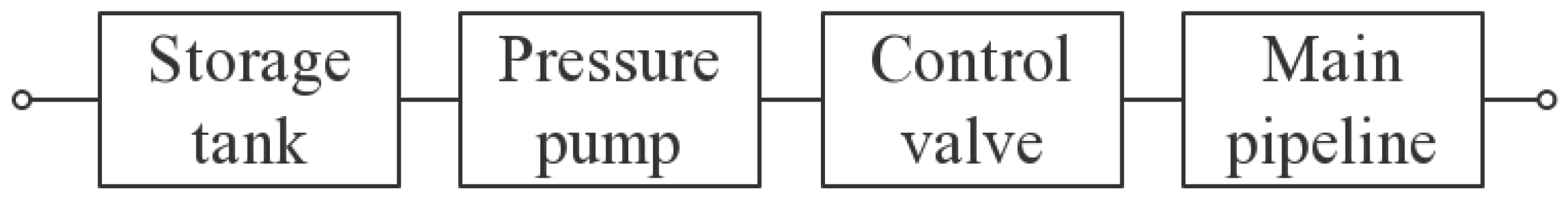
3.3. Structural Analysis of the Water, Oil, and Gas Supply System
3.3.1. Water Supply System and Oil Supply System
3.3.2. Gas Supply System
3.3.3. Reliability Model Analysis of the Water, Oil and Gas Supply System
3.4. Reliability Model Establishment of the Building Real Fire Simulation Training System
4. Reliability Analysis of the Building Real Fire Simulation Training System
4.1. Failure Rate of Key Components
4.2. Reliability Results Analysis of the Fume–Heat Training Room
4.3. Reliability Results Analysis of the Combustion Training Room
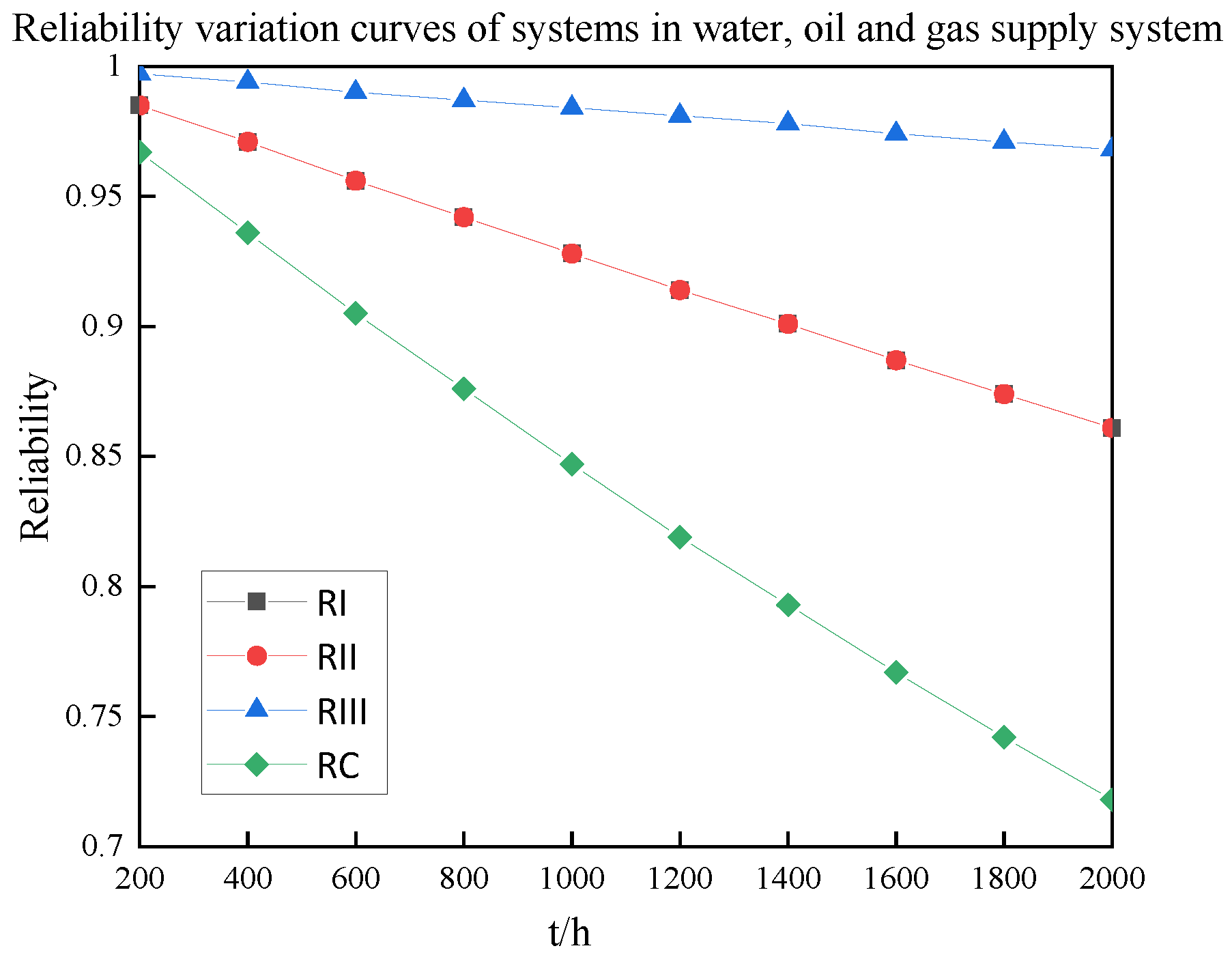
4.4. Reliability Results Analysis of the Water, Oil, and Gas Supply System
5. Conclusions
- (1)
- With a constant correction factor, the failure rate of each system gradually increases and the reliability decreases as the working time increases. At the same time, the larger the correction factor, the harsher the working environment, the higher the failure rate of the system, and the lower the reliability. The water, oil, and gas supply system has the highest reliability, while the combustion training room has the lowest reliability.
- (2)
- The structure with the lowest reliability in the fume–heat training room is the strobe light system. The strobe light system can be changed to a structure with more parallel connections and fewer series connections to improve its reliability. The frequency of maintenance should be increased for heating and fuming device systems after a long period of use to ensure their stability.
- (3)
- The least reliable structure in the combustion training room is the flaring system, which can be improved by introducing a reserve system. The components of a stair fire system need to be optimized to improve overall reliability.
- (4)
- Based on meeting the training requirements, the real fire simulation training system should try to reduce the series link, increase the parallel link, and add reserve systems as necessary. Components with high reliability should be selected as much as possible to increase the overall reliability when economic conditions allow.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Brushlinsky, N.N.; Ahrens, M.; Sokolov, S.V.; Wagner, P. World Fire Statistics, CTIF, International Association of Fire and Rescue Services, No. 22. 2017. Available online: www.ctif.org/sites/default/files/ctif_report22_world_fire_statistics_2017.pdf (accessed on 30 June 2019).
- Bulletin. World Fire Statistics, The Geneva Association, No. 29. 2014. Available online: www.genevaassociation.org/research-topics/world-fire-statistics-bulletin-no-29 (accessed on 30 June 2019).
- GDP. World Bank National Accounts Data, and OECD National Accounts Data Files. 2018. Available online: https://data.worldbank.org/indicator/NY.GDP.MKTP.CD (accessed on 30 June 2019).
- Brushlinsky, N.N.; Ahrens, M.; Sokolov, S.V.; Wagner, P. World Fire Statistics, CTF, International Association of Fire and Rescue Services, No. 21. 2016. Available online: www.ctif.org/sites/default/files/ctif_report21_world_fire_statistics_2016.pdf (accessed on 30 June 2019).
- Meng, Z.F. A brief overview of fires in the world. Fire Prot. Technol. Prod. Inf. 2011, 2, 91. [Google Scholar]
- Feng, Y.P. World fire statistics. Fire Prot. Technol. Prod. Inf. 2009, 3, 75–78. [Google Scholar]
- Kodur, V.; Kumar, P.; Rafi, M.M. Fire hazard in buildings, review, assessment and strategies for improving fire safety. J. PSU Res. Rev. 2019, 4, 1–23. [Google Scholar] [CrossRef]
- Cha, M.; Han, S.; Lee, J.; Choi, B. A virtual reality based fire training simulator integrated with fire dynamics data. Fire Saf. J. 2012, 50, 12–24. [Google Scholar] [CrossRef]
- Beresh, R.; Ciufo, J.; Anders, G. Basic fault tree analysis for use in protection reliability. Int. J. Reliab. Safe 2008, 2, 64–78. [Google Scholar] [CrossRef]
- Stamatelatos, M.; Vesely, W.; Dugan, J.; Fragola, J.; Minarick, J.; Railsback, J. Fault Tree Handbook with Aerospace Applications; NASA Office of Safety and Mission Assurance: Washington, DC, USA, 2002; pp. 109–112.
- Volkanovski, A.; Cepin, M.; Mavko, B. Application of the fault tree analysis for assessment of power system reliability. Reliab. Eng. Syst. Safe 2009, 94, 1116–1127. [Google Scholar] [CrossRef]
- Baig, A.A.; Ruzli, R.; Buang, A.B. Reliability analysis using fault tree analysis, a review. Int. J. Chem. Eng. Appl. 2013, 4, 169. [Google Scholar] [CrossRef]
- D’Auria, F.; Galassi, G.M. Methodology for the Evaluation of the Reliability of Passive Systems; University of Pisa: Pisa, Italy, 2000. [Google Scholar]
- Ricotti, M.E.; Bianchi, F.; Burgazzi, L.; D’Auria, F.; Galassi, G. The REPAS study, reliability evaluation of passive safety systems. Int. Conf. Nucl. Eng. 2002, 35960, 245–254. [Google Scholar]
- Jafari, J.; D’Auria, F.; Kazeminejad, H.; Davilu, H. Reliability evaluation of a natural circulation system. Nucl. Eng. Des. 2003, 224, 79–104. [Google Scholar] [CrossRef]
- Coolen, F.P.A.; Coolen-Maturi, T. Generalizing the Signature to Systems with Multiple Types of Components. In Complex Systems and Dependability; Zamojski, W., Mazurkiewicz, J., Eds.; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2012; Volume 170, pp. 115–130. [Google Scholar]
- Borgonovo, E.; Aliee, H.; Glaß, M.; Teich, J. A new time-independent reliability importance measure. Eur. J. Oper. Res. 2016, 254, 427–442. [Google Scholar] [CrossRef]
- Nguyen, K.; Beugin, J.; Berbineau, M.; Kassab, M. Modelling Communication Based Train control system for dependability analysis of the LTE Communication network in train control application. In Proceedings of the 2014 European Modelling Symposium, Pisa, Italy, 21–23 October 2014. [Google Scholar]
- Tan, C.Q.; Zhang, X.; Zheng, G.X. A method to improve the reliability of CBTC wireless lin. In Proceedings of the IET International Communication Conference on Wireless Mobile and Computing (CCWMC), Shanghai, China, 7–9 December 2009. [Google Scholar]
- Ebersole, J.F.; Furlong, T.J.; Ebersole Jr, J.F. Method of Generating Three-Dimensional Fire and Smoke Plume for Graphical Display. U.S. Patent 6,809,743, 26 October 2004. [Google Scholar]
- Qiu, P.F.; Hao, A.L. Study on the development status of foreign fire safety engineering technology standards. J. Eng. Constr. Stand. 2014, 6, 53–56. [Google Scholar]
- Ministry of Public Security of the People’s Republic of China. Guide for Fire Training Safety, People’s Republic of China Public Safety Industry Standard GA/T 967-2011; Standards Press of China: Beijing, China, 2011. [Google Scholar]
- The Ministry of Public Security of the People’s Republic of China, People’s Republic of China. Public Safety Industry Standard GA/T 623-2006: Standard on Training Facility Construction for Fire Services Training Center; Standards Press of China: Beijing, China, 2006; pp. 1–8. [Google Scholar]
- National People’s Congress Standing Committee. Fire Protection Law of the People’s Republic of China; China Legal Publishing House: Beijing, China, 2021. [Google Scholar]
- Ram, M. On system reliability approaches, a brief survey. Int. J. Syst. Assur. Eng. 2013, 4, 101–117. [Google Scholar] [CrossRef]
- Birolini, A. Basic Concepts, Quality & Reliability (RAMS) Assurance of Complex Equipment & Systems. In Reliability Engineering; Springer: Berlin/Heidelberg, Germany, 2017; pp. 1–24. [Google Scholar]
- Breneman, J.E.; Sahay, C.; Lewis, E.E. Introduction to Reliability Engineering; John Wiley & Sons: Hoboken, NJ, USA, 2022; pp. 1–8. [Google Scholar]
- Bourouni, K. Availability assessment of a reverse osmosis plant, comparison between reliability block diagram and fault tree analysis methods. Desalination 2013, 313, 66–76. [Google Scholar] [CrossRef]
- Souza, F.A.; de Paula, H.; Cardoso Filho, B.J.; Rocha, A.V.; Lobato, G.I. Influence of environmental factors on the reliability of electronic components used in motor drive systems. In Proceedings of the 2013 Brazilian Power Electronics Conference, Gramado, Brazil, 27–31 October 2013. [Google Scholar]
- Rausand, M.; Hoyland, A. System Reliability Theory: Models, Statistical Methods, and Applications; John Wiley & Sons: Hoboken, NJ, USA, 2003; pp. 15–19. [Google Scholar]
- Čepin, M. Assessment of Power System Reliability, Methods and Applications; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2011; pp. 119–123. [Google Scholar]
- Ruijters, E.; Stoelinga, M. Fault tree analysis, A survey of the state-of-the-art in modeling. Anal. Tools Comput. Sci. Rev. 2015, 15, 29–62. [Google Scholar] [CrossRef]
- Kim, M.C. Reliability block diagram with general gates and its application to system reliability analysis. Ann. Nucl. Energy 2011, 38, 2456–2461. [Google Scholar] [CrossRef]
- Kaczor, G.; Młynarski, S.; Szkoda, M. Verification of safety integrity level with the application of Monte Carlo simulation and reliability block diagrams. J. Loss Prev. Process Ind. 2016, 41, 31–39. [Google Scholar] [CrossRef]
- Verma, A.K.; Ajit, S.; Karanki, D.R. Reliability and Safety Engineering; Springer: London, UK, 2010; pp. 373–392. [Google Scholar]








| Structure | Mathematical Models |
|---|---|
| Series system | |
| Parallel system | |
| Series-parallel system | |
| Parallel-series system | |
| Voting system |
| Reliability of the fume—heat training room: | ||
| Heating system | : Reliability of electric controls : Reliability of manual controls : Reliability of heat blower : Reliability of fume emitter : Reliability of industrial heater : Reliability of fume generator | |
| Fuming system | ||
| Air supply and exhaust system | : Reliability of supply fan : Reliability of exhaust fan | |
| Strobe light system | : Reliability of light | |
| Sound system | : Reliability of sound | |
| Thermostat system | : Reliability of thermostat : Reliability of temperature monitor | |
| Ground flow fire system | : Reliability of starting devices : Reliability of valves : Reliability of nozzles : Reliability of ignition indicators : Reliability of ignition devices : Reliability of closing devices : Two operating conditions for the injector and ignition system : Reliability of injectors and ignition system : Reliability of spraying system | |
| Hearth fire system | : Reliability of gas ignition system : Reliability of fuel injection system : Reliability of spraying system | |
| Flaring system | : Reliability of flaring permitting device | |
| Combustion bed system | : Reliability of plumbing system : Reliability of pipeline | |
| Stair fire system | ||
| TV fire system | ||
| Water supply system | : Reliability of water tank : Reliability of water pump : Reliability of control valves : Reliability of main pipeline | |
| Oil supply system | : Reliability of oil tank : Reliability of oil pump | |
| Gas supply system | : Reliability of gas cylinder | |
| Systems | Components | ||||
|---|---|---|---|---|---|
| Upper Limit | Average | Lower Limit | |||
| Fume–heat training room | Electric controls | 10.81 | 7.3 | 1.5 | |
| Manual controls | 8.0 | 7.2 | 1.6 | ||
| Heat blower | 11.4 | 6.9 | 0.2 | ||
| Fume emitter | 15.8 | 5.13 | 0.32 | ||
| Temperature monitor | 9.2 | 5.74 | 1.3 | ||
| Supply fan | 24.3 | 3.22 | 1.76 | ||
| Exhaust fan | 19.7 | 2.43 | 1.32 | ||
| Light | 6.43 | 1.62 | 0.6 | ||
| Sound | 15.24 | 8.1 | 0.91 | ||
| Thermostat | 10.4 | 8.52 | 1.21 | ||
| Combustion training room | Starting devices | 1.5 | 1.32 | 0.72 | |
| Valves | 2.71 | 1.05 | 0.45 | ||
| Nozzles | 0.77 | 0.3 | 0.01 | ||
| Ignition indicators | 1.77 | 1.59 | 0.41 | ||
| Ignition devices | 2.34 | 1.7 | 0.27 | ||
| Closing device | 2.4 | 1.2 | 0.1 | ||
| Pipeline | 2.01 | 0.03 | 0.012 | ||
| Flaring permitting device | 1.22 | 0.5 | 0.4 | ||
| Water, oil, and gas supply system | Tanks | 2.52 | 1.5 | 0.48 | |
| Valves | 2.71 | 1.05 | 0.45 | ||
| Pumps | 5.54 | 2.41 | 1.34 | ||
| Gas cylinder | 0.8 | 0.3 | 0.041 | ||
| Pipeline | 2.01 | 0.03 | 0.012 | ||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Huang, Z.; Yu, R.; Huang, Y.; Li, J.; Ding, H.; Lei, Y.; Wang, P.; Jameel, D. Reliability Analysis of a Building Real Fire Simulation Training System. Fire 2023, 6, 369. https://doi.org/10.3390/fire6100369
Huang Z, Yu R, Huang Y, Li J, Ding H, Lei Y, Wang P, Jameel D. Reliability Analysis of a Building Real Fire Simulation Training System. Fire. 2023; 6(10):369. https://doi.org/10.3390/fire6100369
Chicago/Turabian StyleHuang, Zhian, Rongxia Yu, Yang Huang, Jinyang Li, Hao Ding, Yukun Lei, Pengfei Wang, and Danish Jameel. 2023. "Reliability Analysis of a Building Real Fire Simulation Training System" Fire 6, no. 10: 369. https://doi.org/10.3390/fire6100369
APA StyleHuang, Z., Yu, R., Huang, Y., Li, J., Ding, H., Lei, Y., Wang, P., & Jameel, D. (2023). Reliability Analysis of a Building Real Fire Simulation Training System. Fire, 6(10), 369. https://doi.org/10.3390/fire6100369





