Simulation of Thermomechanical Coupling and Evaluation of the Fire Resistance for the Joints of Fabricated Frame Tunnel
Abstract
1. Introduction
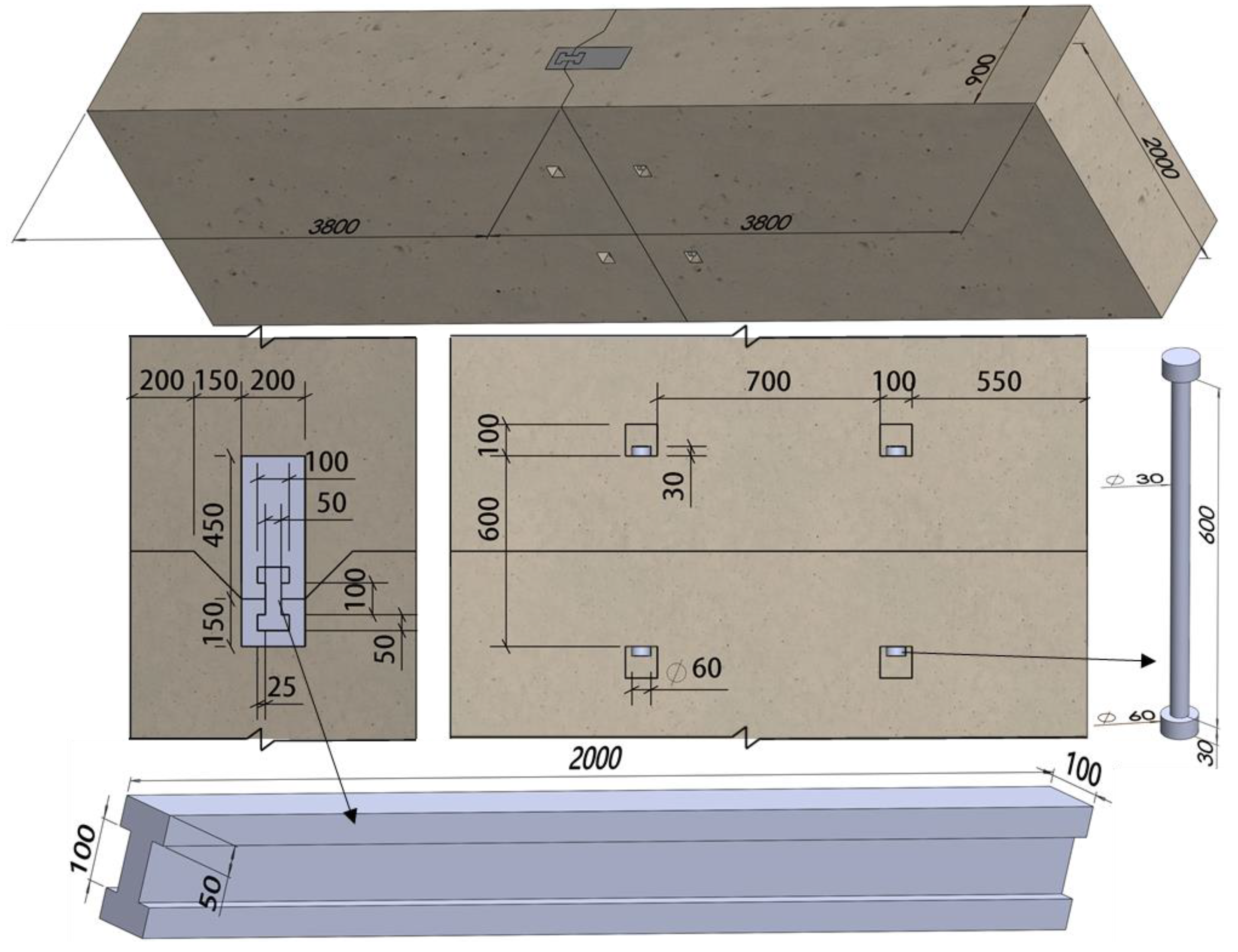
2. Fabricated Frame Tunnel Joints
2.1. Establishment of the Numerical Simulation Model
2.1.1. Joint Construction and Dimensions
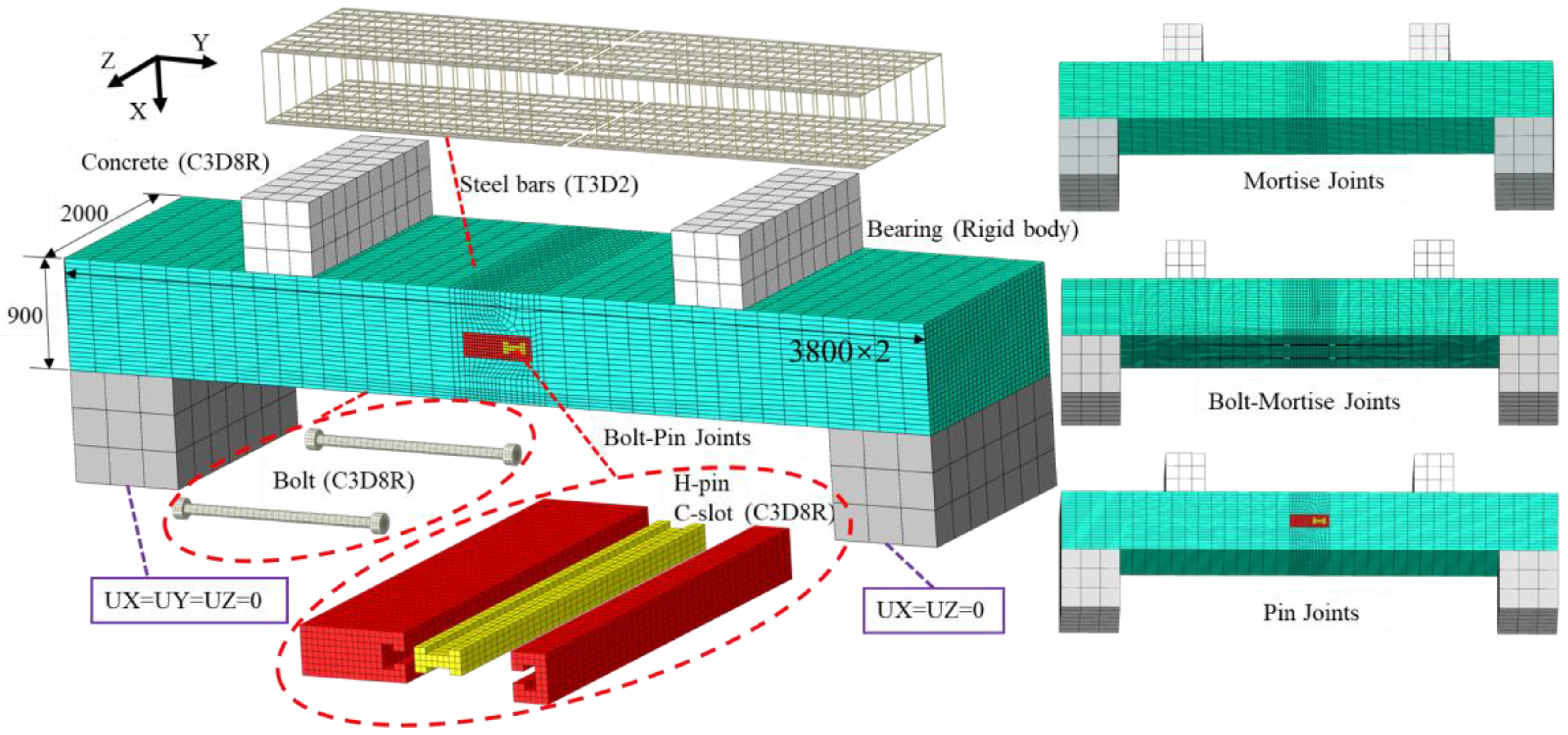
2.1.2. Meshing and Contact Properties
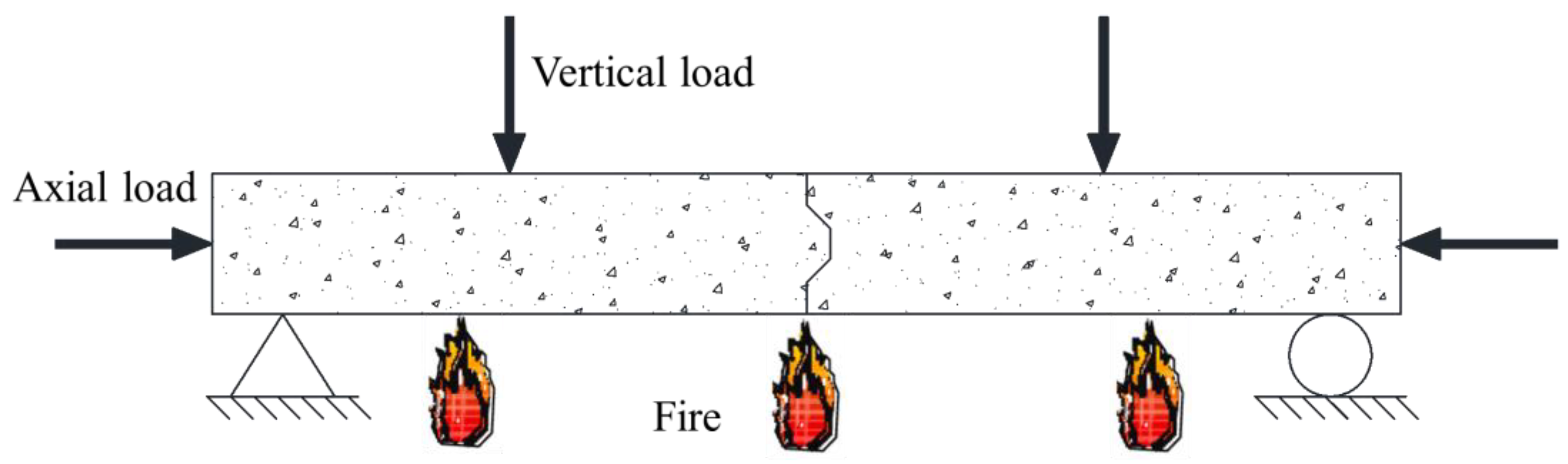
2.1.3. Boundary Conditions and Loading Methods
- A predetermined axial load is applied at the joints at room temperature;
- The first model of the temperature field is imported into the second model;
- A vertical load is applied at the joints to calculate the bearing capacity of the joint under fire, as shown in Figure 4.
2.2. Material Constitutive
2.2.1. Concrete
2.2.2. Steel
2.3. Verification of the Numerical Simulation Method
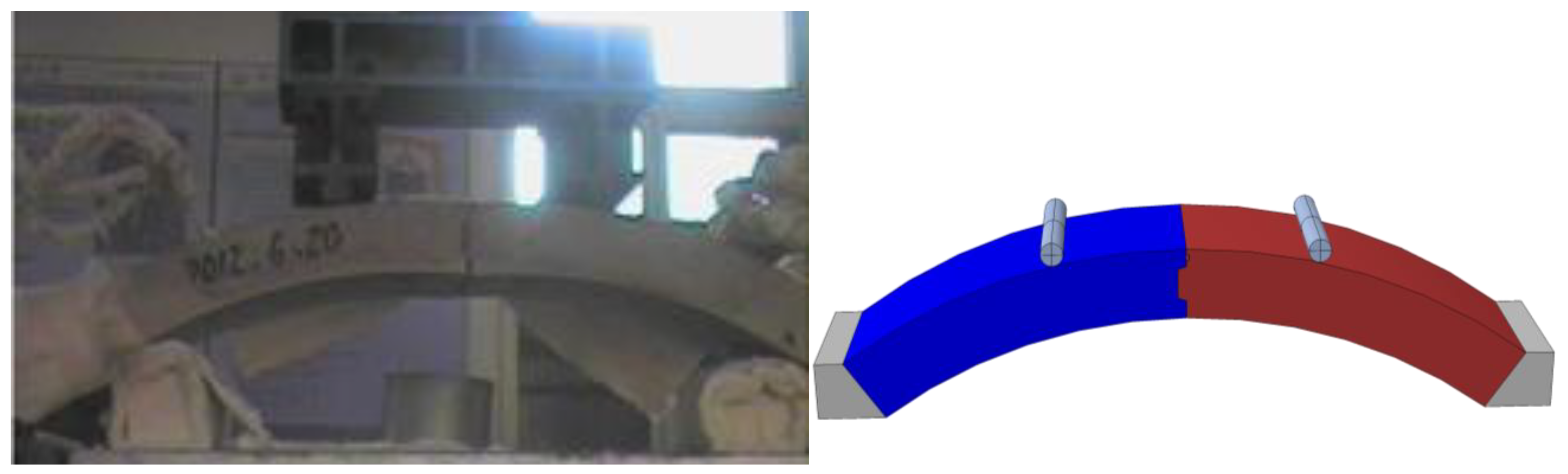
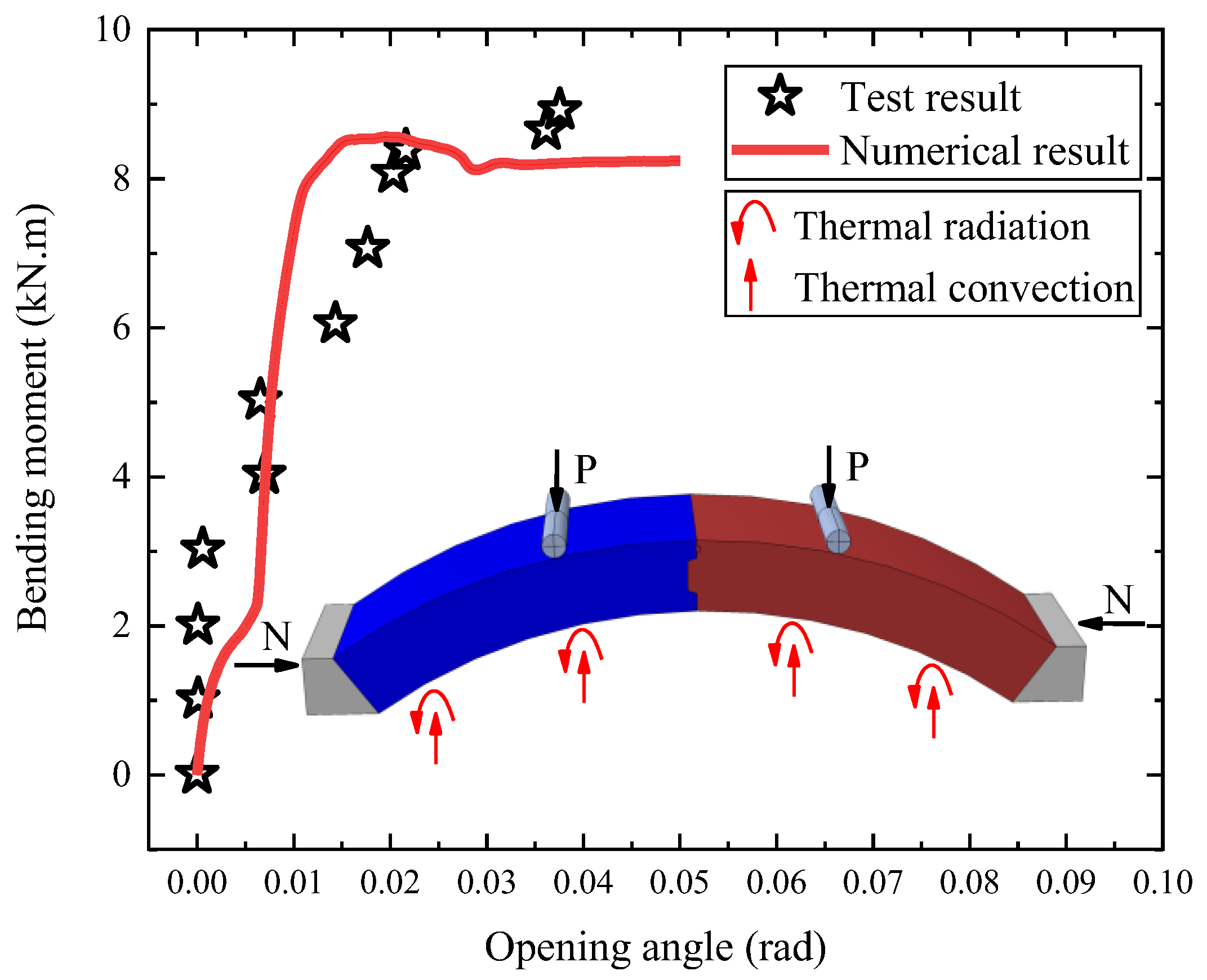
2.3.1. Verification of the Flexural Bearing Capacity of the Joints at Room Temperature
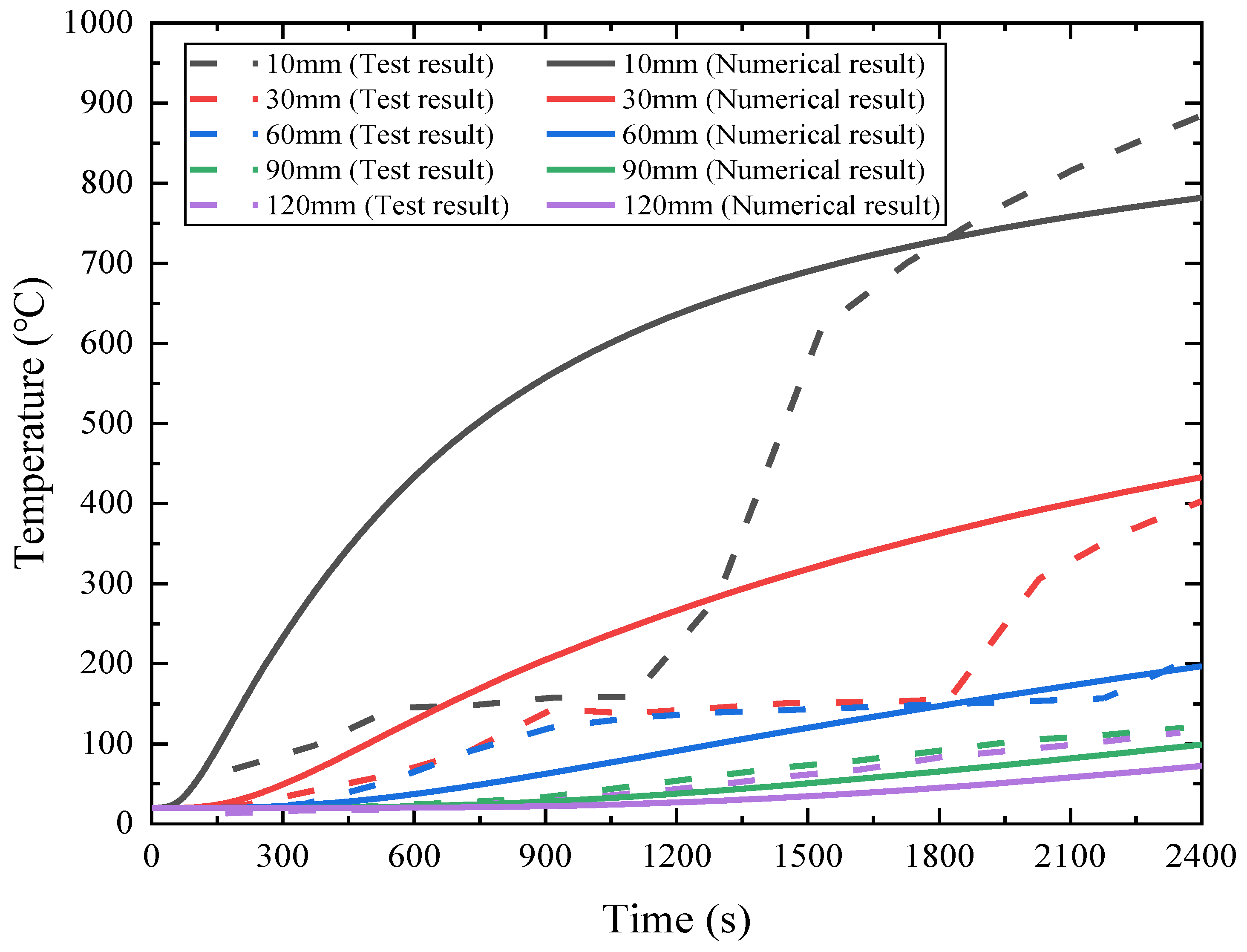
2.3.2. Verification of the Temperature Field Analysis Model
2.3.3. Verification of the Flexural Bearing Capacity of Joints under Fire
3. Results and Analysis
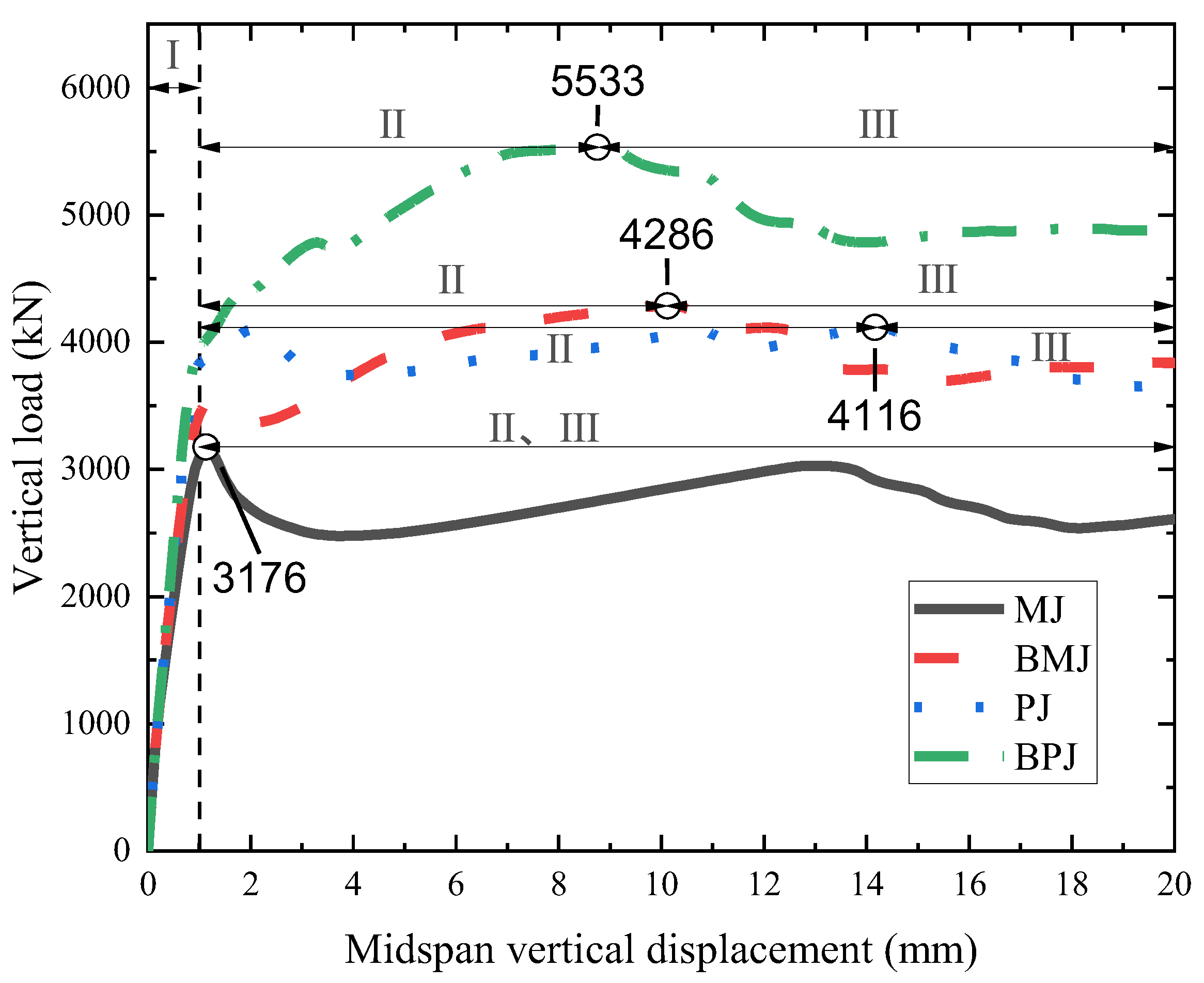
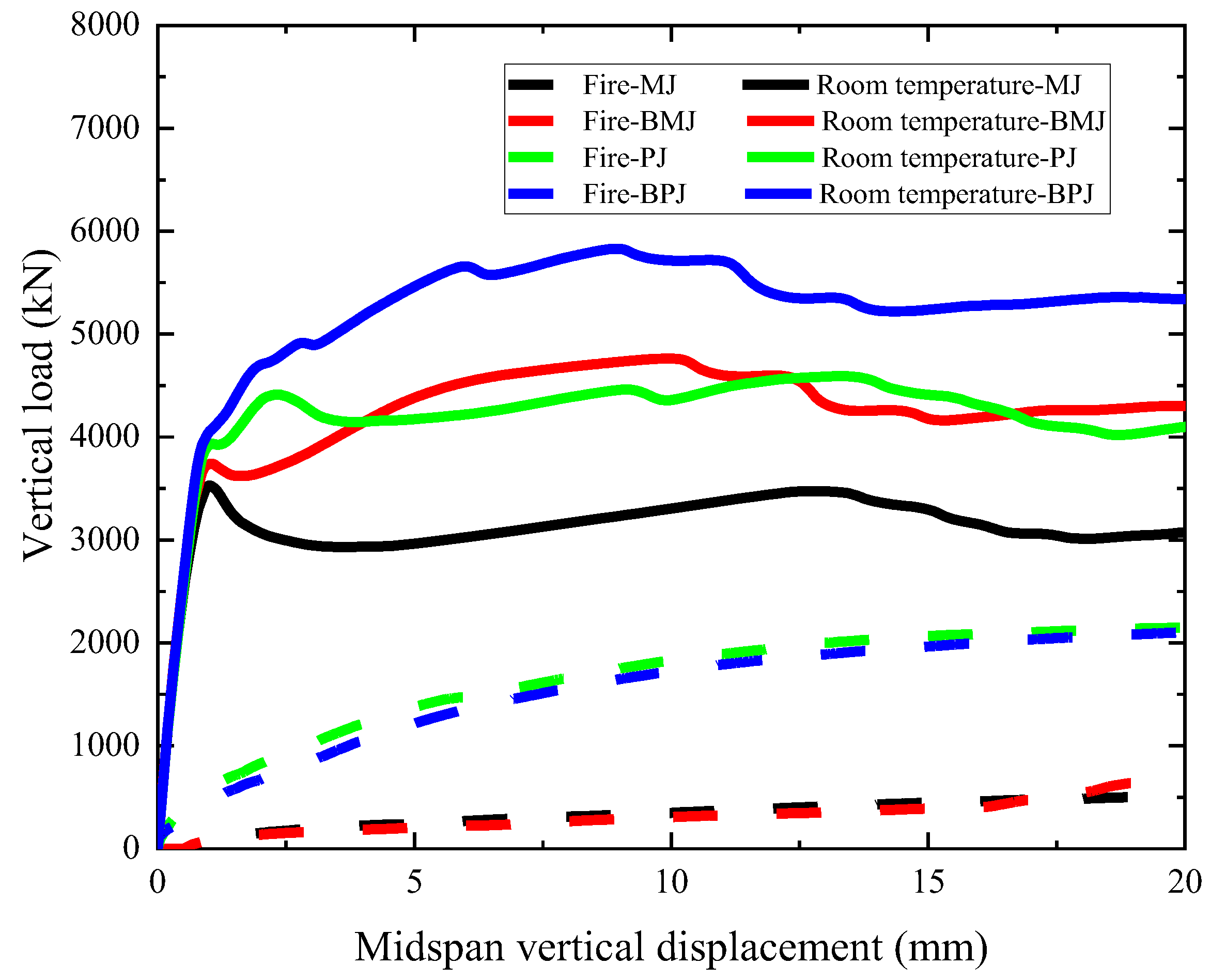
3.1. Flexural Bearing Capacity of Joints at Room Temperature
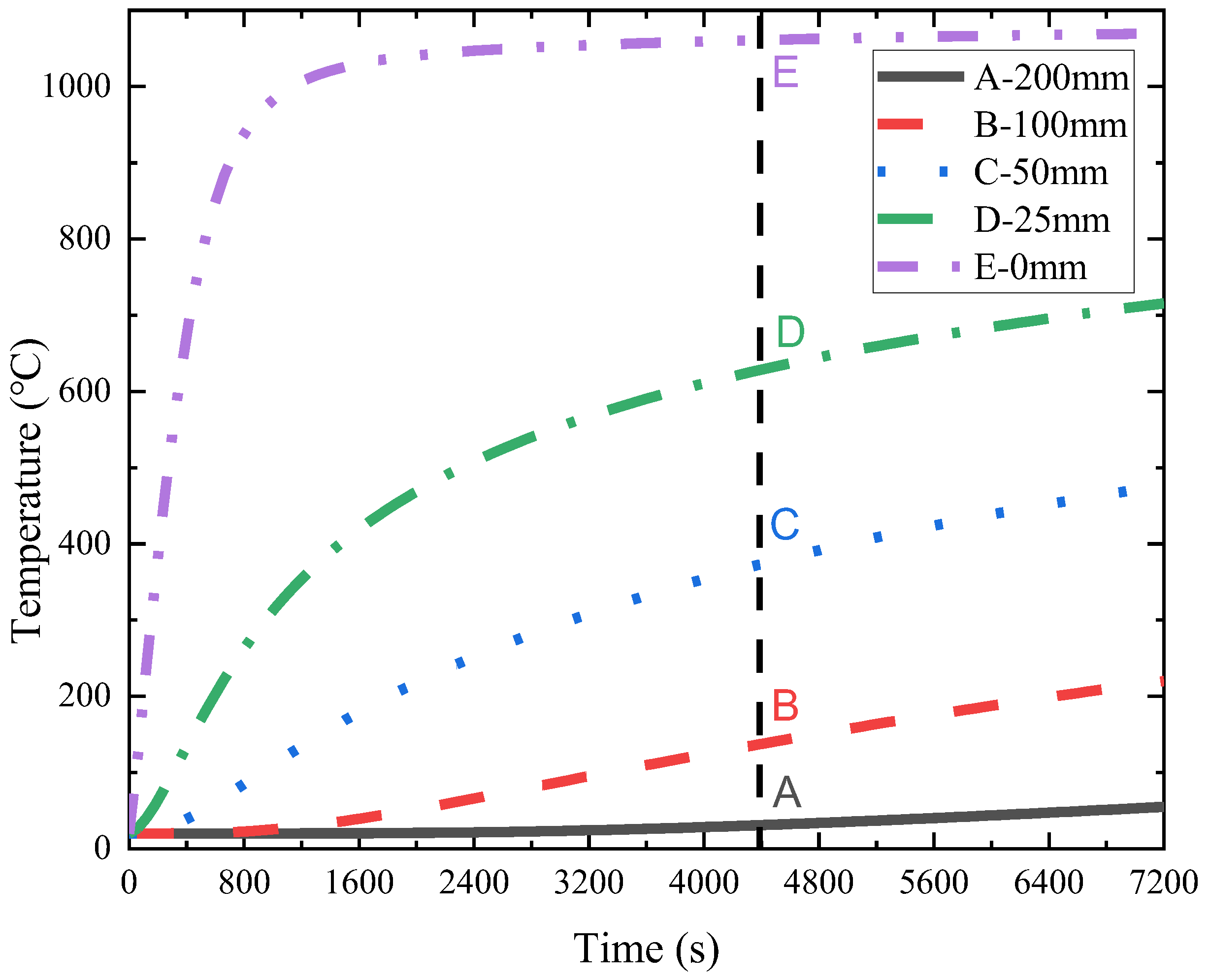
3.2. Temperature Field Analysis Model
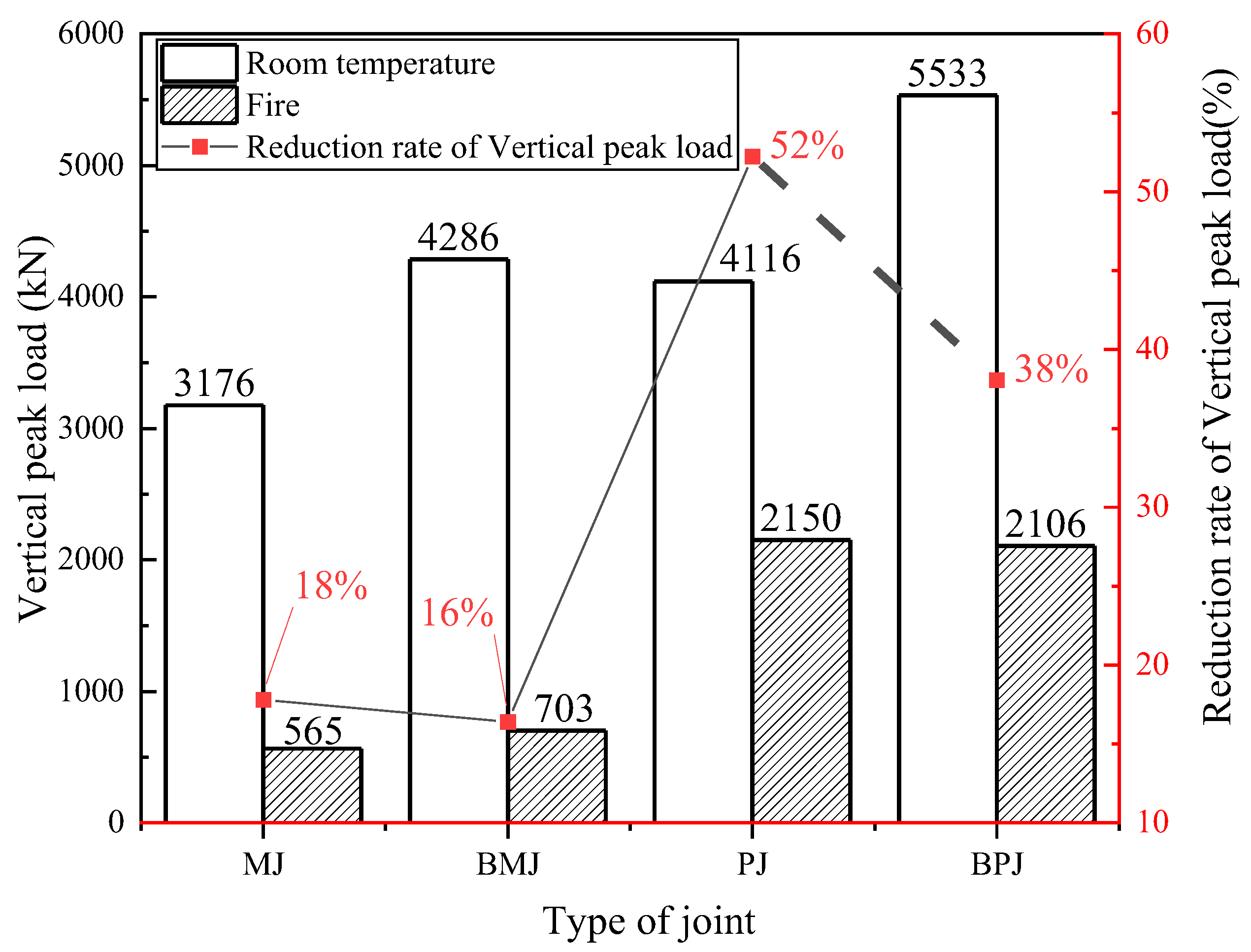
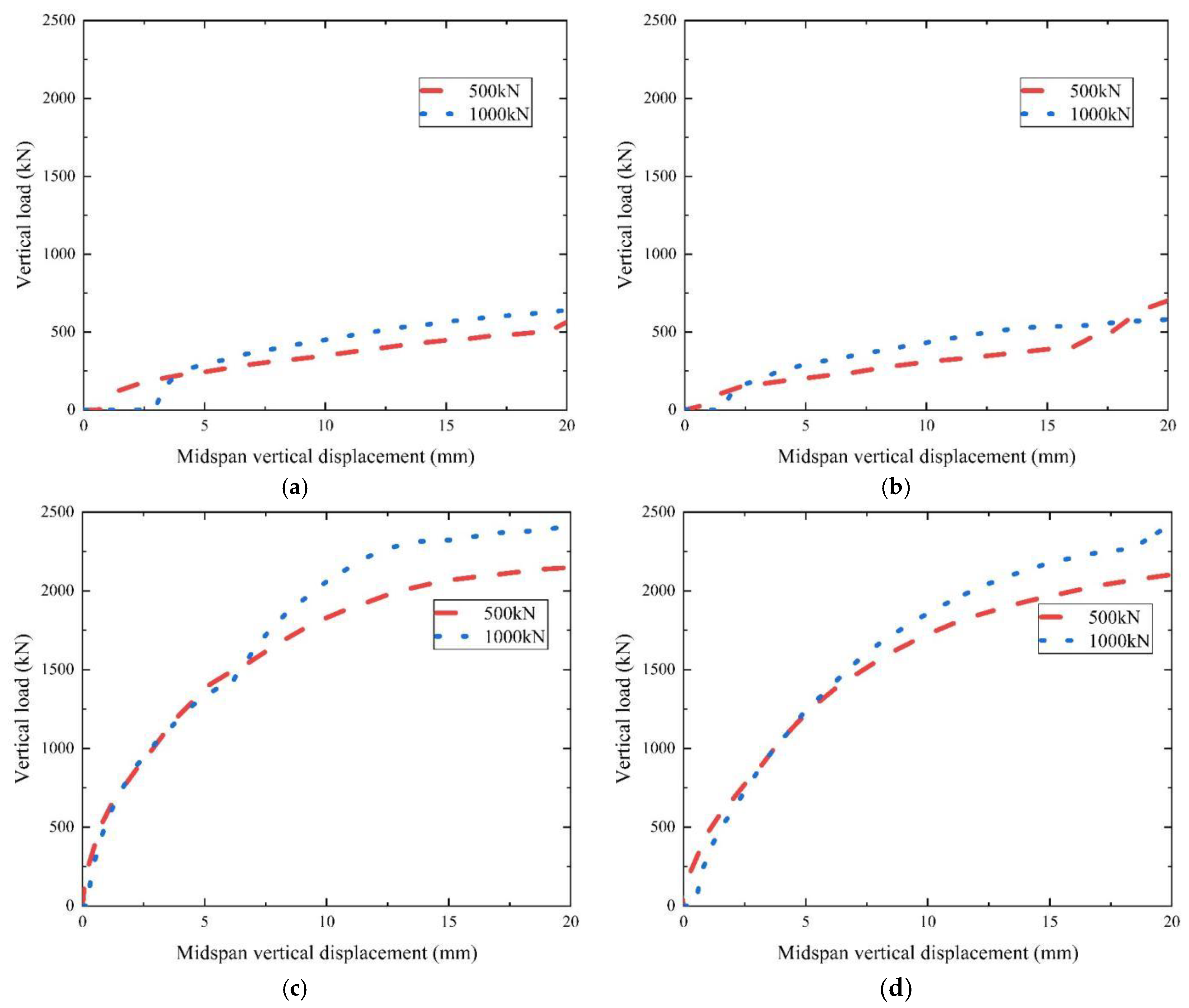
3.3. Flexural Bearing Capacity of Joints under Fire
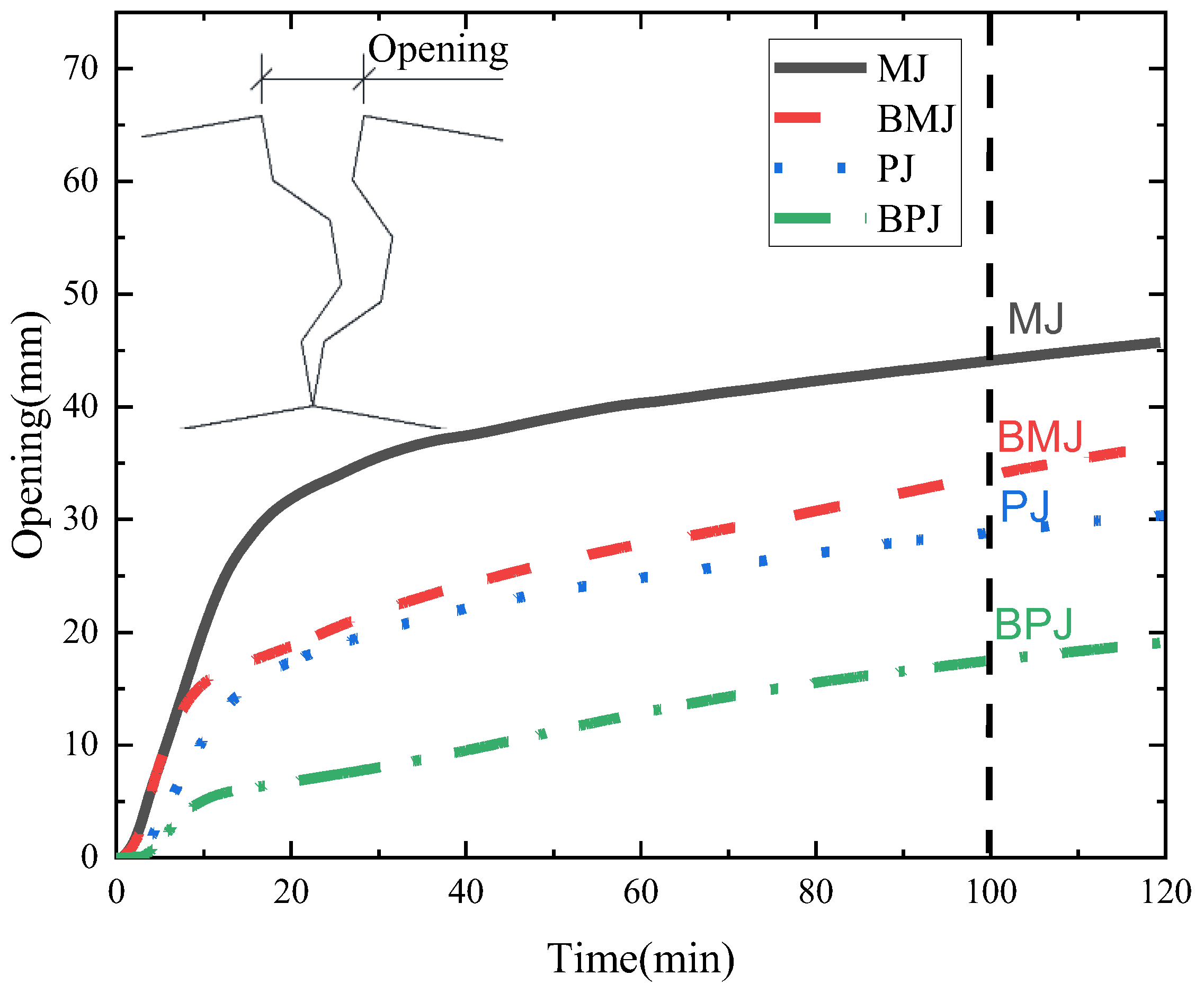
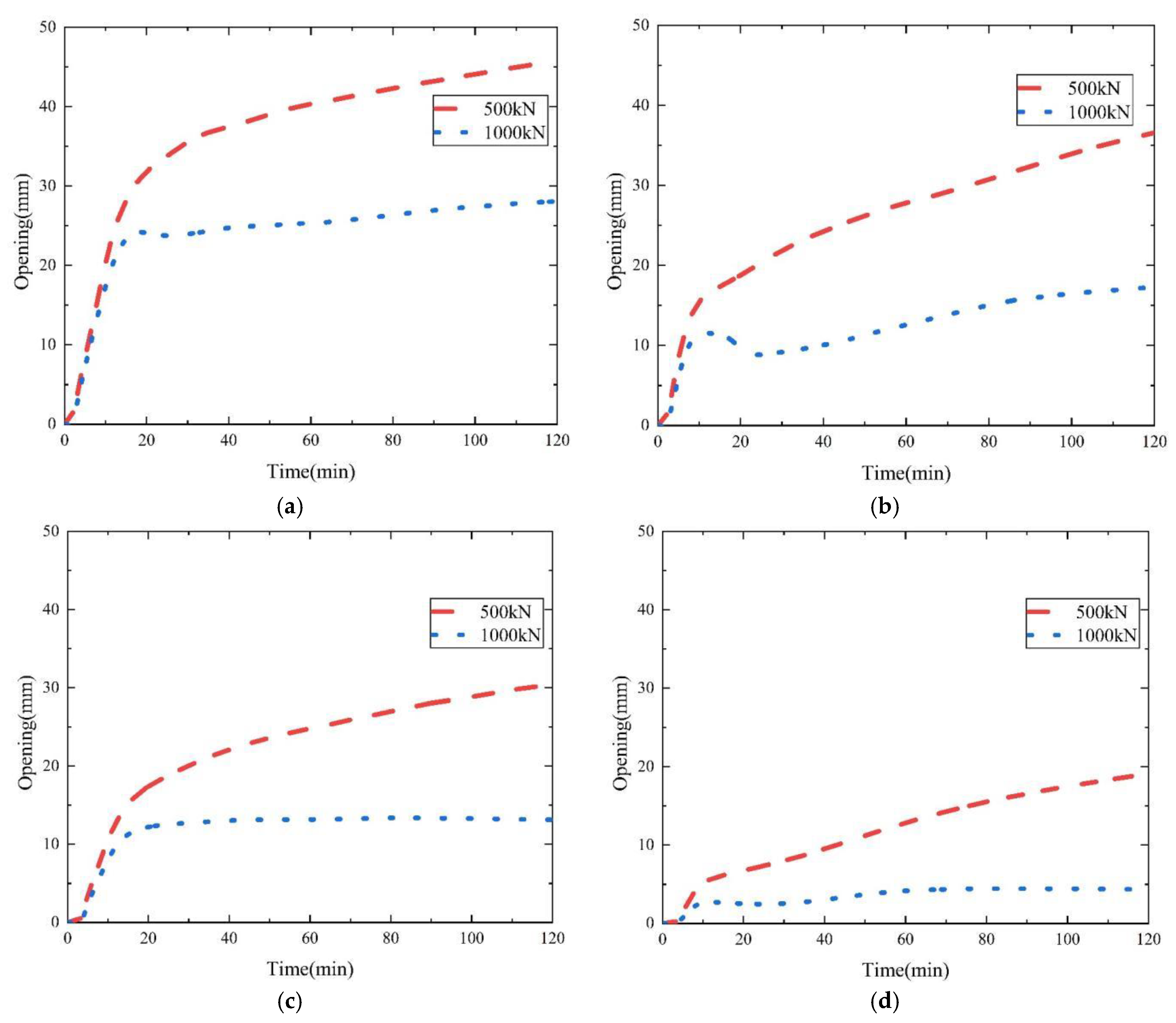
3.4. Opening
3.5. Influence of Axial Force
4. Conclusions
- (1)
- The vertical peak load of the BPJ is higher than that of the other three joints at room temperature, and the combined action of the pin and bolts and the tongue groove can effectively increase the vertical peak load of joints and reduce the midspan vertical displacement.
- (2)
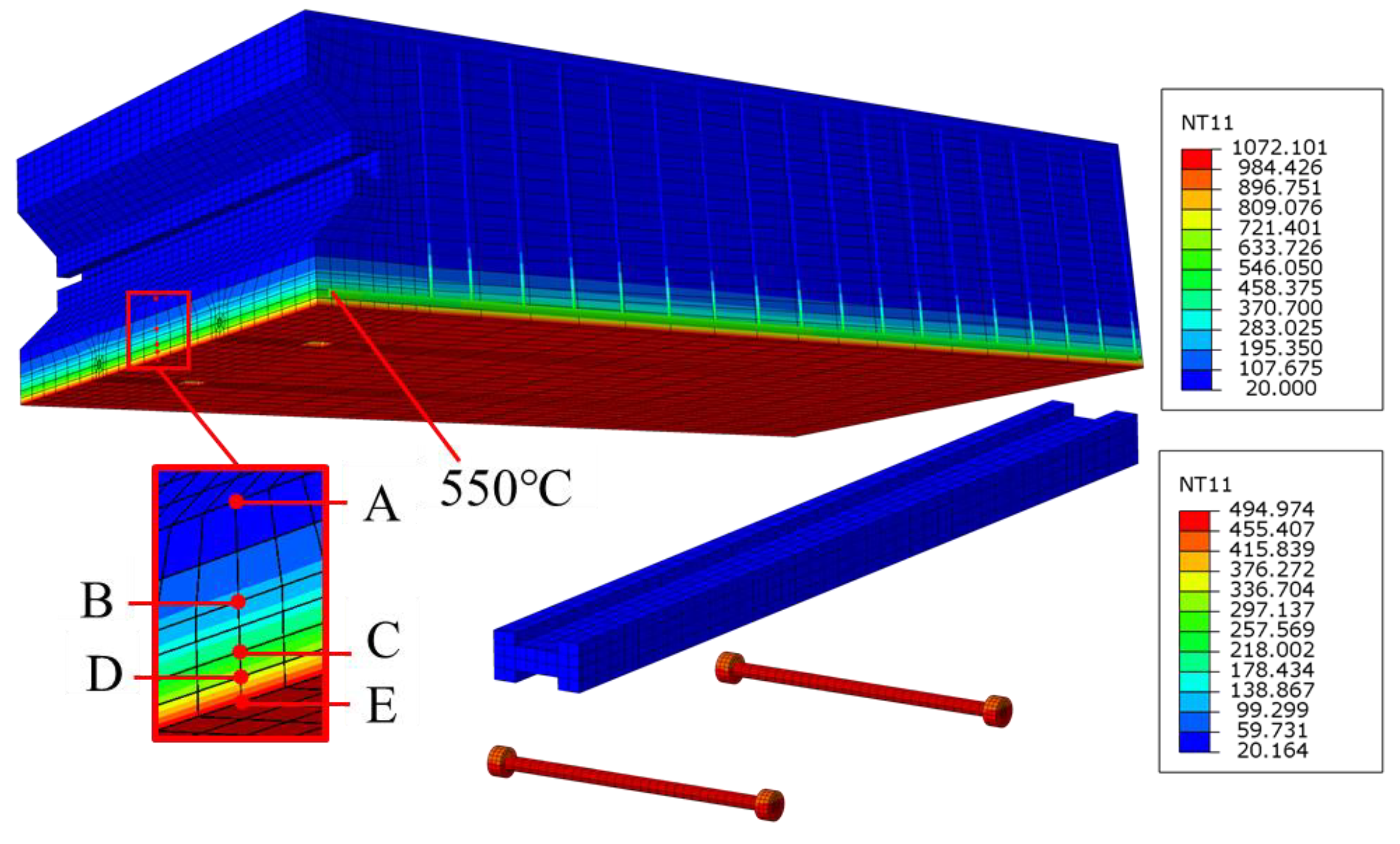
- Under a fire of 120 min, the bolts of BPJ reach 495 °C, while the temperature of the pin is kept at 20 °C. The external concrete can prevent the pin from overheating.
- (3)
- The load–displacement curves of the MJ and BMJ under fire are basically the same, the load–displacement curves of the PJ and BPJ are basically the same, the decrease degree of the vertical peak load of the MJ and BMJ is greater than that of the PJ and BPJ joints, and the opening of the BPJ is much smaller than that of the other three types of fabricated frame tunnel joints.
- (4)
- When the initial axial force is increased, the openings of the four joints under fire are reduced, and the vertical peak loads of PJ and BPJ are increased, while the vertical peak loads of MJ and BMJ are not significantly increased.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Kirkland, C.J. The fire in the Channel Tunnel. Tunn. Undergr. Space Technol. Inc. Trenchless Technol. Res. 2002, 17, 129–132. [Google Scholar] [CrossRef]
- Dorgarten, H.W.; Balthaus, H.; Dahl, J.; Billig, B. Fire resistant tunnel construction: Results of fire behaviour tests and criteria of application. Tunn. Undergr. Space Technol. 2004, 19, 314. [Google Scholar] [CrossRef]
- Lai, H.P.; Wang, S.Y.; Xie, Y.L. Experimental research on temperature field and structure performance under different lining water contents in road tunnel fire. Tunn. Undergr. Space Technol. Inc. Trenchless Technol. Res. 2014, 43, 327–335. [Google Scholar] [CrossRef]
- Zhang, Y.; Zeiml, M.; Maier, M.; Yuan, Y. Lackner Roman Fast assessing spalling risk of tunnel linings under RABT fire: From a coupled thermo-hydro-chemo-mechanical model towards an estimation method. Eng. Struct. 2017, 142, 1–19. [Google Scholar] [CrossRef]
- Rui, R.; Hui, Z.; Zhao, H.; He, S.; Wang, X. Statistical analysis of fire accidents in Chinese highway tunnels 2000–2016. Tunn. Undergr. Space Technol. 2019, 83, 452–460. [Google Scholar] [CrossRef]
- Huang, Z.; Zhang, C.L.; Ma, S.K.; Zhang, J.B.; Zhu, Q.X. Study of the mechanical behaviour and damage characteristics of three new types of joints for fabricated rectangular tunnels using a numerical approach. Tunn. Undergr. Space Technol. 2021, 118, 104184. [Google Scholar] [CrossRef]
- Huang, Z.; Zhang, C.L.; Ma, S.K.; Zhang, H.; Zhou, Z.; Li, H.Z. Parameter Sensitivity Analysis of a New Fabricated Rectangular Tunnel Joint Using Numerical Method. KSCE J. Civ. Eng. 2021, 26, 907–920. [Google Scholar] [CrossRef]
- Huang, Z.; Bai, H.W.; Ma, S.K.; Zhang, J.W.; Chen, Y.M.; Li, H.Z. Large-scale testing of the mortise and tenon joint performance of the tunnel lining of prefabricated frame tunnels. Tunn. Undergr. Space Technol. 2023, 131, 104828. [Google Scholar] [CrossRef]
- Lin, J.; Dong, Y.; Duan, J.; Zhang, D.; Zheng, W. Experiment on single-tunnel fire in concrete immersed tunnels. Tunn. Undergr. Space Technol. 2021, 116, 104059. [Google Scholar] [CrossRef]
- Duan, J.; Dong, Y.; Xiao, J.; Zhang, D.; Zheng, W.; Zhang, S. A large-scale fire test of an immersed tunnel under the protection of fire resistive coating. Tunn. Undergr. Space Technol. 2021, 111, 103844. [Google Scholar] [CrossRef]
- Guo, J.; Jiang, S.; Zhang, Z. Fire Thermal Stress and its Damage to Subsea Immersed Tunnel. Procedia Eng. 2016, 166, 296–306. [Google Scholar] [CrossRef]
- Yan, Z.G.; Zhu, H.H.; Ju, J.W.; Ding, W.Q. Full-scale fire tests of RC metro shield TBM tunnel linings. Constr. Build. Mater. 2012, 36, 484–494. [Google Scholar] [CrossRef]
- Yan, Z.G.; Zhu, H.H.; Ju, J.W. Behavior of reinforced concrete and steel fiber reinforced concrete shield TBM tunnel linings exposed to high temperatures. Constr. Build. Mater. 2013, 38, 610–618. [Google Scholar] [CrossRef]
- Yan, Z.; Shen, Y.; Zhu, H.; Lu, Y. Experimental study of tunnel segmental joints subjected to elevated temperature. Tunn. Undergr. Space Technol. 2016, 53, 46–60. [Google Scholar] [CrossRef]
- Ye, J.; Li, J. Fire Experiments of Shield Tunnel Segments and Joints. China J. Highw. Transp. 2022, in press. [Google Scholar]
- Guo, Z.; Song, J.; Liu, Y.; Wang, X.; Liu, Y.; Ma, C.; Li, F. Experimental study on mechanical behavior of splicing joints of shield tunnel lining subjected to fire. China Civ. Eng. J. 2022, 55, 94–104. [Google Scholar] [CrossRef]
- Yin, Y.; Guo, Z.; Song, J.; Liu Yi Wang, X.; Liu, Y.; Li, F. Mechanical behaviour of splicing joints in shield tunnel lining subjected to fire. Tunn. Undergr. Space Technol. 2022, 123, 104404. [Google Scholar] [CrossRef]
- Zhang, W.; Zhang, X.; Song, X. Mechanical properties of shield tunnel with inclined bolt joint and temperature distribution law under fire. J. Traffic Transp. Eng. 2018, 18, 37–49. [Google Scholar] [CrossRef]
- Hua, N.; Tessari, A.; Khorasani, N.E. The effect of geologic conditions on the fire behavior of tunnels considering soil-structure interaction. Tunn. Undergr. Space Technol. 2022, 122, 104380. [Google Scholar] [CrossRef]
- Zhang, Q.; Zhou, C.; Sun, C.; Li, H.; Guo, Z. Study on the Pattern of Temperature Propagation of Reinforced Concrete Structure under High-Temperature Environment. Mod. Tunn. Technol. 2021, 58, 185–193. [Google Scholar] [CrossRef]
- Ding, R.; Fan, S.; Wu, M. Numerical study on fire resistance of rectangular section stainless steel-concrete composite beam. Fire Saf. J. 2021, 125, 103436. [Google Scholar] [CrossRef]
- Zhang, G.; Zhang, W.; Yu, G.; Lel, J. Model Test on Thermomechanical Coupling of Shield Tunnel Lining Under High Fire Temperature. China J. Highw. Transp. 2019, 32, 120–128. [Google Scholar] [CrossRef]
- Saleheen, Z.; Krishnamoorthy, R.R.; Nadjai, A. A review on behavior, material properties and finite element simulation of concrete tunnel linings under fire. Tunn. Undergr. Space Technol. 2022, 126, 104534. [Google Scholar] [CrossRef]
- Eurocode 3; Eurocode 3: Design of Steel Structures—Part 1–2: General Rules—Structural Fire Design. European Committee for Standardization (CEN): Brussels, Belgium, 2005.
- Eurocode 4; Eurocode 4: Design of Composite Steel and Concrete Structures—Part 1–2: General Rules—Structural Fire Design. European Committee for Standardization (CEN): Brussels, Belgium, 2005.
- Guo, Z.; Li, W. Experimental investigation of strength and deformation of concrete at elevated temperature. J. Build. Struct. 1993, 14, 8–16. [Google Scholar]


| Density (kg/m3) | The Axial Compressive Strength of Concrete (N/mm2) | Elasticity Modulus (N/mm2) | Poisson Ratio |
|---|---|---|---|
| 2400 | 40.2 | 35,992.8 | 0.2 |
| Type | Elasticity Modulus (MPa) | Yield Stress (MPa) | Yield Strain | Ultimate Strain | Poisson Ratio |
|---|---|---|---|---|---|
| Steel bar | 2 × 10−11 | 500 | 0.02 | 0.15 | 0.28 |
| H-pin C-slot | 2 × 10−11 | 335 | 0.02 | 0.2 | 0.28 |
| Bolt | 2 × 10−11 | 900 | 0.02 | 0.2 | 0.28 |
| Temperature/°C | 20 | 100 | 200 | 300 | 400 | 500 | 600 | 700 | 800 | 900 |
|---|---|---|---|---|---|---|---|---|---|---|
| 1 | 1 | 0.9 | 0.8 | 0.7 | 0.6 | 0.31 | 0.13 | 0.09 | 0.0675 | |
| 1 | 1 | 1 | 1 | 1 | 0.78 | 0.47 | 0.23 | 0.11 | 0.06 | |
| 1 | 1 | 0.807 | 0.613 | 0.42 | 0.36 | 0.18 | 0.075 | 0.05 | 0.0375 |
| No. | Fire Load | Mid-Span Ultimate Bending Moment (kN·m) | ||
|---|---|---|---|---|
| MA | MB | |||
| RCJ1 | - | 11.38 | 12.30 | 8.08% |
| RCJ3 | HC | 9.04 | 8.56 | 5.31% |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Huang, Z.; Zhang, J.; Peng, Z.; Hu, H.; An, H.; Yang, X.; Xiong, T. Simulation of Thermomechanical Coupling and Evaluation of the Fire Resistance for the Joints of Fabricated Frame Tunnel. Fire 2023, 6, 3. https://doi.org/10.3390/fire6010003
Huang Z, Zhang J, Peng Z, Hu H, An H, Yang X, Xiong T. Simulation of Thermomechanical Coupling and Evaluation of the Fire Resistance for the Joints of Fabricated Frame Tunnel. Fire. 2023; 6(1):3. https://doi.org/10.3390/fire6010003
Chicago/Turabian StyleHuang, Zhen, Jiawei Zhang, Zimao Peng, Hongbo Hu, Huiping An, Xulong Yang, and Tianxiang Xiong. 2023. "Simulation of Thermomechanical Coupling and Evaluation of the Fire Resistance for the Joints of Fabricated Frame Tunnel" Fire 6, no. 1: 3. https://doi.org/10.3390/fire6010003
APA StyleHuang, Z., Zhang, J., Peng, Z., Hu, H., An, H., Yang, X., & Xiong, T. (2023). Simulation of Thermomechanical Coupling and Evaluation of the Fire Resistance for the Joints of Fabricated Frame Tunnel. Fire, 6(1), 3. https://doi.org/10.3390/fire6010003








