Experimental and Numerical Analysis of Thermo-Mechanical Behaviour of Glass Panes Exposed to Radiant Heating
Abstract
1. Introduction
1.1. Background
1.2. Aims, Scope and Limitations
2. Breakage of Glass at Elevated Temperature
2.1. Strength of Structural Glass
2.2. Previous Studies on Glass Breakage at Elevated Temperature
3. Summary of Experiments
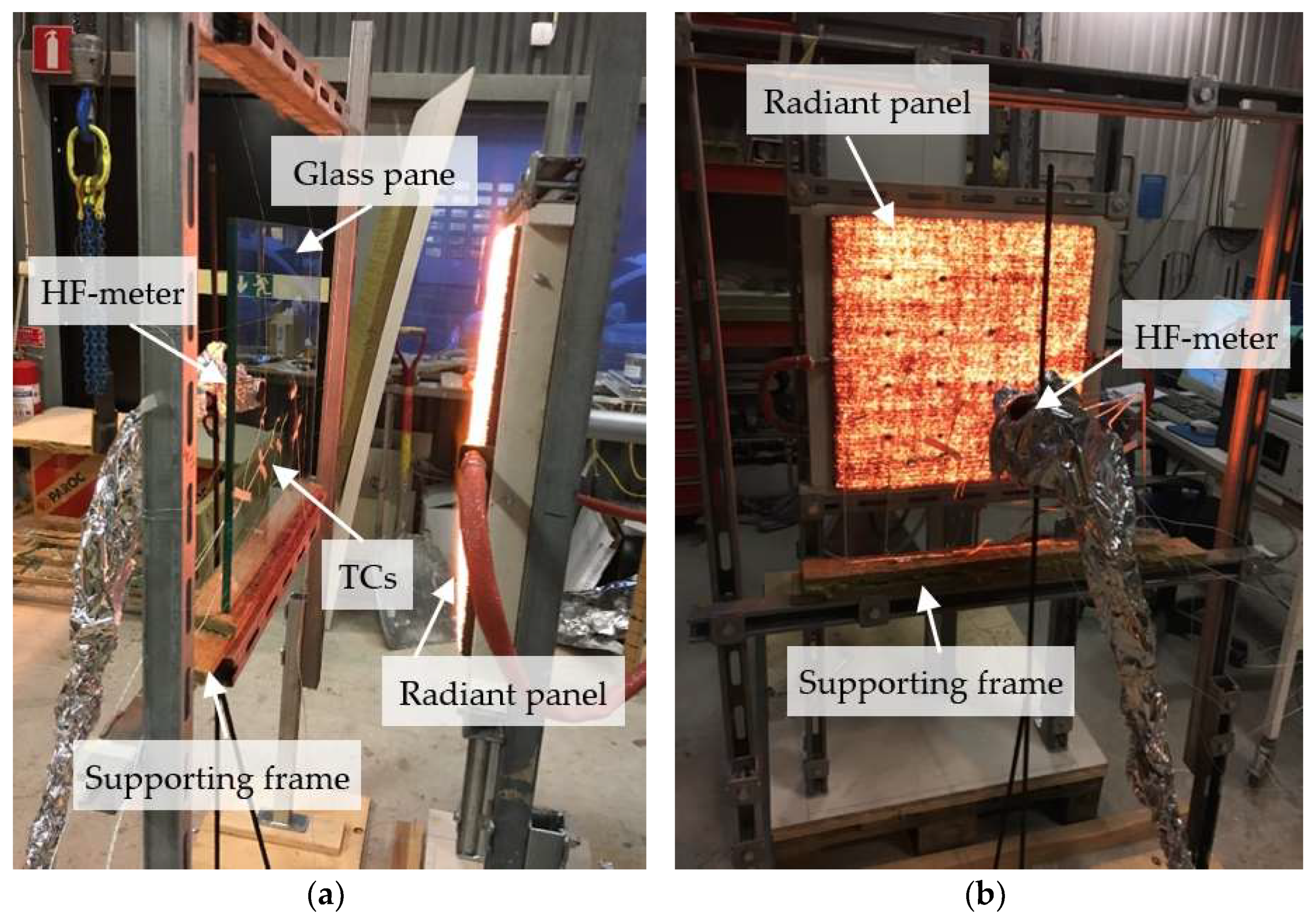
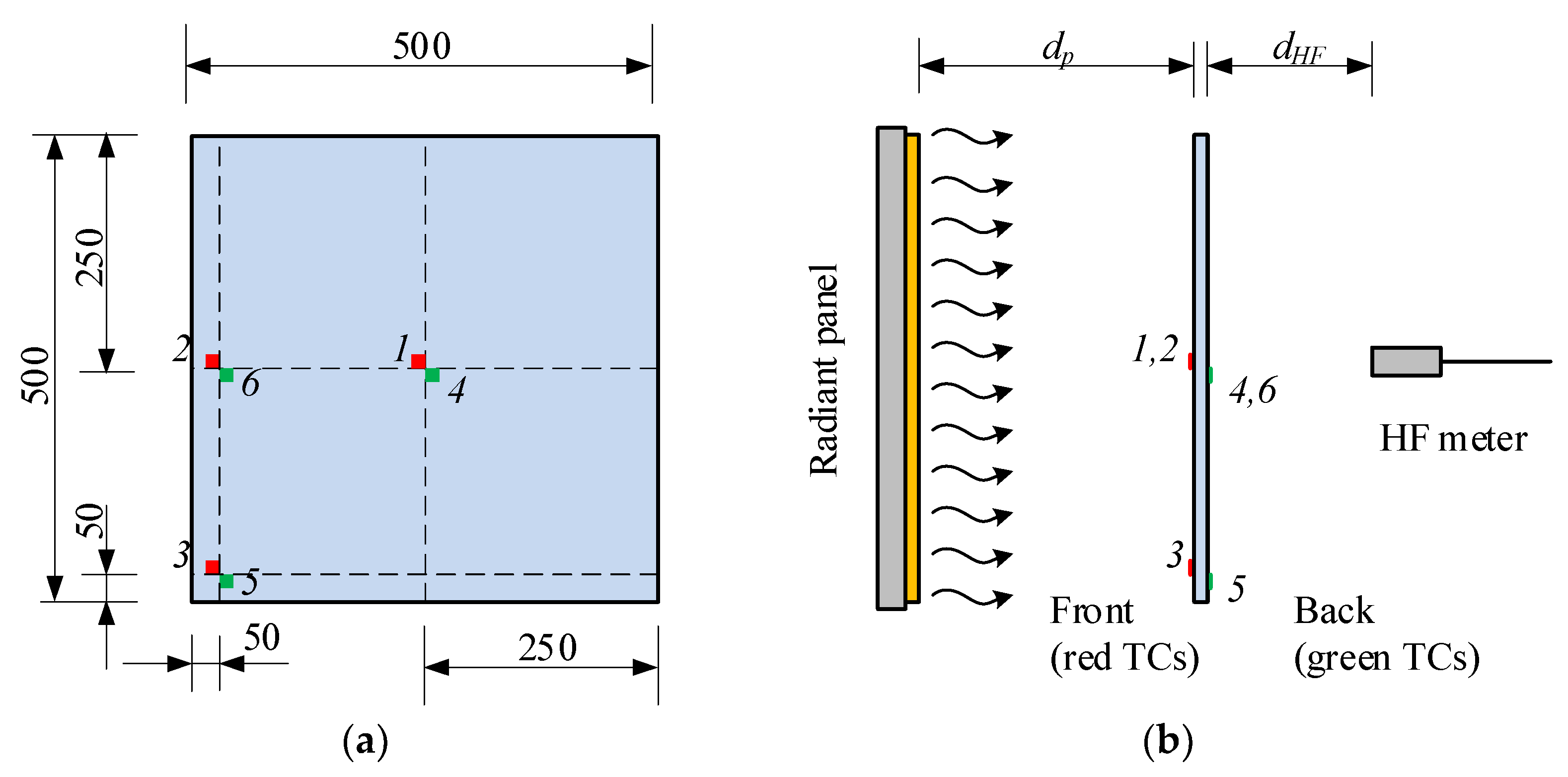
3.1. Testing Programme
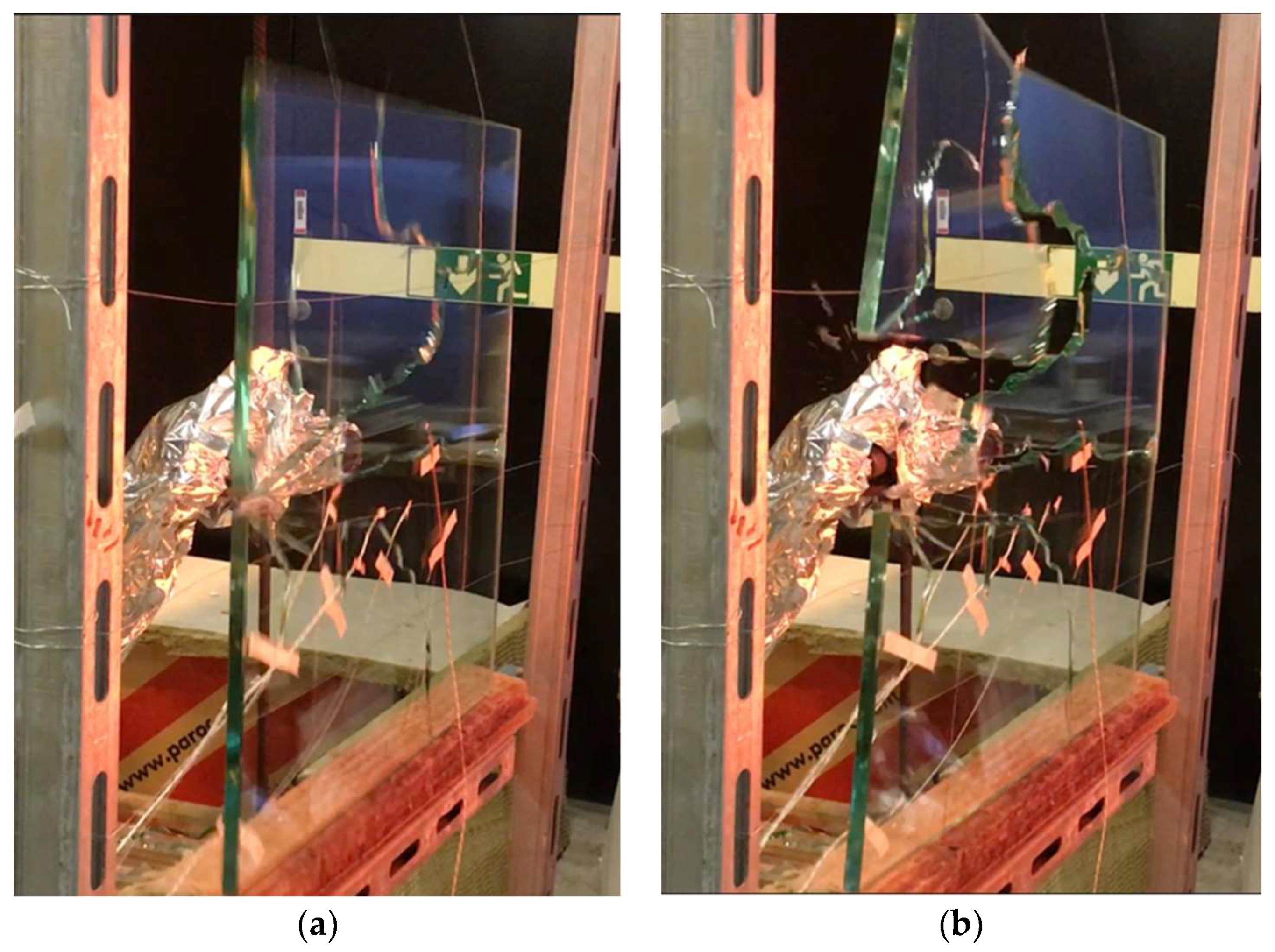
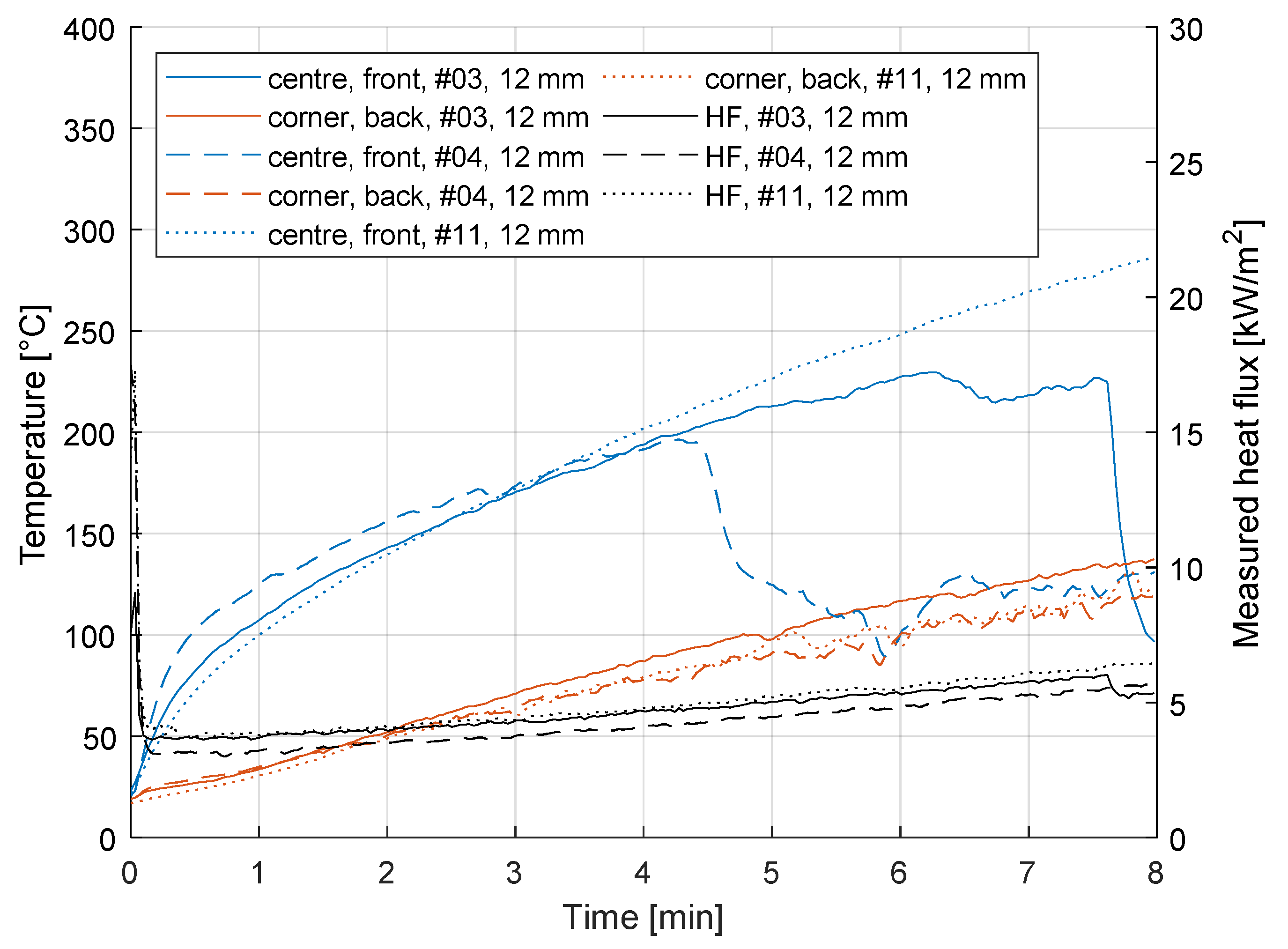
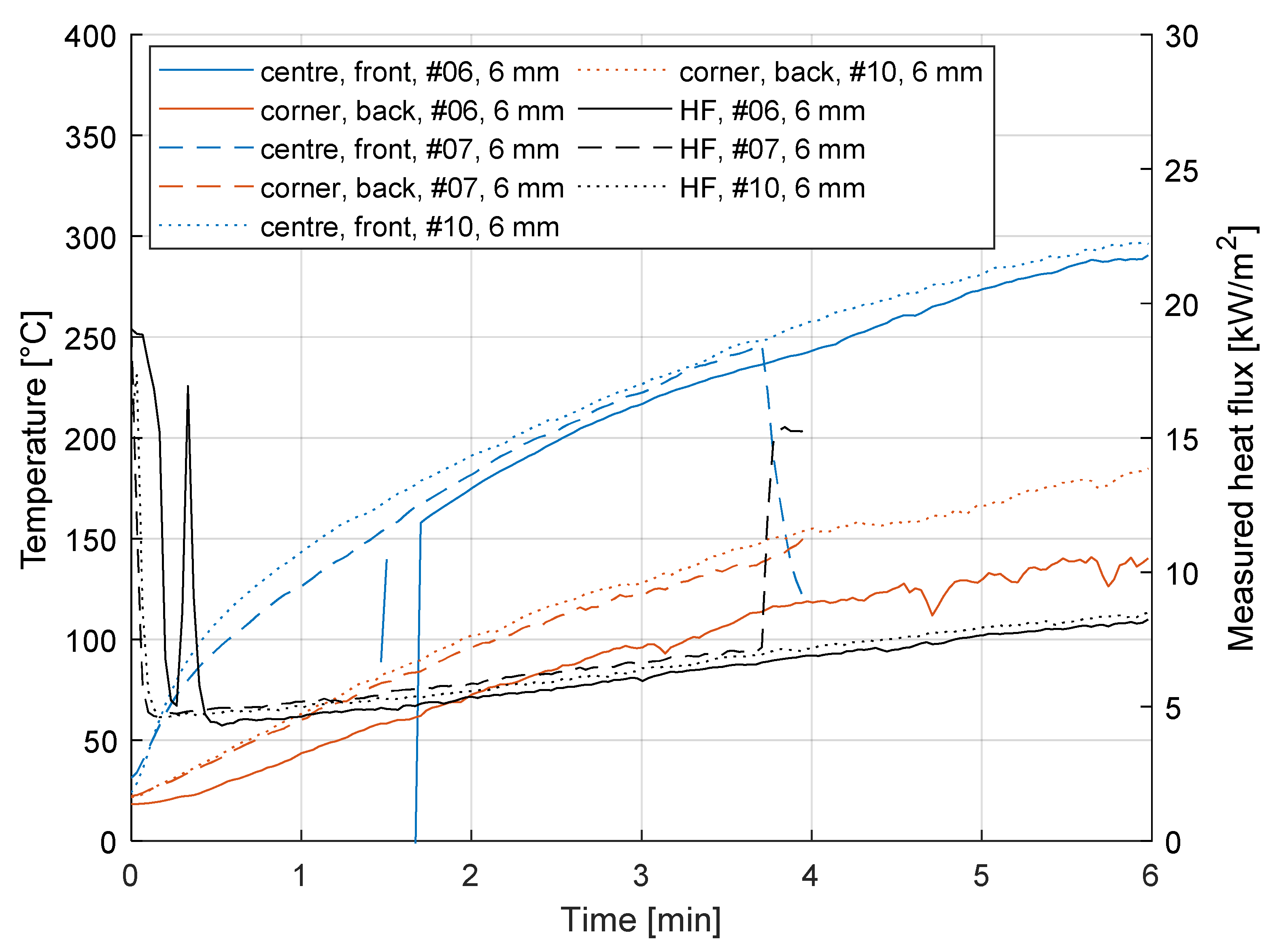
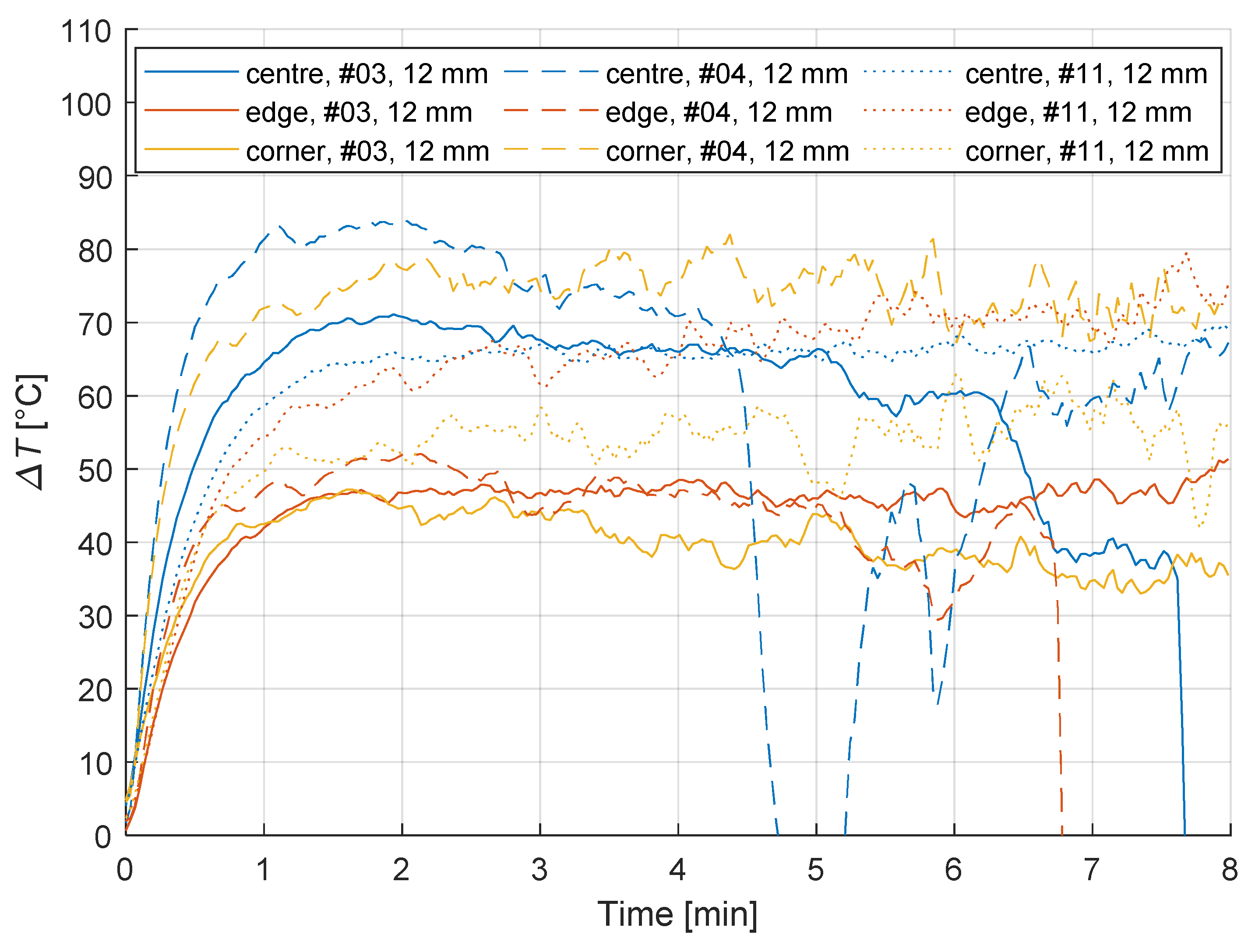
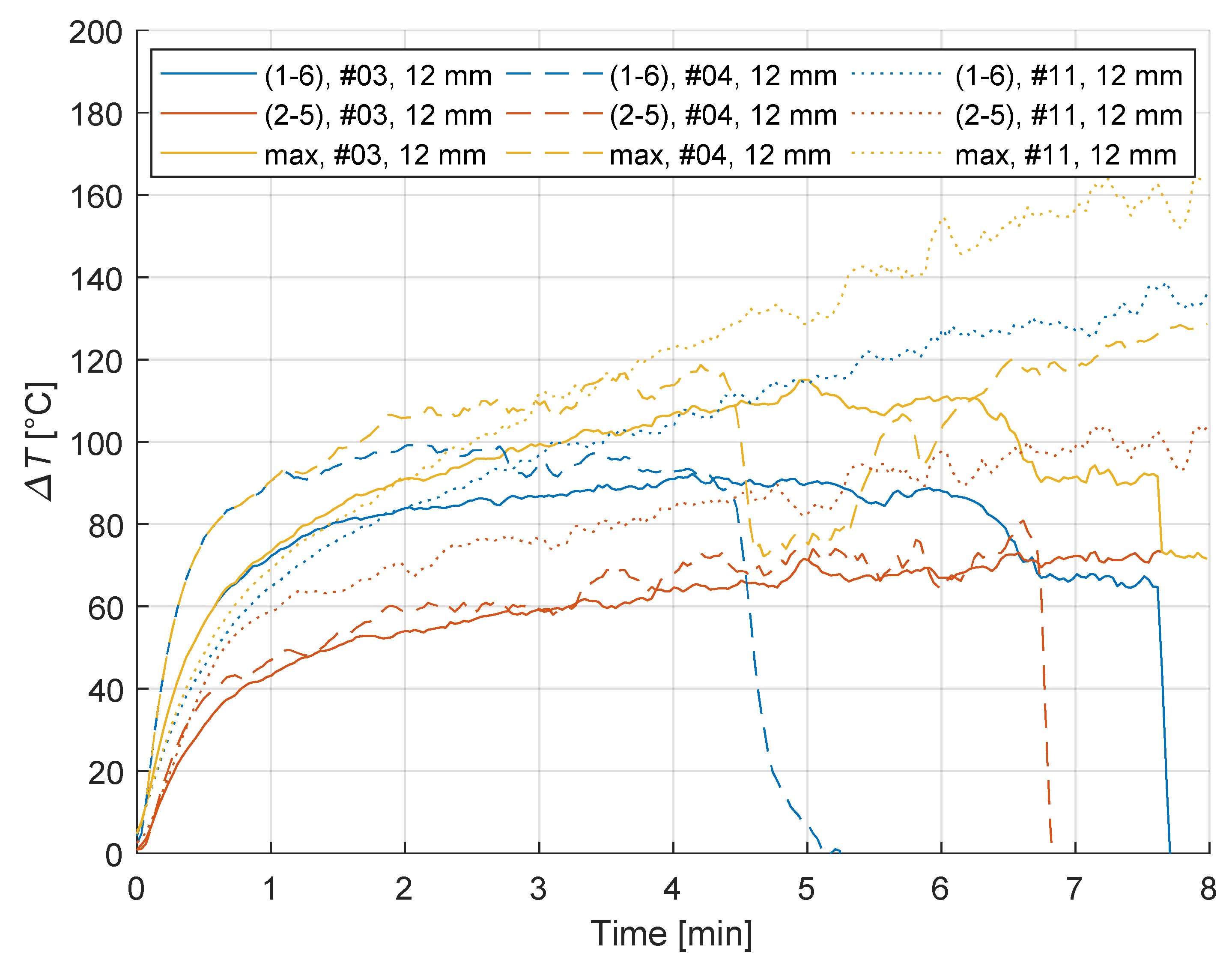
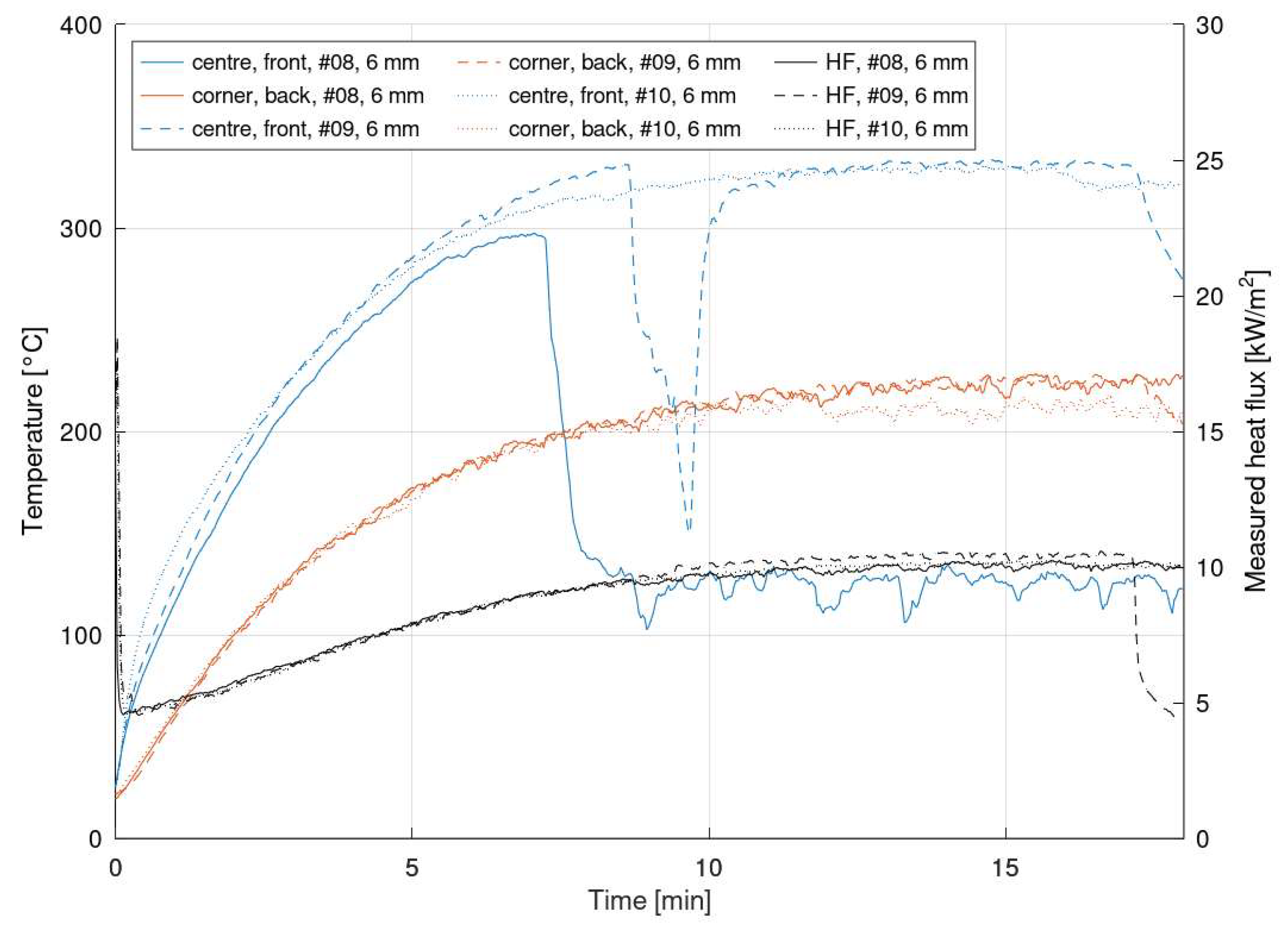
3.2. Test Results
3.3. Transmittance and Effect of Coating
4. Heat Transfer in Glass and Numerical Modelling
4.1. Heat Transfer in Glass
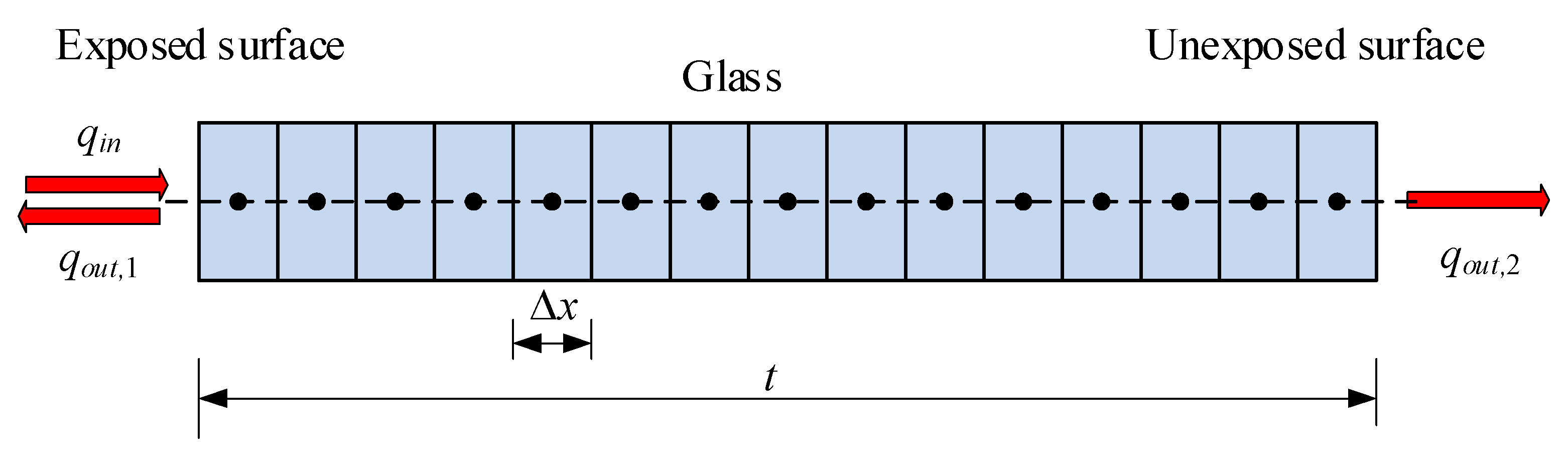
4.2. Description of the 1D Model
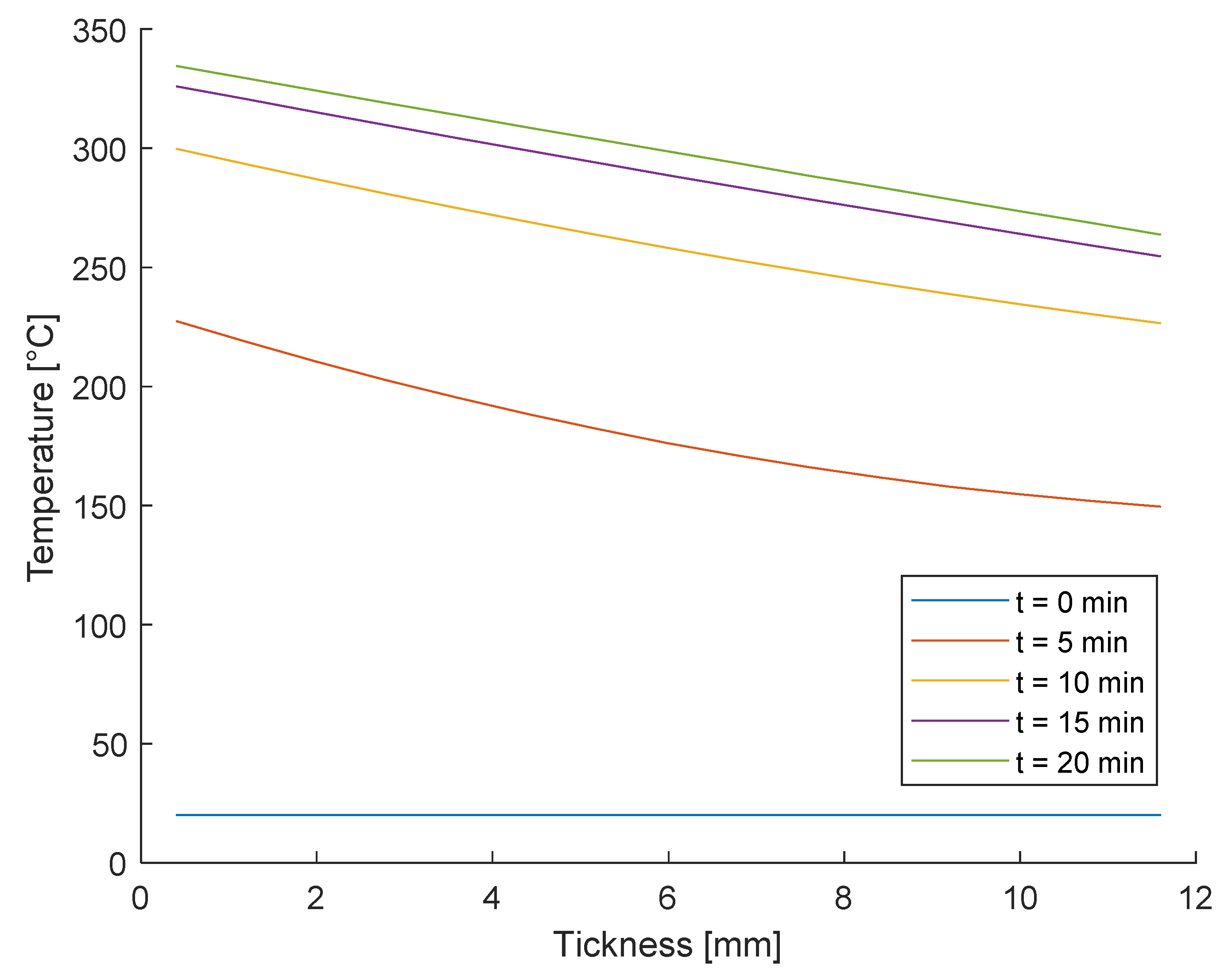
4.3. 1D model Results
5. Thermo-Mechanical Finite Element Model
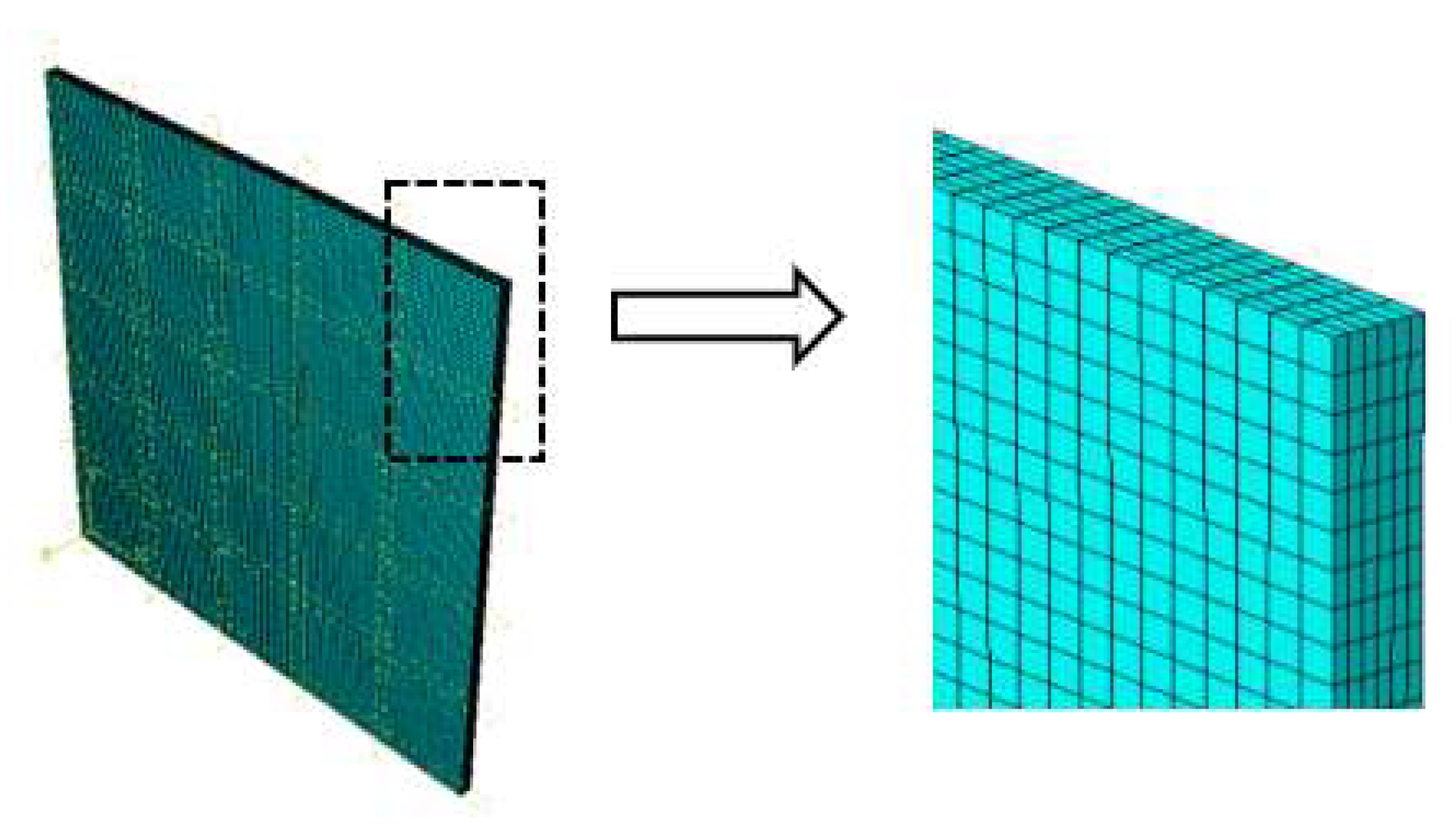
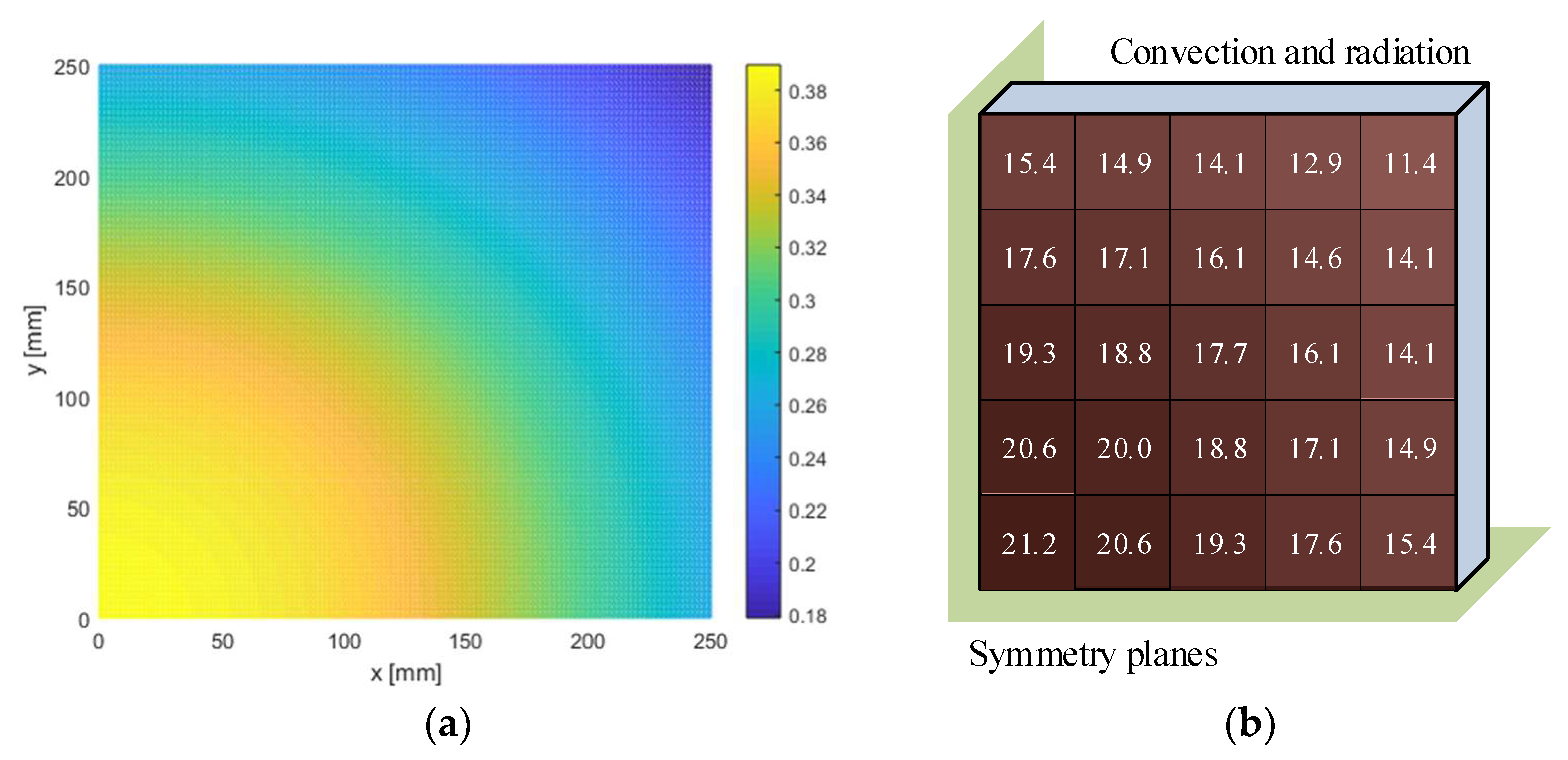
5.1. Modelling Assumptions
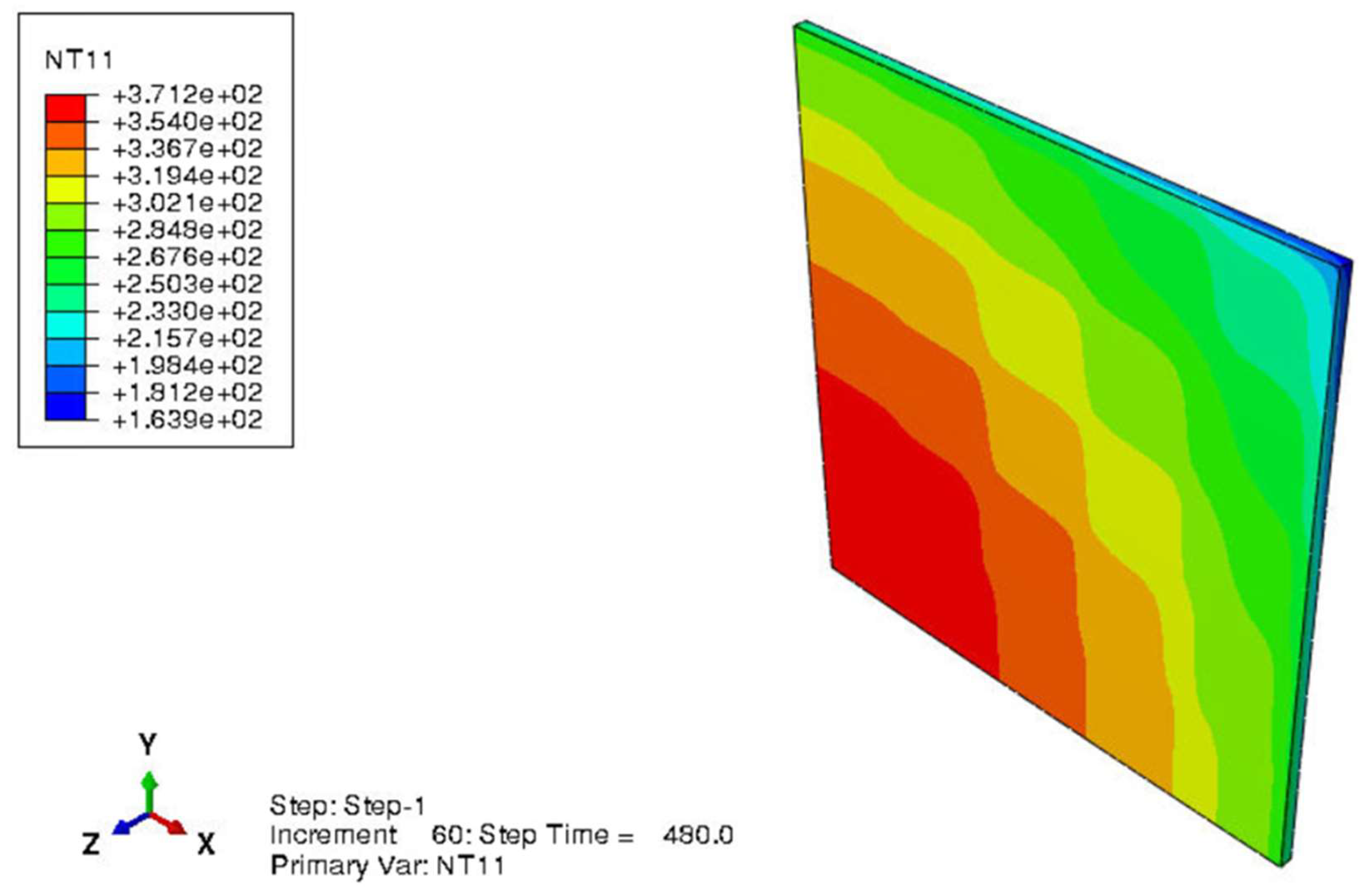
5.2. 3D Modelling Results and Discussion
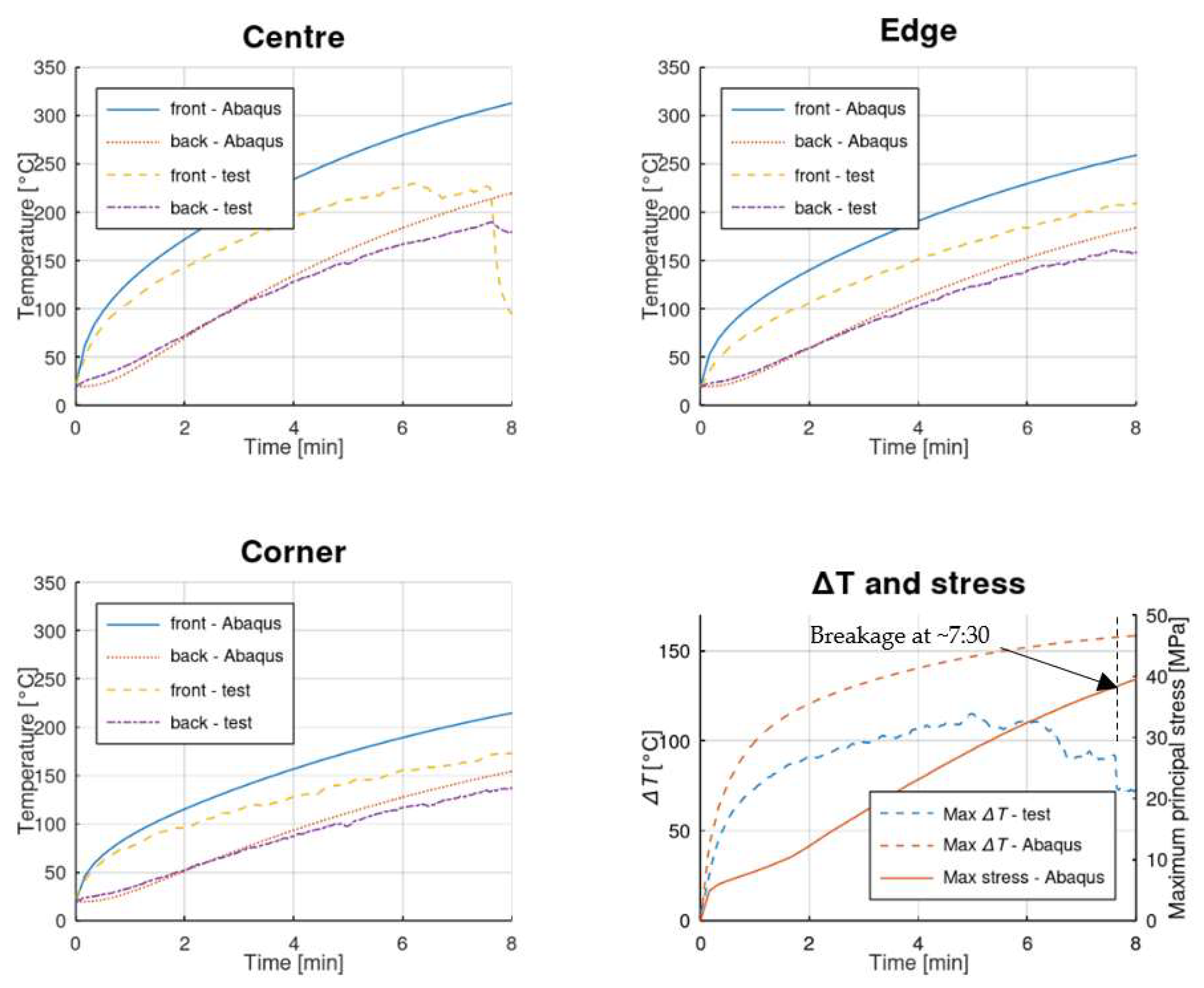
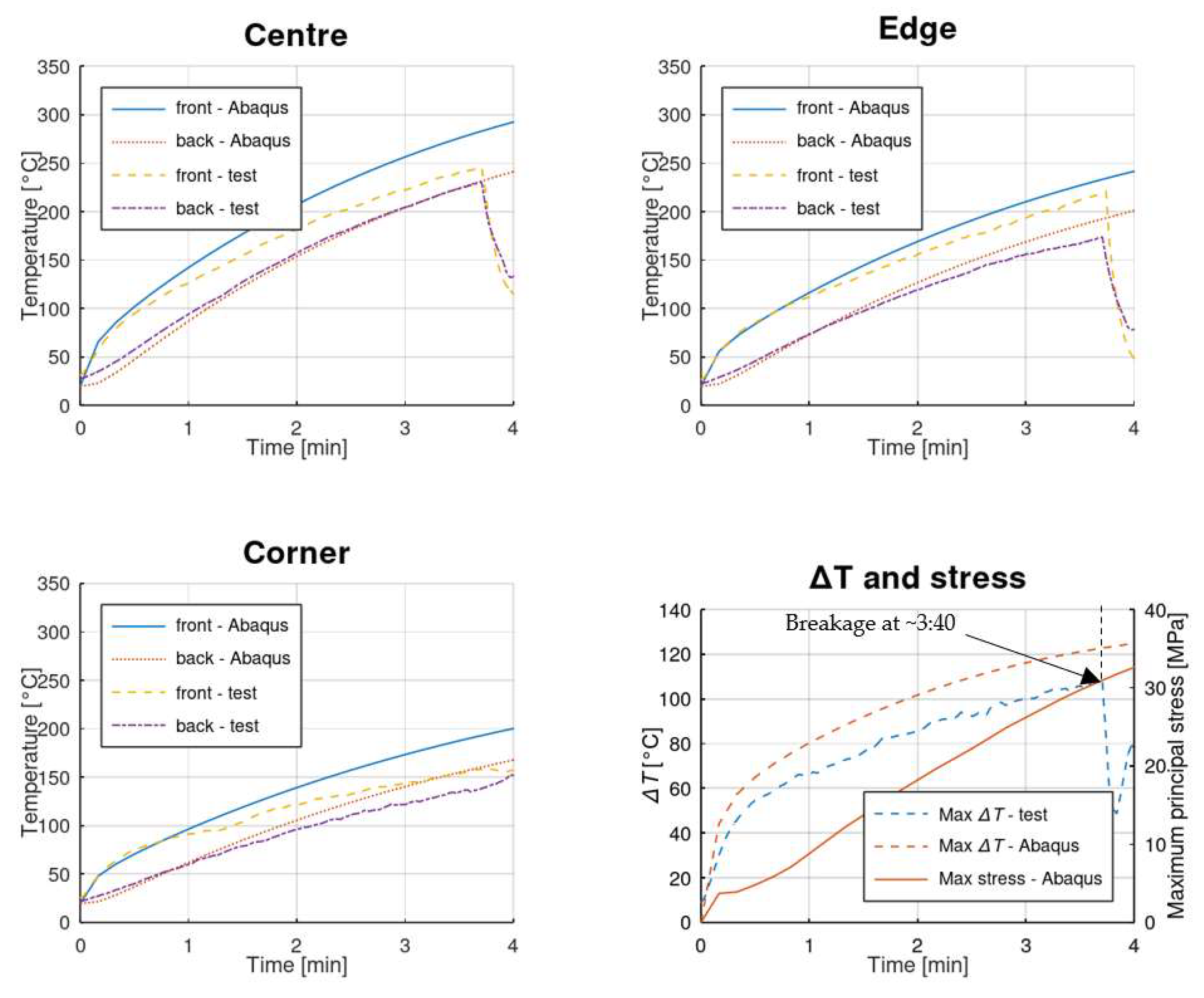
5.3. 3D Model vs. Test Results
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Brzezicki, M. Dynamic Perception of Transparency in Architecture: Mechanisms of the Proper Recognition of Light Permeable Surfaces. Compr. Psychology Compr. Psychol. 2014, 3, 7. [Google Scholar] [CrossRef]
- Haldimann, M.; Luible, A.; Overend, M. Structural Use of Glass; International Association for Bridge and Structural Engineering (IABSE): Zurich, Switzerland, 2008. [Google Scholar]
- Bos, F.P. Safety Concepts in Structural Glass Engineering—Towards an Integrated approach. Ph.D. Thesis, TU Delft, Delft, The Netherlands, 2009. [Google Scholar]
- Honfi, D.; Lange, D.; Kozłowski, M.; Sjöström, J.; Lenk, P. Behavior of load-bearing glass at elevated temperature. ce/papers 2018, 2, 533–540. [Google Scholar] [CrossRef]
- Feldmann, M.; Kasper, R.; Abeln, B.; Cruz, P.; Belis, J.; Beyer, J.; Colvin, J.; Ensslen, F.; Eliasova, M.; Galuppi, L.; et al. Guidance for European Structural Design of Glass Components: Support to the Implementation, Harmonization and Further Development of the Eurocodes. Scientific and Policy Report by the Joint Research Centre of the European Commission; EUR: Luxembourg, 2014. [Google Scholar]
- Bedon, C. Structural Glass Systems under Fire: Overview of Design Issues, Experimental Research, and Developments, Advances in Civil Engineering. Adv. Civ. Eng. 2017, 2017, 2120570. [Google Scholar]
- Bao, M.; Gregson, S. Sensitivity study on climate induced internal pressure within cylindrical curved IGUs. Glass Struct. Eng. 2019, 4, 29. [Google Scholar] [CrossRef]
- Bedon, C.; Zhang, X.; Santos, F.; Honfi, D.; Kozłowski, M.; Arrigoni, M.; Figuli, L.; Lange, D. Performance of structural glass facades under extreme loads—Design methods, existing research, current issues and trends. Constr. Build. Mater. 2018, 163, 921–937. [Google Scholar] [CrossRef]
- Wang, Q.; Wang, Y.; Zhang, Y.; Chen, H.; Sun, J.; He, L. A stochastic analysis of glass crack initiation under thermal loading. Appl. Therm. Eng. 2014, 67, 447–457. [Google Scholar] [CrossRef]
- Wang, Y.; Wang, Q.; Sun, J.; He, L.; Liew, K.M. Influence of fire location on the thermal performance of glass façades. Appl. Therm. Eng. 2016, 106, 438–442. [Google Scholar] [CrossRef]
- Vandebroek, M. Thermal Fracture of Glass. Ph.D. Thesis, Ghent University, Ghent, Belgium, University of Antwerp, Antwerp, Belgium, 2014. [Google Scholar]
- Wang, Y.; Wang, Q.; Shao, G.; Chen, H.; Sun, J.; He, L. Experimental study on critical breaking stress of float glass under elevated temperature. Mater. Des. 2014, 60, 41–49. [Google Scholar]
- Debuyser, M.; Sjöström, J.; Lange, D.; Honfi, D.; Sonck, D.; Belis, J. Behaviour of monolithic and laminated glass exposed to radiant heating. Constr. Build. Mater. 2017, 130, 212–229. [Google Scholar] [CrossRef]
- Wang, Y. Experimental and Numerical Study of Glass Façades Breakage Behavior under Fire Conditions; Springer: Singapore, 2019; p. 137. [Google Scholar]
- prEN Thstr: 2004; Glass in Building: Thermal Stress Calculation Method. European Committee for Standardization (CEN): Brussels, Belgium, 2014.
- Louter, C.; Bedon, C.; Kozłowski, M.; Nussbaumer, A. Structural response of fire-exposed laminated glass beams under sustained loads; exploratory experiments and FE-Simulations. Fire Saf. J. 2021, 123, 103353. [Google Scholar] [CrossRef]
- Vedrtnam, A.; Bedon, C.; Youssef, M.; Chaturvedi, S. Effect of non-uniform temperature exposure on the out-of-plane bending performance of ordinary laminated glass panels. Comp. Struct. 2021, 275, 114517. [Google Scholar] [CrossRef]
- Kozłowski, M.; Bedon, C. Sensitivity to Input Parameters of Failure Detection Methods for Out-of-Plane Loaded Glass Panels in Fire. Fire 2021, 4, 5. [Google Scholar] [CrossRef]
- Ferreira, D.M.; Araújo, A.; Fonseca, E.M.M.; Piloto, P.A.G.; Pinto, J. Behaviour of non-loadbearing tabique wall subjected to fire—Experimental and numerical analysis. J. Build. Eng. 2017, 9, 164–176. [Google Scholar] [CrossRef][Green Version]
- Rossi, M.L.; Simões, Y.S.; Munaiar Neto, J.; Castro, A.L. Thermal numerical analysis of steel and steel and concrete composite columns in a fire situation aimed at the evaluation of the simplified method of ABNT NBR 14323:2013. Rev. IBRACON Estrut. Mater. 2021, 14, e14502. [Google Scholar] [CrossRef]
- Drozdzol, K. CFD thermal analysis of a three-layer chimney used in residential buildings. J. Build. Eng. 2021, 44, 102665. [Google Scholar] [CrossRef]
- Mckeen, P.; Liao, Z. The impact of horizontal projections on lateral fire spread in multi-unit residential buildings—comparison of numerical and similarity correlations. Fire Saf. J. 2021, 126, 103441. [Google Scholar] [CrossRef]
- Mathworks, Inc. Release 2018a; The MathWorks Inc.: Natick, MA, USA, 2018. [Google Scholar]
- Moses, M. Numerically Solving the 1D Transient Heat Equation. 2017. Available online: https://hackaday.io/project/21642-repkiln/log/65537-numerically-solving-the-1d-transient-heat-equation (accessed on 15 July 2019).
- Kozłowski, M.; Bedon, C.; Honfi, D. Numerical Analysis and 1D/2D Sensitivity Study for Monolithic and Laminated Structural Glass Elements under Thermal Exposure. Materials 2018, 11, 1447. [Google Scholar] [CrossRef] [PubMed]
- Simulia. ABAQUS v. 6.14; Computer Software and Online Documentation; Dassault Systems: Providence, RI, USA, 2018. [Google Scholar]
- Howell, J.R. A Catalog of Radiation Configuration Factors; McGraw-Hill: New York, NY, USA, 1982. [Google Scholar]
- Sjöström, J.; Kozłowski, M.; Honfi, D.; Lange, D.; Albrektsson, J.; Lenk, P.; Eriksson, J. Fire Resistance Testing of a Timber-Glass Composite Beam. Int. J. Struct. Glass Adv. Mater. Res. 2020, 4, 24–40. [Google Scholar] [CrossRef]




| Glass Type | Thickness [mm] | Surface | Number of Specimens * |
|---|---|---|---|
| AN | 6 | uncoated | 3 |
| AN | 12 | uncoated | 2 |
| HS | 12 | uncoated | 3 |
| FT | 6 | uncoated | 2 |
| FT | 6 | low-e coating | 1 |
| Test No. | Type/Thickness | Emissive Power (kW/m2) | dp/dHF | Breakage | Remark |
|---|---|---|---|---|---|
| #1 | AN.12 | 64.7 | N/A | Yes | Measurement only in the middle |
| #2 | AN.12 | 64.7 | N/A | Yes | Measurement only in the middle |
| #3 | AN.12 | 64.7 | 330/90 | 7:30 | TC connection problems |
| #4 | HS.12 | 64.7 | 350/70 | No | Panel off for 1–2 s, TC connection problems |
| #5 | HS.12 | 58.0, 64.7, 70.2 | 350/70 | No | Same specimen as in #4 |
| #6 | AN.6 | 64.7 | 350/69 | 5:30 | Problems with glass placing |
| #7 | AN.6 | 64.7 | 350/69 | 3:40 | |
| #8 | FT.6 | 64.7 | 350/65 | No | TC1 lost during the test |
| #9 | FT.6 | 64.7 | 340/70 | No | Specimen with low-e coating, some issues with the tape, TC1 lost |
| #10 | FT.6 | 64.7 | 350/80 | No | Hall gate was opened during the test |
| #11 | HS.12 | 64.7 | 350/75 | TCs: no glue applied, some HF measurement through the glass in the beginning |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Honfi, D.; Sjöström, J.; Bedon, C.; Kozłowski, M. Experimental and Numerical Analysis of Thermo-Mechanical Behaviour of Glass Panes Exposed to Radiant Heating. Fire 2022, 5, 124. https://doi.org/10.3390/fire5040124
Honfi D, Sjöström J, Bedon C, Kozłowski M. Experimental and Numerical Analysis of Thermo-Mechanical Behaviour of Glass Panes Exposed to Radiant Heating. Fire. 2022; 5(4):124. https://doi.org/10.3390/fire5040124
Chicago/Turabian StyleHonfi, Dániel, Johan Sjöström, Chiara Bedon, and Marcin Kozłowski. 2022. "Experimental and Numerical Analysis of Thermo-Mechanical Behaviour of Glass Panes Exposed to Radiant Heating" Fire 5, no. 4: 124. https://doi.org/10.3390/fire5040124
APA StyleHonfi, D., Sjöström, J., Bedon, C., & Kozłowski, M. (2022). Experimental and Numerical Analysis of Thermo-Mechanical Behaviour of Glass Panes Exposed to Radiant Heating. Fire, 5(4), 124. https://doi.org/10.3390/fire5040124







