Geometrical Analysis of the Stagnation Zone in Laterally Colliding Plasmas: Effects of Plasma Plume Separation and Ablating Target Material
Abstract
1. Introduction
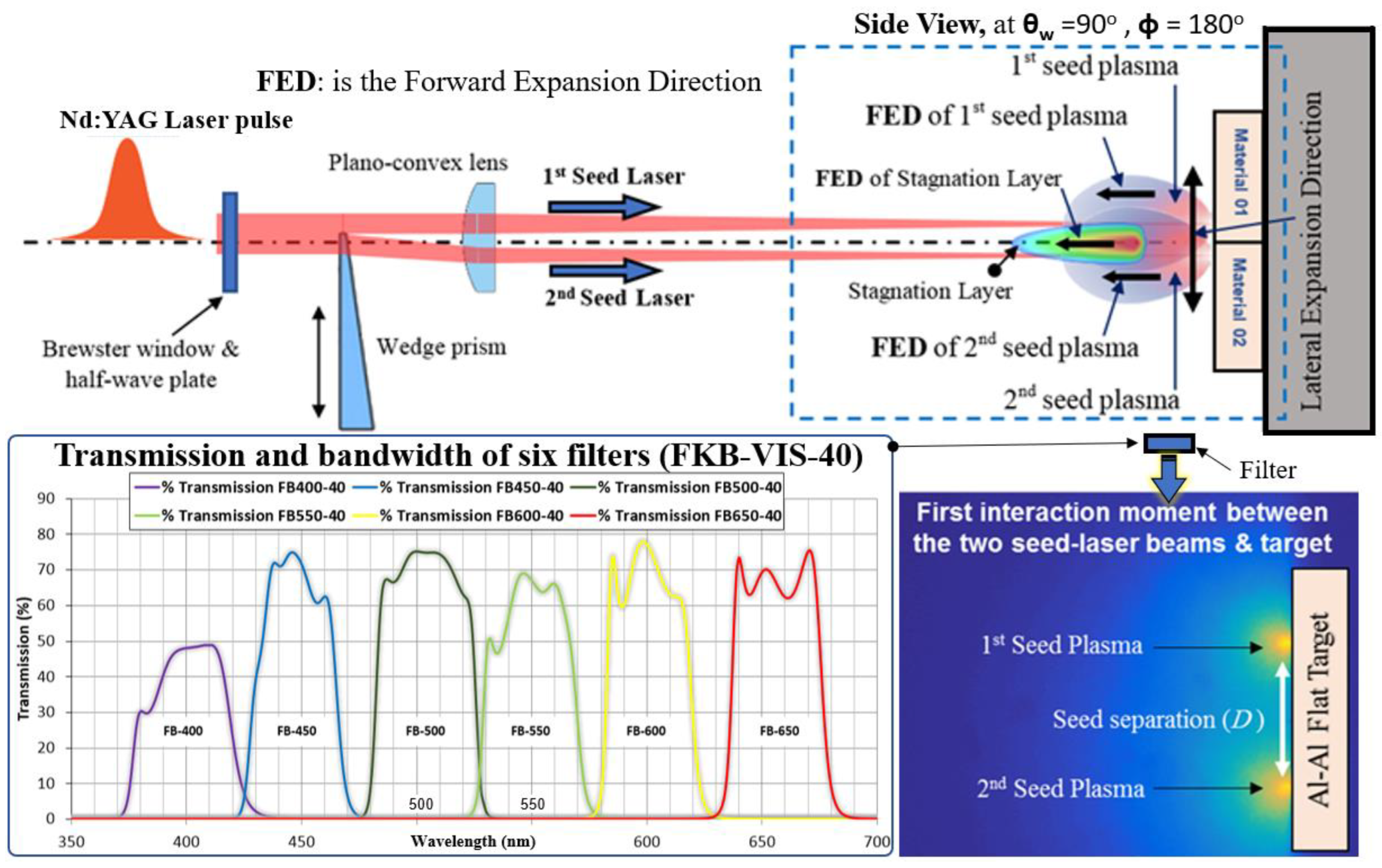
2. Materials and Methods
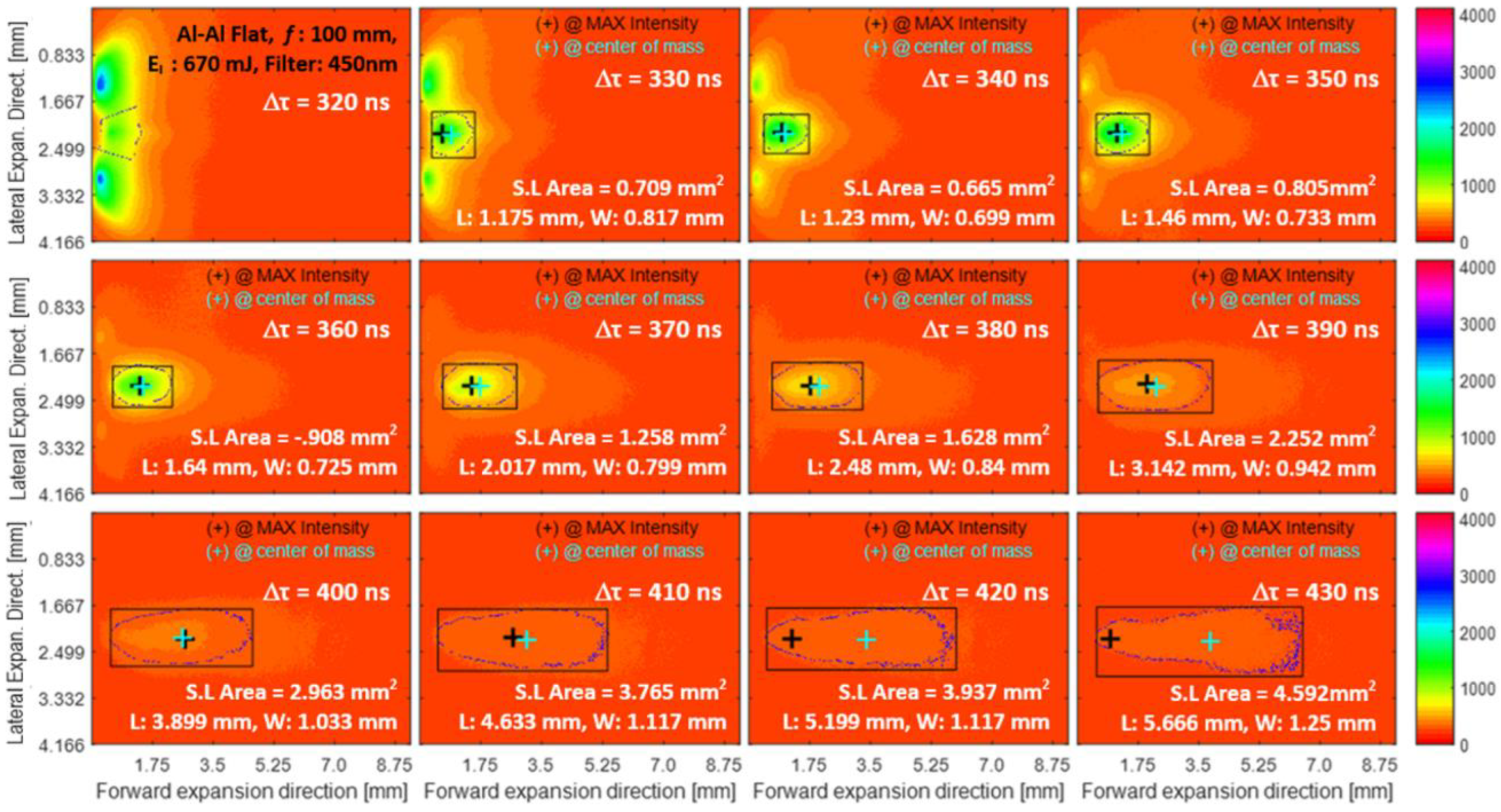
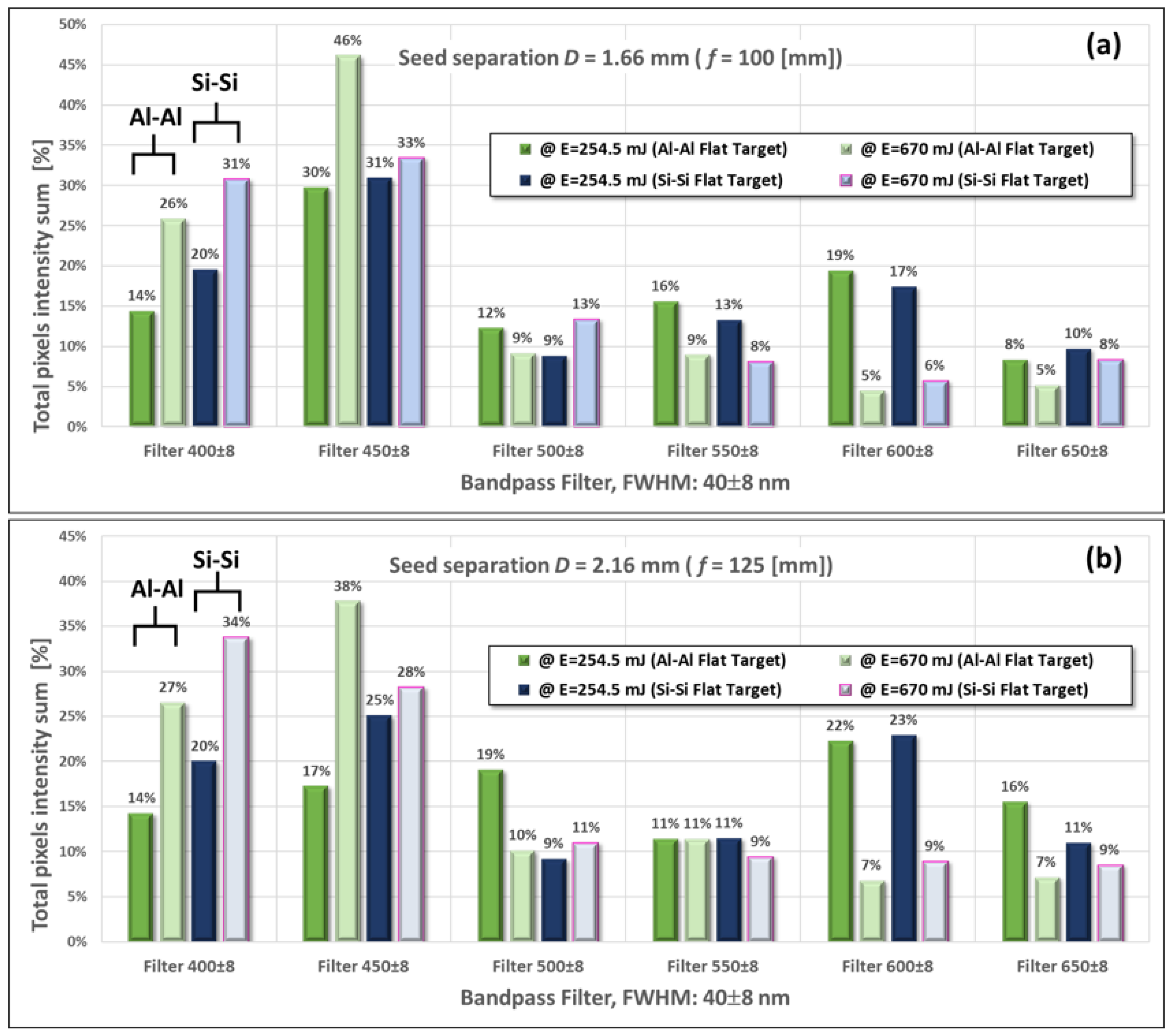
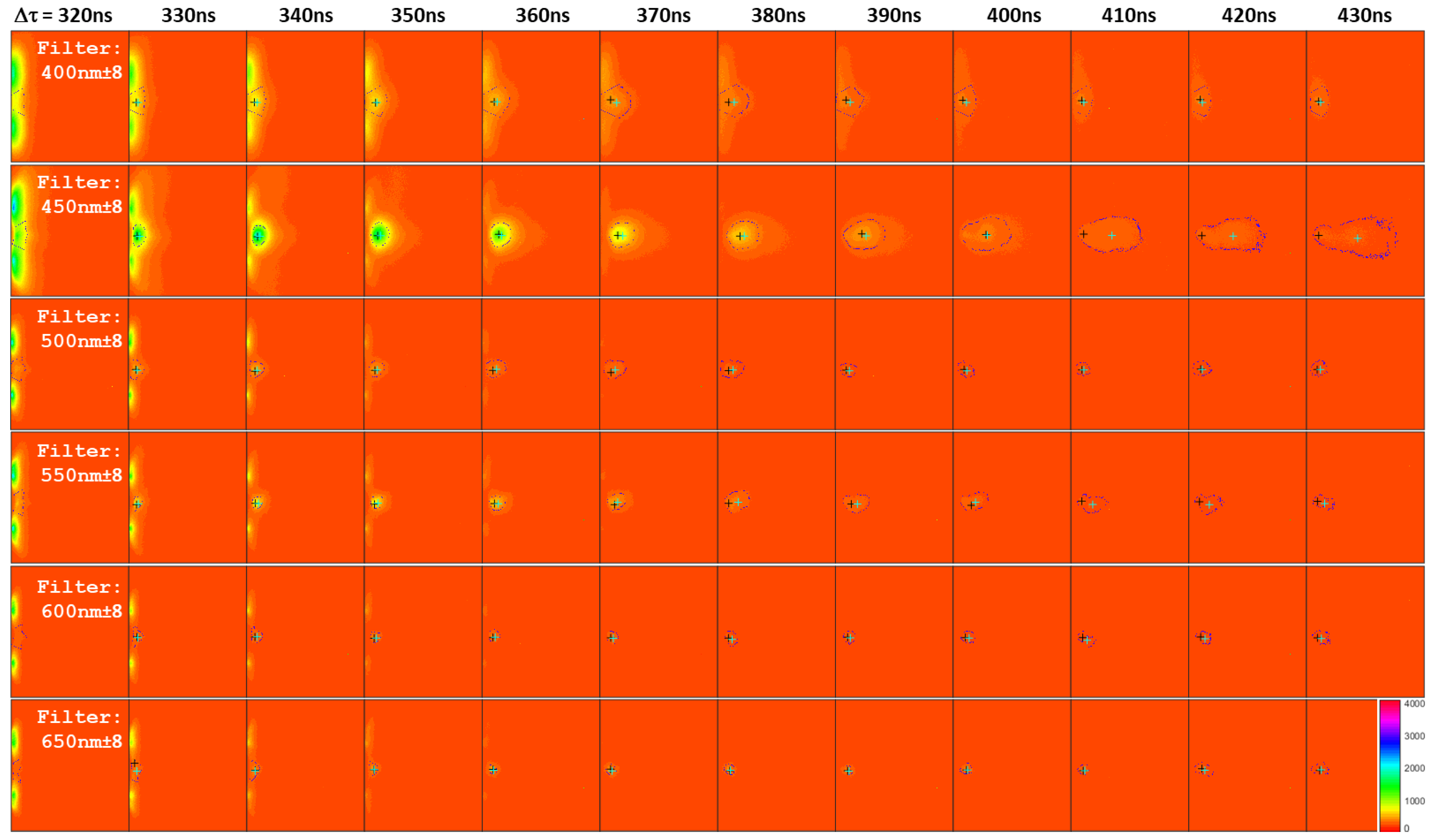
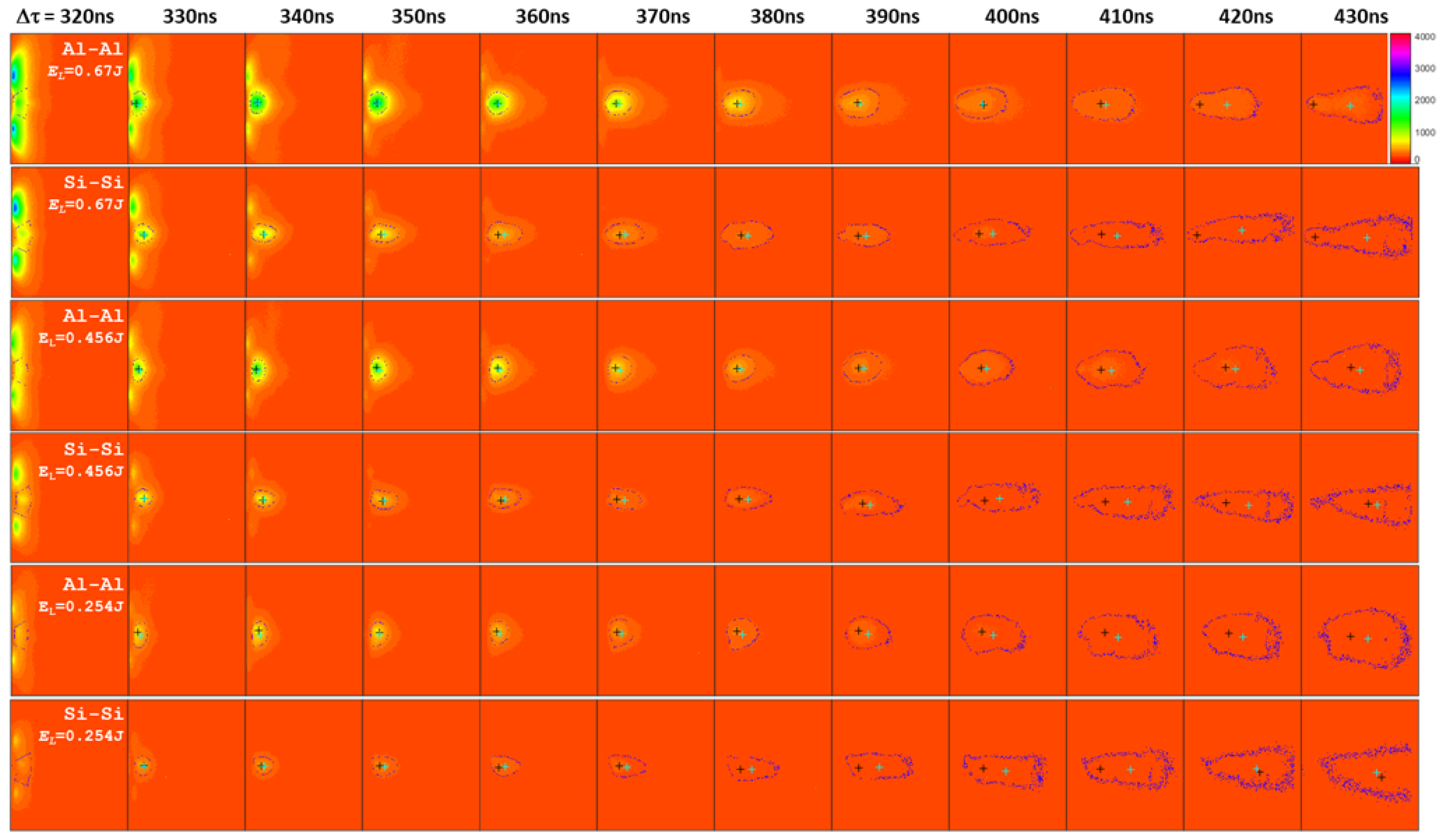
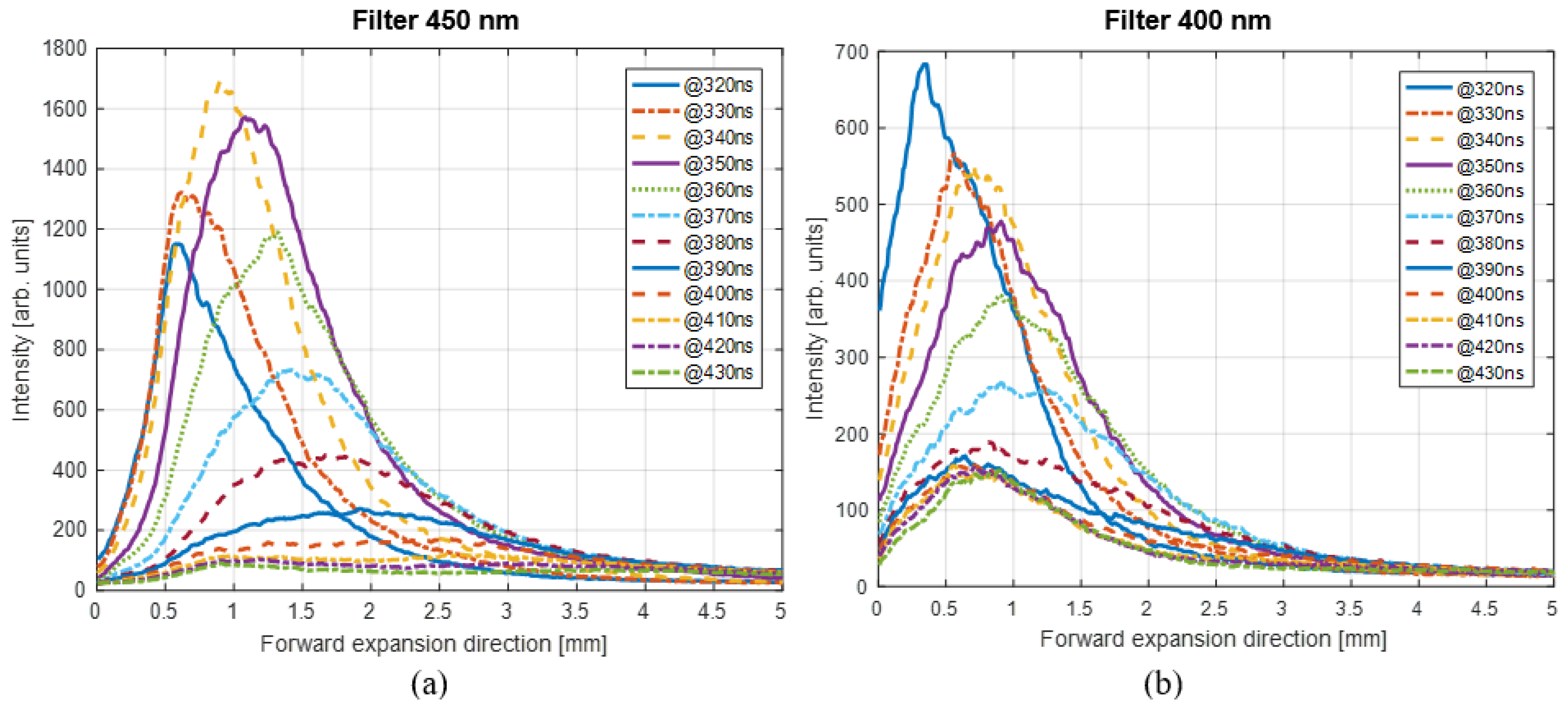
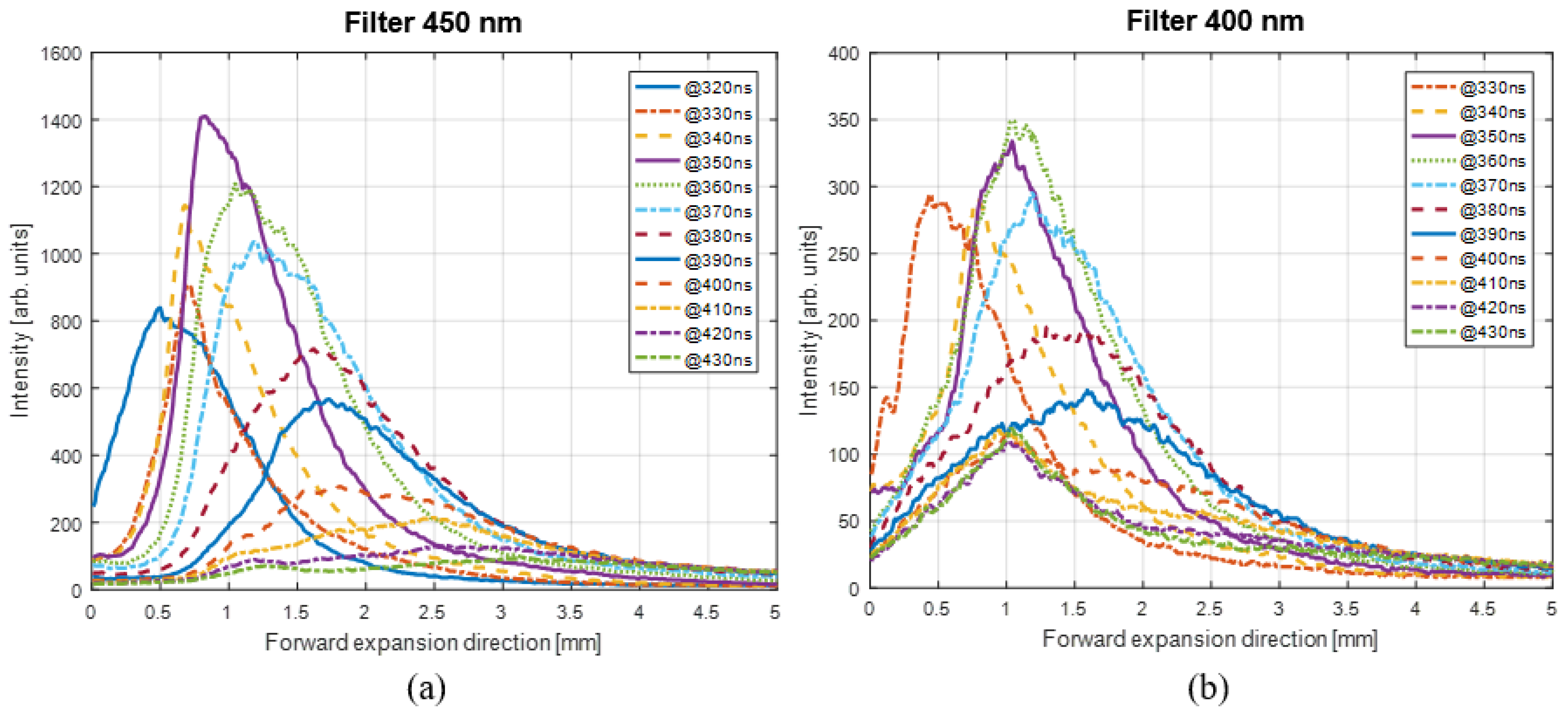
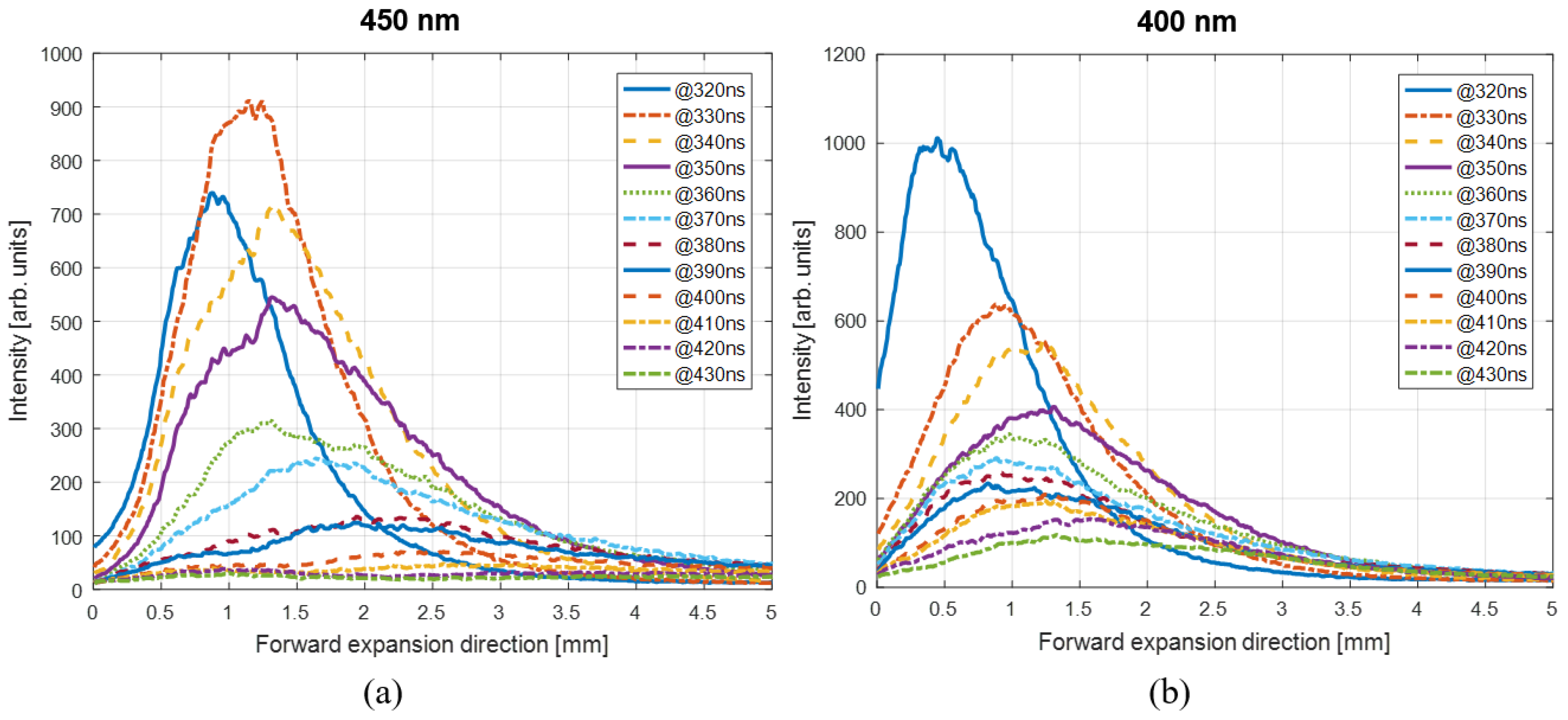
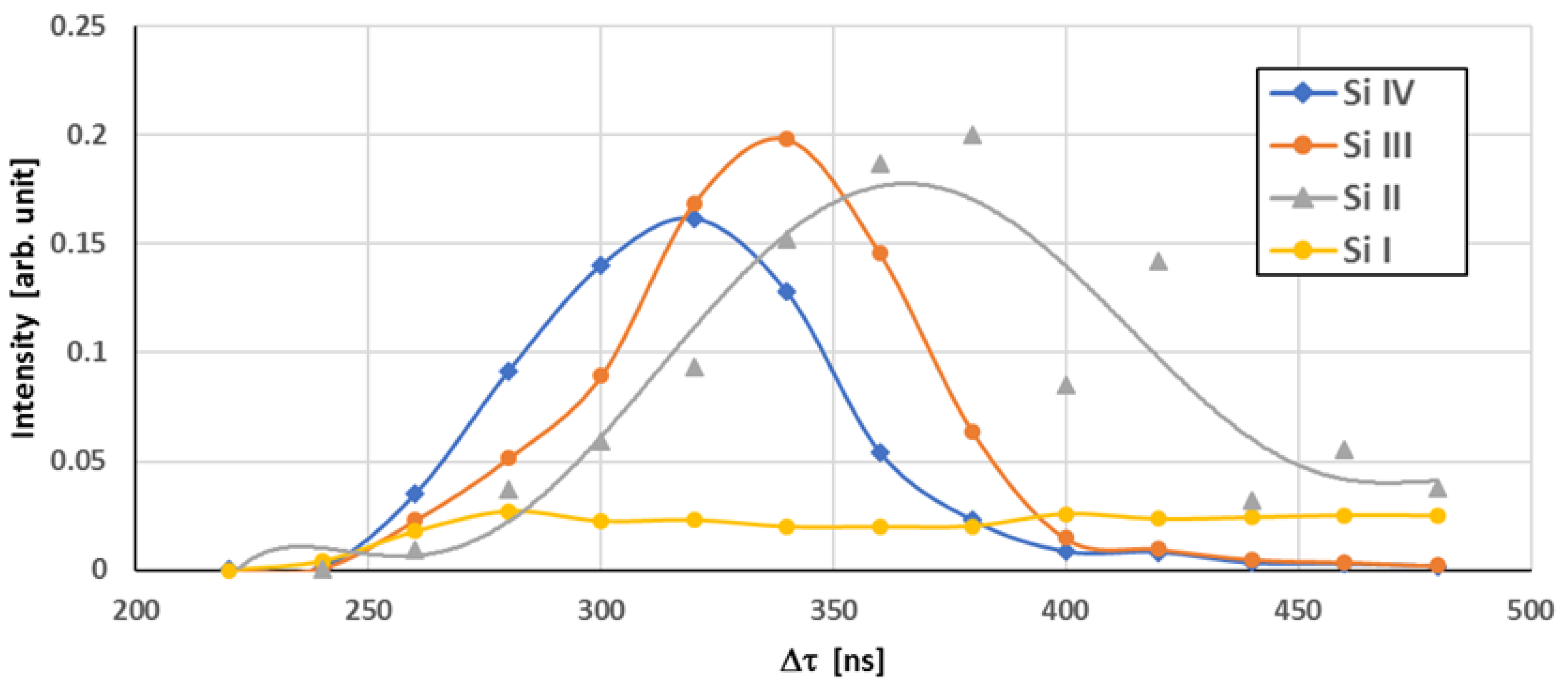
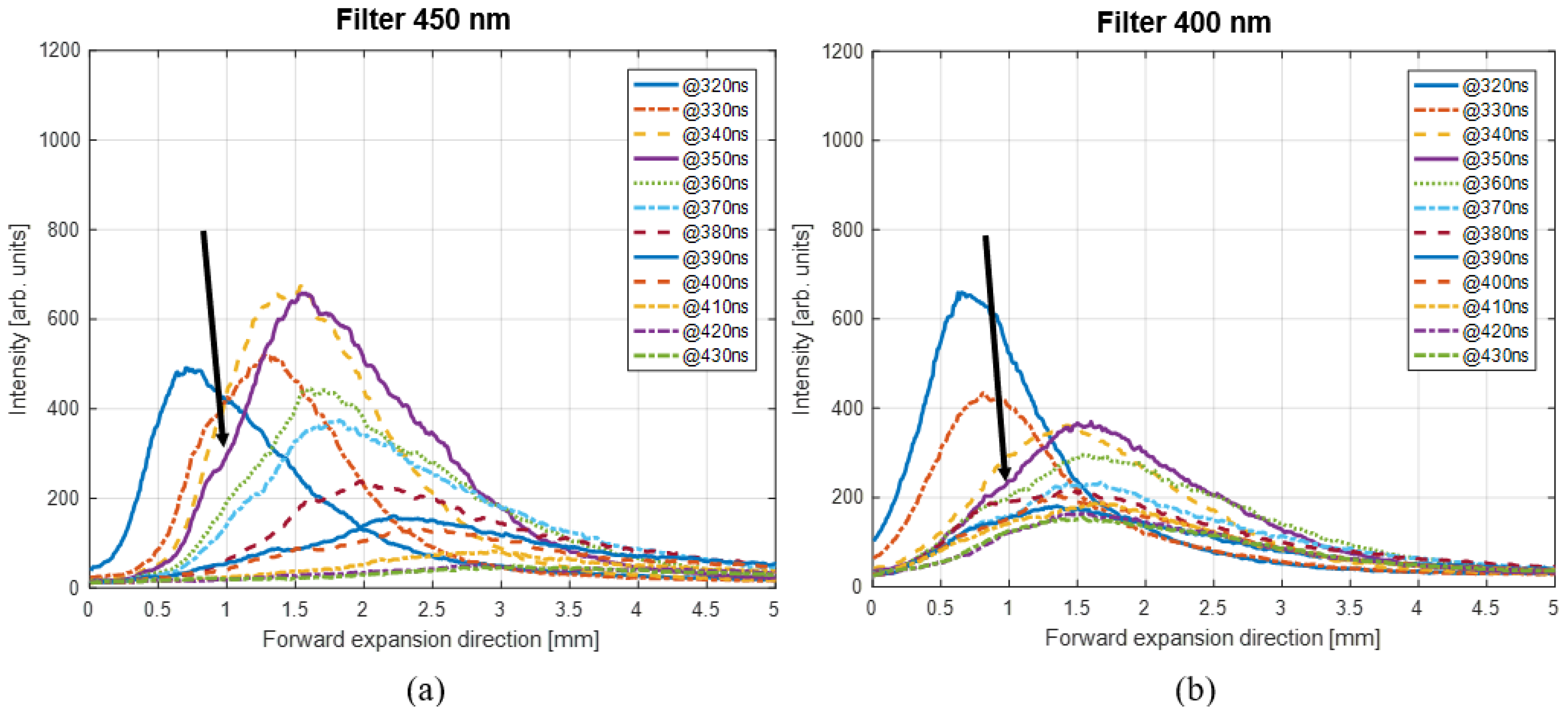
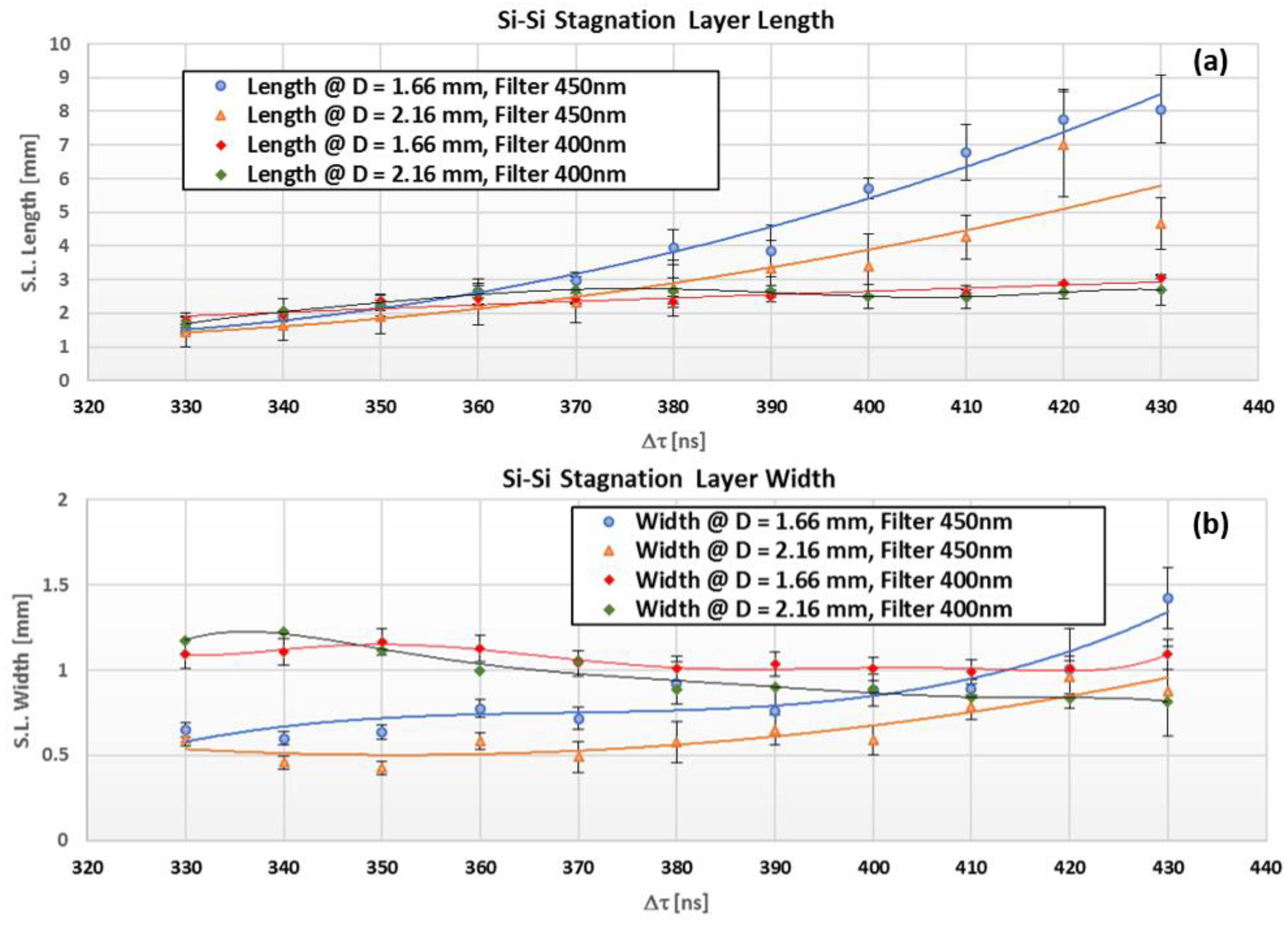
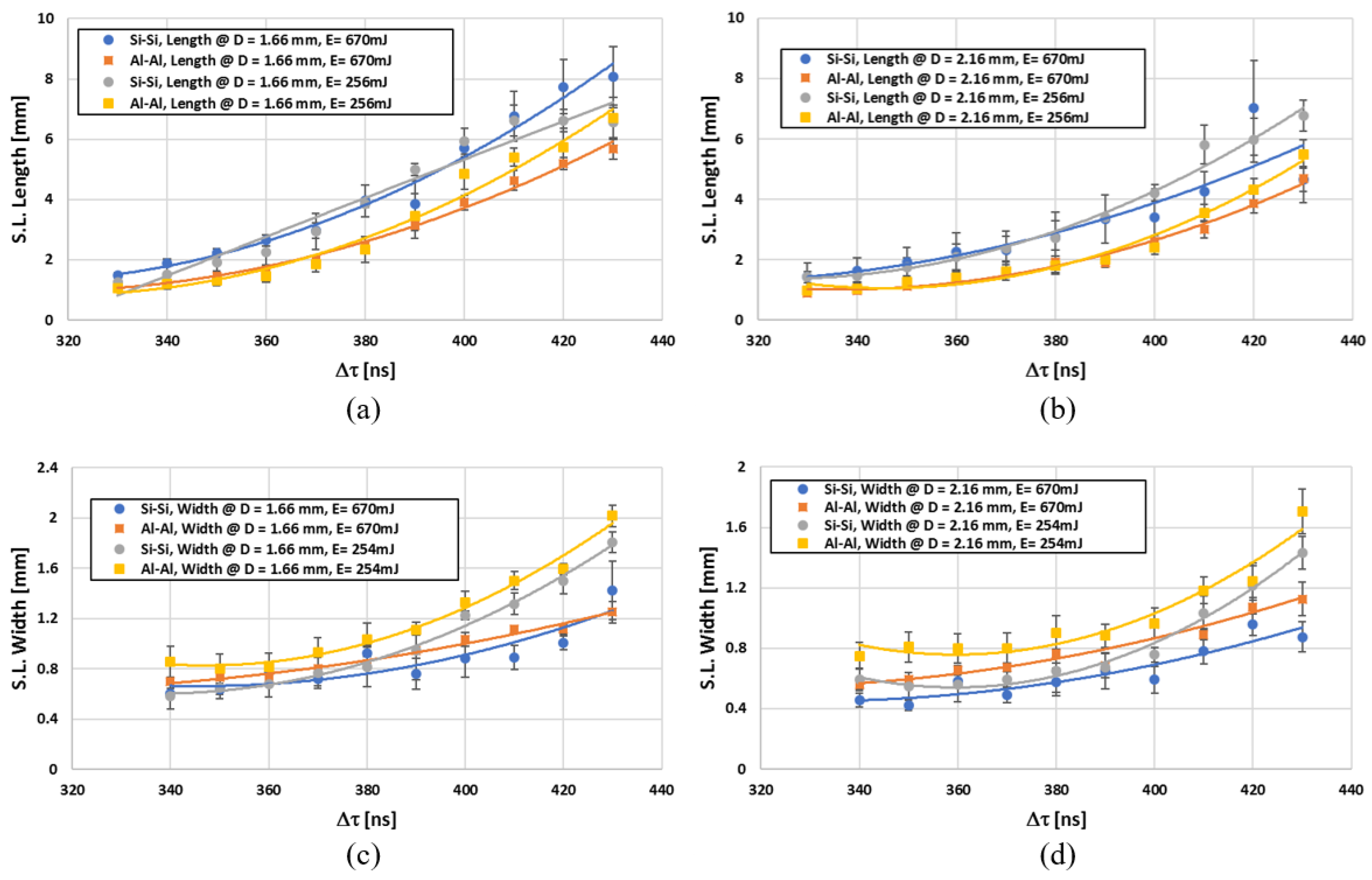
3. Results and Discussions
4. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Rambo, P.W.; Denavit, J. Interpenetration and ion separation in colliding plasmas. Phys. Plasmas 1994, 1, 4050–4060. [Google Scholar] [CrossRef]
- Camps, E.; Escobar-Alarcón, L.; Haro-Poniatowski, E.; Fernández-Guasti, M. Spectroscopic studies of two perpendicularly interacting carbon plasmas generated by laser ablation. Appl. Surf. Sci. 2002, 197, 239–245. [Google Scholar] [CrossRef]
- Zepf, M.; Clark, E.L.; Krushelnick, K.; Beg, F.N.; Escoda, C.; Dangor, A.E.; Santala, M.I.K.; Tatarakis, M.; Watts, I.F.; Norreys, P.A.; et al. Fast particle generation and energy transport in laser-solid interactions. Phys. Plasmas 2001, 8, 2323–2330. [Google Scholar] [CrossRef]
- Katayama, K.; Kinoshita, T.; Okada, R.; Fukuoka, H.; Yoshida, T.; Yaga, M.; Aoki-Matsumoto, T.; Umezu, I. Mixing of laser-induced plumes colliding in a background gas. Appl. Phys. A 2022, 128, 1007. [Google Scholar] [CrossRef]
- Hough, P.; McLoughlin, C.; Harilal, S.S.; Mosnier, J.P.; Costello, J.T. Emission characteristics and dynamics of the stagnation layer in colliding laser produced plasmas. J. Appl. Phys. 2010, 107, 024904. [Google Scholar] [CrossRef]
- Shilpa, S.; Gopinath, P. Dynamics of angularly colliding laser-produced plasmas in air and argon ambient. Vacuum 2023, 208. [Google Scholar] [CrossRef]
- Lee, H.; Kim, N.-K.; Lee, M.-G.; Kwon, J.-W.; Son, S.H.; Bae, N.; Park, T.; Park, S.; Kim, G.-H. Investigation of ion collision effect on electrostatic sheath formation in weakly ionized and weakly collisional plasma. Plasma Sources Sci. Technol. 2022, 31, 084006. [Google Scholar] [CrossRef]
- Kabacinski, A.; Tissandier, F.; Gautier, J.; Goddet, J.-P.; Andriyash, I.; Zeitoun, P.; Sebban, S.; Oliva, E.; Kozlová, M. Femtosecond soft x-ray lasing in dense collisionaly-pumped plasma. Phys. Rev. Res. 2022, 4, L032009. [Google Scholar] [CrossRef]
- Wang, Y.; Chen, A.; Zhang, D.; Wang, Q.; Li, S.; Jiang, Y.; Jin, M. Enhanced optical emission in laser-induced breakdown spectroscopy by combining femtosecond and nanosecond laser pulses. Phys. Plasmas 2020, 27, 023507. [Google Scholar] [CrossRef]
- Kumar, B.; Singh, R.K.; Sengupta, S.; Kaw, P.K.; Kumar, A. Propagation dynamics of laterally colliding plasma plumes in laser-blow-off of thin film. Phys. Plasmas 2014, 21, 083510. [Google Scholar] [CrossRef]
- George, S.; Singh, R.; Nampoori, V.; Kumar, A. Fast imaging of the laser-blow-off plume driven shock wave: Dependence on the mass and density of the ambient gas. Phys. Lett. A 2013, 377, 391–398. [Google Scholar] [CrossRef]
- Singh, R.P.; Patel, D.; Thareja, R.K. Investigation of ion dynamics of laser ablated single and colliding carbon plasmas using Faraday cup. Heliyon 2022, 8, e10621. [Google Scholar] [CrossRef] [PubMed]
- Al-Juboori, H.M.; McCormack, T. Digital nanosecond imaging architecture and analytical tracking technique of colliding laser-produced plasma. Opt. Quantum Electron. 2022, 54, 321. [Google Scholar] [CrossRef]
- Mondal, A.; Kumar, B.; Singh, R.K.; Joshi, H.C.; Kumar, A. Spectroscopic investigation of stagnation region in laterally colliding plasmas: Dependence of ablating target material and plasma plume separation. Phys. Plasmas 2019, 26, 022102. [Google Scholar] [CrossRef]
- Kramida, R.Y. NIST Atomic Spectra Database (Version 5.6.1). 2022. Available online: http://physics.nist.gov (accessed on 12 January 2023).
- Al-Juboori, H.M.; Malik, N.A.; McCormack, T. Investigations on the effect of target angle on the stagnation layer of colliding laser produced plasmas of aluminum and silicon. Phys. Plasmas 2021, 28, 123515. [Google Scholar] [CrossRef]
- Sizyuk, T.; Oliver, J.; Diwakar, P.K. Mechanisms of carbon dimer formation in colliding laser-produced carbon plasmas. J. Appl. Phys. 2017, 122, 023303. [Google Scholar] [CrossRef]




| Atomic Species | λo [nm] | Filter | Transition Levels |
|---|---|---|---|
| Al I | 393.20 | 400 nm | 3s24d – 3s3p(3po)3d – 2D5/2 – 2Do5/2 |
| Al I | 394.40 | 3s23p – 3s24s – 2P1/2 – 2S1/2 | |
| Al I | 396.15 | 3s23p – 3s24s – 2P3/2 – 2S1/2 | |
| Al III | 415.02 | 2p64d – 2p65f – 2D3/2 – 2Fo5/2 | |
| Al II | 466.30 | 450 nm | 3p2 – 3s24p – 1D2 – 1P1 |
| Al III | 447.99 | 2p64f – 2p65g – 2F7/2 – 2G9/2 | |
| Al III | 451.25 | 2p64p – 2p64d – 2P1/2 – 2D3/2 | |
| Al III | 452.89 | 2p64p – 2p64d – 2P3/2 – 2D3/2 |
| Atomic Species | λo [nm] | Filter | Transition Levels |
|---|---|---|---|
| Si III | 380.654 | 400 nm | 3s4p – 3s4d – 3P2 – 3D3 |
| Si II | 385.336 | 3s3p2 – 3s24p – 2D3/2 – 2P3/2 | |
| Si I | 390.552 | 3s2 3p2 – 3s23p4s – 1S0 1P1 | |
| Si III | 392.447 | 3s4f – 3s5g – 1F3 – 1G4 | |
| Si IV | 408.885 | 2p64s – 2p64p – 2D1/2 – 2P3/2 | |
| Si IV | 411.610 | 2p64s – 2p64p – 2S1/2 – 2P1/2 | |
| Si II | 412.807 | 3s23d – 3s24f – 2D3/2 – 2F5/2 | |
| Si II | 413.089 | 3s23d2 – 3s24f2 – 2D5/2 – 2F7/2 | |
| Si IV | 432.818 | 450 nm | 2p65p – 2p66s – 2P3/2 – 2S1/2 |
| Si II | 455.262 | 3s4s – 3s4p – 3S1 – 3P2 | |
| Si III | 456.782 | 3s4s – 3s4p – 3S1 – 3P1 | |
| Si II | 462.172 | 3s24d – 3s27f – 2D5/2 – 2F7/2 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Al-Juboori, H.M.; McCormack, T. Geometrical Analysis of the Stagnation Zone in Laterally Colliding Plasmas: Effects of Plasma Plume Separation and Ablating Target Material. Plasma 2023, 6, 198-211. https://doi.org/10.3390/plasma6020015
Al-Juboori HM, McCormack T. Geometrical Analysis of the Stagnation Zone in Laterally Colliding Plasmas: Effects of Plasma Plume Separation and Ablating Target Material. Plasma. 2023; 6(2):198-211. https://doi.org/10.3390/plasma6020015
Chicago/Turabian StyleAl-Juboori, Haider M., and Tom McCormack. 2023. "Geometrical Analysis of the Stagnation Zone in Laterally Colliding Plasmas: Effects of Plasma Plume Separation and Ablating Target Material" Plasma 6, no. 2: 198-211. https://doi.org/10.3390/plasma6020015
APA StyleAl-Juboori, H. M., & McCormack, T. (2023). Geometrical Analysis of the Stagnation Zone in Laterally Colliding Plasmas: Effects of Plasma Plume Separation and Ablating Target Material. Plasma, 6(2), 198-211. https://doi.org/10.3390/plasma6020015







