A Probable Approach to Reliability Assessment of Reinforced Plates
Abstract
:1. Introduction
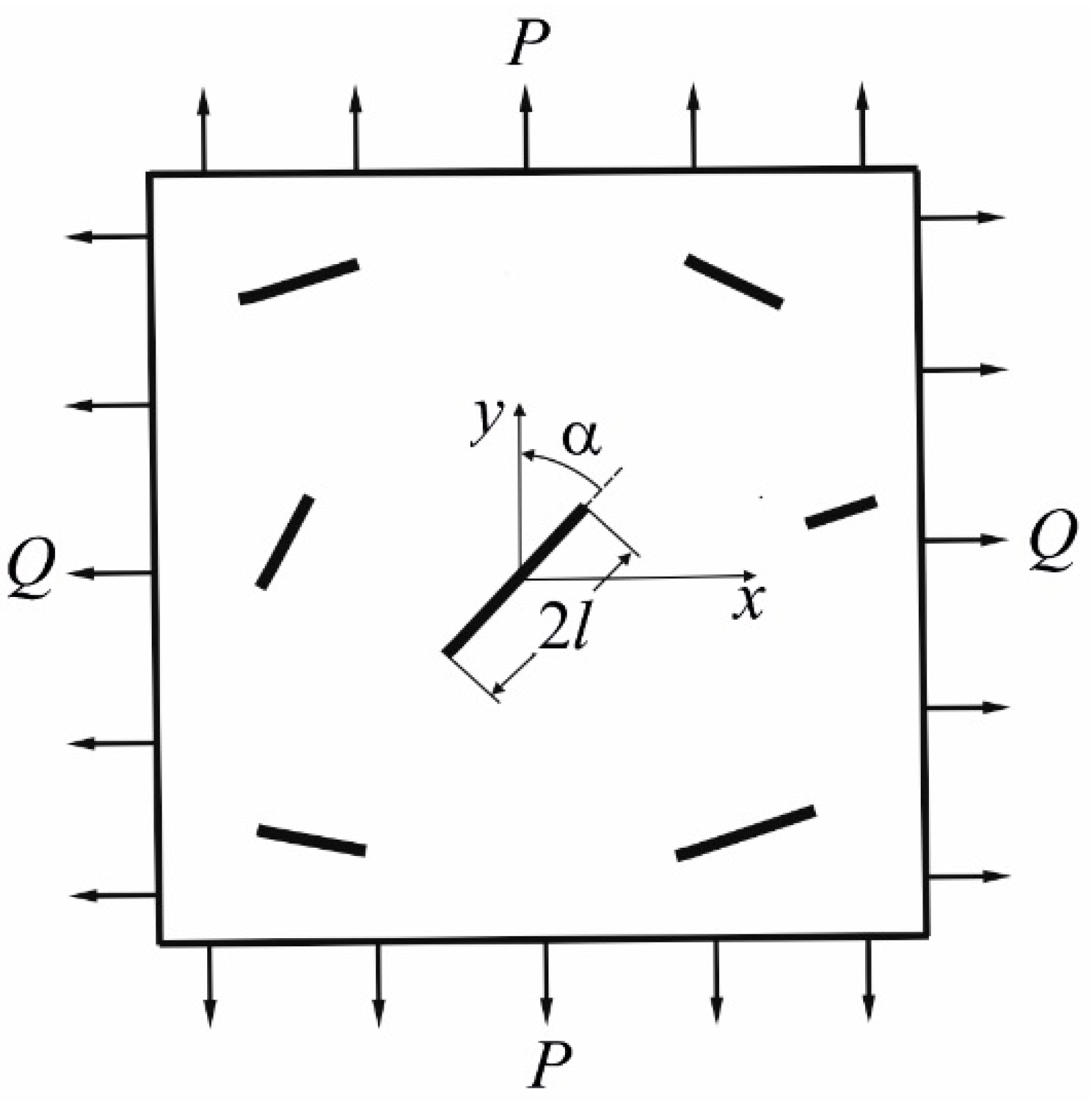
2. Materials and Methods
3. Failure Loading Probability Distribution Function
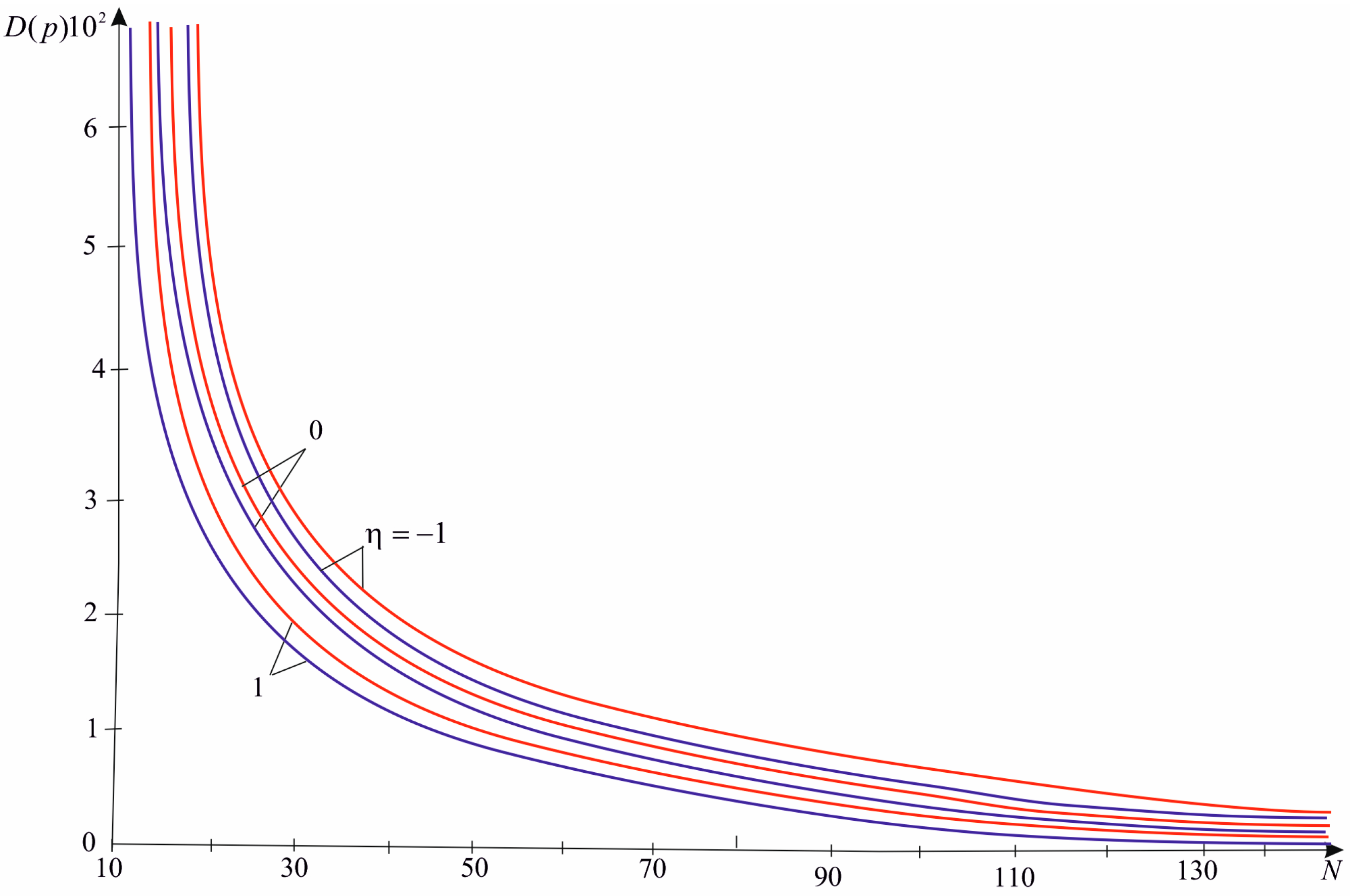
- at
- at
- at
- at we obtain the Expression (20) with a replacement in its left part by .
- at (biaxial symmetric tension)
- at (biaxial symmetric compression)
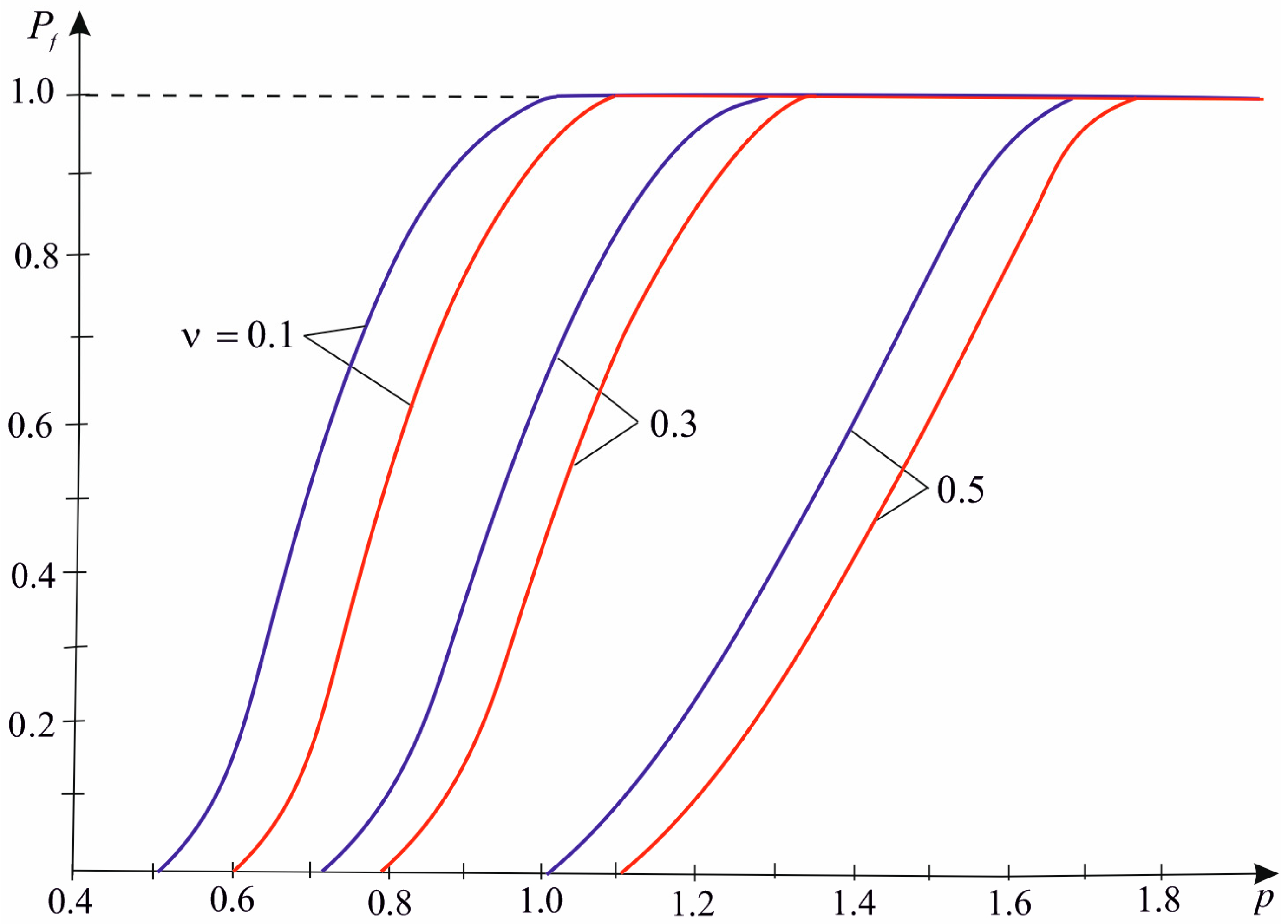
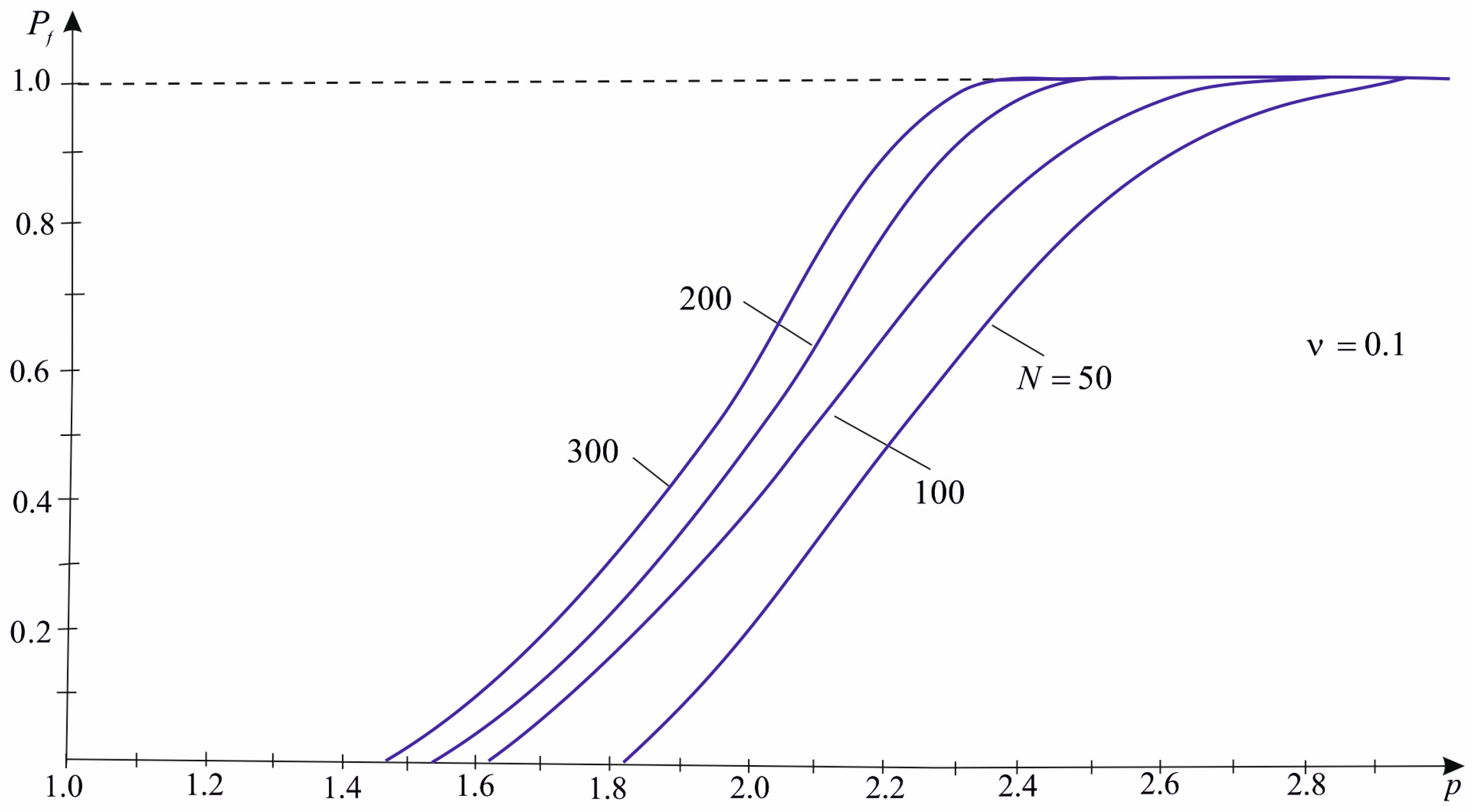
4. The Probability of Failure of the Reinforced Plates
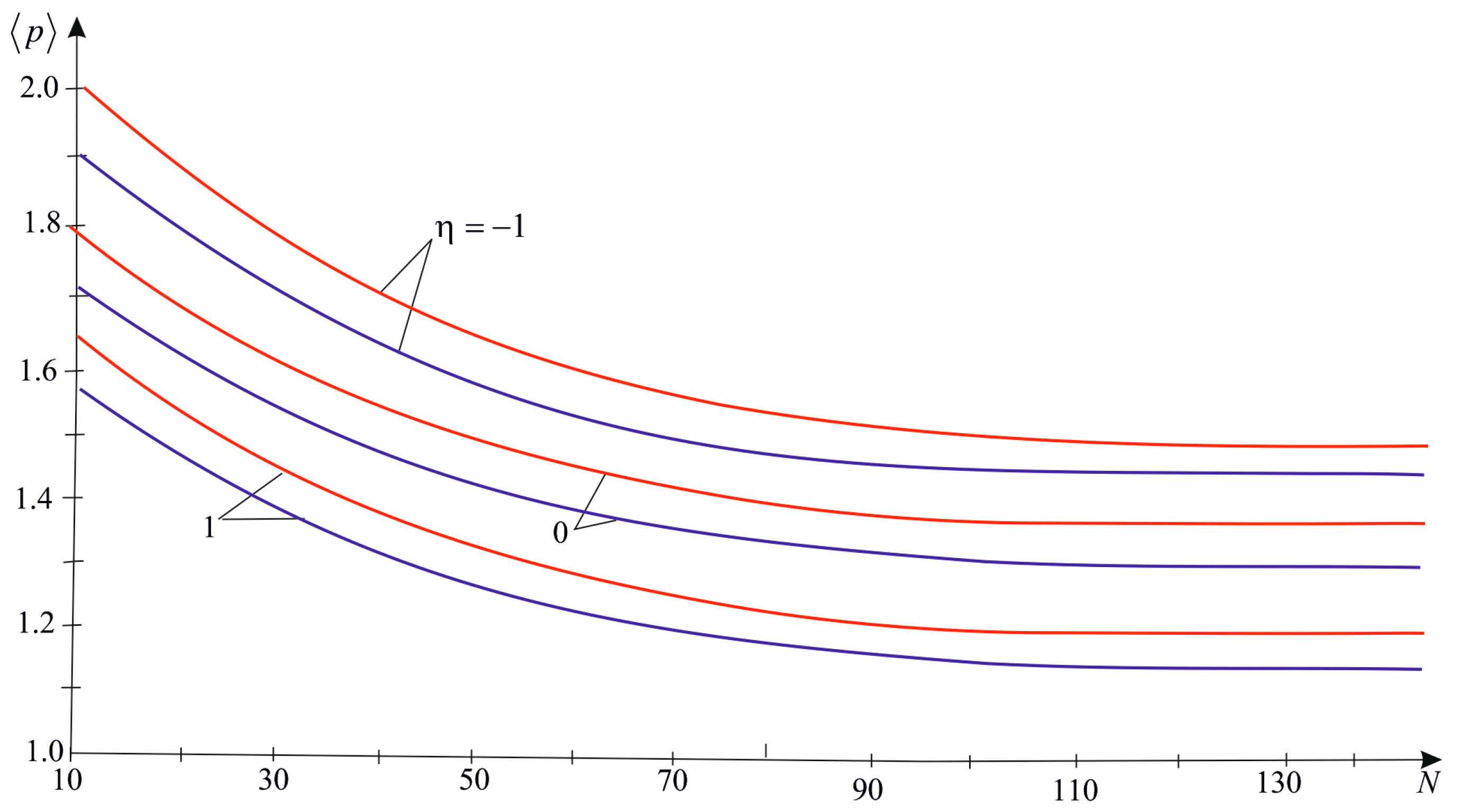
5. Mean Value and Dispersion of Failure Loading
6. Discussion
7. Conclusions
- In this paper, a new class of problems for the study of composite material failure is considered, taking into account the stochasticity of their structure.
- According to the obtained results, it is stated that the dependence of the probability of failure of the plate on the loading and the number of rigid linear inclusions have the same features as for a plate with cracks, while the dependence on the type of loading (from ) will be different.
- Further research directions could examine inclusions and their spatial placement in three-dimensional bodies.
- According to (18)–(20), in future studies, the criteria for failure under plane stress will be analyzed. In particular, the limit state curves expressed in failure loading mean values, taking into account that and the limit state curves corresponding to the given probability of failure .
- Similar probabilistic studies of strength also can be applied to structural fiberglass since reinforced fiberglass is used as a load-bearing support element for mining and construction equipment.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Zhi, J.; Tay, T.E. Interrogating failure mechanisms of notched composites through a discrete crack modeling approach. Compos. Sci. Technol. 2020, 196, 108203. [Google Scholar] [CrossRef]
- Xu, J.-H.; Zhou, G.-D.; Zhu, T.-Y. Fatigue reliability assessment for orthotropic steel bridge decks considering load sequence effects. Front. Mater. 2021, 8, 678855. [Google Scholar] [CrossRef]
- Magar, A.; Lal, A. Progressive failure analysis of laminated plate containing elliptical cutout. Int. J. Struct. Integr. 2021, 12, 569–588. [Google Scholar] [CrossRef]
- Martinez, J.; Bishay, P. On the stochastic first-ply failure analysis of laminated composite plates under in-plane tensile loading. Compos. Part C Open Access 2021, 4, 100102. [Google Scholar] [CrossRef]
- Saberi, S.; Abdollahi, A.; Inam, F. Reliability analysis of bistable composite laminates. AIMS Mater. Sci. 2021, 8, 29–41. [Google Scholar] [CrossRef]
- Tan, W.; Martínez-Pañeda, E. Phase-field fracture predictions of microscopic bridging behavior of composite materials. Compos. Struct. 2022, 286, 115242. [Google Scholar] [CrossRef]
- Rakesh, P.; More, A.; Kumar, M.; Muthu, N. Probabilistic failure prediction in a double composite cantilever beam with single and double source uncertainty. Compos. Struct. 2022, 279, 114870. [Google Scholar] [CrossRef]
- Leong, K.H.; Zhi, J.; Lee, H.P.; Tan, V.B.C.; Tay, T.E. Adaptive multi-fidelity (AMF) modeling of progressive damage in notched composite laminates. Compos. Part A Appl. Sci. Manuf. 2022, 154, 106790. [Google Scholar] [CrossRef]
- Papanicolaou, G.; Bakos, D. Effect of treatment conditions on the mode delamination fracture toughness of sandwich structures. J. Compos. Mater. 1995, 29, 2295–2316. [Google Scholar] [CrossRef]
- Kontaxis, L.; Kozaniti, F.; Papanicolaou, G. Mechanical behavior modelling and filler geometry effect of glass filler reinforced starch-epoxy hybrid matrix composites. Materials 2021, 14, 6587. [Google Scholar] [CrossRef]
- Kozlov, M.; Sheshenin, S. Modeling the progressive failure of laminated composites. Mech. Compos. Mater. 2016, 51, 695–706. [Google Scholar] [CrossRef]
- Drygaś, P.; Mityushev, V. Effective elastic properties of random two-dimensional composites. Int. J. Solids Struct. 2016, 97–98, 543–553. [Google Scholar] [CrossRef]
- Drygaś, P.; Gluzman, S.; Mityushev, V.; Nawalaniec, W. Applied Analysis of Composite Media: Analytical and Computational Results for Materials Scientists and Engineers; Woodhead Publishing: Sawston, UK, 2019; ISBN 9780081027325. [Google Scholar]
- Morassi, A.; Rosset, E.; Vessella, S. Stable determination of a rigid inclusion in an anisotropic elastic plate. SIAM J. Math. Anal. 2012, 44, 2204–2235. [Google Scholar] [CrossRef]
- Alessandrini, G.; Di Cristo, M.; Morassi, A.; Rosset, E. Stable determination of an inclusion in an elastic body by boundary measurements. SIAM J. Math. Anal. 2014, 46, 2692–2729. [Google Scholar] [CrossRef]
- Morassi, A.; Rosset, E.; Vessella, S. Optimal stability in the identification of a rigid inclusion in an isotropic Kirchhoff–Love plate. SIAM J. Math. Anal. 2019, 51, 731–747. [Google Scholar] [CrossRef]
- Alessandrini, G.; Rosset, E.; Vessella, S. Optimal three spheres inequality at the boundary for the Kirchhoff-Love plate’s equations with Dirichlet conditions. Arch. Ration. Mech. Anal. 2019, 231, 1455–1486. [Google Scholar] [CrossRef]
- Salasiya, P.; Sundaram, K. Receding contact between an inclusion of generalized shape and a remotely-stressed plate. Int. J. Solids Struct. 2022, 252, 111826. [Google Scholar] [CrossRef]
- Carpinteri, A.; Fernandez-Canteli, A.; Fortese, G.; Muñiz-Calvente, M.; Ronchei, C.; Scorza, D.; Vantadori, S. Probabilistic failure assessment of fiberglass composites. Compos. Struct. 2017, 160, 1163–1170. [Google Scholar] [CrossRef]
- Panasyuk, V.; Berezhnytskyi, L.; Trush, I. Stress distribution about defects such as rigid sharp-angled inclusions. Strength Mater. 1972, 4, 771–777. [Google Scholar] [CrossRef]
- Berezhnytskyi, L.; Panasyuk, V.; Trush, I. Local failure of a brittle body with a sharp-ended rigid inclusion. Strength Mater. 1973, 5, 1166–1170. [Google Scholar] [CrossRef]
- Vytvytskyi, P.; Popina, S. Strength and Criteria of Brittle Fracture of Stochastically Defective Bodies; Naukova Dumka: Kyiv, Ukraine, 1980. (In Ukrainian) [Google Scholar]
- Kvit, R. Investigation of probabilistic aspects reliability of isotropic bodies with internal defects. J. Appl. Math. Comput. Mech. 2022, 21, 73–84. [Google Scholar] [CrossRef]
- Petersen, T. The laws of defect-size distribution in the failure of materials. Dokl. Phys. 2001, 46, 369–372. [Google Scholar] [CrossRef]
- Ignatovich, S.; Bouraou, N. Power law of crack length distribution in the multiple damage process. Strength Mater. 2019, 51, 735–745. [Google Scholar] [CrossRef]
- Vitvitskiy, P.; Kvit, R. Probabilistic description of experimental statistical strength characteristics. Mater. Sci. 1992, 28, 83–86. [Google Scholar] [CrossRef]
- Kvit, R. Development of orthotropic composite materials statistical model failure. J. Appl. Math. Comput. Mech. 2023, 22, 26–35. [Google Scholar] [CrossRef]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Pukach, P.; Kvit, R.; Salo, T.; Vovk, M. A Probable Approach to Reliability Assessment of Reinforced Plates. Appl. Syst. Innov. 2023, 6, 73. https://doi.org/10.3390/asi6040073
Pukach P, Kvit R, Salo T, Vovk M. A Probable Approach to Reliability Assessment of Reinforced Plates. Applied System Innovation. 2023; 6(4):73. https://doi.org/10.3390/asi6040073
Chicago/Turabian StylePukach, Petro, Roman Kvit, Tetyana Salo, and Myroslava Vovk. 2023. "A Probable Approach to Reliability Assessment of Reinforced Plates" Applied System Innovation 6, no. 4: 73. https://doi.org/10.3390/asi6040073
APA StylePukach, P., Kvit, R., Salo, T., & Vovk, M. (2023). A Probable Approach to Reliability Assessment of Reinforced Plates. Applied System Innovation, 6(4), 73. https://doi.org/10.3390/asi6040073






