Design of Type-IV Composite Pressure Vessel Based on Comparative Analysis of Numerical Methods for Modeling Type-III Vessels
Abstract
1. Introduction
2. Materials and Methods
2.1. Materials
2.2. Micromechanics Models
2.3. Constitutive Models for the Liner and the Overwrapped Composite Layers
3. Numerical Simulations
3.1. Simulation Using 3D Elements
3.2. Simulation Using Conventional Shell Elements
3.3. Simulation Using Continuum Shell Elements
3.4. Simulation Using Mixed Method
4. Comparison between Methods and Discussion
5. New Design of Type-IV Hydrogen Tank
6. Concluding Remarks
- In this study, we presented a comparative analysis of various numerical methods for modeling composite pressure vessels, aiming to provide a comprehensive understanding of their performance. The methods under scrutiny include finite element analysis in Abaqus with conventional shell element, continuum shell element, three-dimensional solid element, and homogenization approaches for multilayered composite pressure vessels. Through a systematic comparison, this research offers insights into the strengths and limitations of each method. It is crucial to emphasize that the results achieved could be replicated with a lower mesh density when utilizing only a quarter of the model.
- The findings of this study indicate that three-dimensional solid elements yield the highest accuracy in modeling composite pressure vessels. However, their practicality diminishes as the number of layers in the composite increases. Following closely are the continuum shell elements, which strike a balance between accuracy and computational efficiency due to their intermediate nature, combining features of both 3D and conventional shell elements. Meanwhile, the method relying solely on conventional shell elements proves to be accurate for specific applications but lacks universality.
- Moreover, this research underscores the significance of the homogenization technique used in the mixed method as an alternative, particularly for damage-free applications, as it consistently delivers highly accurate results. The approach involves treating the composite shell section of the tank as a straightforward homogenized layer.
- A new design dedicated to a type-IV hydrogen tank, composed of carbon fibers, epoxy resin, and a high-density polyethylene (HDPE) liner, is proposed. The study concentrates on predicting damage onset and behavior within the tank and burst pressure prediction. With this new design, we demonstrated that the tank can endure a pressure of 1000 bar when using 36 plies, resulting in a composite shell thickness of 7.2 mm. Undoubtedly, future optimization is essential as this exploration aligns with the broader scope of a significant project where we are concurrently working on new materials development.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Xu, P.; Xu, P.; Zheng, J.; Liu, P. Finite element analysis of burst pressure of composite hydrogen storage vessels. Mater. Des. 2009, 30, 2295–2301. [Google Scholar] [CrossRef]
- Rafiee, R.; Torabi, M.A. Stochastic prediction of burst pressure in composite pressure vessels. Compos. Struct. 2018, 185, 573–583. [Google Scholar] [CrossRef]
- Zheng, J.; Liu, P. Elasto-plastic stress analysis and burst strength evaluation of al-carbon fiber/epoxy composite cylindrical laminates. Comput. Mater. Sci. 2008, 42, 453–461. [Google Scholar] [CrossRef]
- Liu, P.; Zheng, J. Progressive failure analysis of carbon fiber/epoxy composite laminates using continuum damage mechanics. Mater. Sci. Eng. -Struct. Mater. Prop. Microstruct. Process. 2008, 485, 711–717. [Google Scholar] [CrossRef]
- Liu, P.; Liu, P.; Chu, J.; Chu, J.; Chu, J.; Hou, S.; Xu, P.; Xu, P.; Xu, P.; Zheng, J. Numerical simulation and optimal design for composite high-pressure hydrogen storage vessel: A review. Renew. Sustain. Energy Rev. 2012, 16, 1817–1827. [Google Scholar] [CrossRef]
- Ramirez, J.P.B.; Halm, D.; Grandidier, J.-C.; Villalonga, S.; Villalonga, S. A fixed directions damage model for composite materials dedicated to hyperbaric type iv hydrogen storage vessel—Part I: Model formulation and identification. Int. J. Hydrogen Energy 2015, 40, 13165–13173. [Google Scholar] [CrossRef]
- Humberto, J.; Almeida, J.; St-Pierre, L.; Wang, Z.; Ribeiro, M.L.; Tita, V.; Amico, S.C.; Castro, S.G. Design, modelling, optimization, manufacturing, and testing of variable-angle filament-wound cylinders. Compos. Part 2021, 225, 109224. [Google Scholar]
- Zhanga, Q.; Xua, H.; Jiab, X.; Zua, L.; Chenga, S.; Wanga, H. Design of a 70 mpa type iv hydrogen storage vessel using accurate modelling techniques for dome thickness prediction. Compos. Struct. 2020, 236, 111915. [Google Scholar] [CrossRef]
- Jois, K.C.; Welsh, M.; Gries, T.; Sackmann, J. Numerical analysis of filament wound cylindrical composite pressure vessels accounting for variable dome contour. J. Compos. Sci. 2021, 5, 56. [Google Scholar] [CrossRef]
- Zhang, N.; Gao, S.; Song, M.; Chen, Y.; Zhao, X.; Liang, J.; Feng, J. A multiscale study of cfrp based on asymptotic homogenization with application to mechanical analysis of composite pressure vessels. Polymers 2022, 14, 2817. [Google Scholar] [CrossRef]
- Kang, H.; He, P.; Zhang, C.; Dai, Y.; Lv, H.; Zhang, M.; Yang, D. Stress–strain and burst failure analysis of fibre wound composite material high-pressure vessel. Polym. Polym. Compos. 2021, 29, 1291–1303. [Google Scholar] [CrossRef]
- Landi, A.V.D.; Borriello, S.; Scafà, M.; Germani, M. A methodological approach for the design of composite tanks produced by filament winding. Comput.-Aided Des. Appl. 2020, 17, 1229–1240. [Google Scholar] [CrossRef]
- Jebeli, M.A.; Heidari-Rarani, M. Development of abaqus wcm plugin for progressive failure analysis of type iv composite pressure vessels based on puck failure criterion. Eng. Fail. Anal. 2022, 131, 105851. [Google Scholar] [CrossRef]
- Wang, H.; Fu, S.; Chen, Y.; Hua, L. Thickness-prediction method involving tow redistribution for the dome of composite hydrogen storage vessels. Polymers 2021, 14, 902. [Google Scholar] [CrossRef] [PubMed]
- Zhang, M.; Lv, H.; Kang, H.; Zhou, W.; Zhang, C. A literature review of failure prediction and analysis methods for composite high-pressure hydrogen storage tanks. Int. J. Hydrogen Energy 2019, 44, 25777–25799. [Google Scholar] [CrossRef]
- Kartav, O.; Kangal, S.; Yücetürk, K.; Tanoglu, M.; Aktas, E.; Artem, H.S. Development and analysis of composite overwrapped pressure vessels for hydrogen storage. J. Compos. Mater. 2021, 55, 002199832110335. [Google Scholar] [CrossRef]
- Regassa, Y.; Gari, J.; Lemu, H.G. Composite overwrapped pressure vessel design optimization using numerical method. J. Compos. Sci. 2022, 6, 229. [Google Scholar] [CrossRef]
- Kangal, S.; Kartav, O.; Tanoglu, M.; Aktas, E.; Artem, H.S. Investigation of interlayer hybridization effect on burst pressure performance of composite overwrapped pressure vessels with load-sharing metallic liner. J. Compos. Mater. 2020, 54, 961–980. [Google Scholar] [CrossRef]
- Nguyen, B.N.; Roh, H.S.; Merkel, D.R.; Simmons, K.L. A predictive modelling tool for damage analysis and design of hydrogen storage composite pressure vessels. Int. J. Hydrogen Energy 2021, 46, 20573–20585. [Google Scholar] [CrossRef]
- Moradi, R.; Growth, K.M. Hydrogen storage and delivery: Review of the state-of-the-art technologies and risk and reliability analysis. Int. J. Hydrogen Energy 2019, 44, 12254–12269. [Google Scholar] [CrossRef]
- Modesto, A.J.; Birgul, R.; Werlink, R.J.; Catbas, F.N. Damage detection of composite overwrapped pressure vessels using arx models. Int. J. Press. Vessel. Pip. 2021, 192, 104410. [Google Scholar] [CrossRef]
- Guo, L.W.K.; Xiao, J.; Lei, M.; Wang, S.; Zhang, C.; Hou, X. Design of winding pattern of filament wound composite pressure vessel with unequal openings based on non-geodesics. J. Eng. Fibers Fabr. 2020, 15, 1–17. [Google Scholar] [CrossRef]
- Air, A.; Oromiehie, E.; Prusty, G. Design and manufacture of a type v composite pressure vessel using automated fibre placement. Compos. Part Eng. 2023, 266, 111027. [Google Scholar] [CrossRef]
- Belardi, V.; Ottaviano, M.; Vivio, F. Bending theory of composite pressure vessels: A closed-form analytical approach. Compos. Struct. 2024, 329, 117799. [Google Scholar] [CrossRef]
- Lin, J.; Zheng, C.; Dai, Y.; Wang, Z.; Lu, J. Prediction of composite pressure vessel dome contour and strength analysis based on a new fiber thickness calculation method. Compos. Struct. 2023, 306, 116590. [Google Scholar] [CrossRef]
- Rohatgi, A. Webplotdigitizer: Version 4.6. 2022. Available online: https://automeris.io/WebPlotDigitizer (accessed on 2 January 2024).
- Bouhala, L.; Makradi, A.; Belouettar, S.; Kiefer-Kamal, H.; Fréres, P. Modelling of failure in long fibres reinforced composites by xfem and cohesive zone model. Compos. Part B 2013, 55, 352–361. [Google Scholar] [CrossRef]
- Bouhala, L.; Shao, Q.; Koutsawa, Y.; Younes, A.; Núnez, P.; Makradi, A.; Belouettar, S. An xfem crack-tip enrichment for a crack terminating at a bi-material interface. Eng. Fract. Mech. 2013, 102, 51–64. [Google Scholar] [CrossRef]
- Dassault Systèmes. Micromechanics Plugin for Abaqus/CAE, Version 1.18; Dassault Systèmes Simulia Corp.: Providence, RI, USA, 2022. [Google Scholar]
- Azoti, W.L.; Tchalla, A.; Koutsawa, Y.; Makradi, A.; Rauchs, G.; Belouettar, S.; Zahrouni, H. Mean-field constitutive modeling of elasto-plastic composites using two (2) incremental formulations. Compos. Struct. 2013, 105, 256–262. [Google Scholar] [CrossRef]
- Bouhala, L.; Koutsawa, Y.; Makradi, A.; Belouettar, S. An advanced numerical method for predicting effective elastic properties of heterogeneous composite materials. Compos. Struct. 2014, 117, 114–123. [Google Scholar] [CrossRef]
- Dassault Systèmes. User’s Manual; Version 2022; Dassault Systèmes Simulia Corp.: Providence, RI, USA, 2022. [Google Scholar]
- Lapczyk, I.; Hurtado, J.A. Progressive damage modeling in fiber-reinforced materials. Compos. Part A Appl. Sci. Manuf. 2007, 38, 2333–2341. [Google Scholar] [CrossRef]
- Sapre, S.; Pareek, K.; Vyas, M. Investigation of structural stability of type iv compressed hydrogen storage tank during refueling of fuel cell vehicle. Energy Storage 2020, 2, 150. [Google Scholar] [CrossRef]

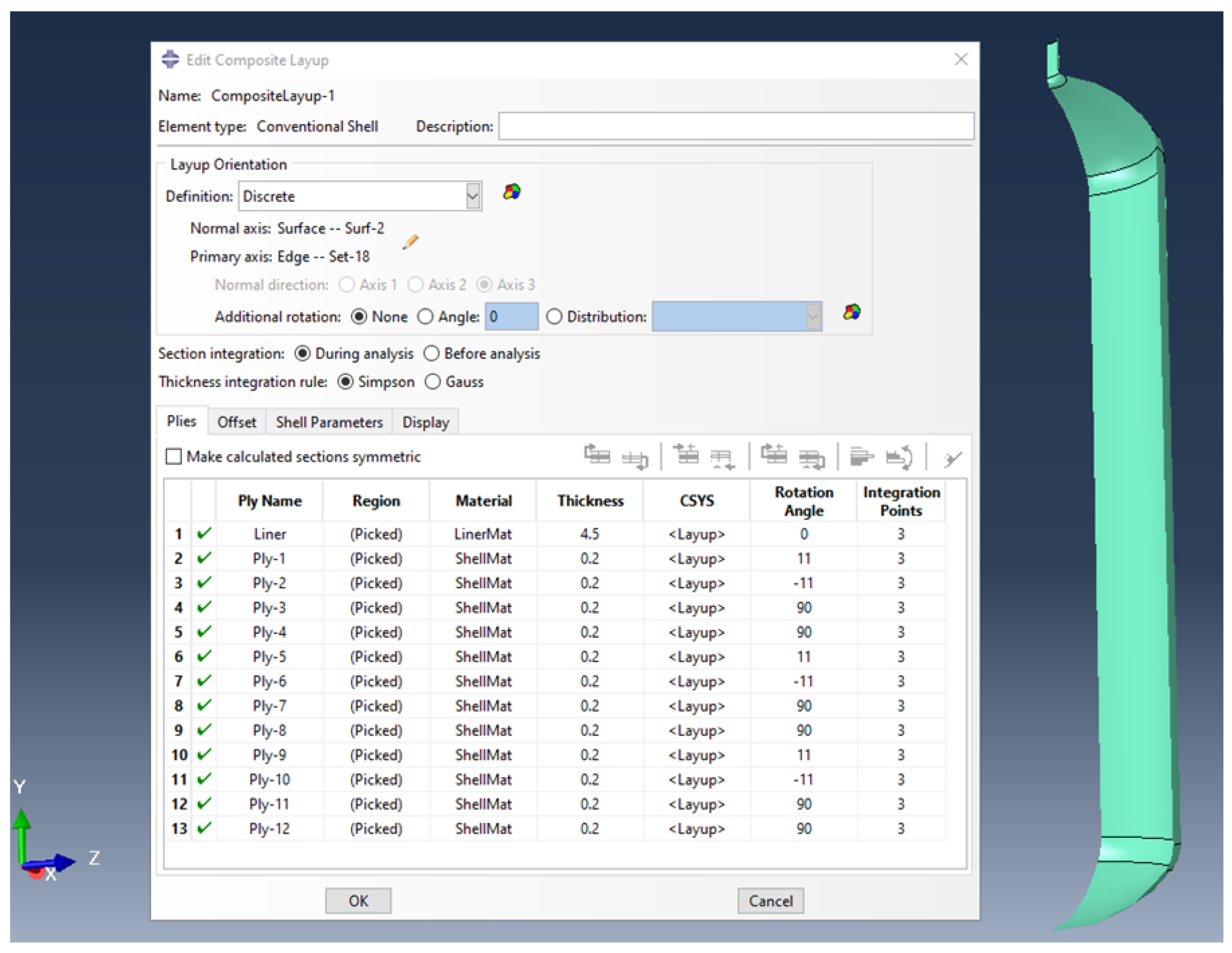

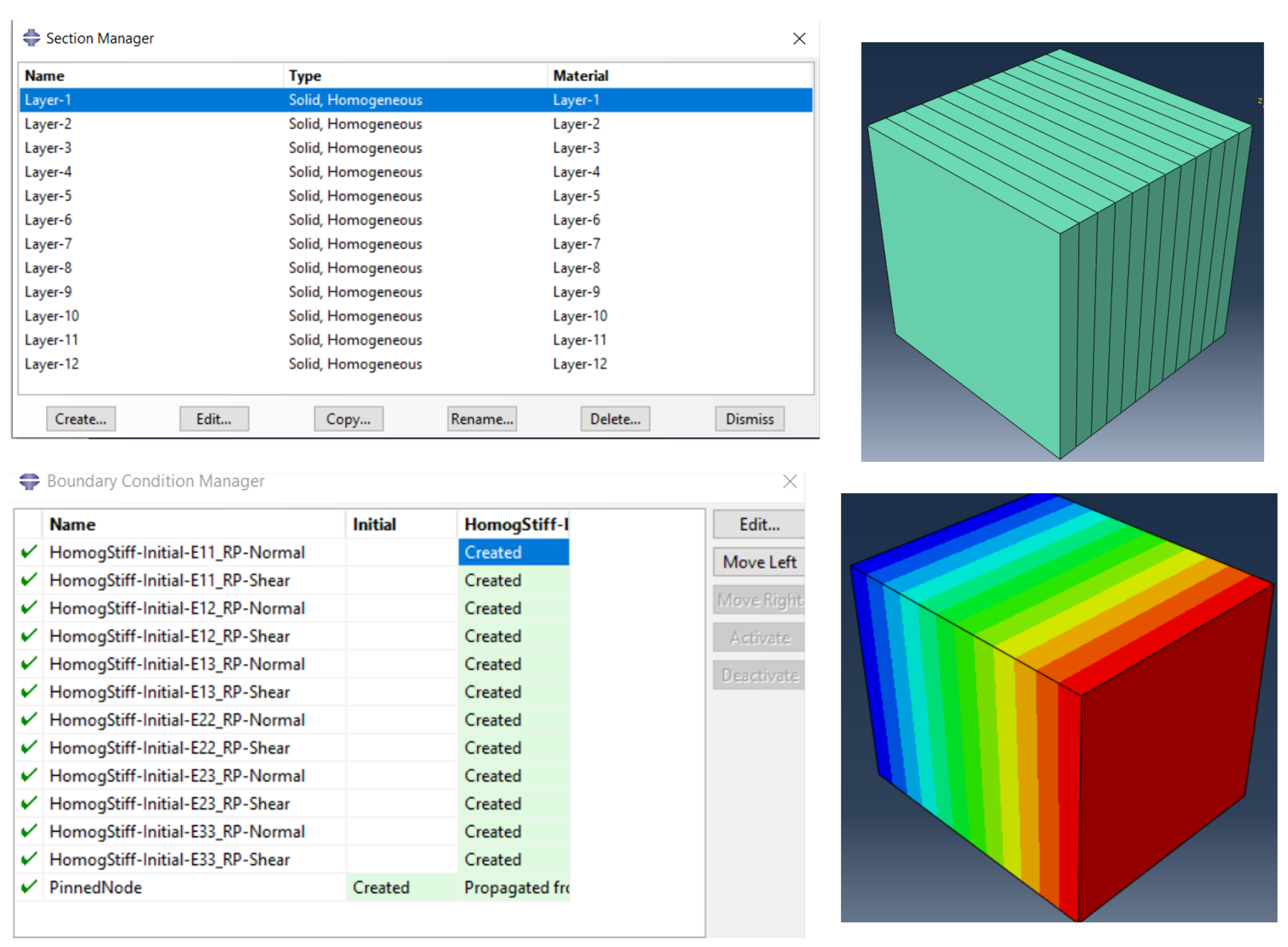



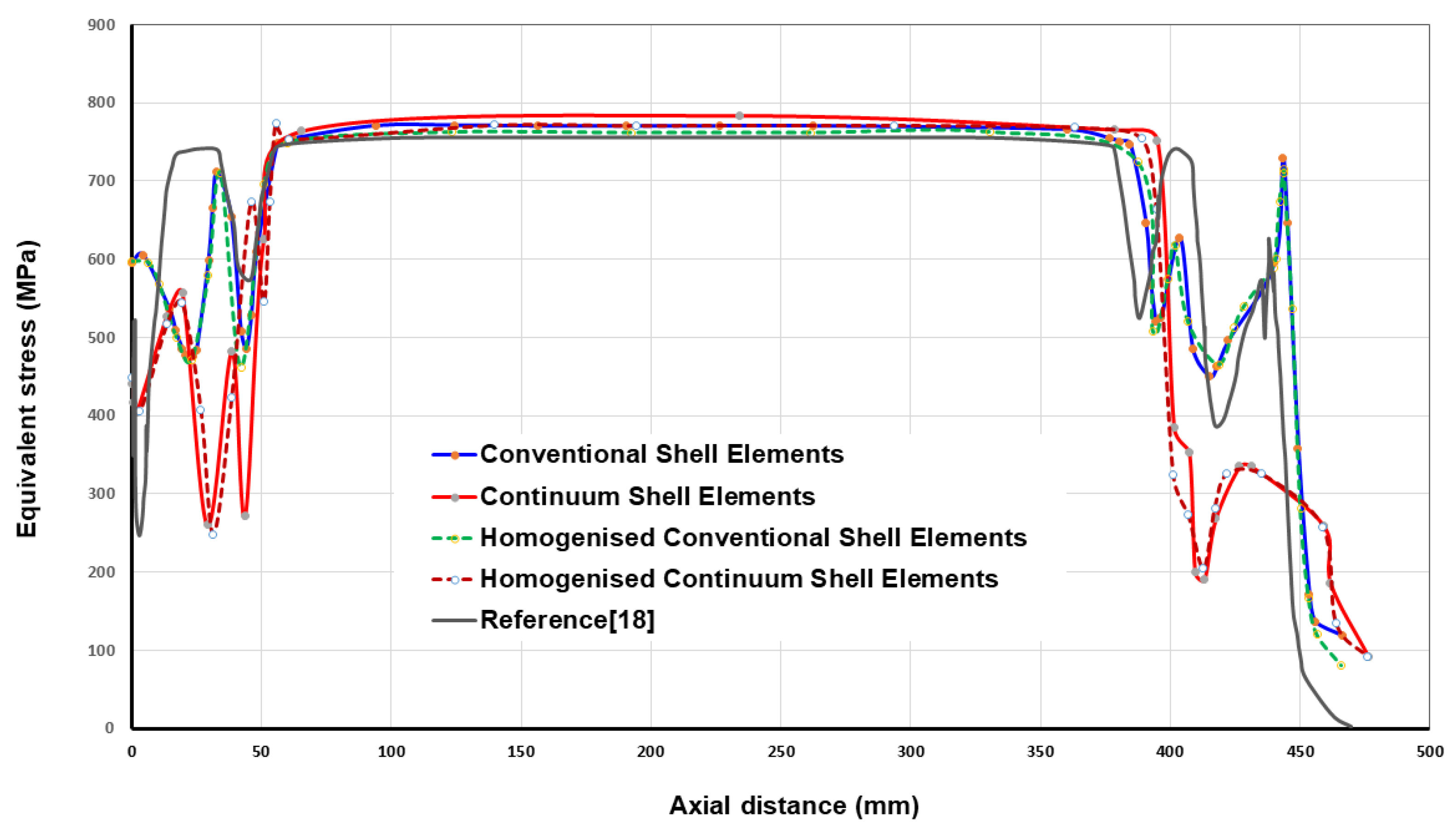
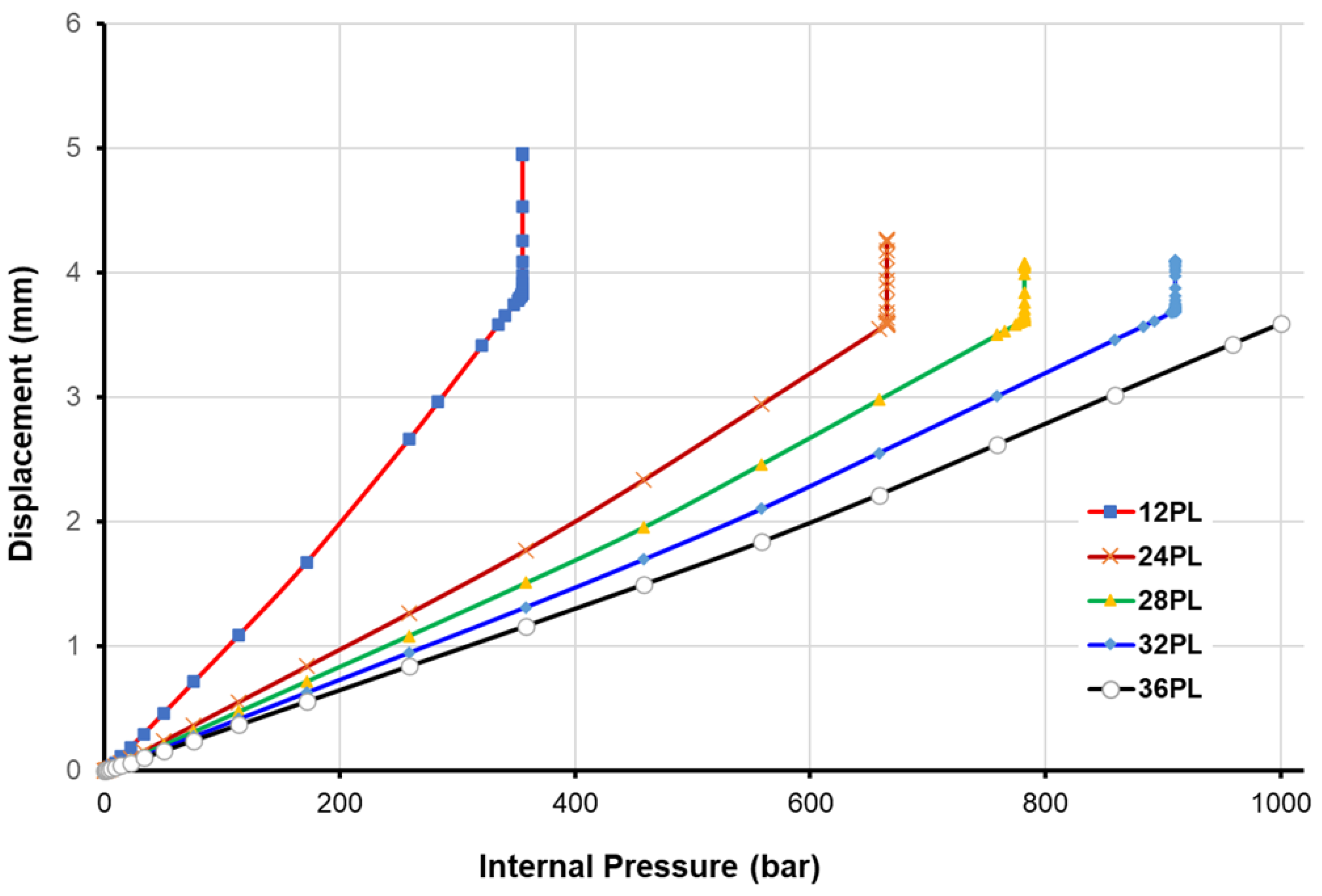

| Symbol | Description | Unit | Value |
|---|---|---|---|
| Glass fiber/epoxy composite | |||
| Longitudinal (fiber-dominated) modulus | MPa | 38,500 | |
| Transverse (matrix-dominated) modulus | MPa | 16,500 | |
| Poisson’s ratio (in-plane) | - | 0.27 | |
| Poisson’s ratio (planes 2–3) | - | 0.28 | |
| In-plane shear modulus | MPa | 4700 | |
| Shear modulus (planes 2–3) | MPa | 5000 | |
| Longitudinal (fiber-dominated) tensile strength | MPa | 1250 | |
| Longitudinal (fiber-dominated) compressive strength | MPa | −650 | |
| Transverse (matrix-dominated) tensile strength | MPa | 36 | |
| Transverse (matrix-dominated) compressive strength | MPa | −165 | |
| In-plane shear strength | MPa | 86 | |
| Fracture energy of the fiber | N/mm | 12.5 | |
| Fracture energy of the matrix | N/mm | 1 | |
| Steel liner (SL) | |||
| Young’s modulus | MPa | 205,000 | |
| Poisson’s ratio | - | 0.3 | |
| Yield strength | MPa | 743 | |
| Bilinear isotropic hardening tangent modulus | MPa | 2600 | |
| (MPa) | (MPa) | (MPa) | (-) | (-) | (-) | (MPa) | (MPa) | (MPa) |
|---|---|---|---|---|---|---|---|---|
| 26,548.24 | 27,347.34 | 17,343.40 | 0.180 | 0.344 | 0.339 | 5204.77 | 4700 | 4700 |
| Symbol | Description | Unit | Value | ||||
|---|---|---|---|---|---|---|---|
| Carbon fiber/epoxy composite | |||||||
| Longitudinal (fiber-dominated) modulus | MPa | 141,000 | |||||
| Transverse (matrix-dominated) modulus | MPa | 11,400 | |||||
| Poisson’s ratio (in-plane) | - | 0.28 | |||||
| Poisson’s ratio (planes 2–3) | - | 0.40 | |||||
| In-plane shear modulus | MPa | 5000 | |||||
| Shear modulus (planes 2–3) | MPa | 3080 | |||||
| Longitudinal (fiber-dominated) tensile strength | MPa | 2080 | |||||
| Longitudinal (fiber-dominated) compressive strength | MPa | −1250 | |||||
| Transverse (matrix-dominated) tensile strength | MPa | 60 | |||||
| Transverse (matrix-dominated) compressive strength | MPa | −290 | |||||
| In-plane shear strength | MPa | 110 | |||||
| Fracture energy of the fiber | N/mm | 78 | |||||
| Fracture energy of the matrix | N/mm | 1 | |||||
| Isotropic elastic properties for the high-density polyethylene liner (HDPE) [32] | |||||||
| Young’s modulus | MPa | 903.114 | |||||
| Poisson’s ratio | - | 0.39 | |||||
| Isotropic plastic hardening data for the HDPE liner material [32] | |||||||
| Yield stress (MPa) | 8.618 | 13.064 | 16.787 | 18.476 | 20.337 | 24.543 | 26.887 |
| Plastic strain (-) | 0 | 0.007 | 0.025 | 0.044 | 0.081 | 0.28 | 0.59 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bouhala, L.; Koutsawa, Y.; Karatrantos, A.; Bayreuther, C. Design of Type-IV Composite Pressure Vessel Based on Comparative Analysis of Numerical Methods for Modeling Type-III Vessels. J. Compos. Sci. 2024, 8, 40. https://doi.org/10.3390/jcs8020040
Bouhala L, Koutsawa Y, Karatrantos A, Bayreuther C. Design of Type-IV Composite Pressure Vessel Based on Comparative Analysis of Numerical Methods for Modeling Type-III Vessels. Journal of Composites Science. 2024; 8(2):40. https://doi.org/10.3390/jcs8020040
Chicago/Turabian StyleBouhala, Lyazid, Yao Koutsawa, Argyrios Karatrantos, and Claus Bayreuther. 2024. "Design of Type-IV Composite Pressure Vessel Based on Comparative Analysis of Numerical Methods for Modeling Type-III Vessels" Journal of Composites Science 8, no. 2: 40. https://doi.org/10.3390/jcs8020040
APA StyleBouhala, L., Koutsawa, Y., Karatrantos, A., & Bayreuther, C. (2024). Design of Type-IV Composite Pressure Vessel Based on Comparative Analysis of Numerical Methods for Modeling Type-III Vessels. Journal of Composites Science, 8(2), 40. https://doi.org/10.3390/jcs8020040








